Boussinesq Modelling of Shallow Water Phenomena †
Abstract
:1. Introduction
2. Boussinesq-Type Models
3. Numerical Experiments
3.1. Incipient Breaking on a Plane Beach
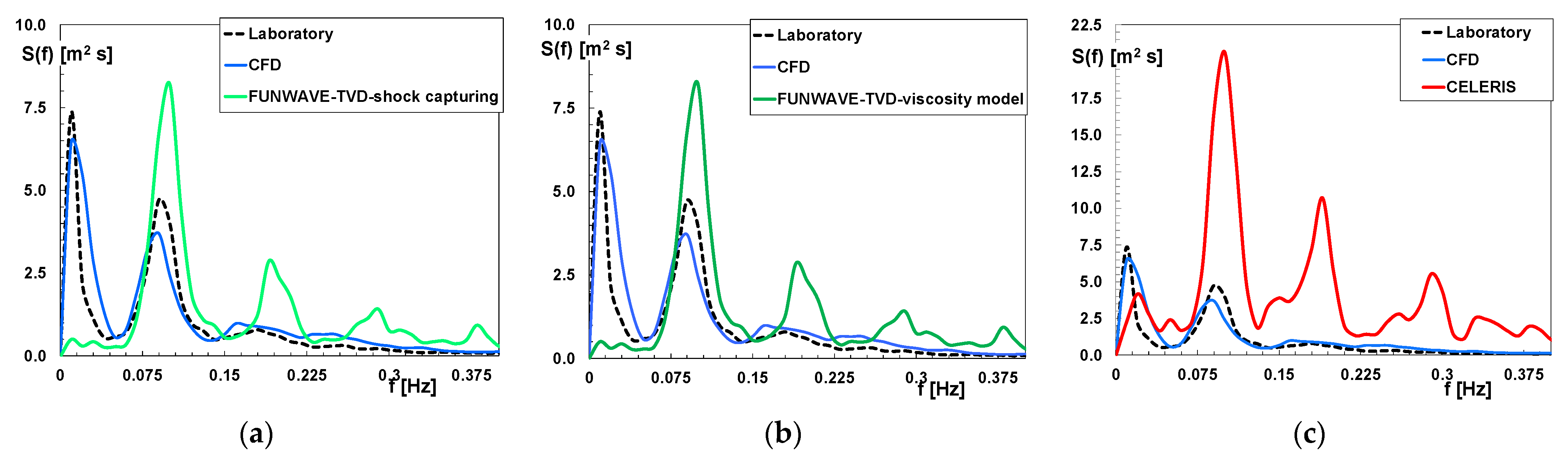
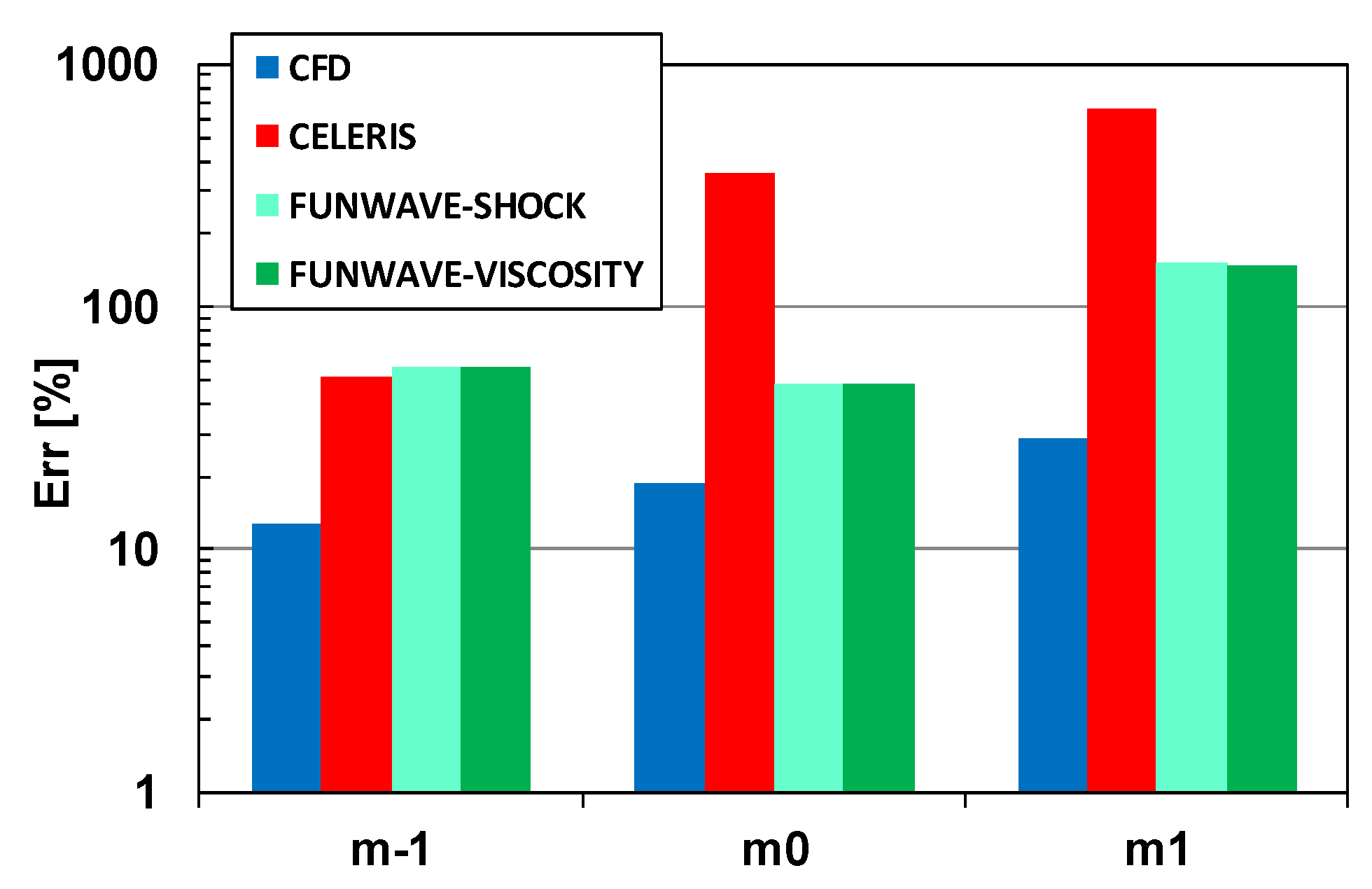
3.2. Wave Breaking of Irregular Waves
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Brocchini, M. A reasoned overview on Boussinesq-type models: The interplay between physics, mathematics and numerics. Proc. R. Soc. A 2013, 469, 20130496. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Svendsen, I.A. Mass flux and undertow in a surf zone. Coast. Eng. 1984, 8, 347–365. [Google Scholar] [CrossRef]
- Kennedy, A.B.; Chen, Q.; Kirby, J.T.; Dalrymple, R.A. Boussinesq modeling of wave transformation, breaking, end runup. I: 1D. J. Waterw. Port Coast. Ocean Eng. 2000, 126, 39–47. [Google Scholar] [CrossRef] [Green Version]
- Veeramony, J.; Svendsen, I.A. The flow in surf-zone waves. Coast. Eng. 2000, 39, 93–122. [Google Scholar] [CrossRef]
- Tonelli, M.; Petti, M. Hybrid finite volume: Finite difference scheme for 2DH improved Boussinesq equations. Coast. Eng. 2009, 56, 609–622. [Google Scholar] [CrossRef]
- Tavakkol, S.; Lynett, P. Celeris: A GPU-accelerated open source software with a Boussinesq-type wave solver for real-time interactive simulation and visualization. Comput. Phys. Commun 2017, 217, 117–127. [Google Scholar] [CrossRef] [Green Version]
- Shi, F.; Kirby, J.T.; Harris, J.C.; Geiman, J.D.; Grilli, S.T. A high-order adaptive time-stepping TVD solver for Boussinesq modeling of breaking waves and coastal inundation. Ocean. Model. 2012, 43–44, 36–51. [Google Scholar] [CrossRef]
- Madsen, P.A.; Sørensen, O.R. A new form of the Boussinesq equations with improved linear dispersion characteristics. Part 2: A slowly varying bathymetry. Coast. Eng. 1992, 18, 183–204. [Google Scholar] [CrossRef]
- Chen, Q. Fully nonlinear Boussinesq-type equations for waves and currents over porous beds. J. Eng. Mech. 2006, 132, 220–230. [Google Scholar] [CrossRef]
- Peregrine, D.H. Long waves on a beach. J. Fluid Mech. 1967, 27, 815–827. [Google Scholar] [CrossRef]
- Weggel, J.R. Maximum breaker height. J. Waterw. Harb. Coast. Eng. Div. 1972, 98, 529–548. [Google Scholar] [CrossRef]
- Goda, Y. Irregular wave deformation in the surf zone. Coast. Eng. Jpn. 1975, 18, 13–26. [Google Scholar] [CrossRef]
- Moore, B.D. Beach Profile Evolution in Response to Changes in Water Level and Wave Height. Master’s Thesis, University of Delaware, Newark, NJ, USA, 1982. [Google Scholar]
- Choi, Y.K.; Shi, F.; Malej, M.; Smith, J.M. Performance of various shock-capturing-type reconstruction schemes in the Boussinesq wave model, FUNWAVE-TVD. Ocean. Model. 2018, 131, 86–100. [Google Scholar] [CrossRef]
- Kamphuis, J.W. Incipient wave breaking. Coast. Eng. 1991, 15, 185–203. [Google Scholar] [CrossRef]
- FLOW-3D®; Version 12.0; Flow Science, Inc.: Santa Fe, NM, USA, 2019.
- Buccino, M.; Daliri, M.; Dentale, F.; Di Leo, A.; Calabrese, M. CFD experiments on a low crested sloping top caisson breakwater. Part 1. nature of loadings and global stability. Ocean. Eng. 2019, 182, 259–282. [Google Scholar] [CrossRef]
- Buccino, M.; Daliri, M.; Dentale, F.; Calabrese, M. CFD experiments on a low crested sloping top caisson breakwater. Part 2. Analysis of plume impact. Ocean. Eng. 2019, 173, 345–357. [Google Scholar] [CrossRef]
- Di Leo, A.; Buccino, M.; Dentale, F.; Pugliese Carratelli, E. CFD Analysis of Wind Effect on Wave Overtopping. In Proceedings of the 32nd International Ocean and Polar Engineering Conference, Shanghai, China, 5–10 June 2022. [Google Scholar]
- Cordova Lopez, L.F.; Salerno, D.; Dentale, F.; Capobianco, A.; Buccino, M. Wave overtopping at Malecòn tradicional, La Habana, Cuba. Coast. Eng. Proc. 2016, 35, 24–39. [Google Scholar]


| m [-] | s0 [-] | Δx [m] |
|---|---|---|
| 0.1 | 0.01; 0.03; 0.05 | 0.5 |
| 0.033 | 0.01; 0.03; 0.05 | 1.0 |
| 0.025 | 0.01; 0.03; 0.05 | 1.0 |
| 0.02 | 0.01; 0.03; 0.05 | 2.0 |
| 0.0125 | 0.01; 0.03; 0.05 | 2.0 |
| 0.01 | 0.01; 0.03; 0.05 | 2.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tuozzo, S.; Di Leo, A.; Buccino, M.; Calabrese, M. Boussinesq Modelling of Shallow Water Phenomena. Environ. Sci. Proc. 2022, 21, 64. https://doi.org/10.3390/environsciproc2022021064
Tuozzo S, Di Leo A, Buccino M, Calabrese M. Boussinesq Modelling of Shallow Water Phenomena. Environmental Sciences Proceedings. 2022; 21(1):64. https://doi.org/10.3390/environsciproc2022021064
Chicago/Turabian StyleTuozzo, Sara, Angela Di Leo, Mariano Buccino, and Mario Calabrese. 2022. "Boussinesq Modelling of Shallow Water Phenomena" Environmental Sciences Proceedings 21, no. 1: 64. https://doi.org/10.3390/environsciproc2022021064
APA StyleTuozzo, S., Di Leo, A., Buccino, M., & Calabrese, M. (2022). Boussinesq Modelling of Shallow Water Phenomena. Environmental Sciences Proceedings, 21(1), 64. https://doi.org/10.3390/environsciproc2022021064





