The Swelling–Shrinkage Properties of Intact and Disturbed Clayey and Marly Soils: The Density Effect
Abstract
:1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Sample Preparation
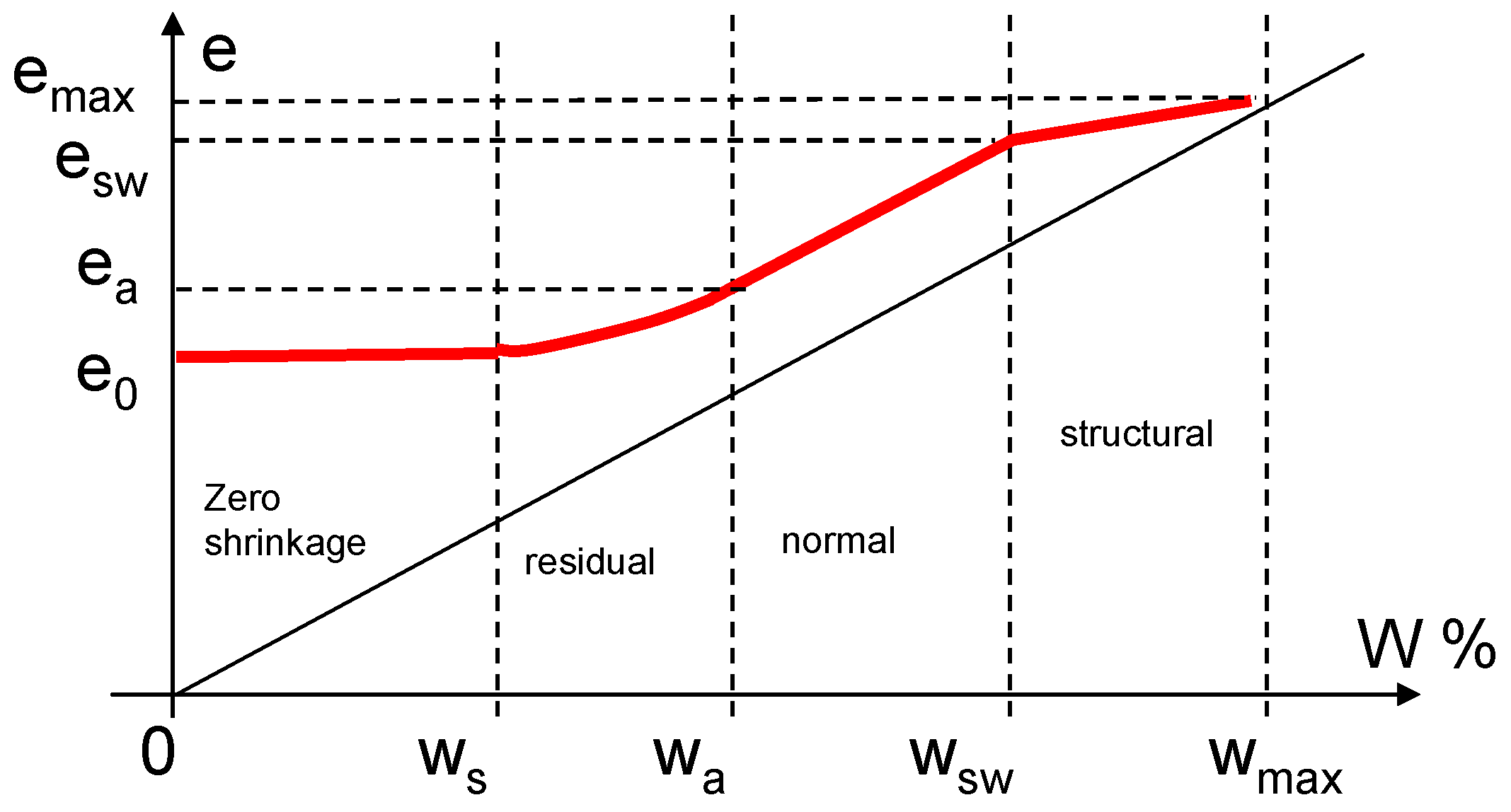
2.3. The swelling and Shrinkage Protocol
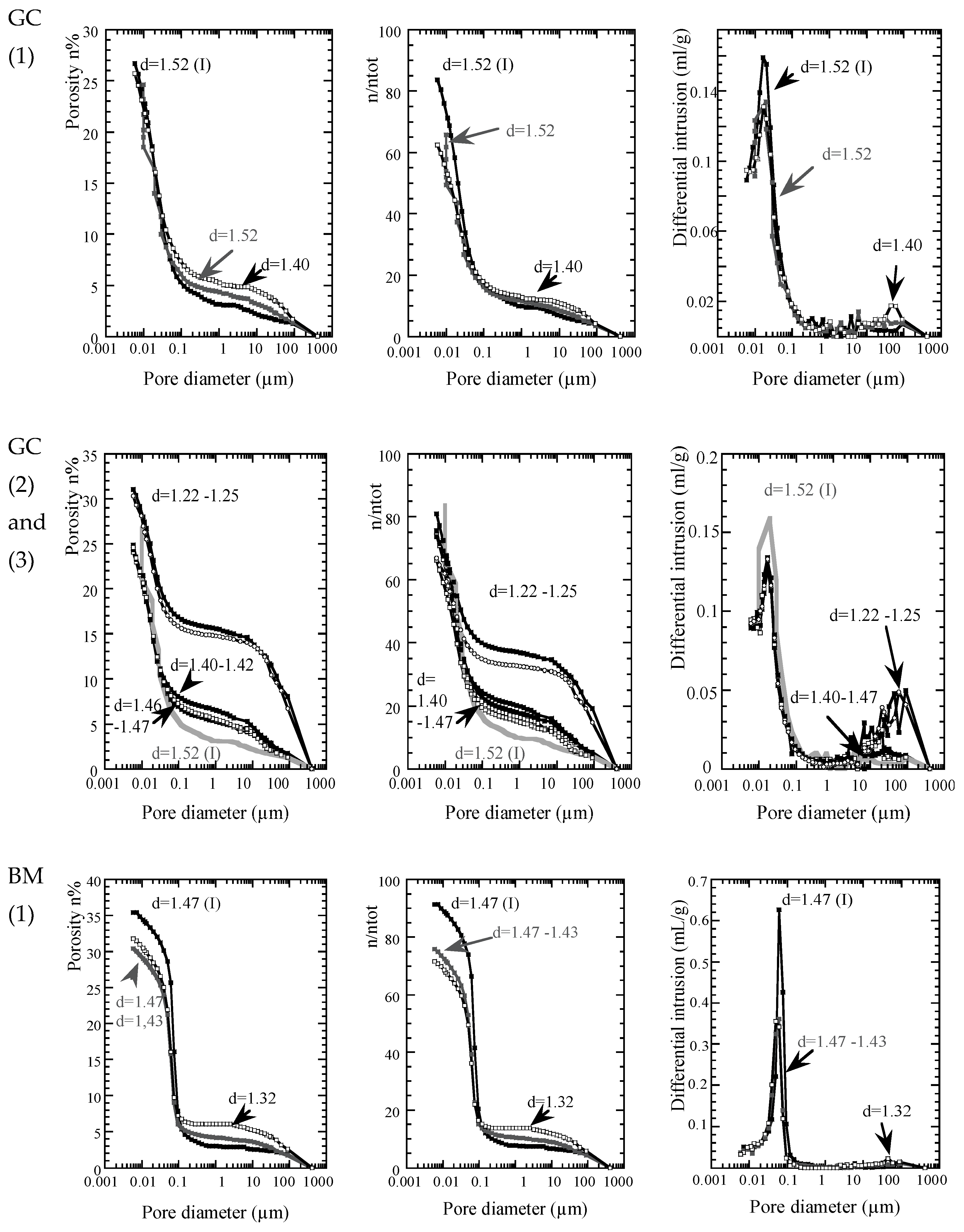
2.4. Microstructural Observations
3. Results and Discussion
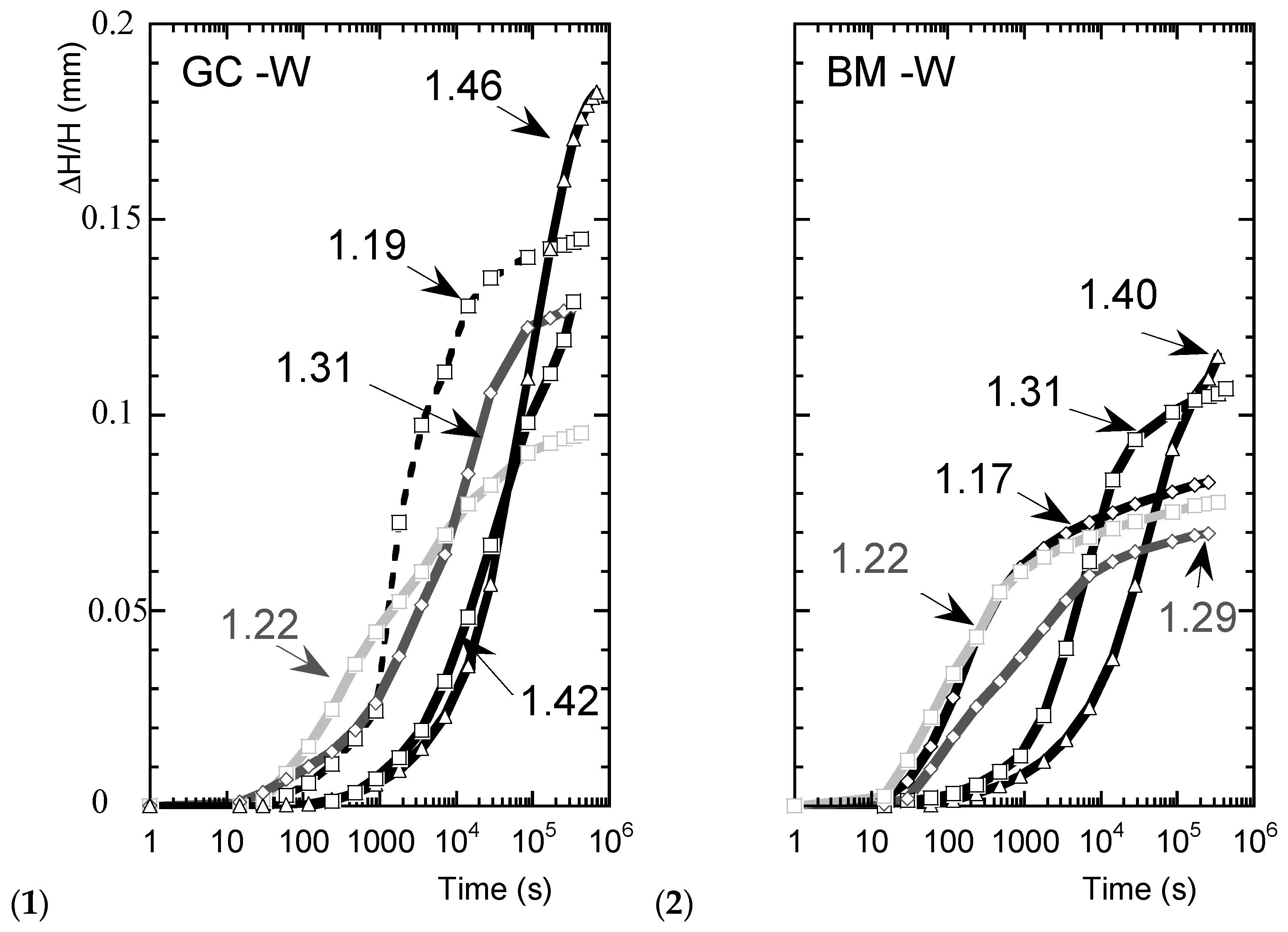
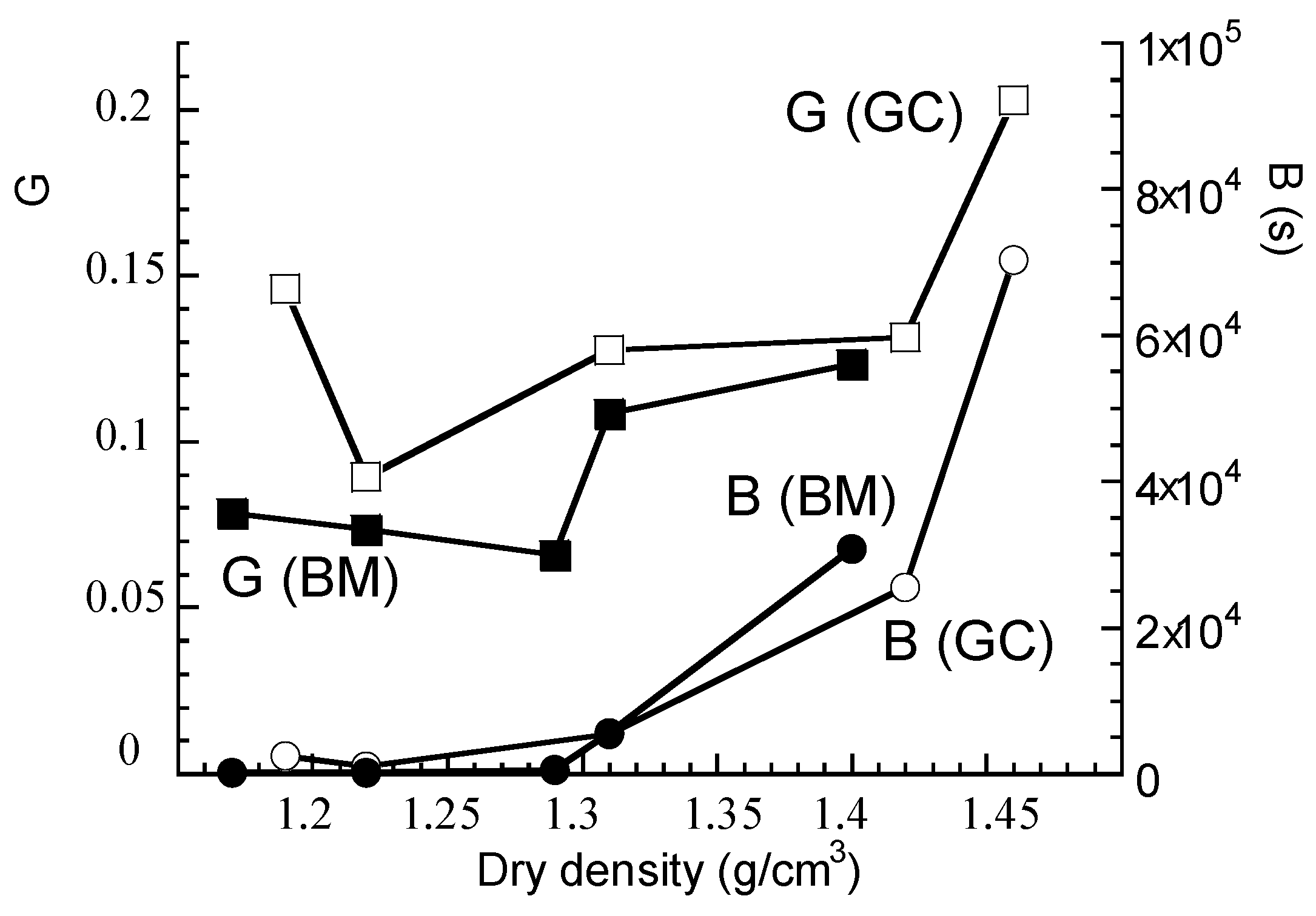
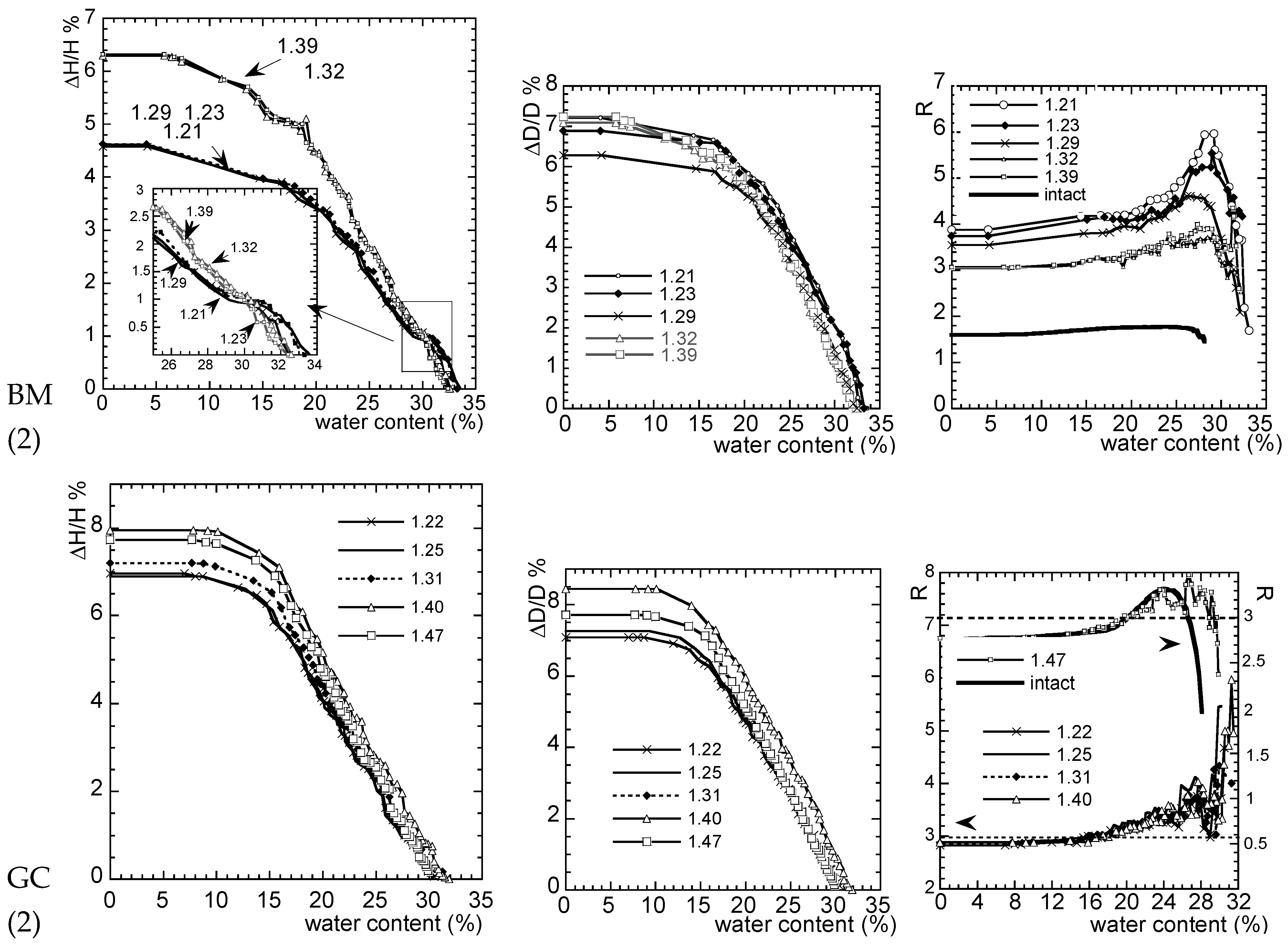
3.1. The Swelling of Statically Compacted W-Disturbed Soils at Various Densities
3.2. Effect of the Density on Shrinkage Amplitude Measured on Statically Compacted W-Disturbed Soils (Test Is Preceded or Not by a Free Swelling)
- a variability in the local composition of the sample (in particular, the calcium carbonate content of marl samples—the material may come from different natural blocks extracted from the quarry);
- a variability in the preparation by the manipulator during the step of manual mixing in the hermetic bag before compaction or during the compaction step (i.e., the way to filling the oedometer ring…), or a variability in the particle size distribution of the ground soil after drying.
3.3. Effect of the Density on Shrinkage Amplitude on Dynamically Compacted W- or C-Disturbed Soils (Test Is Preceded by a Free Swelling)
4. Conclusions
- The dry density remains a major parameter that governs the swelling–shrinkage behavior of the soil. The denser the soil is (the soil is characterized by a low void ratio or by low initial water content), the lower the amplitude of shrinkage, and the higher the swelling amplitude appears. On the contrary, looser soils (above all those combined with high-moisture content) favor a high shrinkage strain amplitude, while the swelling deformation decreases (as swelling clay may expand into the soil porosity without modifying the whole sample volume). However, some data sets may not follow this trend and their behavior (even if an explanation may be found) needs to be confirmed by testing other soils. In all cases, dry density should be taken into account in the swelling–shrinkage soil classification;
- The study of intact soils is recommended, especially when the material is characterized by a cemented microstructure, as in the presence of carbonates in marl. Nevertheless, the work on disturbed samples in this paper demonstrated that the shrinkage behavior of intact soils can be obtained from the testing of disturbed soils (extrapolation of swelling behavior gives results with poor accuracy). This is an interesting element to consider in order to develop a methodology for predicting the behavior of intact soils when the direct testing of undisturbed soils is not possible;
- The mode of compaction (static or dynamic) clearly has an effect on the amplitude of swelling–shrinkage (for similar dry density). Further study at microscale will need to distinguish the soil microstructure responsible for the observed behavior;
- The results indicate also that conducting a swelling test on W-disturbed samples and a shrinkage test on C-disturbed samples allows us to assess more closely the behavior of intact soil samples. Finally, in situations where working with undisturbed soils is not feasible or too costly, disturbed samples can be used.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Holtz Wesley, G.; Gibbs Harold, J. Engineering Properties of Expansive Clays. Trans. Am. Soc. Civ. Eng. 1956, 121, 641–663. [Google Scholar] [CrossRef]
- Seed, H.B.; Woodward Richard, J.; Lundgren, R. Prediction of Swelling Potential for Compacted Clays. J. Soil Mech. Found. Div. 1962, 88, 53–87. [Google Scholar] [CrossRef]
- Gromko Gerald, J. Review of Expansive Soils. J. Geotech. Eng. Div. 1974, 100, 667–687. [Google Scholar] [CrossRef]
- Djeran-Maigre, I.; Tessier, D.; Grunberger, D. Importance de La Nature Minéralogique et de La Texture Des Argiles Dans Leur Comportement Mécanique et Hydraulique. In Proceedings of the Colloque Magi, Nancy, France, 21–22 September 1998; pp. 87–92. [Google Scholar]
- Al-Shayea, N.A. The Combined Effect of Clay and Moisture Content on the Behavior of Remolded Unsaturated Soils. Eng. Geol. 2001, 62, 319–342. [Google Scholar] [CrossRef]
- Mitchell James, K.; Hooper Don, R.; Campenella Richard, G. Permeability of Compacted Clay. J. Soil Mech. Found. Div. 1965, 91, 41–65. [Google Scholar] [CrossRef]
- Christodoulias, J. Engineering Properties and Shrinkage Limit of Swelling Soils in Greece. J. Earth Sci. Clim. Chang. 2015, 6, 279. [Google Scholar]
- Delage, P.; Audiguier, M.; Cui, Y.-J.; Howat, M.D. Microstructure of a Compacted Silt. Can. Geotech. J. 1996, 33, 150–158. [Google Scholar] [CrossRef]
- Audiguier, M.; Geremew, Z.; Laribi, S.; Cojean, R. Caractérisation Au Laboratoire de La Sensibilité Au Retrait-Gonflement Des Sols Argileux. Rev. Fr. Géotech. 2007, 120–121, 67–82. [Google Scholar] [CrossRef]
- Diamond, S. Pore Size Distributions in Clays. Clays Clay Miner. 1970, 18, 7–23. [Google Scholar] [CrossRef]
- Sridharan, A.; Altschaefflf, A.; Diamond, S. Pore Size Distribution Studies. J. Soil Mech. Found. Div. 1971, 97, 771–787. [Google Scholar] [CrossRef]
- Delage, P.; Lefebvre, G. Study of the Structure of the Sensitive Champlain Clay and of Its Evolution during Consolidation. Can. Geotech. J. 1984, 21, 21–35. [Google Scholar] [CrossRef]
- Kouassi, P.; Poulin, D.; Girard, H.; Breysse, D. Etude Comparative de Différentes Méthodes de Compactage Par Pétrissage En Laboratoire: Application Au Limon de Xeuilley. In Proceedings of the Magi’50, Nancy, France, 21–22 September 1998; pp. 191–196. [Google Scholar]
- Albrecht Brian, A.; Benson Craig, H. Effect of Desiccation on Compacted Natural Clays. J. Geotech. Geoenviron. Eng. 2001, 127, 67–75. [Google Scholar] [CrossRef]
- Jain, A.K.; Punmia, B.C.; Jain, E.A.K. Soil Mechanics and Foundations; Laxmi Publications: New Delhi, India, 2005. [Google Scholar]
- Villar, M.V.; Lloret, A. Influence of Dry Density and Water Content on the Swelling of a Compacted Bentonite. Appl. Clay Sci. 2008, 39, 38–49. [Google Scholar] [CrossRef]
- Dejong, E.D.; Warkentin, B.P. Shrinkage of Soil Samples with Varying Clay Concentration. Can. Geotech. J. 1965, 2, 16–22. [Google Scholar] [CrossRef]
- Kleppe, J.; Olson, R.E. Desiccation Cracking of Soil Barriers; ASTM: Philadelphia, PA, USA, 1985; pp. 263–275. [Google Scholar]
- Daniel David, E.; Wu, Y.-K. Compacted Clay Liners and Covers for Arid Sites. J. Geotech. Eng. 1993, 119, 223–237. [Google Scholar] [CrossRef]
- Komine, H.; Ogata, N. Experimental Study on Swelling Characteristics of Sand-Bentonite Mixture for Nuclear Waste Disposal. Soils Found. 1999, 39, 83–97. [Google Scholar] [CrossRef] [PubMed]
- Mrad, M. Modelling the Hydromechanical Behavior of Swelling Unsaturated Soils. Ph.D. Thesis, Institut National Polytechnique, Nancy, France, 2005. [Google Scholar]
- Louafi, B.; Dafalla, M. Moisture and Dry Density Influence on Compacted Clay and Clay-Sand Mixtures. Rev. Compos. Matériaux Avancés 2022, 32, 33. [Google Scholar] [CrossRef]
- Hamilton, J.J. Foundations on Swelling or Shrinking Subsoils; Canadian Building Digest; no. CBD-184; National Research Council: Ottawa, ON, Canada, 1977. [Google Scholar] [CrossRef]
- Subba Rao, K.S. Swell-shrink behaviour of expansive soils-geotechnical challenges. Indian Geotech. J. 2000, 30, 1–68. [Google Scholar]
- Gharsalli, J.; Mzali, H. Hazard susceptibility related to clay shrinkage–swelling phenomena in north-eastern Tunisia (Grombalia area). Model. Earth Syst. Environ. 2017, 3, 963–976. [Google Scholar] [CrossRef]
- Li, Z.S.; Benchouk, A.; Derfouf, F.E.M.; Abou-Bekr, N.; Taibi, S.; Souli, H.; Fleureau, J.M. Global representation of the drying–wetting curves of four engineering soils: Experiments and correlations. Acta Geotech. 2018, 13, 51–71. [Google Scholar] [CrossRef]
- NF P 94-093; Sols: Reconnaissance et Essais—Détermination Des Références de Compactage d’un Matériau Essai Proctor Normal. Essai Proctor Modifié, AFNOR: Paris, France, 2014.
- Ferber, V.; Auriol, J.-C.; Cui, Y.-J.; Magnan, J.-P. Wetting-Induced Volume Changes in Compacted Silty Clays and High-Plasticity Clays. Can. Geotech. J. 2008, 45, 252–265. [Google Scholar] [CrossRef]
- ASTM D 4546-03; Standard Test Methods for One-Dimensional Swell or Settlement Potential of Cohesive Soils. ASTM International: West Conshohocken, PA, USA, 2003.
- AS 1289.7.1.1; Method of Testing Soils for Engineering Purpose. Standards Australia: Sydney, Australia, 2003.
- Alonso, E.E.; Lloret, A.; Gens, A.; Battle, F. A New Approach for the Prediction of Long Term Heave. In Proceedings of the Congrès International de Mécanique des sols et des Travaux de Fondations, Rio de Janeiro, Brazil, 13 August 1989; Volume 1, pp. 571–574. [Google Scholar]
- Alonso, E.E.; Lloret, A.; Gens, A. Double Structure Model for the Prediction of Long Term Movements in Expansive Materials. In Geomechanics; Beer, G., Booker, J.R., Carter, J.P., Eds.; Balkema: Rotterdam, The Netherlands, 1991; Volume 1, pp. 541–548. [Google Scholar]
- Parcevaux, P. Etude Microscopique et Macroscopique Du Gonflement de Sols Argileux; Université de Paris VI/Ecole Nationale Supérieure des Mines de Paris: Paris, France, 1980. [Google Scholar]
- Sridharan, A.; Rao, A.; Puvvadi, S. Swelling Pressure of Clays. Geotech. Test. J. 1986, 9, 24–33. [Google Scholar] [CrossRef]
- Chaney, R.; Demars, K.; Rao, K.; Rao, S.; Gangadhara, S. Swelling Behavior of a Desiccated Clay. Geotech. Test. J. 2000, 23, 193–198. [Google Scholar] [CrossRef]
- Mollins, L.H. The Design of Bentonite-Sand Mixtures. Doctoral Dissertation, University of Leeds, Leeds, UK, 1996. [Google Scholar]
- Rao, A.S.; Phanikumar, B.R.; Sharma, R.S. Prediction of Swelling Characteristics of Remoulded and Compacted Expansive Soils Using Free Swell Index. Q. J. Eng. Geol. Hydrogeol. 2004, 37, 217–226. [Google Scholar] [CrossRef]
- Tripathy, S.; Rao, K.S.; Fredlund, D.G. Water Content—Void Ratio Swell-Shrink Paths of Compacted Expansive Soils. Can. Geotech. J. 2002, 39, 938–959. [Google Scholar] [CrossRef]
- Cornelis, W.M.; Corluy, J.; Medina, H.; Díaz, J.; Hartmann, R.; Van Meirvenne, M.; Ruiz, M.E. Measuring and Modelling the Soil Shrinkage Characteristic Curve. Geoderma 2006, 137, 179–191. [Google Scholar] [CrossRef]
- XP P 94-060-2; Essai de Dessiccation: Partie 2: Détermination Effective de La Limite de Retrait Sur Un Prélèvement Non Remanié. AFNOR: Paris, France, 1997.
- Peng, X.; Zhang, Z.B.; Wang, L.L.; Gan, L. Does Soil Compaction Change Soil Shrinkage Behavior? Soil Tillage Res. 2012, 125, 89–95. [Google Scholar] [CrossRef]
- Monroy, R.; Zdrakovic, L.; Ridley, A. Evolution of Microstructure in Compacted London Clay during Wetting and Loading. Géotechnique 2010, 60, 105–119. [Google Scholar] [CrossRef]
- Alaoui, A.; Lipiec, J.; Gerke, H.H. A Review of the Changes in the Soil Pore System Due to Soil Deformation: A Hydrodynamic Perspective. Soil Tillage Res. 2011, 115–116, 1–15. [Google Scholar] [CrossRef]
- Mbemba, F.S. Évaluation de La Dessiccation, Du Retrait et de La Fissuration de Matériaux Silteux Peu Plastiques. Master’s Thesis, École Polytechnique de Montréal, Montréal, QC, Canada, 2010. [Google Scholar]
- Ying, Z.; Cui, Y.J.; Benahmed, N.; Duc, M. Drying effect on the microstructure of compacted salted silt. Géotechnique 2023, 73, 62–70. [Google Scholar] [CrossRef]
- Chertkov, V. The Shrinkage Geometry Factor of a Soil Layer. Soil Sci. Soc. Am. J. 2005, 69, 1671–1683. [Google Scholar] [CrossRef]
- Ferber, V. Sensibilité Des Sols Fins Compactés à l’humidification: Apport d’un Modèle de Microstructure; Ecole Centrale Nantes: Nantes, France, 2005. [Google Scholar]
- Fabre, R.; Kouassi, P.; Riss, J. Variabilité de Propriétés Physiques Des Sols Argileux à Différents Échelles: Application à Des Sols Compactés Utilisés Pour La Construction de Barrages; Journée métier Géo-Entreprise: Nancy, France, 1998; pp. 27–31. [Google Scholar]
- Serratrice, J.F. Retrait-Gonflement Des Sols Argileux et Des Marnes. Rev. Fr. Géotech. 2007, 120–121, 107–120. [Google Scholar] [CrossRef]


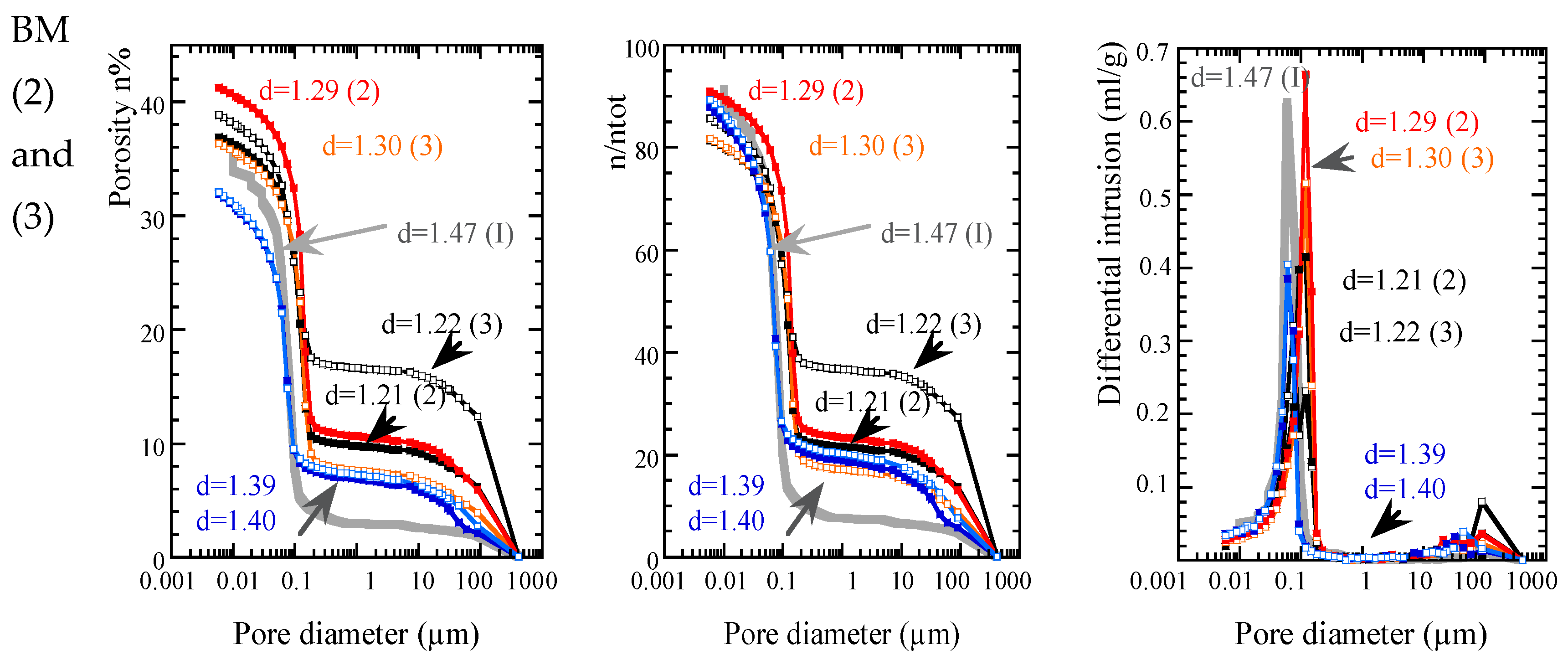



| Specimen Name | Preparation | Compaction Mode | Test | Size of the Cylindrical Specimen (mm) | wo % | Initial Density ρ0 (g/cm3) | Initial Dry Density ρd,0 (g/cm3) | |
|---|---|---|---|---|---|---|---|---|
| (1) | GC (1) BM (1) | I, W, C I, W, C | Static | Sw(S) Sh (S) (A) | D 50 H 30 | 27 30 | 1.75–1.98 1.77–1.97 | 1.34–1.56 1.32–1.56 |
| (2) | GC (2) BM (2) | W W | Static | Sh(US) (A) | D 50 H 30 | 30 32.5 | 1.22–1.48 1.21–1.39 | 1.59–1.92 1.61–1.84 |
| (3) | GC (3) BM (3) | W W | Static | Sw (US) followed by Sh (A) | D 50 H 30 | 30 32.5 | 1.22–1.48 1.21–1.39 | 1.59–1.92 1.61–1.84 |
| (4) | GC (4) BM (4) | W, C W, C | Dynamic | Sw (US) followed by Sh (M) | D 70 H19 D 60 H 20 | 27 30 | 1.42–1.78 1.65–1.86 | 1.14–1.40 1.24–1.43 |
| Initial State | Shrunk State | |
|---|---|---|
| GC (1) | −0.9 | −0.76 |
| GC (2) | −1.19 | −1.39 |
| GC (3) | −1.47 | −1.39 |
| GC (4) | −1.76 to −1.84 | −0.30 to −0.48 |
| BM (1) | −0.81 | −0.52 |
| BM (2) | −1.36 | −1.56 |
| BM (3) | −1.36 | −1.16 |
| BM (4) wi = 27% | −1.60 | −1.15 |
| BM (4) wi = 30% | −1.48 | −0.70 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Makki, L.; Duc, M.; Coppée, T.; Szymkiewicz, F. The Swelling–Shrinkage Properties of Intact and Disturbed Clayey and Marly Soils: The Density Effect. Geotechnics 2024, 4, 512-529. https://doi.org/10.3390/geotechnics4020028
Makki L, Duc M, Coppée T, Szymkiewicz F. The Swelling–Shrinkage Properties of Intact and Disturbed Clayey and Marly Soils: The Density Effect. Geotechnics. 2024; 4(2):512-529. https://doi.org/10.3390/geotechnics4020028
Chicago/Turabian StyleMakki, Lamis, Myriam Duc, Thibault Coppée, and Fabien Szymkiewicz. 2024. "The Swelling–Shrinkage Properties of Intact and Disturbed Clayey and Marly Soils: The Density Effect" Geotechnics 4, no. 2: 512-529. https://doi.org/10.3390/geotechnics4020028
APA StyleMakki, L., Duc, M., Coppée, T., & Szymkiewicz, F. (2024). The Swelling–Shrinkage Properties of Intact and Disturbed Clayey and Marly Soils: The Density Effect. Geotechnics, 4(2), 512-529. https://doi.org/10.3390/geotechnics4020028










