Synthesis and Physical Properties of NbMnP Single Crystals
Abstract
:1. Introduction
2. Experimental Methods
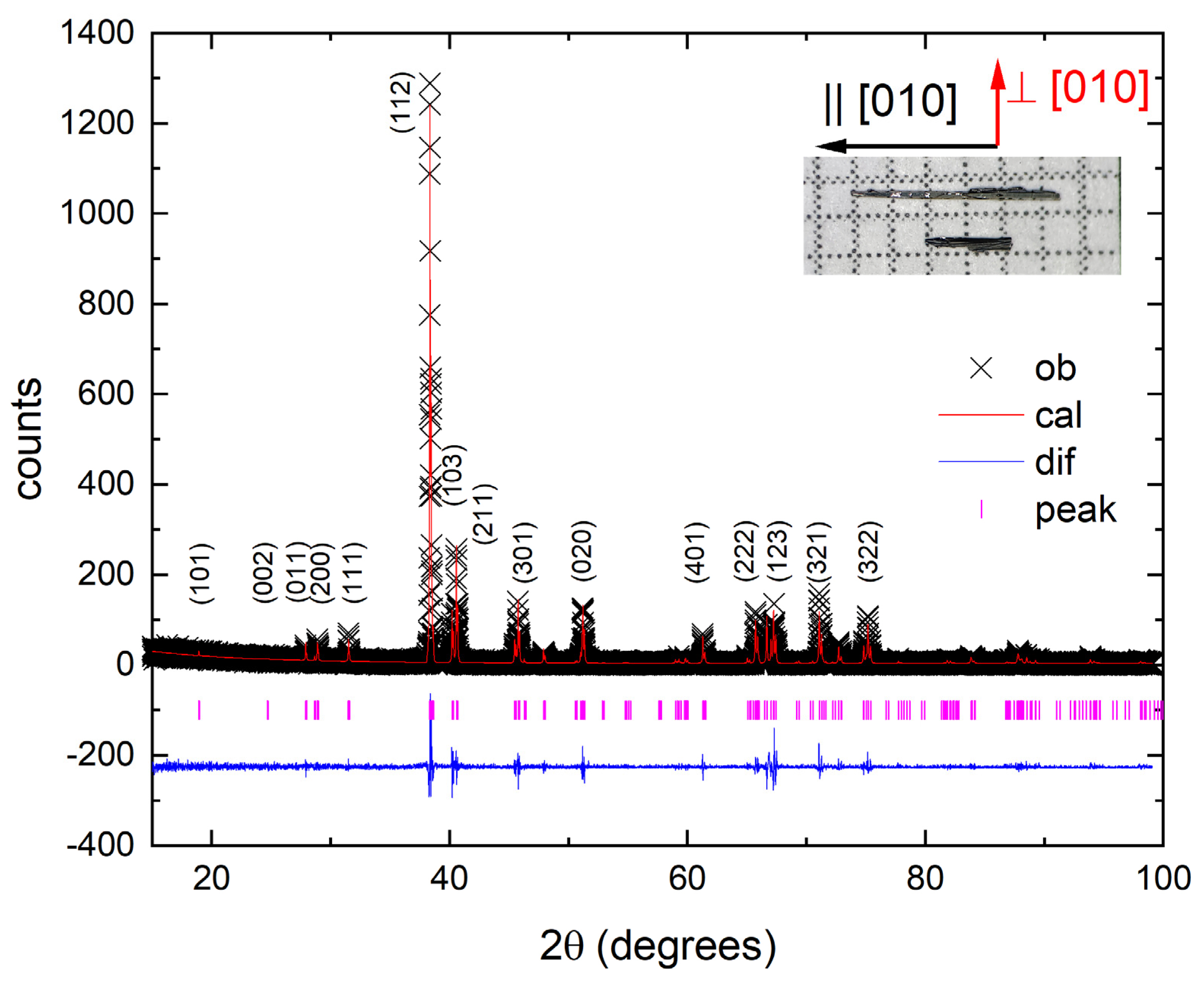
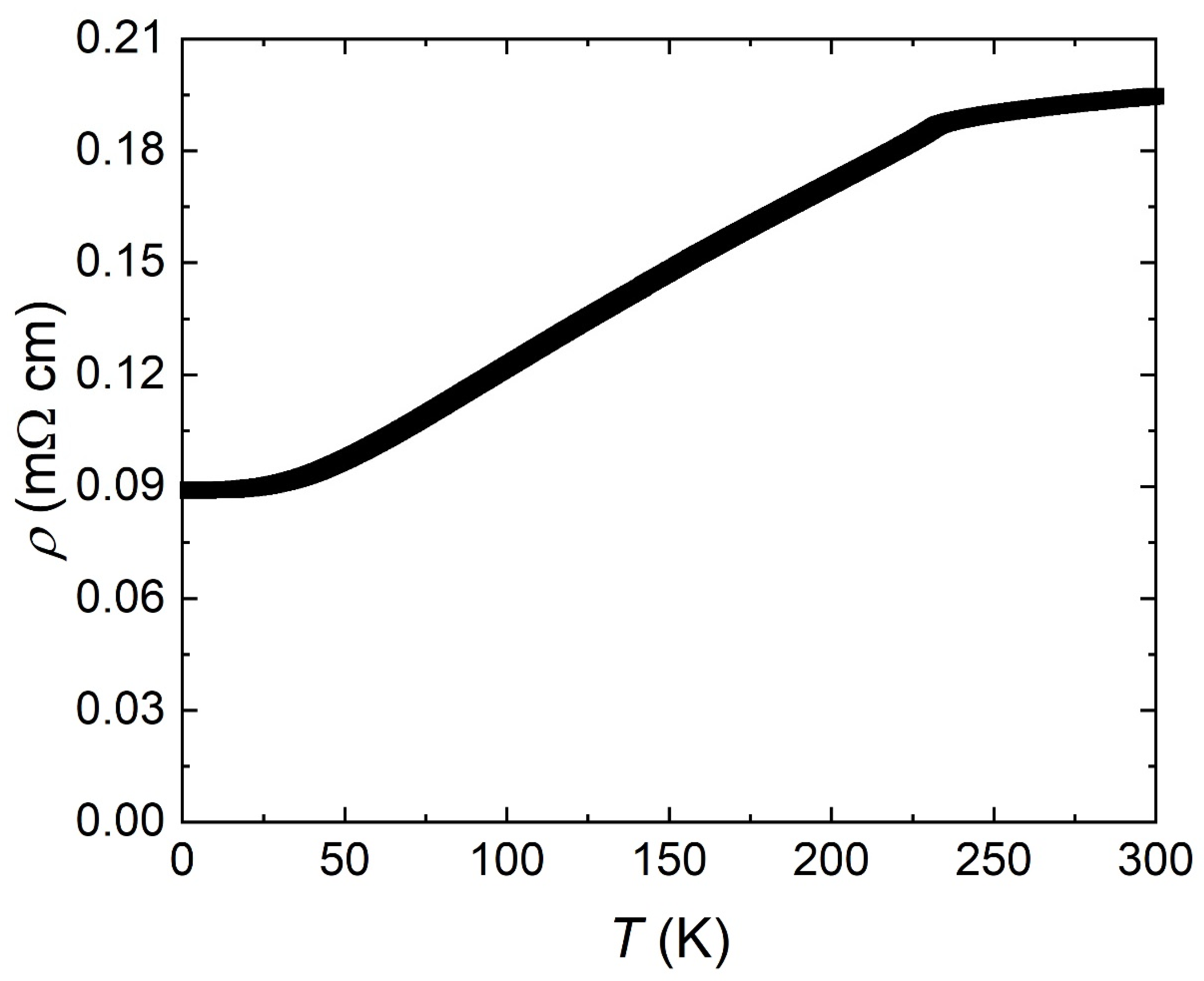
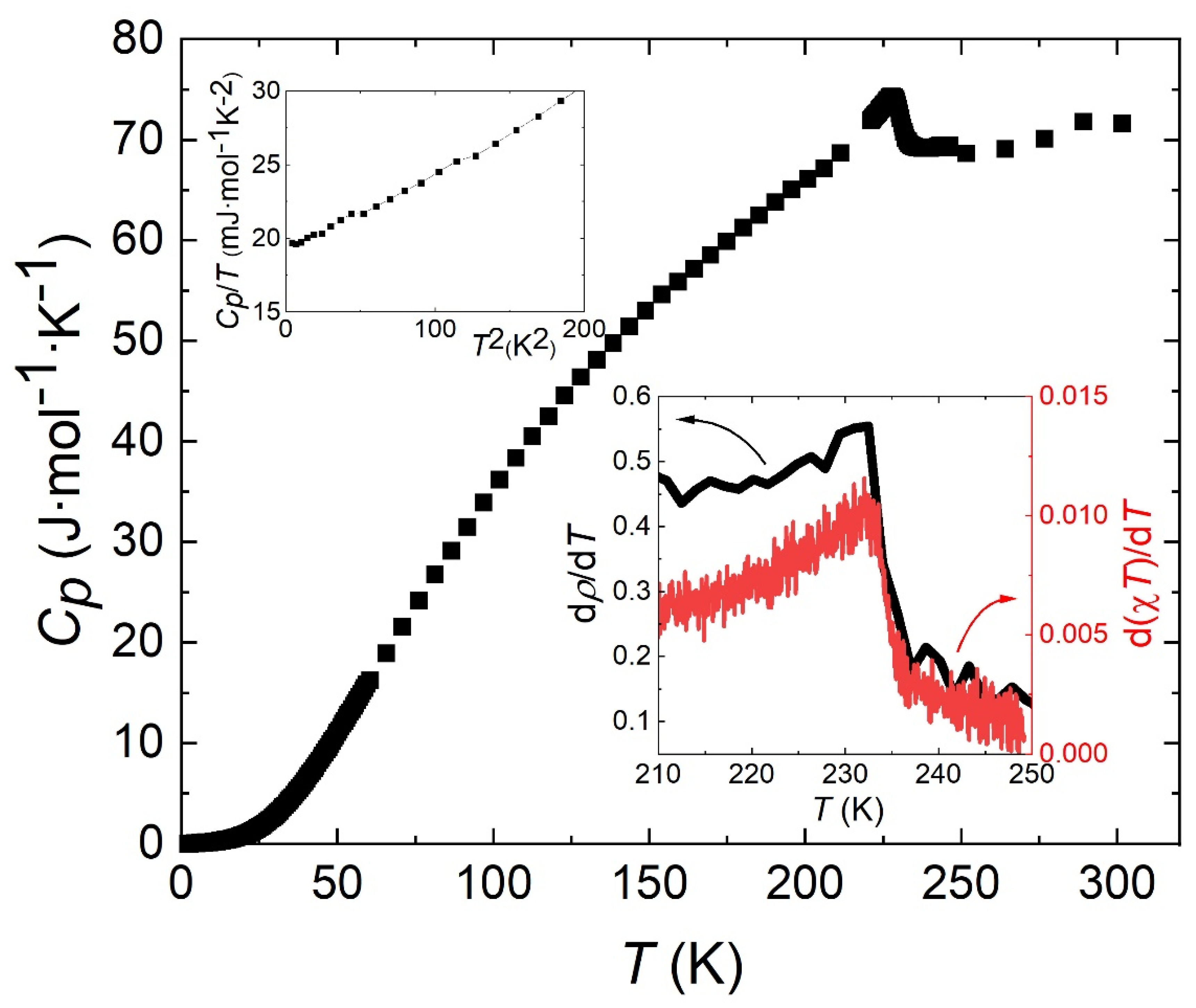
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Anderson, P.W. Localized Magnetic States in Metals. Phys. Rev. 1961, 124, 41. [Google Scholar] [CrossRef]
- Anderson, P.W. The Resonating Valence Bond State in La2CuO4 and Superconductivity. Science 1987, 235, 1196–1198. [Google Scholar] [CrossRef] [PubMed]
- Ramirez, A.P. Strongly Geometrically Frustrated Magnets. Annu. Rev. Mater. Sci. 1994, 24, 453–480. [Google Scholar] [CrossRef]
- Canfield, P.C. New materials physics. Rep. Prog. Phys. 2020, 83, 016501. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Lomnytska, Y.F.; Kuz’ma, Y.B. New phosphides of IVa and Va group metals with TiNiSi-type. J. Alloys Compd. 1998, 269, 133–137. [Google Scholar] [CrossRef]
- Lamichhane, T.N.; Taufour, V.; Masters, M.W.; Parker, D.S.; Kaluarachchi, U.S.; Thimmaiah, S.; Bud’Ko, S.L.; Canfield, P.C. Discovery of ferromagnetism with large magnetic anisotropy in ZrMnP and HfMnP. Appl. Phys. Lett. 2016, 109, 092402. [Google Scholar] [CrossRef] [Green Version]
- Welter, R.; Venturini, G.; Ijjaali, I.; Malaman, B. Magnetic study of the new TiNiSi-type TbMnSi, DyMnSi and NdMnGe compounds. J. Magn. Magn. Mater. 1999, 205, 221. [Google Scholar] [CrossRef]
- Müller, R.; Shelton, R.N.; Richardson, J.W.; Jacobson, R.A.J. Superconductivity and crystal structure of a new class of ternary transition metal phosphides TT′P (T ≡ Zr, Nb, Ta and T′ ≡ Ru, Rh). Less-Comm. Met. 1983, 92, 177–183. [Google Scholar] [CrossRef]
- Matsuda, M.; Zhang, D.; Kuwata, Y.; Zhang, Q.; Sakurai, T.; Ohta, H.; Sugawara, H.; Takeda, K.; Hayashi, J.; Kotegawa, H. Noncollinear spin structure with weak ferromagnetism in NbMnP. Phys. Rev. B 2021, 104, 174413. [Google Scholar] [CrossRef]
- Canfield, P.C.; Fisk, Z. Growth of single crystals from metallic fluxes. Phil. Mag. B 1992, 65, 1117. [Google Scholar] [CrossRef]
- Canfield, P.C.; Kong, T.; Kaluarachchi, U.S.; Jo, N.H. Use of frit-disc crucibles for routine and exploratory solution growth of single crystalline samples. Philos. Mag. 2016, 96, 84–92. [Google Scholar] [CrossRef] [Green Version]
- Toby, B.H. EXPGUI, a graphical user interface for GSAS. J. Appl. Crystallogr. 2001, 34, 210–213. [Google Scholar] [CrossRef] [Green Version]
- Larson, A.C.; Von Dreele, R.B. General Structure Analysis System (GSAS). In Los Alamos National Laboratory Report LAUR 86-748; Los Alamos National Laboratory: Los Alamos, NM, USA, 2000. [Google Scholar]
- Lomnitskaya, Y.F.; Kondratyuk, G.D.; Zakharets, L.I. Niobium and phosphorus interaction with manganese and copper. Zh. Neorg. Khim. 1988, 33, 734–737. [Google Scholar]
- Kong, T.; Cunningham, C.E.; Taufour, V.; Bud’ko, S.L.; Buffon, M.L.C.; Lin, X.; Emmons, H.; Canfield, P.C. Thermodynamic and transport properties of single crystalline RCo2Ge2 (R = Y, La–Nd, Sm–Tm). J. Magn. Magn. Mater. 2014, 358–359, 212. [Google Scholar] [CrossRef] [Green Version]
- Fisher, M.E.; Langer, J.S. Resistive Anomalies at Magnetic Critical Points. Phys. Rev. Lett. 1968, 20, 665. [Google Scholar] [CrossRef]
- Fisher, M.E. Relation between the specific heat and susceptibility of an antiferromagnet. Philos. Mag. 1962, 7, 1731–1743. [Google Scholar] [CrossRef]
- Tari, A. The Specific Heat of Matter at Low Temperatures; World Scientific: Singapore, 2003. [Google Scholar]

| Empirical Formula | NbMnP |
|---|---|
| Formula weight | 178.82 |
| Temperature | 299 (2) K |
| Wavelength | 0.71073 Å |
| Crystal system, space group | Orthorhombic, Pnma |
| Unit cell dimensions | a = 6.184 (7) Å b = 3.560 (4) Å c = 7.217 (9) Å |
| Volume | 158.9 (3) |
| Z, Calculated density | 4 |
| Absorption coefficient | 15.508 |
| F (000) | 324 |
| Crystal size | ~20 µm × 20 µm × 20 µm |
| θ range (°) | 4.340 to 34.946 |
| Limiting indices | h = −9 to 7 k = −5 to 5 l = −9 to 9 |
| Reflection collected | 2316 |
| Independent reflections | 393 |
| Completeness to θ = 25.242° | 100 |
| Absorption correction | None |
| Refinement method | Full-matrix least-squares on |
| Data/restraints/parameters | 393/0/20 |
| Goodness-of-fit on | 1.173 |
| Final R indices [I > 2 (I)] | R1 = 0.0365, wR2 = 0.0577 |
| R indices (all data) | R1 = 0.0460, wR2 = 0.0598 |
| Extinction coefficient | 0.042 (3) |
| Largest diff. peak and hole | 1.735 and −2.419 e. |
| Atom | Wyckoff. | Occ. | x | y | z | Ueq |
|---|---|---|---|---|---|---|
| Nb1 | 4c | 1 | 0.03093 (9) | ¼ | 0.67220 (9) | 0.0048 (2) |
| Mn2 | 4c | 1 | 0.14117 (15) | ¼ | 0.05925 (15) | 0.0034 (2) |
| P3 | 4c | 1 | 0.2676 (3) | ¼ | 0.3695 (2) | 0.0041 (3) |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, J.; Shu, Z.; Mudiyanselage, R.S.D.; Xie, W.; Kong, T. Synthesis and Physical Properties of NbMnP Single Crystals. Magnetism 2022, 2, 179-185. https://doi.org/10.3390/magnetism2020013
Zhao J, Shu Z, Mudiyanselage RSD, Xie W, Kong T. Synthesis and Physical Properties of NbMnP Single Crystals. Magnetism. 2022; 2(2):179-185. https://doi.org/10.3390/magnetism2020013
Chicago/Turabian StyleZhao, Jianda, Zhixue Shu, Ranuri S. Dissanayaka Mudiyanselage, Weiwei Xie, and Tai Kong. 2022. "Synthesis and Physical Properties of NbMnP Single Crystals" Magnetism 2, no. 2: 179-185. https://doi.org/10.3390/magnetism2020013
APA StyleZhao, J., Shu, Z., Mudiyanselage, R. S. D., Xie, W., & Kong, T. (2022). Synthesis and Physical Properties of NbMnP Single Crystals. Magnetism, 2(2), 179-185. https://doi.org/10.3390/magnetism2020013






