Multiscale Simulation on Product Distribution from Pyrolysis of Styrene-Butadiene Rubber
Abstract
:1. Introduction
2. Computational Details
2.1. DFT Calculations
2.2. ReaxFF MD Simulations
3. Results and Discussions
3.1. Analysis of Bond Dissociation Energy by DFT
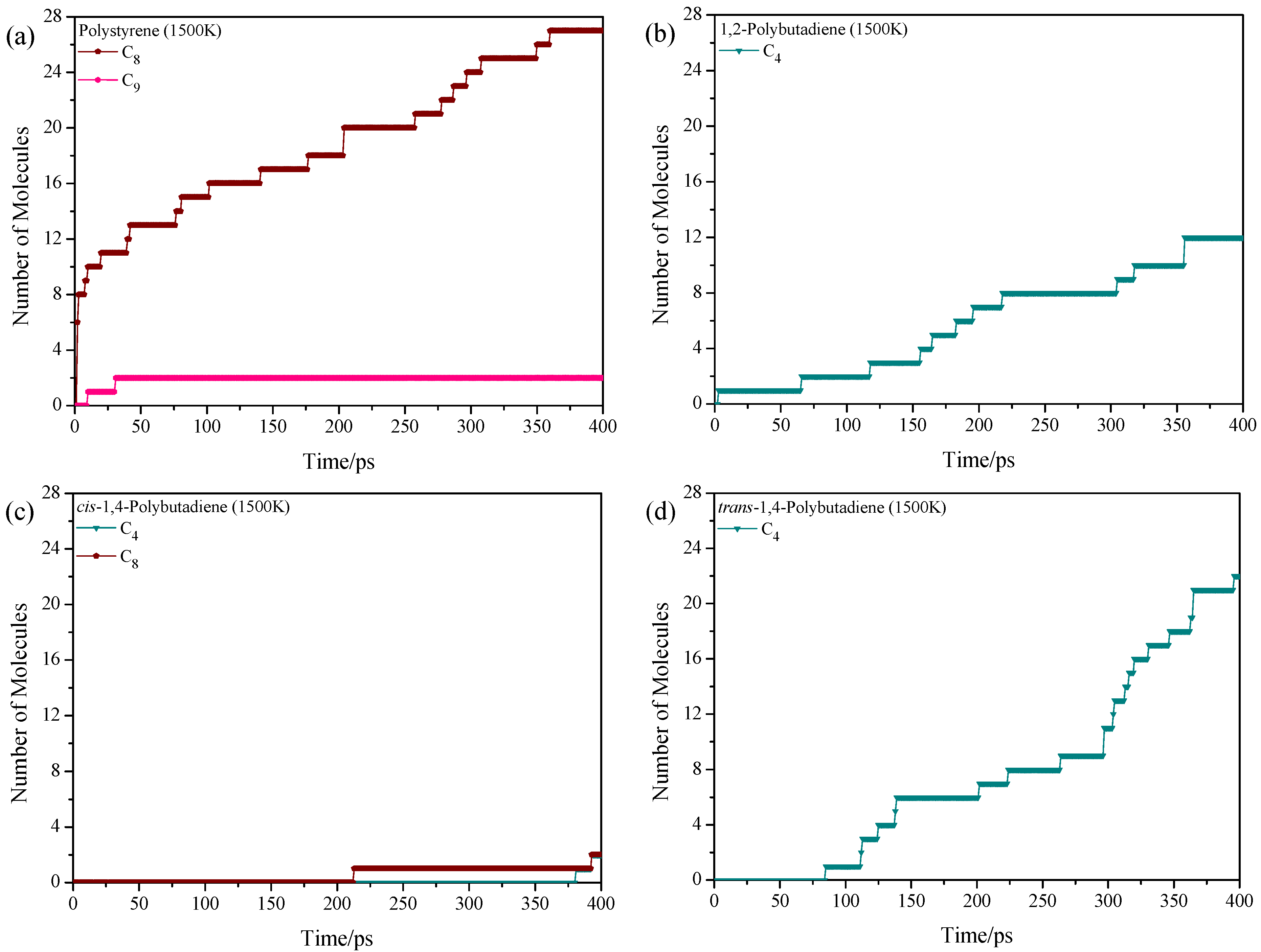
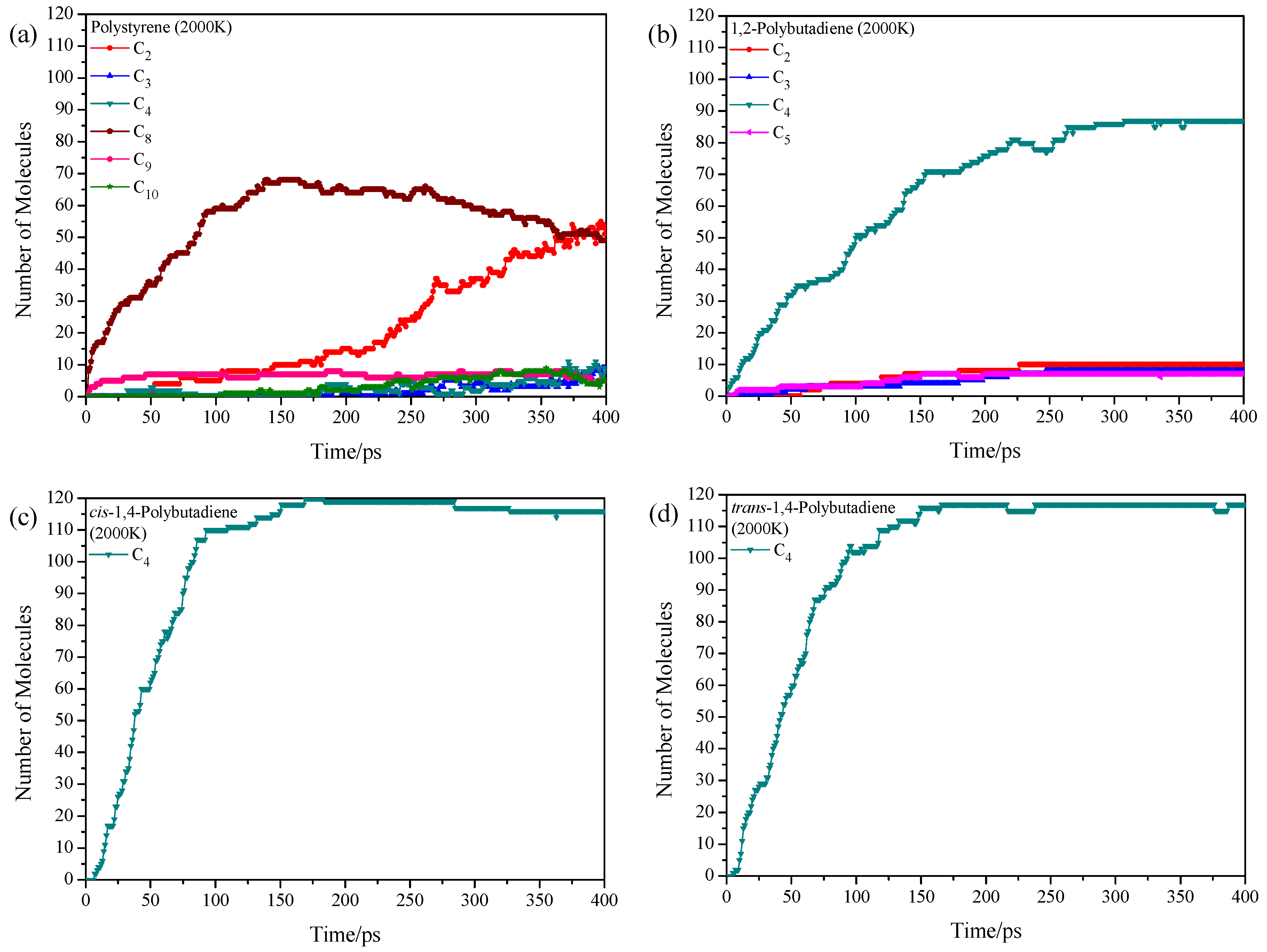
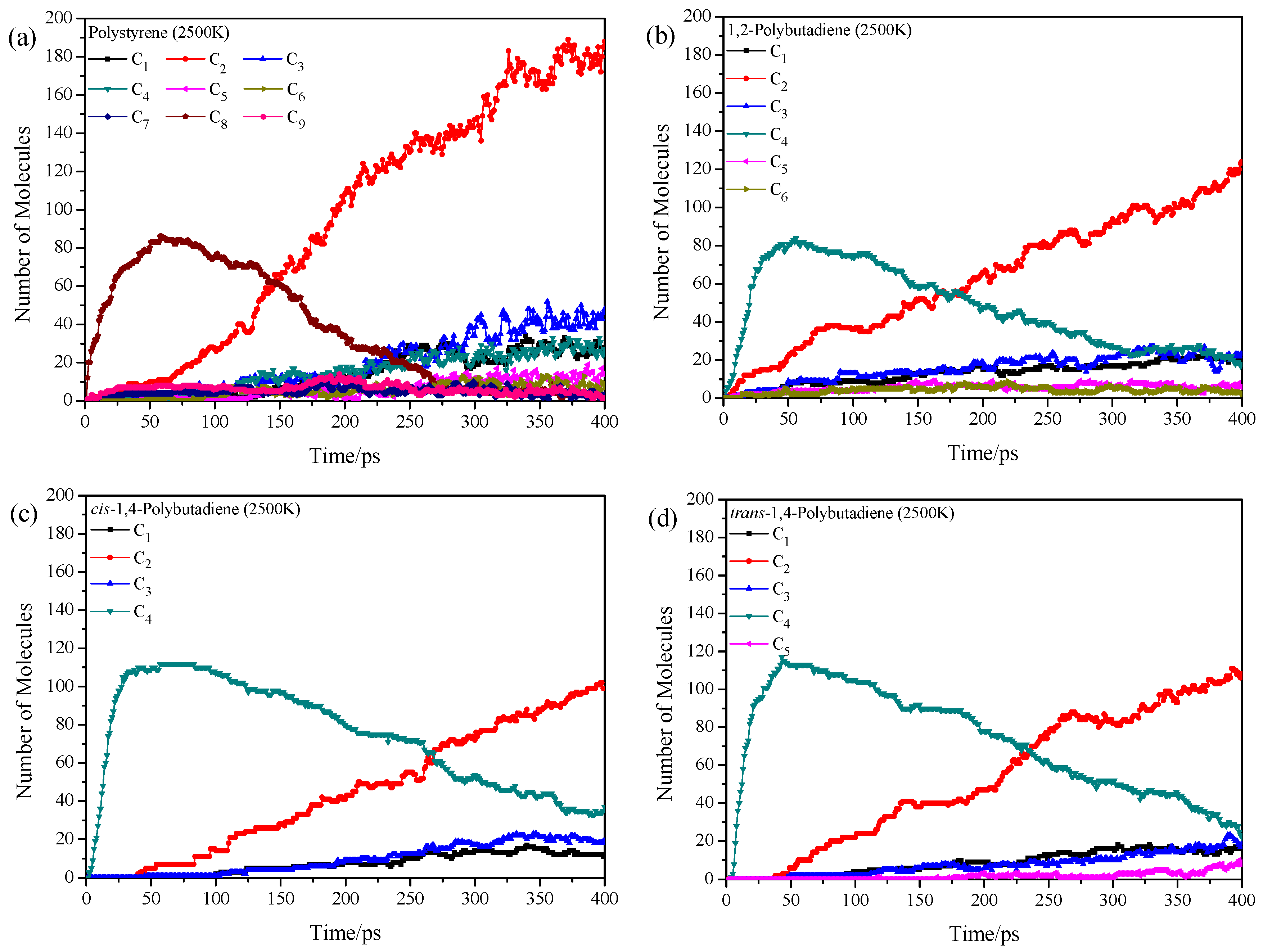
3.2. Pyrolysis Product Distributions of Homopolymers via ReaxFF MD
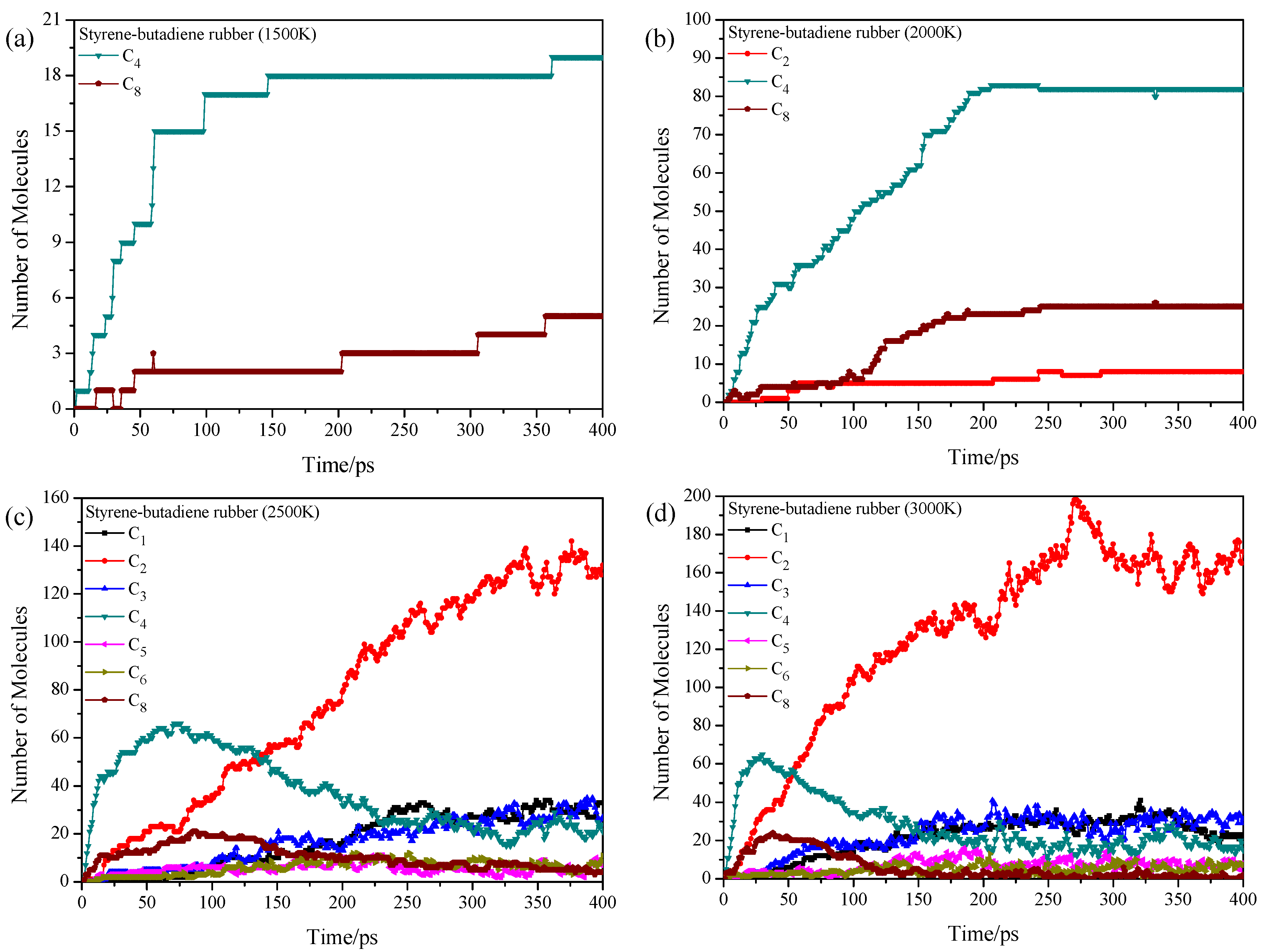
3.3. Pyrolysis Product Distributions of Styrene-Butadiene Rubber via ReaxFF MD
3.4. Effect of Initial Density on Pyrolysis Product Distributions
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Lah, B.; Klinar, D.; Likozar, B. Pyrolysis of natural, butadiene, styrene-butadiene rubber and tyre components: Modelling kinetics and transport phenomena at different heating rates and formulations. Chem. Eng. Sci. 2013, 87, 1–13. [Google Scholar] [CrossRef]
- Rhyner, C.R.; Schwartz, L.J.; Wenger, R.B.; Kohrell, M.G. Waste Management and Resource Recovery; CRC Press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Fang, Y.; Zhan, M.; Wang, Y. The status of recycling of waste rubber. Mater. Des. 2001, 22, 123–128. [Google Scholar] [CrossRef]
- Quek, A.; Balasubramanian, R. Liquefaction of waste tires by pyrolysis for oil and chemicals—A review. J. Anal. Appl. Pyrolysis 2013, 101, 1–16. [Google Scholar] [CrossRef]
- Williams, P.T. Pyrolysis of waste tyres: A review. Waste Manag. 2013, 33, 1714–1728. [Google Scholar] [CrossRef] [PubMed]
- Martínez, J.D.; Puy, N.; Murillo, R.; García, T.; Navarro, M.V.; Mastral, A.M. Waste tyre pyrolysis—A review. Renew. Sustain. Energy Rev. 2013, 23, 179–213. [Google Scholar] [CrossRef]
- Mui, E.L.; Ko, D.C.; McKay, G. Production of active carbons from waste tyres—A review. Carbon 2004, 42, 2789–2805. [Google Scholar] [CrossRef]
- Cypres, R.; Bettens, B. Pyrolysis and Gasification; Wiley Online Library: Hoboken, NJ, USA, 1989; pp. 209–229. [Google Scholar]
- Murillo, R.; Aylón, E.; Navarro, M.; Callén, M.; Aranda, A.; Mastral, A. The application of thermal processes to valorise waste tyre. Fuel Process. Technol. 2006, 87, 143–147. [Google Scholar] [CrossRef]
- Lopez, G.; Olazar, M.; Aguado, R.; Elordi, G.; Amutio, M.; Artetxe, M.; Bilbao, J. Vacuum pyrolysis of waste tires by continuously feeding into a conical spouted bed reactor. Ind. Eng. Chem. Res. 2010, 49, 8990–8997. [Google Scholar] [CrossRef]
- Barbooti, M.M.; Mohamed, T.J.; Hussain, A.A.; Abas, F.O. Optimization of pyrolysis conditions of scrap tires under inert gas atmosphere. J. Anal. Appl. Pyrolysis 2004, 72, 165–170. [Google Scholar] [CrossRef]
- Senneca, O.; Salatino, P.; Chirone, R. A fast heating-rate thermogravimetric study of the pyrolysis of scrap tyres. Fuel 1999, 78, 1575–1581. [Google Scholar] [CrossRef]
- Mastral, A.; Murillo, R.; Callén, M.; Garcıa, T. Application of coal conversion technology to tire processing. Fuel Process. Technol. 1999, 60, 231–242. [Google Scholar] [CrossRef]
- Leung, D.; Wang, C. Kinetic study of scrap tyre pyrolysis and combustion. J. Anal. Appl. Pyrolysis 1998, 45, 153–169. [Google Scholar] [CrossRef]
- Conesa, J.A.; Font, R.; Marcilla, A. Gas from the pyrolysis of scrap tires in a fluidized bed reactor. Energy Fuels 1996, 10, 134–140. [Google Scholar] [CrossRef]
- Ohno, K.; Esfarjani, K.; Kawazoe, Y. Computational Materials Science: From ab initio to Monte Carlo Methods; Springer: Heidelberg, Germany, 2018. [Google Scholar]
- Zheng, M.; Li, X.; Liu, J.; Guo, L. Initial chemical reaction simulation of coal pyrolysis via ReaxFF molecular dynamics. Energy Fuels 2013, 27, 2942–2951. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, C.; Chen, X. Unveiling the initial pyrolytic mechanisms of cellulose by DFT study. J. Anal. Appl. Pyrolysis 2015, 113, 621–629. [Google Scholar] [CrossRef]
- Qi, T.; Bauschlicher, C.W., Jr.; Lawson, J.W.; Desai, T.G.; Reed, E.J. Comparison of ReaxFF, DFTB, and DFT for phenolic pyrolysis. 1. Molecular dynamics simulations. J. Phys. Chem. A 2013, 117, 11115–11125. [Google Scholar] [CrossRef]
- Strachan, A.; Kober, E.M.; Van Duin, A.C.; Oxgaard, J.; Goddard, W.A., III. Thermal decomposition of RDX from reactive molecular dynamics. J. Chem. Phys. 2005, 122, 054502. [Google Scholar] [CrossRef]
- Zhong, Y.; Jing, X.; Wang, S.; Jia, Q.-X. Behavior investigation of phenolic hydroxyl groups during the pyrolysis of cured phenolic resin via molecular dynamics simulation. Polym. Degrad. Stab. 2016, 125, 97–104. [Google Scholar] [CrossRef]
- Lu, X.; Wang, X.; Li, Q.; Huang, X.; Han, S.; Wang, G. A ReaxFF-based molecular dynamics study of the pyrolysis mechanism of polyimide. Polym. Degrad. Stab. 2015, 114, 72–80. [Google Scholar] [CrossRef]
- Zheng, M.; Wang, Z.; Li, X.; Qiao, X.; Song, W.; Guo, L. Initial reaction mechanisms of cellulose pyrolysis revealed by ReaxFF molecular dynamics. Fuel 2016, 177, 130–141. [Google Scholar] [CrossRef]
- Chenoweth, K.; Cheung, S.; Van Duin, A.C.; Goddard, W.A.; Kober, E.M. Simulations on the thermal decomposition of a poly (dimethylsiloxane) polymer using the ReaxFF reactive force field. J. Am. Chem. Soc. 2005, 127, 7192–7202. [Google Scholar] [CrossRef] [PubMed]
- Zhang, J.; Weng, X.; Han, Y.; Li, W.; Cheng, J.; Gan, Z.; Gu, J. The effect of supercritical water on coal pyrolysis and hydrogen production: A combined ReaxFF and DFT study. Fuel 2013, 108, 682–690. [Google Scholar] [CrossRef]
- Kan, T.; Strezov, V.; Evans, T. Fuel production from pyrolysis of natural and synthetic rubbers. Fuel 2017, 191, 403–410. [Google Scholar] [CrossRef]
- Frisch, M.J.; Trucks, G.W.; Schlegel, H.B.; Scuseria, G.E.; Robb, M.A.; Cheeseman, J.R.; Scalmani, G.; Barone, V.; Mennucci, B.; Petersson, G.A.; et al. Gaussian 09, Revision D.01; Gaussian, Inc.: Wallingford, CT, USA, 2009. [Google Scholar]
- Lee, C.; Yang, W.; Parr, R.G. Development of the Colle-Salvetti correlation-energy formula into a functional of the electron density. Phys. Rev. B 1988, 37, 785–789. [Google Scholar] [CrossRef] [PubMed]
- Becke, A.D. Density-functional thermochemistry. III. The role of exact exchange. J. Chem. Phys. 1993, 98, 5648–5652. [Google Scholar] [CrossRef]
- Liu, L.; Bai, C.; Sun, H.; Goddard, W.A., III. Mechanism and kinetics for the initial steps of pyrolysis and combustion of 1,6-dicyclopropane-2,4-hexyne from ReaxFF reactive dynamics. J. Phys. Chem. A 2011, 115, 4941–4950. [Google Scholar] [CrossRef]
- Van Duin, A.C.; Dasgupta, S.; Lorant, F.; Goddard, W.A. ReaxFF: A reactive force field for hydrocarbons. J. Phys. Chem. A 2001, 105, 9396–9409. [Google Scholar] [CrossRef]
- Senftle, T.P.; Hong, S.; Islam, M.M.; Kylasa, S.B.; Zheng, Y.; Shin, Y.K.; Junkermeier, C.; Engel-Herbert, R.; Janik, M.J.; Aktulga, H.M. The ReaxFF reactive force-field: Development, applications and future directions. NPJ Comput. Mater. 2016, 2, 15011. [Google Scholar] [CrossRef]
- Chenoweth, K.; Van Duin, A.C.; Goddard, W.A. ReaxFF reactive force field for molecular dynamics simulations of hydrocarbon oxidation. J. Phys. Chem. A 2008, 112, 1040–1053. [Google Scholar] [CrossRef]
- Ding, J.; Zhang, L.; Zhang, Y.; Han, K.-L. A reactive molecular dynamics study of n-heptane pyrolysis at high temperature. J. Phys. Chem. A 2013, 117, 3266–3278. [Google Scholar] [CrossRef]
- Chenoweth, K.; Van Duin, A.C.; Dasgupta, S.; Goddard, W.A., III. Initiation mechanisms and kinetics of pyrolysis and combustion of JP-10 hydrocarbon jet fuel. J. Phys. Chem. A 2009, 113, 1740–1746. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Bhoi, S.; Banerjee, T.; Mohanty, K. Insights on the combustion and pyrolysis behavior of three different ranks of coals using reactive molecular dynamics simulation. RSC Adv. 2016, 6, 2559–2570. [Google Scholar] [CrossRef]
- FrantzDale, B.; Plimpton, S.J.; Shephard, M.S. Software components for parallel multiscale simulation: An example with LAMMPS. Eng. Comput. 2010, 26, 205–211. [Google Scholar] [CrossRef]
- Song, K.-S.; Liu, L.; Guo, Q.-X. Effects of geminal disubstitution on C–H and N–H bond dissociation energies. Tetrahedron 2004, 60, 9909–9923. [Google Scholar] [CrossRef]
- Mulliken, R.S.; Rieke, C.A.; Brown, W.G. Hyperconjugation. J. Am. Chem. Soc. 1941, 63, 41–56. [Google Scholar] [CrossRef]
- Berliner, E.; Bondhus, F.J. Hyperconjugation. II. The Competitive Bromination of Benzene and t-Butylbenzene. J. Am. Chem. Soc. 1948, 70, 854–858. [Google Scholar] [CrossRef]
- Ashraf, C.; Shabnam, S.; Jain, A.; Xuan, Y.; van Duin, A.C. Pyrolysis of binary fuel mixtures at supercritical conditions: A ReaxFF molecular dynamics study. Fuel 2019, 235, 194–207. [Google Scholar] [CrossRef]
- Joshi, K.L.; Raman, S.; Van Duin, A.C. Connectivity-based parallel replica dynamics for chemically reactive systems: From femtoseconds to microseconds. J. Phys. Chem. Lett. 2013, 4, 3792–3797. [Google Scholar] [CrossRef]
- Miron, R.A.; Fichthorn, K.A. Accelerated molecular dynamics with the bond-boost method. J. Chem. Phys. 2003, 119, 6210–6216. [Google Scholar] [CrossRef] [Green Version]
- Song, P.; Wu, X.; Wang, S. Effect of styrene butadiene rubber on the light pyrolysis of the natural rubber. Polym. Degrad. Stab. 2018, 147, 168–176. [Google Scholar] [CrossRef]
- Choi, S.-S. Characteristics of pyrolysis patterns of polybutadienes with different microstructures. J. Anal. Appl. Pyrolysis 2001, 57, 249–259. [Google Scholar] [CrossRef]
- Zhou, J.; Qiao, Y.; Wang, W.; Leng, E.; Huang, J.; Yu, Y.; Xu, M. Formation of styrene monomer, dimer and trimer in the primary volatiles produced from polystyrene pyrolysis in a wire-mesh reactor. Fuel 2016, 182, 333–339. [Google Scholar] [CrossRef]
- Grieco, E.; Bernardi, M.; Baldi, G. Styrene-butadiene rubber pyrolysis: Products, kinetics, modelling. J. Anal. Appl. Pyrolysis 2008, 82, 304–311. [Google Scholar] [CrossRef]
- López, G.; Olazar, M.; Aguado, R.; Bilbao, J. Continuous pyrolysis of waste tyres in a conical spouted bed reactor. Fuel 2010, 89, 1946–1952. [Google Scholar] [CrossRef]
- Buekens, A. Introduction to Feedstock Recycling of Plastics; Wiley Online Library: Hoboken, NJ, USA, 2006; Volume 6, pp. 1–41. [Google Scholar]



| Trimer | C1–C2 | C2–C3 | C3–C4 | C4–C5 | C5–C6 | C6–C7 | C7–C8 | C8–C9 | C9–C10 | C10–C11 | C11–C12 |
|---|---|---|---|---|---|---|---|---|---|---|---|
| CCC | 429.4 | 711.8 | 420.7 | 221.4 | 420.5 | 712.7 | 420.5 | 220.6 | 420.7 | 711.8 | 429.4 |
| CDC | 428.6 | 715.6 | 416.6 | 233.1 | 421.2 | 719.9 | 422.4 | 236.7 | 416.7 | 716.4 | 428.6 |
| DCD | 434.1 | 718.9 | 424.4 | 232.5 | 417.1 | 715.5 | 415.9 | 235.3 | 424.6 | 721.0 | 435.7 |
| DDD | 435.9 | 721.3 | 426.2 | 235.4 | 421.0 | 719.6 | 423.2 | 236.1 | 421.5 | 719.9 | 434.0 |
| CAC | 427.5 | 714.0 | 409.6 | 229.3 | 300.0 | 292.1 | 413.0 | 716.3 | 428.1 | ||
| CBC | 417.2 | 703.2 | 402.8 | 210.6 | 282.5 | 289.5 | 403.3 | 703.3 | 416.9 | ||
| DAD | 435.8 | 720.2 | 417.3 | 231.7 | 299.5 | 294.2 | 420.3 | 720.8 | 435.6 | ||
| DBD | 435.9 | 718.7 | 422.4 | 220.3 | 287.8 | 302.3 | 428.5 | 720.4 | 439.5 | ||
| ACA | 316.8 | 300.1 | 413.8 | 713.9 | 412.9 | 235.9 | 319.1 | ||||
| ADA | 316.0 | 302.6 | 422.1 | 720.6 | 420.9 | 238.7 | 320.2 | ||||
| BCB | 305.7 | 303.0 | 413.2 | 713.6 | 414.9 | 223.5 | 306.1 | ||||
| BDB | 305.4 | 303.3 | 425.5 | 718.9 | 419.4 | 223.5 | 303.6 | ||||
| AAA | 317.0 | 307.0 | 301.9 | 300.5 | 316.0 | ||||||
| ABA | 311.3 | 278.3 | 276.1 | 290.3 | 307.0 | ||||||
| BAB | 290.0 | 283.0 | 281.4 | 277.7 | 296.3 | ||||||
| BBB | 292.2 | 268.9 | 269.6 | 278.5 | 294.0 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, S.; Zhuo, H.; Wang, Y.; Leng, S.; Zhuang, G.; Zhong, X.; Wei, Z.; Yao, Z.; Wang, J.-g. Multiscale Simulation on Product Distribution from Pyrolysis of Styrene-Butadiene Rubber. Polymers 2019, 11, 1967. https://doi.org/10.3390/polym11121967
Deng S, Zhuo H, Wang Y, Leng S, Zhuang G, Zhong X, Wei Z, Yao Z, Wang J-g. Multiscale Simulation on Product Distribution from Pyrolysis of Styrene-Butadiene Rubber. Polymers. 2019; 11(12):1967. https://doi.org/10.3390/polym11121967
Chicago/Turabian StyleDeng, Shengwei, Han Zhuo, Yinbin Wang, Shuai Leng, Guilin Zhuang, Xing Zhong, Zhongzhe Wei, Zihao Yao, and Jian-guo Wang. 2019. "Multiscale Simulation on Product Distribution from Pyrolysis of Styrene-Butadiene Rubber" Polymers 11, no. 12: 1967. https://doi.org/10.3390/polym11121967
APA StyleDeng, S., Zhuo, H., Wang, Y., Leng, S., Zhuang, G., Zhong, X., Wei, Z., Yao, Z., & Wang, J.-g. (2019). Multiscale Simulation on Product Distribution from Pyrolysis of Styrene-Butadiene Rubber. Polymers, 11(12), 1967. https://doi.org/10.3390/polym11121967






