Detector Response to D-D Neutrons and Stability Measurements with 4H Silicon Carbide Detectors
Abstract
:1. Introduction
2. Detectors and Front-End Electronics
3. Irradiation Facilities
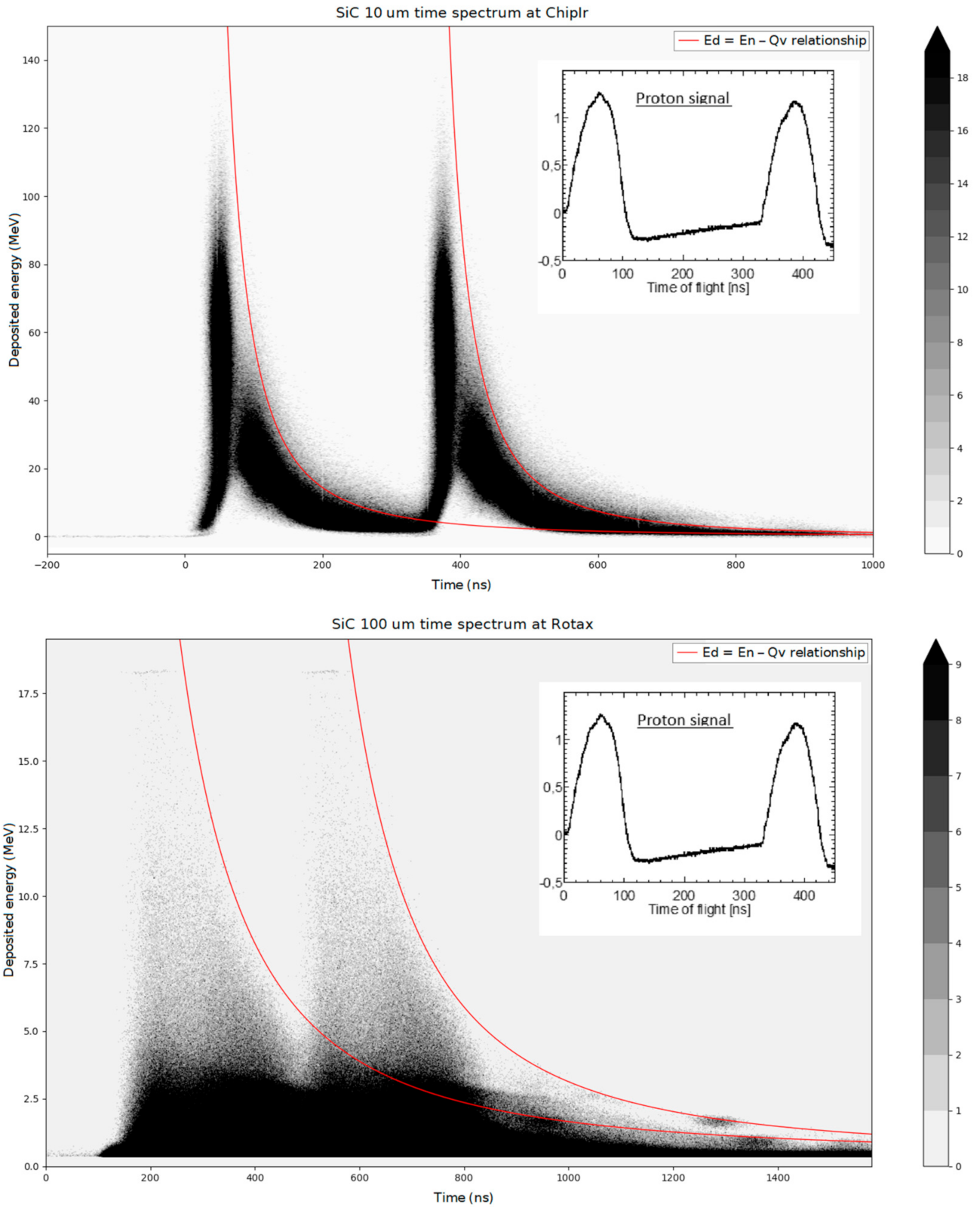
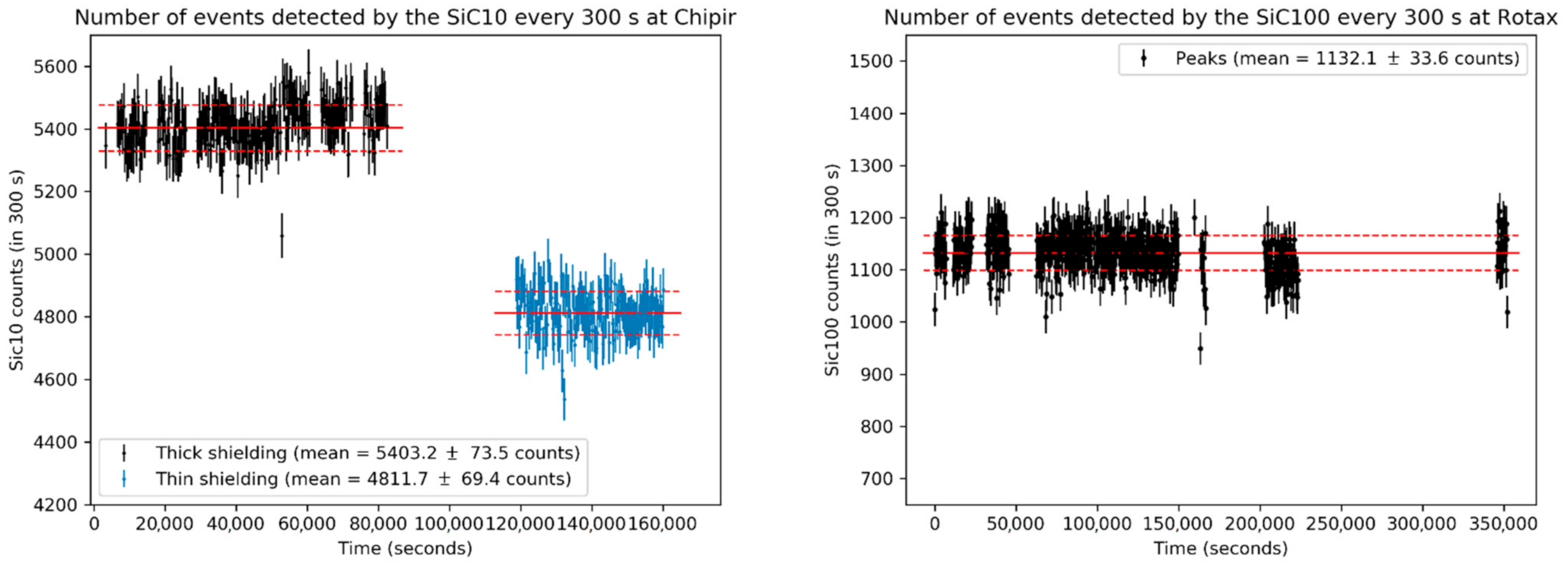
3.1. ISIS (ChipIr and ROTAX)
3.2. FNG
3.3. Alpha Irradiation
4. Stability Measurements
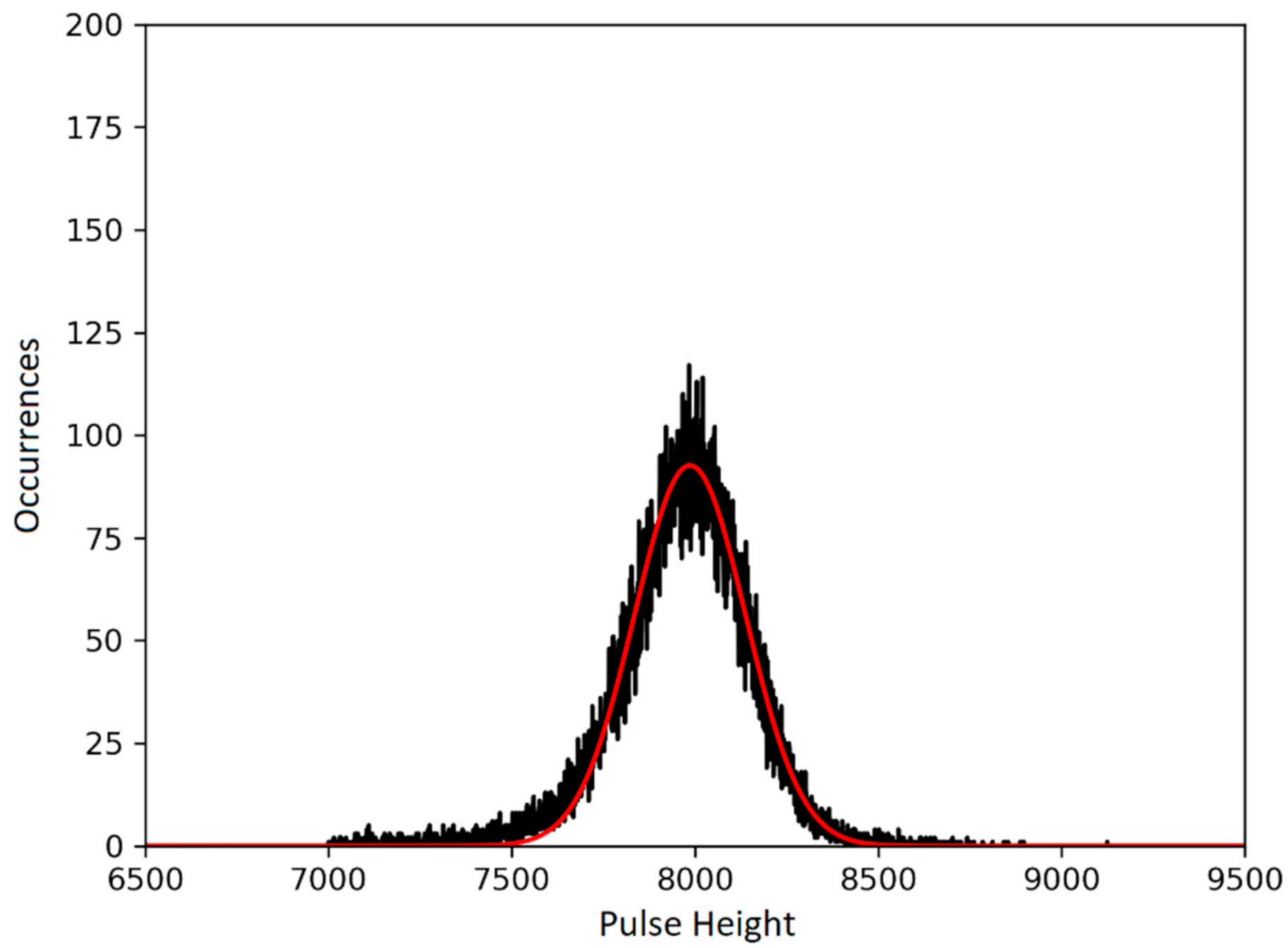
4.1. Results for Stability with Alpha Particles
4.2. Results for Stability with Neutrons
5. Neutron Spectroscopy (3.0 MeV)
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ericsson, G. Advanced Neutron Spectroscopy in Fusion Research. J. Fusion Energy 2019, 38, 330–355. [Google Scholar] [CrossRef] [Green Version]
- Rigamonti, D.; Giacomelli, L.; Gorini, G.; Nocente, M.; Rebai, M.; Tardocchi, M.; Angelone, M.; Batistoni, P.; Cufar, A.; Ghani, Z.; et al. Neutron spectroscopy measurements of 14 MeV neutrons at unprecedented energy resolution and implications for deuterium–tritium fusion plasma diagnostics. Meas. Sci. Technol. 2018, 29, 045502. [Google Scholar] [CrossRef] [Green Version]
- JET: EUROfusion’s Flagship Device. Available online: https://www.euro-fusion.org/devices/jet/ (accessed on 20 December 2020).
- Giacomelli, L.; Nocente, M.; Rebai, M.; Rigamonti, D.; Milocco, A.; Tardocchi, M.; Chen, Z.J.; Du, T.F.; Fan, T.S.; Hu, Z.M.; et al. Neutron emission spectroscopy of D-T plasmas at enhanced energy resolution with diamond detectors. Rev. Sci. Instrum. 2016, 87, 11D822. [Google Scholar] [CrossRef] [PubMed]
- Thomason, J.W.G. The ISIS spallation neutron and muon source—the first thirty-three years. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2019, 917, 61–67. [Google Scholar] [CrossRef]
- Giacomelli, L.; Rebai, M.; Fazzi, A.; Cippo, E.P.; Tardocchi, M.; Frost, C.D.; Pietropaolo, A.; Rhodes, N.; Schooneveld, E.; Gorini, G. Pulsed neutron beam measurements with diamond detectors. Nucl. Instrum. Methods Phys. Res. Sect. A 2013, 720, 125–127. [Google Scholar] [CrossRef]
- Rebai, M.; Andreani, C.; Fazzi, A.; Frost, C.D.; Giacomelli, L.; Gorini, G.; Milani, E.; Cippo, E.P.; Pietropaolo, A.; Prestopino, G.; et al. Fission diamond detector tests at the ISIS spallation neutron source. Nucl. Phys. B 2011, 215, 313–315. [Google Scholar] [CrossRef] [Green Version]
- ITER. Available online: www.iter.org (accessed on 20 December 2020).
- Franceschini, F.; Ruddy, F.H. Silicon Carbide Neutron Detectors. Prop. Appl. Silicon Carbide 2018. [Google Scholar] [CrossRef] [Green Version]
- Szalkai, D.; Ferone, R.; Issa, F.; Klix, A.; Lazar, M.; Lyoussi, A.; Ottaviani, L.; Tutto, P.; Vervisch, V. Fast Neutron Detection with 4H-SiC Based Diode Detector up to 500 °C Ambient Temperature. IEEE Trans. Nucl. Sci. 2016, 63, 1491–1498. [Google Scholar] [CrossRef]
- Ruddy, F.H.; Siedel, J.G. Effects of gamma irradiation on silicon carbide semiconductor radiation detectors. IEEE Nucl. Sci. Symp. Conf. Rec. 2006, 1, 583–587. [Google Scholar] [CrossRef]
- Moscatelli, F.; Scorzoni, A.; Poggi, A.; Bruzzi, M.; Sciortino, S.; Lagomarsino, S.; Wagner, G.; Nipoti, R. Radiation hardness of minimum ionizing particle detectors based on SiC p+n junctions. IEEE Nucl. Sci. Symp. Conf. Rec. 2005, 1, 490–494. [Google Scholar] [CrossRef]
- Tudisco, S.; Altana, C.; Boscardin, M.; Calcagno, L.; Ciampi, C.; Cirrone, G.A.P.; Fazzi, A.; Giove, D.; Gorini, G.; Labate, L.; et al. Silicon carbide for future intense luminosity nuclear physics investigations. Nuovo Cimento Della Societa Italiana Di Fisica C 2019, 42, 1–4. [Google Scholar] [CrossRef]
- Rebai, M.; Rigamonti, D.; Cancelli, S.; Croci, G.; Gorini, G.; Cippo, E.P.; Putignano, O.; Tardocchi, M.; Altana, C.; Angelone, M.; et al. New thick silicon carbide detectors: Response to 14 MeV neutrons and comparison with single-crystal diamonds. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2019, 946, 162637. [Google Scholar] [CrossRef]
- Kushoro, M.H.; Rebai, M.; Dicorato, M.; Rigamonti, D.; Altana, C.; Cazzaniga, C.; Croci, G.; Gorini, G.; Lanzalone, G.; la Via, F.; et al. Silicon Carbide characterization at the n_TOF spallation source with quasi-monoenergetic fast neutrons. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2020, 983, 164578. [Google Scholar] [CrossRef]
- Sato, Y.; Shimaoka, T.; Kaneko, J.H.; Murakami, H.; Isobe, M.; Osakabe, M.; Tsubota, M.; Ochiai, K.; Chayahara, A.; Umezawa, H.; et al. Radiation hardness of a single crystal CVD diamond detector for MeV energy protons. Nucl. Instrum. Methods Phys. Res., Sect. A 2015, 784, 147–150. [Google Scholar] [CrossRef]
- Rebai, M.; Fazzi, A.; Cazzaniga, C.; Croci, G.; Tardocchi, M.; Cippo, E.P.; Frost, C.D.; Zaccagnino, D.; Varoli, V.; Gorini, G. Time-stability of a Single-crystal Diamond Detector for fast neutron beam diagnostic under alpha and neutron irradiation. Diam. Relat. Mater. 2016, 61, 1–6. [Google Scholar] [CrossRef] [Green Version]
- Ruddy, F.H.; Dulloo, A.R.; Seidel, J.G.; Das, M.K.; Ryu, S.H.; Agarwal, A.K. The fast neutron response of silicon carbide semiconductor radiation detectors. IEEE Nucl. Sci. Symp. Conf. Rec. 2004, 7, 4575–4579. [Google Scholar] [CrossRef]
- Tudisco, S.; La Via, F.; Agodi, C.; Altana, C.; Borghi, G.; Boscardin, M.; Bussolino, G.; Calcagno, L.; Camarda, M.; Cappuzzello, F.; et al. Sicilia—silicon carbide detectors for intense luminosity investigations and applications. Sensors 2018, 18, 2289. [Google Scholar] [CrossRef] [Green Version]
- Cividec. Available online: https://civideC.At/ (accessed on 20 December 2020).
- Pietropaolo, A.; Andreani, C.; Rebai, M.; Giacomelli, L.; Gorini, G.; Cippo, E.P.; Tardocchi, M.; Fazzi, A.; Rinati, G.V.; Verona, C.; et al. Single-crystal diamond detector for time-resolved measurements of a pulsed fast-neutron beam. Europhys 2010, 92, 68003. [Google Scholar] [CrossRef]
- Schäfer, W.; Jansen, E.; Skowronek, R.; Will, G.; Kockelmann, W.; Schmidt, W.; Tietze-Jaensch, H. Setup and use of the ROTAX instrument at ISIS as angle-dispersive neutron powder and texture diffractometer. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 1995, 364, 179–185. [Google Scholar] [CrossRef]
- Cazzaniga, C.; Frost, C.D. Progress of the Scientific Commissioning of a fast neutron beamline for Chip Irradiation. J. Phys. Conf. Ser. 2018, 1021, 012037. [Google Scholar] [CrossRef]
- Chiesa, D.; Nastasi, M.; Cazzaniga, C.; Rebai, M.; Arcidiacono, L.; Previtali, E.; Gorini, G.; Frost, C.D. Measurement of the neutron flux at spallation sources using multi-foil activation. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2018, 902, 14–24. [Google Scholar] [CrossRef] [Green Version]
- ASTAR-NIST Catalogue. Available online: https://physics.nist.gov/PhysRefData/Star/Text/ASTAR.html (accessed on 1 November 2020).
- Rebai, M.; Milocco, A.; Giacomelli, L.; Cippo, E.P.; Tardocchi, M.; Fazzi, A.; Pietropaolo, A.; Gorini, G. Response of a single-crystal diamond detector to fast neutrons. J. Instrum. 2013, 8. [Google Scholar] [CrossRef]
- Evaluated Nuclear Data File (ENDF). Available online: https://www-nds.iaea.org/exfor/endf.htm (accessed on 1 November 2020).
- Angelone, M.; Cardarelli, R.; Paolozzi, L.; Pillon, M. Development of a low-noise amplifier for neutron detection in harsh environment. Eur. Phys. J. Plus 2014, 129, 1–5. [Google Scholar] [CrossRef]
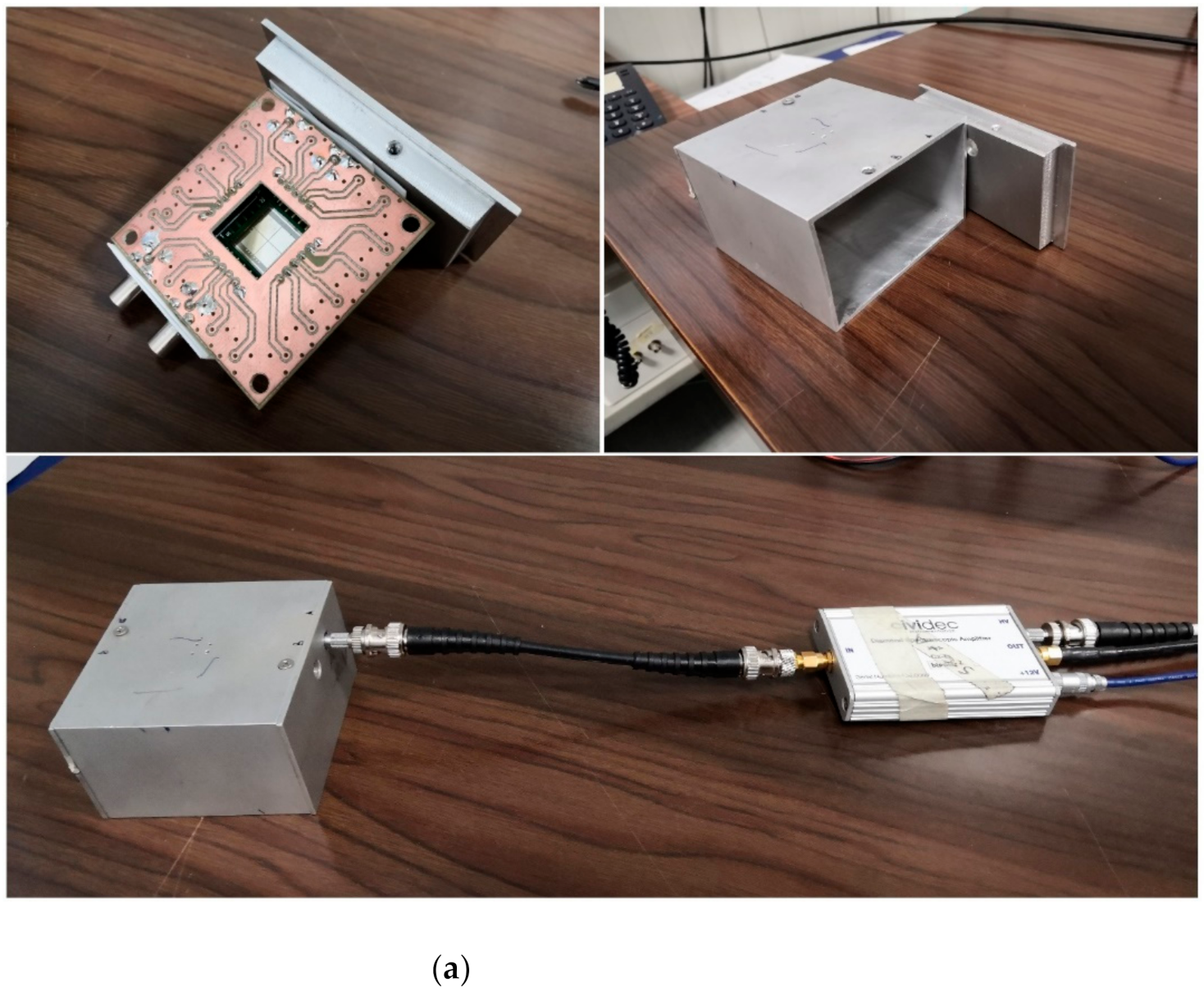
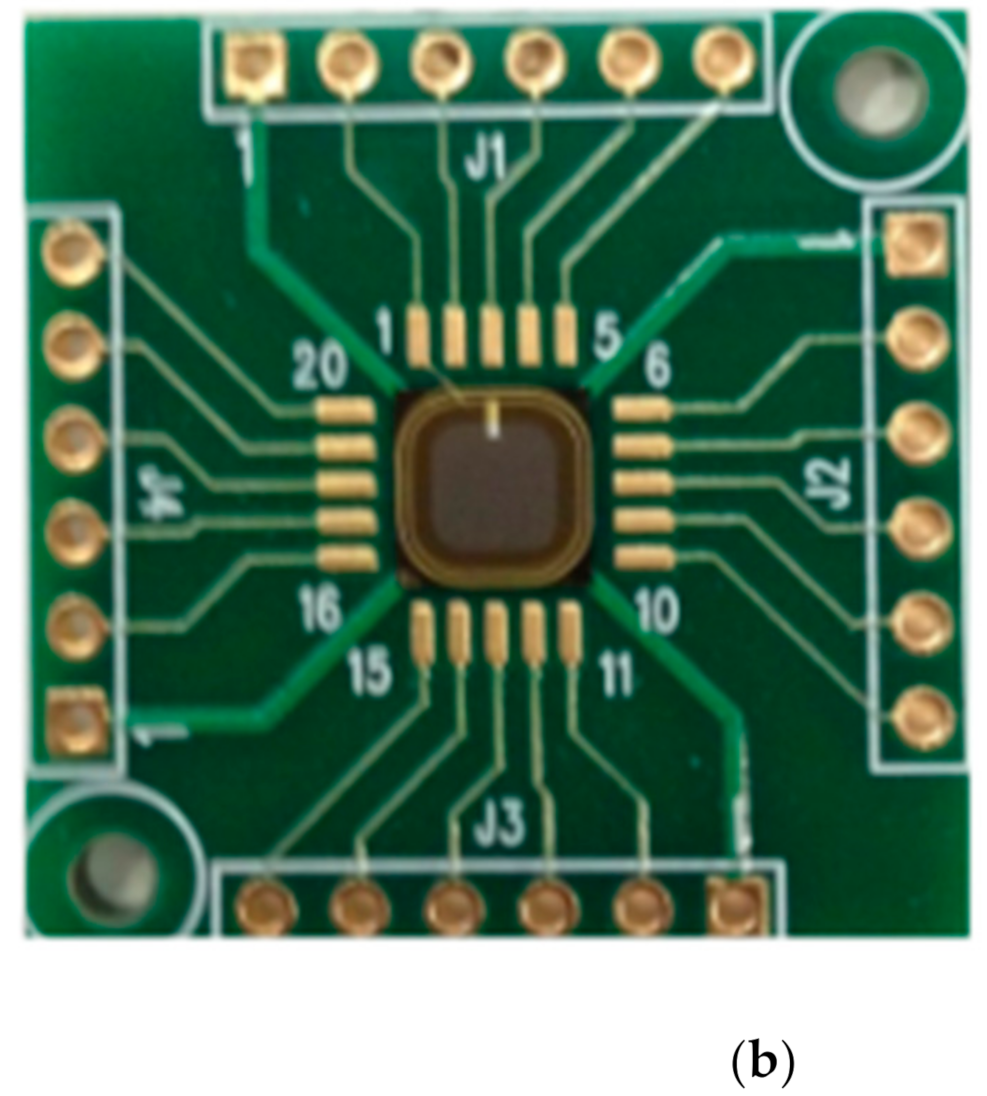



| Mean No. of Counts, | Dispersion (σ) | Poisson Uncertainty () | |
| SiC10 | 36,460.55 | 195.02 | 190.95 |
| SiC100 | 94,737.97 | 313.11 | 307.80 |
| Mean Pulse Height | Dispersion (σ) | Max PH—Min PH | |
| SiC10 | 7995.52 | 8.09 | - |
| SiC100 | 9672.03 | 27.60 | 9709 − 9625 = 84 (0.87%) |
| Mean Energy Resolution | Dispersion (σ) | ||
| SiC10 | 4.356% | 0.113% | - |
| SiC100 | 2.893% | 0.036% | - |
| 12C(n,n’)12C | 28Si(n,n’)28Si | |||
|---|---|---|---|---|
| Angle (deg) | Meas. (MeV) | Theor. (MeV) | Meas. (MeV) | Theor. (MeV) |
| 0 | 0.91 | 0.882 | 0.43 | 0.4095 |
| 30 | 0.9 | 0.854 | - | - |
| 50 | 0.86 | 0.826 | - | - |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kushoro, M.H.; Rebai, M.; Tardocchi, M.; Altana, C.; Cazzaniga, C.; De Marchi, E.; La Via, F.; Meda, L.; Meli, A.; Parisi, M.; et al. Detector Response to D-D Neutrons and Stability Measurements with 4H Silicon Carbide Detectors. Materials 2021, 14, 568. https://doi.org/10.3390/ma14030568
Kushoro MH, Rebai M, Tardocchi M, Altana C, Cazzaniga C, De Marchi E, La Via F, Meda L, Meli A, Parisi M, et al. Detector Response to D-D Neutrons and Stability Measurements with 4H Silicon Carbide Detectors. Materials. 2021; 14(3):568. https://doi.org/10.3390/ma14030568
Chicago/Turabian StyleKushoro, Matteo Hakeem, Marica Rebai, Marco Tardocchi, Carmen Altana, Carlo Cazzaniga, Eliana De Marchi, Francesco La Via, Laura Meda, Alessandro Meli, Miriam Parisi, and et al. 2021. "Detector Response to D-D Neutrons and Stability Measurements with 4H Silicon Carbide Detectors" Materials 14, no. 3: 568. https://doi.org/10.3390/ma14030568
APA StyleKushoro, M. H., Rebai, M., Tardocchi, M., Altana, C., Cazzaniga, C., De Marchi, E., La Via, F., Meda, L., Meli, A., Parisi, M., Perelli Cippo, E., Pillon, M., Trotta, A., Tudisco, S., & Gorini, G. (2021). Detector Response to D-D Neutrons and Stability Measurements with 4H Silicon Carbide Detectors. Materials, 14(3), 568. https://doi.org/10.3390/ma14030568









