Search for Articles:
Title / Keyword
Author / Affiliation / Email
Journal
Article Type
 
 
Advanced Search
 
Section
Special Issue
Volume
Issue
Number
Page
 
4.0
2.3
Journals
Mathematics
Sections
Topology and Foundations
share announcement

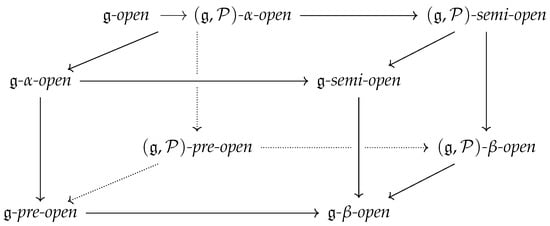
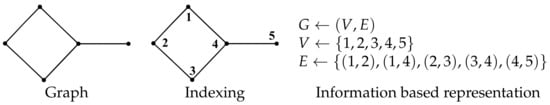
Topology and Foundations

A topical collection in Mathematics (ISSN 2227-7390). This collection belongs to the section "Algebra, Geometry and Topology".

Viewed by 9535

Editors

Prof. Dr. Lorentz Jäntschi

E-Mail Website
Collection Editor
Department of Physics and Chemistry, Technical University of Cluj-Napoca, 400641 Cluj-Napoca, Romania
Interests: applied mathematics; applied informatics
Special Issues, Collections and Topics in MDPI journals
Prof. Dr. Dušanka Janežič

E-Mail Website1 Website2
Collection Editor
Faculty of Mathematics, Natural Sciences and Information Technologies, University of Primorska, Glagoljaška 8, SI-6000 Koper, Slovenia
Interests: mathematics in natural science; computational
Special Issues, Collections and Topics in MDPI journals

Topical Collection Information

Dear Colleagues,

A foundational example in topology leading to graph theory as a new branch of mathematics is when Leonhard Euler demonstrated that it was impossible to find a route through the town of Königsberg (now Kaliningrad) that would cross each of its seven bridges exactly once (with the result depending only on connectivity properties—which bridges connect to which islands or riverbanks).

Subjects included in topology are graph theory and algebraic topology. Topology is foundational in number theory, algebraic geometry, category theory and homological algebra, K-theory, group theory and generalizations, topological groups and Lie groups, dynamical systems and ergodic theory, functional analysis, quantum theory, game theory, etc. Topology is in all fields of engineering, physical sciences, life sciences, social sciences, medicine and even arts, economics, finance, and finally mathematics-related sciences: informatics, physics, chemistry and biology.

Foundations extend beyond topology as the basis or groundwork of anything. Foundations are under algebraic geometry, tropical geometry, logic and deductive systems, functions, algebraic topology, homotopy theory, probability theory, stochastic processes, statistics, physics (fluid mechanics, optics, electromagnetic theory, thermodynamics, heat transfer, equilibrium and time-dependent statistical mechanics) and finally quantum information and its processing.

The aim of this Topical Collection is to bring together recent scientific advances, reviews, communications and short notes dealing with topology and foundations.

Prof. Dr. Lorentz Jäntschi
Prof. Dr. Dušanka Janežič
Collection Editors

Manuscript Submission Information

Manuscripts should be submitted online at www.mdpi.com by registering and logging in to this website. Once you are registered, click here to go to the submission form. Manuscripts can be submitted until the deadline. All submissions that pass pre-check are peer-reviewed. Accepted papers will be published continuously in the journal (as soon as accepted) and will be listed together on the collection website. Research articles, review articles as well as short communications are invited. For planned papers, a title and short abstract (about 100 words) can be sent to the Editorial Office for announcement on this website.

Submitted manuscripts should not have been published previously, nor be under consideration for publication elsewhere (except conference proceedings papers). All manuscripts are thoroughly refereed through a single-blind peer-review process. A guide for authors and other relevant information for submission of manuscripts is available on the Instructions for Authors page. Mathematics is an international peer-reviewed open access semimonthly journal published by MDPI.

Please visit the Instructions for Authors page before submitting a manuscript. The Article Processing Charge (APC) for publication in this open access journal is 2600 CHF (Swiss Francs). Submitted papers should be well formatted and use good English. Authors may use MDPI's English editing service prior to publication or during author revisions.

Published Papers (3 papers)

Download All Papers

2024

Jump to: 2023, 2021

14 pages, 314 KiB  
Article
Categories of Open Sets in Generalized Primal Topological Spaces
by Hanan Al-Saadi and Huda Al-Malki
Mathematics 2024, 12(2), 207; https://doi.org/10.3390/math12020207 - 8 Jan 2024
Cited by 7 | Viewed by 1488
Abstract
In this research article, we define some categories of open sets over a generalized topological space given together with a primal collection. In addition, we clarify some of its characteristics and investigate the relationships between these concepts in the space under consideration. The [...] Read more.
In this research article, we define some categories of open sets over a generalized topological space given together with a primal collection. In addition, we clarify some of its characteristics and investigate the relationships between these concepts in the space under consideration. The topic of continuity occupies a large space in topological theory and is one of the most important topics therein. Researchers have examined it in light of many variables. We followed the same approach by studying the concept of continuity between two generalized topological spaces in light of the primal collection under the name (g,P)-continuity. We also made a decomposition of this type of function in light of these weak categories of open sets. Full article
►▼ Show Figures

Figure 1

2023

Jump to: 2024, 2021

16 pages, 337 KiB  
Article
On Bishop–Phelps and Krein–Milman Properties
by Francisco Javier García-Pacheco
Mathematics 2023, 11(21), 4473; https://doi.org/10.3390/math11214473 - 28 Oct 2023
Viewed by 1021
Abstract
A real topological vector space is said to have the Krein–Milman property if every bounded, closed, convex subset has an extreme point. In the case of every bounded, closed, convex subset is the closed convex hull of its extreme points, then we say [...] Read more.
A real topological vector space is said to have the Krein–Milman property if every bounded, closed, convex subset has an extreme point. In the case of every bounded, closed, convex subset is the closed convex hull of its extreme points, then we say that the topological vector space satisfies the strong Krein–Milman property. The strong Krein–Milman property trivially implies the Krein–Milman property. We provide a sufficient condition for these two properties to be equivalent in the class of Hausdorff locally convex real topological vector spaces. This sufficient condition is the Bishop–Phelps property, which we introduce for real topological vector spaces by means of uniform convergence linear topologies. We study the inheritance of the Bishop–Phelps property. Nontrivial examples of topological vector spaces failing the Krein–Milman property are also given, providing us with necessary conditions to assure that the Krein–Milman property is satisfied. Finally, a sufficient condition to assure the Krein–Milman property is discussed. Full article

2021

Jump to: 2024, 2023

25 pages, 764 KiB  
Communication
Figures of Graph Partitioning by Counting, Sequence and Layer Matrices
by Mihaela Aurelia Tomescu, Lorentz Jäntschi and Doina Iulia Rotaru
Mathematics 2021, 9(12), 1419; https://doi.org/10.3390/math9121419 - 18 Jun 2021
Cited by 20 | Viewed by 3626
Abstract
A series of counting, sequence and layer matrices are considered precursors of classifiers capable of providing the partitions of the vertices of graphs. Classifiers are given to provide different degrees of distinctiveness for the vertices of the graphs. Any partition can be represented [...] Read more.
A series of counting, sequence and layer matrices are considered precursors of classifiers capable of providing the partitions of the vertices of graphs. Classifiers are given to provide different degrees of distinctiveness for the vertices of the graphs. Any partition can be represented with colors. Following this fundamental idea, it was proposed to color the graphs according to the partitions of the graph vertices. Two alternative cases were identified: when the order of the sets in the partition is relevant (the sets are distinguished by their positions) and when the order of the sets in the partition is not relevant (the sets are not distinguished by their positions). The two isomers of C28 fullerenes were colored to test the ability of classifiers to generate different partitions and colorings, thereby providing a useful visual tool for scientists working on the functionalization of various highly symmetrical chemical structures. Full article
►▼ Show Figures

Figure 1

Back to TopTop