Amplified Spontaneous Emission Threshold Dependence on Determination Method in Dye-Doped Polymer and Lead Halide Perovskite Waveguides
Abstract
:1. Introduction
2. Results
2.1. State of Art
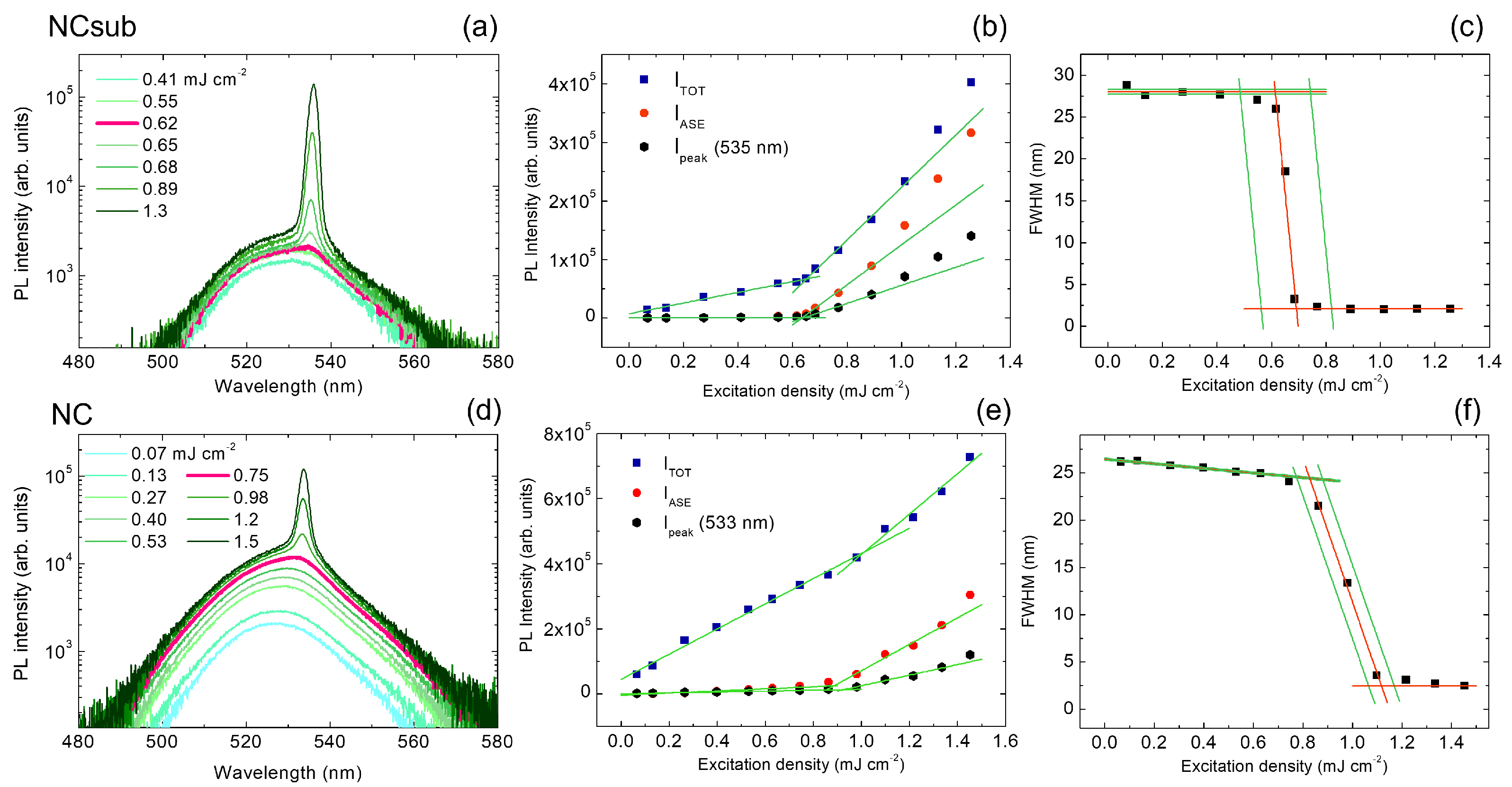
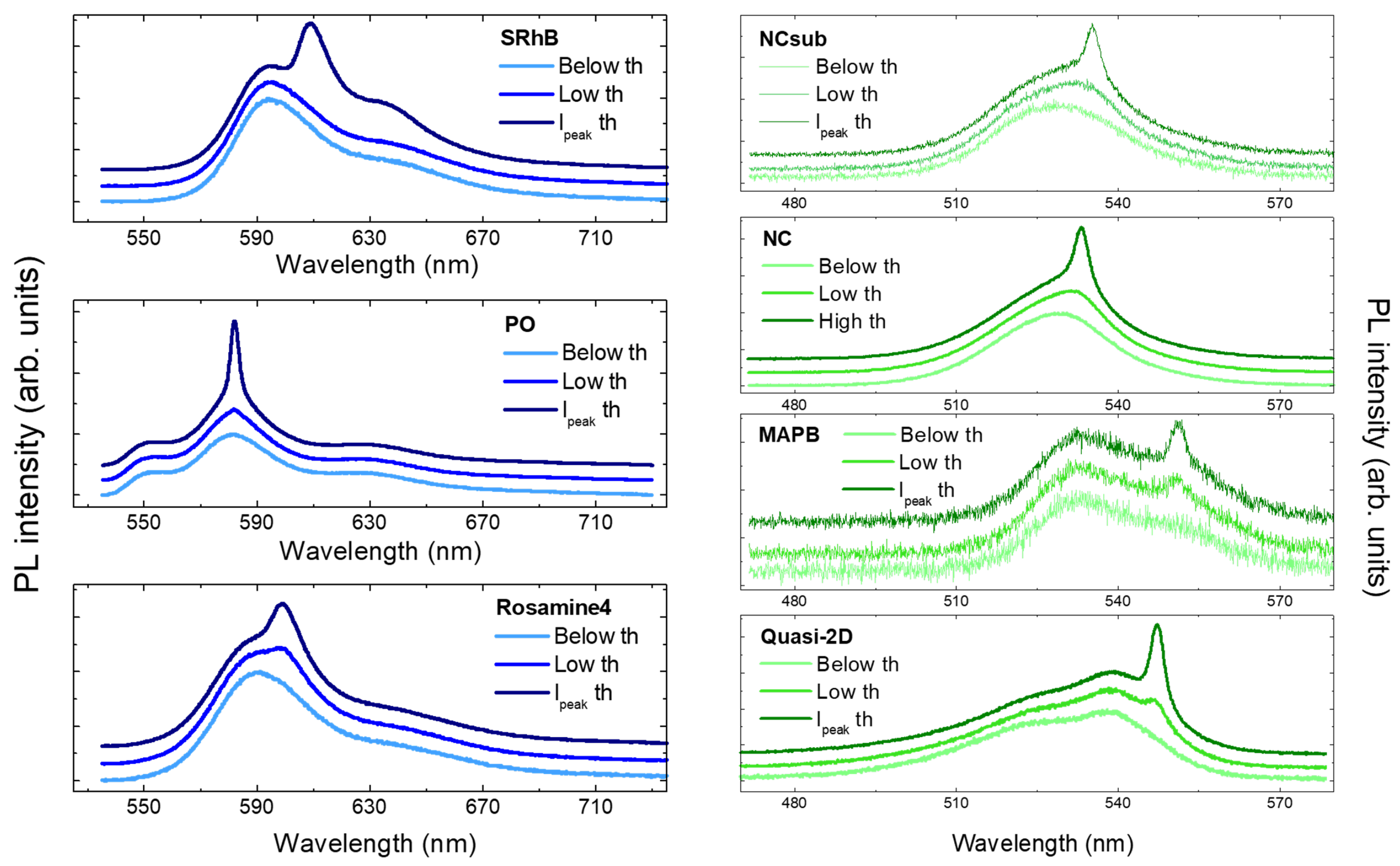
2.2. ASE in Lead Halide Perovskites
2.3. ASE in Dye-Polymer Blends
3. Discussion
4. Materials and Methods
4.1. Sample Preparation and Characterization
- VPI measurements for perovskite samples (NC, NCsub, MAPB, and Quasi-2D)
- VPI measurements for dye-polymer samples (SRhB, PO and rosamine4)
4.2. Methods Used to Extract the ASE Threshold
- 1.
- Visual method
- 2.
- Slope variation in the output intensity plot-based methods
- 3.
- FWHM-based methods
- method: starting from the first point that deviates for more than one standard deviation from the initial constant/linear best fit, the ASE threshold value is calculated as the average between the excitation density value of this point and the one immediately before; semidispersion is used as maximum error and converted to statistical error;
- method: the ASE threshold is given by the excitation density value of the crossing point between the initial constant/linear fit at low excitation densities and the following decreasing linear fit. The intersection of the error lines are used for the determination of the ASE threshold error;
- FWHM/2 method: the ASE threshold is given by the excitation density at which the FWHM halves with respect to the initial value obtained at low excitation density (line-width of the spontaneous emission spectrum). In the samples showing a FWHM variation before the ASE-induced line narrowing, we considered as reference the spectral linewidth of the PL spectrum at the lowest excitation density.
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References and Note
- Schäfer, F.P.; Schmidt, W.; Volze, J. Organic Dye Solution Laser. Appl. Phys. Lett. 1966, 9, 306–309. [Google Scholar] [CrossRef]
- Sorokin, P.P.; Lankard, J.R. Stimulated Emission Observed from an Organic Dye, Chloro-aluminum Phthalocyanine. IBM J. Res. Dev. 1966, 10, 162–163. [Google Scholar] [CrossRef]
- Anni, M.; Lattante, S. Organic Lasers: Fundamentals, Developments, and Applications; Jenny Stanford Publishing: Dubai, United Arab Emirates, 2018. [Google Scholar]
- Singh, S.; Kanetkar, V.; Sridhar, G.; Muthuswamy, V.; Raja, K. Solid-state polymeric dye lasers. J. Lumin. 2003, 101, 285–291. [Google Scholar] [CrossRef]
- Kuehne, A.J.C.; Gather, M.C. Organic Lasers: Recent Developments on Materials, Device Geometries, and Fabrication Techniques. Chem. Rev. 2016, 116, 12823–12864. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Q.; Tao, W.; Huang, J.; Xia, R.; Cabanillas-Gonzalez, J. Toward Electrically Pumped Organic Lasers: A Review and Outlook on Material Developments and Resonator Architectures. Adv. Photonics Res. 2021, 2, 2000155. [Google Scholar] [CrossRef]
- Jiang, Y.; Liu, Y.Y.; Liu, X.; Lin, H.; Gao, K.; Lai, W.Y.; Huang, W. Organic solid-state lasers: A materials view and future development. Chem. Soc. Rev. 2020, 49, 5885–5944. [Google Scholar] [CrossRef]
- Ruan, B.; Liu, H.L.; Xie, L.; Ding, H.; Zhang, Y.; Wu, J.; Huang, Z.; Shi, D.; Jiang, T.; Tsai, F.C. The Fluorescence Property of Zirconium-Based MOFs Adsorbed Sulforhodamine B. J. Fluoresc. 2020, 30, 427–435. [Google Scholar] [CrossRef]
- Vasileva, E.; Ye, F.; Marinins, A.; Etcheverry, S.; Toprak, M.S.; Popov, S. Optimization of optical gain in composite materials containing Rh6G dye and gold nanoparticles. In Proceedings of the Asia Communications and Photonics Conference, Hong Kong, China, 19–23 November 2015. [Google Scholar] [CrossRef]
- Gupta, M.; Mula, S.; Ghanty, T.K.; Naik, D.; Ray, A.K.; Sharma, A.; Chattopadhyay, S. Structure and solvent-induced tuning of laser property and photostability of a boradiazaindacene (BODIPY) dye. J. Photochem. Photobiol. A 2017, 349, 162–170. [Google Scholar] [CrossRef]
- Gaylord, B.S.; Wang, S.; Heeger, A.J.; Bazan, G.C. Water-Soluble Conjugated Oligomers: Effect of Chain Length and Aggregation on Photoluminescence-Quenching Efficiencies. J. Am. Chem. Soc. 2001, 123, 6417–6418. [Google Scholar] [CrossRef]
- Zhang, M.; Chen, J.; Wang, M.; Yuan, M.; Li, R.; Feng, X.; He, Y.; Mao, X.; Li, Y.; Xiong, Z.; et al. Pyrene-Based Nonwoven Fabric with Tunable Fluorescence Properties by Employing the Aggregation-Caused Quenching Effect. ACS Appl. Mater. Interfaces 2021, 13, 9036–9042. [Google Scholar] [CrossRef]
- Hu, R.; Qin, A.; Tang, B.Z. AIE polymers: Synthesis and applications. Prog. Polym. Sci. 2020, 100, 101176. [Google Scholar] [CrossRef]
- Kojima, A.; Teshima, K.; Shirai, Y.; Miyasaka, T. Organometal Halide Perovskites as Visible-Light Sensitizers for Photovoltaic Cells. J. Am. Chem. Soc. 2009, 131, 6050–6051. [Google Scholar] [CrossRef] [PubMed]
- Stranks, S.D.; Snaith, H.J. Metal-halide perovskites for photovoltaic and light-emitting devices. Nat. Nanotechnol. 2015, 10, 391–402. [Google Scholar] [CrossRef] [PubMed]
- Kovalenko, M.V.; Protesescu, L.; Bodnarchuk, M.I. Properties and potential optoelectronic applications of lead halide perovskite nanocrystals. Science 2017, 358, 745–750. [Google Scholar] [CrossRef] [Green Version]
- Kim, E.B.; Akhtar, M.S.; Shin, H.S.; Ameen, S.; Nazeeruddin, M.K. A review on two-dimensional (2D) and 2D-3D multidimensional perovskite solar cells: Perovskites structures, stability, and photovoltaic performances. J. Photochem. Photobiol. C 2021, 48, 100405. [Google Scholar] [CrossRef]
- Xing, G.; Mathews, N.; Lim, S.S.; Yantara, N.; Liu, X.; Sabba, D.; Grätzel, M.; Mhaisalkar, S.; Sum, T.C. Low-temperature solution-processed wavelength-tunable perovskites for lasing. Nat. Mater. 2014, 13, 476–480. [Google Scholar] [CrossRef]
- Chen, Q.; De Marco, N.; Yang, Y.M.; Song, T.B.; Chen, C.C.; Zhao, H.; Hong, Z.; Zhou, H.; Yang, Y. Under the spotlight: The organic–inorganic hybrid halide perovskite for optoelectronic applications. Nano Today 2015, 10, 355–396. [Google Scholar] [CrossRef] [Green Version]
- Fu, Y.; Zhu, H.; Chen, J.; Hautzinger, M.P.; Zhu, X.Y.; Jin, S. Metal halide perovskite nanostructures for optoelectronic applications and the study of physical properties. Nat. Rev. Mater. 2019, 4, 169–188. [Google Scholar] [CrossRef]
- Fan, Q.; Biesold-McGee, G.V.; Ma, J.; Xu, Q.; Pan, S.; Peng, J.; Lin, Z. Lead-Free Halide Perovskite Nanocrystals: Crystal Structures, Synthesis, Stabilities, and Optical Properties. Angew. Chem. Int. Ed. 2020, 59, 1030–1046. [Google Scholar] [CrossRef]
- Zhu, H.; Fu, Y.; Meng, F.; Wu, X.; Gong, Z.; Ding, Q.; Gustafsson, M.V.; Trinh, M.T.; Jin, S.; Zhu, X.Y. Lead halide perovskite nanowire lasers with low lasing thresholds and high quality factors. Nat. Mater. 2015, 14, 636–642. [Google Scholar] [CrossRef]
- Yakunin, S.; Protesescu, L.; Krieg, F.; Bodnarchuk, M.I.; Nedelcu, G.; Humer, M.; De Luca, G.; Fiebig, M.; Heiss, W.; Kovalenko, M.V. Erratum: Low-threshold amplified spontaneous emission and lasing from colloidal nanocrystals of caesium lead halide perovskites. Nat. Commun. 2015, 6, 8515. [Google Scholar] [CrossRef] [PubMed]
- Brenner, P.; Bar-On, O.; Jakoby, M.; Allegro, I.; Richards, B.S.; Paetzold, U.W.; Howard, I.A.; Scheuer, J.; Lemmer, U. Continuous wave amplified spontaneous emission in phase-stable lead halide perovskites. Nat. Commun. 2019, 10, 988. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Hu, Z.; Zhang, Z.; Du, J.; Yang, J.; Tang, X.; Liu, W.; Leng, Y. Two-Photon Pumped Amplified Spontaneous Emission and Lasing from Formamidinium Lead Bromine Nanocrystals. ACS Photonics 2019, 6, 3150–3158. [Google Scholar] [CrossRef]
- De Giorgi, M.L.; Cretì, A.; La-Placa, M.G.; Boix, P.P.; Bolink, H.J.; Lomascolo, M.; Anni, M. Amplified spontaneous emission in thin films of quasi-2D BA3MA3Pb5Br16 lead halide perovskites. Nanoscale 2021, 13, 8893–8900. [Google Scholar] [CrossRef]
- Athanasiou, M.; Papagiorgis, P.; Manoli, A.; Bernasconi, C.; Bodnarchuk, M.I.; Kovalenko, M.V.; Itskos, G. Efficient Amplified Spontaneous Emission from Solution-Processed CsPbBr3 Nanocrystal Microcavities under Continuous Wave Excitation. ACS Photonics 2021, 8, 2120–2129. [Google Scholar] [CrossRef]
- Deschler, F.; Price, M.; Pathak, S.; Klintberg, L.E.; Jarausch, D.D.; Higler, R.; Hüttner, S.; Leijtens, T.; Stranks, S.D.; Snaith, H.J.; et al. High Photoluminescence Efficiency and Optically Pumped Lasing in Solution-Processed Mixed Halide Perovskite Semiconductors. J. Phys. Chem. Lett. 2014, 5, 1421–1426. [Google Scholar] [CrossRef]
- Leyden, M.R.; Terakawa, S.; Matsushima, T.; Ruan, S.; Goushi, K.; Auffray, M.; Sandanayaka, A.S.D.; Qin, C.; Bencheikh, F.; Adachi, C. Distributed Feedback Lasers and Light-Emitting Diodes Using 1-Naphthylmethylamnonium Low-Dimensional Perovskite. ACS Photonics 2019, 6, 460–466. [Google Scholar] [CrossRef]
- Shang, Q.; Li, M.; Zhao, L.; Chen, D.; Zhang, S.; Chen, S.; Gao, P.; Shen, C.; Xing, J.; Xing, G.; et al. Role of the Exciton–Polariton in a Continuous-Wave Optically Pumped CsPbBr3 Perovskite Laser. Nano Lett. 2020, 20, 6636–6643. [Google Scholar] [CrossRef]
- Chen, S.; Nurmikko, A. Stable Green Perovskite Vertical-Cavity Surface-Emitting Lasers on Rigid and Flexible Substrates. ACS Photonics 2017, 4, 2486–2494. [Google Scholar] [CrossRef]
- Pourdavoud, N.; Mayer, A.; Buchmüller, M.; Brinkmann, K.; Häger, T.; Hu, T.; Heiderhoff, R.; Shutsko, I.; Görrn, P.; Chen, Y.; et al. Distributed Feedback Lasers Based on MAPbBr3. Adv. Mater. Technol. 2018, 3, 1700253. [Google Scholar] [CrossRef]
- Qin, C.; Sandanayaka, A.S.D.; Zhao, C.; Matsushima, T.; Zhang, D.; Fujihara, T.; Adachi, C. Stable room-temperature continuous-wave lasing in quasi-2D perovskite films. Nature 2020, 585, 53–57. [Google Scholar] [CrossRef] [PubMed]
- De Giorgi, M.L.; Anni, M. Amplified Spontaneous Emission and Lasing in Lead Halide Perovskites: State of the Art and Perspectives. Appl. Sci. 2019, 9, 4591. [Google Scholar] [CrossRef] [Green Version]
- Milanese, S.; De Giorgi, M.L.; Anni, M. Determination of the Best Empiric Method to Quantify the Amplified Spontaneous Emission Threshold in Polymeric Active Waveguides. Molecules 2020, 25, 2992. [Google Scholar] [CrossRef] [PubMed]
- De Giorgi, M.L.; Krieg, F.; Kovalenko, M.V.; Anni, M. Amplified Spontaneous Emission Threshold Reduction and Operational Stability Improvement in CsPbBr3 Nanocrystals Films by Hydrophobic Functionalization of the Substrate. Sci. Rep. 2019, 9, 17964. [Google Scholar] [CrossRef] [PubMed]
- De Giorgi, M.L.; Lippolis, T.; Jamaludin, N.F.; Soci, C.; Bruno, A.; Anni, M. Origin of Amplified Spontaneous Emission Degradation in MAPbBr3 Thin Films under Nanosecond-UV Laser Irradiation. J. Phys. Chem. C 2020, 124, 10696–10704. [Google Scholar] [CrossRef]
- Cerdán, L.; Costela, A.; Durán-Sampedro, G.; García-Moreno, I.; Calle, M.; Juan-y Seva, M.; de Abajo, J.; Turnbull, G.A. New perylene-doped polymeric thin films for efficient and long-lasting lasers. J. Mater. Chem. 2012, 22, 8938–8947. [Google Scholar] [CrossRef] [Green Version]
- Cerdán, L.; Costela, A.; Durán-Sampedro, G.; García-Moreno, I. Random lasing from sulforhodamine dye-doped polymer films with high surface roughness. Appl. Phys. B 2012, 108, 839–850. [Google Scholar] [CrossRef] [Green Version]
- Cerdán, L.; Martínez-Martínez, V.; García-Moreno, I.; Costela, A.; Pérez-Ojeda, M.E.; Arbeloa, I.L.; Wu, L.; Burgess, K. Naturally Assembled Excimers in Xanthenes as Singular and Highly Efficient Laser Dyes in Liquid and Solid Media. Adv. Opt. Mater. 2013, 1, 984–990. [Google Scholar] [CrossRef]
- Dudley, C. Efficient generation of blue light in oligothiophene chromophores doped in polymer films and fiber. Opt. Mater. 2013, 35, 2115–2121. [Google Scholar] [CrossRef]
- Chen, F.; Wang, J. LDS dye-doped zirconia-organically modified silicate distributed feedback planar waveguide lasers. Appl. Phys. B 2013, 113, 259–264. [Google Scholar] [CrossRef]
- Wu, X.; Li, Y.; Li, W.; Wu, L.; Fu, B.; Wang, W.; Liu, G.; Zhang, D.; Zhao, J.; Chen, P. Enhancing Optically Pumped Organic-Inorganic Hybrid Perovskite Amplified Spontaneous Emission via Compound Surface Plasmon Resonance. Crystals 2018, 8, 124. [Google Scholar] [CrossRef] [Green Version]
- Zarins, E.; Puciriuss, T.; Pervenecka, J.; Vembris, A.; Kokars, V. Glass-forming nonsymmetric DWK-dyes with 5,5,5-triphenylpentyl and piparazine moieties for light-amplification studies. J. Photonics Energy 2018, 8, 046001. [Google Scholar] [CrossRef]
- Litvinas, D.; Aleksiejunas, R.; Scajev, P.; Baronas, P.; Soriute, V.; Qin, C.; Fujihara, T.; Matsushima, T.; Adachi, C.; Jursenas, S. Energy transfer in (PEA)2FAn-1PbnBr3n+1 quasi-2D perovskites. J. Mater. Chem. C 2021, 9, 4782–4791. [Google Scholar] [CrossRef]
- Aoki, R.; Komatsu, R.; Goushi, K.; Mamada, M.; Ko, S.Y.; Wu, J.W.; Placide, V.; D’Aléo, A.; Adachi, C. Realizing Near-Infrared Laser Dyes through a Shift in Excited-State Absorption. Adv. Opt. Mater. 2021, 9, 2001947. [Google Scholar] [CrossRef]
- Zarins, E.; Pervenecka, J.; Misina, E.; Bezvikonnyi, O.; Vembris, A.; Balodis, K.; Volyniuk, D.; Grazulevicius, J.V.; Kokars, V. HAPPY Dyes as Light Amplification Media in Thin Films. J. Org. Chem. 2021, 86, 3213–3222. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Luo, M.; Liu, Y.; Li, M.; Pi, M.; Yang, J.; Chen, Y.; Zhang, Z.; Du, J.; Zhang, D.; et al. Air-Processed MAPbBr3 Perovskite Thin Film with Ultrastability and Enhanced Amplified Spontaneous Emission. Small 2021, 17, 2101107. [Google Scholar] [CrossRef]
- Pourdavoud, N.; Haeger, T.; Mayer, A.; Cegielski, P.J.; Giesecke, A.L.; Heiderhoff, R.; Olthof, S.; Zaefferer, S.; Shutsko, I.; Henkel, A.; et al. Room-Temperature Stimulated Emission and Lasing in Recrystallized Cesium Lead Bromide Perovskite Thin Films. Adv. Mater. 2019, 31, 1903717. [Google Scholar] [CrossRef] [Green Version]
- Li, S.; Yu, Z.; Xiao, X.; Geng, H.; Wang, K.; Jin, X.; Liao, Q.; Liao, Y.; Wu, Y.; Yao, J.; et al. Modulation of Amplified Spontaneous Emissions between Singlet Fluorescence and Triplet Phosphorescence Channels in Organic Dye Lasers. Laser Photonics Rev. 2019, 13, 1900036. [Google Scholar] [CrossRef]
- Udayan, S.; Sha, M.M.R.; Sebastian, M.; Nampoori, V.; Thomas, S. Two photon induced amplified spontaneous emission at low threshold from Styryl 7 dye incorporated DNA template. Opt. Mater. 2018, 86, 492–497. [Google Scholar] [CrossRef]
- Liu, R.; Chen, W.; Liu, L.; Lu, Y.; Li, W.; Fu, T.; Liu, X.; Hang, X.C.; Sun, Z. Air-stable and low threshold amplified spontaneous emission via CsBr aqueous solution processed all-inorganic CsPbBr3 perovskite films. Appl. Phys. Lett. 2021, 118, 251106. [Google Scholar] [CrossRef]
- Pina, J.M.; Parmar, D.H.; Bappi, G.; Zhou, C.; Choubisa, H.; Vafaie, M.; Najarian, A.M.; Bertens, K.; Sagar, L.K.; Dong, Y.; et al. Deep-Blue Perovskite Single-Mode Lasing through Efficient Vapor-Assisted Chlorination. Adv. Mater. 2021, 33, 2006697. [Google Scholar] [CrossRef] [PubMed]
- Munoz-Mármol, R.; Boj, P.G.; Villalvilla, J.M.; Quintana, J.A.; Zink-Lorre, N.; Sastre-Santos, A.; Arago, J.; Ortì, E.; Baronas, P.; Litvinas, D.; et al. Effect of Substituents at Imide Positions on the Laser Performance of 1,7-Bay-Substituted Perylenediimide Dyes. J. Phys. Chem. C 2021, 125, 12277–12288. [Google Scholar] [CrossRef]
- Kazlauskas, K.; Kreiza, G.; Bobrovas, O.; Adomėnienė, O.; Adomėnas, P.; Jankauskas, V.; Juršėnas, S. Fluorene- and benzofluorene-cored oligomers as low threshold and high gain amplifying media. Appl. Phys. Lett. 2015, 107, 043301. [Google Scholar] [CrossRef]
- Lei, L.; Seyitliyev, D.; Stuard, S.; Mendes, J.; Dong, Q.; Fu, X.; Chen, Y.A.; He, S.; Yi, X.; Zhu, L.; et al. Efficient Energy Funneling in Quasi-2D Perovskites: From Light Emission to Lasing. Adv. Mater. 2020, 32, 1906571. [Google Scholar] [CrossRef]
- Navarro-Arenas, J.; Suárez, I.; Chirvony, V.S.; Gualdrón-Reyes, A.F.; Mora-Seró, I.; Martínez-Pastor, J. Single-Exciton Amplified Spontaneous Emission in Thin Films of CsPbX3 (X = Br, I) Perovskite Nanocrystals. J. Phys. Chem. Lett. 2019, 10, 6389–6398. [Google Scholar] [CrossRef]
- Zou, Y.; Bonal, V.; Moles Quintero, S.; Boj, P.G.; Villalvilla, J.M.; Quintana, J.A.; Li, G.; Wu, S.; Jiang, Q.; Ni, Y.; et al. Perylene-Fused, Aggregation-Free Polycyclic Aromatic Hydrocarbons for Solution-Processed Distributed Feedback Lasers. Angew. Chem. Int. Ed. 2020, 59, 14927–14934. [Google Scholar] [CrossRef]
- Bonal, V.; Morales-Vidal, M.; Boj, P.G.; Villalvilla, J.M.; Quintana, J.A.; Lin, N.; Watanabe, S.; Tsuji, H.; Nakamura, E.; Díaz-García, M.A. Kinetically Protected Carbon-Bridged Oligo(p-phenylenevinylene) Derivatives for Blue Color Amplified Spontaneous Emission. Bull. Chem. Soc. Jpn. 2020, 93, 751–758. [Google Scholar] [CrossRef]
- Kallinger, C.; Riechel, S.; Holderer, O.; Lemmer, U.; Feldmann, J.; Berleb, S.; Mückl, A.G.; Brütting, W. Picosecond amplified spontaneous emission bursts from a molecularly doped organic semiconductor. J. Appl. Phys. 2002, 91, 6367–6370. [Google Scholar] [CrossRef]
- Liu, Z.; Wang, C.; Hu, Z.; Du, J.; Yang, J.; Zhang, Z.; Shi, T.; Liu, W.; Tang, X.; Leng, Y. Mode selection and high-quality upconversion lasing from perovskite CsPb2Br5 microplates. Photonics Res. 2020, 8, A31–A38. [Google Scholar] [CrossRef]
- Mysliwiec, J.; Szukalski, A.; Sznitko, L.; Miniewicz, A.; Haupa, K. Studies of new organic molecules and hybrid systems for lasing applications. In Proceedings of the Organic Photonic Materials and Devices XV; SPIE: Bellingham, WA, USA, 2013; Volume 8622, pp. 30–35. [Google Scholar] [CrossRef]
- Li, J.; Liu, J.; Mei, E.; Du, J.; Liang, X.; Liu, Z.; Wang, X.; Xiang, W. Low threshold amplified spontaneous emission in ultrastable CsPbBr3-TS-1 nanocomposite films under two-photon excitation. Appl. Phys. Lett. 2021, 119, 091102. [Google Scholar] [CrossRef]
- De Giorgi, M.L.; Perulli, A.; Yantara, N.; Boix, P.P.; Anni, M. Amplified Spontaneous Emission Properties of Solution Processed CsPbBr3 Perovskite Thin Films. J. Phys. Chem. C 2017, 121, 14772–14778. [Google Scholar] [CrossRef]
- Thompson, J.; Anni, M.; Lattante, S.; Pisignano, D.; Blyth, R.; Gigli, G.; Cingolani, R. Amplified spontaneous emission in the near infrared from a dye-doped polymer thin film. Synth. Met. 2004, 143, 305–307. [Google Scholar] [CrossRef]
- Anni, M.; Lattante, S.; Cingolani, R.; Gigli, G.; Barbarella, G.; Favaretto, L. Emission properties of organic random lasers. Phys. Status Solidi C 2004, 1, 450–453. [Google Scholar] [CrossRef]
- Murzin, A.O.; Stroganov, B.V.; Günnemann, C.; Hammouda, S.B.; Shurukhina, A.V.; Lozhkin, M.S.; Emeline, A.V.; Kapitonov, Y.V. Amplified Spontaneous Emission and Random Lasing in MAPbBr3 Halide Perovskite Single Crystals. Adv. Opt. Mater. 2020, 8, 2000690. [Google Scholar] [CrossRef]
- Cerdán, L.; Costela, A.; García-Moreno, I.; García, O.; Sastre, R. Waveguides and quasi-waveguides based on pyrromethene 597-doped poly(methyl methacrylate). Appl. Phys. B 2009, 97, 73–83. [Google Scholar] [CrossRef]
- Cerdán, L. Simultaneous retrieval of optical gains, losses, and threshold in active waveguides. Opt. Laser Technol. 2020, 121, 105814. [Google Scholar] [CrossRef]
- In order to allow an easier comparison with the previously published threshold values, we used power density and energy density to quantify the ASE threshold of dye-polymer and perovskite samples, respectively.
- Li, Y.; Allegro, I.; Kaiser, M.; Malla, A.J.; Richards, B.S.; Lemmer, U.; Paetzold, U.W.; Howard, I.A. Exciton versus free carrier emission: Implications for photoluminescence efficiency and amplified spontaneous emission thresholds in quasi-2D and 3D perovskites. Mater. Today 2021, 49, 35–47. [Google Scholar] [CrossRef]


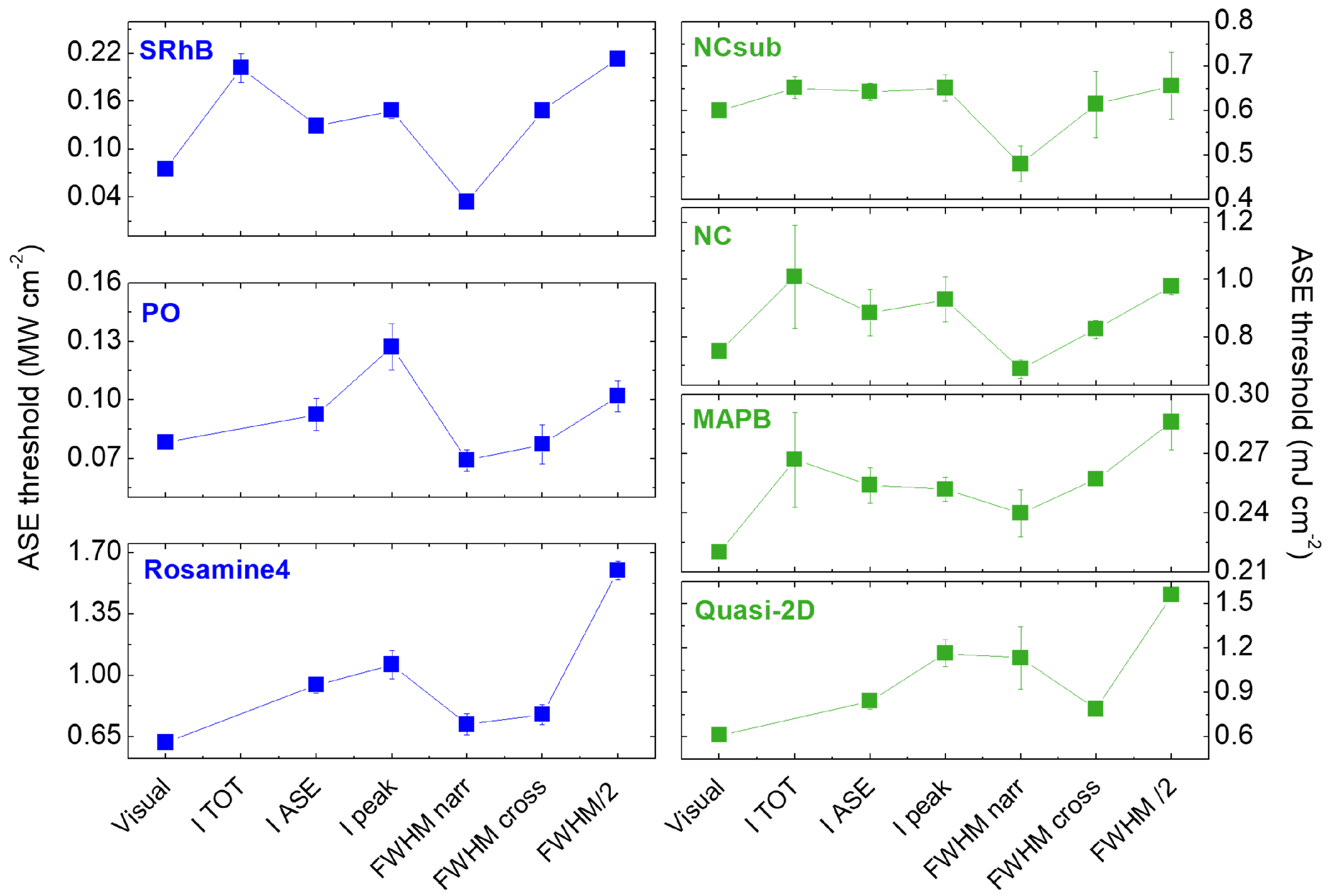
| ASE Threshold () | ||||
|---|---|---|---|---|
| NCsub | NC | MAPB | Quasi-2D | |
| Visual | ||||
| – | ||||
| ASE Threshold () | |||
|---|---|---|---|
| SRhB | PO | Rosamine4 | |
| Visual | ∼0.075 | ∼0.078 | ∼0.62 |
| – | – | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Milanese, S.; De Giorgi, M.L.; Cerdán, L.; La-Placa, M.-G.; Jamaludin, N.F.; Bruno, A.; Bolink, H.J.; Kovalenko, M.V.; Anni, M. Amplified Spontaneous Emission Threshold Dependence on Determination Method in Dye-Doped Polymer and Lead Halide Perovskite Waveguides. Molecules 2022, 27, 4261. https://doi.org/10.3390/molecules27134261
Milanese S, De Giorgi ML, Cerdán L, La-Placa M-G, Jamaludin NF, Bruno A, Bolink HJ, Kovalenko MV, Anni M. Amplified Spontaneous Emission Threshold Dependence on Determination Method in Dye-Doped Polymer and Lead Halide Perovskite Waveguides. Molecules. 2022; 27(13):4261. https://doi.org/10.3390/molecules27134261
Chicago/Turabian StyleMilanese, Stefania, Maria Luisa De Giorgi, Luis Cerdán, Maria-Grazia La-Placa, Nur Fadilah Jamaludin, Annalisa Bruno, Henk J. Bolink, Maksym V. Kovalenko, and Marco Anni. 2022. "Amplified Spontaneous Emission Threshold Dependence on Determination Method in Dye-Doped Polymer and Lead Halide Perovskite Waveguides" Molecules 27, no. 13: 4261. https://doi.org/10.3390/molecules27134261








