The Solubility Parameters of Ionic Liquids

Abstract

The Hildebrand’s solubility parameters have been calculated for 18 ionic liquids from the inverse gas chromatography measurements of the activity coefficients at infinite dilution. Retention data were used for the calculation. The solubility parameters are helpful for the prediction of the solubility in the binary solvent mixtures. From the solubility parameters, the standard enthalpies of vaporization of ionic liquids were estimated.

1. Introduction

Ionic liquids (ILs) have become the subject of an increasing number of investigations due to their unique properties such as wide liquid range, stability at high temperatures, no flammability and negligible vapor pressure. Ionic liquids as green solvents can be used in separation processes, synthesis, catalysis and electrochemistry, successfully replacing the conventional volatile, flammable and toxic organic solvents. Since the ILs have a negligible vapor pressure, the inverse gas chromatography (IGC) is a suitable method for measuring thermodynamic properties of pure substances and their mixtures [1]. From the retention data, the activity coefficients at infinite dilution, Flory-Huggins interaction parameters as well as the Hildebrand’s solubility parameters can be determined. Activity coefficients at infinite dilution are very important for calculations of selectivity and capacity of entrainers for the different separation problems, characterizing the behavior of liquid mixtures, estimation of mutual solubilities, fitting the excess molar energy (G^E) model parameters (e.g., Wilson, NRTL, UNIQUAC), predicting the existence of an azeotrope, analytical chromatography, calculation of Henry constant and partition coefficients, development of thermodynamic models based on the group contribution methods such as mod. UNIFAC [2]. The values of the activity coefficients at infinite dilution for the investigated ionic liquids were published earlier [3–18].

The Hildebrand’s solubility parameters have numerous applications including gas-liquid solubility, solvent extraction and many others as described in detail in the literature [19,20]. Solubility parameters are available for only some of the ionic liquids determined by IGC [21–24], intrinsic viscosity method [25] or estimated from Kamlet-Taft equation [26]. This paper provides information on the Hildebrand’s solubility parameters determined for 18 ionic liquids as a function of temperature and the standard enthalpies of vaporization calculated from the values of the solubility parameters.

2. Results and Discussion

The Hildebrand’s solubility parameters (δ₂) were calculated for the ionic liquids presented (with abbreviations and structures) in

Table 1. Abbreviations, names and structures of investigated ionic liquids.

Abbreviation	Name	Structure	Reference
[emim][TFA]	1-Ethyl-3-methyl-imidazolium trifluoroacetate		[3]
[emim][SCN]	1-Ethyl-3-methyl-imidazolium thiocyanate		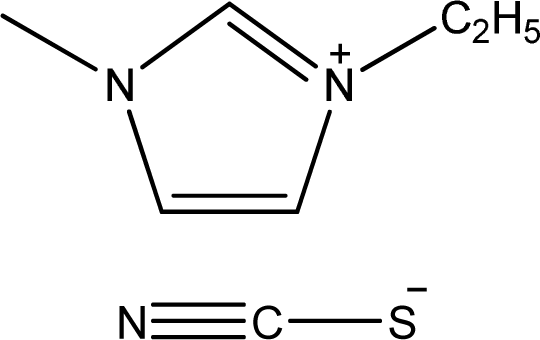[4]
[bmim][SCN]	1-Butyl-3-methyl-imidazolium thiocyanate		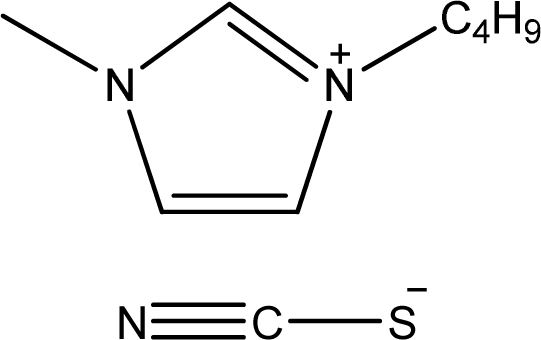[5]
[hmim][SCN]	1-Hexyl-3-methyl-imidazolium thiocyanate		[6]
[1,4bmPY][SCN]	1-Butyl-4-methyl-pyridinium thiocyanate		[7]
[bmPYR][SCN]	1-Butyl-1-methyl-pyrrolidinium thiocyanate		[7]
[bmim][CF3SO3]	1-Butyl-3-methyl-imidazolium trifluoromethanesulfonate		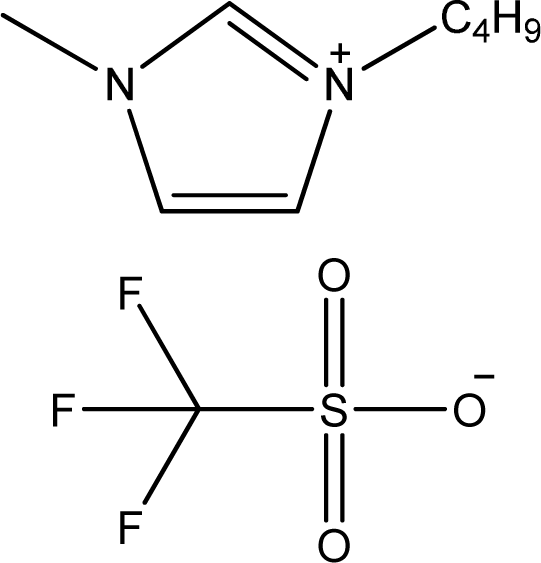[8]
[1,3bmPY][CF3SO3]	1-Butyl-3-methyl-pyridinium trifluoromethanesulfonate		[9]
[bmPYR][CF3SO3]	1-Butyl-1-methyl-pyrrolidinium trifluoromethanesulfonate		[10]
[bmim][MDEGSO4]	1-Butyl-3-methyl-imidazolium 2-(2-methoxyethoxy)ethyl sulfate		[11]
[bmim][OcSO4]	1-Butyl-3-methyl-imidazolium octyl sulfate		[12]
[P1,i4,i4,i4][TOS]	Triisobutyl-methyl-phosphonium tosylate		[13]
[1,4bmPY][TOS]	1-Butyl-4-methyl-pyridinium tosylate		[14]
[1,4bmPY][NTf2]	1-Butyl-4-methyl-pyridinium bis(trifluoromethylsulfonyl)-amide		[15]
[C6OCmim][NTf2]	1-Hexyloxymethyl-3-methyl-imidazolium bis(trifluoromethylsulfonyl)-amide		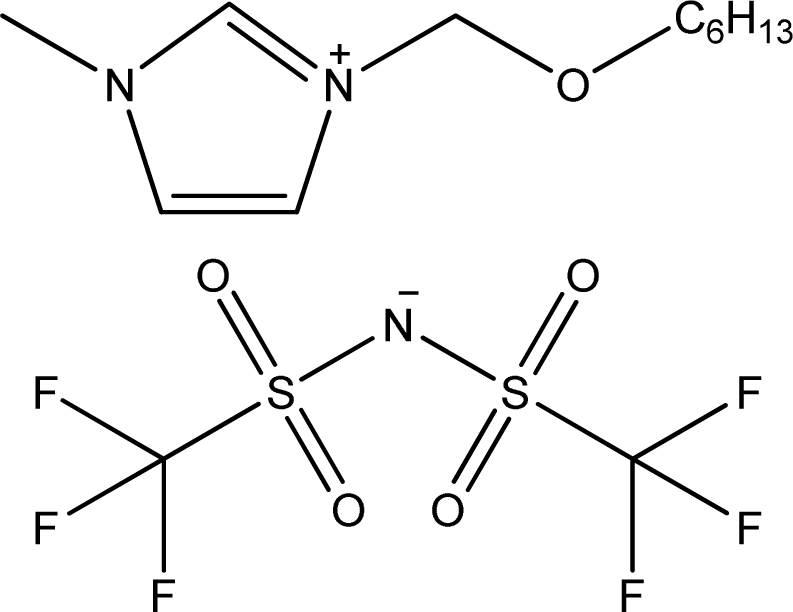[16]
[(C6OC)2im][NTf2]	1,3-Dihexyloxymethyl-imidazolium bis(trifluoromethylsulfonyl)-amide		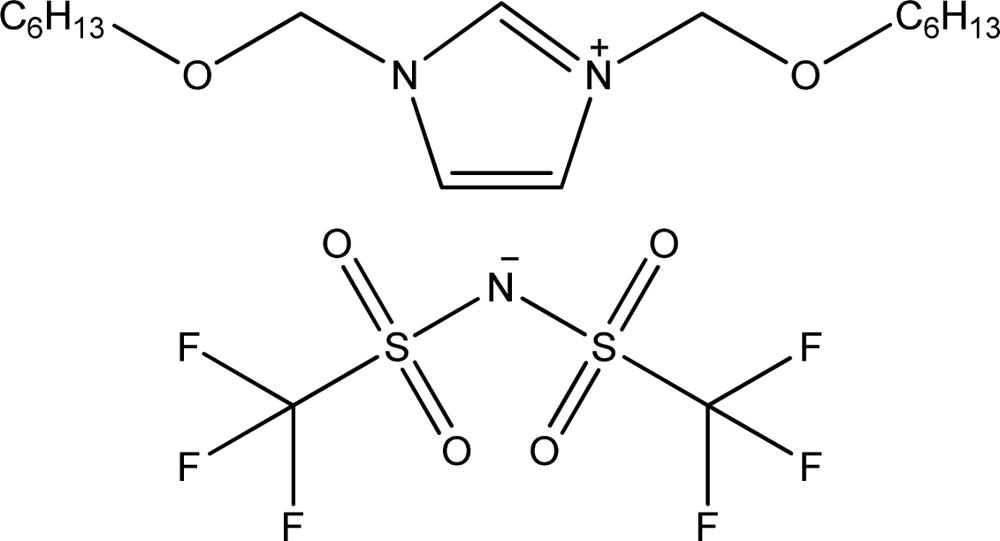[16]
[Et3S][NTf2]	Triethyl-sulfonium bis(trifluoromethylsulfonyl)-amide		[17]
[hmim][NTf2]	1-Hexyl-3-methyl-imidazolium bis(trifluoromethylsulfonyl)-amide		[18]

. The solubility parameters show a slight dependence on the temperature, which was also observed by Mutelet et al. [21–23]. The results are presented in

Table 2. Hildebrand’s solubility parameters δ₂ for the different ionic liquids.

Ionic liquid	T/K	δ2/MPa0.5
[emim][TFA]	298.15	25.561
	328.15	25.58
	338.15	25.59
	348.15	25.60
	358.15	25.60
[emim][SCN]	298.15	25.191
	308.15	25.24
	318.15	25.33
	328.15	25.41
	338.15	25.46
	348.15	25.55
	358.15	25.57
[bmim][SCN]	298.15	24.641
	318.15	24.70
	328.15	24.72
	338.15	24.75
	348.15	24.77
	358.15	24.80
[hmim][SCN]	298.15	23.651
	318.15	23.74
	328.15	23.79
	338.15	23.84
	348.15	23.90
	358.15	23.93
	368.15	23.98
[1,4bmPY][SCN]	298.15	24.53
	308.15	24.57
	318.15	24.62
	328.15	24.67
	338.15	24.71
	348.15	24.74
	358.15	24.77
[bmPYR][SCN]	298.15	24.96
	308.15	24.98
	318.15	25.00
	328.15	25.01
	338.15	25.02
	348.15	25.04
	358.15	25.05
[bmPYR][SCN]	298.15	24.96
	308.15	24.98
	318.15	25.00
	328.15	25.01
	338.15	25.02
	348.15	25.04
	358.15	25.05
[bmim][CF3SO3]	298.15	22.671
	308.15	22.74
	318.15	22.81
	328.15	22.87
	338.15	22.97
	348.15	23.03
	358.15	23.09
[1,3bmPY][CF3SO3]	298.15	22.471
	318.15	22.61
	328.15	22.68
	338.15	22.75
	348.15	22.84
	358.15	22.89
[bmPYR][CF3SO3]	298.15	22.831
	318.15	22.94
	328.15	23.01
	338.15	23.06
	348.15	23.13
	358.15	23.17
	368.15	23.24
[bmim][MDEGSO4]	298.15	24.80
	303.15	24.80
	308.15	24.81
[bmim][OcSO4]	298.15	22.83
	313.15	23.00
	328.15	23.25
[P1,i4,i4,i4][TOS]	298.15	24.331
	318.15	24.20
	328.15	24.13
	338.15	24.05
	348.15	23.99
	358.15	23.93
[1,4bmPY][TOS]	298.15	23.061
	328.15	23.24
	333.15	23.27
	338.15	23.29
	343.15	23.33
[1,4bmPY][NTf2]	298.15	20.611
	318.15	20.82
	328.15	20.92
	338.15	21.05
	348.15	21.15
	358.15	21.25
	368.15	21.35
[C6OCmim][NTf2]	298.15	20.261
	318.15	20.48
	328.15	20.59
	338.15	20.71
	348.15	20.82
	358.15	20.93
	368.15	21.05
[(C6OC)2im][NTf2]	298.15	19.601
	318.15	19.81
	328.15	19.92
	338.15	20.03
	348.15	20.14
	358.15	20.25
	368.15	20.35
[Et3S][NTf2]	298.15	21.051
	308.15	21.13
	318.15	21.25
	328.15	21.35
	338.15	21.47
	348.15	21.55
	358.15	21.66
	368.15	21.72
[hmim][NTf2]	298.15	20.25
	308.15	20.36
	313.15	20.44
	328.15	20.58
	333.15	20.64
	338.15	20.70
	348.15	20.83
Solubility parameters taken from the literature
[mmim][(CH3)2PO4] [21]	312.55	26.54
	332.65	25.96
	352.75	25.16
[emim][(C2H5)2PO4] [21]	312.65	25.81
	332.55	25.44
	352.65	25.32
[emim][NTf2] [23]	313.15	22.31
[emim][NTf2] [25]	298.15	27.6
[emim][BF4] [24]	298.15	24.4
[bmim][BF4] [24]	298.15	24.3
[bmim][BF4] [25]	298.15	31.6
[bmim][NTf2] [25]	298.15	26.7
[bmim][NTf2] [26]	298.15	25.5
[bmim][CF3SO3] [25]	298.15	24.9
[bmim][CF3SO3] [26]	298.15	25.4
[bmim][PF6] [23]	313.15	23.2
	323.15	22.62
	333.15	22.05
[bmim][PF6] [25]	298.15	29.8
[bmim][PF6] [26]	298.15	30.2
[bmim][SbF6] [26]	298.15	31.5
[bmmim][NTf2] [26]	298.15	24.2
[hmim][BF4] [24]	298.15	23.3
[hmim][NTf2] [25]	298.15	25.6
[hmim][PF6] [25]	298.15	28.6
[omim][BF4] [24]	298.15	22.5
[omim][NTf2] [25]	298.15	25.0
[omim][PF6] [25]	298.15	27.8
[omim][Cl] [23]	313.15	17.91
[C16mim][BF4] [22]	323.15	19.52
	333.15	19.61
	343.15	19.60
[OH-C2mim][BF4] [21]	302.55	22.77
	312.65	22.87
	332.65	22.88
[OH-C2mim][PF6] [21]	302.65	21.84
	312.55	21.93
	332.45	22.45

¹extrapolated values.

and are compared to results taken from the literature [21–26].

The values of δ₂ calculated using the IGC method are not consistent with those obtained by the intrinsic viscosity method or estimated from the Kamlet-Taft equation. For ionic liquid [bmim][CF₃SO₃] the values of δ₂ are 22.67, 24.9 [25] and 25.4 [26] obtained by IGC, intrinsic viscosity method or estimated from Kamlet-Taft equation, respectively. For ionic liquid [hmim][NTf₂] the difference is much greater, values of δ₂ are 20.25 and 25.6 [25] for the IGC and intrinsic viscosity methods, respectively. It was found that values of δ₂ determined using the IGC method by Mutelet et al. [21–23] and Foco et al. [24] are also not consistent with those determined by the two methods mentioned above (Table 2). On the other hand, values obtained by different research groups by IGC are coherent as is shown in Figure 1. From Figure 1, it is obvious that for an ionic liquid of general cation formula [Rmim]⁺, the solubility parameter decreases with an increasing of the alkyl chain R. In the other words, the more aliphatic the cation character, the lower the solubility parameter. The slope of all three lines is similar – it confirms that the data are consistent (except for [emim][BF₄] ionic liquid).

Figure 2 shows the anion influence on the solubility parameter for ionic liquids based on 1-alkyl-3-methyl-imidazolium cations [Rmim]⁺, 1-butyl-(3 or 4)-methyl-pyridinium [bmPY]⁺ and 1-butyl-1-methyl-pyrrolidinium [bmPYR]⁺ cations. The solubility parameter increases in the following order: [Cl]⁻ < [NTf₂]⁻ < [CF₃SO₃]⁻ < [OcSO₄]⁻ < [PF₆]⁻ < [BF₄]⁻ < [TOS]⁻ < [SCN]⁻ < [MDEGSO₄]⁻ < [TFA]⁻. The highest values of δ₂ are for [BF₄]⁻, [TOS]⁻, [SCN]⁻, [MDEGSO₄]⁻ and [TFA]⁻ anions, whilst the lowest value is for the [Cl]⁻ anion.

Figure 3 shows influence of the cation structure on the solubility parameter for ionic liquids based on [SCN]⁻ and [CF₃SO₃]⁻ anions. The lowest values of δ₂ are for butyl-methyl-pyridinium [bmPY]⁺ cations ([1,3bmPY][CF₃SO₃] and [1,4bmPY][SCN]).

The influence of the cation on the solubility parameter for the bis(trifluoromethylsulfonyl)-amide based ionic liquids ([NTf₂]⁻) is shown in Figure 4. The solubility parameter increases in the following order: [(C₆OC)₂im]⁺ < [hmim]⁺ < [C₆OCmim]⁺ < [1,4bmPY]⁺ < [Et₃S]⁺ < [emim]⁺. The difference in solubility parameters between [hmim]⁺ and [C₆OCmim]⁺ cations are very small. It is caused by the similar structure of these two cations. The [C₆OCmim]⁺ cation has an additional methoxy group (–O–CH₂–) in the structure, which causes a little augmentation of δ₂ value. From this figure, it can be concluded again that the solubility parameter is higher for the ionic liquids with less aliphatic character. It is also presented in Figure 1 and was mentioned previously.

Standard enthalpies of vaporization Δ_vapH_298.15 calculated according to equation 8 and molar volumes of ionic liquids necessary in enthalpy calculations are presented in

Table 3. Molar volumes V_m at T = 298.15 K and standard enthalpies of vaporization Δ_vapH_298.15 for investigated ionic liquids.

Ionic liquid	Vm/cm3·mol−1	ΔvapH298.15/kJ·mol−1
[emim][TFA]	173.71	115.97
[emim][SCN]	151.62	98.67	1518
[bmim][SCN]	184.43	114.57	1488
[hmim][SCN]	200.04	114.37
[1,4bmPY][SCN]	196.25	120.5
[bmPYR][SCN]	188.85	120.1
[bmim][CF3SO3]	222.05	116.67	1398	130.29	140.110	145.711
[1,3bmPY][CF3SO3]	234.75	121.07
[bmPYR][CF3SO3]	232.65	123.77
[bmim][MDEGSO4]	284.25	177.6
[bmim][OcSO4]	327.75	173.0
[P1,i4,i4,i4][TOS]	363.46	217.67
[1,4bmPY][NTf2]	304.85	132.07	1528
[C6OCmim][NTf2]	349.95	146.07
[(C6OC)2im][NTf2]	460.25	179.27
[Et3S][NTf2]	273.75	123.77
[hmim][NTf2]	326.45	136.7	1398	141.612	216.410

¹from reference [30];

²from reference [31];

³from reference [32];

⁴from reference [33];

⁵from density measurements performed on Anton Paar Density Meter DMA 4500;

⁶from reference [34];

⁷calculated from extrapolated values of δ₂;

⁸from reference [27];

⁹from reference [28];

¹⁰calculated from δ₂ from reference [25];

¹¹calculated from δ₂ from reference [26];

¹²from reference [29]

, and are contrasted the results taken from the literature [25–29]. The larger differences in values of enthalpies of vaporization are for ionic liquids based on the [SCN]⁻ anion. For ionic [bmim][CF₃SO₃] the difference is not so high: 22 and 13 kJ·mol⁻¹ according to references [27] and [28], respectively. Due to the difference in solubility parameters, values of the enthalpies of vaporization calculated from data from references [25,26] are of course different and larger. For ionic liquid [1,4bmPY][NTf₂] value of the enthalpy of vaporization is lower by 20 kJ·mol⁻¹ than for that obtained by Deyko et al. [27]. A very good consistency in results of enthalpies of vaporization is found for [hmim][NTf₂] ionic liquid. Result obtained from IGC measurements is only of about 2 and 4 kJ·mol⁻¹ lower than for that obtained by Deyko et al. [27] and Zaitsau et al. [29], whilst the enthalpy of vaporization obtained from the solubility parameter determined by intrinsic viscosity method is much higher at of 216.4 kJ·mol⁻¹ [25].

3. Calculation of Solubility Parameters

3.1. Experimental Procedure

The activity coefficients at infinite dilution for all investigated ionic liquids were measured using inverse gas chromatography. Detailed descriptions of materials, apparatus and methods used in each experiment are presented in the certain papers [3–18]. On the basis of the experimental data from the activity coefficients at infinite dilution measurements, the Hildebrand’s solubility parameters have been calculated using equations presented below.

3.2. Theoretical Basis

Retention data were used for the calculation of Hildebrand’s solubility parameters, δ₂. According to the Flory-Huggins theory the interaction parameter at infinite dilution can be determined using the following expression:

$${\chi }_{12}^{\infty }=\text{ln}\left(\frac{273.15R}{P_1^{*}{V}_g{M}_1}\right)-\frac{P_1^{*}\left(B_{11}-V_1^{*}\right)}{RT}+\text{ln}\left(\frac{{\rho }_1}{{\rho }_2}\right)-\left(1-\frac{V_1^{*}}{V_2^{*}}\right)$$

(1)

where R denotes the gas constant, T the temperature, P₁^* the saturated vapor pressure of the solute at temperature T, B₁₁ the second virial coefficient of pure solute, V₁^* and V₂^* the molar volume of the solute and solvent respectively, M₁ the molar mass of solute, ρ₁ and ρ₂ density of solute and solvent respectively, V_g specific retention volume which is given by:

$$V_g=\frac{273.15V_{\text{N}}}{T{m}_2}$$

(2)

where m₂ denotes the mass of the solvent on the column packing and V_N the net retention volume of the solute given by:

$$V_{\text{N}}=J_2^3{U}_{\text{o}}(t_{\text{R}}-t_{\text{G}})$$

(3)

where t_R and t_G are the retention times for the solute and an unretained gas, respectively, U_o is the column outlet flow rate, $J_2^3$ the pressure correction term given by:

$$J_2^3=\frac{2}{3}\frac{{(P_{\text{i}}/P_{\text{o}})}^3-1}{{(P_{\text{i}}/P_{\text{o}})}^2-1}$$

(4)

where P_i and P_o denote the inlet and the outlet pressure, respectively.

The column outlet flow rate corrected for the vapor pressure of water U_o is given by:

$$U_{\text{o}}=U\left(1-\frac{P_w}{P_o}\right)\frac{T}{T_f}$$

(5)

where T_f is the temperature of the flow meter, P_w is the vapor pressure of water at T_f and U is the flow rate measured with the bubble flow meter.

The interaction parameter ${\chi }_{12}^{\infty }$ may be expressed as a function of δ₁ and δ₂ which denote the solubility parameters of the solute and of the solvent, respectively by:

$${\chi }_{12}^{\infty }=\frac{V_1^{*}{({\delta }_1-{\delta }_2)}^2}{RT}$$

(6)

Equation 6 can be rewritten as:

$$\left(\frac{{\delta }_1^2}{RT}-\frac{{\chi }_{12}^{\infty }}{V_1^{*}}\right)=\left(\frac{2{\delta }_2}{RT}\right){\delta }_1-\frac{{\delta }_2^2}{RT}$$

(7)

The solubility parameters δ₁ of the solutes were calculated using following equation:

$${\delta }^2=\frac{{\Delta }_{\text{vap}}H-RT}{\upsilon }$$

(8)

where Δ_vapH denotes enthalpy of vaporization and υ the molar volume. Enthalpies of vaporization of solutes were taken from literature [35] and molar volumes were calculated from densities taken from literature [36]. The values of B₁₁ were calculated using the McGlashan and Potter [37] equation for alkanes and Tsonopolous [38] equation for the rest of solvents. The vapor pressure values were calculated using equation and constants taken from the literature [36,39,40]. Critical data used to calculate B₁₁ were obtained from literature [41,42].

Values of ${\chi }_{12}^{\infty }$ were determined from equation 1. If the left side of equation 7 is plotted against δ₁, a straight line having a slope of 2δ₂/RT and an intercept of $-{\delta }_2^2/RT$ is obtained. The solubility parameter of the solvent δ₂ (ionic liquid) can be calculated from the slope and from the intercept of the straight line. The agreement of both δ₂ values confirms the applicability of the method to the considered system. An example plot $\frac{{\delta }_1^2}{RT}-\frac{{\chi }_{12}^{\infty }}{V_1^{*}}$ versus δ₁ is given in Figure 5 for ionic liquid [(C₆OC)₂im][NTf₂] at T = 368.15 K. From the slope and interception of straight line the solubility parameter was determined, giving results of 20.30 and 20.40, respectively. Then the average of these values was taken as a final result. The correlation coefficient in this example is 0.996. Hildebrand’s solubility parameters of the investigated ionic liquids and the estimated standard enthalpy of vaporization calculated using equation 8 are listed in Tables 2 and 3, respectively.

4. Conclusions

Inverse gas chromatography is a reliable method to determine Hildebrand’s solubility parameters. Data obtained for 18 ionic liquids are coherent with those obtained by different research group by the same method. From the solubility parameters the standard enthalpies of vaporization can be calculated. Obtained values of enthalpies of vaporization are in acceptable consistency with the data available in literature except for ionic liquids based on thiocyanate anion.