Energies 2009, 2(2), 307-319; doi:10.3390/en20200307
Article

Global Assessment of High-Altitude Wind Power

Cristina L. Archer 1,* email and Ken Caldeira 2 email
1 Department of Geological and Environmental Sciences, California State University – Chico, Chico, CA 95929, USA 2 Department of Global Ecology, Carnegie Institution of Washington, Stanford, CA 94305, USA
* Author to whom correspondence should be addressed.
Received: 20 April 2009; in revised form: 15 May 2009 / Accepted: 18 May 2009 / Published: 26 May 2009
(This article belongs to the Special Issue Wind Energy)
PDF Full-text Download PDF Full-Text [818 KB, uploaded 26 May 2009 17:00 CEST]
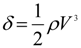
Abstract: The available wind power resource worldwide at altitudes between 500 and 12,000 m above ground is assessed for the first time. Twenty-eight years of wind data from the reanalyses by the National Centers for Environmental Prediction and the Department of Energy are analyzed and interpolated to study geographical distributions and persistency of winds at all altitudes. Furthermore, intermittency issues and global climate effects of large-scale extraction of energy from high-altitude winds are investigated.
Keywords: wind power; high altitude; reanalyses; jet streams; intermittency

Supplementary Files

Article Statistics

Click here to load and display the download statistics.

Cite This Article

MDPI and ACS Style

Archer, C.L.; Caldeira, K. Global Assessment of High-Altitude Wind Power. Energies 2009, 2, 307-319.

AMA Style

Archer CL, Caldeira K. Global Assessment of High-Altitude Wind Power. Energies. 2009; 2(2):307-319.

Chicago/Turabian Style

Archer, Cristina L.; Caldeira, Ken. 2009. "Global Assessment of High-Altitude Wind Power." Energies 2, no. 2: 307-319.

Energies EISSN 1996-1073 Published by MDPI AG, Basel, Switzerland RSS E-Mail Table of Contents Alert