Multiscale Modeling of Polycrystalline NiTi Shape Memory Alloy under Various Plastic Deformation Conditions by Coupling Microstructure Evolution and Macroscopic Mechanical Response
Abstract
:1. Introduction
2. Modeling Methodology
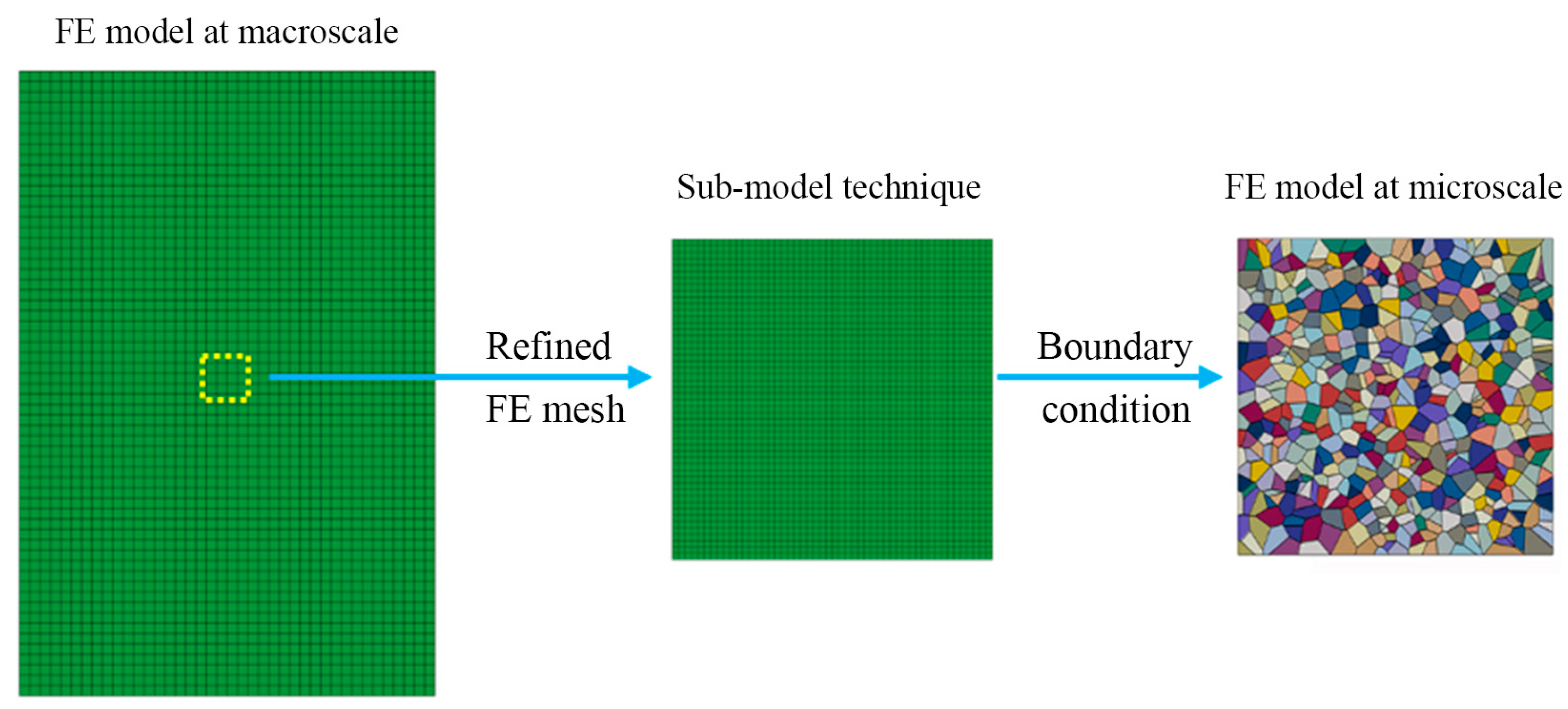
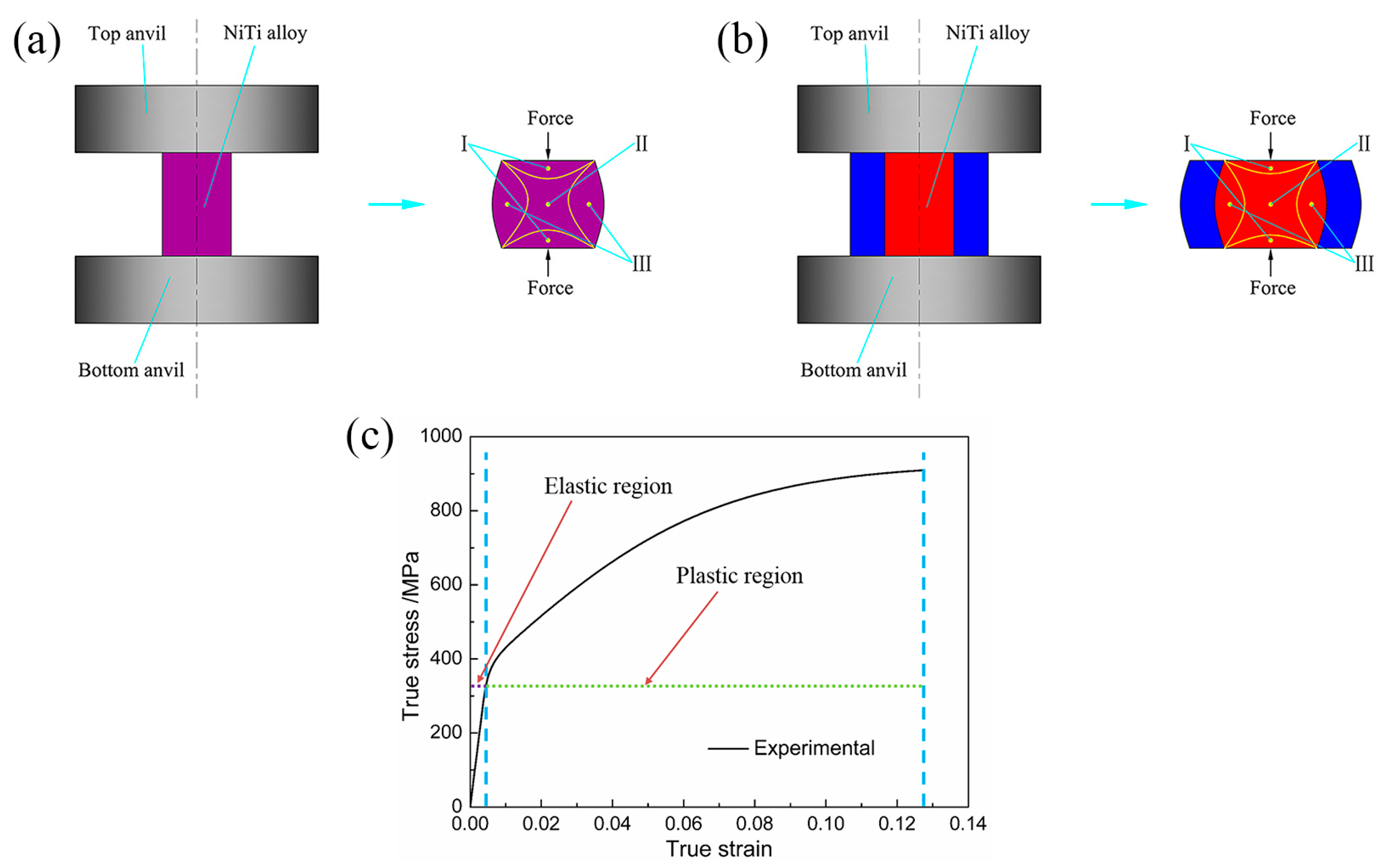
2.1. Macroscale Finite Element Model
2.2. Microscale Finite Element Model
2.3. Crystal Plasticity Constitutive Model
3. Results and Discussion
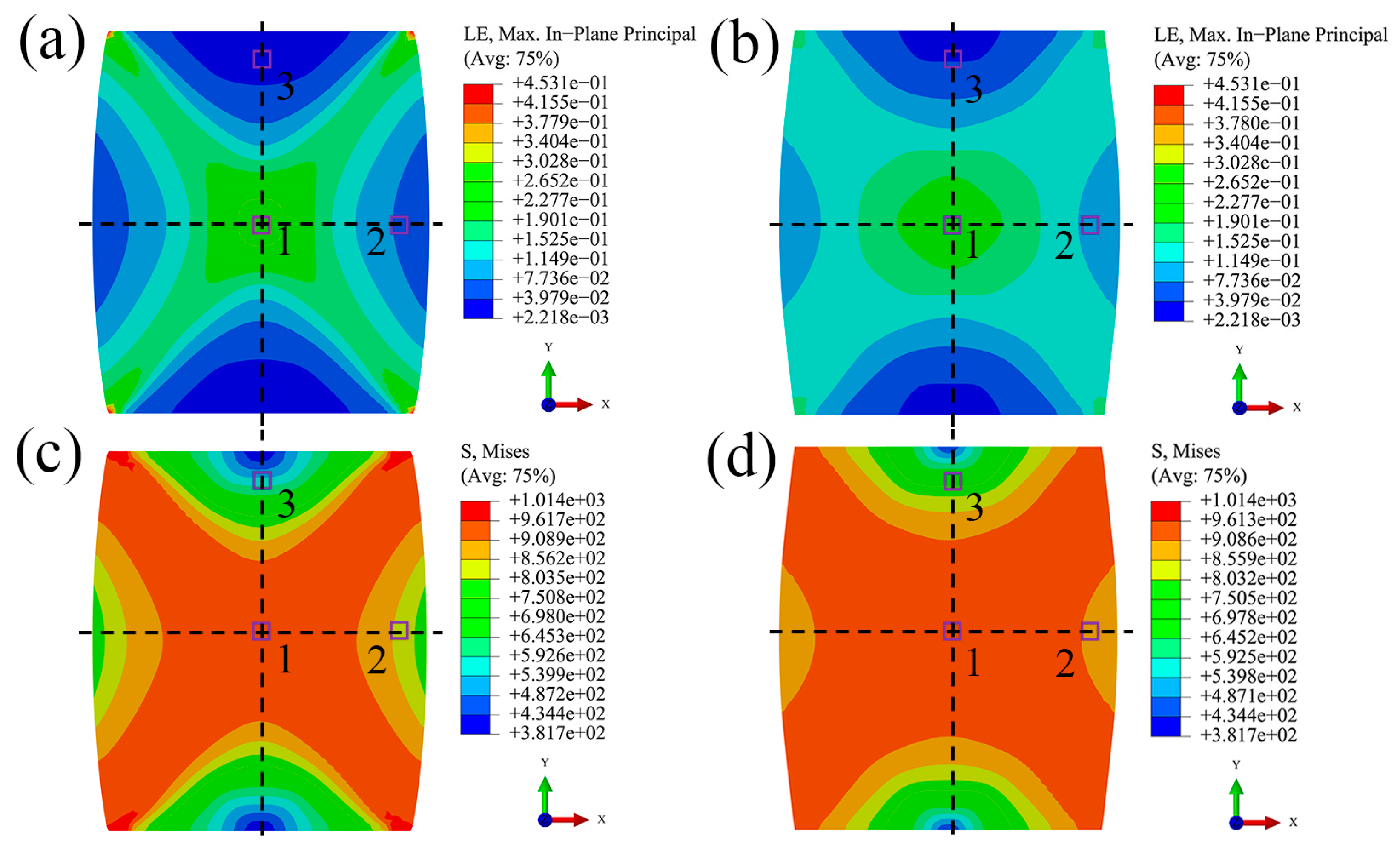
3.1. Distribution of Stress and Strain at Macroscale
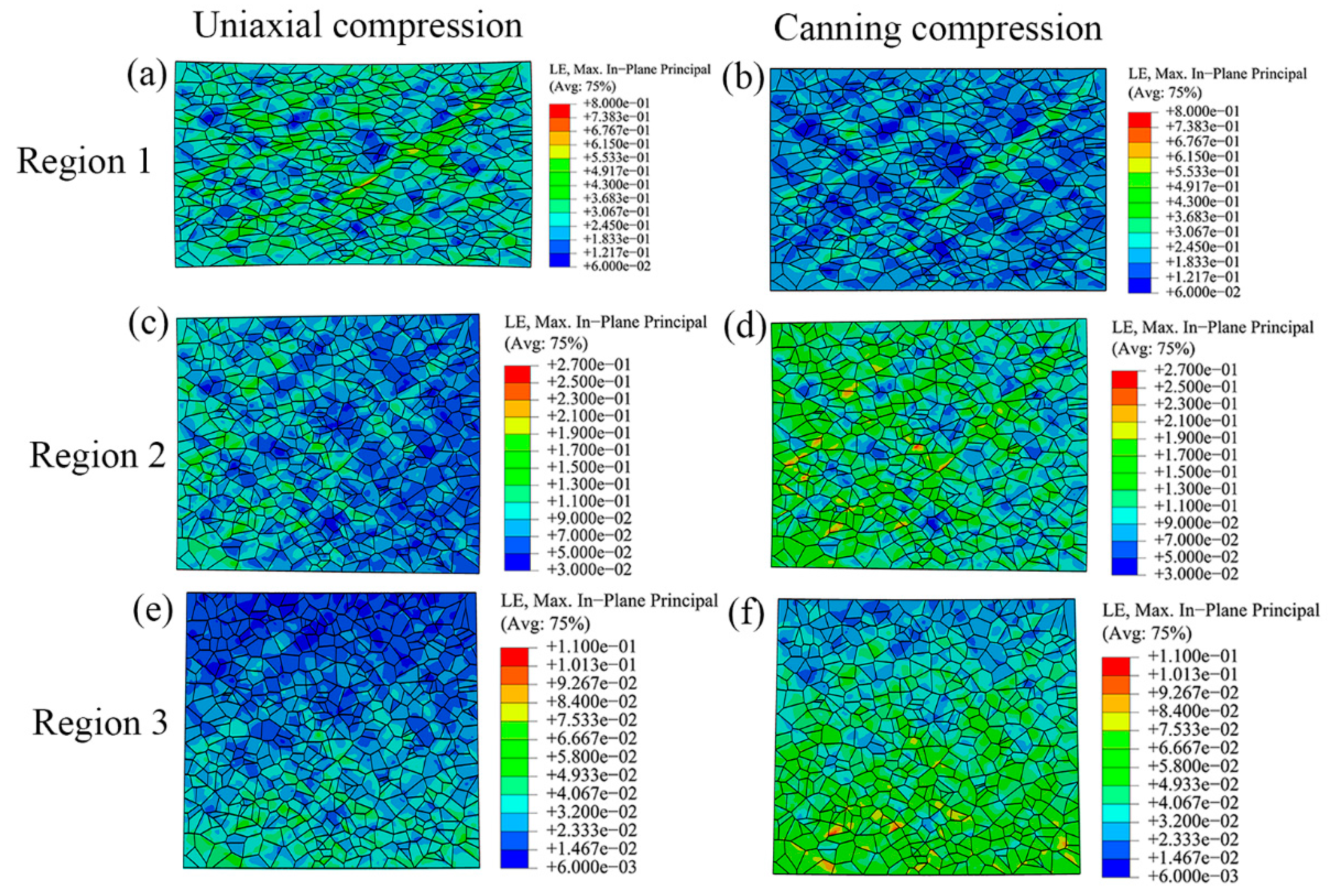
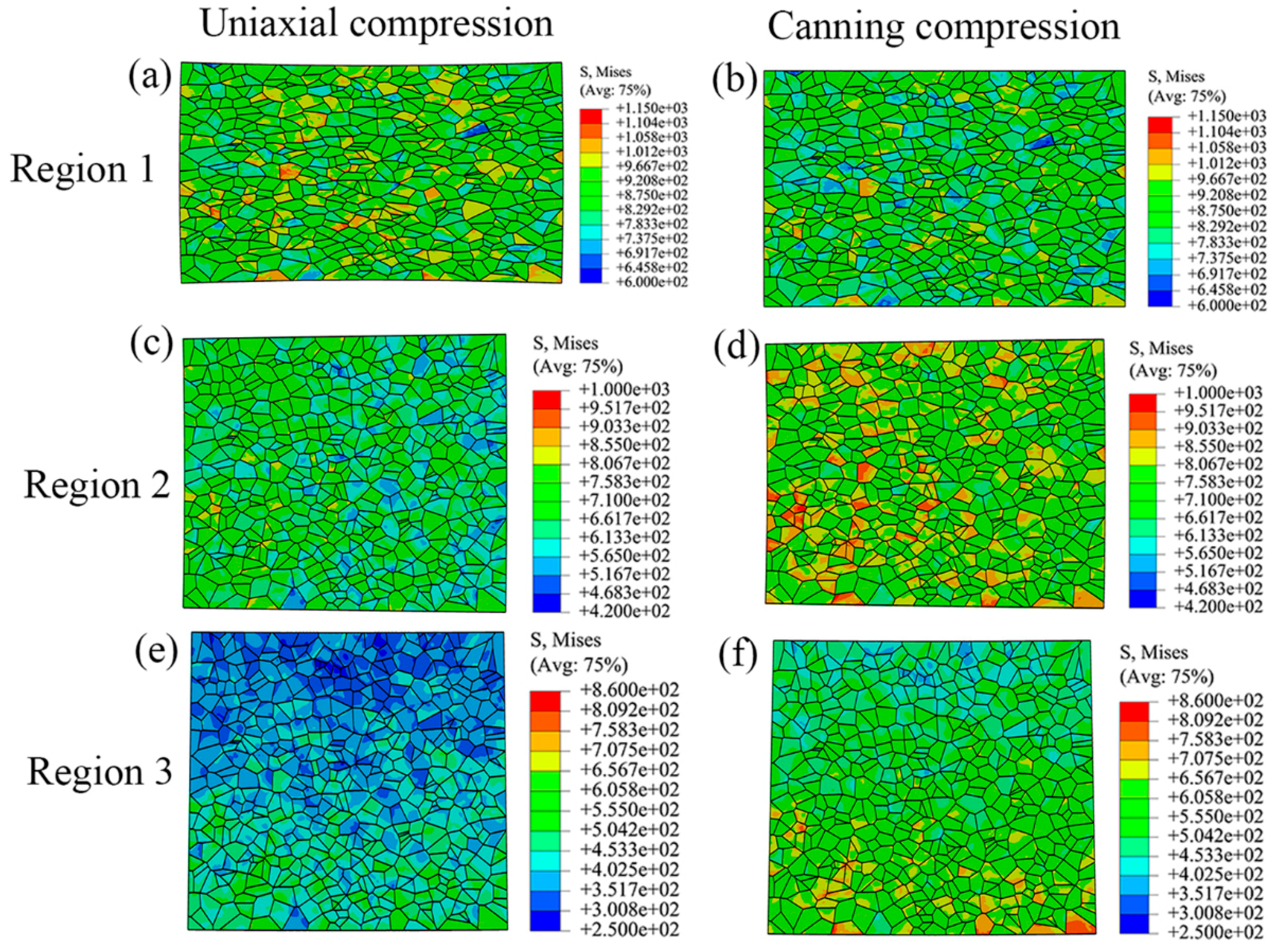
3.2. Distribution of Stress and Strain at Microscale
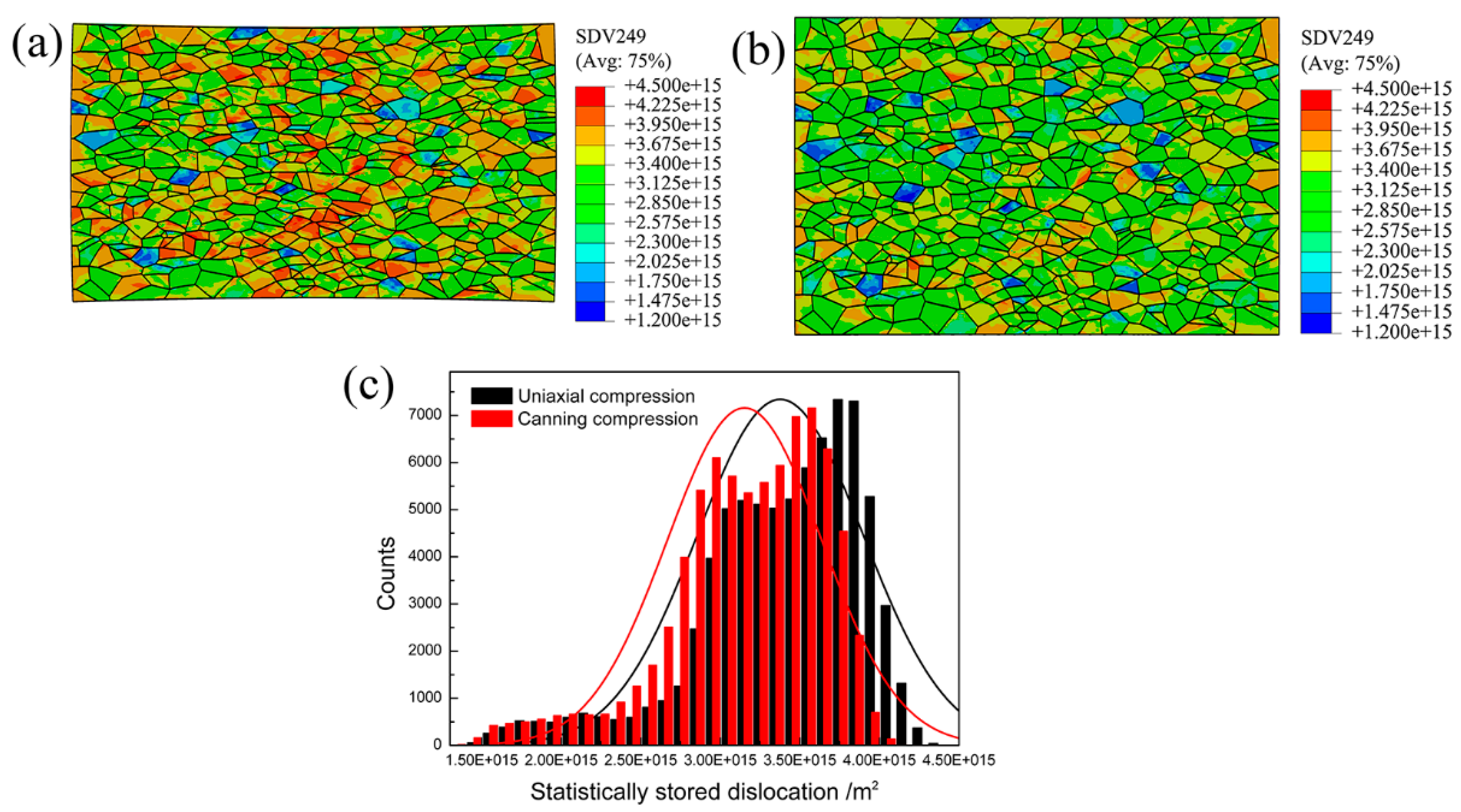
3.3. Distribution of Dislocation at Microscale
4. Conclusions
- (1)
- At the macroscale, it can be concluded that canning compression contributes to relieving the inhomogeneous plastic strain as the compression of the low carbon steel can results in a three-dimensional compressive loading state of NiTi SMA sample.
- (2)
- The sub-model technique in the finite element code ABAQUS is used to extracted the accurate deformation history of selected regions at the macroscale simulation. Then, this deformation history, which reflects the complex interaction between the polycrystalline aggregation and neighboring grains, can serve as boundary conditions used at the microsacle simulation.
- (3)
- At the microscale, it can be concluded that the mitigation of inhomogeneous plastic deformation in canning compression contributes to various microstructure evolution in selected regions. Moreover, the ease of inhomogeneity in plastic strain contributes to reducing the difference of the stress level in various regions and reducing statistically stored dislocation (SSD) density within the deformed NiTi SMA sample. Therefore, canning compression is favorable of sustaining large plastic deformation.
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Isalgue, A.; Auguet, C.; Grau, R.; Torra, V.; Cinca, N.; Fernadez, J. Behavior of NiTi wires for dampers and actuators in extreme conditions. J. Mater. Eng. Perform. 2015, 24, 3323–3327. [Google Scholar] [CrossRef]
- Liu, Y. The superelastic anisotropy in a NiTi shape memory alloy thin sheet. Acta Mater. 2015, 95, 411–427. [Google Scholar] [CrossRef]
- Twohig, E.; Tiernan, P.; Butler, J.; Dickinson, C.; Tofail, S.A.M. Mechanical, microstructural and thermal properties of a 50:50 at.% nickel–titanium alloy subjected to a dieless drawing process. Acta Mater. 2014, 68, 140–149. [Google Scholar] [CrossRef]
- Paranjape, H.M.; Manchiraju, S.; Anderson, P.M. A phase field-Finite element approach to model the interaction between phase transformations and plasticity in shape memory alloys. Int. J. Plast. 2016, 80, 1–18. [Google Scholar] [CrossRef]
- Weafer, F.M.; Guo, Y.; Bruzzi, M.S. The effect of crystallographic texture on stress-induced martensitic transformation in NiTi: A computational analysis. J. Mech. Behav. Biomed. Mater. 2016, 53, 210–217. [Google Scholar] [CrossRef] [PubMed]
- Gall, K.; Tyber, J.; Wilkesanders, G.; Robertson, S.W.; Ritchie, R.O.; Maier, H.J. Effect of microstructure on the fatigue of hot-rolled and cold-drawn NiTi shape memory alloys. Mater. Sci. Eng. A 2008, 486, 389–403. [Google Scholar] [CrossRef]
- Manchiraju, S.; Gaydosh, D.; Benafan, O.; Noebe, R.; Vaidyanathan, R.; Anderson, P.M. Thermal cycling and isothermal deformation response of polycrystalline NiTi: Simulations vs. experiment. Acta Mater. 2011, 59, 5238–5249. [Google Scholar] [CrossRef]
- Kazemi-Choobi, K.; Khalil-Allafi, J.; Abbasi-Chianeh, V. Investigation of the recovery and recrystallization processes of Ni 50.9 Ti 49.1 shape memory wires using in situ electrical resistance measurement. Mater. Sci. Eng. A 2012, 551, 122–127. [Google Scholar] [CrossRef]
- Benafan, O.; Noebe, R.D.; Padula, S.A., II; Garg, A.; Clausen, B.; Vogel, S.; Vaidyanathan, R. Temperature dependent deformation of the B2 austenite phase of a NiTi shape memory alloy. Int. J. Plast. 2013, 51, 103–121. [Google Scholar] [CrossRef]
- Hu, L.; Jiang, S.Y.; Zhang, Y.Q.; Zhao, Y.N.; Liu, S.W.; Zhao, C.Z. Multiple plastic deformation mechanisms of NiTi shape memory alloy based on local canning compression at various temperatures. Intermetallics 2016, 70, 45–52. [Google Scholar] [CrossRef]
- Zaki, W.; Moumni, Z. A three-dimensional model of the thermomechanical behavior of shape memory alloys. J. Mech. Phys. Solids 2007, 55, 2455–2490. [Google Scholar] [CrossRef]
- Auricchio, F.; Reali, A.; Stefanelli, U. A three-dimensional model describing stress-induced solid phase transformation with permanent inelasticity. Int. J. Plast. 2007, 23, 207–226. [Google Scholar] [CrossRef]
- Yu, C.; Kang, G.; Song, D.; Kan, Q. Micromechanical constitutive model considering plasticity for super-elastic NiTi shape memory alloy. Comput. Mater. Sci. 2012, 56, 1–5. [Google Scholar] [CrossRef]
- Armattoe, K.M.; Haboussi, M.; Zineb, T.B. A 2D finite element based on a nonlocal constitutive model describing localization and propagation of phase transformation in shape memory alloy thin structures. Int. J. Solids Struct. 2012, 51, 1208–1220. [Google Scholar] [CrossRef]
- Haroush, S.; Priel, E.; Moreno, D.; Busiba, A.; Silverman, I.; Turgeman, A.; Shneck, R.; Gelbstein, Y. Evaluation of the mechanical properties of SS-316L thin foils by small punch testing and finite element analysis. Mater. Des. 2015, 83, 75–84. [Google Scholar] [CrossRef]
- Roters, F.; Eisenlohr, P.; Hantcherli, L.; Tjahjanto, D.D.; Bieler, T.R.; Raabe, D. Overview of constitutive laws, kinematics, homogenization and multiscale methods in crystal plasticity finite-element modeling: Theory, experiments, applications. Acta Mater. 2010, 58, 1152–1211. [Google Scholar] [CrossRef]
- Liu, M.; Tieu, A.K.; Lu, C.; Zhu, H.; Deng, G.A. Crystal plasticity study of the effect of friction on the evolution of texture and mechanical behaviour in the nano-indentation of an aluminium single crystal. Comput. Mater. Sci. 2014, 81, 30–38. [Google Scholar] [CrossRef]
- Manchiraju, S.; Anderson, P.M. Coupling between martensitic phase transformations and plasticity: A microstructure-based finite element model. Int. J. Plast. 2010, 26, 1508–1526. [Google Scholar] [CrossRef]
- Abdolvand, H.; Daymond, M.R.; Mareau, C. Incorporation of twinning into a crystal plasticity finite element model: Evolution of lattice strains and texture in Zircaloy-2. Int. J. Plast. 2011, 27, 1721–1738. [Google Scholar] [CrossRef]
- Sheikh, H.; Ebrahimi, R.; Bagherpour, E. Crystal plasticity finite element modeling of crystallographic textures in simple shear extrusion (SSE) process. Mater. Des. 2016, 109, 289–299. [Google Scholar] [CrossRef]
- Hibbitt, H.; Karlsson, B.; Sorensen, P. Abaqus. Analysis User’s Manual Version 6.10; Dassault Systèmes Simulia Corp.: Providence, RI, USA, 2011. [Google Scholar]
- Quey, R.; Dawson, P.R.; Barbe, F. Large-scale 3D random polycrystals for the finite element method: Generation, meshing and remeshing. Comput. Meth. Appl. Mech. Eng. 2011, 200, 1729–1745. [Google Scholar] [CrossRef]
- Hill, R.; Rice, J.R. Constitutive analysis of elastic plastic crystals at arbitrary strain. J. Mech. Phys. Solids 1972, 20, 401–413. [Google Scholar] [CrossRef]
- Huang, Y.G. A User-Material Subroutine Incroporating Single Crystal Plasticity in the Abaqus Finite Element Program; Harvard University: Cambridge, MA, USA, 1991. [Google Scholar]
- Jiang, S.Y.; Zhang, Y.Q.; Zhao, Y.N.; Tang, M.; Yi, W.L. Constitutive behavior of Ni-Ti shape memory alloy under hot compression. J. Cent. South Univ. 2013, 20, 24–29. [Google Scholar] [CrossRef]
- Cailletaud, G. A micromechanical approach to inelastic behaviour of metals. Int. J. Plast. 1992, 8, 55–73. [Google Scholar] [CrossRef]
- Li, L.T.; Lin, Y.C.; Li, L.; Shen, L.M.; Wen, D.X. Three-Dimensional Crystal Plasticity Finite Element Simulation of Hot Compressive Deformation Behaviors of 7075 Al Alloy. J. Mater. Eng. Perform. 2015, 24, 1294–1304. [Google Scholar] [CrossRef]
- Lan, Y.J.; Xiao, N.M.; Li, D.Z.; Li, Y.Y. Mesoscale simulation of deformed austenite decomposition into ferrite by coupling a cellular automaton method with a crystal plasticity finite element model. Acta Mater. 2005, 53, 991–1003. [Google Scholar] [CrossRef]

| q | n | |||||||
|---|---|---|---|---|---|---|---|---|
| 130 GPa | 98 GPa | 34 GPa | 1200 MPa | 322 MPa | 160 MPa | 0.001 s−1 | 1.4 | 20 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, L.; Jiang, S.; Zhou, T.; Tu, J.; Shi, L.; Chen, Q.; Yang, M. Multiscale Modeling of Polycrystalline NiTi Shape Memory Alloy under Various Plastic Deformation Conditions by Coupling Microstructure Evolution and Macroscopic Mechanical Response. Materials 2017, 10, 1172. https://doi.org/10.3390/ma10101172
Hu L, Jiang S, Zhou T, Tu J, Shi L, Chen Q, Yang M. Multiscale Modeling of Polycrystalline NiTi Shape Memory Alloy under Various Plastic Deformation Conditions by Coupling Microstructure Evolution and Macroscopic Mechanical Response. Materials. 2017; 10(10):1172. https://doi.org/10.3390/ma10101172
Chicago/Turabian StyleHu, Li, Shuyong Jiang, Tao Zhou, Jian Tu, Laixin Shi, Qiang Chen, and Mingbo Yang. 2017. "Multiscale Modeling of Polycrystalline NiTi Shape Memory Alloy under Various Plastic Deformation Conditions by Coupling Microstructure Evolution and Macroscopic Mechanical Response" Materials 10, no. 10: 1172. https://doi.org/10.3390/ma10101172
APA StyleHu, L., Jiang, S., Zhou, T., Tu, J., Shi, L., Chen, Q., & Yang, M. (2017). Multiscale Modeling of Polycrystalline NiTi Shape Memory Alloy under Various Plastic Deformation Conditions by Coupling Microstructure Evolution and Macroscopic Mechanical Response. Materials, 10(10), 1172. https://doi.org/10.3390/ma10101172





