Comprehensive Noise Modeling of Piezoelectric Charge Accelerometer with Signal Conditioning Circuit
Abstract
1. Introduction
2. Methodology
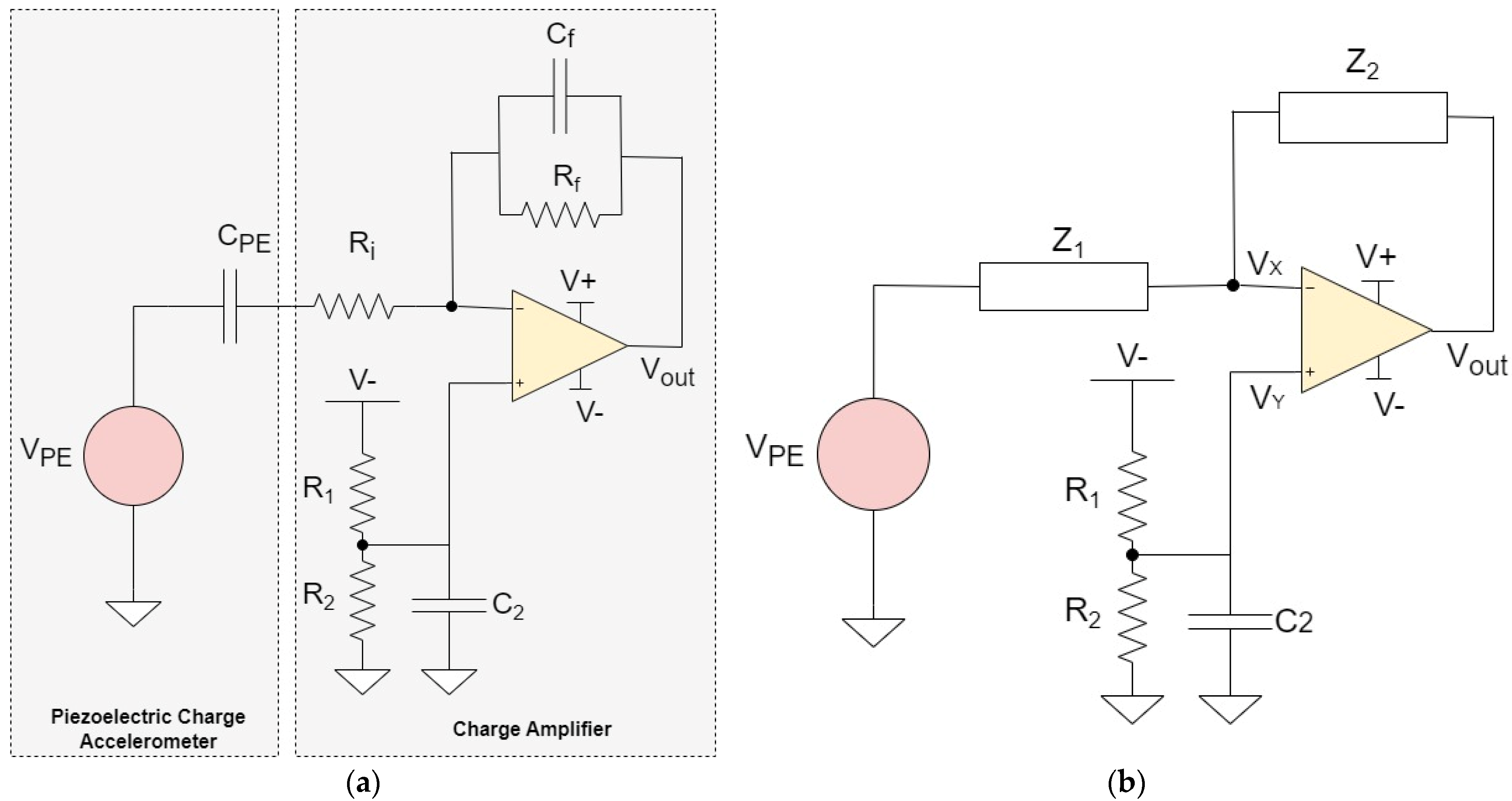
2.1. Circuit Modeling
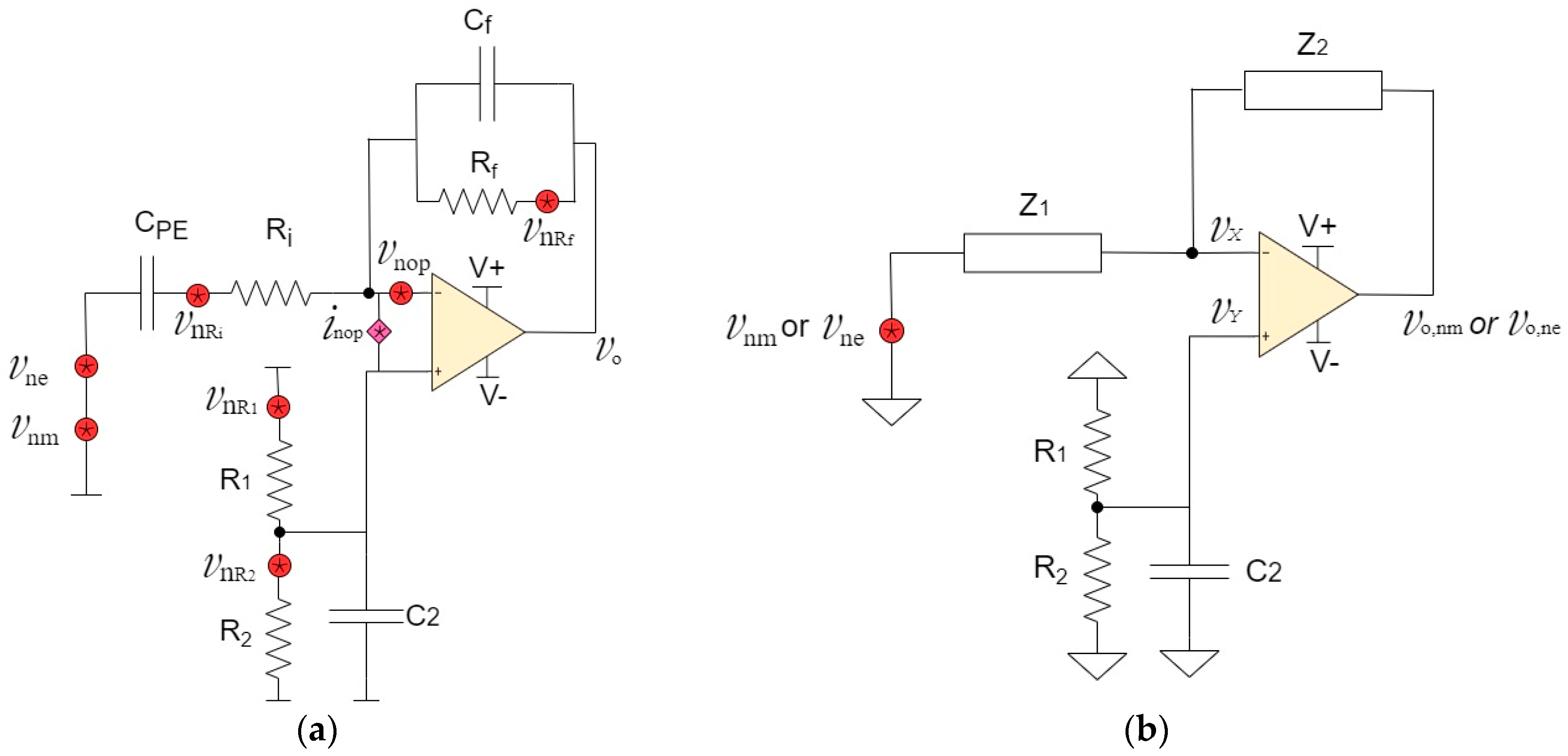
2.2. Noise Modeling
2.2.1. Intrinsic Noise Sources from Accelerometer ( and )
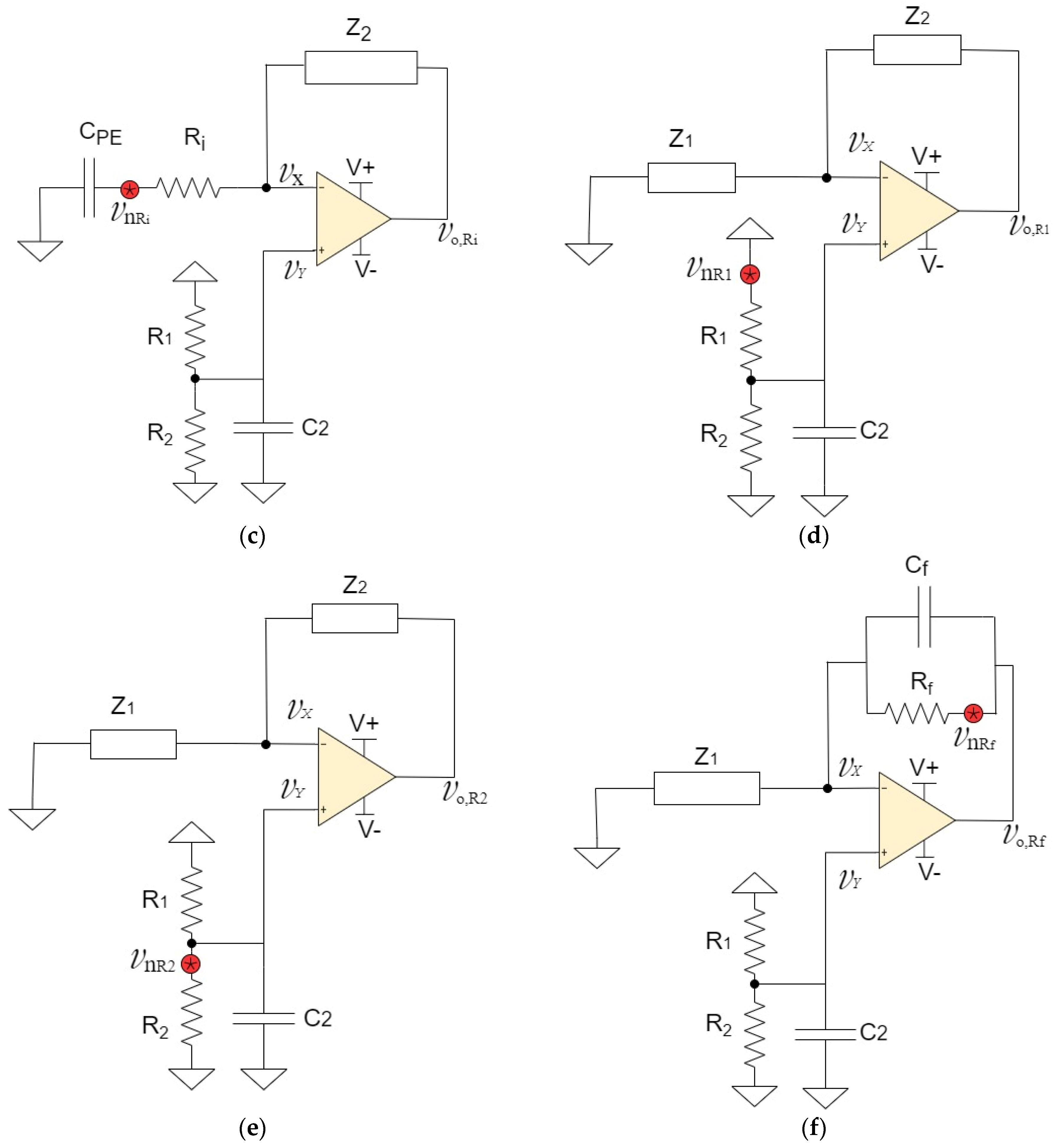
2.2.2. Noise Sources Due to Resistors ()
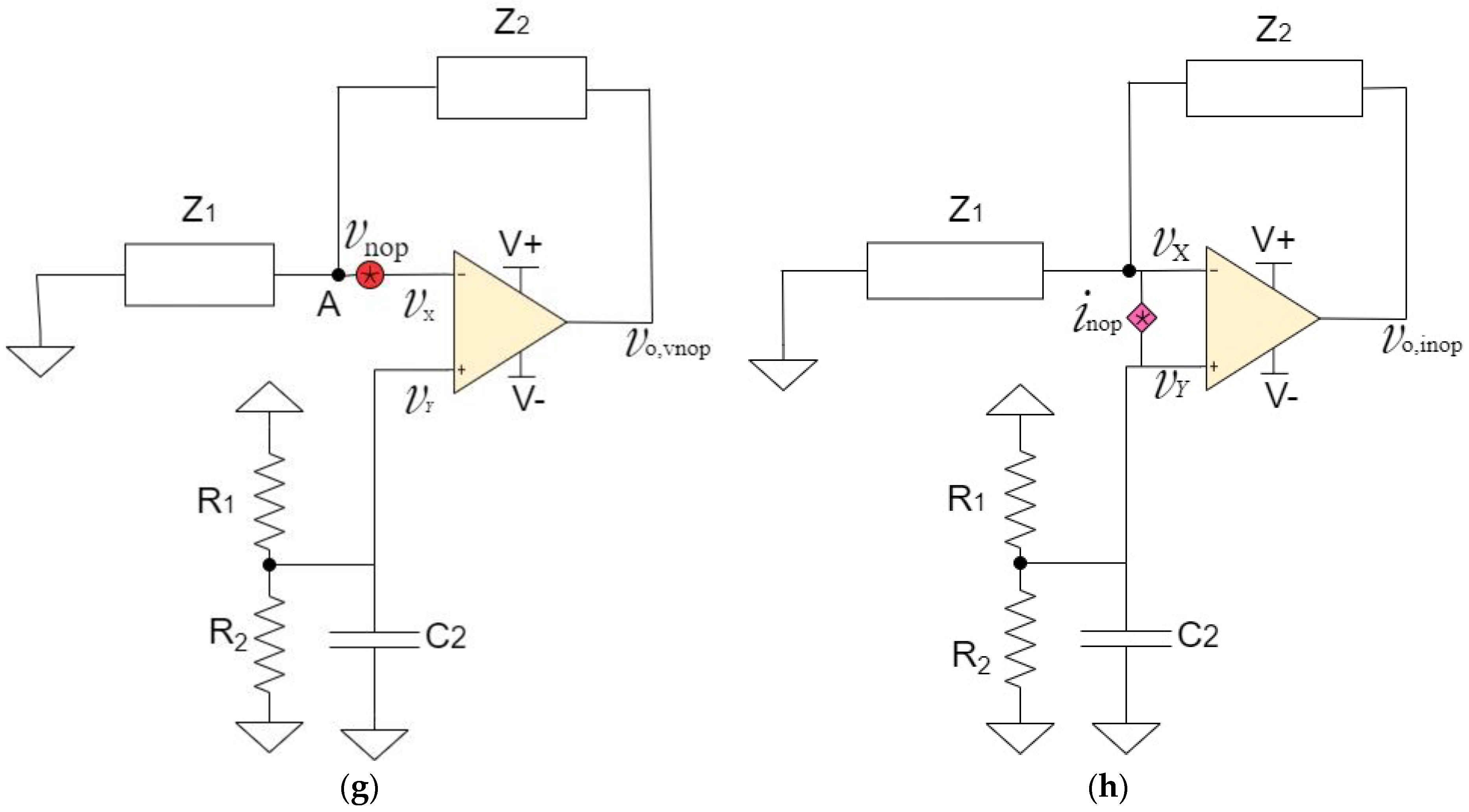
2.2.3. Noise Sources Due to the JFET Operational Amplifier ()
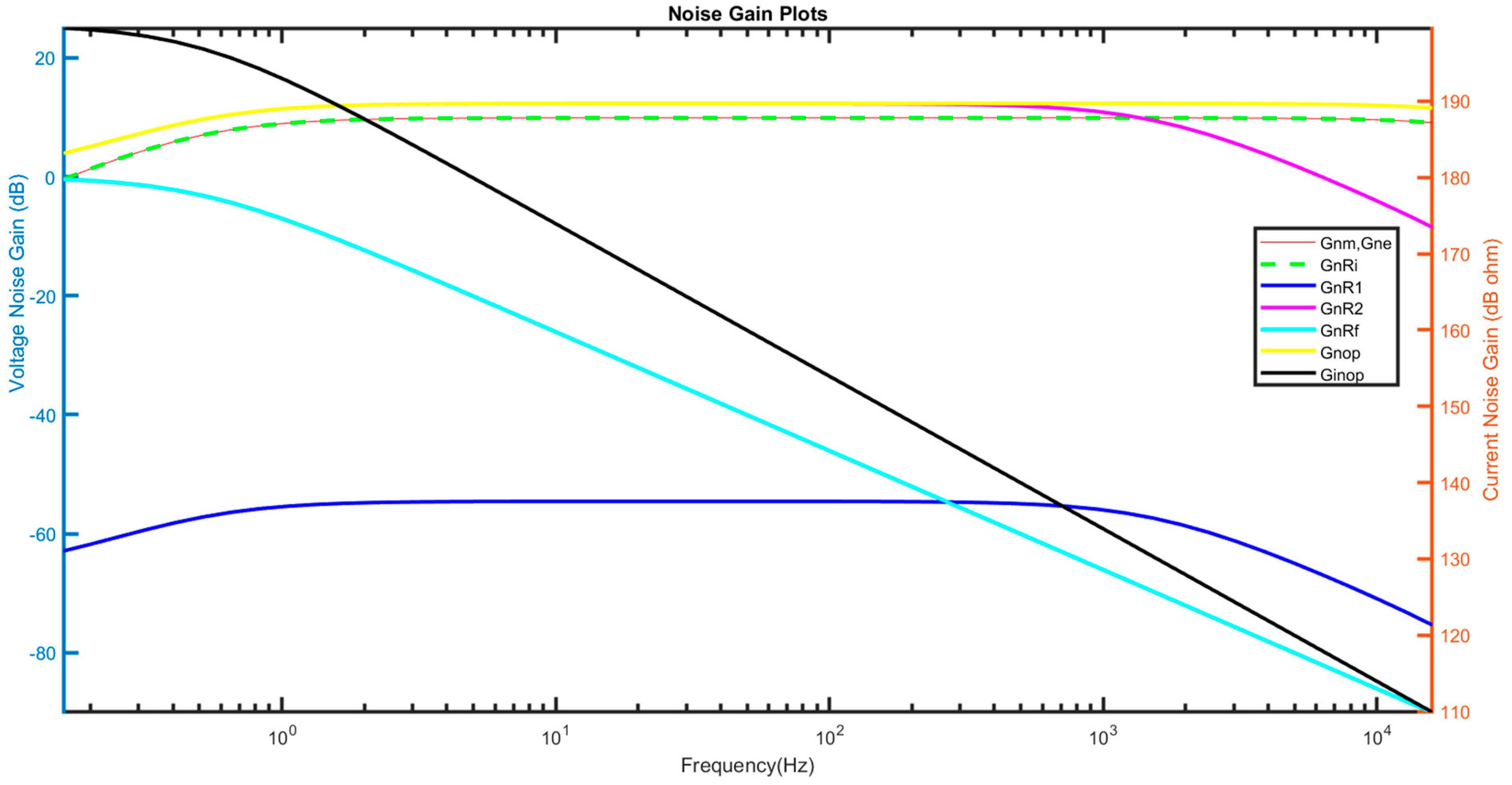
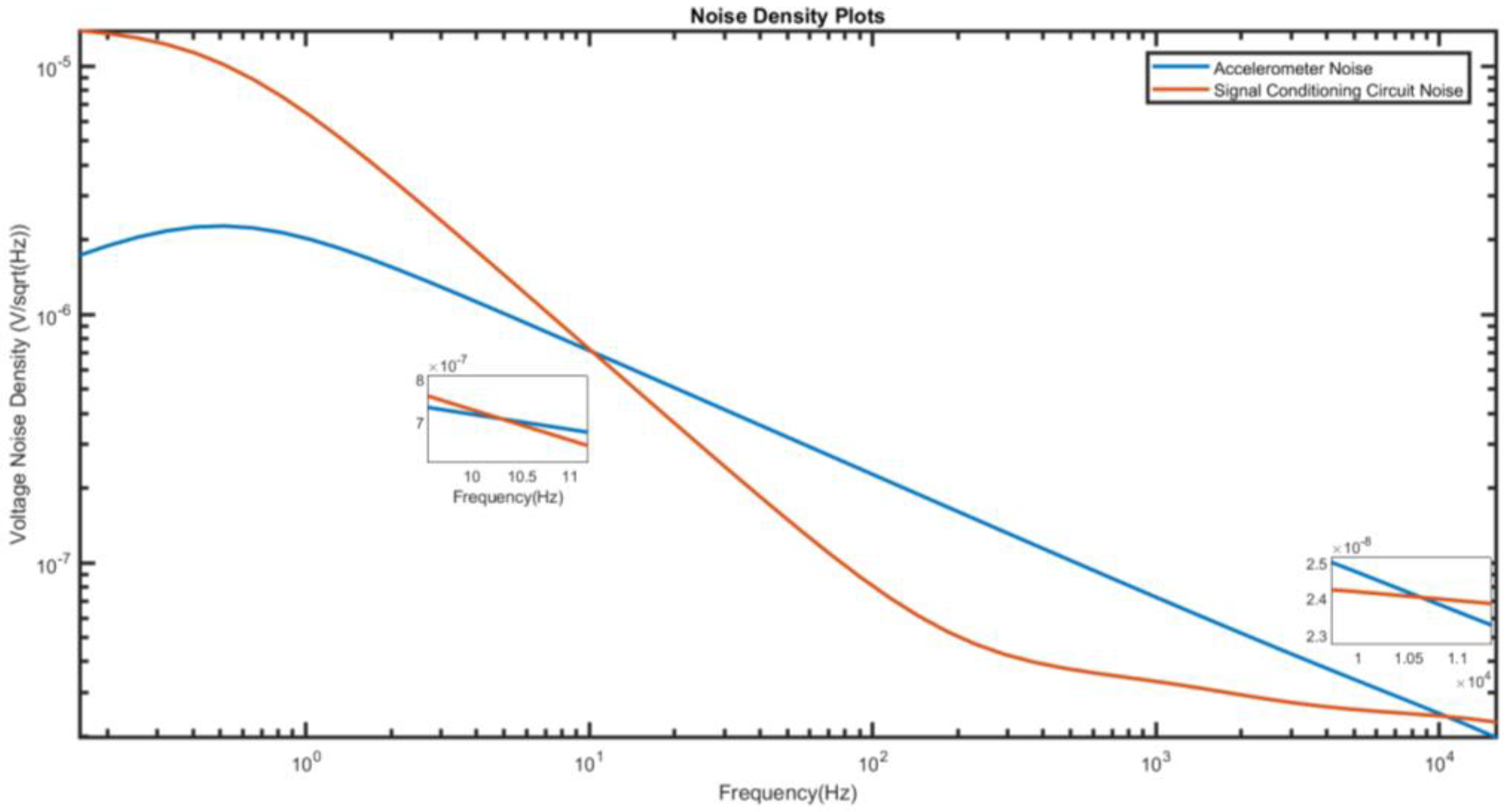
3. Results and Discussion
4. Practical Considerations
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Correction Statement
References
- Zhang, S.; Jiang, X.; Lapsley, M.; Moses, P.; Shrout, T.R. Piezoelectric accelerometers for ultrahigh temperature application. Appl. Phys. Lett. 2010, 96, 013506. [Google Scholar] [CrossRef]
- Han, R.-H.; Wang, J.-Y.; Xu, M.-H.; Guo, H. Design of a tri-axial micro piezoelectric accelerometer. In Proceedings of the 2016 Symposium on Piezoelectricity, Acoustic Waves, and Device Applications (SPAWDA), Xi’an, China, 21–24 October 2016; pp. 66–70. [Google Scholar]
- Elies, S. Performance analysis of commercial accelerometers: A parameter review. Sens. Transducers 2015, 193, 179. [Google Scholar]
- Wu, T.; You, D.; Gao, H.; Lian, P.; Ma, W.; Zhou, X.; Wang, C.; Luo, J.; Zhang, H.; Tan, H. Research Status and Development Trend of Piezoelectric Accelerometer. Crystals 2023, 13, 1363. [Google Scholar] [CrossRef]
- Yu, J.-C.; Lan, C.-B. System modeling of microaccelerometer using piezoelectric thin films. Sens. Actuators A Phys. 2001, 88, 178–186. [Google Scholar] [CrossRef]
- Chen, Z.-H.; Li, C.-Y.; Chu, S.-Y.; Tsai, C.-C.; Wang, Y.-H.; Kao, H.-Y.; Wei, C.-L.; Huang, Y.-H.; Hsiao, P.-Y.; Liu, Y.-H. The design of aluminum nitride-based lead-free piezoelectric MEMS accelerometer system. IEEE Trans. Electron Devices 2020, 67, 4399–4404. [Google Scholar] [CrossRef]
- Hindrichsen, C.C.; Larsen, J.; Thomsen, E.; Hansen, K.; Lou-Møller, R. Circular piezoelectric accelerometer for high band width application. In Proceedings of the IEEE SENSORS, Christchurch, New Zealand, 25–28 October 2009; pp. 475–478. [Google Scholar]
- Zhou, H.; Han, R.-H.; Xu, M.-H.; Guo, H. Study of a piezoelectric accelerometer based on d33 mode. In Proceedings of the 2016 Symposium on Piezoelectricity, Acoustic Waves, and Device Applications (SPAWDA), Xi’an, China, 21–24 October 2016; pp. 61–65. [Google Scholar]
- Zhou, H.; Xu, M.-H.; Peng, H.; Zhu, L.-H.; Zeng, Y.-B.; Guo, H. On the measuring circuit of piezoelectric accelerometers. In Proceedings of the 2017 Symposium on Piezoelectricity, Acoustic Waves, and Device Applications (SPAWDA), Chengdu, China, 27–30 October 2017; pp. 524–527. [Google Scholar]
- Durdaut, P.; Penner, V.; Kirchhof, C.; Quandt, E.; Knöchel, R.; Höft, M. Noise of a JFET charge amplifier for piezoelectric sensors. IEEE Sens. J. 2017, 17, 7364–7371. [Google Scholar] [CrossRef]
- Levinzon, F.A. Ultra-low-noise high-input impedance amplifier for low-frequency measurement applications. IEEE Trans. Circuits Syst. I Regul. Pap. 2008, 55, 1815–1822. [Google Scholar] [CrossRef]
- Sackinger, E. On the noise optimum of FET broadband transimpedance amplifiers. IEEE Trans. Circuits Syst. I Regul. Pap. 2012, 59, 2881–2889. [Google Scholar] [CrossRef]
- Abidi, A.A. On the noise optimum of gigahertz FET transimpedance amplifiers. IEEE J. Solid-State Circuits 1987, 22, 1207–1209. [Google Scholar] [CrossRef]
- Lin, T.-Y.; Green, R.J.; O’Connor, P.B. A gain and bandwidth enhanced transimpedance preamplifier for Fourier-transform ion cyclotron resonance mass spectrometry. Rev. Sci. Instrum. 2011, 82, 124101. [Google Scholar] [CrossRef] [PubMed]
- Fonck, R.; Ashley, R.; Durst, R.; Paul, S.; Renda, G. Low-noise photodiode detector for optical fluctuation diagnostics. Rev. Sci. Instrum. 1992, 63, 4924–4926. [Google Scholar] [CrossRef]
- Levinzon, F. Piezoelectric Accelerometers with Integral Electronics; Springer: Berlin/Heidelberg, Germany, 2015. [Google Scholar]
- Walter, P.L. The Handbook of Dynamic Force, Pressure and Acceleration Measurement; Meggitt, Endevco: San Juan Capistrano, CA, USA, 2001. [Google Scholar]
- Linear Technology. Data Sheet LT1169; Linear Technology: Milpitas, CA, USA, 1994. [Google Scholar]
- Levinzon, F.A. Fundamental noise limit of piezoelectric accelerometer. IEEE Sens. J. 2004, 4, 108–111. [Google Scholar] [CrossRef]
- Mohd-Yasin, F.; Korman, C.E.; Nagel, D.J. Measurement of noise characteristics of MEMS accelerometers. Solid-State Electron. 2003, 47, 357–360. [Google Scholar] [CrossRef]
- Ali, G.; Mohd-Yasin, F. A Simulink Model of Noise of Piezoelectric Charge Amplifier. In Proceedings of the 2023 IEEE Regional Symposium on Micro and Nanoelectronics, Langkawi, Malaysia, 28–29 August 2023; pp. 13–16. [Google Scholar]
- Yasin, F.M.; Tye, K.F.; Reaz, M.B.I. Design and implementation of interface circuitry for CMOS-based SAW gas sensors. In Proceedings of the 2005 IEEE International SOC Conference, Herndon, VA, USA, 25–28 September 2005; pp. 161–164. [Google Scholar]


| Parameter | Value |
|---|---|
| Resonant frequency ‘fo’ | 30 KHz |
| Mass ‘M’ | 3 × 10−4 Kg |
| Quality factor ‘Q’ | 70 |
| Charge Sensitivity ‘QPE’ | 1 pC/g |
| Capacitance ‘CPE’ | 100 pF |
| Dissipation factor ‘η’ | 0.02 |
| Temperature ‘T’ | 300 K |
| Operating frequency | 0.5 Hz to 10 KHz |
| Parameter | Value |
|---|---|
| Ri | 45 KΩ |
| R1 | 2.2 KΩ |
| R2 | 1 Ω |
| Cf | 32 pF |
| Rf | 10 GΩ |
| C2 | 100 uF |
| V+ | 5 V |
| V- | −5 V |
| Aop | 1.2 × 106 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ali, G.; Mohd-Yasin, F. Comprehensive Noise Modeling of Piezoelectric Charge Accelerometer with Signal Conditioning Circuit. Micromachines 2024, 15, 283. https://doi.org/10.3390/mi15020283
Ali G, Mohd-Yasin F. Comprehensive Noise Modeling of Piezoelectric Charge Accelerometer with Signal Conditioning Circuit. Micromachines. 2024; 15(2):283. https://doi.org/10.3390/mi15020283
Chicago/Turabian StyleAli, Ghulam, and Faisal Mohd-Yasin. 2024. "Comprehensive Noise Modeling of Piezoelectric Charge Accelerometer with Signal Conditioning Circuit" Micromachines 15, no. 2: 283. https://doi.org/10.3390/mi15020283
APA StyleAli, G., & Mohd-Yasin, F. (2024). Comprehensive Noise Modeling of Piezoelectric Charge Accelerometer with Signal Conditioning Circuit. Micromachines, 15(2), 283. https://doi.org/10.3390/mi15020283






