Numerical Simulation of the Influence of Non-Uniform ζ Potential on Interfacial Flow
Abstract
:1. Introduction
2. Theory
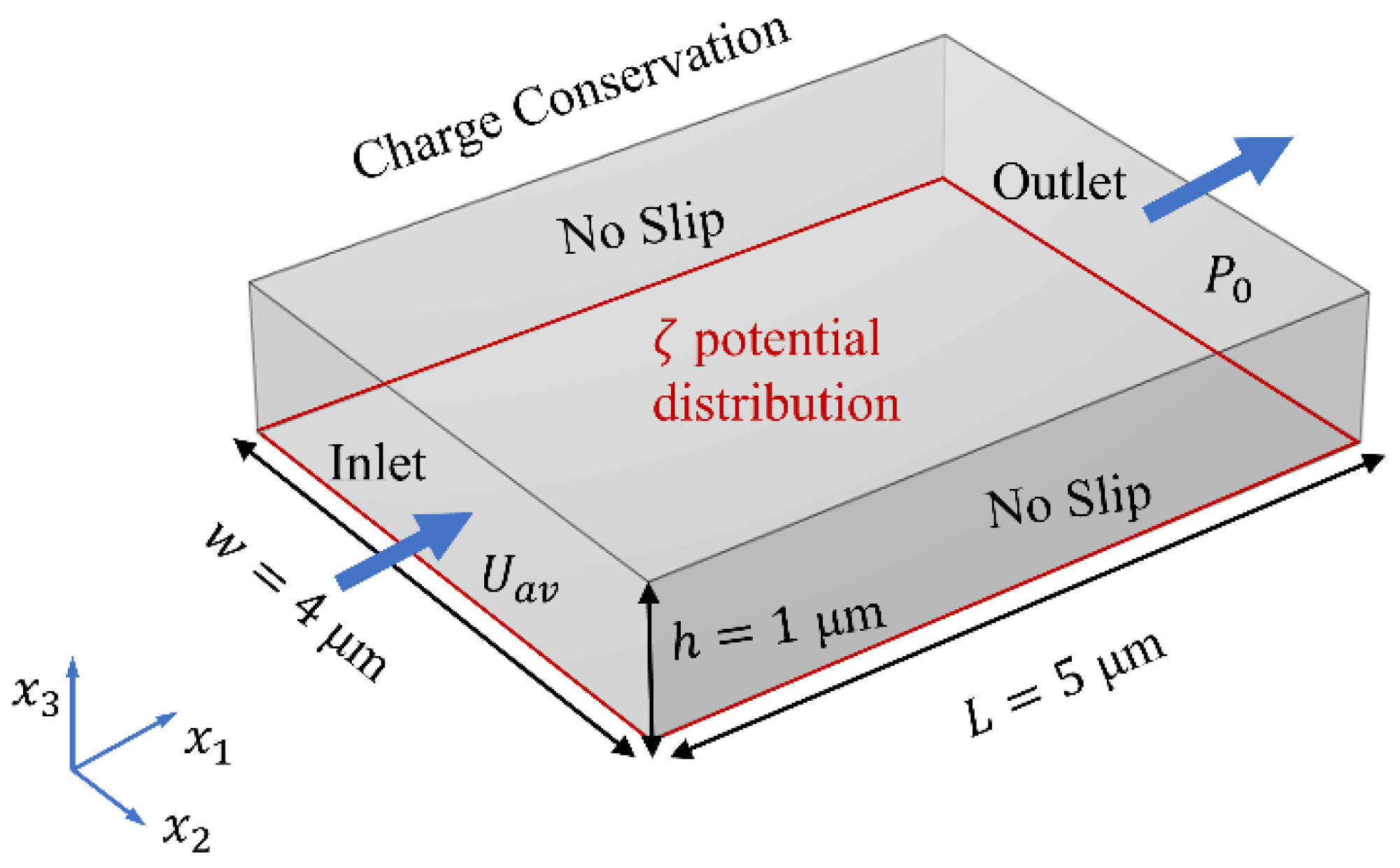
3. Numerical Simulation
3.1. Pressure-Driven Laminar Flow
3.2. Electrostatics Module
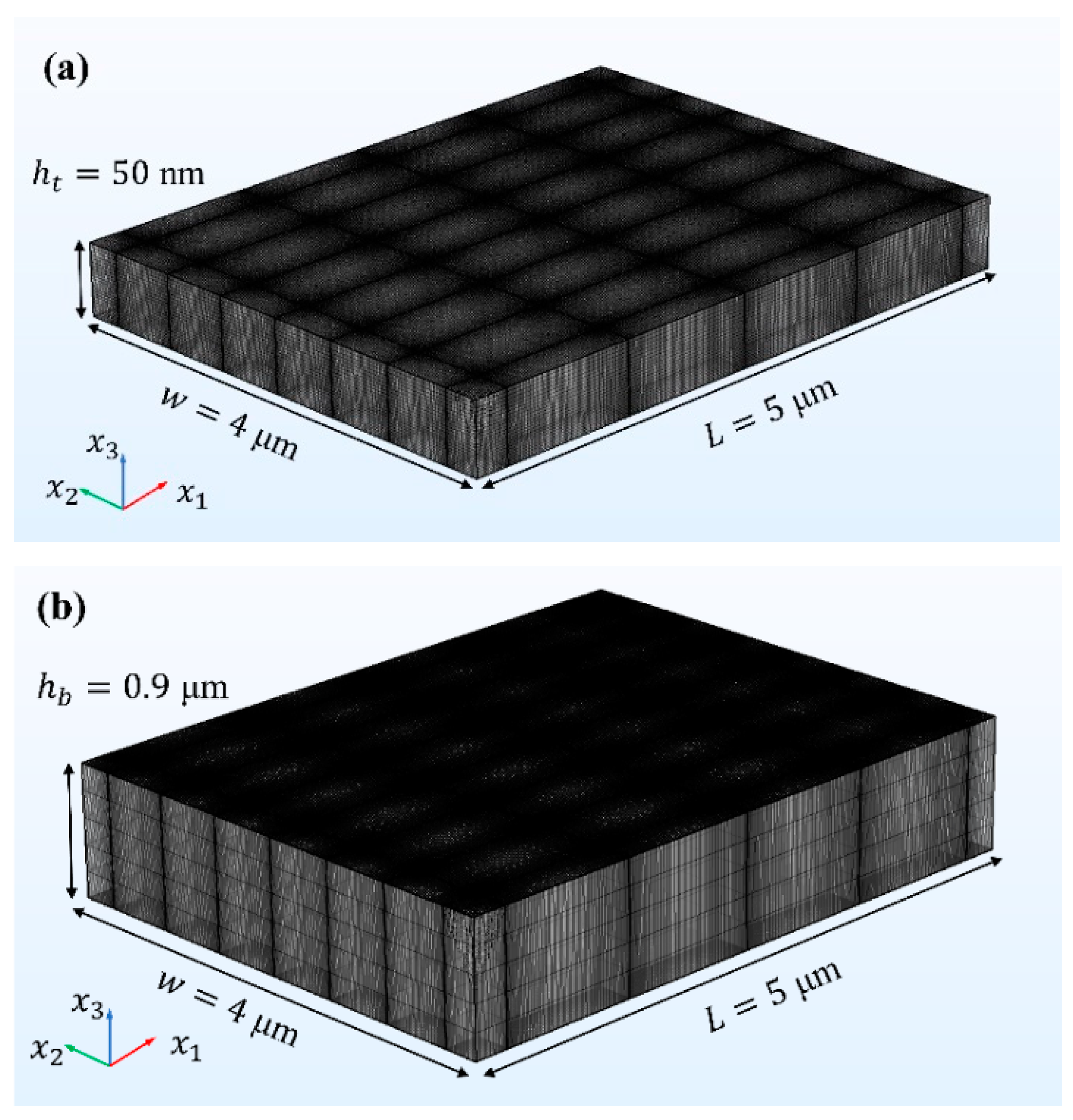
3.3. Modeling and Meshing
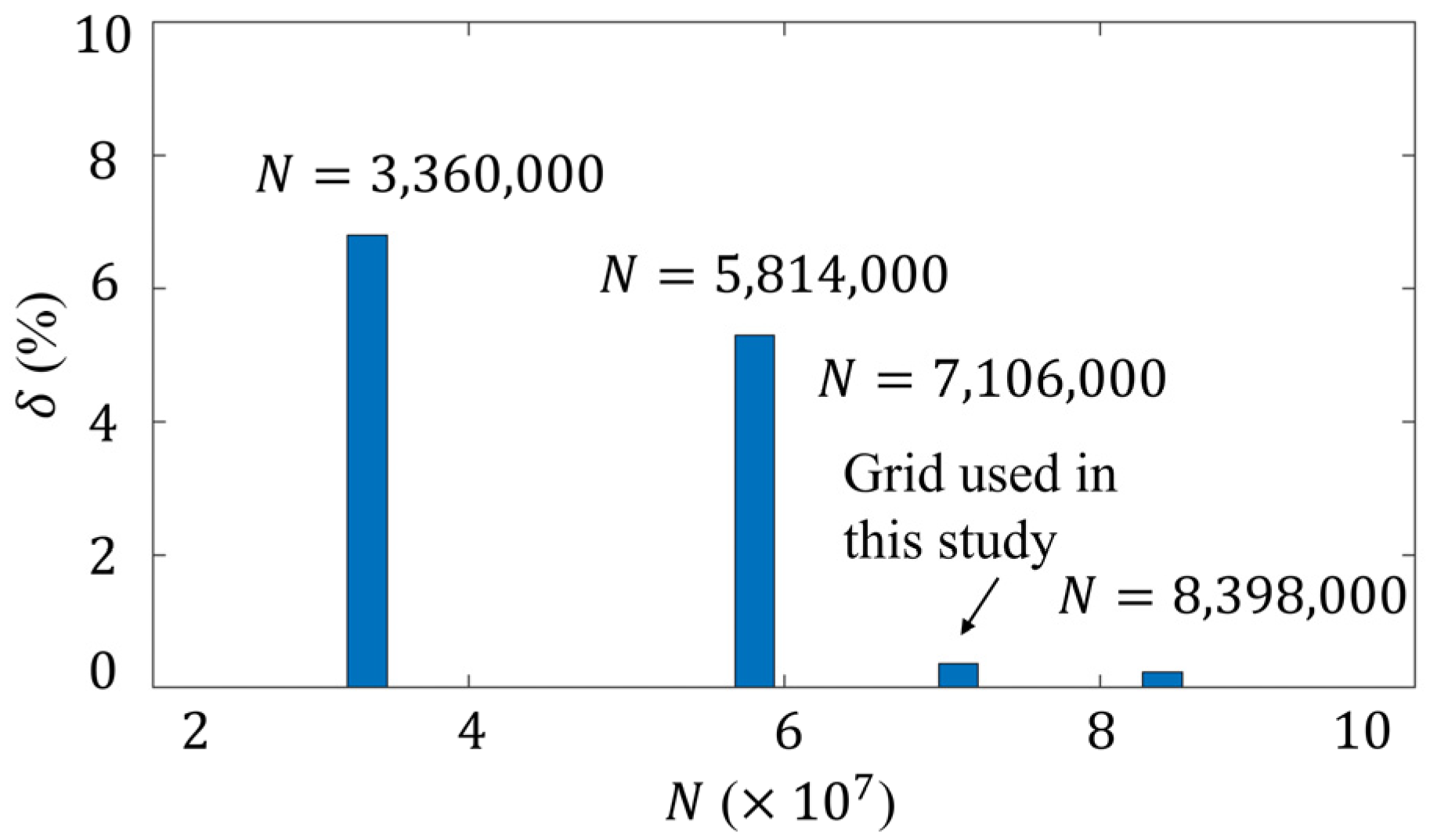
3.4. Grid Independence Analysis
4. Results
4.1. Pressure-Driven Basic Flow
4.2. Uniform ζ Distribution
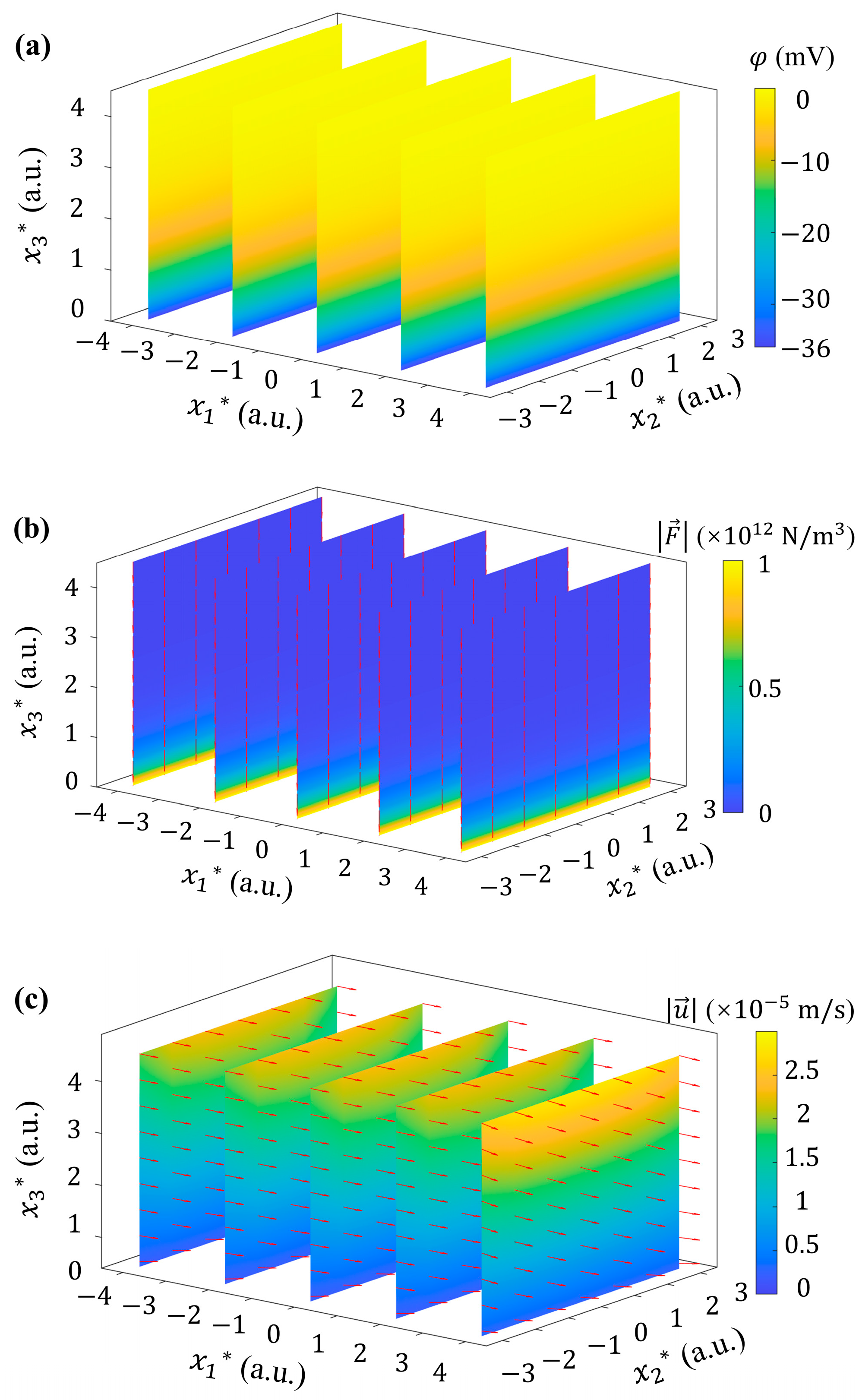
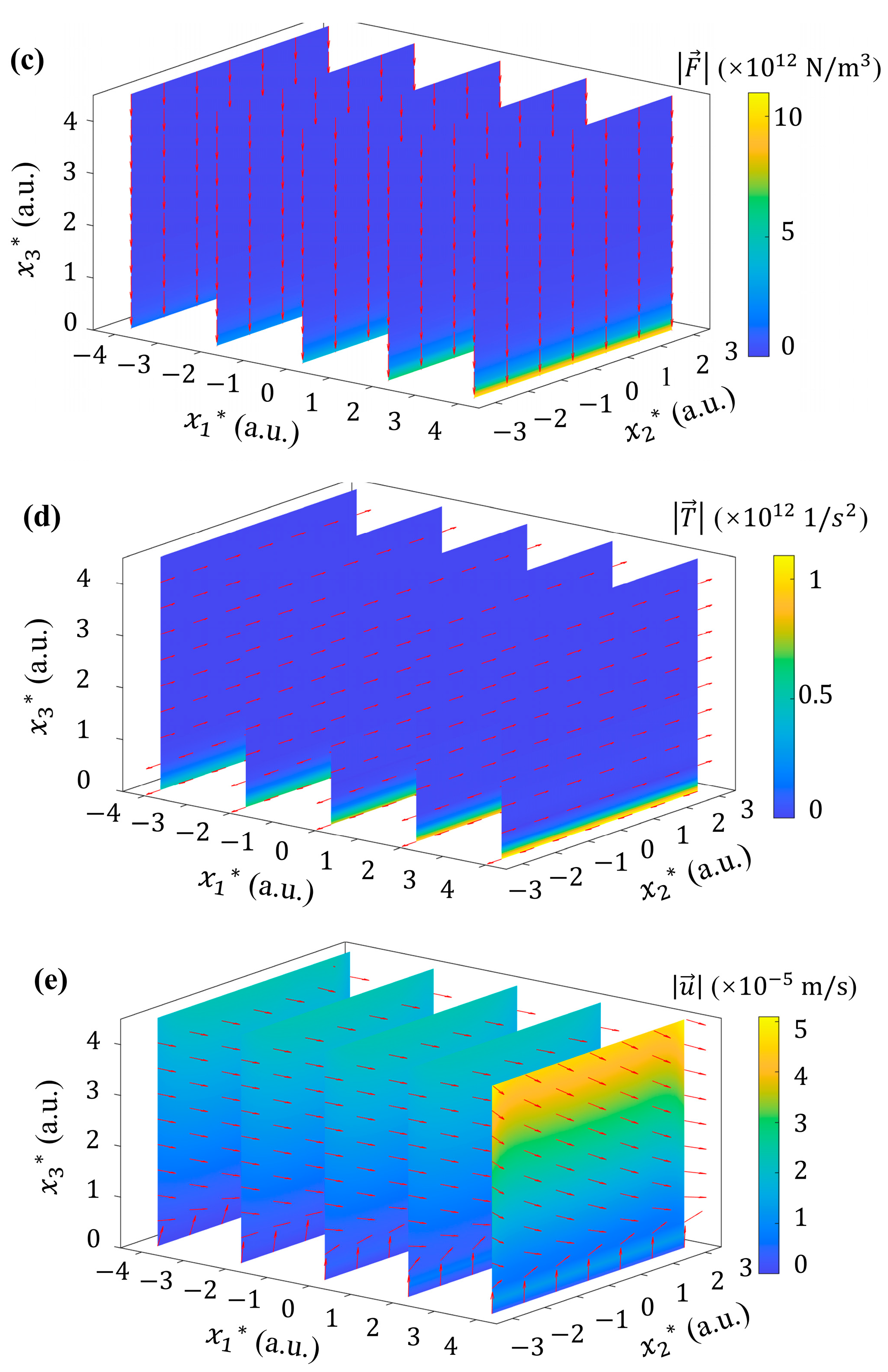
4.3. One-Dimensional ζ Distribution
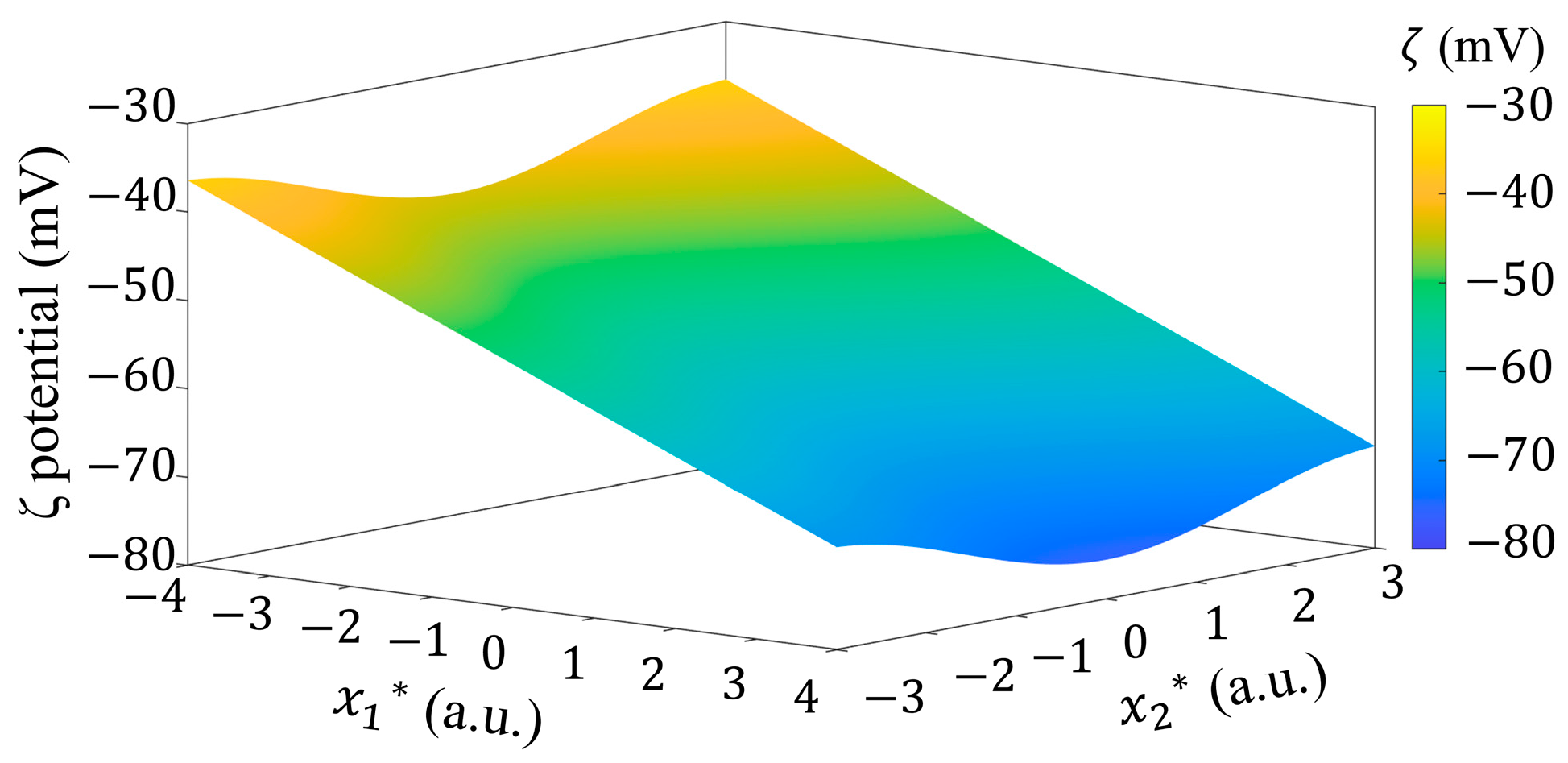
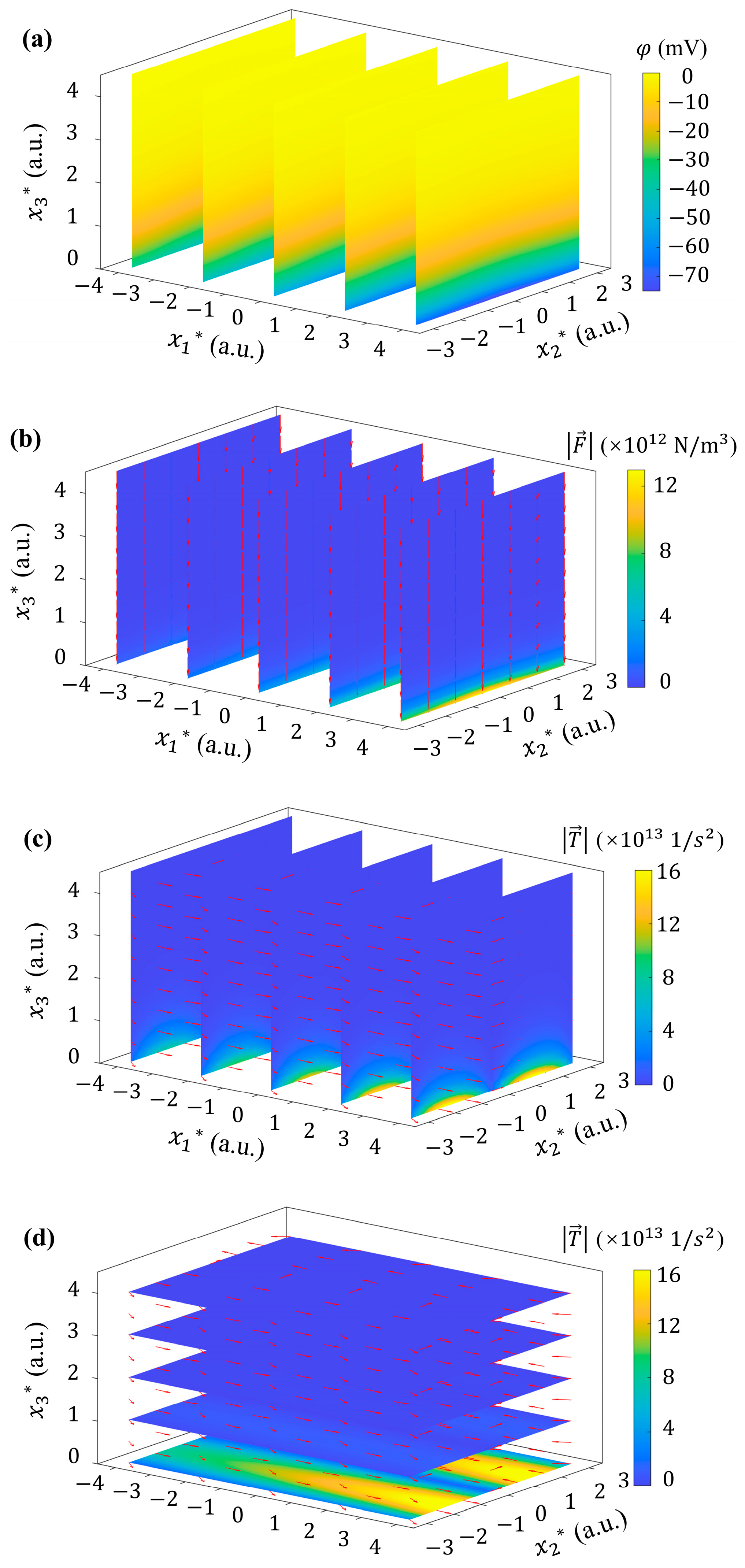
4.4. Two-Dimensional ζ Distribution
5. Discussion
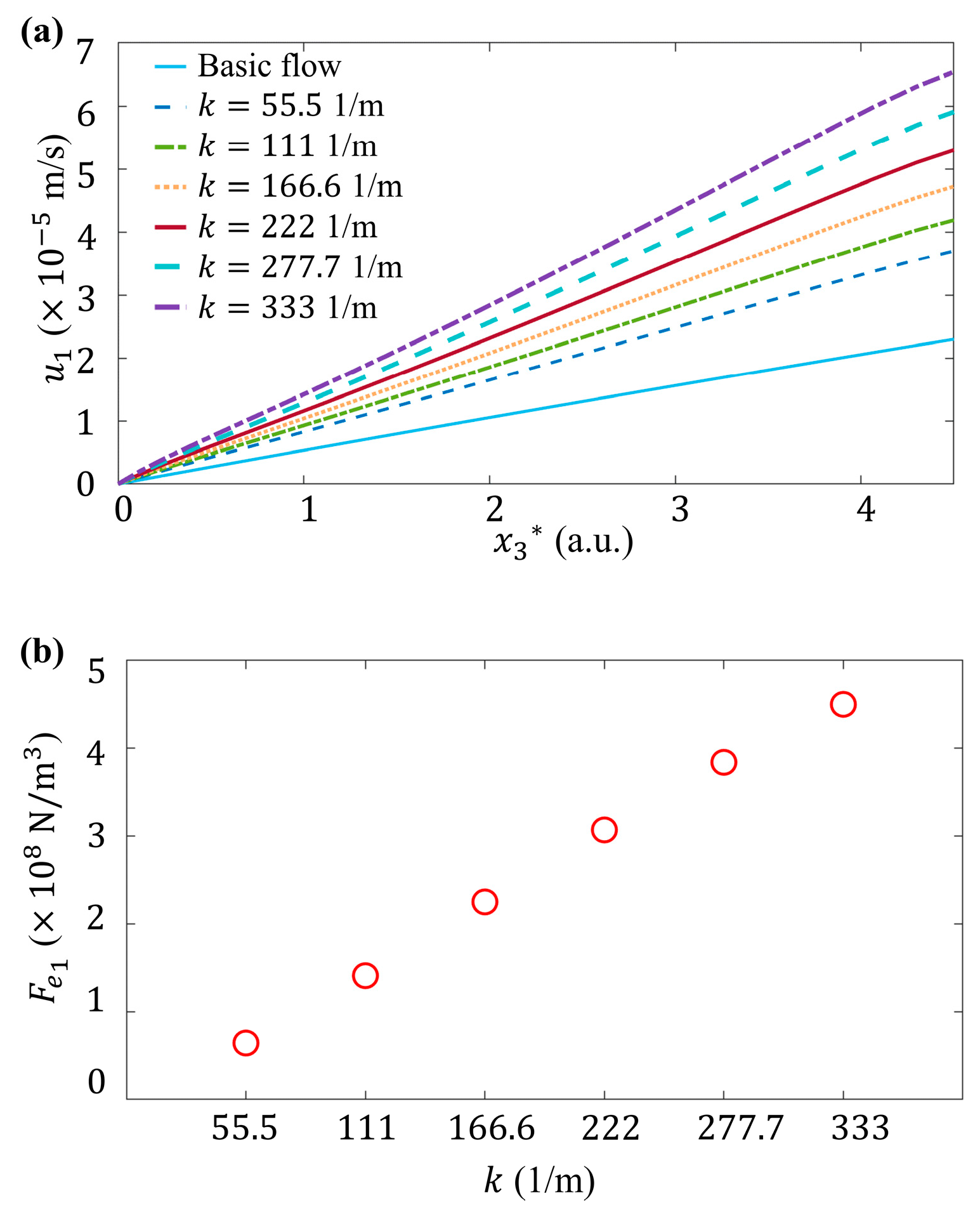
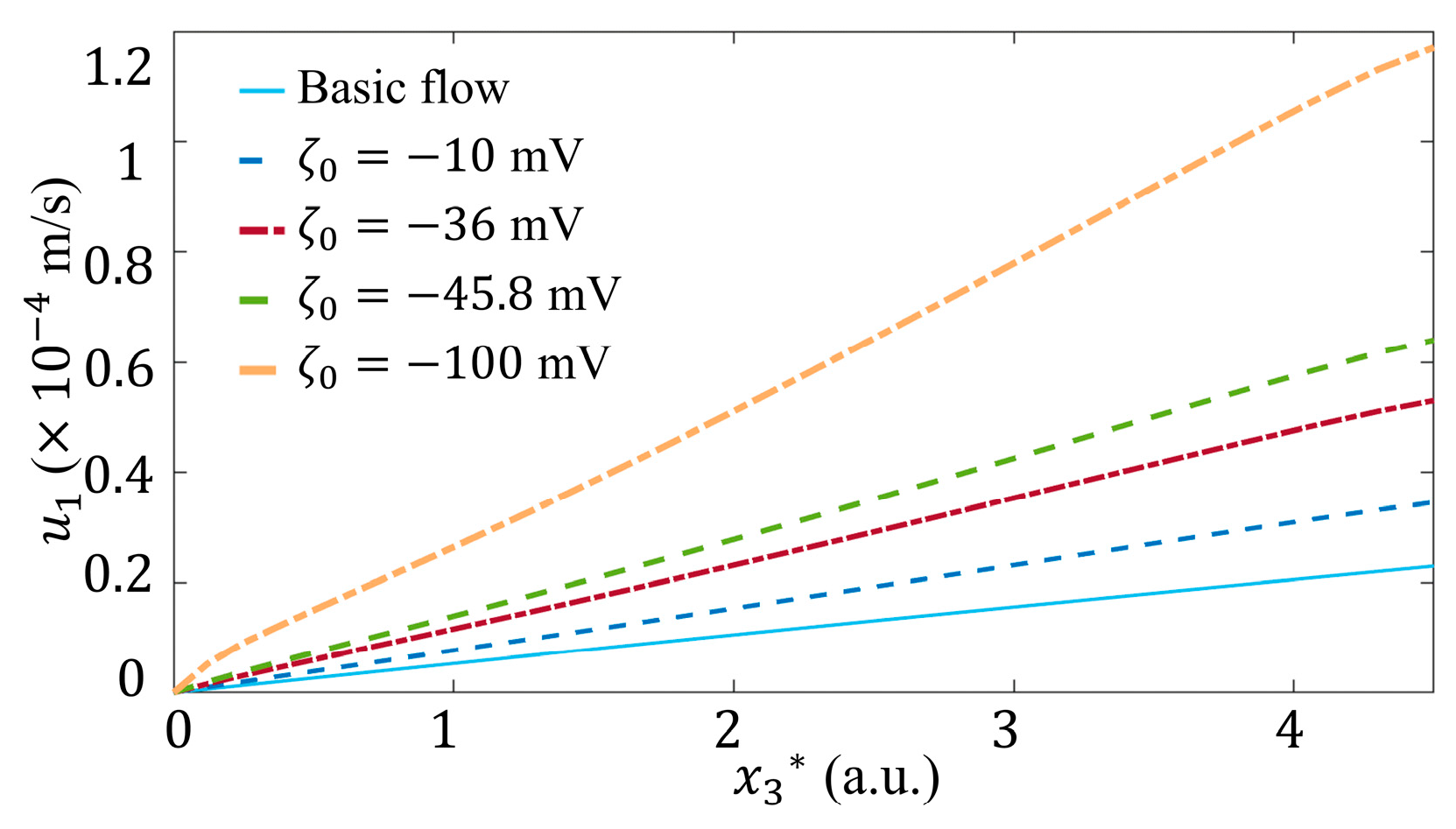
5.1. Influence of
5.2. Influence of
5.3. Influence of
5.4. Influence of
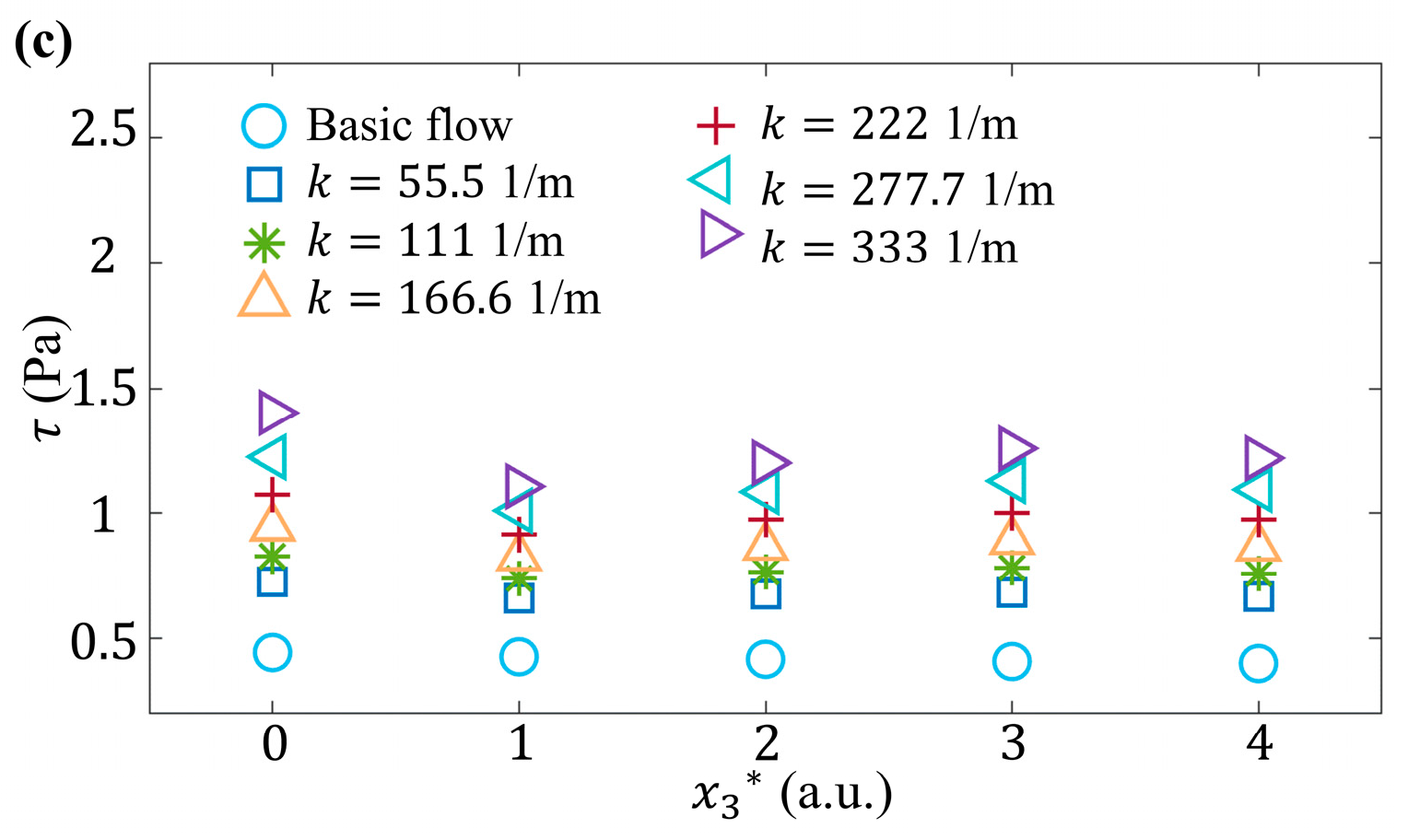
5.5. Influence of Other Parameters
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Toney, M.F.; Howard, J.N.; Richer, J.; Borges, G.L.; Gordon, J.G.; Melroy, O.R.; Wiesler, D.G.; Yee, D.; Sorensen, L.B. Voltage-dependent ordering of water molecules at an electrode–electrolyte interface. Nature 1994, 368, 444–446. [Google Scholar] [CrossRef]
- Bazant, M.Z.; Squires, T.M. Induced-Charge Electrokinetic Phenomena: Theory and Microfluidic Applications. Phys. Rev. Lett. 2004, 92, 066101. [Google Scholar] [CrossRef]
- Davidson, S.M.; Andersen, M.B.; Mani, A. Chaotic Induced-Charge Electro-Osmosis. Phys. Rev. Lett. 2014, 112, 128302. [Google Scholar] [CrossRef]
- Fernández-Mateo, R.; Calero, V.; Morgan, H.; Ramos, A.; García-Sánchez, P. Concentration–Polarization Electroosmosis near Insulating Constrictions within Microfluidic Channels. Anal. Chem. 2021, 93, 14667–14674. [Google Scholar] [CrossRef]
- Sparreboom, W.; Berg, A.v.d.; Eijkel, J.C.T. Principles and applications of nanofluidic transport. Nat. Nanotechnol. 2009, 4, 713–720. [Google Scholar] [CrossRef]
- Whitesides, G.M. The origins and the future of microfluidics. Nature 2006, 442, 368–373. [Google Scholar] [CrossRef] [PubMed]
- Wu, Y.; Ma, X.; Li, K.; Yue, Y.; Zhang, Z.; Meng, Y.; Wang, S. Bipolar Electrode-based Sheath-Less Focusing and Continuous Acoustic Sorting of Particles and Cells in an Integrated Microfluidic Device. Anal. Chem. 2024, 96, 3627–3635. [Google Scholar] [CrossRef] [PubMed]
- Wu, Y.; Zhang, H.; Yue, Y.; Wang, S.; Meng, Y. Controllable and Scaffold-Free Formation of 3D Multicellular Architectures Using a Bipolar Electrode Array. Adv. Mater. Technol. 2024, 9, 2301626. [Google Scholar] [CrossRef]
- Escosura-Muñiz, A.d.l.; Ambrosi, A.; Merkoçi, A. Electrochemical analysis with nanoparticle-based biosystems. TrAC Trends Anal. Chem. 2008, 27, 568–584. [Google Scholar] [CrossRef]
- Karden, E.; Ploumen, S.; Fricke, B.; Miller, T.; Snyder, K. Energy storage devices for future hybrid electric vehicles. J. Power Sources 2007, 168, 2–11. [Google Scholar] [CrossRef]
- Hu, Y.; Yang, J.; Hu, J.; Wang, J.; Liang, S.; Hou, H.; Wu, X.; Liu, B.; Yu, W.; He, X.; et al. Synthesis of Nanostructured PbO@C Composite Derived from Spent Lead-Acid Battery for Next-Generation Lead-Carbon Battery. Adv. Funct. Mater. 2018, 28, 1705294. [Google Scholar] [CrossRef]
- Cui, P.; Wang, S. Application of microfluidic chip technology in pharmaceutical analysis: A review. J. Pharm. Anal. 2019, 9, 238–247. [Google Scholar] [CrossRef]
- Wu, Y.; Gu, Q.; Wang, Z.; Tian, Z.; Wang, Z.; Liu, W.; Han, J.; Liu, S. Electrochemiluminescence Analysis of Multiple Glycans on Single Living Cell with a Closed Bipolar Electrode Array Chip. Anal. Chem. 2024, 96, 2165–2172. [Google Scholar] [CrossRef]
- Tiflidis, C.; Westerbeek, E.Y.; Jorissen, K.F.A.; Olthuis, W.; Eijkel, J.C.T.; Malsche, W.D. Inducing AC-electroosmotic flow using electric field manipulation with insulators. Lab Chip 2021, 21, 3105–3111. [Google Scholar] [CrossRef]
- Pikal, M.J. The role of electroosmotic flow in transdermal iontophoresis. Adv. Drug Deliv. Rev. 2001, 46, 281–305. [Google Scholar] [CrossRef] [PubMed]
- Wu, M.; Gao, Y.; Ghaznavi, A.; Zhao, W.; Xu, J. AC electroosmosis micromixing on a lab-on-a-foil electric microfluidic device. Sens. Actuators B 2022, 359, 131611. [Google Scholar] [CrossRef]
- Kneller, A.R.; Haywood, D.G.; Jacobson, S.C. AC Electroosmotic Pumping in Nanofluidic Funnels. Anal. Chem. 2016, 88, 6390–6394. [Google Scholar] [CrossRef] [PubMed]
- Porada, S.; Weinstein, L.; Dash, R.; Wal, A.v.d.; Bryjak, M.; Gogotsi, Y.; Biesheuvel, P.M. Water Desalination Using Capacitive Deionization with Microporous Carbon Electrodes. ACS Appl. Mater. Interfaces 2012, 4, 1194–1199. [Google Scholar] [CrossRef]
- Wang, C.; Gao, Q. 3D Numerical Study of the Electrokinetic Motion of a Microparticle Adsorbed at a Horizontal Oil/Water Interface in an Infinite Domain. ACS Omega 2022, 7, 4062–4070. [Google Scholar] [CrossRef] [PubMed]
- Santiago, J.G. Electroosmotic Flows in Microchannels with Finite Inertial and Pressure Forces. Anal. Chem. 2001, 73, 2353–2365. [Google Scholar] [CrossRef]
- Schoch, R.B.; Lintel, H.v.; Renaud, P. Effect of the surface charge on ion transport through nanoslits. Phys. Fluids 2005, 17, 100604. [Google Scholar] [CrossRef]
- Brown, M.A.; Abbas, Z.; Kleibert, A.; Green, R.G.; Goel, A.; May, S.; Squires, T.M. Determination of Surface Potential and Electrical Double-Layer Structure at the Aqueous Electrolyte-Nanoparticle Interface. Phys. Rev. X 2016, 6, 011007. [Google Scholar] [CrossRef]
- Saha, P.; Zenyuk, I.V. Electrokinetic Streaming Current Method to Probe Polycrystalline Gold Electrode-Electrolyte Interface Under Applied Potentials. J. Electrochem. Soc. 2021, 168, 046511. [Google Scholar] [CrossRef]
- Putnis, C.V.; Ruiz-Agudo, E. The Mineral–Water Interface: Where Minerals React with the Environment. Elements 2013, 9, 177–182. [Google Scholar] [CrossRef]
- Schaefer, J.; Gonella, G.; Bonn, M.; Backus, E.H.G. Surface-specific vibrational spectroscopy of the water/silica interface: Screening and interference. Phys. Chem. Chem. Phys. 2017, 19, 16875–16880. [Google Scholar] [CrossRef]
- Zhang, S.; Chen, J.; Liu, J.; Pyles, H.; Baker, D.; Chen, C.-L.; Yoreo, J.J.D. Engineering Biomolecular Self-Assembly at Solid–Liquid Interfaces. Adv. Mater. 2021, 33, 1905784. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Chen, C.; Niu, Q.; Li, N.-W.; Yu, L.; Wang, B. Self-assembly of nanoparticles at solid–liquid interface for electrochemical capacitors. Rare Met. 2022, 41, 3591–3611. [Google Scholar] [CrossRef]
- Xue, J.; Zhao, W.; Nie, T.; Zhang, C.; Ma, S.; Wang, G.; Liu, S.; Li, J.; Gu, C.; Bai, J.; et al. Abnormal Rheological Phenomena in Newtonian Fluids in Electroosmotic Flows in a Nanocapillary. Langmuir 2018, 34, 15203–15210. [Google Scholar] [CrossRef] [PubMed]
- Zhao, W.; Liu, X.; Yang, F.; Wang, K.; Bai, J.; Qiao, R.; Wang, G. Study of Oscillating Electroosmotic Flows with High Temporal and Spatial Resolution. Anal. Chem. 2018, 90, 1652–1659. [Google Scholar] [CrossRef] [PubMed]
- Dutta, P.; Beskok, A. Analytical Solution of Time Periodic Electroosmotic Flows: Analogies to Stokes’ Second Problem. Anal. Chem. 2001, 73, 5097–5102. [Google Scholar] [CrossRef] [PubMed]
- Huang, G.; Xu, B.; Qiu, J.; Peng, L.; Luo, K.; Liu, D.; Han, P. Symmetric electrophoretic light scattering for determination of the zeta potential of colloidal systems. Colloids Surf. A 2020, 587, 124339–124345. [Google Scholar] [CrossRef]
- Dukhin, A.S.; Parlia, S. Studying homogeneity and zeta potential of membranes using electroacoustics. J. Membr. Sci. 2012, 415, 587–595. [Google Scholar] [CrossRef]
- Bellaistre, M.C.d.; Renaud, L.; Kleimann, P.; Morin, P.; Randon, J.; Rocca, J.-L. Streaming current measurements in zirconia-coated capillaries. Electrophoresis 2004, 25, 3086–3091. [Google Scholar] [CrossRef]
- Gallardo-Moreno, A.M.; Vadillo-Rodríguez, V.; Perera-Núñez, J.; Bruqueab, J.M.; González-Martín, M.L. The zeta potential of extended dielectrics and conductors in terms of streaming potential and streaming current measurements. Phys. Chem. Chem. Phys. 2012, 14, 9758–9767. [Google Scholar] [CrossRef] [PubMed]
- Lis, D.; Backus, E.H.G.; Hunger, J.; Parekh, S.H.; Bonn, M. Liquid flow along a solid surface reversibly alters interfacial chemistry. Science 2014, 344, 1138–1142. [Google Scholar] [CrossRef]
- Werkhoven, B.L.; Everts, J.C.; Samin, S.; Roij, R.v. Flow-Induced Surface Charge Heterogeneity in Electrokinetics due to Stern-Layer Conductance Coupled to Reaction Kinetics. Phys. Rev. Lett. 2018, 120, 264502. [Google Scholar] [CrossRef]
- Ober, P.; Boon, W.Q.; Dijkstra, M.; Backus, E.H.G.; Roij, R.v.; Bonn, M. Liquid flow reversibly creates a macroscopic surface charge gradient. Nat. Commun. 2021, 12, 4102. [Google Scholar] [CrossRef] [PubMed]
- Meng, S.; Han, Y.; Zhao, W.; Zhu, Y.; Zhang, C.; Feng, X.; Zhang, C.; Zang, D.; Jing, G.; Wang, K. Electrokinetic origin of swirling flow on nanoscale interface. arXiv 2024, arXiv:2402.04279. [Google Scholar]
- Paratore, F.; Boyko, E.; Kaigala, G.V.; Bercovici, M. Electroosmotic Flow Dipole: Experimental Observation and Flow Field Patterning. Phys. Rev. Lett. 2019, 122, 224502. [Google Scholar] [CrossRef]
- Yang, X.; Rong, C.; Zhang, L.; Ye, Z.; Wei, Z.; Huang, C.; Zhang, Q.; Yuan, Q.; Zhai, Y.; Xuan, F.-Z.; et al. Mechanistic insights into C-C coupling in electrochemical CO reduction using gold superlattices. Nat. Commun. 2024, 15, 720. [Google Scholar] [CrossRef]
- Ramos, A.; Morgan, H.; Green, N.G.; Castellanos, A. Ac electrokinetics: A review of forces in microelectrode structures. J. Phys. D Appl. Phys. 1998, 31, 2338–2353. [Google Scholar] [CrossRef]
- Zhao, W.; Wang, G. Scaling of velocity and scalar structure functions in ac electrokinetic turbulence. Phys. Rev. E 2017, 95, 023111. [Google Scholar] [CrossRef]
- Nan, K.; Shi, Y.; Zhao, T.; Tang, X.; Zhu, Y.; Wang, K.; Bai, J.; Zhao, W. Mixing and Flow Transition in an Optimized Electrokinetic Turbulent Micromixer. Anal. Chem. 2022, 94, 12231–12239. [Google Scholar] [CrossRef]
- Hu, Z.; Zhao, W.; Chen, Y.; Han, Y.; Zhang, C.; Feng, X.; Jing, G.; Wang, K.; Bai, J.; Wang, G.; et al. Onset of Nonlinear Electroosmotic Flow under an AC Electric Field. Anal. Chem. 2022, 94, 17913–17921. [Google Scholar] [CrossRef] [PubMed]
- Lee, M.; Park, G.; Park, C.; Kim, C. Improvement of Grid Independence Test for Computational Fluid Dynamics Model of Building Based on Grid Resolution. Adv. Civ. Eng. 2020, 2020, 8827936. [Google Scholar] [CrossRef]
- Suresh, V.; Homsy, G.M. Stability of time-modulated electroosmotic flow. Phys. Fluids 2004, 16, 2349–2356. [Google Scholar] [CrossRef]
- Hu, Z.; Zhao, T.; Zhao, W.; Yang, F.; Wang, H.; Wang, K.; Bai, J.; Wang, G. Transition from periodic to chaotic AC electroosmotic flows near electric double layer. AIChE J. 2021, 67, e17148. [Google Scholar] [CrossRef]




| Material | ) | ) | |
|---|---|---|---|
| Liquid water | 997 | 89.57 | 80 |
| Parameter | Value |
|---|---|
| Number of grid elements | 7,106,000 |
| Number of grid vertices | 7,275,576 |
| Minimum grid element quality | 1.0 |
| Average grid element quality | 1.0 |
| Grid element volume ratio | |
| Grid volume | μm3 |
| direction | 6.68 nm |
| direction | 20 nm |
| direction | 4.42 nm |
| direction | 13.26 nm |
| direction | 1.33 nm |
| direction | 180 nm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, Y.; Zhao, W. Numerical Simulation of the Influence of Non-Uniform ζ Potential on Interfacial Flow. Micromachines 2024, 15, 419. https://doi.org/10.3390/mi15030419
Han Y, Zhao W. Numerical Simulation of the Influence of Non-Uniform ζ Potential on Interfacial Flow. Micromachines. 2024; 15(3):419. https://doi.org/10.3390/mi15030419
Chicago/Turabian StyleHan, Yu, and Wei Zhao. 2024. "Numerical Simulation of the Influence of Non-Uniform ζ Potential on Interfacial Flow" Micromachines 15, no. 3: 419. https://doi.org/10.3390/mi15030419






