Ballistic Response of a Glass Fiber Composite for Two Levels of Threat
Abstract
1. Introduction
2. Materials and Methods
3. Results of the Simulation
3.1. The Model
- Macro, with the target being one body, made of a single equivalent material, used especially for metallic shields [41];
- Meso, implying the layers as a continuous body with equivalent properties determined experimentally [27] and paying attention to modeling the bonding between them, being applied the cohesive zone model [42,43,44,45,46,47,48,49], as the designer wanted to have the thickness of the entire panel as small as possible; in this group, we can include fabrics modeled with yarns. This model is difficult to calibrate, taking into account the statistical response of such a multitude of bodies;
3.2. Material Models of the Bodies Involved in the Model
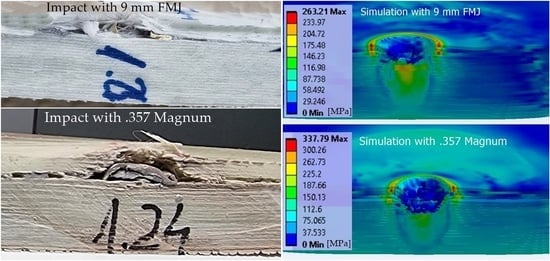
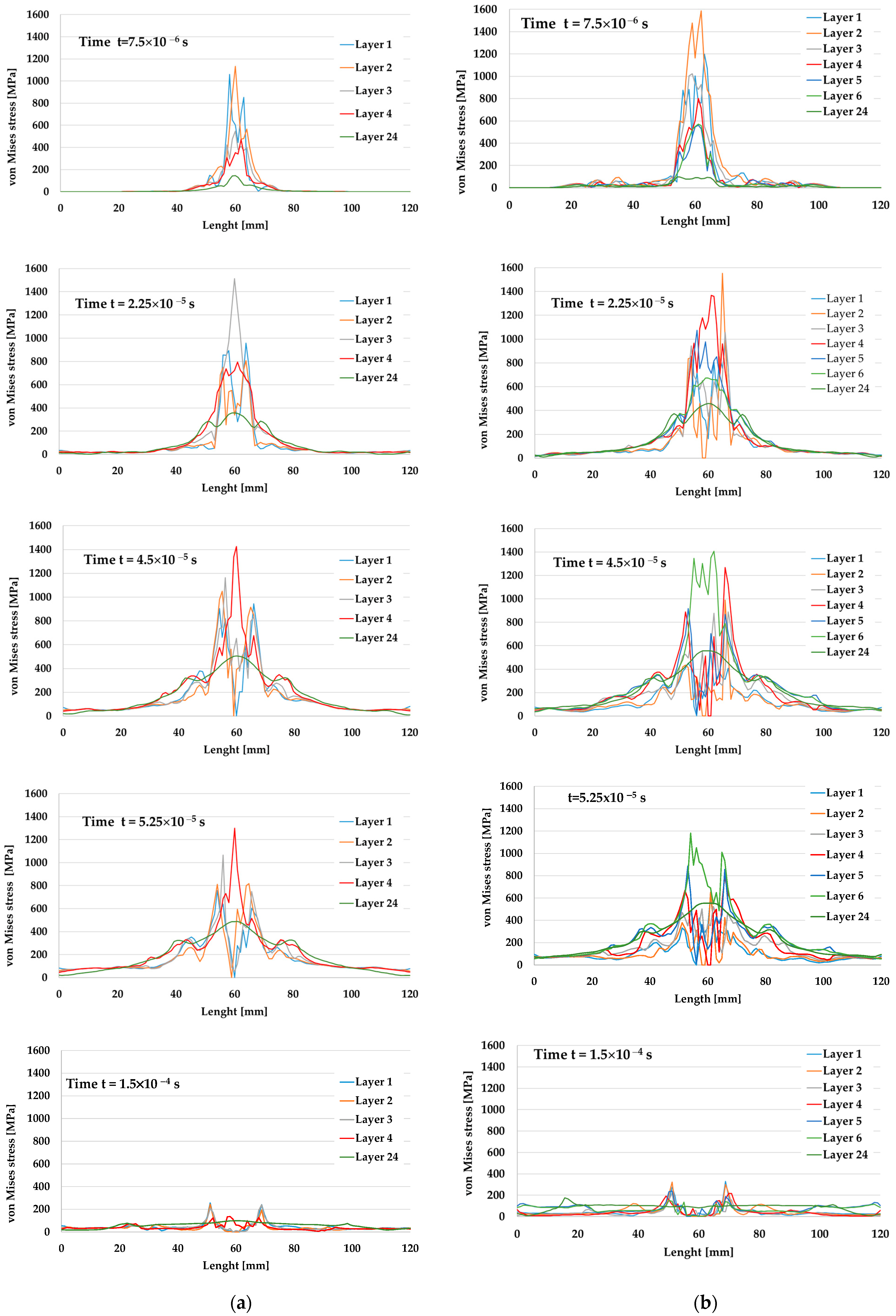
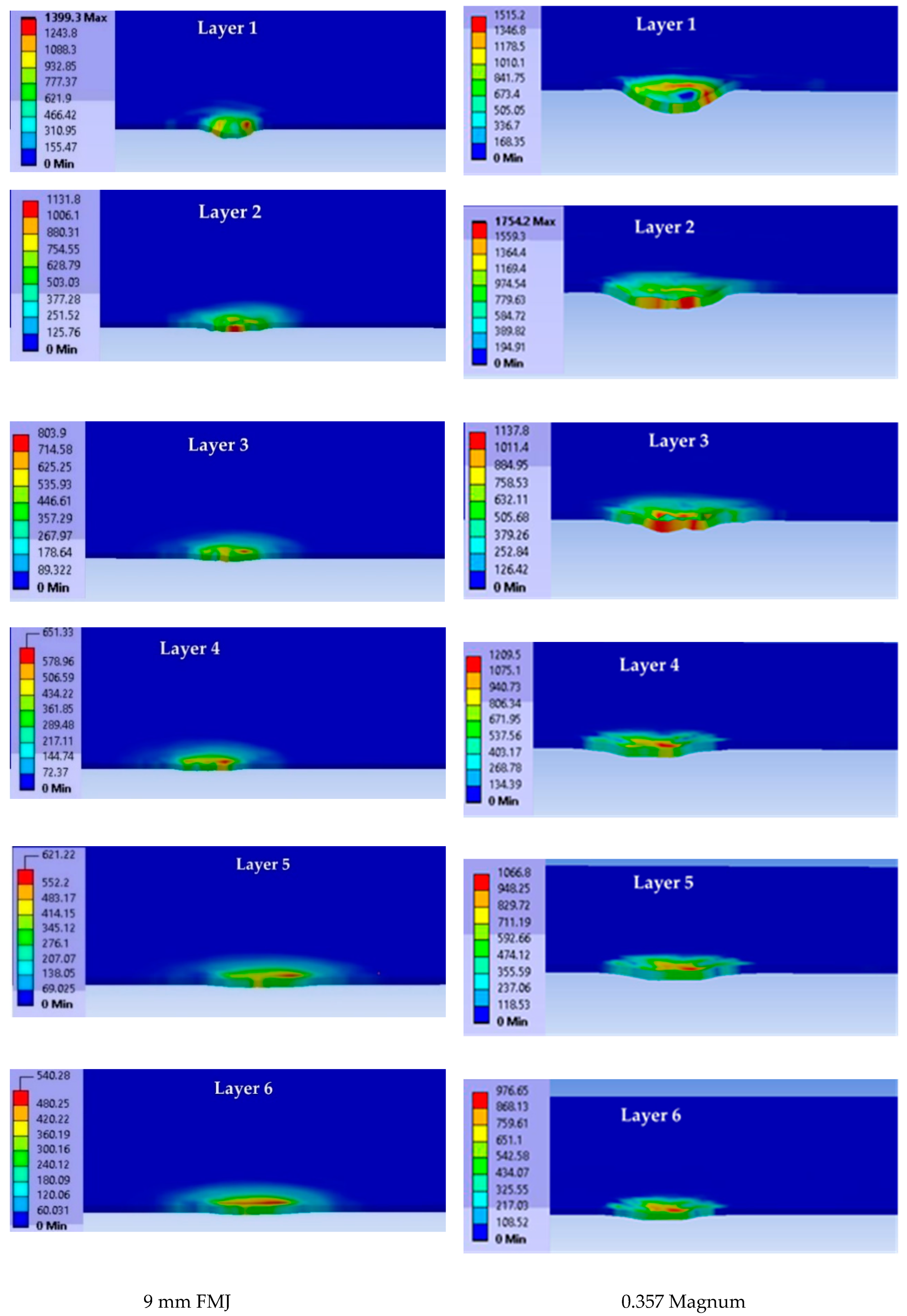
3.3. Analysis of the Simulation Results
4. Analysis of the Failure Mechanisms after Actual Tests
- Micro, including glass fibers and resin damage;
- Meso, here including delamination and failure of the projectile;
- Macro, including qualification of the composite and partial or total penetration, evidenced by photos taken of the entire panel or large areas or sections of it.
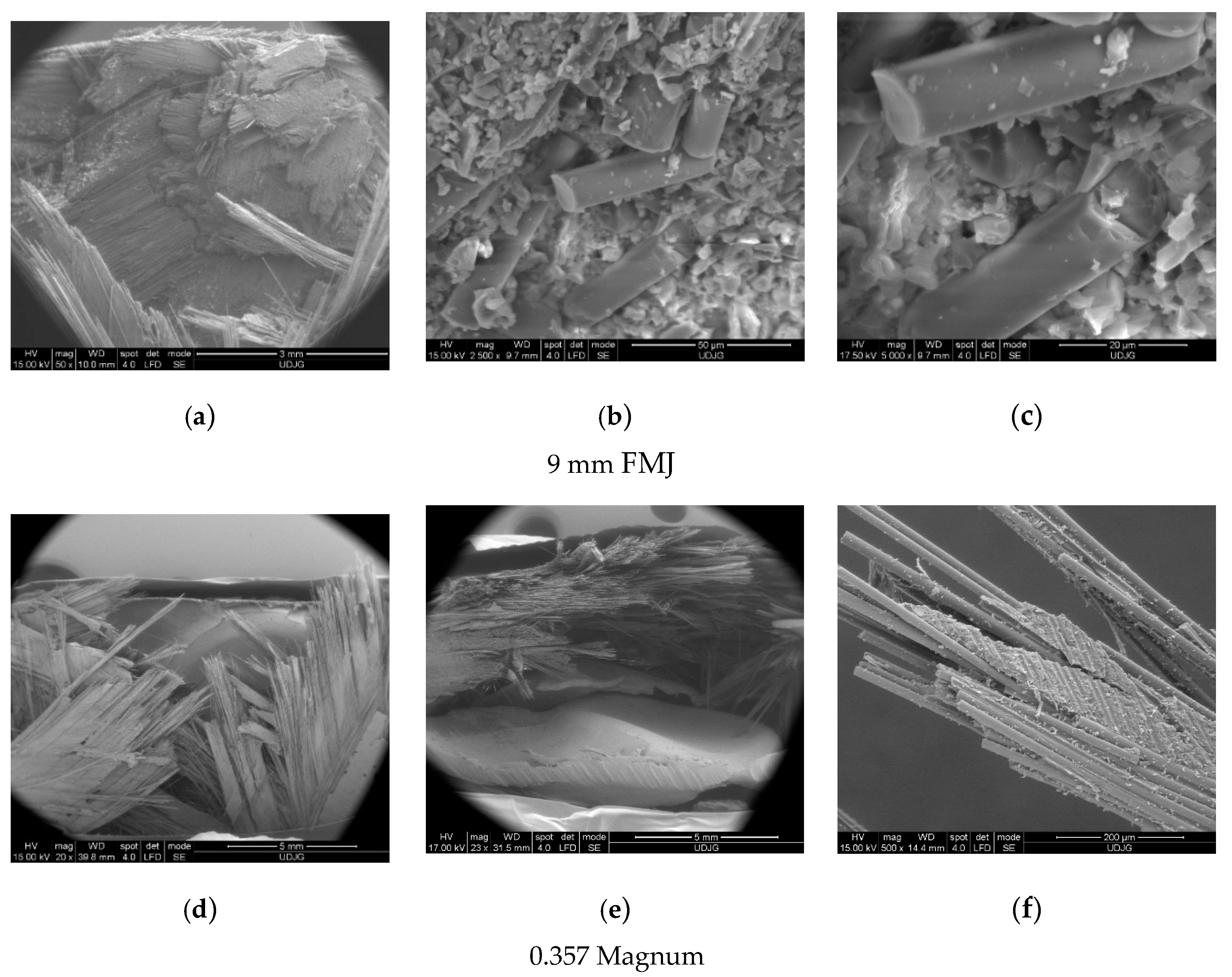
- (a)
- Top view of the penetration hole produced by the 9 mm FMJ;
- (b)
- Detail of the surface that the projectile was stopped on (fragments of the projectile are not in this image, but there are glass fiber fragments);
- (c)
- Shear cut of a glass fiber (up), typically for impacted glass fibers, meaning a cut surface almost perpendicular to the fiber axis;
- (d)
- Top view of the penetration hole produced by the 0.357 Magnum. On the bottom the flattened projectile is visible, less fragmented compared to the 9 mm FMJ;
- (e)
- Detail in cross section, with flattened projectile and hole, not very cylindrical due to the different orientation of the yarns in each sub-layer;
- (f)
- Detail of cut fibers and the aspect of delamination, revealing the detaching of the fibers of different orientation and other fibers remaining in the matrix.
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ou, Y.; Zhu, D.; Zhang, H.; Huang, L.; Yao, Y.; Li, G.; Mobasher, B. Mechanical Characterization of the Tensile Properties of Glass Fiber and Its Reinforced Polymer (GFRP) Composite under Varying Strain Rates and Temperatures. Polymers 2016, 8, 196. [Google Scholar] [CrossRef]
- Stanciu, M.D.; Draghicescu, H.T.; Rosca, I.C. Mechanical Properties of GFRPs Exposed to Tensile, Compression and Tensile–Tensile Cyclic Tests. Polymers 2021, 13, 898. [Google Scholar] [CrossRef]
- Reddy, R.S.P.; Reddy, S.T.; Madhu, V.; Gogia, A.K.; Rao, V.K. Behavior of E-glass composite laminates under ballistic impact. Mater. Des. 2015, 84, 79–86. [Google Scholar] [CrossRef]
- Czech, K.; Oliwa, R.; Krajewski, D.; Bulanda, K.; Oleksy, M.; Budzik, G.; Mazurkow, A. Hybrid Polymer Composites Used in the Arms Industry: A Review. Materials 2021, 14, 3047. [Google Scholar] [CrossRef]
- Key, C.T.; Alexander, C.S.; Harstad, E.N.; Schumacher, S.C. Dynamic Shock Response of an S2 Glass/SC15 Epoxy Woven Fabric Composite Material System. AIP Conf. Proc. 2018, 1979, 110006. [Google Scholar] [CrossRef]
- Pavlovic, A.; Fragassa, C. Investigating the resistance of reinforced barriers to high velocity projectiles. Eng. Struct. 2018, 174, 384–395. [Google Scholar] [CrossRef]
- Pai, A.; Kini, C.R.; Shenoy, S.B. Development of materials and structures for shielding applications against Blast and Ballistic impact: A Detailed Review. Thin-Walled Struct. 2022, 179, 109664. [Google Scholar] [CrossRef]
- Bilisik, K.; Karaduman, N.S.; Bilisik, N.E. Fiber Architectures for Composite Applications. In Fibrous and Textile Materials for Composite Applications. Textile Science and Clothing Technology; Rana, S., Fangueiro, R., Eds.; Springer: Singapore, 2016; pp. 75–134. [Google Scholar] [CrossRef]
- Alagumalai, V.; Shanmugam, V.; Balasubramanian, N.K.; Krishnamoorthy, Y.; Ganesan, V.; Försth, M.; Sas, G.; Berto, F.; Chanda, A.; Das, O. Impact Response and Damage Tolerance of Hybrid Glass/Kevlar-Fibre Epoxy Structural, Composites. Polymers 2021, 13, 2591. [Google Scholar] [CrossRef]
- Onyechi Pius, C.; Edelugo, S.O.; Ihueze Chukwutoo, C.; Obuka Nnaemeka, S.P.; Chukwumuanya Okechukwu, E. High Velocity Impact Response Evaluation of a Glass Fibre Reinforced Polymer (GFRP) Composite—Amour Body. Int. J. Energy Eng. 2013, 3, 242–255. [Google Scholar] [CrossRef]
- Zhai, Z.; Jiang, B.; Drummer, D. Strain rate-dependent mechanical behavior of quasi-unidirectional E-glass fabric reinforced polypropylene composites under off-axis tensile loading. Polym. Test. 2018, 69, 276–285. [Google Scholar] [CrossRef]
- Kalebek, N.A.; Babaarslan, O. Fiber Selection for the Production of Nonwovens. In Non-Woven Fabrics; Jeon, H., Ed.; InTech: London, UK, 2016; pp. 1–32. [Google Scholar] [CrossRef]
- Aghbash, S.A.; Breite, C.; Mehdikhani, M.; Swolfs, Y. Longitudinal debonding in unidirectional fibre-reinforced composites: Numerical analysis of the effect of interfa-cial properties. Compos. Sci. Technol. 2022, 218, 109117. [Google Scholar] [CrossRef]
- NIJ Standard 0108.01; Ballistic Resistant Protective Materials. National Institute of Justice: Washington, DC, USA, 1985.
- SR EN 1523-2004; Windows, Doors, Shutters and Blinds—Bullet Resistance—Test Method. ASRO: Bucharest, Romania, 2004.
- Nastasescu, V.; Barsan, G. Metoda Particulelor Libere in Analiza Numerica a Mediilor Continue; AGIR Publishing House: Bucharest, Romania, 2015. (In Romanian) [Google Scholar]
- Satkar, A.R.; Mache, A.; Kulkarni, A. Numerical investigation on perforation resistance of glass-carbon/epoxy hybrid composite laminate under ballistic impact. Mater. Today Proc. 2022, 59, 734–741. [Google Scholar] [CrossRef]
- Ansari, M.M.; Chakrabarti, A. Ballistic performance of unidirectional glass fiber laminated composite plate under normal and oblique impact, 11th International Symposium on Plasticity and Impact Mechanics. Proc. Eng. 2017, 173, 161–168. [Google Scholar] [CrossRef]
- Pirvu, C. Contribution on Experimental and Numerical Studying Ballistic Protection Packages Made of Aramid Fibers. PhD Thesis, “Dunarea de Jos” University of Galati, Galati, Romania, 2015. [Google Scholar]
- NIJ Standard 0101.07; Ballistic Resistance of Body Armor, National Institute of Justice. National Institute of Justice: Washington, DC, USA, January 2018.
- Naik, N.K.; Shrirao, P. Composite structures under ballistic impact. Compos. Struct. 2004, 66, 579–590. [Google Scholar] [CrossRef]
- Bilisik, K. Two-dimensional (2D) fabrics and three-dimensional (3D) preforms for ballistic and stabbing protection: A review. Text. Res. J. 2017, 87, 2275–2304. [Google Scholar] [CrossRef]
- Briscoe, B.J.; Motamedi, F. Role of Interfacial Friction and Lubrication in Yarn and Fabric Mechanics. Text. Res. J. 1990, 60, 697–708. [Google Scholar] [CrossRef]
- Zhou, Y.; Yao, W.; Zhang, Z.; Sun, M.; Xiong, Z.; Lin, Y.; Wang, D.; Wang, M. The effect of cumulative damage on the ballistic performance of plain weaves. Compos. Struct. 2022, 297, 115978. [Google Scholar] [CrossRef]
- Signetti, S.; Ryu, S.; Pugno, M.N. Impact mechanics of multilayer composite armors: Analytical modeling, FEM numerical simulation, and ballistic experiments. Compos. Struct. 2022, 297, 115916. [Google Scholar] [CrossRef]
- Ingle, S.; Chandra, S.Y.; Anirban, G.; Sushil, M. Effect of material properties on ballistic energy absorption of woven fabrics subjected to different levels of inter-yarn friction. Compos. Struct. 2021, 266, 113824. [Google Scholar] [CrossRef]
- Vescovini, A.; Balen, L.; Scazzosi, R.; da Silva, A.A.X.; Amico, S.C.; Giglio, M.; Manes, A. Numerical investigation on the hybridization effect in inter-ply S2-glass and aramid woven composites subjected to ballistic impacts. Compos. Struct. 2021, 276, 114506. [Google Scholar] [CrossRef]
- Mohan, S.; Velu, S. Ballistic impact behaviour of unidirectional fibre reinforced composites. Int. J. Impact Eng. 2014, 63, 164–176. [Google Scholar] [CrossRef]
- Ma, D.; Manes, A.; Campos Amico, S.; Giglio, M. Ballistic strain-rate-dependent material modelling of glass-fibre woven composite based on the prediction of a meso-heterogeneous approach. Compos. Struct. 2019, 216, 187–200. [Google Scholar] [CrossRef]
- Ma, D.; Wang, Z.; Giglio, M.; Amico, S.C.; Manes, A. Influence of strain-rate related parameters on the simulation of ballistic impact in woven composites. Compos. Struct. 2022, 300, 116142. [Google Scholar] [CrossRef]
- Karthick, P.; Ramajeyathilagam, K. Numerical study on ballistic impact behavior of hybrid composites. Mater. Today: Proc. 2022, 59, 995–1003. [Google Scholar] [CrossRef]
- Meyer, C.S.; O’Brien, D.J.; Haque, B.Z.G.; Gillespie, J.W. Mesoscale modeling of ballistic impact experiments on a single layer of plain weave composite. Compos. Part B 2022, 235, 109753. [Google Scholar] [CrossRef]
- National Research Council. Opportunities in Protection Materials Science and Technology for Future Army Applications; The National Academies Press: Washington, DC, USA, 2011; Available online: http://nap.edu/13157 (accessed on 12 January 2022). [CrossRef]
- Ojoc, G.G. A Theoretical and Experimental Study of Ballistic Protection Packages Made of Glass Fibers. PhD Thesis, “Dunarea de Jos” University of Galati, Galati, Romania, 2022. [Google Scholar]
- Glass. Available online: https://www.castrocompositesshop.com/en/63-glass (accessed on 22 February 2022).
- 1200 g/m2 Quadriaxial Stitched Glass Fabric (0°/+45°/90°/−45°), 127 cm Wide. Available online: https://www.castrocompositesshop.com/en/fibre-reinforcements/1204-1200-gm2-quadriaxial-stitched-glass-fabric-0%C2%BA45%C2%BA90%C2%BA-45%C2%BA-127-cm-wide.html (accessed on 22 February 2022).
- Gibson, R. Principles of Composite Material Mechanics, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2007. [Google Scholar]
- Sika Group. About Us. Available online: https://www.sika.com/en/home.html. (accessed on 3 October 2021).
- Biresin® CR82. Composite Resin System. Available online: https://industry.sika.com/dms/getdocument.get/93a3a9b1-7291-47ce-8ca7-18ae3f458043/Biresin-CR82-New.pdf. (accessed on 4 October 2020).
- Abdulmajeed, A.A.; Närhia, T.O.; Vallittu, P.K.; Lassila, L.V. The effect of high fiber fraction on some mechanical properties of unidirectional glass fiber-reinforced composite. Dent. Mater. 2011, 27, 313–321. [Google Scholar] [CrossRef]
- Masri, R.; Ryan, S. Ballistic limit predictions of non-identical layered targets perforated in ductile hole formation. Int. J. Impact Eng. 2023, 171, 104391. [Google Scholar] [CrossRef]
- Jinescu, V.V. Application in Mechanical Engineering of Principle of Critical Energy; Lambert Academic Publishing: Saarbrucken, Germany, 2015. [Google Scholar]
- Jinescu, V.V.; Nicolof, V.I.; Chelu, A.; Manea, S.E. Calculation of the local critical state taking into account the deterioration and the residual stresses. Int. J. Eng. Sci. 2017, 2, 9–21. [Google Scholar] [CrossRef]
- Cohesive Zone Material (CZM) Model, Release 18.2 © ANSYS, Inc. Available online: https://www.mm.bme.hu/~gyebro/files/ans_help_v182/ans_thry/thy_mat11.html (accessed on 22 February 2022).
- Li, S.; Thouless, M.D.; Waas, A.M.; Schroeder, J.A.; Zavattieri, P.D. Use of a cohesive-zone model to analyze the fracture of a fiber-reinforced polymer–matrix composite. Compos. Sci. Technol. 2005, 65, 537–549. [Google Scholar] [CrossRef]
- Harper, P.W.; Hallett, S.R. Cohesive zone length in numerical simulations of composite delamination. Eng. Fract. Mech. 2008, 75, 4774–4792. [Google Scholar] [CrossRef]
- Harper, P.W.; Sun, L.; Hallett, S.R. A study on the influence of cohesive zone interface element strength parameters on mixed mode behaviour. Composites Part A 2012, 43, 722–734. [Google Scholar] [CrossRef]
- Joki, R.K.; Grytten, F.; Hayman, B.; Sørensen, B.F. Determination of a cohesive law for delamination modelling—Accounting for variation in crack opening and stress state across the test specimen width. Compos. Sci. Technol. 2016, 128, 49–57. [Google Scholar] [CrossRef]
- Chowdhury, U.; Wu, X.-F. Cohesive zone modeling of the elastoplastic and failure behavior of polymer nanoclay composites. J. Compos. Sci. 2021, 5, 131. [Google Scholar] [CrossRef]
- Sockalingam, S. Transverse Impact of Ballistic Fibers and Yarns—Fiber Length-Scale Finite Element Modeling and Experiments. Ph.D. Thesis, University of Delaware, Newark, DE, USA, 2016. Available online: https://udspace.udel.edu/handle/19716/19972 (accessed on 22 February 2022).
- Mishnaevsky, L., Jr. Micromechanical modelling of wind turbine blade materials. In Advances in Wind Turbine Blade Design and Materials; Brøndsted, P., Nijssen, R.P.L., Eds.; Woodhead Publishing Limited: Cambridge, UK, 2013; pp. 298–324. [Google Scholar] [CrossRef]
- Totolici Rusu, V.; Ojoc, G.G.; Pirvu, C.; Deleanu, L. Influence of element size in a case of impact simulation. Mech. Test. Diagn. 2021, 10, 24–29. Available online: https://www.gup.ugal.ro/ugaljournals/index.php/mtd/article/view/406 (accessed on 22 February 2022). [CrossRef]
- ANSYS Explicit Dynamics Analysis Guide; ANSYS, Inc.: Canonsburg, PA, USA, 2021.
- Buyuk, M.; Kan, C.-D.S.; Bedewi, N.E.; Durmus, A.; Ulku, S. Moving Beyong the Finite Elements, a Comparison between the Finite Element Methods and Meshless Methods for a Ballistic Impact Simulation. In Proceedings of the 8th International LS-Dyna Users Conference, DynaLook, Detroit, MI, USA, 2–4 May 2004. [Google Scholar]
- Scazzosi, R.; Manesa, A.; Giglio, M. An Enhanced Material Model for the Simulation of High-Velocity Impact on Fiber-Reinforced Composites. Procedia Struct. Integr. 2019, 24, 53–65. [Google Scholar] [CrossRef]
- Wiśniewski, A.; Gmitrzuk, M. Validation of numerical model of the Twaron CT709 ballistic fabric. In Proceedings of the 27th International Symposium on Ballistics, Freiburg, Germany, 22–26 April 2013; Ballistic Publishing: Adelaide, Australia, 2013; Volume 2, pp. 1535–1544. [Google Scholar]
- Børvik, T.; Dey, S.; Clausen, A.H. Perforation resistance of five different high-strength steel plates subjected to small-arms projectiles. Int. J. Impact Eng. 2009, 36, 948–964. [Google Scholar] [CrossRef]
- Giglio, M.; Gilioli, A.; Manes, A.; Peroni, L.; Scapin, M. Investigation about the influence of the mechanical properties of lead. EPJ Web Conf. 2012, 26, 04010. [Google Scholar] [CrossRef]
- Peroni, L.; Scapin, M.; Fichera, C.; Manes, A.; Giglio, M. Mechanical properties at high strain-rate of lead core and brass jacket of a NATO 7.62 mm ball bullet in numerical simulations of ballistic impacts. Proc. DYMAT 2012, 26, 01060. [Google Scholar] [CrossRef]
- Naresh, K.; Shankar, K.; Rao, B.S.; Velmurugan, R. Effect of high strain rate on glass fiber reinforced epoxy laminated composites. Composites Part B 2016, 100, 125–135. [Google Scholar] [CrossRef]
- Kumar, M.; Naik, N.K. Prediction of mechanical behavior of composites under high strain rate tensile loading. Mech. Res. Commun. 2018, 90, 1–7. [Google Scholar] [CrossRef]
- Tao, Y.; Chen, H.; Yao, K.; Lei, H.; Pei, Y.; Fang, D. Experimental and theoretical studies on inter-fiber failure of unidirectional polymer-matrix composites under different strain rates. Int. J. Solids Struct. 2017, 113–114, 37–46. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, X.; Young, R.; Kinloch, I.; Wells, G. A numerical study of ply orientation on ballistic impact resistance of multi-ply fabric panels. Composites Part B 2015, 68, 259–265. [Google Scholar] [CrossRef]
- Raghavendra, G.; Naidu, P.P.; Ojha, S.; Vasavi, B.; Pachal, M.; Acharya, S.K. Effect of bi-directional and multi-directional fibers on the mechanical properties of glass fiber-epoxy composites. Mater. Res. Express 2019, 6, 5353. [Google Scholar] [CrossRef]
- Montenegro, D.M.; Pappas, G.; Botsis, J.; Zogg, M.; Wegener, K. A comparative study of mode I delamination behavior of unidirectional glass fiber-reinforced polymers with epoxy and polyurethane matrices using two methods. Eng. Fract. Mech. 2019, 206, 485–500. [Google Scholar] [CrossRef]
- Rosenberg, Z.; Erez Dekel, E. Terminal Ballistics, 3rd ed.; Springer Nature Switzerland AG: Cham, Switzerland, 2020. [Google Scholar]
- Nunes, S.G.; Scazzosi, R.; Manes, A.; Amico, S.C.; de Amorim, J.W.F.; Giglio, M. Influence of projectile and thickness on the ballistic behavior of aramid composites: Experimental and numerical study. Int. J. Impact Eng. 2019, 132, 103307. [Google Scholar] [CrossRef]








| Layer | Yarn Orientation | Fiber Type | Area Weight |
|---|---|---|---|
| 1. | 0° | 600 Tex | 283 g/m2 |
| 2. | 45° | 300 + 600 Tex | 300 g/m2 |
| 3. | 90° | 600 Tex | 307 g/m2 |
| 4. | −45° | 300 + 600 Tex | 300 g/m2 |
| Stitch | 76 Dtex | 10 g/m2 | |
| Total surface weight: 1200 g/m2 (±%3) | |||
| Element Average (wt. %) (9 Measurement on Cross Section of Fibers on the Same Yarn) | |||||||||||||
| B | C | O | Na | Mg | Al | Si | S | Cl | K | Ca | Ti | Fe | Zn |
| 29.3 | 24.7 | 9.0 | 0.3 | 0.4 | 3.5 | 14.2 | 1.7 | 0.2 | 0.2 | 8.8 | 0.5 | 2.1 | 4.4 |
| Element Average (Wt. %) (4 Measurement on External Surface of Fibers on the Same Yarn) | |||||||||||||
| 30.8 | 24.6 | 9.7 | 0.5 | 0.6 | 3.1 | 10.6 | 1.5 | 0.2 | 0.5 | 7.8 | 1.0 | 2.8 | 5.6 |
| Characteristics | Resin (A) Biresin® CR82 | Hardener (B) Biresin® CH80-2 | |
| Mixing ratio, parts by weight | 100 | 27 | |
| Viscosity at 25 °C, mPa·s | ~1.600 | ~80 | |
| Density at 25 °C, g/mL | 1.11 | 0.99 | |
| Mixture | |||
| Potlife, 100 g/RT (approx.), minutes | ~80 | ||
| Mix viscosity, 25 °C (approx.), mPa·s | 800 | ||
| Characteristics | Tested According to | Units | Resin Biresin® CR82 (A) with hardener CH80-2 |
| Tensile strength | ISO 527 | MPa | 85 |
| Tensile elasticity modulus | ISO 527 | MPa | 3250 |
| Elongation at break | ISO 527 | % | 5.0 |
| Flexural strength | ISO 178 | MPa | 125 |
| Flexural E-Modulus | ISO 178 | MPa | 3200 |
| Compressive strength | ISO 604 | MPa | 107 |
| Density | ISO 1183 | g/cm3 | 1.16 |
| Shore hardness | ISO 868 | - | D 84 |
| Impact resistance | ISO 179 | kJ/m2 | 21 |
| Typical thermal properties of fully cured neat resin | |||
| Heat distortion temperature | ISO 75A | °C | 77 |
| Glass transition temperature | ISO 11357 | °C | 89 |
| Panel | Fabrics Mass | Panel Mass | Resin Mass * | Mass Ratio Fabrics/Panel ** | Surface Density *** | Thickness in 4 Points | ||||
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 2 | 3 | 4 | Average | ||||||
| [g] | [g] | [g] | [kg/m2] | [mm] | ||||||
| 0 | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| 1 | 2500 | 3273 | 773 | 0.763 | 27.77 | 18.37 | 18.46 | 18.60 | 18.12 | 18.38 |
| 2 | 2510 | 3118 | 608 | 0.805 | 27.88 | 17.21 | 18.53 | 17.37 | 18.23 | 17.83 |
| 3 | 2460 | 3150 | 690 | 0.781 | 27.33 | 18.61 | 18.04 | 18.96 | 18.20 | 18.45 |
| 4 | 2460 | 3174 | 714 | 0.775 | 27.33 | 18.24 | 18.02 | 18.76 | 17.97 | 18.24 |
| 5 | 2450 | 3200 | 750 | 0.765 | 27.22 | 18.53 | 18.23 | 18.46 | 18.37 | 18.39 |
| Average | 2476 | 3183 | 707 | 0.778 | 27.51 | 18.26 | ||||
| Max | 2510 | 3273 | 773 | 0.805 | 27.88 | |||||
| Min | 2450 | 3118 | 608 | 0.763 | 27.22 | |||||
| Standard deviation | 24.17 | 52.51 | 57.17 | 0.015 | 0.266 | 0.225 | ||||
| Class | Type of Weapon | Caliber | Bullet | Test Condition | ||
|---|---|---|---|---|---|---|
| Type | Mass [g] | Test Range [m] | Bullet Velocity [m/s] | |||
| FB2 | Hand gun | 9 mm Luger | FJ/RN/SC | 8.0 ± 0.1 | 5 ± 0.5 | 400 ± 10 |
| FB3 | Hand gun | 0.357 Magnum | FJ/RN/SC | 10.2 ± 0.1 | 5 ± 0.5 | 430 ± 10 |
| FJ—full metal jacket, RN—round-nose bullet, SC—soft core (lead). | ||||||
| Property | Jacket (Brass) | Core (Lead Alloy) |
|---|---|---|
| Density [kg/m3] | 8450 * | 11350 * |
| Specific heat at constant pressure [mJ/(kg °C)] | 380 | 1.288 × 105 |
| Young modulus [MPa] | 90,000 * (115,000, [59]) | 16,000 [59] |
| Poisson coefficient | 0.344 | 0.44 |
| Temperature [°C] | 22 | 22 |
| Constants for Johnson–Cook model | ||
| Initial yield limit [MPa] | 90 [58] (80 [59]) | 1 [59] (0, [58]) |
| Hardening constant [MPa] | 628 [58] | 55 [58] |
| Hardening exponent | 0.72 [58] | 9.8 × 10−2 [58] |
| Constant for strain rate | 0.266 [58] | 0.231 [58] |
| Thermal softening exponent | 1 [58] | 1 [58] |
| Quasi-static strain rate threshold (s−1) | 604 [59] | 221 [59] |
| Melting temperature [°C] | 927 * | 327.5 * |
| Equivalent plastic strain at break (EPS) | 0.75 * | 0.75 * |
| Mechanical properties of a layer | ||
| Property | Value | |
| Density [kg/m3] | 1904 * | |
| Specific heat at constant pressure [mJ/(kg °C)] | 6 × 105 * | |
| Young modulus [MPa] | 50,000 [60] | |
| Poisson coefficient | 0.3065 * | |
| Temperature [°C] | 22 | |
| Isotropic bilinear hardening model | ||
| Initial yield limit [MPa] | 550 * (577 for 495 s−1, in [60]) | |
| Tangent modulus [MPa] | 10,000 * | |
| Temperature [°C] | 22 | |
| Equivalent plastic strain at break (EPS) | 0.12 * | |
| Parameters for Modeling the Bilinear Strength in Interlaminar Delamination | |||||
| Tempe-rature, °C | Maximum normal traction stress at the interface, MPa | Normal displacement jump at completion of debonding, mm | Maximum tangential traction stress at the interface, MPa | Tangential displacement jump at completion of debonding, mm | Ratio |
| 22 | 70 | 5 | 50 | 0.1 | 0.3 |
| Parameters for Energy at Break in Interlaminar Delamination | |||||
| Tempe-rature, °C | Maximum normal contact stress, MPa | Critical fracture energy for normal separation, J/m2 | Maximum equivalent tangential contact stress, MPa | Critical fracture energy for tangential slip, J/m2 | Artificial damping coefficient, s |
| 22 | 100 | 3000 [65] | - | - | 0.1 |
| Case (Projectile) | Number of Broken Layers | |
|---|---|---|
| Experimental | Numerical | |
| 9 mm FMJ | 2–3 | 3 |
| 0.357 Magnum | 5 | 5 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ojoc, G.G.; Chiper Titire, L.; Munteniță, C.; Pîrvu, C.; Sandu, S.; Deleanu, L. Ballistic Response of a Glass Fiber Composite for Two Levels of Threat. Polymers 2023, 15, 1039. https://doi.org/10.3390/polym15041039
Ojoc GG, Chiper Titire L, Munteniță C, Pîrvu C, Sandu S, Deleanu L. Ballistic Response of a Glass Fiber Composite for Two Levels of Threat. Polymers. 2023; 15(4):1039. https://doi.org/10.3390/polym15041039
Chicago/Turabian StyleOjoc, George Ghiocel, Larisa Chiper Titire, Cristian Munteniță, Cătălin Pîrvu, Simona Sandu, and Lorena Deleanu. 2023. "Ballistic Response of a Glass Fiber Composite for Two Levels of Threat" Polymers 15, no. 4: 1039. https://doi.org/10.3390/polym15041039
APA StyleOjoc, G. G., Chiper Titire, L., Munteniță, C., Pîrvu, C., Sandu, S., & Deleanu, L. (2023). Ballistic Response of a Glass Fiber Composite for Two Levels of Threat. Polymers, 15(4), 1039. https://doi.org/10.3390/polym15041039