An Analytical Solution for Block Toppling Failure of Rock Slopes during an Earthquake
Abstract
:Featured Application
Abstract
1. Introduction
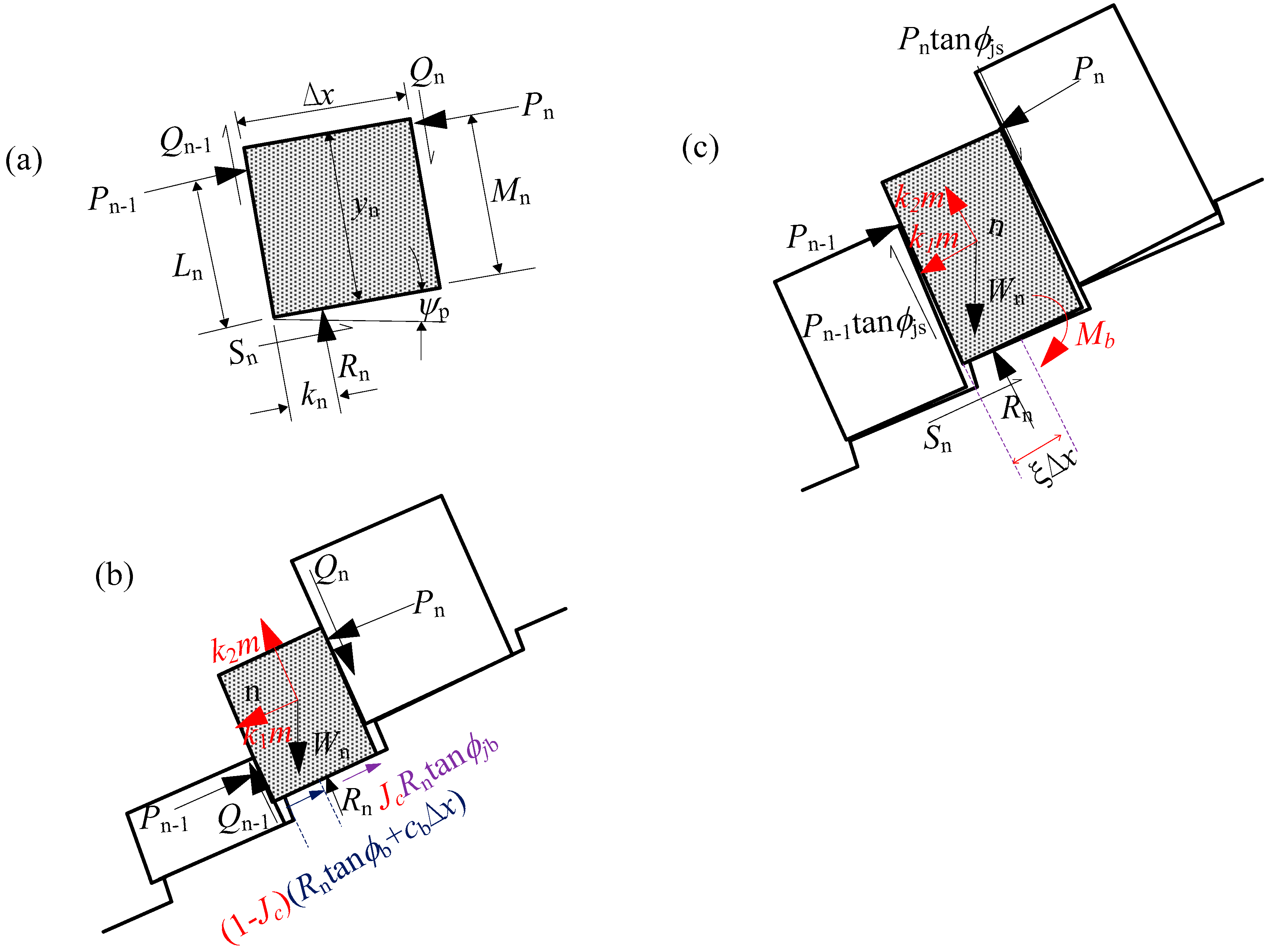
2. Mechanical Model and Analytical Solution to Toppling Slope Failure
- (1)
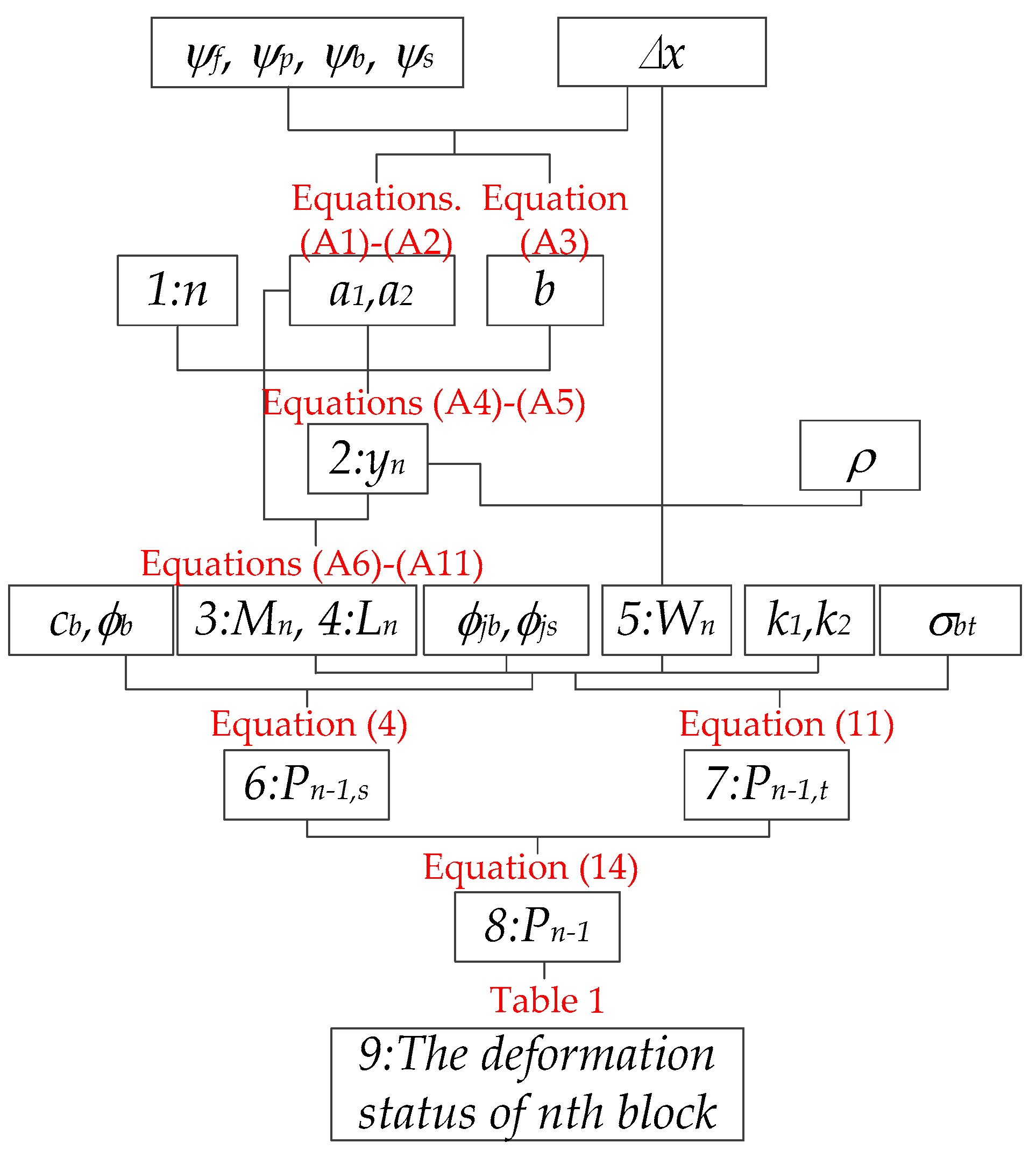
- Reach the basic geometric parameters ψb, ψp, ψs, ψd, ψf and ∆x from in- situ measurement work and calculating other geometric parameters a1, a2, b, yn, Mn and Ln according to Equations (A1)–(A11).
- (2)
- Determining all the physical parameters of the joints and blocks, e.g., ρ, φjb, φjs, φb, cb, σbt, and the joint connectivity rate (Jc) of the block base on the basis of lab tests, in-situ measurement or experience.
- (3)
- Calculating Pn−1,S and Pn−1,t from the top block downwards on the basis of Equations (4) and (11).
- (4)
- Determining Pn−1 and the deformation status of the nth block.
- If Pn−1,s < 0 and Pn−1,t < 0, Pn−1 = 0. It means that the nth block is stable and does not need the support of the (n − 1)th block.
- If Pn−1,s < 0 and Pn−1,t > 0, Pn−1 = Pn−1,t. It means that the nth block needs the support of the (n − 1)th block to inhibit toppling deformation.
- If Pn−1,t > Pn−1,s > 0, Pn−1 = Pn−1,t. It means that the nth block needs more support from the (n − 1)th block to inhibit toppling failure rather than sliding failure.
- If Pn−1,s > 0 and Pn−1,t < 0, Pn−1 = Pn−1,s. It means that the nth block needs the support of the (n − 1)th block to inhibit sliding deformation.
- If Pn−1,s > Pn−1,t > 0, Pn−1 = Pn−1,s. It means that the nth block needs more support from the (n − 1)th block to inhibit sliding failure rather than toppling failure.In summary, Pn−1 can also be simply obtained according to Equation (14):
- If Pn−1 = 0, the nth block is stable;
- If Pn−1 = Pn−1,t, the nth block is toppling;
- If Pn−1 = Pn−1,s, the nth block is sliding.
- Pn−1 and the deformation status can also be determined according to Table 1.
- (5)
- Estimating the stability of the slope. If the deformation status of the lowest block (n = 1) is stable (or P0 = 0), the slope is stable. If the deformation status of the lowest block (n = 1) is unstable (or P0 > 0), the slope is unstable.
- (6)
- Determining the FOS. The FOS of the slope can be determined using the methods as follows:A ratio Ra is adopted to obtain the parameters in calculation based on the actual parameters, which are shown as Equations (15)–(19).
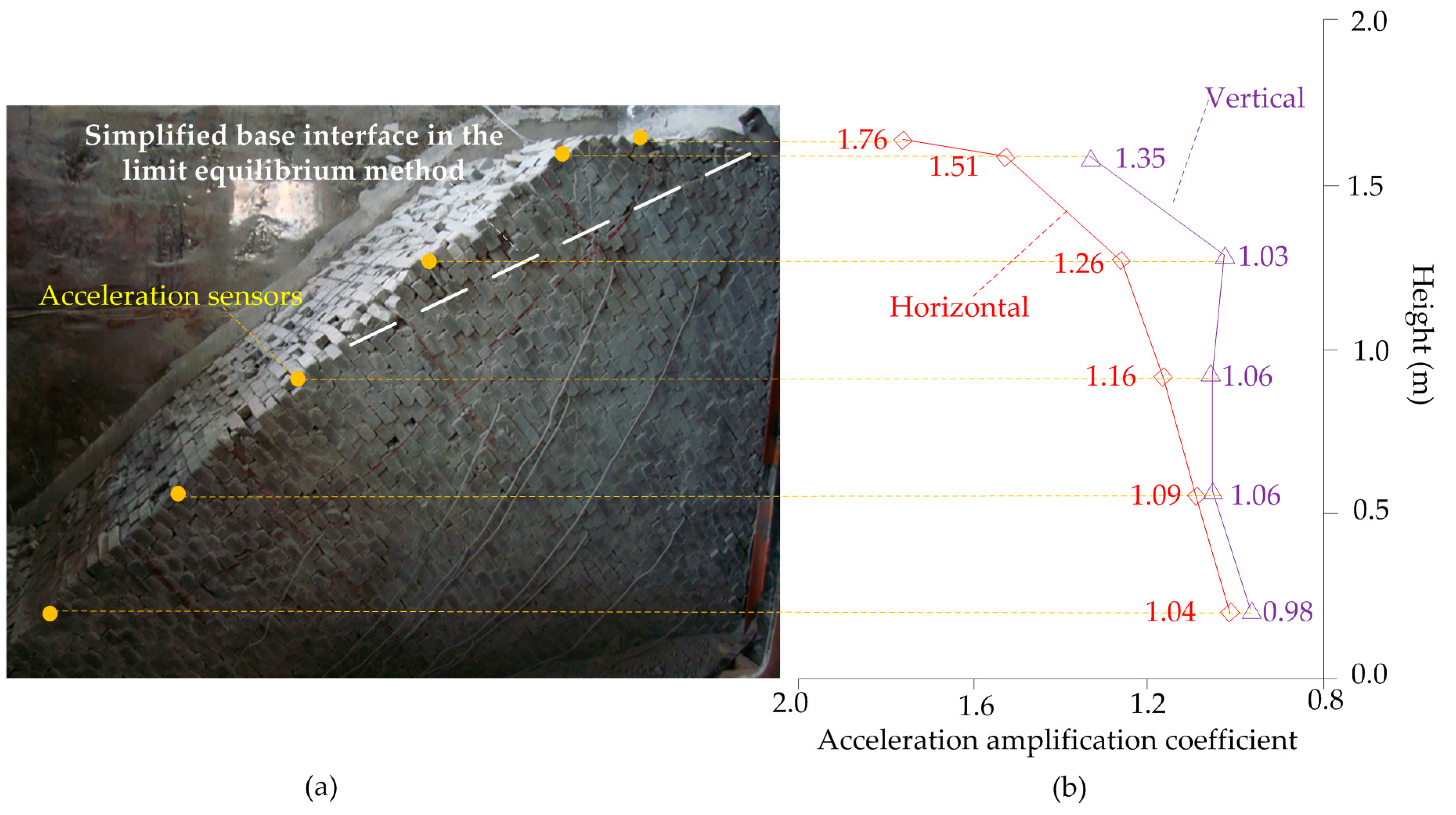
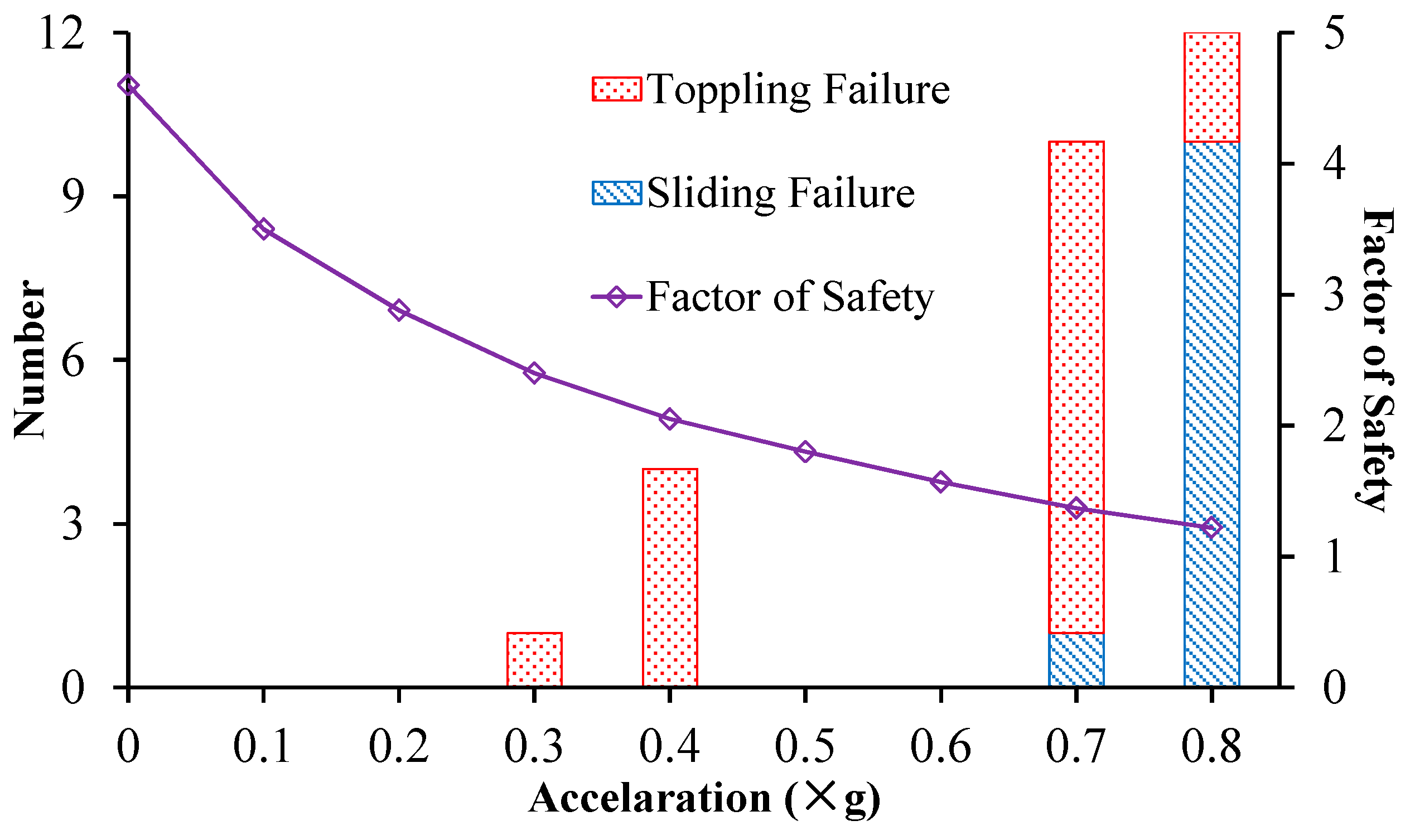
3. An Application Research on an Anti-Dip Rock Slope Model under Shake Table Test
- (1)
- As the inputting acceleration (horizontal direction) increased to 0.3 g, some flexible toppling deformation initiated around the slope crest.
- (2)
- As the inputting accelerations (both horizontal and vertical direction) increased to 0.3 g, the number of toppling blocks increased a little and several blocks fell down.
- (3)
- As the inputting acceleration (horizontal direction) increased to 0.4 g, the toppling deformation was observed obviously and continued to develop noticeably around the slope crest.
- (4)
- As the inputting acceleration (horizontal direction) increased to 0.7 g, shear sliding deformation initiated in the slope, i.e., a curved-linear sliding surface was observed.
- (5)
- As the inputting acceleration (horizontal direction) increased to 0.8 g, the sliding surface propagated to nearly go through the crest area and some cracks parallel to the sliding surface were observed in the slope (Figure 6a).
- (1)
- (2)
- As the accelerations (both horizontal and vertical direction) increased to 0.3 g, two toppling blocks were estimated (Table 4b).
- (3)
- (4)
- (5)
4. Discussion
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Abbreviations
| H | Slope height |
| n | The number of the blocks (the block at the slope toe is numbered as 1 and n = 1, 2 …) |
| yn | The height of nth block |
| The width of block | |
| The dip of block base | |
| The dip of the orthogonal planes forming the faces of the blocks | |
| The overall dip of base plane | |
| The slope angle under the crest | |
| The slope angle above the crest | |
| The height difference at the top of adjacent blocks under the slope crest | |
| The height difference at the top of adjacent blocks above the slope crest | |
| The height difference at the base of adjacent blocks | |
| Wn | The weight of nth block |
| Pn | The normal force of (n + 1)th block to nth block |
| Qn | The shear force of (n + 1)th block to nth block |
| Pn−1 | The normal force of (n − 1)th block to nth block |
| Qn−1 | The shear force of (n − 1)th block to nth block |
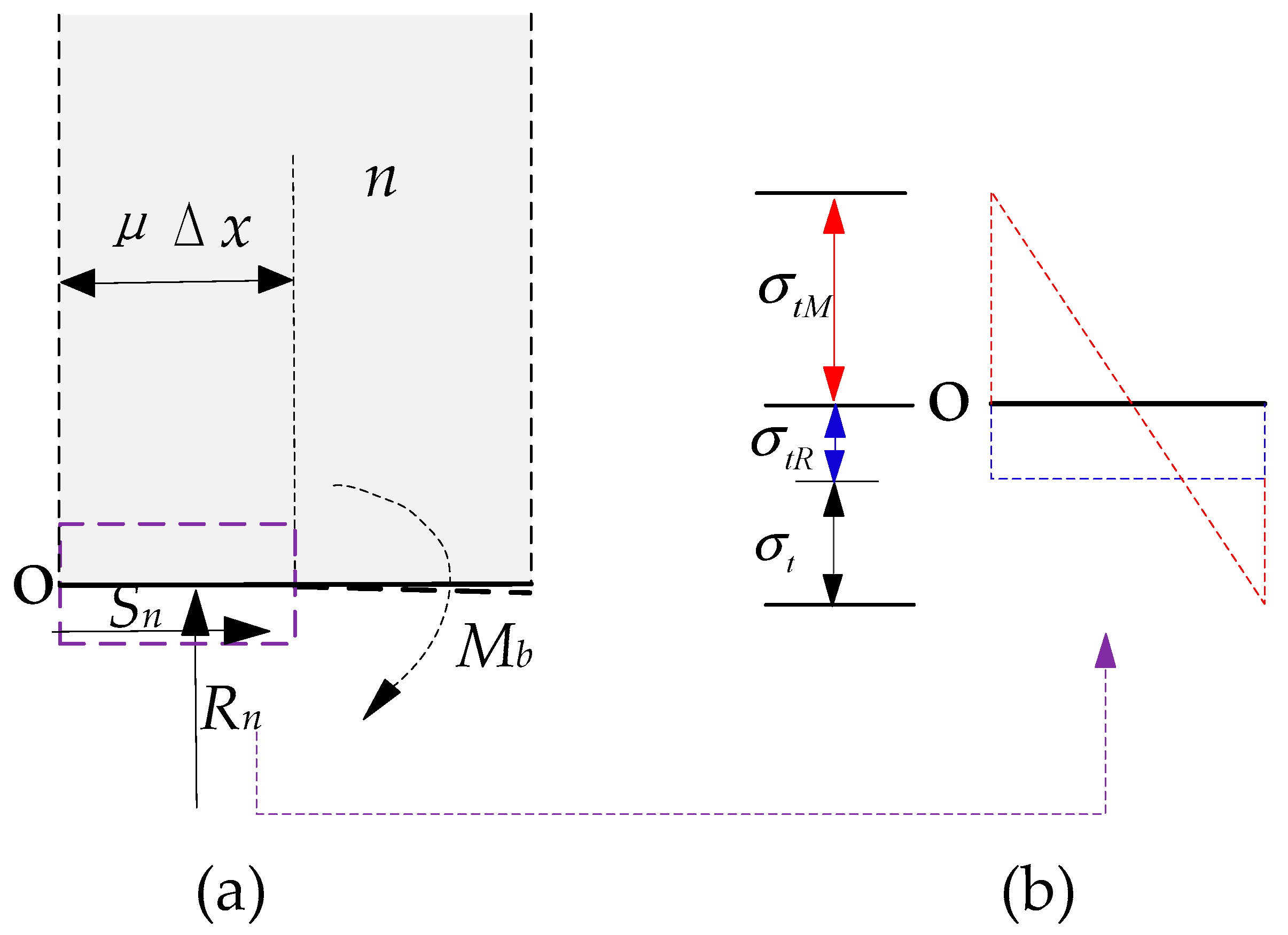
| σtM | The maximum tensile stress induced by moment Mb |
| σtR | The compressive stress induced byRn |
| Rn | The normal force of base to nth block |
| Sn | The shear force of base to nth block |
| Mn | The length from the point Pn to the base for the toppling block |
| Ln | The length from the point Pn−1 to the base for the toppling block |
| Mb | The moment acting at the base of the toppling blocks |
| Jc | The joint connectivity rate of the block base |
| 1 − Jc | |
| k1 | Seismic acceleration component parallel to the block base |
| k2 | Seismic acceleration component orthogonal to the block base |
| kx | Seismic acceleration component in horizontal direction |
| ky | Seismic acceleration component in vertical direction |
| g | Gravitational acceleration |
| φjs | Friction angle of block side |
| φjb | Friction angle of block base |
| φb | Internal friction angle of block |
| cb | Cohesion of block |
| σbt | Tensile strength of block |
| ρ | Density of block |
Appendix A
References
- Chen, Z.; Wang, X.; Yang, J.; Jia, Z.; Wang, Y. Rock Slope Stability Analysis–Theory, Methods and Programs; China Water & Power Press: Beijing, China, 2005. [Google Scholar]
- Zhang, Z.; Liu, G.; Wu, S.; Tang, H.; Wang, T.; Li, G.; Liang, C. Rock slope deformation mechanism in the Cihaxia Hydropower Station, Northwest China. Bull. Eng. Geol. Environ. 2015, 74, 943–958. [Google Scholar] [CrossRef]
- Gu, D.; Huang, D. A complex rock topple-rock slide failure of an anticlinal rock slope in the Wu Gorge, Yangtze River, China. Eng. Geol. 2016, 208, 165–180. [Google Scholar] [CrossRef]
- Alejano, L.; Gómez-Márquez, I.; Martínez-Alegría, R. Analysis of a complex toppling-circular slope failure. Eng. Geol. 2010, 114, 93–104. [Google Scholar] [CrossRef]
- Wyllie, D.C. Toppling rock slope failures examples of analysis and stabilization. Rock Mech. 1980, 13, 89–98. [Google Scholar] [CrossRef]
- De Freitas, M.H.; Watters, R.J. Some field examples of toppling failure. Geotechnique 1973, 23, 495–513. [Google Scholar] [CrossRef]
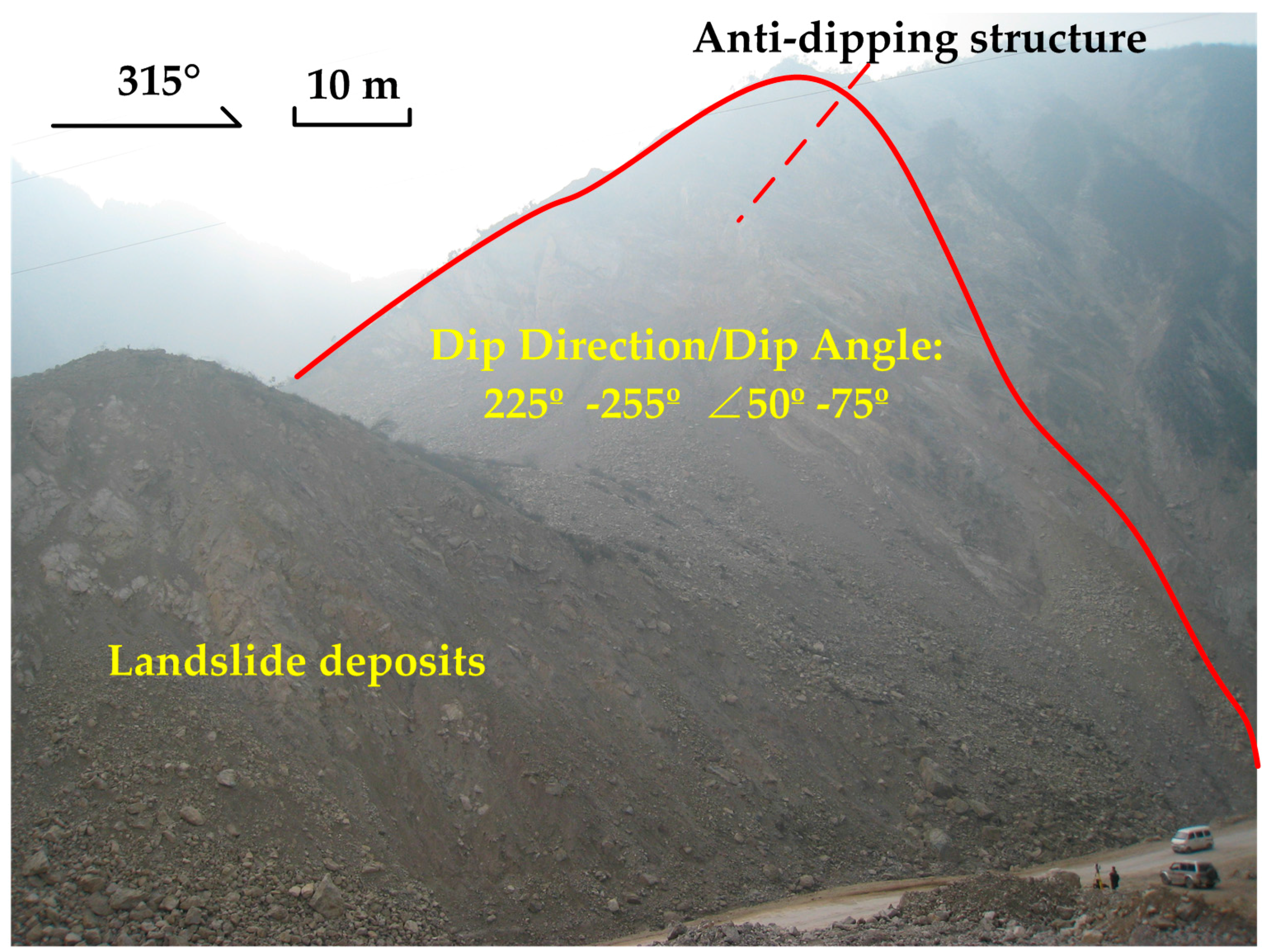
- Huang, R.; Zhao, J.; Ju, N.; Li, G.; Lee, M.; Li, Y. Analysis of an anti-dip landslide triggered by the 2008 Wenchuan earthquake in China. Nat. Hazards 2013, 68, 1021–1039. [Google Scholar] [CrossRef]
- Goodman, R.E.; Bray, J.W. Toppling of rock slopes. In Rock Engineering for Foundations & Slopes; ASCE: Reston, VA, USA, 1976; pp. 201–234. [Google Scholar]
- Zanbak, C. Design charts for rock slopes susceptible to toppling. J. Geotech. Eng. 1983, 109, 1039–1062. [Google Scholar] [CrossRef]
- Bobet, A. Analytical solutions for toppling failure. Int. J. Rock Mech. Min. Sci. 1999, 36, 971–980. [Google Scholar] [CrossRef]
- Sagaseta, C.; Sanchez, J.; Canizal, J. A general analytical solution for the required anchor force in rock slopes with toppling failure. Int. J. Rock Mech. Min. Sci. 2001, 38, 421–435. [Google Scholar] [CrossRef]
- Liu, C.; Jaksa, M.; Meyers, A. Improved analytical solution for toppling stability analysis of rock slopes. Int. J. Rock Mech. Min. Sci. 2008, 45, 1361–1372. [Google Scholar] [CrossRef]
- Liu, C.; Chen, C. Analysis of toppling failure of rock slopes of rock slopes due to earthquakes. Chin. J. Rock Mech. Eng. 2010, 29, 3193–3198. [Google Scholar]
- Aydan, Ö.; Kawamoto, T. The stability of slopes and underground openings against flexural toppling and their stabilisation. Rock Mech. Rock Eng. 1992, 25, 143–165. [Google Scholar] [CrossRef]
- Adhikary, D.; Dyskin, A.; Jewell, R.; Stewart, D.P. A study of the mechanism of flexural toppling failure of rock slopes. Rock Mech. Rock Eng. 1997, 30, 75–93. [Google Scholar] [CrossRef]
- Majdi, A.; Amini, M. Analysis of geo-structural defects in flexural toppling failure. Int. J. Rock Mech. Min. Sci. 2011, 48, 175–186. [Google Scholar] [CrossRef]
- Yagoda-Biran, G.; Hatzor, Y. A new failure mode chart for toppling and sliding with consideration of earthquake inertia force. Int. J. Rock Mech. Min. Sci. 2013, 64, 122–131. [Google Scholar] [CrossRef]
- Zheng, Y.; Chen, C.; Zhu, X.; Liu, T.T.; Liu, X.M.; Song, Y.F.; Zhou, Y.C. Analysis of toppling failure of rock slopes subjected to seismic loads. Rock Soil Mech. 2014, 35, 1025–1032. [Google Scholar]
- Maiorano, R.M.S.; Adinolfi, M.; Aversa, S. Rocking of slender rock blocks under seismic excitation. Ital. Heotech. J. 2015, 49, 87–99. [Google Scholar]
- Zhang, Z.; Wang, T.; Wu, S.; Tang, H. Rock toppling failure mode influenced by local response to earthquakes. Bull. Eng. Geol. Environ. 2016, 75, 1361–1375. [Google Scholar] [CrossRef]
- ROCSIENCE INC. RocTopple Version 1.0-Toppling Analysis of Rock Slopes; ROCSIENCE INC.: Toronto, ON, Canada, 2014. Available online: www.rocscience.com (accessed on 8 July 2017).
- Wyllie, D.C.; Mah, C. Rock Slope Engineering; CRC Press: Boca Raton, FL, USA, 2004. [Google Scholar]
- Pritchard, M.; Savigny, K. Numerical modelling of toppling. Can. Geotech. J. 1990, 27, 823–834. [Google Scholar] [CrossRef]
- Alzo’ubi, A.; Martin, C.; Cruden, D. Influence of tensile strength on toppling failure in centrifuge tests. Int. J. Rock Mech. Min. Sci. 2010, 47, 974–982. [Google Scholar] [CrossRef]
- Chen, Z.; Gong, W.; Ma, G.; Wang, J.; He, L.; Xing, Y.C.; Xing, J.Y. Comparisons between centrifuge and numerical modeling results for slope toppling failure. Sci. China Technol. Sci. 2015, 58, 1497–1508. [Google Scholar] [CrossRef]
- Yang, G.; Ye, H.; Wu, F.; Qi, S.; Dong, J. Shaking table model test on dynamic response characteristics and failure mechanism of anti-dip layered rock slope. Chin. J. Rock Mech. Eng. 2012, 31, 2214–2221. [Google Scholar]
- Qi, S.; Wu, F.Q.; Sun, J.Z. General regularity of dynamic responses of slopes under dynamic input. Sci. China Ser. E 2003, 46, 120–132. [Google Scholar] [CrossRef]
- Bi, Z.W.; Zhang, M.; Jin, F.; Ding, D.X. Dynamic response of slopes under earthquakes. Rock Soil Mech. 2009, 30, 180–183. [Google Scholar]
- Huang, R.; Li, G.; Ju, N. Shaking table test on strong earthquake response of stratified rock slopes. Chin. J. Rock Mech. Eng. 2013, 32, 865–876. [Google Scholar]
- Lanaro, F.; Jing, L.; Stephansson, O.; Barla, G. DEM modelling of laboratory tests of block toppling. Int. J. Rock Mech. Min. Sci. 1997, 34, 209–218. [Google Scholar] [CrossRef]
- Xu, Q.; Pei, X.; Huang, R. Large-Scale Landslides Induced by Wenchuan Earthquake; Science Press: Beijing, China, 2009. (In Chinese) [Google Scholar]

| Calculating Results | Pn−1 | Deformation Status | ||
|---|---|---|---|---|
| Pn−1,t < 0 | Pn−1,s < 0 | - | 0 | stable |
| Pn−1,t > 0 | Pn−1,s < 0 | - | Pn−1,t | toppling |
| Pn−1,t > 0 | Pn−1,s > 0 | Pn−1,t > Pn−1,s | Pn−1,t | toppling |
| Pn−1,t < 0 | Pn−1,s > 0 | - | Pn−1,s | sliding |
| Pn−1,t > 0 | Pn−1,s > 0 | Pn−1,s > Pn−1,t | Pn−1,s | sliding |
| Parameter | Value | Parameter | Value |
|---|---|---|---|
| (m) | 0.04 | (°) | 40 |
| (m) | 0.007 | (°) | 30 |
| (m) | 0.0135 | (°) | 28 |
| (m) | −0.0015 | (°) | 11 |
| 0.51 | - | - |
| 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
|---|---|---|---|---|---|---|---|---|
| n | yn (cm) | Mn (cm) | Ln (cm) | Wn (N) | Pn,t (N) | Pn,s (N) | Pn (N) | MODE |
| 26 | 1.5 | 0.2 | 1.5 | 15.1 | 0.0 | 0.0 | 0.0 | stable |
| 25 | 2.7 | 1.4 | 2.7 | 27.1 | −390.2 | −225.3 | 0.0 | |
| 24 | 3.9 | 2.6 | 3.9 | 39.2 | −233.0 | −226.6 | 0.0 | |
| 23 | 5.1 | 3.8 | 5.1 | 51.2 | −165.9 | −227.8 | 0.0 | |
| 22 | 6.3 | 5.0 | 6.3 | 63.3 | −127.9 | −229.1 | 0.0 | |
| 21 | 7.5 | 6.2 | 7.5 | 75.3 | −102.7 | −230.4 | 0.0 | |
| 20 | 8.7 | 7.4 | 8.7 | 87.3 | −84.5 | −231.6 | 0.0 | |
| 19 | 9.9 | 8.6 | 9.9 | 99.4 | −70.3 | −232.9 | 0.0 | |
| 18 | 11.1 | 9.8 | 11.1 | 111.4 | −58.8 | −234.2 | 0.0 | |
| 17 | 12.3 | 11.0 | 12.3 | 123.5 | −49.1 | −235.4 | 0.0 | |
| 16 | 13.5 | 12.2 | 12.8 | 135.5 | −40.7 | −236.7 | 0.0 | |
| 15 | 12.7 | 12.7 | 12.0 | 127.0 | −35.1 | −238.0 | 0.0 | |
| 14 | 11.8 | 11.8 | 11.1 | 118.5 | −40.7 | −237.1 | 0.0 | |
| 13 | 11.0 | 11.0 | 10.3 | 109.9 | −46.8 | −236.2 | 0.0 | |
| 12 | 10.1 | 10.1 | 9.4 | 101.4 | −53.6 | −235.3 | 0.0 | |
| 11 | 9.3 | 9.3 | 8.6 | 92.9 | −61.3 | −234.4 | 0.0 | |
| 10 | 8.4 | 8.4 | 7.7 | 84.3 | −70.0 | −233.5 | 0.0 | |
| 9 | 7.6 | 7.6 | 6.9 | 75.8 | −80.1 | −232.6 | 0.0 | |
| 8 | 6.7 | 6.7 | 6.0 | 67.3 | −92.1 | −231.7 | 0.0 | |
| 7 | 5.9 | 5.9 | 5.2 | 58.7 | −106.9 | −230.8 | 0.0 | |
| 6 | 5.0 | 5.0 | 4.3 | 50.2 | −125.6 | −229.9 | 0.0 | |
| 5 | 4.2 | 4.2 | 3.5 | 41.7 | −150.7 | −229.0 | 0.0 | |
| 4 | 3.3 | 3.3 | 2.6 | 33.1 | −186.4 | −228.1 | 0.0 | |
| 3 | 2.5 | 2.5 | 1.8 | 24.6 | −242.4 | −227.2 | 0.0 | |
| 2 | 1.6 | 1.6 | 0.9 | 16.1 | −345.3 | −226.3 | 0.0 | |
| 1 | 0.8 | 0.8 | 0.1 | 7.5 | −603.4 | −225.4 | 0.0 |
| (a) kx = 0.3 g, ky = 0 | ||||||||
|---|---|---|---|---|---|---|---|---|
| n | yn (cm) | Mn (cm) | Ln (cm) | Wn (N) | Pn,t(N) | Pn,s (N) | Pn (N) | MODE |
| 26 | 1.5 | 0.2 | 1.5 | 15.1 | 0.0 | 0.0 | 0.0 | Stable |
| 25 | 2.7 | 1.4 | 2.7 | 27.1 | −362.9 | −212.3 | 0.0 | |
| 24 | 3.9 | 2.6 | 3.9 | 39.2 | −210.8 | −203.2 | 0.0 | |
| 23 | 5.1 | 3.8 | 5.1 | 51.2 | −144.7 | −194.1 | 0.0 | |
| 22 | 6.3 | 5.0 | 6.3 | 63.3 | −106.2 | −185.0 | 0.0 | |
| 21 | 7.5 | 6.2 | 7.5 | 75.3 | −79.9 | −175.8 | 0.0 | |
| 20 | 8.7 | 7.4 | 8.7 | 87.3 | −60.2 | −166.7 | 0.0 | |
| 19 | 9.9 | 8.6 | 9.9 | 99.4 | −44.3 | −157.6 | 0.0 | |
| 18 | 11.1 | 9.8 | 11.1 | 111.4 | −30.9 | −148.5 | 0.0 | |
| 17 | 12.3 | 11.0 | 12.3 | 123.5 | −19.3 | −139.4 | 0.0 | |
| 16 | 13.5 | 12.2 | 12.8 | 135.5 | −8.8 | −130.3 | 0.0 | |
| 15 | 12.7 | 12.7 | 12.0 | 127.0 | 0.8 | −121.1 | 0.8 | Toppling |
| 14 | 11.8 | 11.8 | 11.1 | 118.5 | −5.6 | −126.8 | 0.0 | Stable |
| 13 | 11.0 | 11.0 | 10.3 | 109.9 | −13.9 | −134.1 | 0.0 | |
| 12 | 10.1 | 10.1 | 9.4 | 101.4 | −22.0 | −140.5 | 0.0 | |
| 11 | 9.3 | 9.3 | 8.6 | 92.9 | −31.0 | −147.0 | 0.0 | |
| 10 | 8.4 | 8.4 | 7.7 | 84.3 | −41.0 | −153.4 | 0.0 | |
| 9 | 7.6 | 7.6 | 6.9 | 75.8 | −52.3 | −159.9 | 0.0 | |
| 8 | 6.7 | 6.7 | 6.0 | 67.3 | −65.4 | −166.3 | 0.0 | |
| 7 | 5.9 | 5.9 | 5.2 | 58.7 | −81.0 | −172.8 | 0.0 | |
| 6 | 5.0 | 5.0 | 4.3 | 50.2 | −100.4 | −179.3 | 0.0 | |
| 5 | 4.2 | 4.2 | 3.5 | 41.7 | −125.8 | −185.7 | 0.0 | |
| 4 | 3.3 | 3.3 | 2.6 | 33.1 | −161.2 | −192.2 | 0.0 | |
| 3 | 2.5 | 2.5 | 1.8 | 24.6 | −215.8 | −198.6 | 0.0 | |
| 2 | 1.6 | 1.6 | 0.9 | 16.1 | −314.9 | −205.1 | 0.0 | |
| 1 | 0.8 | 0.8 | 0.1 | 7.5 | −562.4 | −211.5 | 0.0 | |
| (b) kx = 0.3 g, ky = 0.3 g | ||||||||
| n | yn (cm) | Mn (cm) | Ln (cm) | Wn (N) | Pn,t(N) | Pn,s (N) | Pn (N) | MODE |
| 26 | 1.5 | 0.2 | 1.5 | 15.1 | 0.0 | 0.0 | 0.0 | Stable |
| 25 | 2.7 | 1.4 | 2.7 | 27.1 | −367.3 | −212.8 | 0.0 | |
| 24 | 3.9 | 2.6 | 3.9 | 39.2 | −214.6 | −204.1 | 0.0 | |
| 23 | 5.1 | 3.8 | 5.1 | 51.2 | −147.7 | −195.3 | 0.0 | |
| 22 | 6.3 | 5.0 | 6.3 | 63.3 | −108.3 | −186.6 | 0.0 | |
| 21 | 7.5 | 6.2 | 7.5 | 75.3 | −81.2 | −177.9 | 0.0 | |
| 20 | 8.7 | 7.4 | 8.7 | 87.3 | −60.6 | −169.1 | 0.0 | |
| 19 | 9.9 | 8.6 | 9.9 | 99.4 | −43.8 | −160.4 | 0.0 | |
| 18 | 11.1 | 9.8 | 11.1 | 111.4 | −29.6 | −151.7 | 0.0 | |
| 17 | 12.3 | 11.0 | 12.3 | 123.5 | −17.0 | −142.9 | 0.0 | |
| 16 | 13.5 | 12.2 | 12.8 | 135.5 | −5.7 | −134.2 | 0.0 | |
| 15 | 12.7 | 12.7 | 12.0 | 127.0 | 5.0 | −125.5 | 5.0 | Toppling |
| 14 | 11.8 | 11.8 | 11.1 | 118.5 | 1.5 | −126.6 | 1.5 | |
| 13 | 11.0 | 11.0 | 10.3 | 109.9 | −9.7 | −136.3 | 0.0 | Stable |
| 12 | 10.1 | 10.1 | 9.4 | 101.4 | −19.8 | −144.0 | 0.0 | |
| 11 | 9.3 | 9.3 | 8.6 | 92.9 | −29.4 | −150.2 | 0.0 | |
| 10 | 8.4 | 8.4 | 7.7 | 84.3 | −40.0 | −156.4 | 0.0 | |
| 9 | 7.6 | 7.6 | 6.9 | 75.8 | −52.0 | −162.6 | 0.0 | |
| 8 | 6.7 | 6.7 | 6.0 | 67.3 | −65.8 | −168.8 | 0.0 | |
| 7 | 5.9 | 5.9 | 5.2 | 58.7 | −82.1 | −174.9 | 0.0 | |
| 6 | 5.0 | 5.0 | 4.3 | 50.2 | −102.3 | −181.1 | 0.0 | |
| 5 | 4.2 | 4.2 | 3.5 | 41.7 | −128.3 | −187.3 | 0.0 | |
| 4 | 3.3 | 3.3 | 2.6 | 33.1 | −164.5 | −193.5 | 0.0 | |
| 3 | 2.5 | 2.5 | 1.8 | 24.6 | −220.0 | −199.7 | 0.0 | |
| 2 | 1.6 | 1.6 | 0.9 | 16.1 | −320.3 | −205.9 | 0.0 | |
| 1 | 0.8 | 0.8 | 0.1 | 7.5 | −569.5 | −212.1 | 0.0 | |
| (c) kx = 0.4 g, ky = 0 | ||||||||
| n | yn (cm) | Mn (cm) | Ln (cm) | Wn (N) | Pn,t(N) | Pn,s (N) | Pn (N) | MODE |
| 26 | 1.5 | 0.2 | 1.5 | 15.1 | 0.0 | 0.0 | 0.0 | Stable |
| 25 | 2.7 | 1.4 | 2.7 | 27.1 | −360.5 | −208.0 | 0.0 | |
| 24 | 3.9 | 2.6 | 3.9 | 39.2 | −207.5 | −195.4 | 0.0 | |
| 23 | 5.1 | 3.8 | 5.1 | 51.2 | −140.6 | −182.8 | 0.0 | |
| 22 | 6.3 | 5.0 | 6.3 | 63.3 | −101.3 | −170.2 | 0.0 | |
| 21 | 7.5 | 6.2 | 7.5 | 75.3 | −74.2 | −157.7 | 0.0 | |
| 20 | 8.7 | 7.4 | 8.7 | 87.3 | −53.7 | −145.1 | 0.0 | |
| 19 | 9.9 | 8.6 | 9.9 | 99.4 | −37.0 | −132.5 | 0.0 | |
| 18 | 11.1 | 9.8 | 11.1 | 111.4 | −22.9 | −119.9 | 0.0 | |
| 17 | 12.3 | 11.0 | 12.3 | 123.5 | −10.4 | −107.4 | 0.0 | |
| 16 | 13.5 | 12.2 | 12.8 | 135.5 | 0.8 | −94.8 | 0.8 | Toppling |
| 15 | 12.7 | 12.7 | 12.0 | 127.0 | 12.4 | −81.4 | 12.4 | |
| 14 | 11.8 | 11.8 | 11.1 | 118.5 | 14.5 | −78.7 | 14.5 | |
| 13 | 11.0 | 11.0 | 10.3 | 109.9 | 8.0 | −85.5 | 8.0 | |
| 12 | 10.1 | 10.1 | 9.4 | 101.4 | −6.3 | −101.0 | 0.0 | Stable |
| 11 | 9.3 | 9.3 | 8.6 | 92.9 | −22.2 | −117.8 | 0.0 | |
| 10 | 8.4 | 8.4 | 7.7 | 84.3 | −32.7 | −126.8 | 0.0 | |
| 9 | 7.6 | 7.6 | 6.9 | 75.8 | −44.6 | −135.7 | 0.0 | |
| 8 | 6.7 | 6.7 | 6.0 | 67.3 | −58.2 | −144.6 | 0.0 | |
| 7 | 5.9 | 5.9 | 5.2 | 58.7 | −74.4 | −153.5 | 0.0 | |
| 6 | 5.0 | 5.0 | 4.3 | 50.2 | −94.3 | −162.4 | 0.0 | |
| 5 | 4.2 | 4.2 | 3.5 | 41.7 | −120.2 | −171.3 | 0.0 | |
| 4 | 3.3 | 3.3 | 2.6 | 33.1 | −156.1 | −180.2 | 0.0 | |
| 3 | 2.5 | 2.5 | 1.8 | 24.6 | −211.2 | −189.1 | 0.0 | |
| 2 | 1.6 | 1.6 | 0.9 | 16.1 | −310.7 | −198.0 | 0.0 | |
| 1 | 0.8 | 0.8 | 0.1 | 7.5 | −558.3 | −206.9 | 0.0 | |
| (d) kx = 0.7 g, ky = 0 | ||||||||
| n | yn (cm) | Mn (cm) | Ln (cm) | Wn (N) | Pn,t(N) | Pn,s (N) | Pn (N) | MODE |
| 26 | 1.5 | 0.2 | 1.5 | 15.1 | 0.0 | 0.0 | 0.0 | Stable |
| 25 | 2.7 | 1.4 | 2.7 | 27.1 | −353.3 | −195.0 | 0.0 | |
| 24 | 3.9 | 2.6 | 3.9 | 39.2 | −197.7 | −172.1 | 0.0 | |
| 23 | 5.1 | 3.8 | 5.1 | 51.2 | −128.4 | −149.1 | 0.0 | |
| 22 | 6.3 | 5.0 | 6.3 | 63.3 | −86.6 | −126.2 | 0.0 | |
| 21 | 7.5 | 6.2 | 7.5 | 75.3 | −57.2 | −103.3 | 0.0 | |
| 20 | 8.7 | 7.4 | 8.7 | 87.3 | −34.3 | −80.3 | 0.0 | |
| 19 | 9.9 | 8.6 | 9.9 | 99.4 | −15.3 | −57.4 | 0.0 | |
| 18 | 11.1 | 9.8 | 11.1 | 111.4 | 1.2 | −34.4 | 1.2 | Toppling |
| 17 | 12.3 | 11.0 | 12.3 | 123.5 | 16.8 | −10.2 | 16.8 | |
| 16 | 13.5 | 12.2 | 12.8 | 135.5 | 41.0 | 28.3 | 41.0 | |
| 15 | 12.7 | 12.7 | 12.0 | 127.0 | 75.1 | 75.4 | 75.4 | Sliding |
| 14 | 11.8 | 11.8 | 11.1 | 118.5 | 98.4 | 93.6 | 98.4 | Toppling |
| 13 | 11.0 | 11.0 | 10.3 | 109.9 | 106.7 | 100.3 | 106.7 | |
| 12 | 10.1 | 10.1 | 9.4 | 101.4 | 101.6 | 92.3 | 101.6 | |
| 11 | 9.3 | 9.3 | 8.6 | 92.9 | 84.6 | 71.0 | 84.6 | |
| 10 | 8.4 | 8.4 | 7.7 | 84.3 | 57.3 | 37.7 | 57.3 | |
| 9 | 7.6 | 7.6 | 6.9 | 75.8 | 21.3 | −5.8 | 21.3 | |
| 8 | 6.7 | 6.7 | 6.0 | 67.3 | −21.4 | −58.0 | 0.0 | Stable |
| 7 | 5.9 | 5.9 | 5.2 | 58.7 | −54.5 | −95.6 | 0.0 | |
| 6 | 5.0 | 5.0 | 4.3 | 50.2 | −76.1 | −111.9 | 0.0 | |
| 5 | 4.2 | 4.2 | 3.5 | 41.7 | −103.5 | −128.1 | 0.0 | |
| 4 | 3.3 | 3.3 | 2.6 | 33.1 | −140.9 | −144.4 | 0.0 | |
| 3 | 2.5 | 2.5 | 1.8 | 24.6 | −197.4 | −160.6 | 0.0 | |
| 2 | 1.6 | 1.6 | 0.9 | 16.1 | −298.0 | −176.9 | 0.0 | |
| 1 | 0.8 | 0.8 | 0.1 | 7.5 | −546.0 | −193.1 | 0.0 | |
| (e) kx = 0.8 g, ky = 0 | ||||||||
| n | yn (cm) | Mn (cm) | Ln (cm) | Wn (N) | Pn,t(N) | Pn,s (N) | Pn (N) | MODE |
| 26 | 1.5 | 0.2 | 1.5 | 15.1 | 0.0 | 0.0 | 0.0 | Stable |
| 25 | 2.7 | 1.4 | 2.7 | 27.1 | −350.8 | −190.7 | 0.0 | |
| 24 | 3.9 | 2.6 | 3.9 | 39.2 | −194.4 | −164.3 | 0.0 | |
| 23 | 5.1 | 3.8 | 5.1 | 51.2 | −124.3 | −137.8 | 0.0 | |
| 22 | 6.3 | 5.0 | 6.3 | 63.3 | −81.7 | −111.4 | 0.0 | |
| 21 | 7.5 | 6.2 | 7.5 | 75.3 | −51.5 | −85.0 | 0.0 | |
| 20 | 8.7 | 7.4 | 8.7 | 87.3 | −27.8 | −58.6 | 0.0 | |
| 19 | 9.9 | 8.6 | 9.9 | 99.4 | −8.0 | −32.1 | 0.0 | |
| 18 | 11.1 | 9.8 | 11.1 | 111.4 | 9.3 | −5.7 | 9.3 | Toppling |
| 17 | 12.3 | 11.0 | 12.3 | 123.5 | 30.9 | 30.1 | 30.9 | |
| 16 | 13.5 | 12.2 | 12.8 | 135.5 | 60.3 | 78.1 | 78.1 | Sliding |
| 15 | 12.7 | 12.7 | 12.0 | 127.0 | 113.7 | 151.7 | 151.7 | |
| 14 | 11.8 | 11.8 | 11.1 | 118.5 | 172.6 | 206.5 | 206.5 | |
| 13 | 11.0 | 11.0 | 10.3 | 109.9 | 205.6 | 242.6 | 242.6 | |
| 12 | 10.1 | 10.1 | 9.4 | 101.4 | 221.0 | 260.0 | 260.0 | |
| 11 | 9.3 | 9.3 | 8.6 | 92.9 | 219.0 | 258.7 | 258.7 | |
| 10 | 8.4 | 8.4 | 7.7 | 84.3 | 200.1 | 238.7 | 238.7 | |
| 9 | 7.6 | 7.6 | 6.9 | 75.8 | 164.8 | 200.0 | 200.0 | |
| 8 | 6.7 | 6.7 | 6.0 | 67.3 | 113.9 | 142.5 | 142.5 | |
| 7 | 5.9 | 5.9 | 5.2 | 58.7 | 48.8 | 66.4 | 66.4 | |
| 6 | 5.0 | 5.0 | 4.3 | 50.2 | −28.3 | −28.5 | 0.0 | Stable |
| 5 | 4.2 | 4.2 | 3.5 | 41.7 | −97.9 | −113.6 | 0.0 | |
| 4 | 3.3 | 3.3 | 2.6 | 33.1 | −135.8 | −132.3 | 0.0 | |
| 3 | 2.5 | 2.5 | 1.8 | 24.6 | −192.7 | −151.0 | 0.0 | |
| 2 | 1.6 | 1.6 | 0.9 | 16.1 | −293.8 | −169.8 | 0.0 | |
| 1 | 0.8 | 0.8 | 0.1 | 7.5 | −541.9 | −188.5 | 0.0 | |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, S.; Qi, S.; Yang, G.; Zhang, S.; Saroglou, C. An Analytical Solution for Block Toppling Failure of Rock Slopes during an Earthquake. Appl. Sci. 2017, 7, 1008. https://doi.org/10.3390/app7101008
Guo S, Qi S, Yang G, Zhang S, Saroglou C. An Analytical Solution for Block Toppling Failure of Rock Slopes during an Earthquake. Applied Sciences. 2017; 7(10):1008. https://doi.org/10.3390/app7101008
Chicago/Turabian StyleGuo, Songfeng, Shengwen Qi, Guoxiang Yang, Shishu Zhang, and Charalampos Saroglou. 2017. "An Analytical Solution for Block Toppling Failure of Rock Slopes during an Earthquake" Applied Sciences 7, no. 10: 1008. https://doi.org/10.3390/app7101008
APA StyleGuo, S., Qi, S., Yang, G., Zhang, S., & Saroglou, C. (2017). An Analytical Solution for Block Toppling Failure of Rock Slopes during an Earthquake. Applied Sciences, 7(10), 1008. https://doi.org/10.3390/app7101008








