Complex Spatial Illumination Scheme Optimization of Backscattering Mueller Matrix Polarimetry for Tissue Imaging and Biosensing
Abstract
:1. Introduction
2. Materials and Methods
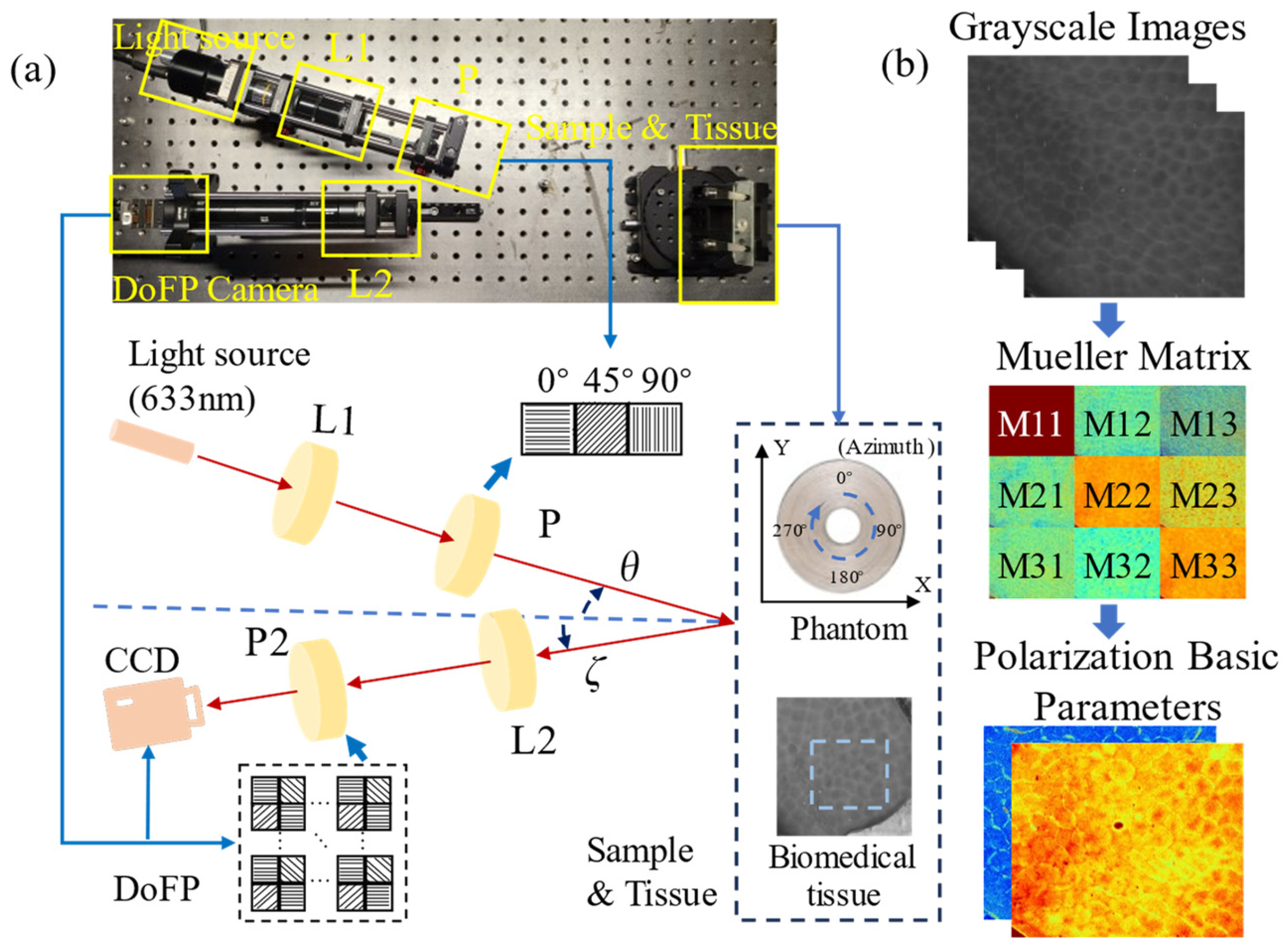
2.1. Experimental Setup and Tissue Samples
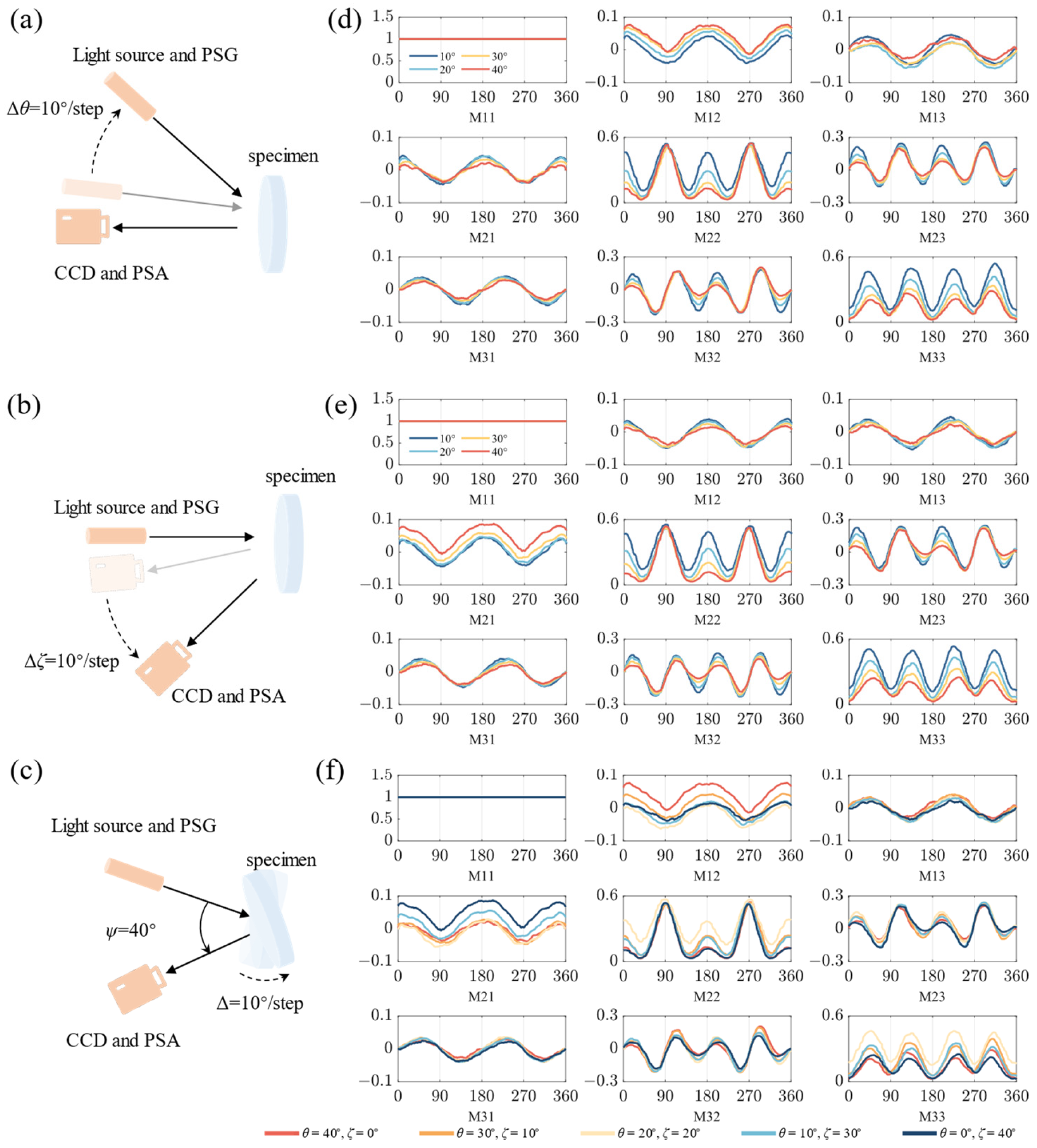
2.2. Azimuthal-Dependent Curves of Mueller Matrix Elements
2.3. Analysis Methods
3. Results
3.1. Aberrations Induced by Complex Spatial Illumination of Two-Periodic MM Elements
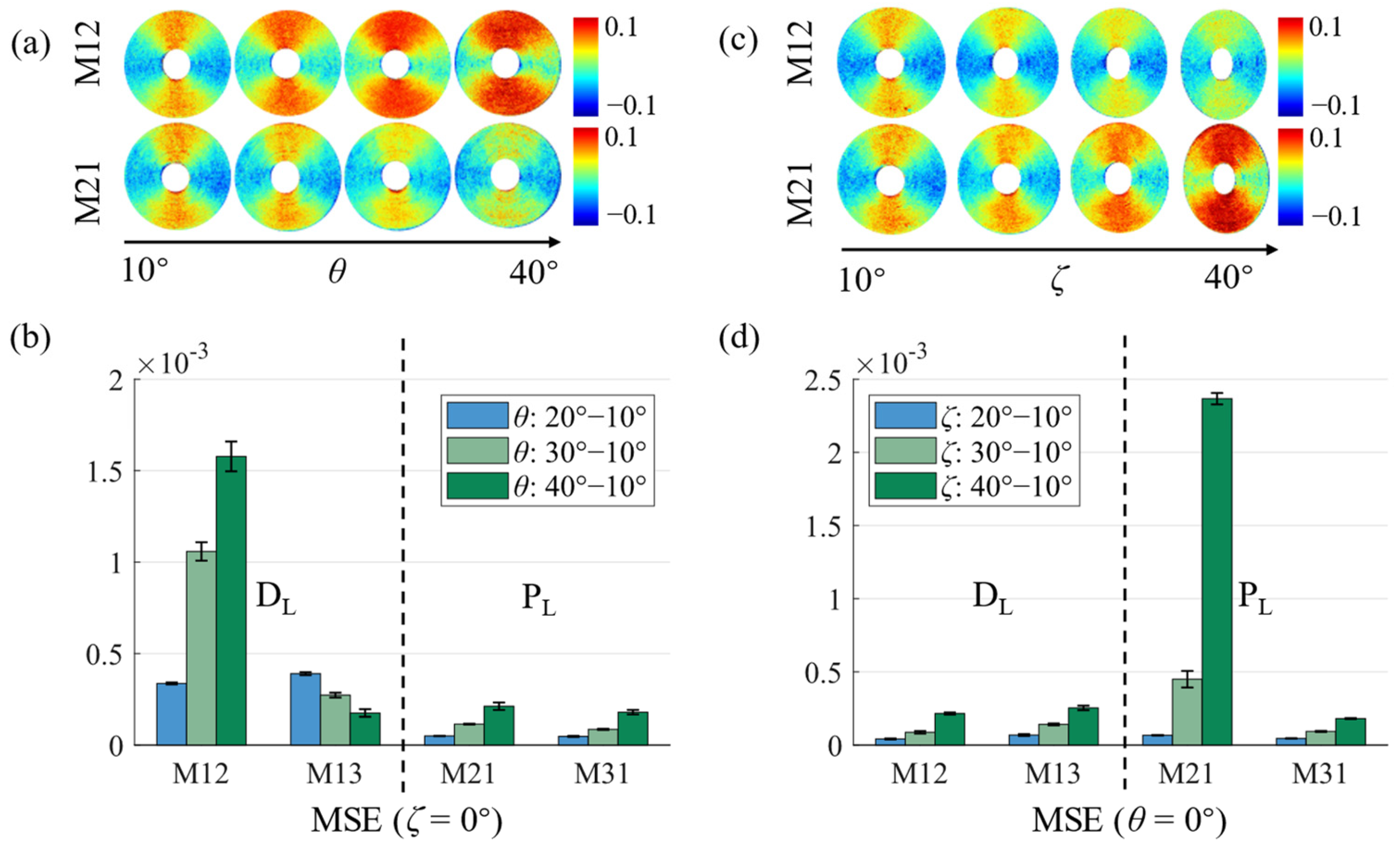
3.2. MM Image Distortions Induced by Oblique Emergent Light
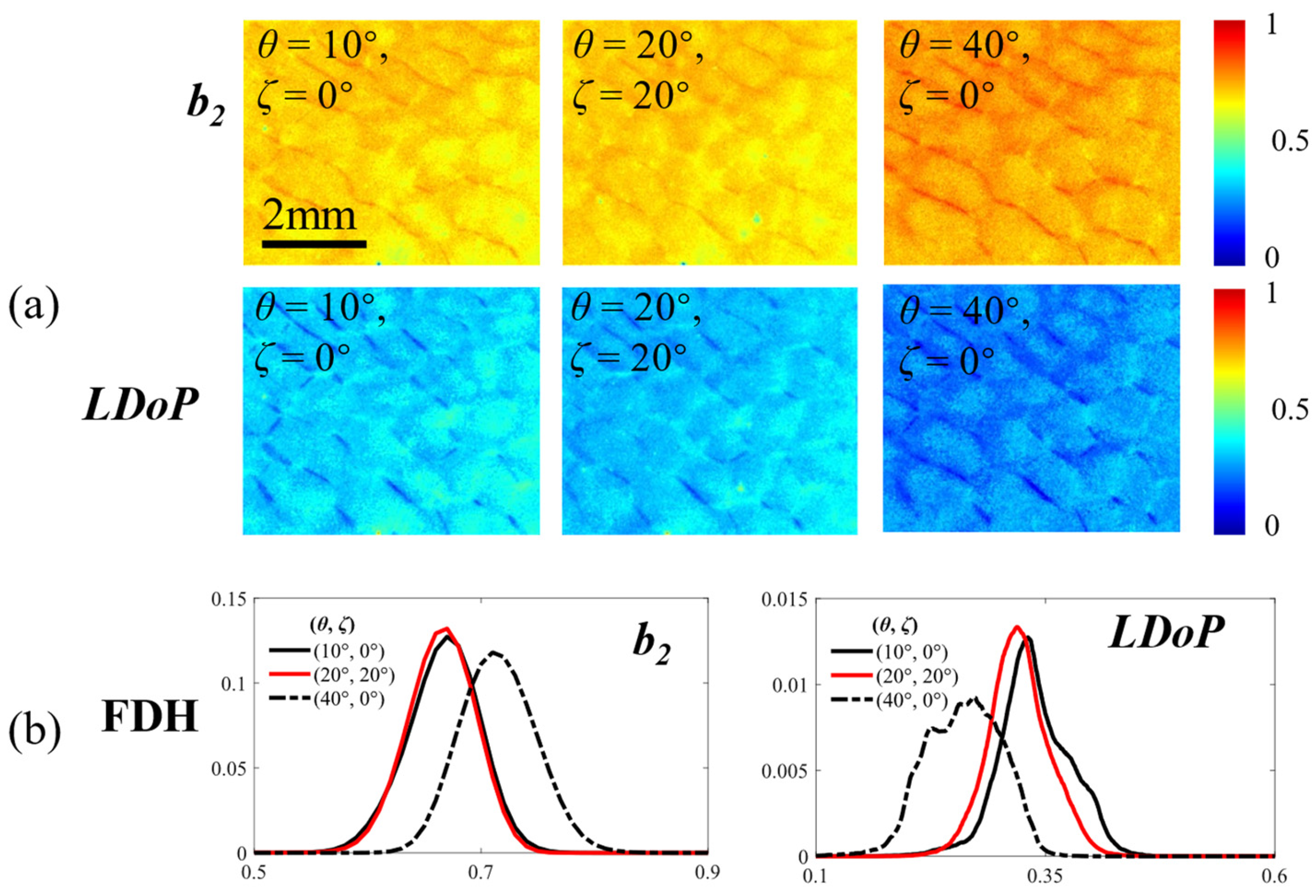
3.3. Calibration of Polarization Properties by Adjusting the Distribution of θ and ζ
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Ramella-Roman, J.C.; Novikova, T. Polarized Light in Biomedical Imaging and Sensing: Clinical and Preclinical Applications; Springer International Publishing: Cham, Switzerland, 2023. [Google Scholar]
- He, C.; He, H.; Chang, J.; Chen, B.; Ma, H.; Booth, M.J. Polarisation Optics for Biomedical and Clinical Applications: A Review. Light. Sci. Appl. 2021, 10, 194. [Google Scholar] [CrossRef] [PubMed]
- Tukimin, S.N.; Karman, S.B.; Ahmad, M.Y.; Wan Kamarul Zaman, W.S. Polarized Light-Based Cancer Cell Detection Techniques: A Review. IEEE Sens. J. 2019, 19, 9010. [Google Scholar] [CrossRef]
- Chue-Sang, J.; Gonzalez, M.; Pierre, A.; Laughrey, M.; Saytashev, I.; Novikova, T.; Ramella-Roman, J.C. Optical Phantoms for Biomedical Polarimetry: A Review. J. Biomed. Opt. 2019, 24, 030901. [Google Scholar] [CrossRef] [PubMed]
- Qi, J.; Elson, D.S. Mueller Polarimetric Imaging for Surgical and Diagnostic Applications: A Review. J. Biophotonics 2017, 10, 950. [Google Scholar] [CrossRef] [PubMed]
- Alali, S.; Vitkin, A. Polarized Light Imaging in Biomedicine: Emerging Mueller Matrix Methodologies for Bulk Tissue Assessment. J. Biomed. Opt. 2015, 20, 061104. [Google Scholar] [CrossRef] [PubMed]
- Lu, S.Y.; Chipman, R.A. Interpretation of Mueller Matrices Based on Polar Decomposition. J. Opt. Soc. Am. A 1996, 13, 1106. [Google Scholar] [CrossRef]
- Ossikovski, R. Analysis of Depolarizing Mueller Matrices through a Symmetric Decomposition. J. Opt. Soc. Am. A 2009, 26, 1109. [Google Scholar] [CrossRef] [PubMed]
- Ossikovski, R. Differential Matrix Formalism for Depolarizing Anisotropic Media. Opt. Lett. 2011, 36, 2330. [Google Scholar] [CrossRef] [PubMed]
- Ortega-Quijano, N.; Arce-Diego, J.L. Mueller Matrix Differential Decomposition. Opt. Lett. 2011, 36, 1942. [Google Scholar] [CrossRef] [PubMed]
- Ahmad, I.; Ahmad, M.; Khan, K.; Ashraf, S.; Ahmad, S.; Ikram, M. Ex Vivo Characterization of Normal and Adenocarcinoma Colon Samples by Mueller Matrix Polarimetry. J. Biomed. Opt. 2015, 20, 056012. [Google Scholar] [CrossRef] [PubMed]
- Yasuda, M.; Akimoto, T. High-Contrast Fluorescence Microscopy for a Biomolecular Analysis Based on Polarization Techniques Using an Optical Interference Mirror Slide. Biosensors 2014, 4, 513. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Hao, R.; Shao, C.; Mi, C.; He, H.; He, C.; Du, E.; Liu, S.; Wu, J.; Ma, H. Analysis and Optimization of Aberration Induced by Oblique Incidence for In-Vivo Tissue Polarimetry. Opt. Lett. 2023, 48, 6136. [Google Scholar] [CrossRef] [PubMed]
- Shang, L.; Tang, J.; Wu, J.; Shang, H.; Huang, X.; Bao, Y.; Xu, Z.; Wang, H.; Yin, J. Polarized Micro-Raman Spectroscopy and 2D Convolutional Neural Network Applied to Structural Analysis and Discrimination of Breast Cancer. Biosensors 2023, 13, 65. [Google Scholar] [CrossRef] [PubMed]
- Phan, Q.H.; Han, C.Y.; Lien, C.H.; Pham, T.T.H. Dual-Retarder Mueller Polarimetry System for Extraction of Optical Properties of Serum Albumin Protein Media. Sensors 2021, 21, 3442. [Google Scholar] [CrossRef] [PubMed]
- Manhas, S.; Vizet, J.; Deby, S.; Vanel, J.C.; Boito, P.; Verdier, M.; Martino, A.D.; Pagnoux, D. Demonstration of Full 4 × 4 Mueller Polarimetry through an Optical Fiber for Endoscopic Applications. Opt. Express 2015, 23, 3047. [Google Scholar] [CrossRef] [PubMed]
- Trout, R.M.; Gnanatheepam, E.; Gado, A.; Reik, C.; Ramella-Roman, J.C.; Hunter, M.; Schnelldorfer, T.; Georgakoudi, I. Polarization Enhanced Laparoscope for Improved Visualization of Tissue Structural Changes Associated with Peritoneal Cancer Metastasis. Biomed. Opt. Express 2022, 13, 571. [Google Scholar] [CrossRef] [PubMed]
- Bargo, P.R.; Kollias, N. Measurement of Skin Texture through Polarization Imaging: Measurement of Skin Texture through Polarization Imaging. Brit. J. Dermatol. 2010, 162, 724. [Google Scholar] [CrossRef] [PubMed]
- Rey-Barroso, L.; Peña-Gutiérrez, S.; Yáñez, C.; Burgos-Fernández, F.J.; Vilaseca, M.; Royo, S. Optical Technologies for the Improvement of Skin Cancer Diagnosis: A Review. Sensors 2021, 21, 252. [Google Scholar] [CrossRef] [PubMed]
- Jütte, L.; Roth, B. Mueller Matrix Microscopy for In Vivo Scar Tissue Diagnostics and Treatment Evaluation. Sensors 2022, 22, 9349. [Google Scholar] [CrossRef] [PubMed]
- Ning, T.; Ma, X.; Li, Y.; Li, Y.; Liu, K. Efficient Acquisition of Mueller Matrix via Spatially Modulated Polarimetry at Low Light Field. Opt. Express 2023, 31, 14532. [Google Scholar] [CrossRef] [PubMed]
- Hu, Z.; Ushenko, Y.A.; Soltys, I.V.; Dubolazov, O.V.; Gorsky, M.P.; Olar, O.V.; Trifonyuk, L.Y. Analytical Review of the Methods of Multifunctional Digital Mueller-Matrix Laser Polarimetry. In Mueller-Matrix Tomography of Biological Tissues and Fluids; Springer: Singapore, 2024; pp. 1–11. [Google Scholar]
- Chen, Z.; Meng, R.; Zhu, Y.; Ma, H. A Collinear Reflection Mueller Matrix Microscope for Backscattering Mueller Matrix Imaging. Opt. Lasers Eng. 2020, 129, 106055. [Google Scholar] [CrossRef]
- Phan, Q.H.; Dinh, Q.H.; Pan, Y.C.; Huang, Y.T.; Hong, Z.H. Decomposition Mueller Matrix Polarimetry for Enhanced miRNA Detection with Antimonene-Based Surface Plasmon Resonance Sensor and DNA-Linked Gold Nanoparticle Signal Amplification. Talanta 2024, 270, 125611. [Google Scholar] [CrossRef] [PubMed]
- Ho, H.P.; Law, W.C.; Wu, S.Y.; Liu, X.H.; Wong, S.P.; Lin, C.; Kong, S.K. Phase-Sensitive Surface Plasmon Resonance Biosensor Using the Photoelastic Modulation Technique. Sens. Actuator B-Chem. 2006, 114, 80. [Google Scholar] [CrossRef]
- Phan, Q.H.; Lo, Y.L. Stokes-Mueller Matrix Polarimetry System for Glucose Sensing. Opt. Lasers Eng. 2017, 92, 120. [Google Scholar] [CrossRef]
- Phan, Q.-H.; Jian, T.H.; Huang, Y.R.; Lai, Y.R.; Xiao, W.Z.; Chen, S.W. Combination of Surface Plasmon Resonance and Differential Mueller Matrix Formalism for Noninvasive Glucose Sensing. Opt. Lasers Eng. 2020, 134, 106268. [Google Scholar] [CrossRef]
- Le, H.M.; Le, T.H.; Phan, Q.H.; Pham, T.T.H. Mueller Matrix Imaging Polarimetry Technique for Dengue Fever Detection. Opt. Commun. 2022, 502, 127420. [Google Scholar] [CrossRef]
- Qi, J.; Elson, D.S.; Stoyanov, D. Eigenvalue Calibration Method for 3 × 3 Mueller Polarimeters. Opt. Lett. 2019, 44, 2362. [Google Scholar] [CrossRef] [PubMed]
- Khaliq, A.; Ashraf, S.; Gul, B.; Ahmad, I. Comparative Study of 3 × 3 Mueller Matrix Transformation and Polar Decomposition. Opt. Commun. 2021, 485, 126756. [Google Scholar] [CrossRef]
- Nguyen, T.V.; Nguyen, T.H.; Nguyen, N.B.T.; Huynh, C.K.; Le, T.H.; Phan, Q.H.; Pham, T.T.H. Evaluation of Optical Features of Fibronectin Fibrils by Backscattering Polarization Imaging. Optik 2023, 272, 170304. [Google Scholar] [CrossRef]
- Iqbal, M.; Ahmad, I.; Khaliq, A.; Khan, S. Comparative Study of Mueller Matrix Transformation and Polar Decomposition for Optical Characterization of Turbid Media. Optik 2020, 224, 165508. [Google Scholar] [CrossRef]
- Gao, W. Independent Differential Depolarization Parameters and Their Physical Interpretations. Opt. Laser Technol. 2023, 161, 109156. [Google Scholar] [CrossRef]
- Wang, Z.; Bovik, A.C.; Sheikh, H.R.; Simoncelli, E.P. Image Quality Assessment: From Error Visibility to Structural Similarity. IEEE Trans. Image Process. 2004, 13, 600. [Google Scholar] [CrossRef] [PubMed]

| Degrees | ||||
|---|---|---|---|---|
| θ = 10°, ζ = 0° | 0.87 | 0.38 | 0.30 | 0.13 |
| θ = 20°, ζ = 0° | 40.83 | 25.66 | 0.29 | 0.0080 |
| θ = 30°, ζ = 0° | 119.83 | 12.61 | 0.07 | 0.03 |
| θ = 40°, ζ = 0° | 173.45 | 2.49 | 2.42 | 0.01 |
| θ = 0°, ζ = 10° | 0.89 | 1.04 | 0.68 | 0.10 |
| θ = 0°, ζ = 20° | 3.11 | 2.98 | 4.27 | 0.19 |
| θ = 0°, ζ = 30° | 4.13 | 0.29 | 48.64 | 1.13 |
| θ = 0°, ζ = 40° | 5.87 | 3.81 | 252.78 | 4.24 |
| Different Degrees | Intercept | X Variable | Significance F | p-Value |
|---|---|---|---|---|
| θ (ζ = 0°) | 0.0066 | 0.000013 | 0.92 | 0.21 |
| θ (ζ = 10°) | 0.028 | −0.00012 | 0.80 | 0.070 |
| θ (ζ = 20°) | 0.070 | 0.00080 | 0.30 | 0.052 |
| ζ (θ = 0°) | 0.95 | −0.93 | 0.0014 | 0.0011 |
| ζ (θ = 10°) | 1.091 | −1.08 | 0.0058 | 0.0051 |
| ζ (θ = Mean) | 0.98 | −0.96 | 0.000054 | 0.000040 |
| Similarity Metrics | b2 (θ, ζ) | LDoP (θ, ζ) | ||
|---|---|---|---|---|
| (20°, 20°) | (40°, 0°) | (20°, 20°) | (40°, 0°) | |
| HD (×10−6) | 0.16 | 10.83 | 1.29 | 10.67 |
| Euclidean norm | 11.53 | 49.67 | 19.96 | 79.15 |
| SSIM | 0.90 | 0.88 | 0.81 | 0.75 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jiao, W.; Zhang, Z.; Zeng, N.; Hao, R.; He, H.; He, C.; Ma, H. Complex Spatial Illumination Scheme Optimization of Backscattering Mueller Matrix Polarimetry for Tissue Imaging and Biosensing. Biosensors 2024, 14, 208. https://doi.org/10.3390/bios14040208
Jiao W, Zhang Z, Zeng N, Hao R, He H, He C, Ma H. Complex Spatial Illumination Scheme Optimization of Backscattering Mueller Matrix Polarimetry for Tissue Imaging and Biosensing. Biosensors. 2024; 14(4):208. https://doi.org/10.3390/bios14040208
Chicago/Turabian StyleJiao, Wei, Zheng Zhang, Nan Zeng, Rui Hao, Honghui He, Chao He, and Hui Ma. 2024. "Complex Spatial Illumination Scheme Optimization of Backscattering Mueller Matrix Polarimetry for Tissue Imaging and Biosensing" Biosensors 14, no. 4: 208. https://doi.org/10.3390/bios14040208






