Controlling the Polymer Ink’s Rheological Properties to Form Single and Stable Droplet
Abstract
1. Introduction
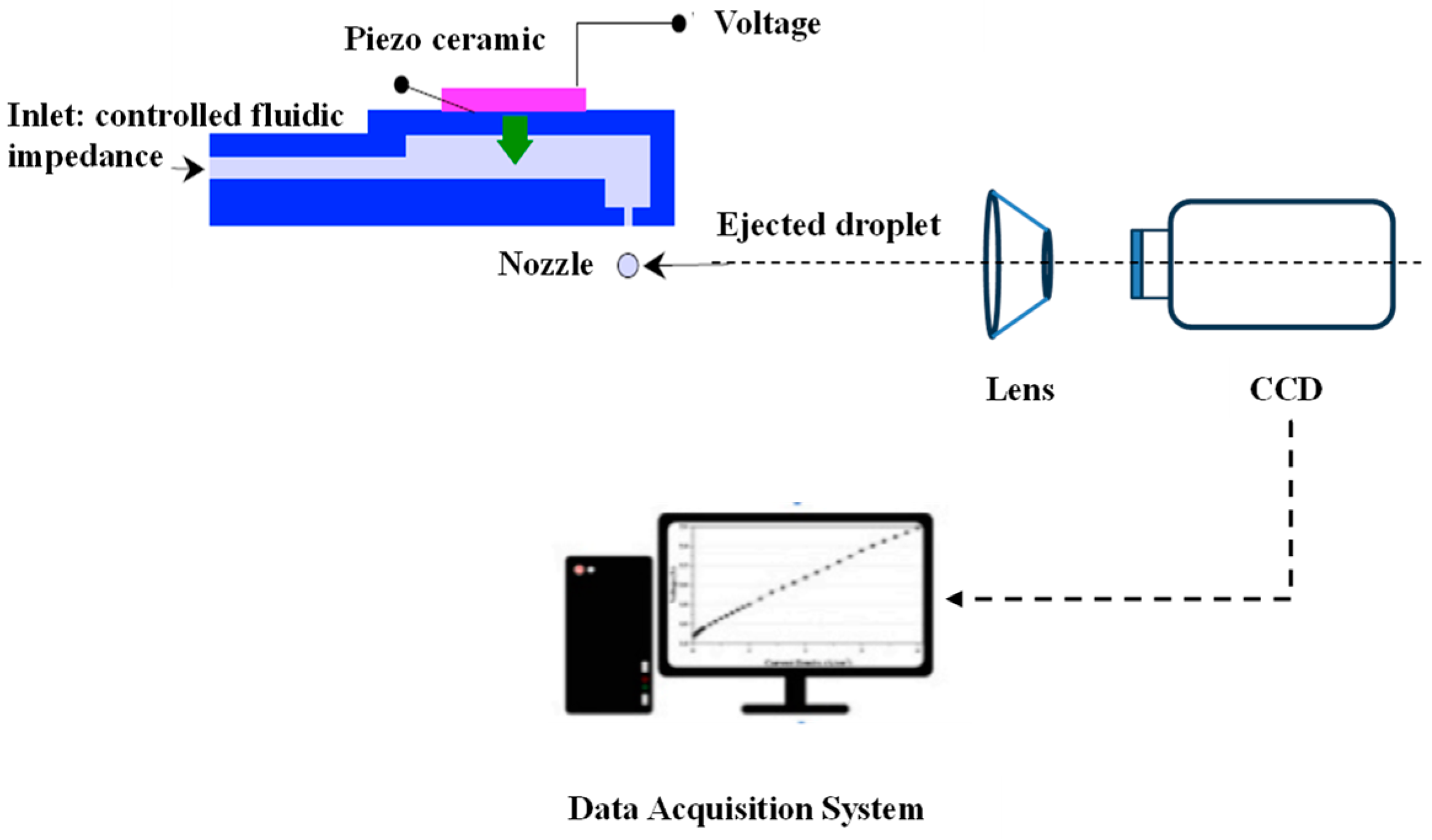
2. Materials and Methods
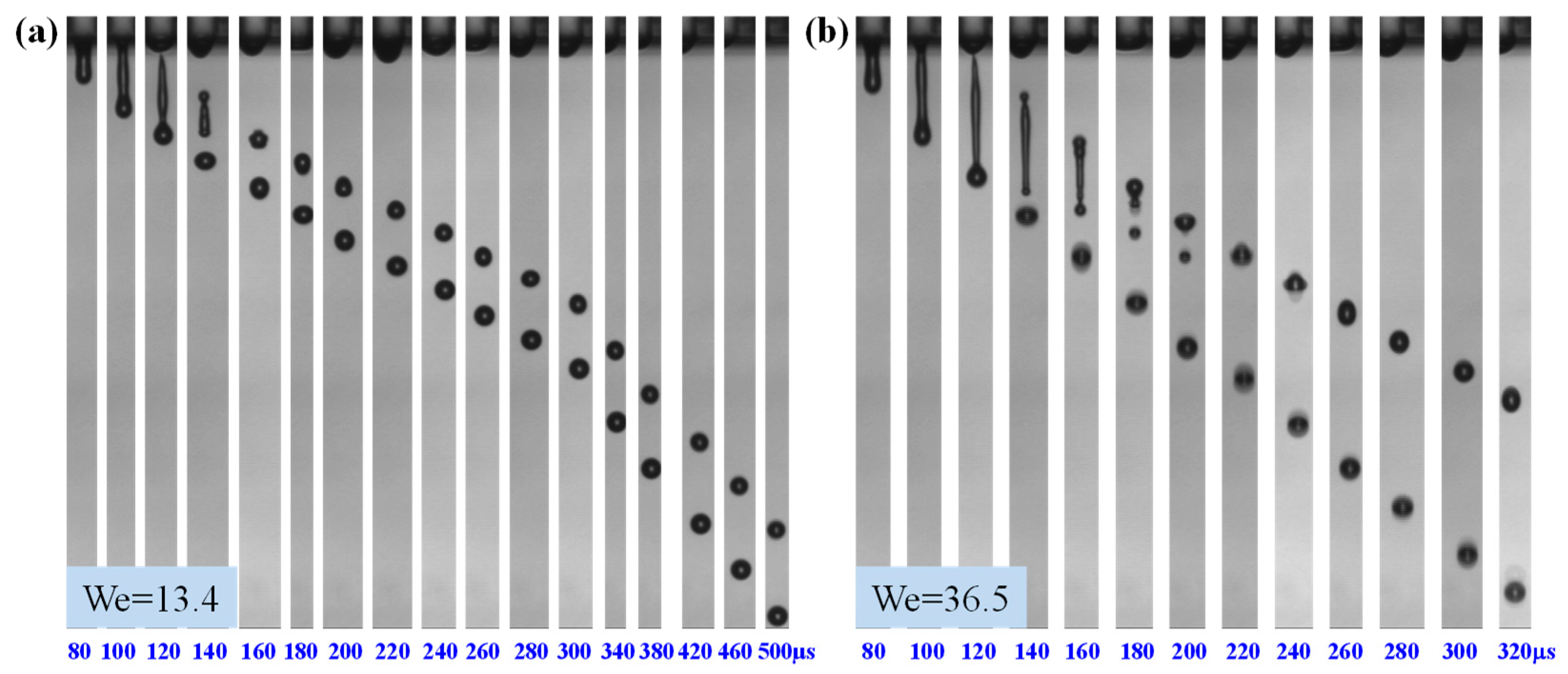
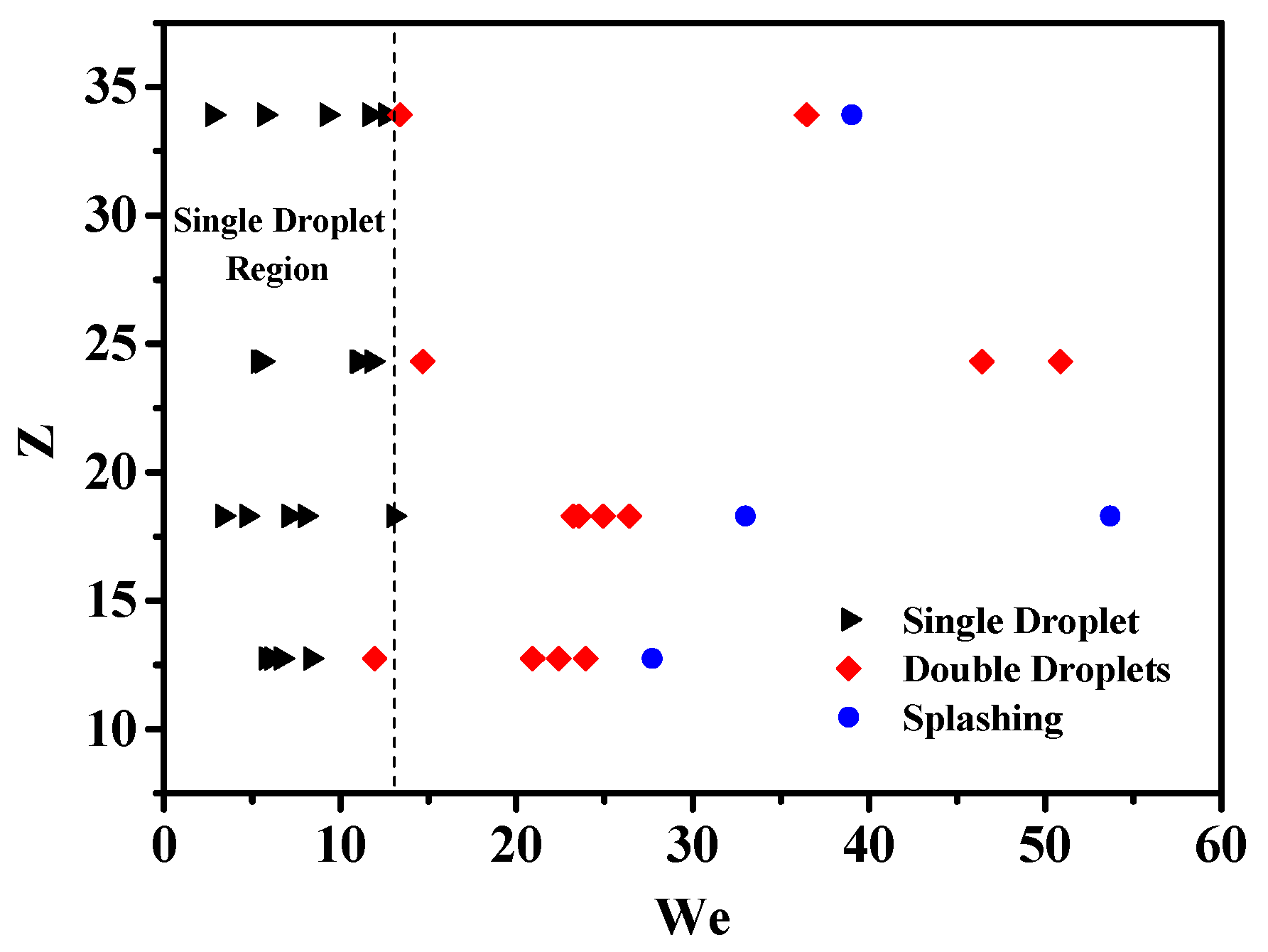
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Saunders, R.E.; Derby, B. Inkjet printing biomaterials for tissue engineering: Bioprinting. Int. Mater. Rev. 2014, 59, 430–448. [Google Scholar] [CrossRef]
- Zhang, G.; Qu, Z.; Tao, W.-Q.; Wang, X.; Wu, L.; Wu, S.; Xie, X.; Tongsh, C.; Huo, W.; Bao, Z.; et al. Porous Flow Field for Next-Generation Proton Exchange Membrane Fuel Cells: Materials, Characterization, Design, and Challenges. Chem. Rev. 2022, 123, 989–1039. [Google Scholar] [CrossRef] [PubMed]
- Xu, C.; Zhang, M.; Huang, Y.; Ogale, A.; Fu, J.; Markwald, R.R. Study of Droplet Formation Process during Drop-on-Demand Inkjetting of Living Cell-Laden Bioink. Langmuir 2014, 30, 9130–9138. [Google Scholar] [CrossRef] [PubMed]
- Wang, J.J.; Li, D.Y.; Mu, L.; Li, M.Z.; Luo, Y.; Zhang, B.B.; Mai, C.H.; Guo, B.A.; Lan, L.F.; Wang, J.; et al. Inkjet-Printed Full-Color Matrix Quasi-Two-Dimensional Perovskite Light-Emitting Diodes. ACS Appl. Mater. Interfaces 2021, 13, 41773–41781. [Google Scholar] [CrossRef] [PubMed]
- Xue, Y.; Wang, L.; Zhang, Y.; Liang, G.; Chu, J.; Han, B.; Cao, W.; Liao, C.; Zhang, S. 31-Inch 4K Flexible Display Employing Gate Driver With Metal Oxide Thin-Film Transistors. IEEE Electron Device Lett. 2021, 42, 188–191. [Google Scholar] [CrossRef]
- Cai, X.; Su, S.-J. Marching Toward Highly Efficient, Pure-Blue, and Stable Thermally Activated Delayed Fluorescent Organic Light-Emitting Diodes. Adv. Funct. Mater. 2018, 28, 1802558. [Google Scholar] [CrossRef]
- Aernouts, T.; Aleksandrov, T.; Girotto, C.; Genoe, J.; Poortmans, J. Polymer based organic solar cells using ink-jet printed active layers. Appl. Phys. Lett. 2008, 92, 03306. [Google Scholar] [CrossRef]
- Hoth, C.N.; Schilinsky, P.; Choulis, S.A.; Brabec, C.J. Printing Highly Efficient Organic Solar Cells. Nano Lett. 2008, 8, 2806–2813. [Google Scholar] [CrossRef]
- Krebs, F.C. Fabrication and processing of polymer solar cells: A review of printing and coating techniques. Sol. Energy Mater. Sol. Cells 2009, 93, 394–412. [Google Scholar] [CrossRef]
- Tu, N.; Lo, J.C.C.; Lee, S.W.R. Development of Uniform Polydimethylsiloxane Arrays through Inkjet Printing. Polymers 2023, 15, 462. [Google Scholar] [CrossRef]
- Gupta, S.; Thombare, M.R.; Patil, N.D. Pinning and Depinning Dynamics of an Evaporating Sessile Droplet Containing Mono- and Bidispersed Colloidal Particles on a Nonheated/Heated Hydrophobic Substrate. Langmuir 2023, 39, 3102–3117. [Google Scholar] [CrossRef] [PubMed]
- Bai, J.Y.; Hu, H.L.; Yu, Y.S.; Zhu, Y.B.; Xu, Z.W.; Zheng, W.C.; Zhao, H.B.; Lin, L.H.; Guo, T.L.; Li, F.S. Achieving high performance InP quantum dot light-emitting devices by using inkjet printing. Org. Electron. 2023, 113, 106705. [Google Scholar] [CrossRef]
- Huang, Q.; Al-Milaji, K.N.; Zhao, H. Inkjet Printing of Silver Nanowires for Stretchable Heaters. ACS Appl. Nano Mater. 2018, 1, 4528–4536. [Google Scholar] [CrossRef]
- Driessen, T.; Jeurissen, R. Drop Formation in Inkjet Printing. In Fundamentals of Inkjet Printing; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2016; pp. 93–116. [Google Scholar]
- Notz, P.K.; Basaran, O.A. Dynamics and breakup of a contracting liquid filament. J. Fluid Mech. 2004, 512, 223–256. [Google Scholar] [CrossRef]
- Schulkes, R. The contraction of liquid filaments. J. Fluid Mech. 1996, 309, 277–300. [Google Scholar] [CrossRef]
- Bernasconi, R.; Brovelli, S.; Viviani, P.; Soldo, M.; Giusti, D.; Magagnin, L. Piezoelectric Drop-On-Demand Inkjet Printing of High-Viscosity Inks. Adv. Eng. Mater. 2022, 24, 2100733. [Google Scholar] [CrossRef]
- Satpathi, N.S.; Malik, L.; Ramasamy, A.S.; Sen, A.K. Drop Impact on a Superhydrophilic Spot Surrounded by a Superhydrophobic Surface. Langmuir 2021, 37, 14195–14204. [Google Scholar] [CrossRef] [PubMed]
- Jung, S.J.; Hoath, S.D.; Hutchings, I.M. The role of viscoelasticity in drop impact and spreading for inkjet printing of polymer solution on a wettable surface. Microfluid. Nanofluidics 2013, 14, 163–169. [Google Scholar] [CrossRef]
- Hoath, S.D.; Jung, S.; Hutchings, I.M. A simple criterion for filament break-up in drop-on-demand inkjet printing. Phys. Fluids 2013, 25, 5. [Google Scholar] [CrossRef]
- Hoath, S.D.; Hutchings, I.M.; Harlen, O.G.; McLlroy, C.; Morrison, N.F. Regimes of Polymer Behaviour in Drop-on-Demand Ink-Jetting; Society for Imaging Science & Technology: Springfield, VA, USA, 2012; pp. 408–411. [Google Scholar]
- Hoath, S.; Martin, G.; Castrejon-Pita, R.; Hutchings, I. Satellite Formation in Drop-on-Demand Printing of Polymer Solutions; Society for Imaging Science & Technology: Springfield, VA, USA, 2007; pp. 331–335. [Google Scholar]
- Dong, H.; Carr, W.W.; Morris, J.F. An experimental study of drop-on-demand drop formation. Phys. Fluids 2006, 18, 072102. [Google Scholar] [CrossRef]
- Jang, D.; Kim, D.; Moon, J. Influence of Fluid Physical Properties on Ink-Jet Printability. Langmuir 2009, 25, 2629–2635. [Google Scholar] [CrossRef]
- de Gans, B.J.; Xue, L.J.; Agarwal, U.S.; Schubert, U.S. Ink-jet printing of linear and star polymers. Macromol. Rapid Commun. 2005, 26, 310–314. [Google Scholar] [CrossRef]
- Suryo, R.; Doshi, P.; Basaran, O.A. Nonlinear dynamics and breakup of compound jets. Phys. Fluids 2006, 18, 082107. [Google Scholar] [CrossRef]
- Aytouna, M.; Paredes, J.; Shahidzadeh-Bonn, N.; Moulinet, S.; Wagner, C.; Amarouchene, Y.; Eggers, J.; Bonn, D. Drop Formation in Non-Newtonian Fluids. Phys. Rev. Lett. 2013, 110, 5. [Google Scholar] [CrossRef]
- Du, Z.; Zhou, H.; Cao, W.; Yu, X.; Han, Y. The effects of coil–stretch transition behavior of polyfluorene inks on single droplet formation during inkjet printing. Chin. Chem. Lett. 2020, 31, 3216–3220. [Google Scholar] [CrossRef]
- Kang, D.J.; Gonzalez-Garcia, L.; Kraus, T. Soft electronics by inkjet printing metal inks on porous substrates. Flex. Print. Electron. 2022, 7, 033001. [Google Scholar] [CrossRef]
- Yan, K.; Li, J.A.; Pan, L.J.; Shi, Y. Inkjet printing for flexible and wearable electronics. APL Mater. 2020, 8, 120705. [Google Scholar] [CrossRef]
- Rivadeneyra, A.; Lopez-Villanueva, J.A. Recent Advances in Printed Capacitive Sensors. Micromachines 2020, 11, 367. [Google Scholar] [CrossRef] [PubMed]
- Smith, P.J.; Stringer, J. Applications in Inkjet Printing. In Fundamentals of Inkjet Printing; Wiley-VCH Verlag GmbH & Co. KGaA: Weinheim, Germany, 2016; pp. 397–418. [Google Scholar]
- Shi, Y.; Liu, J.; Yang, Y. Device performance and polymer morphology in polymer light emitting diodes: The control of thin film morphology and device quantum efficiency. J. Appl. Phys. 2000, 87, 4254–4263. [Google Scholar] [CrossRef]
- Nguyen, T.Q.; Doan, V.; Schwartz, B.J. Conjugated polymer aggregates in solution: Control of interchain interactions. J. Chem. Phys. 1999, 110, 4068–4078. [Google Scholar] [CrossRef]
- Fromm, J.E. Numerical-Calculation of the Fluid-Dynamics of Drop-On-Demand Jets. IBM J. Res. Dev. 1984, 28, 322–333. [Google Scholar] [CrossRef]
- Derby, B.; Reis, N. Inkjet printing of highly loaded particulate suspensions. MRS Bull. 2003, 28, 815–818. [Google Scholar] [CrossRef]
- Derby, B. Inkjet printing ceramics: From drops to solid. J. Eur. Ceram. Soc. 2011, 31, 2543–2550. [Google Scholar] [CrossRef]
- Du, Z.; Zhou, H.; Yu, X.; Han, Y. Controlling the polarity and viscosity of small molecule ink to suppress the contact line receding and coffee ring effect during inkjet printing. Colloids Surf. A Physicochem. Eng. Asp. 2020, 602, 125111. [Google Scholar] [CrossRef]
- Hoath, S.D.; Harlen, O.G.; Hutchings, I.M. Jetting behavior of polymer solutions in drop-on-demand inkjet printing. J. Rheol. 2012, 56, 1109–1127. [Google Scholar] [CrossRef]
- Du, Z.; Yu, X.; Han, Y. Inkjet printing of viscoelastic polymer inks. Chin. Chem. Lett. 2018, 29, 399–404. [Google Scholar] [CrossRef]
- Du, Z.; Lin, Y.; Xing, R.; Cao, X.; Yu, X.; Han, Y. Controlling the polymer ink’s rheological properties and viscoelasticity to suppress satellite droplets. Polymer 2018, 138, 75–82. [Google Scholar] [CrossRef]
- Jiang, C.; Zhong, Z.; Liu, B.; He, Z.; Zou, J.; Wang, L.; Wang, J.; Peng, J.; Cao, Y. Coffee-Ring-Free Quantum Dot Thin Film Using Inkjet Printing from a Mixed-Solvent System on Modified ZnO Transport Layer for Light-Emitting Devices. ACS Appl. Mater. Interfaces 2016, 8, 26162–26168. [Google Scholar] [CrossRef]




| Parameters | Value |
|---|---|
| Density, ρ | 1068.40 Kg/m3 |
| Surface tension, γ | 31.0 mN/m |
| Viscosity, η | 1.20 mPa·s |
| Conductivity, G | 450 S/m |
| Z value | 33.91 |
| Velocity (m/s) | Re | We |
|---|---|---|
| 1.1 | 50.8 | 2.2 |
| 1.8 | 81.1 | 5.7 |
| 2.3 | 103.1 | 9.3 |
| 2.6 | 115.8 | 11.7 |
| Concentration (mg/mL) | Z |
|---|---|
| 5 | 33.91 |
| 10 | 24.31 |
| 15 | 18.30 |
| 20 | 12.75 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Du, Z.; Zhang, L.; Du, Y.; Wei, X.; Du, X.; Lin, X.; Liu, J.; Huang, Y.; Xue, Y.; Zhao, N.; et al. Controlling the Polymer Ink’s Rheological Properties to Form Single and Stable Droplet. Coatings 2024, 14, 600. https://doi.org/10.3390/coatings14050600
Du Z, Zhang L, Du Y, Wei X, Du X, Lin X, Liu J, Huang Y, Xue Y, Zhao N, et al. Controlling the Polymer Ink’s Rheological Properties to Form Single and Stable Droplet. Coatings. 2024; 14(5):600. https://doi.org/10.3390/coatings14050600
Chicago/Turabian StyleDu, Zhonghui, Lu Zhang, Yushuang Du, Xiaoqing Wei, Xiang Du, Xinyan Lin, Jiajun Liu, Yani Huang, Yan Xue, Ning Zhao, and et al. 2024. "Controlling the Polymer Ink’s Rheological Properties to Form Single and Stable Droplet" Coatings 14, no. 5: 600. https://doi.org/10.3390/coatings14050600
APA StyleDu, Z., Zhang, L., Du, Y., Wei, X., Du, X., Lin, X., Liu, J., Huang, Y., Xue, Y., Zhao, N., & Liu, H. (2024). Controlling the Polymer Ink’s Rheological Properties to Form Single and Stable Droplet. Coatings, 14(5), 600. https://doi.org/10.3390/coatings14050600






