Multi-Electric Aero Engine Control and Hardware-in-the-Loop Verification with Starter Generator Coordination
Abstract
:1. Introduction
- The full-state modeling method for the aero engine proposed in this paper enhances the simulation capability at low speeds. The external characteristic modeling method for SG proposed in this paper introduces a new perspective on SG model simplification by identification.
- The control methods with SG coordination proposed in this paper improve the starting performance of multi-electric aero engines and reduce the interference of generator load torque on the acceleration, deceleration, and steady-state processes of multi-electric aero engines.
- The hardware-in-the-loop simulation platform in this paper is designed explicitly for proposed cooperative control methods verification, enabling real-time simulation of a multi-electric aero engine model.
2. Multi-Electric Aero Engine Modeling
2.1. Full-State Modeling of Turbofan Engine
2.2. External Characteristic Modeling of SG
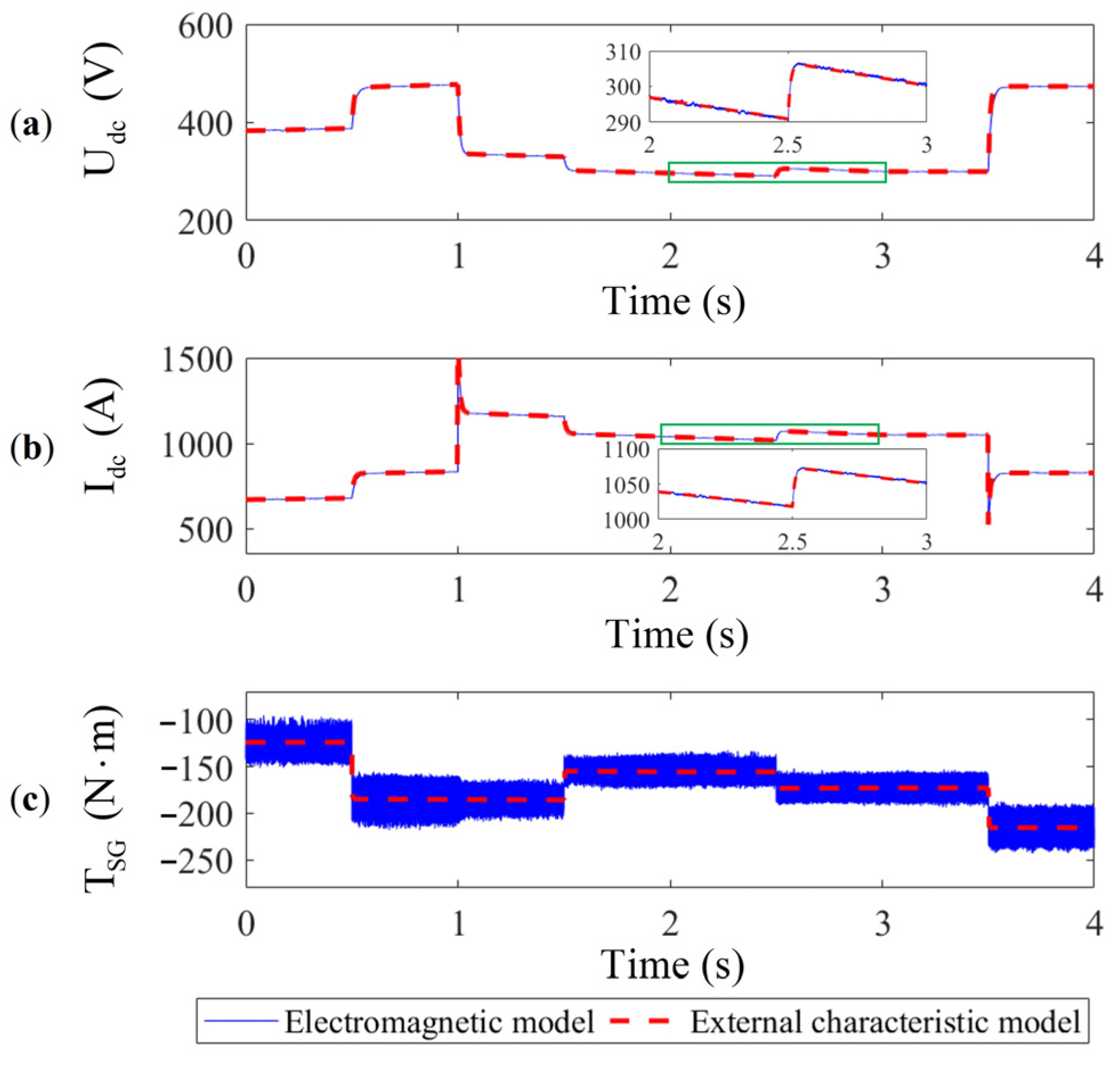
- Compared to the electromagnetic model used in the study of SG control, the external characteristic model of SG allows simulation time steps to be extended to 1 ms. This significantly reduces computational complexity, enhancing the real-time performance of the model.
- The proposed method is an identification approach for a multi-input, multi-output model of SG. This method is general and applicable for simplifying various SG models.
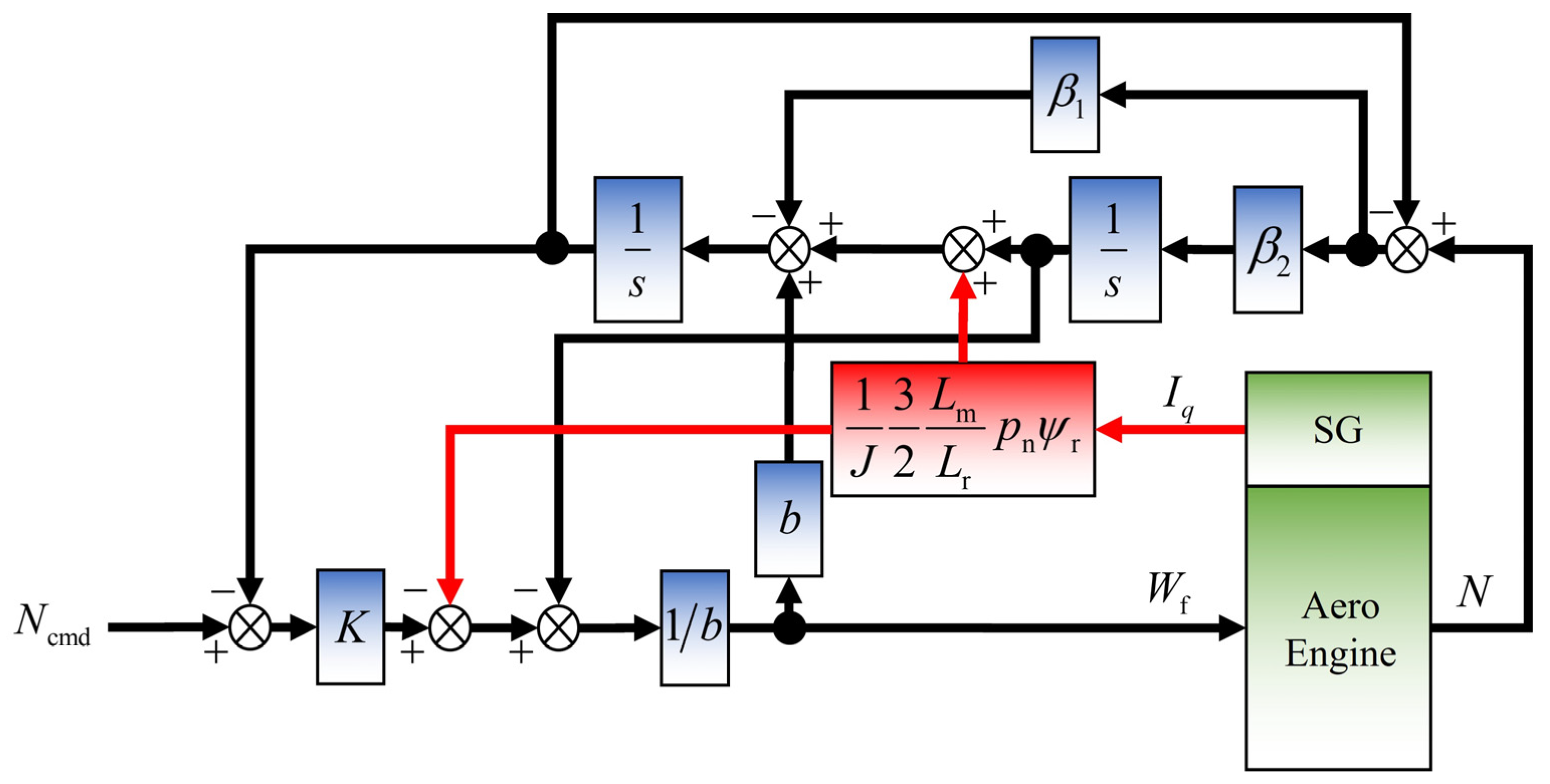
3. Control Methods with SG Coordination
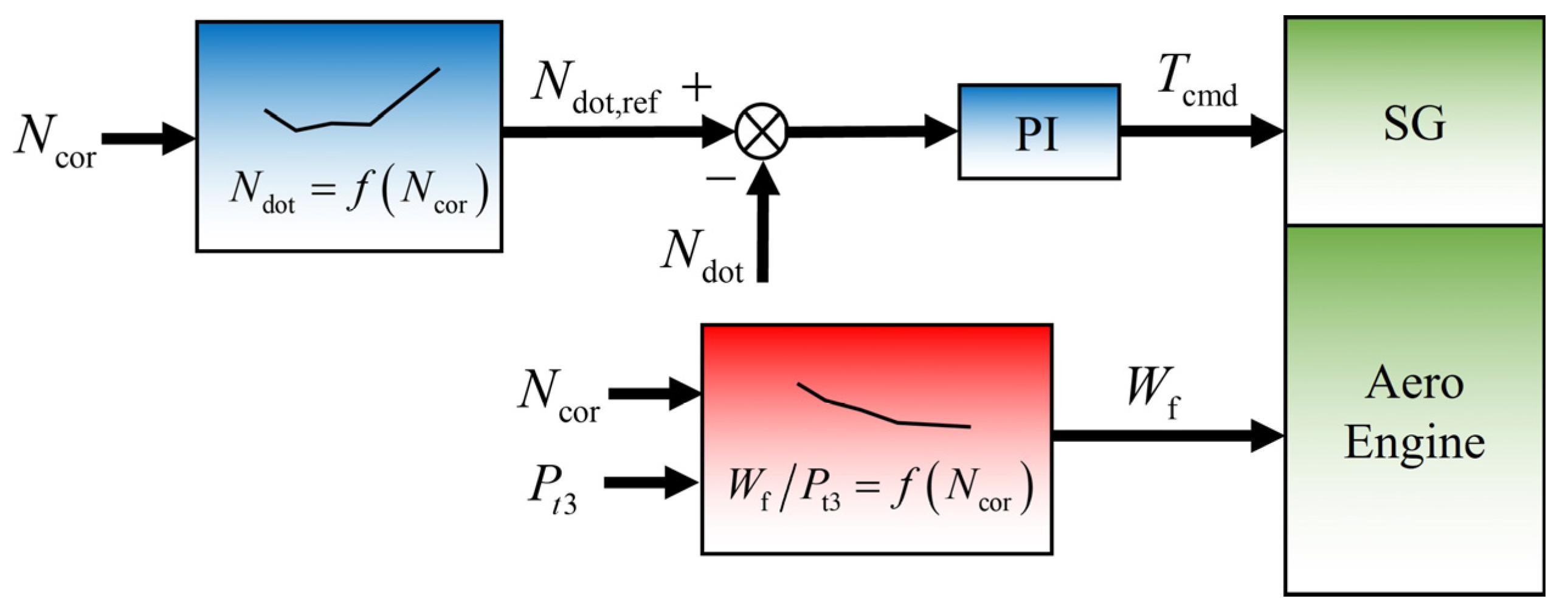
3.1. Cooperative Control Method for Starting Process
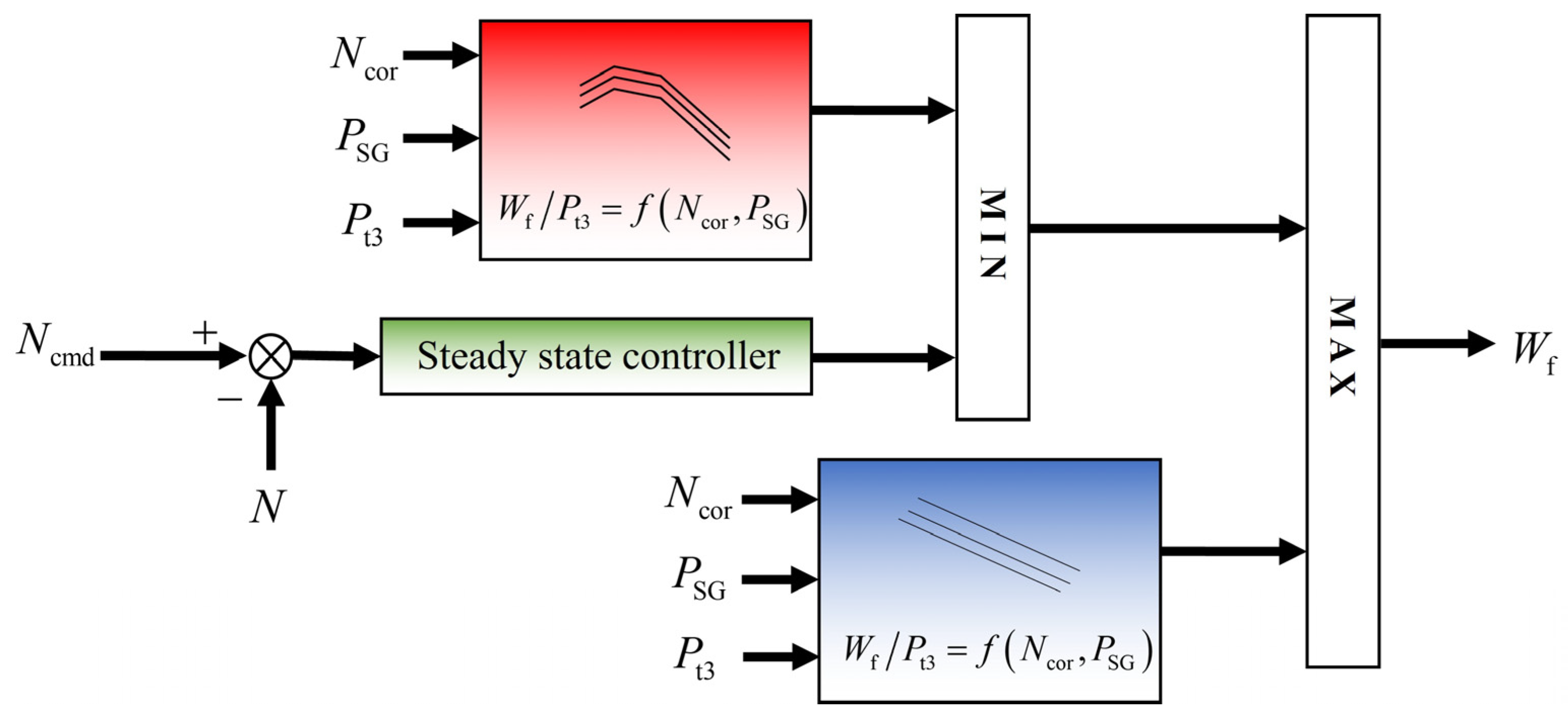
3.2. Cooperative Control Method for Acceleration/Deceleration Process
3.3. Cooperative Control Method for Steady-State Process
4. Hardware-in-the-Loop Simulation Platform
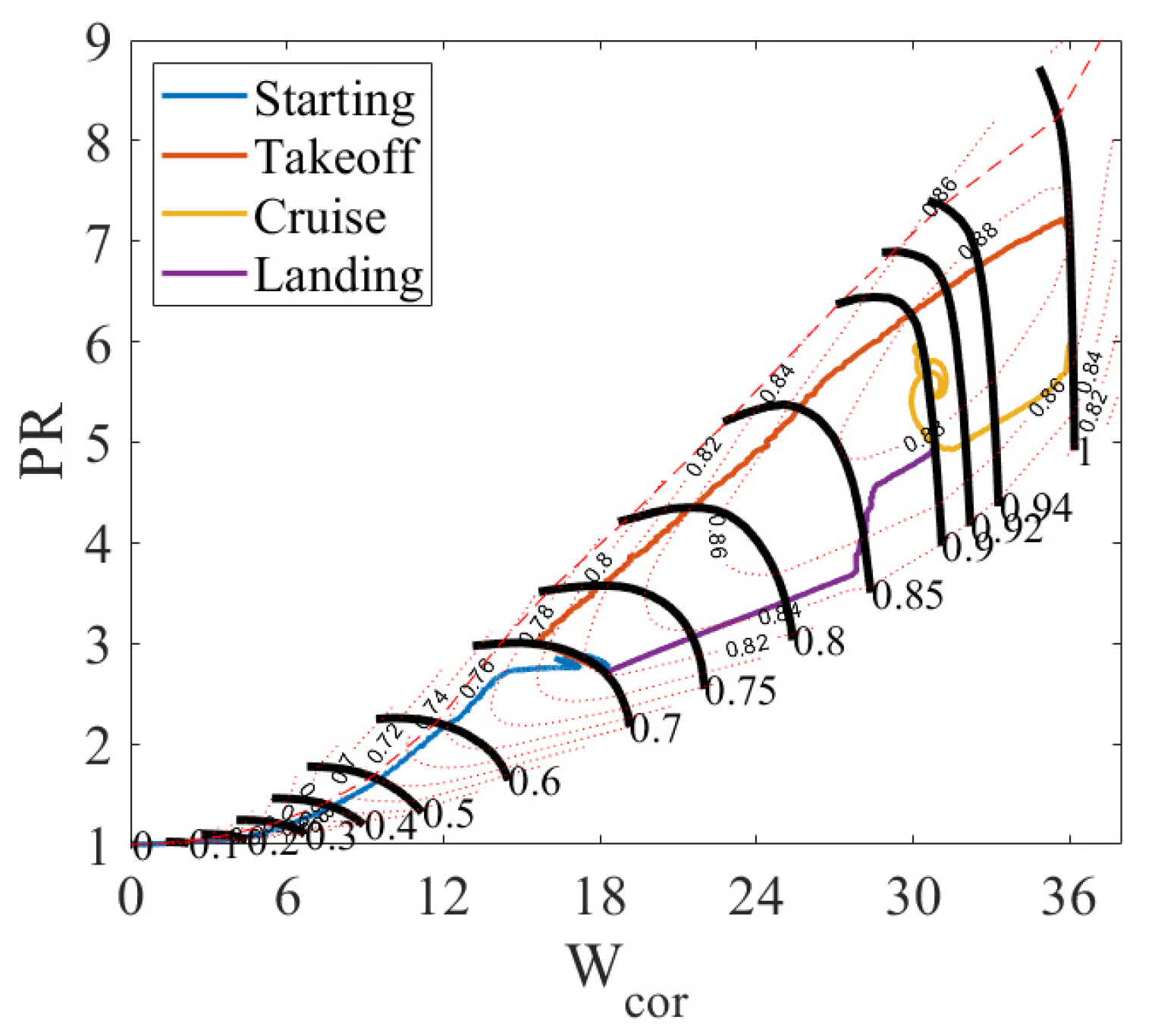
5. Simulation Results and Analysis
5.1. Starting Process
5.2. Acceleration/Deceleration Process
5.3. Steady-State Process
5.4. A Complete Flight Mission
6. Conclusions
- The application of the rotor’s full-state characteristics to the aero engine model enhances the simulation capability at low-speed states.
- The external characteristic modeling method used to establish the SG model sacrifices high-frequency dynamics but enables real-time simulation of the SG model on conventional embedded platforms.
- The cooperative control of SG torque and aero engine fuel during the starting process can reduce the oscillation of the acceleration.
- The adjustment of fuel limiting values based on electrical load power during the acceleration/deceleration process can reduce the impact of electrical load on protection limits such as over-temperature, surging, and flameout, ensuring safety and stability under different electrical load conditions.
- A cooperative control method for the steady-state process is proposed to compensate fuel based on the q-axis current of SG, reducing the amplitude of engine speed fluctuations caused by sudden electrical load disturbances.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DC | Direct current |
| EPT | Electric power transfer |
| FAR | Air–fuel ratio |
| Sliding friction torque | |
| Static friction torque | |
| HP | High-pressure |
| q-axis current | |
| Rotational inertia of the high-pressure shaft | |
| Rotational inertia of the low-pressure shaft | |
| LP | Low-pressure |
| Leakage inductance of rotor winding | |
| Magnetizing inductance | |
| Relative converted speed | |
| High-pressure shaft speed | |
| Low-pressure shaft speed | |
| Rotational acceleration | |
| Speed command | |
| PR | Pressure ratio |
| PLA | Power level angle |
| Power output of the starter generator | |
| Total pressure at the HP compressor outlet section | |
| Inlet total pressure | |
| Outlet total pressure | |
| Number of pole pairs | |
| SG | Starter generator |
| SFC | Specific fuel consumption |
| Fan surge margin | |
| High-pressure compressor surge margin | |
| TEEM | Turbine electrical energy management |
| Fan torque | |
| High-pressure compressor torque | |
| High-pressure turbine torque | |
| Low-pressure turbine torque | |
| Starter generator torque | |
| Torque command | |
| Total temperature at the fan inlet section | |
| Total temperature at the HP turbine inlet section | |
| Inlet total pressure | |
| Outlet total pressure | |
| Converted flow rate | |
| Fuel flow rate | |
| Rotor magnetic flux | |
| Adjustable controller parameters |
References
- Simon, D.L.; Connolly, J.W.; Culley, D.E. Control technology needs for electrified aircraft propulsion systems. J. Eng. Gas Turbines Power-Trans. ASME 2020, 142, 011025. [Google Scholar] [CrossRef]
- Jansen, R.; Bowman, C.; Jankovsky, A.; Dyson, R.; Felder, J. Overview of NASA electrified aircraft propulsion (EAP) research for large subsonic transports. In Proceedings of the 53rd AIAA/SAE/ASEE Joint Propulsion Conference, Atlanta, GA, USA, 10–12 July 2017. [Google Scholar]
- Adibhatla, S.; Garg, S.; Griffith, S.; Karnofski, K.; Payne, N.; Wood, B. Propulsion control technology development roadmaps to address nasa aeronautics research mission goals for thrusts 3a and 4. In Proceedings of the 2018 Joint Propulsion Conference, Cincinnati, OH, USA, 9–11 July 2018. [Google Scholar]
- Welstead, J.R.; Felder, J.L. Conceptual design of a single-aisle turboelectric commercial transport with fuselage boundary layer ingestion. In Proceedings of the 54th AIAA Aerospace Sciences Meeting, San Diego, CA, USA, 4–8 January 2016. [Google Scholar]
- Kratz, J.L.; Thomas, G.L. Dynamic analysis of the STARC-ABL propulsion system. In Proceedings of the AIAA Propulsion and Energy 2019 Forum, Indianapolis, IN, USA, 19–22 August 2019. [Google Scholar]
- Kim, H.D.; Felder, J.L.; Tong, M.T.; Berton, J.J.; Haller, W.J. Turboelectric distributed propulsion benefits on the N3-X vehicle. Aircr. Eng. Aerosp. Technol. 2014, 86, 558–561. [Google Scholar] [CrossRef]
- McNab, I.R. Pulsed power for electric guns. IEEE Trans. Magn. 1997, 33, 453–460. [Google Scholar] [CrossRef]
- Mohamed, M.A.A.; Shen Yeoh, S.; Atkin, J.; Diab, A.M.; Khalaf, M.; Bozhko, S. Enhanced starting control scheme for PMM-based starter/generator system for MEA. Aerospace 2023, 10, 168. [Google Scholar] [CrossRef]
- Liu, H.; Bu, F.; Huang, W.; Xu, H.; Degano, M.; Gerada, C. Control-winding direct power control strategy for five-phase dual-stator winding induction generator DC generating system. IEEE Trans. Transp. Electrif. 2020, 6, 73–82. [Google Scholar] [CrossRef]
- Qiu, X.; Zhang, Y.; Li, Y. Active disturbance rejection control method of auxiliary power unit. J. Aerosp. Power 2024, 39, 20220035. [Google Scholar]
- Simon, D.L.; Bianco, S.J.; Horning, M. Integrated control design for a partially turboelectric aircraft propulsion system. J. Eng. Gas Turbines Power 2023, 146, 1–37. [Google Scholar] [CrossRef]
- Connolly, J.W.; Chapman, J.W.; Stalcup, E.J.; Chicatelli, A.; Hunker, K.R. Modeling and control design for a turboelectric single aisle aircraft propulsion system. In Proceedings of the 2018 AIAA/IEEE Electric Aircraft Technologies Symposium, Cincinnati, OH, USA, 12–13 July 2018. [Google Scholar]
- Seok, J.; Reed, D.M.; Kolmanovsky, I.V.; Girard, A.R. Coordinated model predictive control of aircraft gas turbine engine with simplified electrical system model. In Proceedings of the American Control Conference, Milwaukee, WI, USA, 27–29 June 2018. [Google Scholar]
- Seok, J.; Kolmanovsky, I.; Girard, A. coordinated model predictive control of aircraft gas turbine engine and power system. J. Guid. Control. Dyn. 2017, 40, 2538–2555. [Google Scholar] [CrossRef]
- Seok, J.; Kolmanovsky, I.; Girard, A. Integrated/Coordinated control of aircraft gas turbine engine and electrical power system: Towards large electrical load handling. In Proceedings of the 55th IEEE Conference on Decision and Control (CDC), Las Vegas, NV, USA, 12–14 December 2016. [Google Scholar]
- Richter, H.; Connolly, J.W.; Simon, D.L. Optimal control and energy management for hybrid gas-electric propulsion. J. Eng. Gas Turbines Power 2020, 142, 091009. [Google Scholar] [CrossRef]
- Wei, Z.; Ma, Y.; Xiang, C.; Liu, D.; Li, Z.; Ruan, S. An energy-saving regulation approach based on economic rotational speed and power split feature for more electric turboshaft engine. Aerosp. Sci. Technol. 2022, 130, 107913. [Google Scholar] [CrossRef]
- Culley, D.E.; Kratz, J.L.; Thomas, G.L. Turbine electrified energy management (TEEM) for enabling more efficient engine designs. In Proceedings of the 2018 Joint Propulsion Conference, Cincinnati, OH, USA, 9–11 July 2018. [Google Scholar]
- Enalou, H.B.; Lang, X.; Rashed, M.; Bozhko, S. Time-scaled emulation of electric power transfer in the more electric engine. IEEE Trans. Transp. Electrif. 2020, 6, 1679–1694. [Google Scholar] [CrossRef]
- Electrical Modeling and Thermal Analysis Toolbox (EMTAT) User’s Guide. Available online: https://ntrs.nasa.gov/citations/20205008125 (accessed on 28 October 2020).
- NASA Electric Aircraft Test Bed (NEAT) Development Plan-Design, Fabrication, Installation. Available online: https://ntrs.nasa.gov/citations/20160010440 (accessed on 1 July 2016).
- Hybrid-Electric Aero-Propulsion Controls Testbed Results. Available online: https://ntrs.nasa.gov/citations/20220016701 (accessed on 4 November 2022).
- Bretschneider, S.; Reed, J. Modeling of start-up from engine-off conditions using high fidelity turbofan engine simulations. J. Eng. Gas Turbines Power-Trans. ASME 2016, 138, 051201. [Google Scholar] [CrossRef]
- Ferrer-Vidal, L.E.; Iglesias-Perez, A.; Pachidis, V. Characterization of axial compressor performance at locked rotor and torque-free windmill conditions. Aerosp. Sci. Technol. 2020, 101, 105846. [Google Scholar] [CrossRef]
- Extended Parametric Representation of Compressor Fans and Turbines Volume I—CMGEN User’s Manual. Available online: https://ntrs.nasa.gov/citations/19860014465 (accessed on 1 March 1984).
- Extended Parametric Representation of Compressor Fans and Turbines Volume II—PART User’s Manual. Available online: https://ntrs.nasa.gov/citations/19860014466 (accessed on 1 March 1984).
- Extended Parametric Representation of Compressor Fans and Turbines Volume III MODFAN User’s Manual. Available online: https://ntrs.nasa.gov/citations/19860014467 (accessed on 1 March 1984).
- Shi, Y.; Tu, Q.; Yan, H.; Jiang, P.; Cai, Y. A full states performance model for aero engine. J. Aerosp. Power 2017, 32, 373–381. [Google Scholar] [CrossRef]
- Bu, F.; Hu, Y.; Huang, W.; Zhuang, S.; Shi, K. Wide-speed-range-operation dual stator-winding induction generator dc generating system for wind power applications. IEEE Trans. Power Electron. 2015, 30, 561–573. [Google Scholar] [CrossRef]
- Thomas, G.L.; Culley, D.E.; Kratz, J.L.; Fisher, K.L. Dynamic analysis of the hfan, a parallel hybrid electric turbofan engine. In Proceedings of the 2018 Joint Propulsion Conference, Cincinnati, OH, USA, 9–11 July 2018. [Google Scholar]
- Subsonic Ultra Green Aircraft Research: Phase II—Volume II—Hybrid Electric Design Exploration. Available online: https://ntrs.nasa.gov/citations/20150017039 (accessed on 1 April 2015).
- Kong, X.; Wang, X.; Zhang, S.; Yin, C. Research and experiment on improved sectional combination control scheme for civil turbofan engine’s startup process. J. Aerosp. Power 2014, 29, 2924–2929. [Google Scholar]
- Yao, T.; Wen, W.; Yang, G.; Wang, Y.; Zhu, A. Control law design for n-dot closed control loop for acceleration and deceleration process in turbofan engine. J. Propuls. Technol. 2020, 41, 1404–1410. [Google Scholar]
- Jaw, L.C.; Mattingly, J.D. Aircraft Engine Controls: Design, System Analysis and Health Monitoring; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2009; pp. 119–142. [Google Scholar]

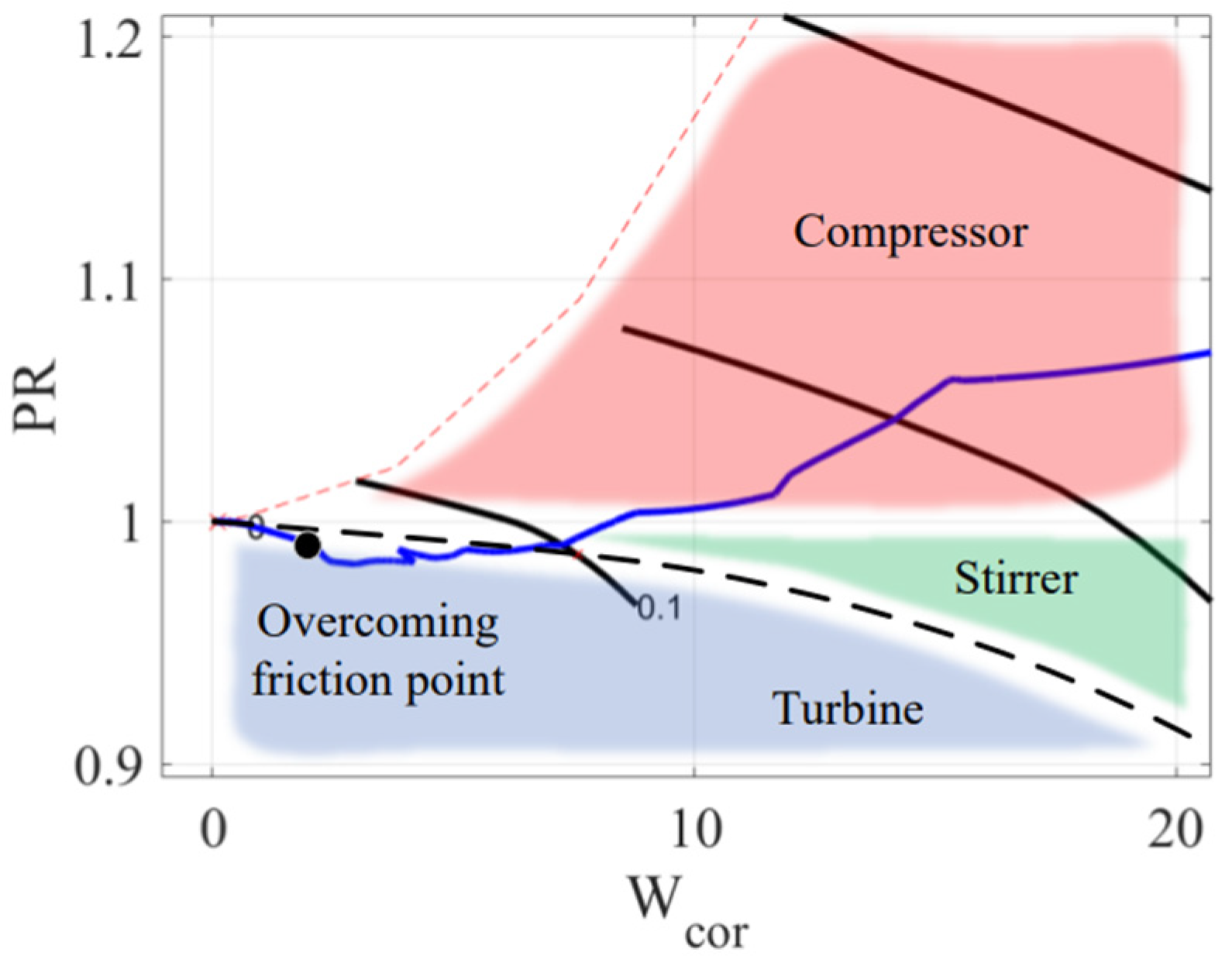










| States | Isentropic Efficiency | Inlet/Outlet Conditions |
|---|---|---|
| Compressor | ||
| Stirrer or Paddle | ||
| Turbine |
| Judgment Criteria | Fan Flow Balance | Schematic Diagram |
|---|---|---|
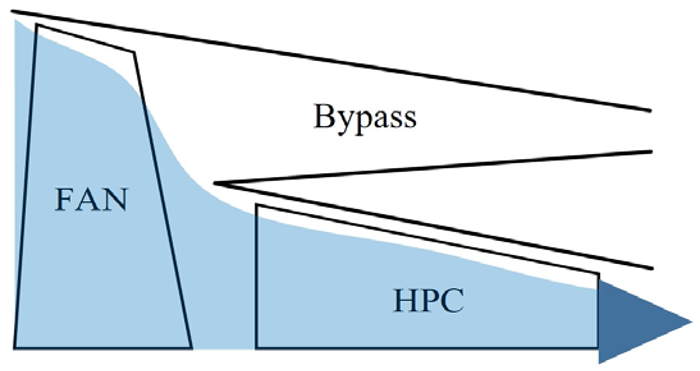 | ||
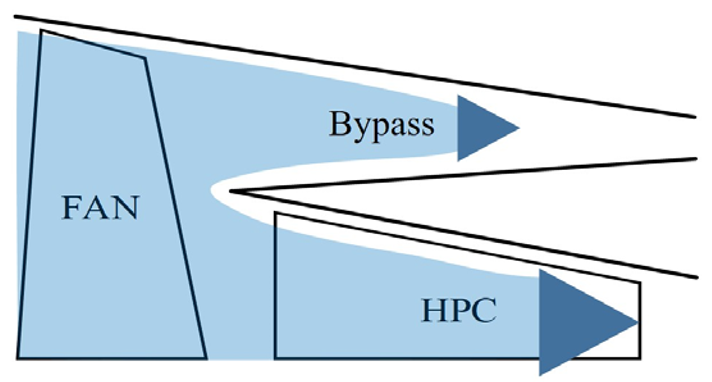 |
| Parameters | Value |
|---|---|
| Bypass Ratio | 0.36 |
| Overall Pressure Ratio | 30.1 |
| Max Thrust (kN) | 106.346 |
| SFC (kg/(N·h)) | 0.0796 |
| Inlet air mass flow (kg/s) | 134.57 |
| Fuel mass flow (kg/s) | 2.35 |
| Inlet HP turbine temperature (K) | 1850 |
| LP shaft and HP shaft speeds (rpm) | 11,000, 15,000 |
| Parameters | Value |
|---|---|
| Rated power (kW) | 420 |
| Rated speed (rpm) | 13,500 |
| Rated voltage of DC bus (V) | 270 |
| Number of pole pairs | 1 |
| Internal resistance of stator winding (mΩ) | 1.107 |
| Internal resistance of rotor winding (mΩ) | 0.907 |
| Magnetizing inductance (mH) | 0.171 |
| Leakage inductance of stator winding (μH) | 2.913 |
| Leakage inductance of rotor winding (μH) | 5.463 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fang, J.; Zhang, T.; Cen, Z.; Tsoutsanis, E. Multi-Electric Aero Engine Control and Hardware-in-the-Loop Verification with Starter Generator Coordination. Aerospace 2024, 11, 271. https://doi.org/10.3390/aerospace11040271
Fang J, Zhang T, Cen Z, Tsoutsanis E. Multi-Electric Aero Engine Control and Hardware-in-the-Loop Verification with Starter Generator Coordination. Aerospace. 2024; 11(4):271. https://doi.org/10.3390/aerospace11040271
Chicago/Turabian StyleFang, Jun, Tianhong Zhang, Zhaohui Cen, and Elias Tsoutsanis. 2024. "Multi-Electric Aero Engine Control and Hardware-in-the-Loop Verification with Starter Generator Coordination" Aerospace 11, no. 4: 271. https://doi.org/10.3390/aerospace11040271
APA StyleFang, J., Zhang, T., Cen, Z., & Tsoutsanis, E. (2024). Multi-Electric Aero Engine Control and Hardware-in-the-Loop Verification with Starter Generator Coordination. Aerospace, 11(4), 271. https://doi.org/10.3390/aerospace11040271







