Influence of Nozzle Exit Conditions on the Near-Field Development of High Subsonic and Underexpanded Axisymmetric Jets
Abstract
1. Introduction
2. Experimental Details
2.1. Experimental Facility
2.2. Test Nozzle Geometries
2.3. Instrumentation
2.3.1. Nozzle Inlet—Pneumatic Probe
2.3.2. Nozzle Exit—Pneumatic Probe
2.3.3. Nozzle Exit and Jet Plume Region—LDA
2.3.4. Jet Plume Region—Schlieren Visualisation
2.3.5. Data Processing/Derived Quantities
2.3.6. Measurement Errors
3. Results
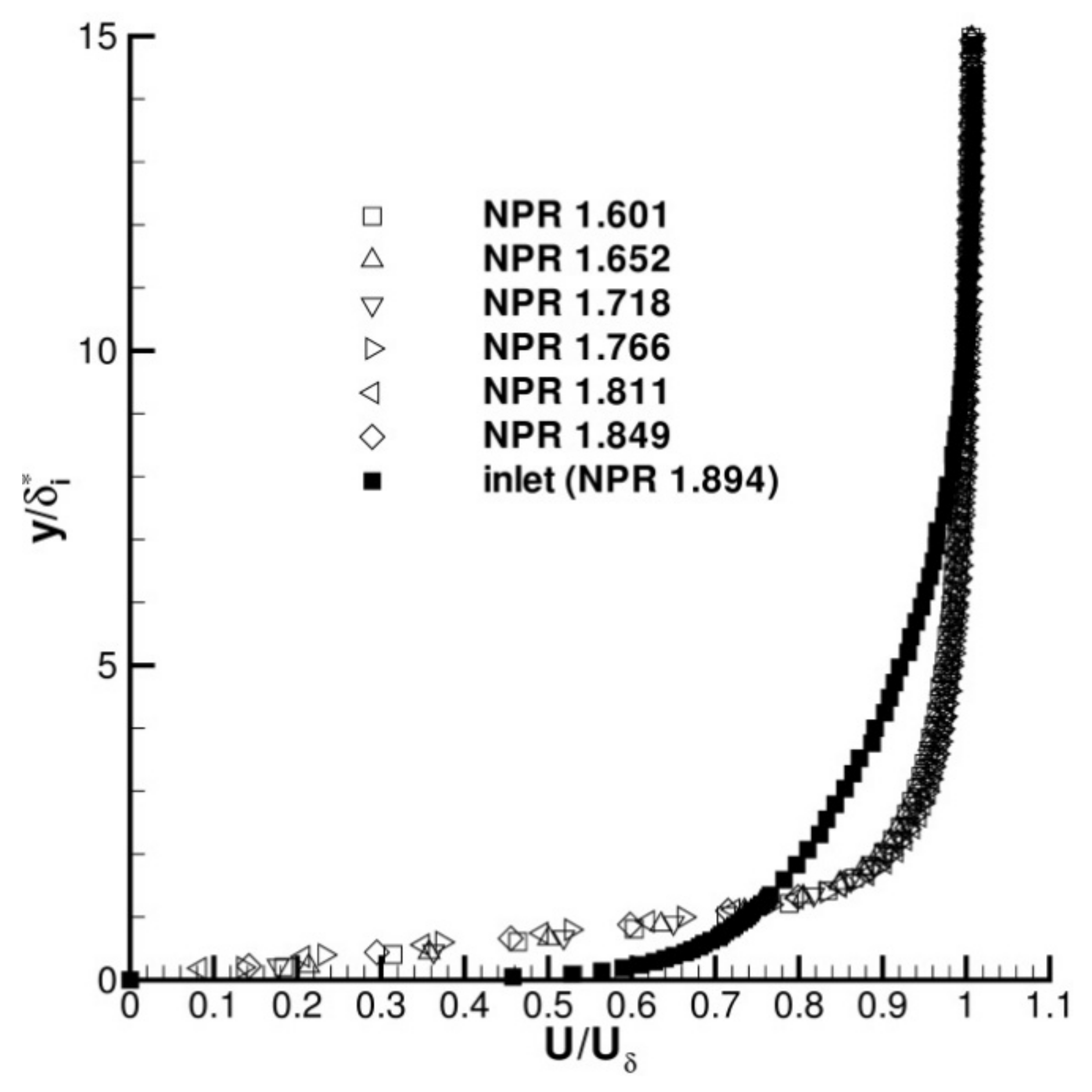
3.1. Nozzle Inlet
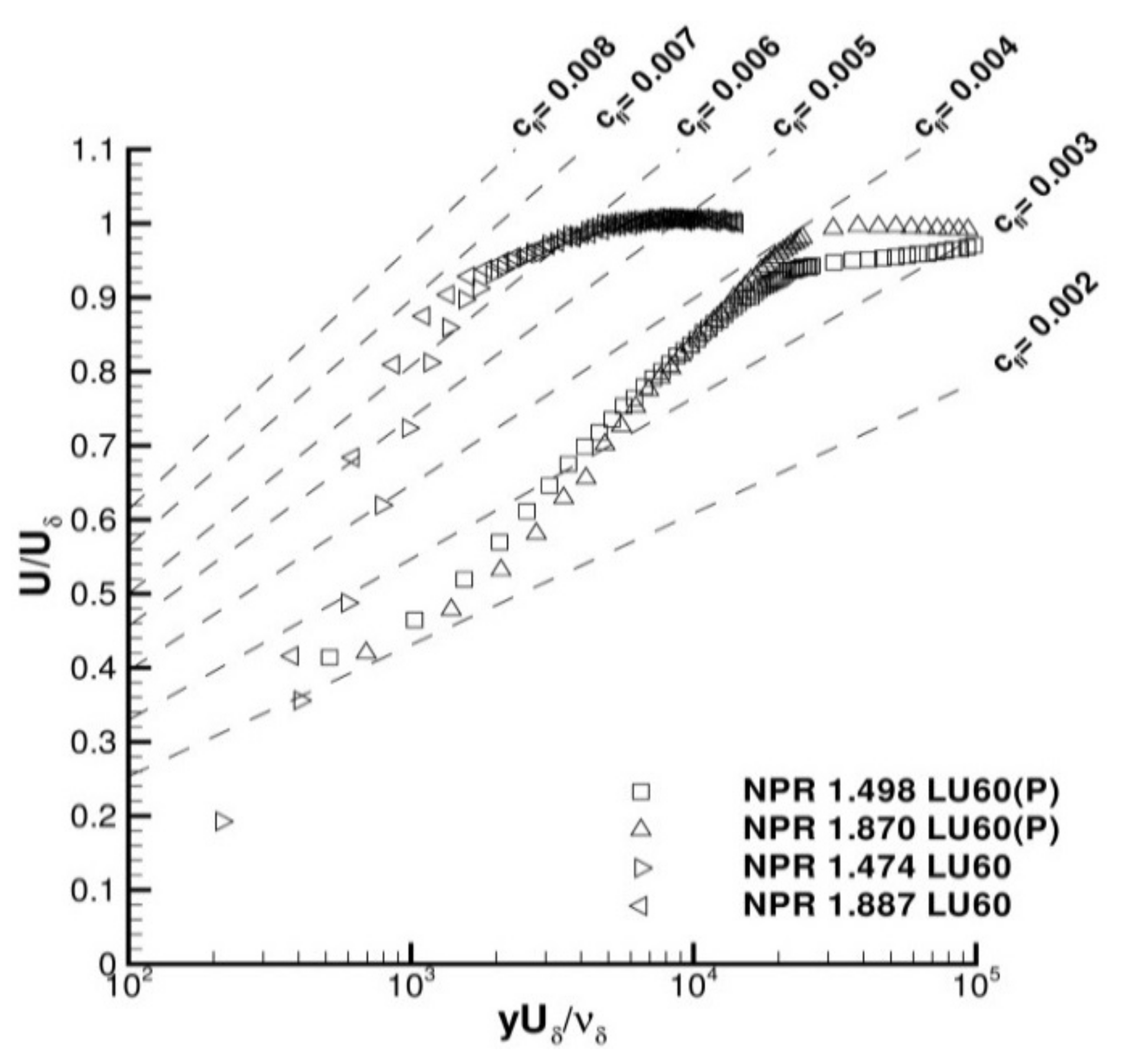
3.2. Nozzle Exit—‘Clean’ Nozzles LU48 and LU60
3.3. Nozzle Exit—The Influence of a Parallel Walled Nozzle Exit Extension—Nozzles LU48P and LU60P
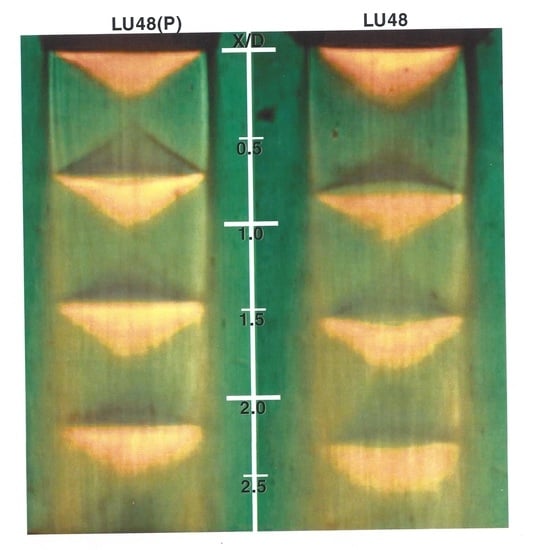
3.4. Jet Plume Region
4. Summary and Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Nomenclature
| a | Speed of sound |
| B | Constant in log-law expression |
| CFD | Computational Fluid Dynamics |
| Skin friction coefficient | |
| D | Nozzle exit diameter |
| dx, dy, dz | LDA measurement volume size |
| Kinematic shape factor = | |
| IR | Infra-Red |
| Boundary layer integral parameters | |
| LDA | Laser Doppler Anemometry |
| L | LDA focal length |
| MC | Convective Mach number |
| MJ | Jet Mach number = UJ/aJ |
| NPR | Nozzle Pressure Ratio |
| Nf | Number of LDA fringes |
| P | Total pressure |
| ps | Static pressure |
| RANS | Reynolds Averaged Navier Stokes |
| ReJ | Jet Reynolds number |
| Reθi | Momentum thickness Reynolds number |
| Rw | Radius of pipe wall |
| s | LDA fringe spacing |
| t | Static temperature |
| T | Total temperature |
| U | Axial mean velocity |
| urms | Axial turbulent rms velocity |
| u+ | Non-dimensional log-law velocity |
| u′v′ | Reynolds shear stress |
| uτ | wall friction velocity = |
| xpc | Potential core length |
| y | Distance normal to wall |
| y+ | Non-dimensional log-law wall distance |
| Greek symbols | |
| Overall boundary layer thickness | |
| Kinematic displacement thickness | |
| κ | Von Karman constant |
| λG | LDA green beam wavelength |
| λB | LDA blue beam wavelength |
| ρ | Density |
| θ | LDA beam angle |
| θi | Kinematic momentum thickness |
| τw | Wall shear stress |
| μ | Absolute viscosity |
| υ | Kinematic viscosity |
| Subscripts | |
| J | Jet properties |
| δ | Boundary layer edge properties |
| amb | Ambient property |
| 1, 2 | Fast and slow moving streams |
References
- Papadopolous, G.; Pitts, W.M. Scaling the near-field centreline mixing behaviour of axisymmetric turbulent jets. AIAA J. 1998, 36, 1635–1642. [Google Scholar] [CrossRef]
- Bradshaw, P. The effect of initial condition on the development of a free shear layer. J. Fluid Mech. 1966, 26, 225–236. [Google Scholar] [CrossRef]
- Ricou, F.P.; Spalding, D.B. Measurements of entrainment by axisymmetrical turbulent jets. J. Fluid Mech. 1961, 11, 21–32. [Google Scholar] [CrossRef]
- Pitts, W.M. Reynolds number effects on the mixing behaviour of axisymmetric turbulent jets. Exp. Fluids 1991, 11, 135–141. [Google Scholar] [CrossRef]
- Xu, G.; Antonia, R.A. Effect of initial conditions on the temperature field of a turbulent round jet. Int. Commun. Heat Mass Transf. 2002, 29, 1057–1068. [Google Scholar] [CrossRef]
- Papamoschou, D.; Roshko, A. The compressible turbulent shear layer: An experimental study. J. Fluid Mech. 1988, 197, 453–477. [Google Scholar] [CrossRef]
- Barone, M.F.; Oberkampf, W.L.; Blottner, F.G. Validation case study: Prediction of compressible turbulent mixing layer growth rate. AIAA J. 2006, 44, 1488–1497. [Google Scholar] [CrossRef]
- Sarkar, S.; Lakshmanan, B. Application of a Reynolds stress turbulence model to the compressible shear layer. AIAA J. 1991, 29, 743–749. [Google Scholar] [CrossRef]
- Yoder, D.A.; DeBonis, J.; Georgiadis, N.J. Modelling of turbulent shear free flows. Comput. Fluids 2015, 117, 212–232. [Google Scholar] [CrossRef]
- Lau, J.C. Mach number and temperature effects on jets. AIAA J. 1980, 18, 609–610. [Google Scholar] [CrossRef]
- Lau, J.C. Effects of exit Mach number and temperature on mean flow and turbulence characteristics in round jets. J. Fluid Mech. 1981, 105, 193–218. [Google Scholar] [CrossRef]
- Feng, T.; McGuirk, J.J. Measurements in the annular shear layer of high subsonic and underexpanded round jets. Exp. Fluids 2016, 57, 7. [Google Scholar] [CrossRef]
- Hill, W.C.; Jenkins, R.C.; Gilbert, B.L. Effects of the Initial Boundary Layer State on Turbulent Jet Mixing; Technical Report AFAPL-TR_75–67; Grumman Aerospace Corp.: New York, NY, USA, 1975. [Google Scholar]
- Hill, W.G.; Jenkins, R.C.; Gilbert, B.L. Effects of the initial boundary layer state on turbulent jet mixing. AIAA J. 1976, 14, 1513–1514. [Google Scholar] [CrossRef]
- Lepicovsky, J.; Ahuja, K.K.; Brown, W.H.; Salikuddin, M.; Morris, P.J. Acoustically Excited Heated Jets; NASA Contractor Report 4129; NASA Lewis Research Centre: Hampton, VA, USA, 1988.
- Lepicovsky, J. The role of nozzle exit boundary layer velocity gradient in mixing entrainment of free shear flows. In Proceedings of the 3rd ASCE/ASME Mechanics Conference, La Jolla, CA, USA, 9–12 July 1989. [Google Scholar]
- Narasimha, R.; Sreenivasan, K.R. Re-laminarisation in highly accelerated turbulent boundary layers. J. Fluid Mech. 1973, 61, 417–447. [Google Scholar] [CrossRef]
- Warnack, D.; Fernholz, H.H. The effects of a favourable pressure gradient and of the Reynolds number on an incompressible axisymmetric turbulent boundary layer—1: The turbulent boundary layer. J. Fluid Mech. 1998, 359, 329–356. [Google Scholar] [CrossRef]
- Warnack, D.; Fernholz, H.H. The effects of a favourable pressure gradient and of the Reynolds number on an incompressible axisymmetric turbulent boundary layer—2: The boundary layer with re-laminarisation. J. Fluid Mech. 1998, 359, 357–381. [Google Scholar] [CrossRef]
- Georgiadis, N.J.; DeBonis, J.R. Navier-Stokes analysis methods for turbulent flows with application to aircraft exhaust nozzle. Prog. Aerosp. Sci. 2006, 42, 377–418. [Google Scholar] [CrossRef]
- Dash, S.M.; Brinkman, K.W.; Ott, J.D. Turbulence modelling advances and validation for high speed aeropropulsive flows. Aeoacoustics 2012, 11, 813–851. [Google Scholar] [CrossRef]
- Bridges, J.; Wernet, M.P. Establishing consensus turbulence statistics for turbulent hot jets. In Proceedings of the 16th AIAA/CEAS Aeroacoustics Conference, Stockholm, Sweden, 7–9 June 2010. [Google Scholar]
- Feng, T.; McGuirk, J.J. LDA measurements of underexpanded jet flow from an axisymmetric nozzle with solid tabs. In Proceedings of the 3rd AIAA Flow Control Conference, San Francisco, CA, USA, 5–8 June 2006. paper AIAA-2006–3702. [Google Scholar]
- Antonia, R.A.; Zhao, Q. Effect of initial conditions on a circular jet. Exp. Fluids 2001, 31, 319–323. [Google Scholar] [CrossRef]
- Morris, S.C.; Foss, J.C. Turbulent boundary layer to single stream shear layer: The transition region. J. Fluid Mech. 2003, 494, 187–221. [Google Scholar] [CrossRef]
- Winter, K.G.; Gaudet, L. Turbulent Boundary Layer Studies at High Reynolds Numbers and Mach Numbers between 0.2 and 2.8; Tech. Report 7213; Aeronautical Research Council Reports and Memoranda: London, UK, 1973. [Google Scholar]
- Gaudet, L. Experimental investigation of a turbulent boundary layer at high Reynolds numbers and a Mach number of 0.8. Aeronaut. J. 1986, 90, 83–94. [Google Scholar]
- Clauser, F.H. Turbulent boundary layers in adverse pressure gradients. J. Aeronaut. Sci. 1951, 21, 91–108. [Google Scholar] [CrossRef]
- Ower, E.; Pankhurst, R.C. The Measurement of Airflow, 5th ed.; Pergamon Press: Oxford, UK, 1977. [Google Scholar]
- McLaughlin, D.K.; Tiedermann, W.G. Biasing correction for individual realisation of laser anemometer measurements in turbulent flows. Phys. Fluids 1973, 16, 2082–2088. [Google Scholar] [CrossRef]
- Fernholz, H.H.; Finley, P.J. The incompressible zero pressure gradient turbulent boundary layer: An assessment of the data. Prog. Aerosp. Sci. 1996, 32, 241–311. [Google Scholar] [CrossRef]
- Young, A.D. Boundary Layers; BSP Professional Books; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1989. [Google Scholar]
- Power, O.; Kerherve, F.; Fitzpatrick, J.; Jordan, P. Measurements of turbulence statistics in high subsonic jets. In Proceedings of the 10th AIAA/CEAS Aeroacoustics Conference, Manchester, UK, 10–12 May 2004. [Google Scholar]



















| Beam Colour | L (mm) | θ (°) | s (μm) | Nf | dx (mm) | dy (mm) | dz (mm) |
|---|---|---|---|---|---|---|---|
| Green beam (λG = 514.5 nm) | 310 | 7.01 | 4.04 | 18 | 0.076 | 0.076 | 1.192 |
| Blue beam (λB = 488.0 nm) | 310 | 7.01 | 3.84 | 18 | 0.072 | 0.072 | 1.131 |
| Parameter | NPR = 1.50 | NPR = 1.88 | ||
|---|---|---|---|---|
| LU60 | LU60P | LU60 | LU60P | |
| θi (mm) | 0.0248 | 0.362 | 0.0177 | 0.239 |
| Reθi | 442 | 7494 | 430 | 6852 |
| H12i | 2.00 | 1.22 | 2.02 | 1.22 |
| (mm) | 0.065 | 0.44 | ||
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Trumper, M.T.; Behrouzi, P.; McGuirk, J.J. Influence of Nozzle Exit Conditions on the Near-Field Development of High Subsonic and Underexpanded Axisymmetric Jets. Aerospace 2018, 5, 35. https://doi.org/10.3390/aerospace5020035
Trumper MT, Behrouzi P, McGuirk JJ. Influence of Nozzle Exit Conditions on the Near-Field Development of High Subsonic and Underexpanded Axisymmetric Jets. Aerospace. 2018; 5(2):35. https://doi.org/10.3390/aerospace5020035
Chicago/Turabian StyleTrumper, Miles T., Parviz Behrouzi, and James J. McGuirk. 2018. "Influence of Nozzle Exit Conditions on the Near-Field Development of High Subsonic and Underexpanded Axisymmetric Jets" Aerospace 5, no. 2: 35. https://doi.org/10.3390/aerospace5020035
APA StyleTrumper, M. T., Behrouzi, P., & McGuirk, J. J. (2018). Influence of Nozzle Exit Conditions on the Near-Field Development of High Subsonic and Underexpanded Axisymmetric Jets. Aerospace, 5(2), 35. https://doi.org/10.3390/aerospace5020035