Higher Order Sliding Mode Control of MIMO Induction Motors: A New Adaptive Approach
Abstract
:1. Introduction
2. Problem Formulation
- Reducing the amplitude of the switching gain.
- Eliminating high frequency switching.
- ; i.e., the input and output dimensions are equal,
- All are functions,
- All are functions,
- .
3. Controller Design
4. Adaptive Approach
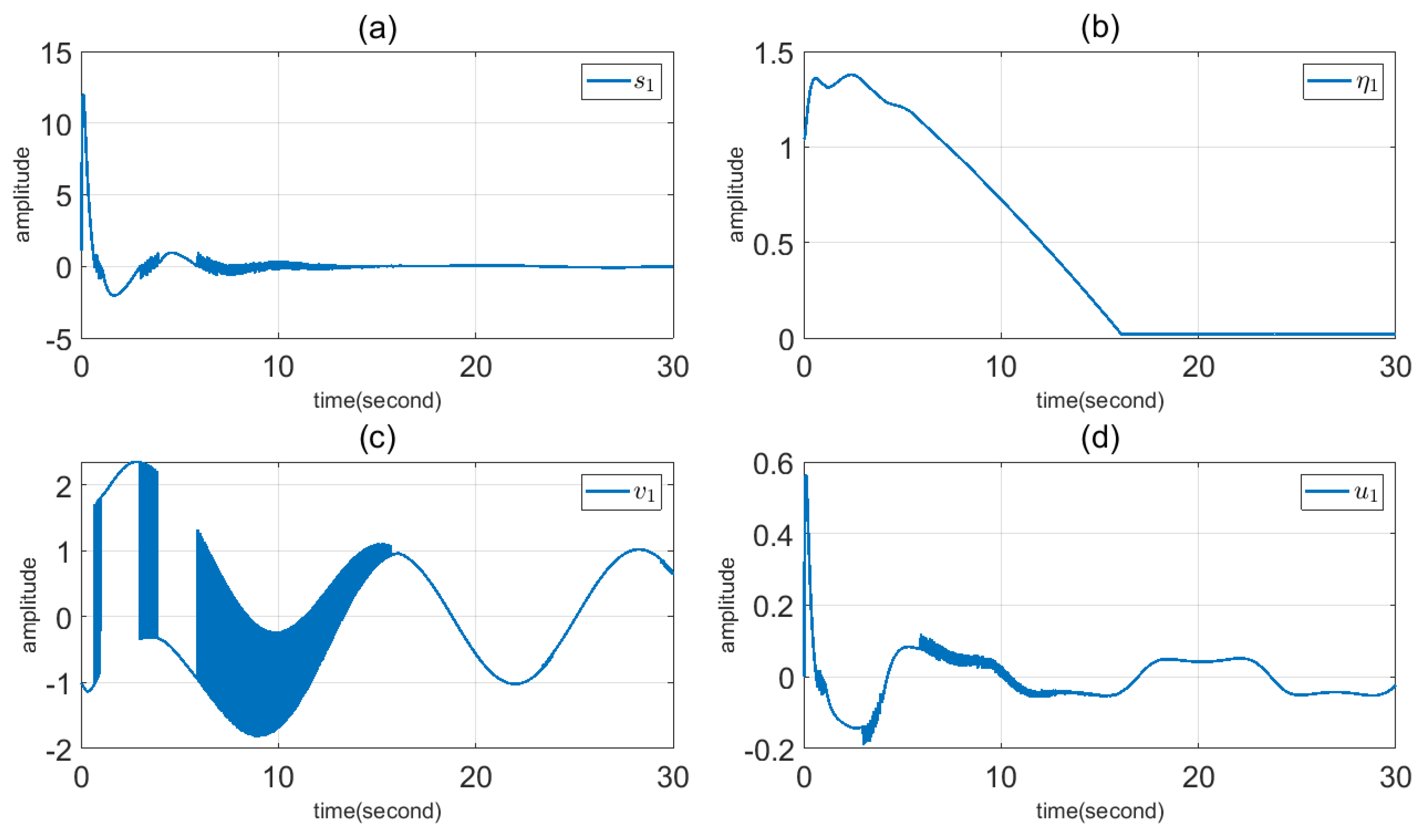
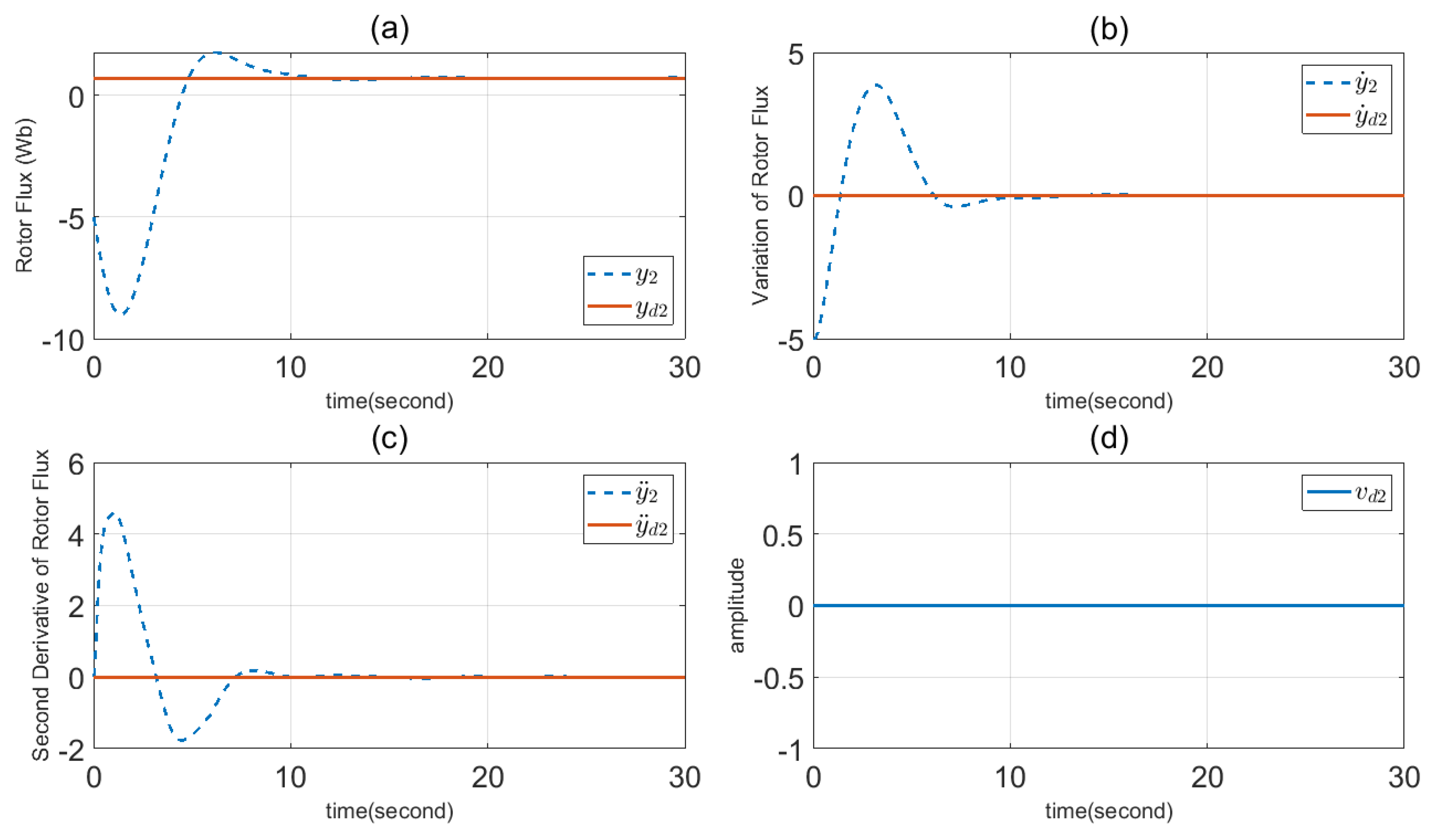
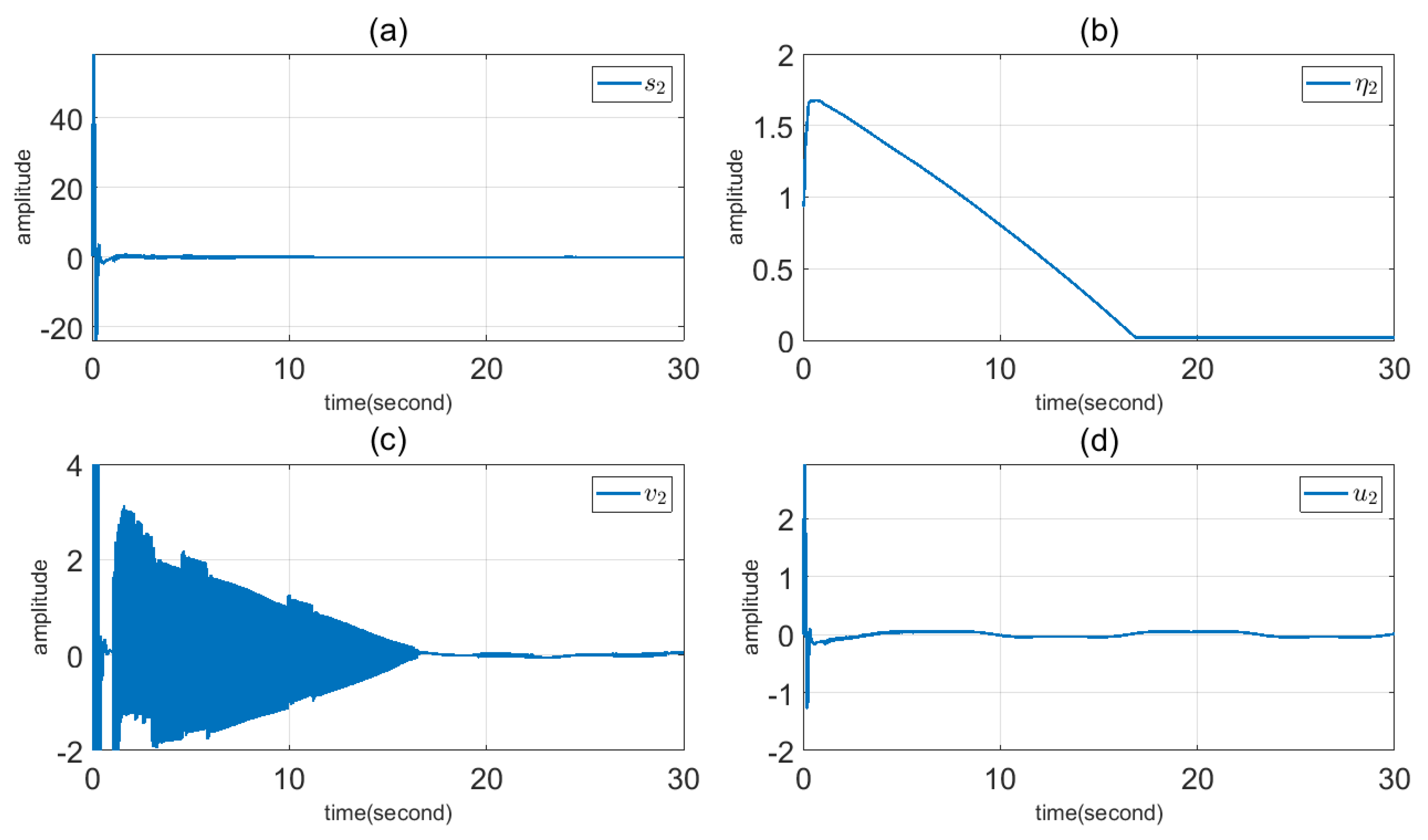
5. Simulation Results
- Calculate and (or one can set ).
- Calculate and also .
- Calculate the sliding surface using Equation (9).
- Calculate the switching gain using Equation (23).
- Calculate via Equation (14).
- Calculate based on the previous parameters.
- Calculate the elements of input vector by numerical integration.
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Young, K.D.; Utkin, V.I.; Ozguner, U. A control engineer’s guide to sliding mode control. IEEE Trans. Control Syst. Technol. 1999, 7, 328–342. [Google Scholar] [CrossRef]
- Li, Y.; Zhang, H.; Xie, X.; Xia, J. Stability analysis of a cart-pendulum model with variable convergence rate: A sliding mode control approach for impulsive stochastic systems. Chaos Solitons Fractals 2023, 175, 114044. [Google Scholar] [CrossRef]
- Rsetam, K.; Al-Rawi, M.; Cao, Z. Robust adaptive active disturbance rejection control of an electric furnace using additional continuous sliding mode component. ISA Trans. 2022, 130, 152–162. [Google Scholar] [CrossRef] [PubMed]
- Liu, K.; Wang, Y.; Ji, H.; Wang, S. Adaptive saturated tracking control for spacecraft proximity operations via integral terminal sliding mode technique. Int. J. Robust Nonlinear Control 2021, 31, 9372–9396. [Google Scholar] [CrossRef]
- Zhao, K.; Liu, W.; Zhou, R.; Dai, W.; Wu, S.; Qiu, P.; Yin, Y.; Jia, N.; Yi, J.; Huang, G. Model-free fast integral terminal sliding-mode control method based on improved fast terminal sliding-mode observer for PMSM with unknown disturbances. ISA Trans. 2023, in press. [Google Scholar] [CrossRef]
- Zhang, L.; Tao, R.; Zhang, Z.-X.; Chien, Y.-R.; Bai, J. PMSM non-singular fast terminal sliding mode control with disturbance compensation. Inf. Sci. 2023, 642, 119040. [Google Scholar] [CrossRef]
- Shao, K.; Zheng, J.; Huang, K.; Wang, H.; Man, Z.; Fu, M. Finite-time control of a linear motor positioner using adaptive recursive terminal sliding mode. IEEE Trans. Ind. Electron. 2020, 67, 6659–6668. [Google Scholar] [CrossRef]
- Slotine, J.-J.E.; Li, W. Applied Nonlinear Control; Prentice-Hall: Hoboken, NJ, USA, 1991. [Google Scholar]
- Perruquetti, W.; Pierre-Barbot, J. Sliding Mode Control in Engineering; Marcel Dekker: New York, NY, USA, 2002. [Google Scholar]
- Gao, W.; Hung, J.C. Variable structure control of nonlinear systems: A new approach. IEEE Trans. Ind. Electron. 1993, 40, 45–55. [Google Scholar]
- Hansen, A.; Li, Y.; Hedrick, J.K. Invariant sliding domains for constrained linear receding horizon tracking control. IFAC J. Syst. Cont. 2017, 2, 12–17. [Google Scholar] [CrossRef]
- Su, J.-P.; Wang, C.-C. Complementary sliding control of non-linear systems. Inter. J. Cont. 2002, 75, 360–368. [Google Scholar] [CrossRef]
- Bartolini, G.; Pydynowski, P. An improved, chattering free, V.S.C. scheme for uncertain dynamical systems. IEEE Trans. Automat. Contr. 1996, 41, 1220–1226. [Google Scholar] [CrossRef]
- Bartolini, G.; Ferrara, A.; Usai, E.; Utkin, V.I. On multi-input chattering-free second-order sliding mode control. IEEE Trans. Automat. Contr. 2000, 45, 1711–1717. [Google Scholar] [CrossRef]
- Boiko, I.; Fridman, L.; Pisano, A.; Usai, E. Analysis of chattering in systems with second-order sliding modes. IEEE Trans. Automat. Contr. 2007, 52, 2085–2102. [Google Scholar] [CrossRef]
- Chen, M.-S.; Hwang, Y.-R.; Tomizuka, M. A state-dependent boundary layer design for sliding mode control. IEEE Trans. Automat. Contr. 2002, 47, 1677–1681. [Google Scholar] [CrossRef]
- Karami-Mollaee, A.; Shojaei, A.A.; Barambones, O.; Fauzi Othman, M. Dynamic sliding mode control of pitch blade wind turbine using sliding mode observer. Trans. Inst. Meas. Control 2022, 44, 3028–3038. [Google Scholar] [CrossRef]
- Emelyanov, S.V.; Korovin, S.K.; Levant, A. Higher-order sliding modes in control systems. Comput. Math. Modeling. 1996, 7, 294–318. [Google Scholar] [CrossRef]
- Barambones, O.; Cortajarena, J.A.; Calvo, I.; Conzales de Durana, J.M.; Alcorta, P.; Karami-Mollaee, A. Real time observer and control scheme for a wind turbine system based on a high order sliding modes. J. Franklin Inst. 2021, 358, 5795–5819. [Google Scholar] [CrossRef]
- Levant, A. Sliding order and sliding accuracy in sliding mode control. Inter. J. Contr. 1993, 58, 1247–1263. [Google Scholar] [CrossRef]
- Bartolini, G.; Ferrara, A.; Usai, E. Chattering avoidance by second-order sliding mode control. IEEE Trans. Automat. Contr. 1998, 43, 241–246. [Google Scholar] [CrossRef]
- Amer, A.F.; Sallam, E.A.; Elawady, W.M. Adaptive fuzzy sliding mode control using supervisory fuzzy control for 3 DOF planar robot manipulators. Appl. Soft Comput. 2011, 11, 4943–4953. [Google Scholar] [CrossRef]
- Xu, G.; Liu, F.; Xiu, C.; Sun, L.; Liu, C. Optimization of hysteretic chaotic neural network based on fuzzy sliding mode control. Neurocomputing 2016, 189, 72–79. [Google Scholar] [CrossRef]
- Yildiz, Y.; Sabanovic, A.; Abidi, K. Sliding-mode neuro-controller for uncertain systems. IEEE Trans. Ind. Electron. 2007, 54, 1676–1685. [Google Scholar] [CrossRef]
- Hao, Z.; Xing-Yuan, W.; Xiao-Hui, L. Synchronization of complex-valued neural network with sliding mode control. J. Franklin Inst. 2016, 353, 345–358. [Google Scholar] [CrossRef]
- Yang, Y.; Yan, Y. Neural network approximation-based nonsingular terminal sliding mode control for trajectory tracking of robotic airships. Aerosp. Sci. Technol. 2016, 54, 192–197. [Google Scholar] [CrossRef]
- Wu, H.; Wang, L.; Niu, P.; Wang, Y. Global projective synchronization in finite time of nonidentical fractional-order neural networks based on sliding mode control strategy. Neurocomputing 2017, 235, 264–273. [Google Scholar] [CrossRef]
- Karami-Mollaee, A.; Tirandaz, H.; Barambones, O. State tracking control of nonlinear systems using neural adaptive dynamic sliding mode. Trans. Inst. Meas. Contr. 2019, 41, 3033–3042. [Google Scholar] [CrossRef]
- Boiko, I.; Fridman, L. Analysis of chattering in continuous sliding-mode controllers. IEEE Trans. Autom. Control 2005, 50, 1442–1446. [Google Scholar] [CrossRef]
- Boiko, I.; Fridman, L.; Iriarte, R. Analysis of chattering in continuous sliding mode control. In Proceedings of the 2005 IEEE American Control Conference (ACC), Portland, OR, USA, 8–10 June 2005; pp. 2439–2444. [Google Scholar]
- Man, Z.; Poplinsky, A.P.; Wu, H.R. A robust terminal sliding-mode control scheme for rigid robot manipulators. IEEE Trans. Autom. Control 2005, 39, 2439–2444. [Google Scholar]
- Shao, K.; Zheng, J.; Tang, R.; Li, X.; Man, Z.; Liang, B. Barrier function based adaptive sliding mode control for uncertain systems with input saturation. IEEE/ASME Trans. Mechatron. 2022, 27, 4258–4268. [Google Scholar] [CrossRef]
- Karami-Mollaee, A.; Tirandaz, H. Estimation of load torque in induction motors via dynamic sliding mode control and new nonlinear state observer. J. Mech. Sci. Tech. 2018, 32, 2283–2288. [Google Scholar] [CrossRef]
- Karami-Mollaee, A.; Tirandaz, H.; Barambones, O. Dynamic sliding mode position control of induction motors based load torque compensation using adaptive state observer. COMPEL 2018, 37, 2249–2262. [Google Scholar] [CrossRef]
- Yousef, H.A.; Wahba, M.A. Adaptive fuzzy MIMO control of induction motors. Expert Sys. Appl. 2009, 13, 4171–4175. [Google Scholar] [CrossRef]
- Lee, H.; Utkin, V.-I. Chattering suppression methods in sliding mode control systems. Annu. Rev. Contr. 2007, 31, 179–188. [Google Scholar] [CrossRef]
- Khalid, K.M.; Spurgeon, S.K. Robust MIMO water level control in interconnected twin-tanks using second order sliding mode control. Contr. Eng. Pract. 2006, 14, 375–386. [Google Scholar]
- Laghrouche, S.; Plestan, F.; Glumineau, A. Higher order sliding mode control based on optimal linear quadratic control. In Proceedings of the 2003 European Control Conference (ECC), Cambridge, UK, 1–4 September 2003. [Google Scholar]
- Levant, A. Universal SISO sliding-mode controllers with finite time convergence. IEEE Trans. Automat. Contr. 2001, 49, 1447–1451. [Google Scholar] [CrossRef]
- Levant, A. Higher-order sliding modes, differentiation and output-feedback control. Int. J. Contr. 2003, 76, 924–941. [Google Scholar] [CrossRef]
- Levant, A. Homogeneity approach to high-order sliding mode design. Automatica 2005, 41, 823–830. [Google Scholar] [CrossRef]
- Edvards, C.; Spurgeon, S. Sliding Mode Control: Theory and Applications; Taylor and Francis: Milton Park, Oxfordshire, 1998. [Google Scholar]
- Zhihong, M.; Glumineau, X.H.Y. Terminal sliding mode control of MIMO linear systems. IEEE Trans. Circuits Syst. 1997, 44, 1065–1070. [Google Scholar] [CrossRef]
- Yang, J.; Li, X.; Fei, J. Intelligent global fast terminal sliding mode control of active power filter. Mathematics 2023, 11, 919. [Google Scholar] [CrossRef]
- Khalil, H.K. Nonlinear Systems; Prentice-Hall: Englewood Cliffs, NJ, USA, 1996. [Google Scholar]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Karami-Mollaee, A.; Barambones, O. Higher Order Sliding Mode Control of MIMO Induction Motors: A New Adaptive Approach. Mathematics 2023, 11, 4558. https://doi.org/10.3390/math11214558
Karami-Mollaee A, Barambones O. Higher Order Sliding Mode Control of MIMO Induction Motors: A New Adaptive Approach. Mathematics. 2023; 11(21):4558. https://doi.org/10.3390/math11214558
Chicago/Turabian StyleKarami-Mollaee, Ali, and Oscar Barambones. 2023. "Higher Order Sliding Mode Control of MIMO Induction Motors: A New Adaptive Approach" Mathematics 11, no. 21: 4558. https://doi.org/10.3390/math11214558
APA StyleKarami-Mollaee, A., & Barambones, O. (2023). Higher Order Sliding Mode Control of MIMO Induction Motors: A New Adaptive Approach. Mathematics, 11(21), 4558. https://doi.org/10.3390/math11214558







