On Appearance of Fast or Late Self-Synchronization between Non-Ideal Sources Mounted on a Rectangular Plate Due to Time Delay
Abstract
:1. Introduction
- By the characteristic curves of the particular energy source
- By the dependence of the motion of the system on the motion of the energy source.
2. Description of the Studied System and Mathematical Formalism
2.1. Presentation of the Studied Systems
2.2. Mathematical Modelling
3. Numerical Investigation of Dynamics
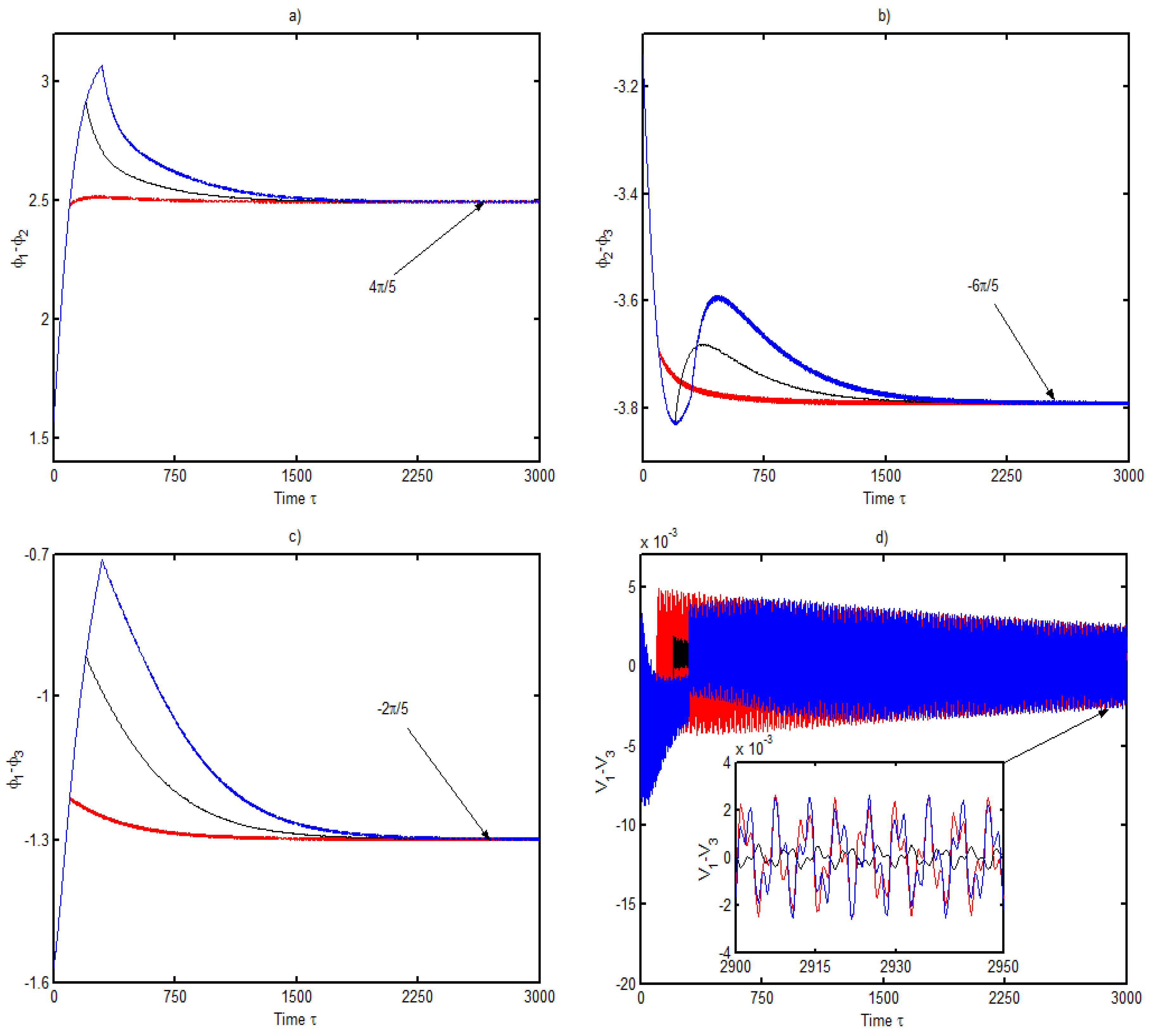
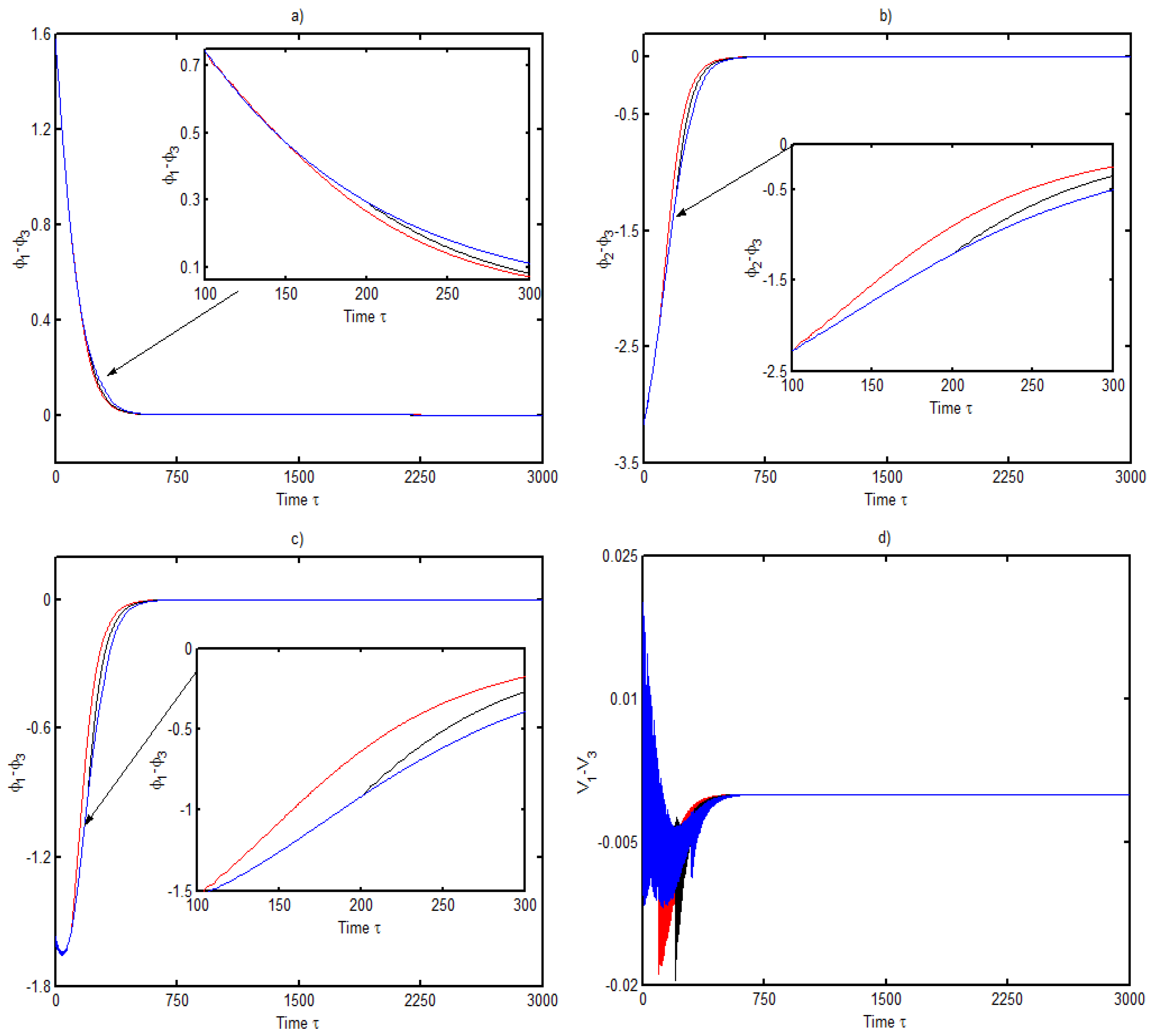
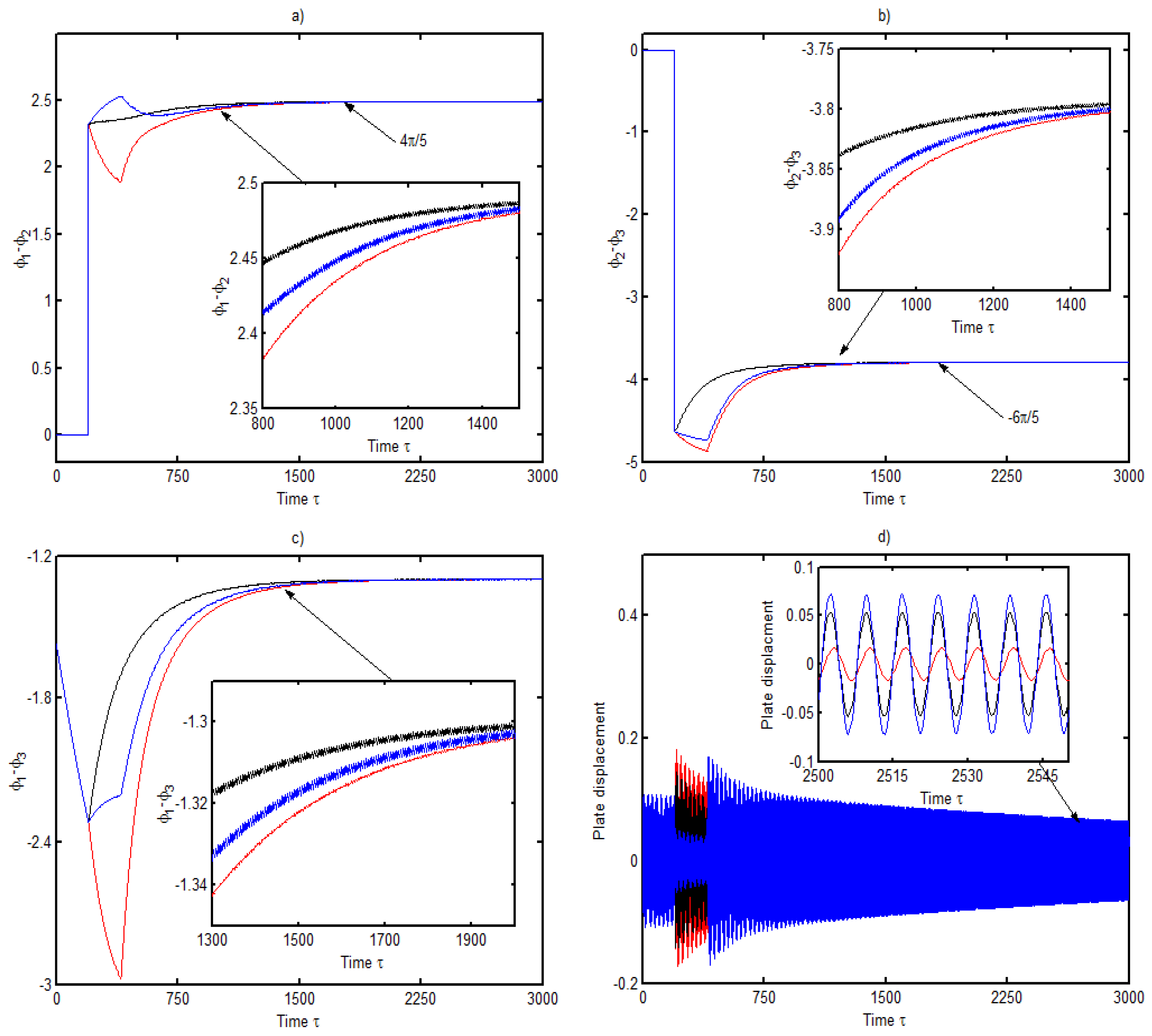
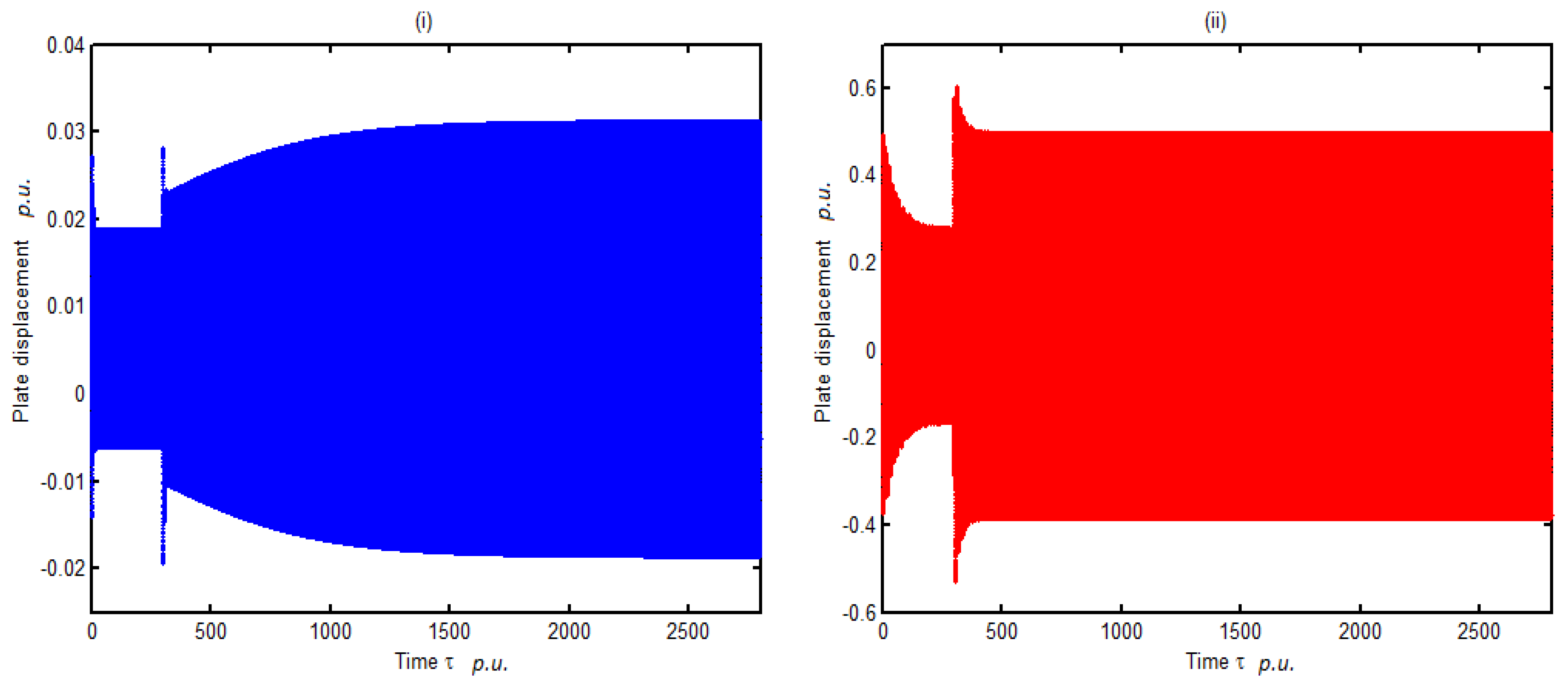
3.1. Dynamic Response of Two DC Electrical Machines
3.2. Dynamic Response of Three DC Electrical Machines
- The first and the second electrical machines start at the same time and the third starts later at ,
- The first and the third electrical machine start at the same time and the second starts later at ,
- The first electrical machine starts at and the other two start later at different time .
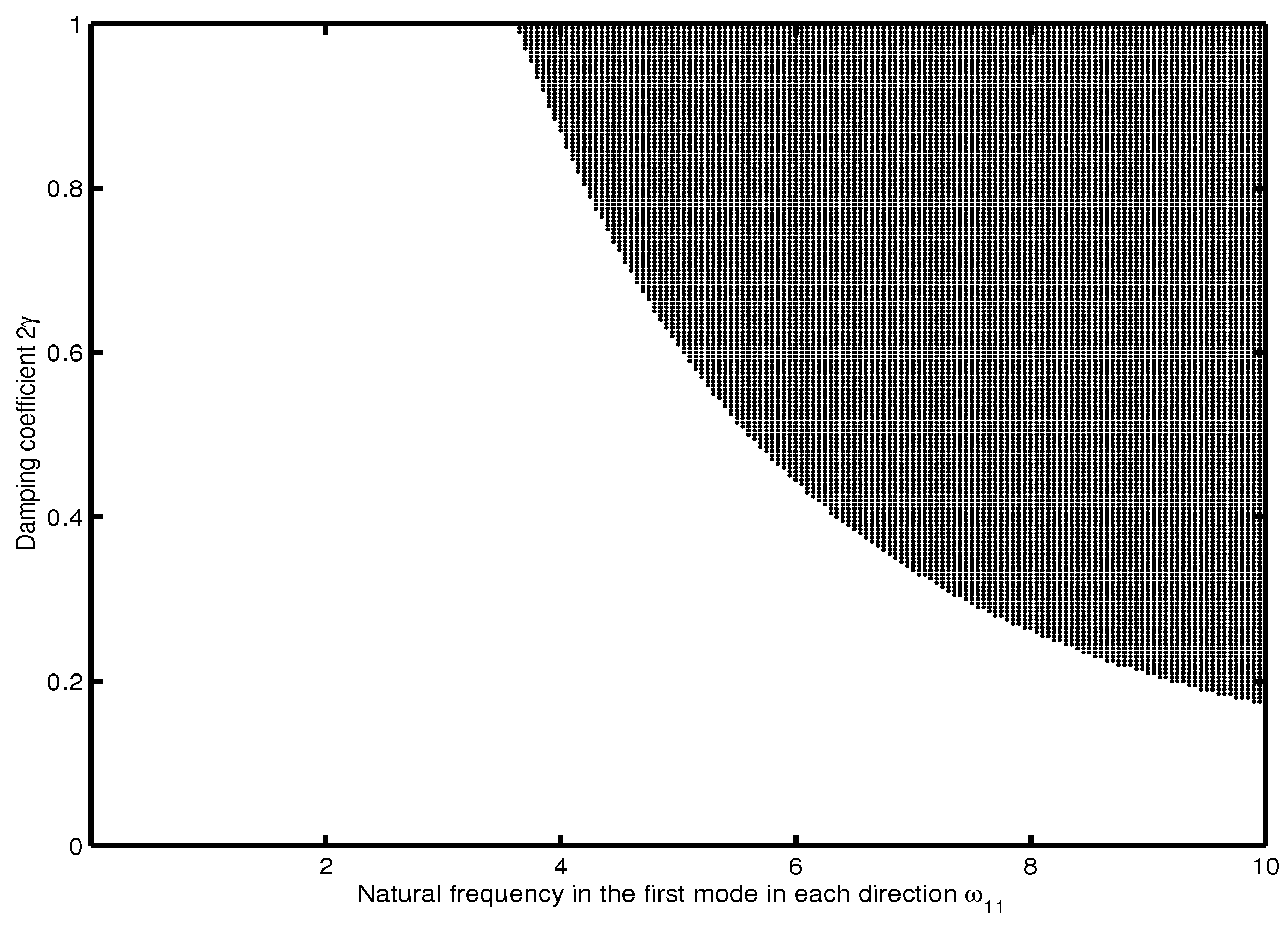
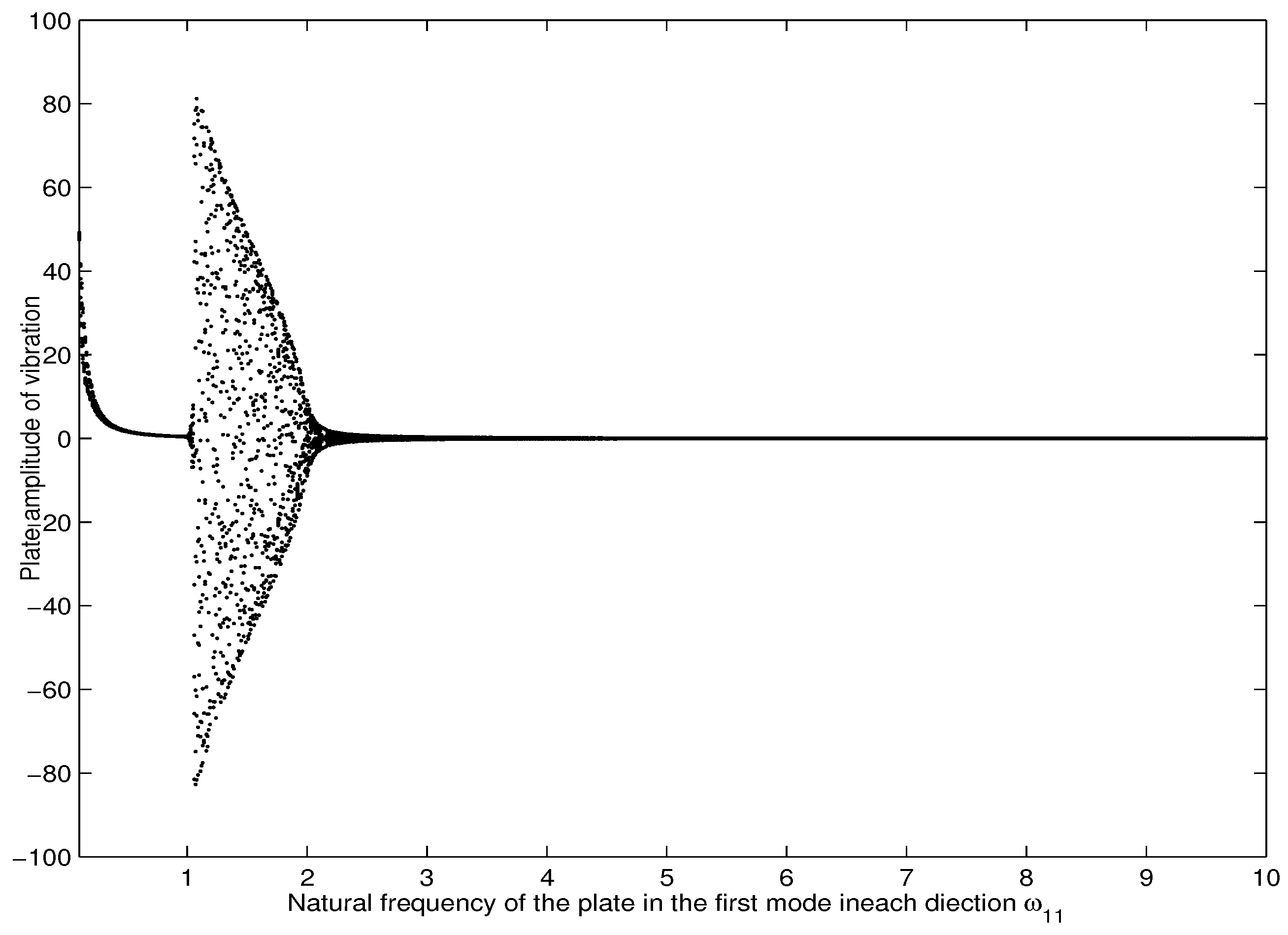
4. Stability Analysis of the System
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
Appendix B
References
- Chen, J.; Hu, W.-H.; Li, Q.-S. Nonlinear dynamics of a foldable multibeam structure with one to two internal resonances. Int. J. Mech. Sci. 2019, 150, 369–378. [Google Scholar] [CrossRef]
- Kecik, K.; Mitura, A. Energy recovery from a pendulum tuned mass damper with two independent harvesting sources. Int. J. Mech. Sci. 2020, 174, 105568. [Google Scholar] [CrossRef]
- Fan, C.-C.; Pan, M.-C. Active elimination of oil and dry whips in a rotating machine with an electromagnetic actuator. Int. J. Mech. Sci. 2011, 53, 126–134. [Google Scholar] [CrossRef]
- Wang, G.; Li, M.; Zhou, J. Modeling soft machines driven by buckling actuators. Int. J. Mech. Sci. 2019, 157, 662–667. [Google Scholar] [CrossRef]
- Nbendjo, B.R.N. Amplitude control on hinged-hinged beam using piezoelectric absorber: Analytical and numerical explanation. Int. J. Non-Linear Mech. 2009, 44, 704–708. [Google Scholar] [CrossRef]
- Tabejieu, L.M.A.; Nbendjo, B.R.N.; Dorka, U. Identification of horseshoes chaos in a cable-stayed bridge subjected to randomly moving loads. Int. J. Non-Linear Mech. 2016, 85, 62–69. [Google Scholar] [CrossRef]
- Pirmoradian, M.; Torkan, E.; Karimpour, H. Parametric resonance analysis of rectangular plates subjected to moving inertial loads via IHB method. Int. J. Mech. Sci. 2018, 142, 191–215. [Google Scholar] [CrossRef]
- Alwis, W.A.M.; Grundy, P. On the carrying capacity of rectangular plates under moving loads. Int. J. Mech. Sci. 1985, 27, 187–197. [Google Scholar] [CrossRef]
- Debbarma, R.; Chakraborty, S.; Ghosh, S.K. Optimum design of tuned liquid column dampers under stochastic earthquake load considering uncertain bounded system parameters. Int. J. Mech. Sci. 2010, 52, 1385–1393. [Google Scholar] [CrossRef]
- Balthazar, J.M.; Mook, D.T.; Weber, H.I.; Fenili, A.; Belato, D.; Felix, J.L.P. An Overview on Non-Ideal Vibrations. Meccanica 2003, 30, 613–621. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Mook, D.T. Nonlinear Oscillations; Wiley: New York, NY, USA, 2008. [Google Scholar]
- Balthazar, J.M.; Felix, J.L.P.; Brasil, R.M.L.R.F. On nonlinear dynamics of a particular portal frame foundation model, excited by a non-ideal motor. Mat. Sci. For. 2003, 440, 371–378. [Google Scholar] [CrossRef]
- Feulefack, S.E.; Djanan, A.A.N.; Nbendjo, B.R.N. Vibration absorption of a rectangular plate supporting a DC motor with a TLCD. Nonlinear Dyn. 2021, 105, 1357–1372. [Google Scholar]
- Cveticanin, L.; Zukovic, M.; Cveticanin, D. Two degree-of-freedom oscillator coupled to a non-ideal source. Int. J. Non-Linear Mech. 2017, 94, 125–133. [Google Scholar] [CrossRef]
- Djanan, A.A.N.; Nbendjo, B.R.N.; Woafo, P. Control of vibration on a hinged-hinged beam under a non-ideal excitation using RLC circuit with variable capacitance. Nonlinear Dyn. 2011, 63, 477–489. [Google Scholar]
- Balthazar, J.M.; Felix, J.L.P.; Brasil, R.M.L.R.F. Short comments on self-synchronization of two non-ideal sources supported by a flexible portal-frame strucure. J. Vib. Control 2004, 10, 1739–1748. [Google Scholar] [CrossRef]
- Balthazar, J.M.; Felix, J.L.P.; Brasil, R.M.L.R.F. Some comments on the numerical simulation of self-synchronization of four non-ideal exciters. Appl. Math. Comput. 2005, 164, 615–625. [Google Scholar] [CrossRef]
- Czolczynski, K.; Perlikowski, P.; Stefanski, A.; Kapitaniak, T. Synchronization of pendula rotating in different directions. Commun. Nonlinear Sci. Numer. Simul. 2012, 17, 3658–3672. [Google Scholar] [CrossRef]
- Czolczynski, K.; Perlikowski, P.; Stefanski, A.; Kapitaniak, T. Synchronization of slowly rotating pendulums. Int. J. Bif. Chaos 2012, 22, 1250128. [Google Scholar] [CrossRef]
- Kapitaniak, M.; Czolczynski, K.; Perlikowski, P.; Stefanski, A.; Kapitaniak, T. Synchronization of clocks. Phys. Rep. 2012, 517, 1–69. [Google Scholar] [CrossRef] [Green Version]
- Djanan, A.A.N.; Nbendjo, B.R.N.; Woafo, P. Self-synchronization of two motors on a rectangular plate and reduction of vibration. J. Vib. Control 2015, 21, 2114–2123. [Google Scholar] [CrossRef]
- Dimentberg, M.F.; Cobb, E.; Mensching, J. Self-synchronization of transient rotations in multiple shaft systems. J. Vib. Control 2001, 7, 221–232. [Google Scholar] [CrossRef]
- Blekhman, I.I. Synchronization in Science and Technology; ASME: New York, NY, USA, 1988. [Google Scholar]
- Dimentberg, M.F.; McGovern, L.; Norton, R.L.; Chapdelaine, J.; Harrison, R. Dynamics of an unbalanced shaft interacting with a limited power supply. Nonlinear Dyn. 1997, 13, 171–187. [Google Scholar] [CrossRef]
- Cveticanin, L.; Zukovic, M.; Cveticanin, D. Oscillator with variable mass excited with non-ideal source. Nonlinear Dyn. 2018, 92, 673–682. [Google Scholar] [CrossRef]
- Litak, G.; Syta, A.; Wasilewski, G.; Kudra, G.; Awrejcewicz, J. Dynamical response of a pendulum driven horizontally by a DC motor with a slider-crank mechanism. Nonlinear Dyn. 2020, 99, 1923–1935. [Google Scholar] [CrossRef]
- Djanan, A.A.N.; Nbendjo, B.R.N. Effects of two moving non-ideal sources on the dynamic of a rectangular plate. Nonlinear Dyn. 2018, 92, 645–657. [Google Scholar] [CrossRef]
- Kong, X.; Zhou, C.; Wen, B. Composite synchronization of four exciters driven by induction motors in a vibration system. Meccanica 2020, 55, 2107–2133. [Google Scholar] [CrossRef]
- Kong, X.; Jiang, J.; Zhou, C.; Xu, Q.; Chen, C. Sommerfeld effect and synchronization analysis in a simply supported beam system excited by two non-ideal induction motors. Nonlinear Dyn. 2020, 100, 2047–2070. [Google Scholar] [CrossRef]
- Djanan, A.A.N.; Nbendjo, B.R.N.; Woafo, P. Electromechanical control of vibration on a plate submitted to a non-ideal excitation. Mech. Res. Comm. 2013, 54, 72–82. [Google Scholar] [CrossRef]
- Djanan, A.A.N.; Nbendjo, B.R.N.; Woafo, P. Effect of self-synchronization of DC motors on the amplitude of vibration of a rectangular plate. Eur. Phys. J. Spec. Top. 2014, 223, 813–825. [Google Scholar] [CrossRef]
- Shaw, J.; Shaw, S.W. Non-linear resonance of an unbalanced rotating shaft with internal damping. J. Sound Vib. 1991, 147, 435–451. [Google Scholar] [CrossRef]
- Haddow, A.G.; Shaw, S.W. Centrifugal Pendulum Vibration Absorbers: An Experimental and Theoretical Investigation. Nonlinear Dyn. 2003, 34, 293–307. [Google Scholar] [CrossRef]
- Ikeda, T. Nonlinear Responses of Dual-Pendulum Dynamic Absorbers. J. Comp. Nonlinear Dyn. 2011, 6, 011012. [Google Scholar] [CrossRef]
- Dudkowski, D.; Czolczynski, K.; Kapitaniak, T. Traveling amplitude death in coupled pendula. Chaos 2019, 29, 083124. [Google Scholar] [CrossRef] [PubMed]
- Dudkowski, D.; Czolczynski, K.; Kapitaniak, T. Traveling chimera states for coupled pendula. Nonlinear Dyn. 2019, 95, 1859–1866. [Google Scholar] [CrossRef] [Green Version]
- Czolczynski, K.; Marynowski, K. Stability of symmetrical rotor supported in flexibly mounted, self-acting gas journal bearings. Wear 1996, 194, 190–197. [Google Scholar] [CrossRef]
- Tan, X.; Chen, G.; He, H.; Chen, W.; Wang, Z.; He, J.; Wang, T. Stability analysis of a rotor system with electromechanically coupled boundary conditions under periodic axial load. Nonlinear Dyn. 2021, 104, 1157–1174. [Google Scholar] [CrossRef]
- Shaw, J.; Shaw, S.W. Instabilities and bifurcations in a rotating shaft. J. Sound Vib. 1989, 132, 227–244. [Google Scholar] [CrossRef]
- Zhang, X.; Wen, B.; Zhao, C. Synchronization of three non-identical coupled exciters with the same rotating directions in a far-resonant vibrating system. J. Sound Vib. 2013, 332, 2300–2317. [Google Scholar] [CrossRef]
- Lai, V.-V.; Brunel, C.O.J.-F.; Dufrnoy, P. The critical effect of rail vertical phase response in railway curve squeal generation. Int. J. Mech. Sci. 2020, 167, 105281. [Google Scholar] [CrossRef]
- Hayashi, C. Nonlinear Oscillations in Physical Systems; Princeton University Press: New York, NY, USA, 1964. [Google Scholar]
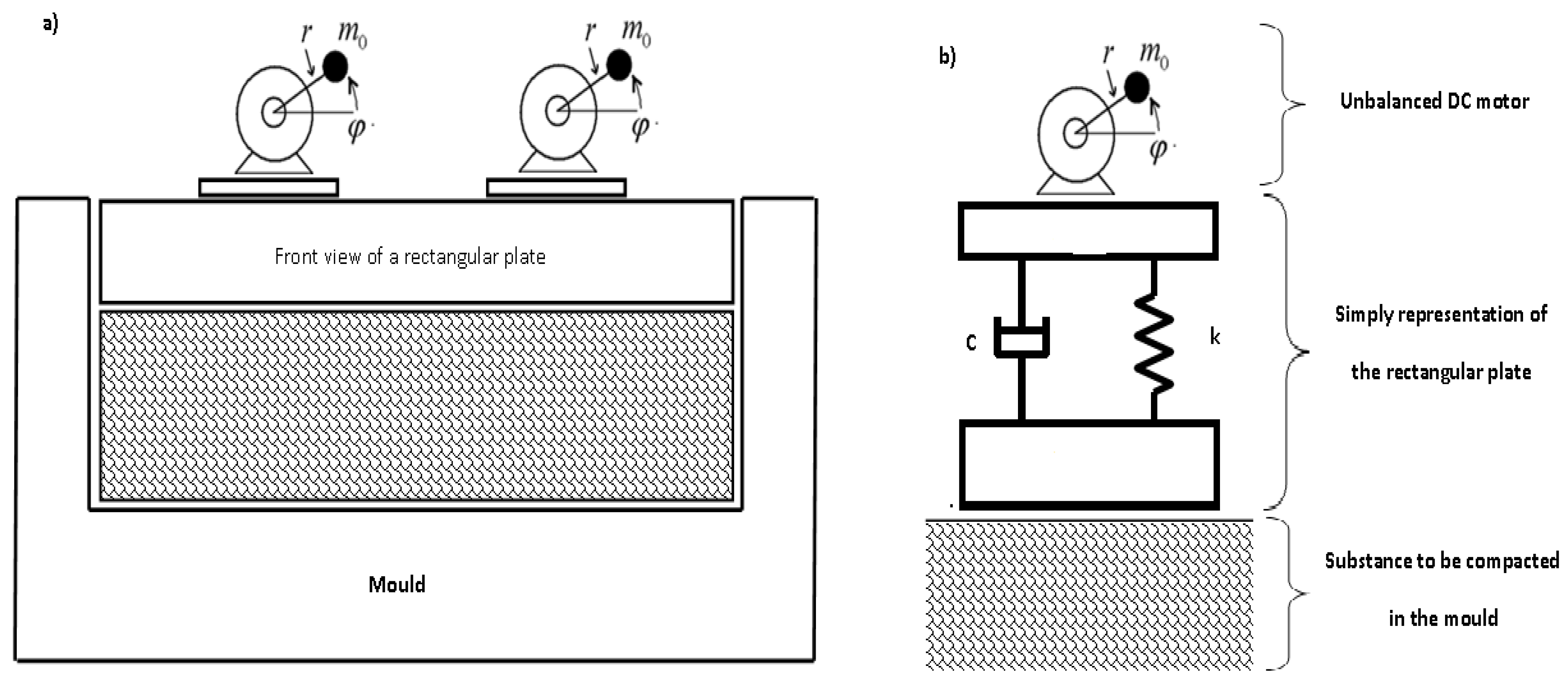

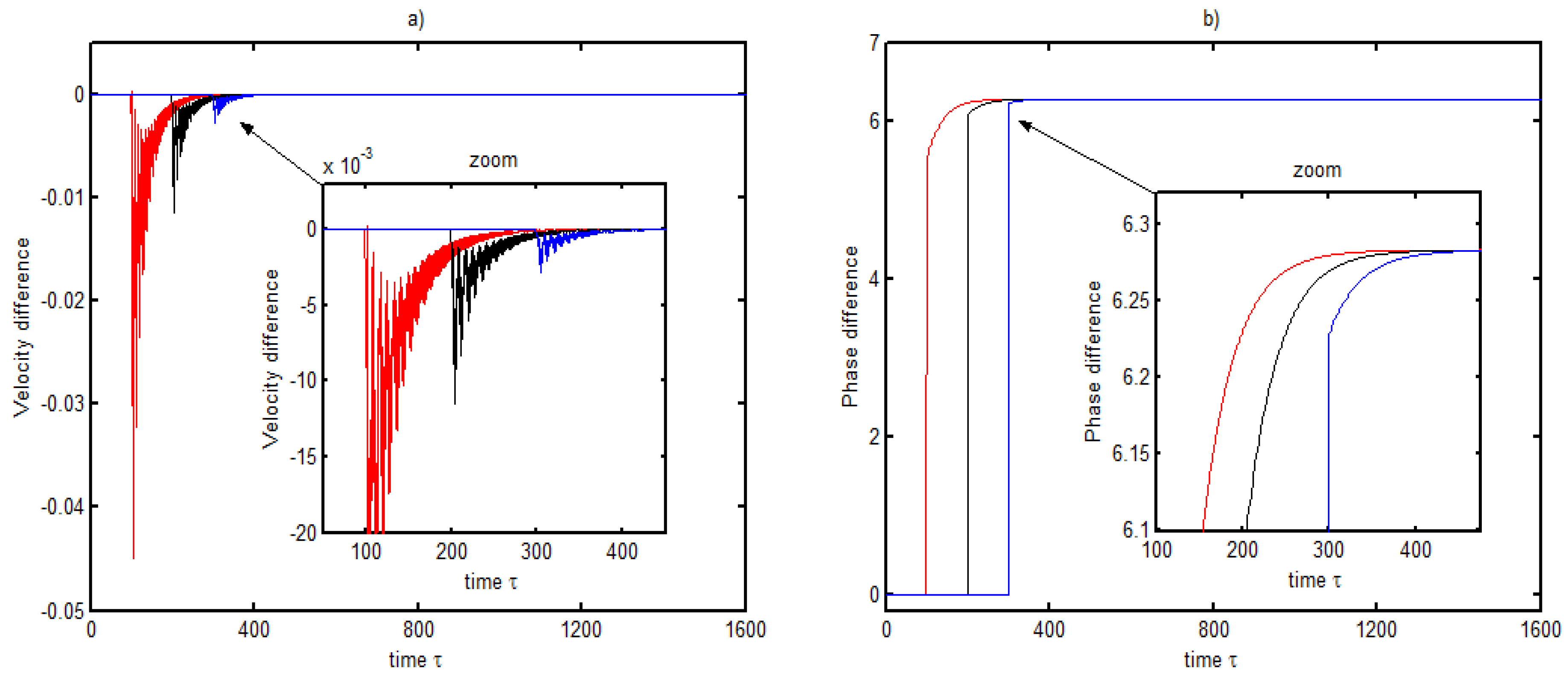
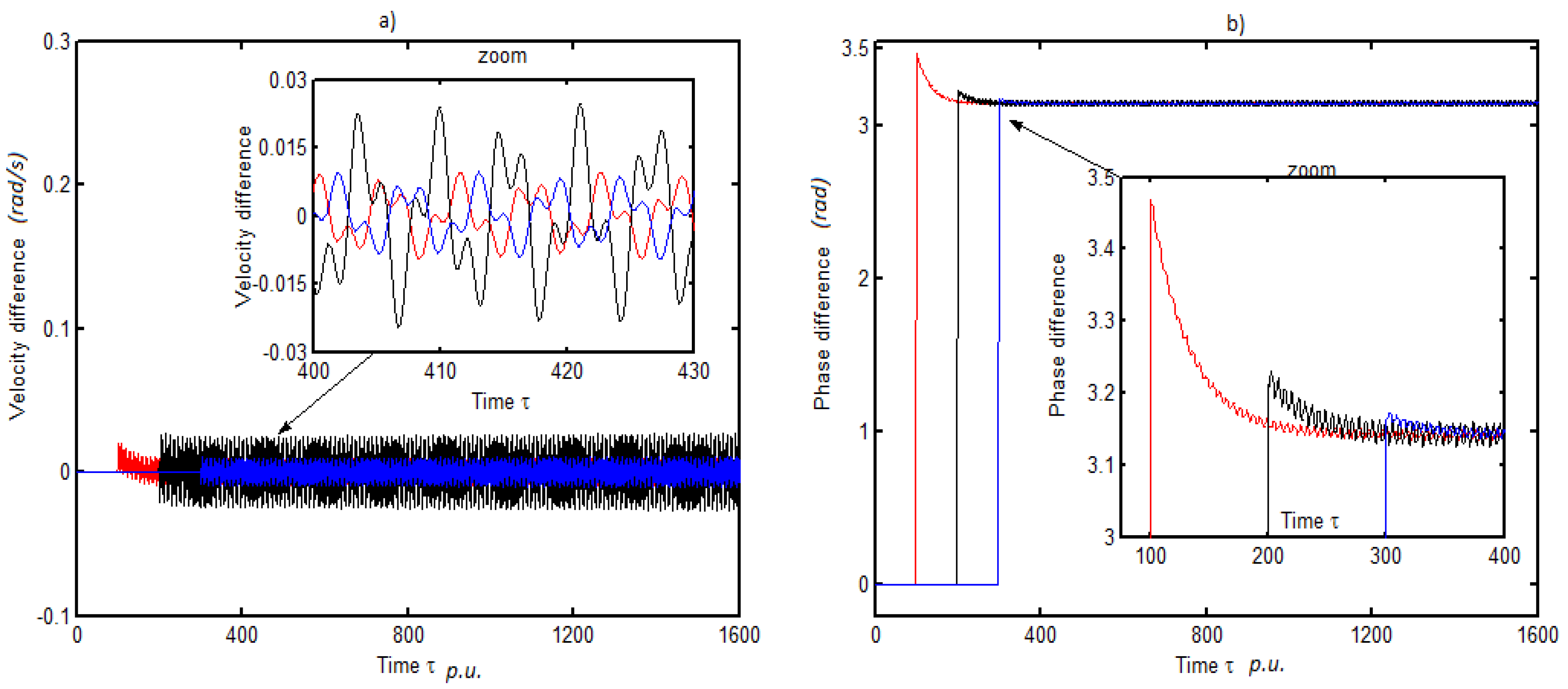
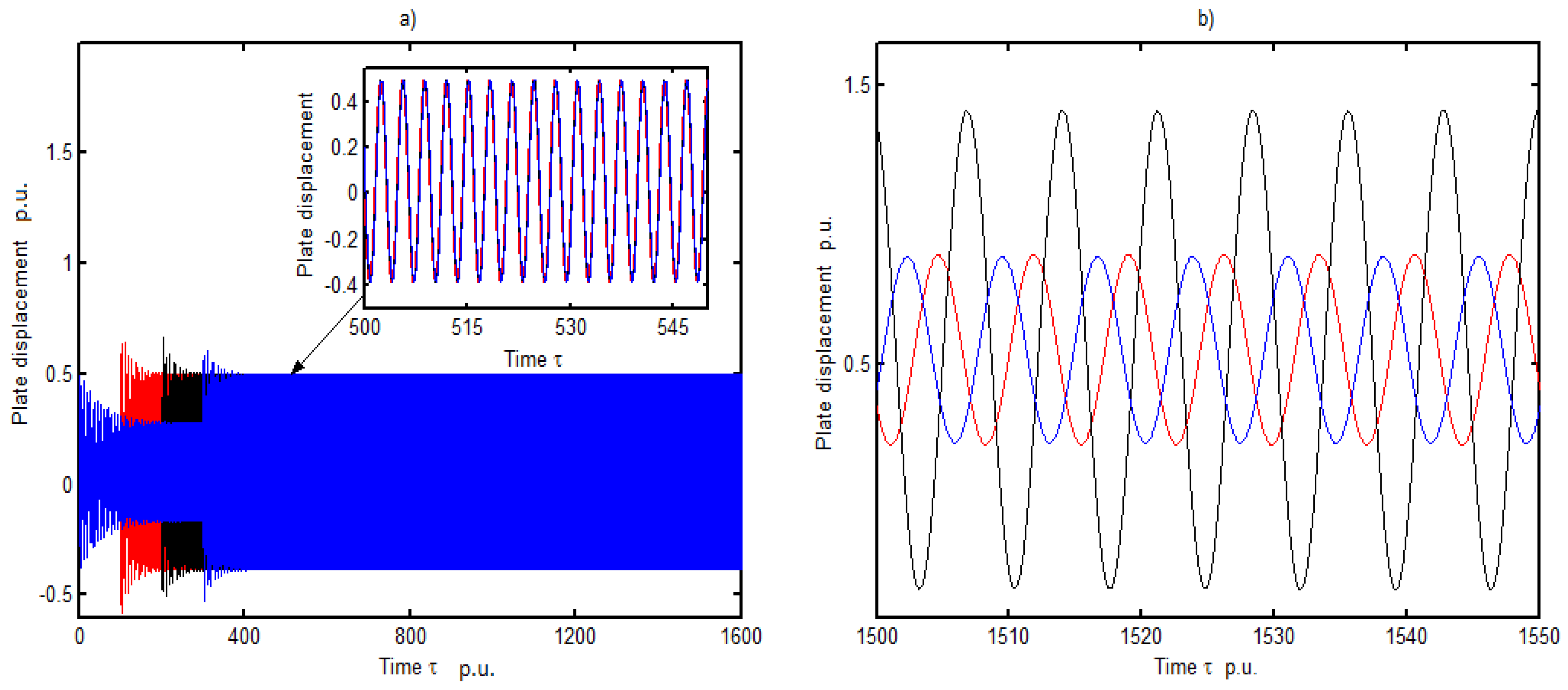

| Quantities | Notations | Values | Units |
|---|---|---|---|
| Dimensions (length, width, thickness) | a × b × h | 1239 × 619.5 × 5 | mm3 |
| Density | 2700 | ||
| Young modulus | E | 6.9 × | |
| Poisson ratio | 0.33 | - |
| Quantities | Notations | Values | Units |
|---|---|---|---|
| Unbalanced mass | g | ||
| Excentricity | cm | ||
| electrical machine mass | 1740 | g | |
| Inertia moment | kg·m2 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nanha Djanan, A.A.; Marburg, S.; Nana Nbendjo, B.R. On Appearance of Fast or Late Self-Synchronization between Non-Ideal Sources Mounted on a Rectangular Plate Due to Time Delay. Math. Comput. Appl. 2022, 27, 20. https://doi.org/10.3390/mca27020020
Nanha Djanan AA, Marburg S, Nana Nbendjo BR. On Appearance of Fast or Late Self-Synchronization between Non-Ideal Sources Mounted on a Rectangular Plate Due to Time Delay. Mathematical and Computational Applications. 2022; 27(2):20. https://doi.org/10.3390/mca27020020
Chicago/Turabian StyleNanha Djanan, Armand Anthelme, Steffen Marburg, and Blaise Roméo Nana Nbendjo. 2022. "On Appearance of Fast or Late Self-Synchronization between Non-Ideal Sources Mounted on a Rectangular Plate Due to Time Delay" Mathematical and Computational Applications 27, no. 2: 20. https://doi.org/10.3390/mca27020020






