Effect of Support Stiffness Nonlinearity on the Low-Frequency Vibro-Acoustic Characteristics for a Mechanical Equipment—Floating Raft—Underwater Cylindrical Shell Coupled System
Abstract
:1. Introduction
2. Materials and Methods
2.1. Model of a Mechanical Equipment—Floating Raft—Cylindrical Shell—Underwater Acoustic Field Coupled System
2.2. State Space Model of the Coupled System
2.3. Expression of Nonlinear Stiffness for Vibration Isolation Supports
2.4. Evaluation Index of Vibro-Acoustic Transfer Characteristics for the Nonlinear Coupled System
3. Results and Discussion
3.1. Modal Parameter Identification for the Cylindrical Shell—Underwater Acoustic Field Coupled Subsystem
3.2. Effect of Nonlinear Stiffness with Softening Characteristic on the Low-Frequency Vibro-Acoustic Characteristics for the Coupled System
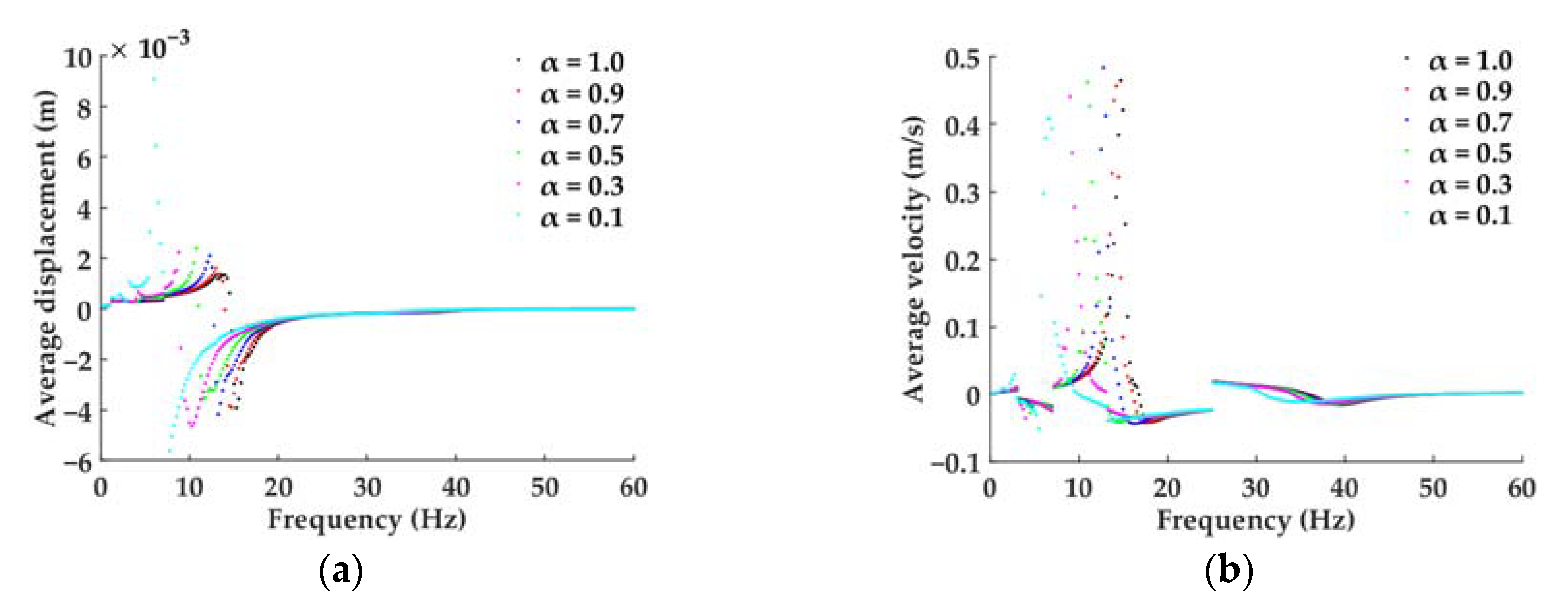
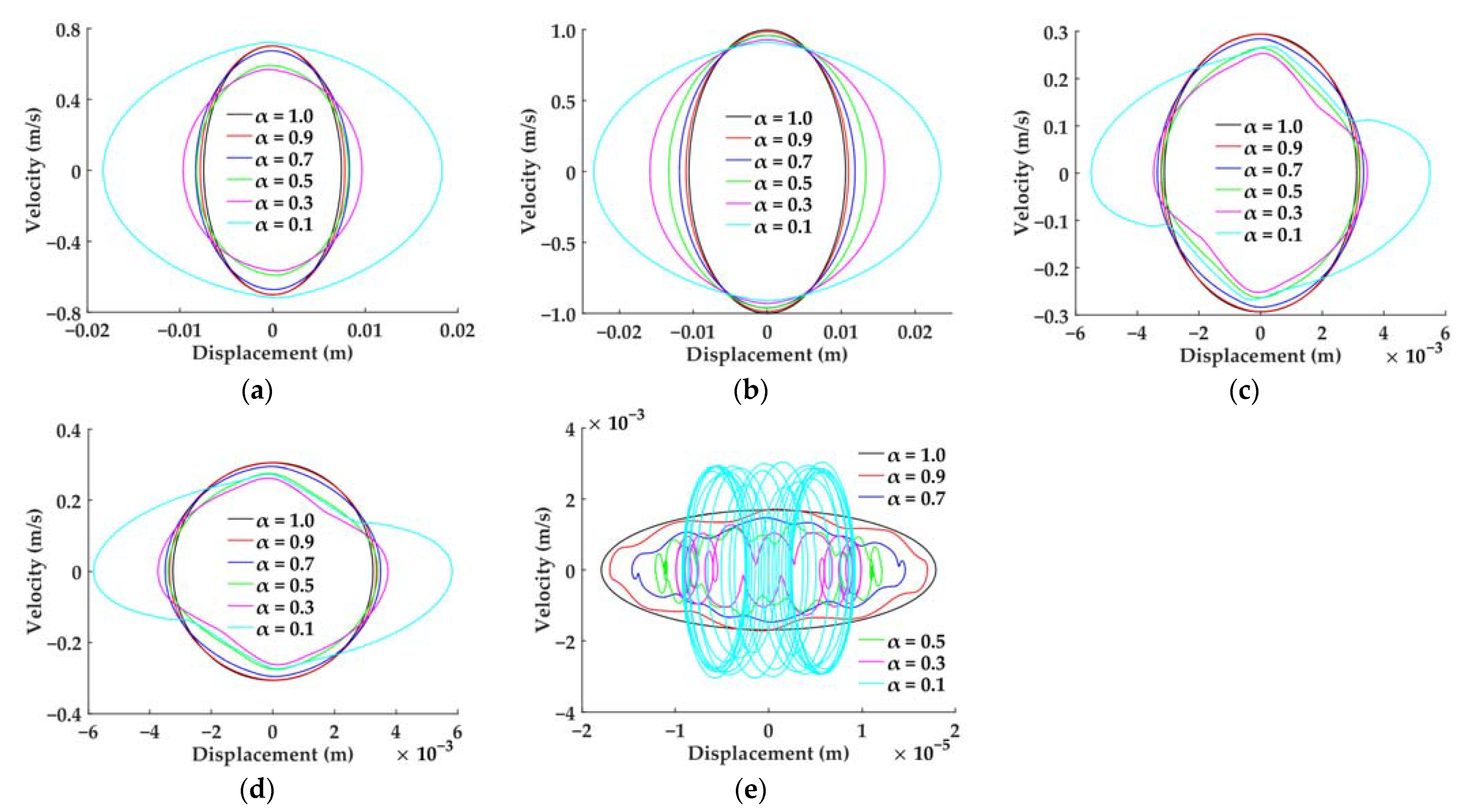
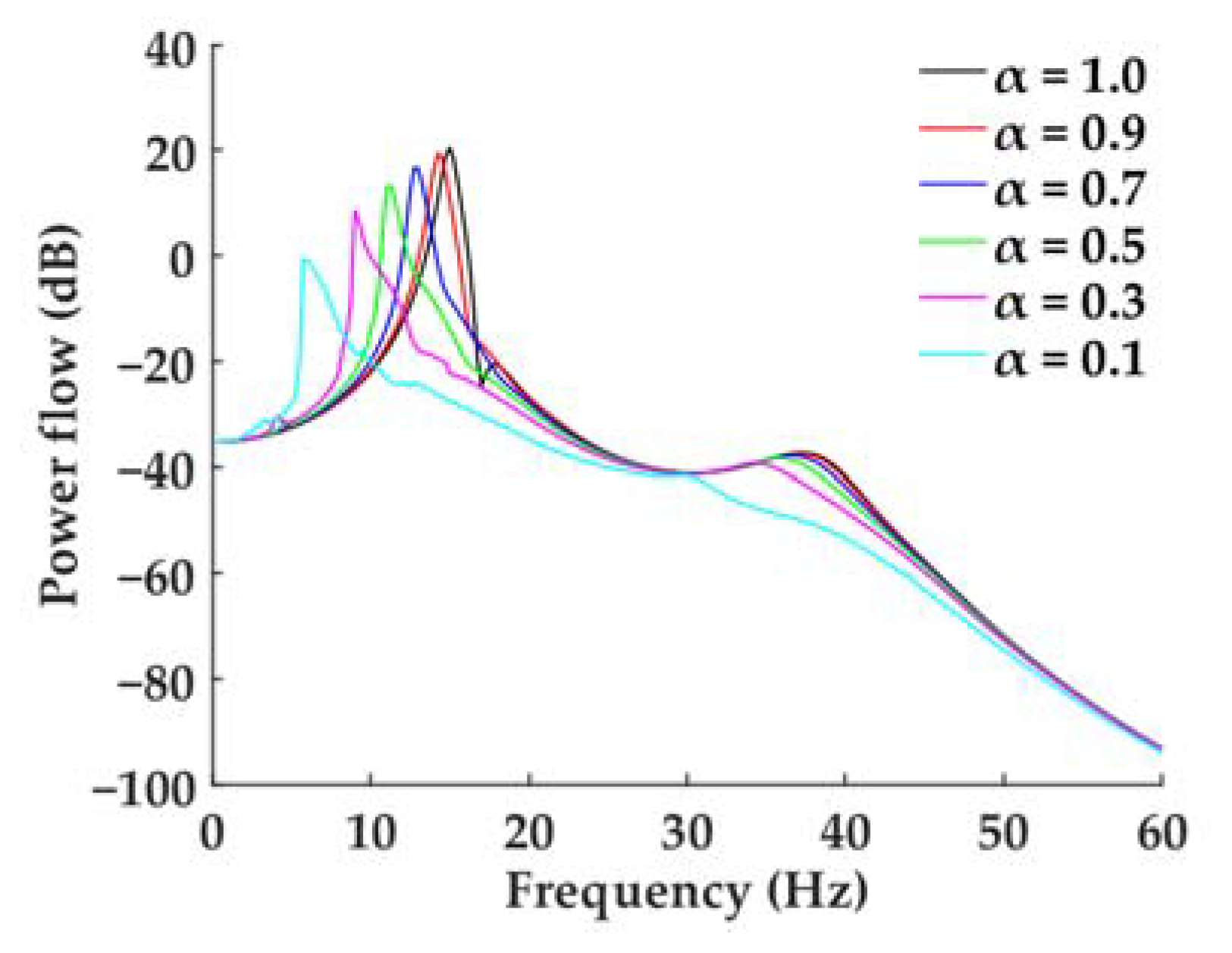
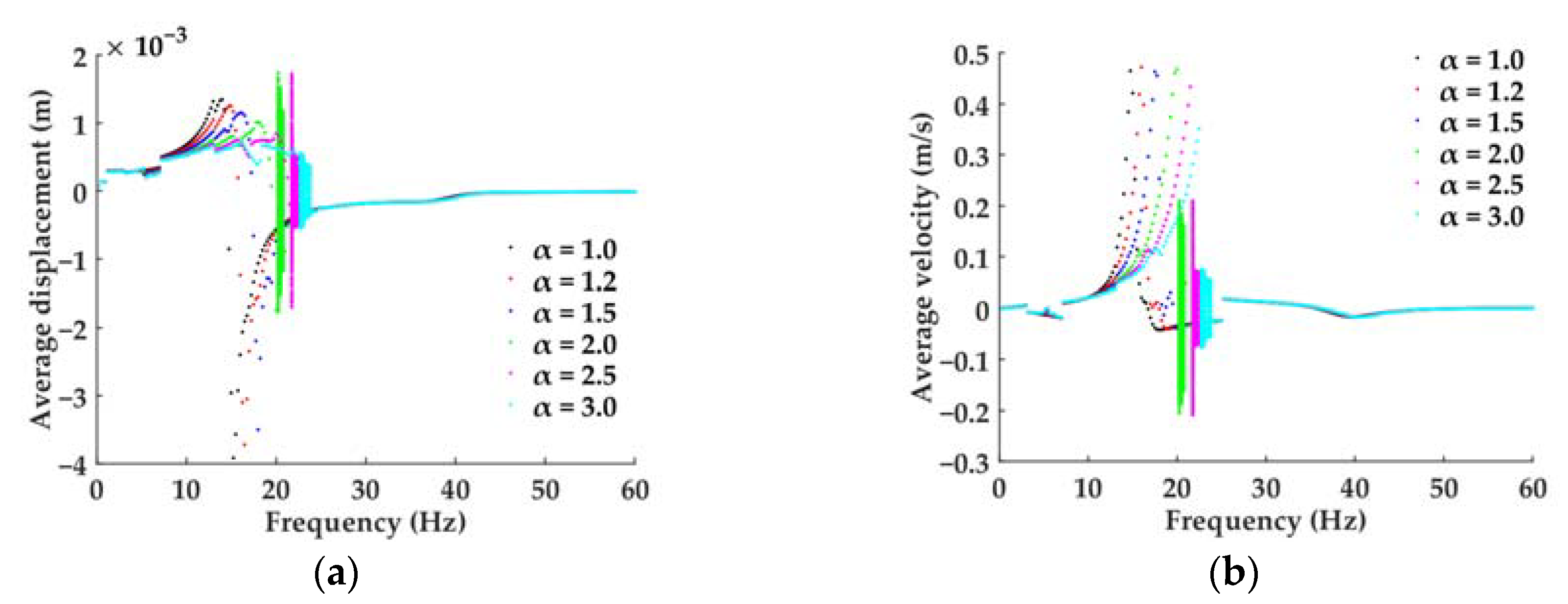
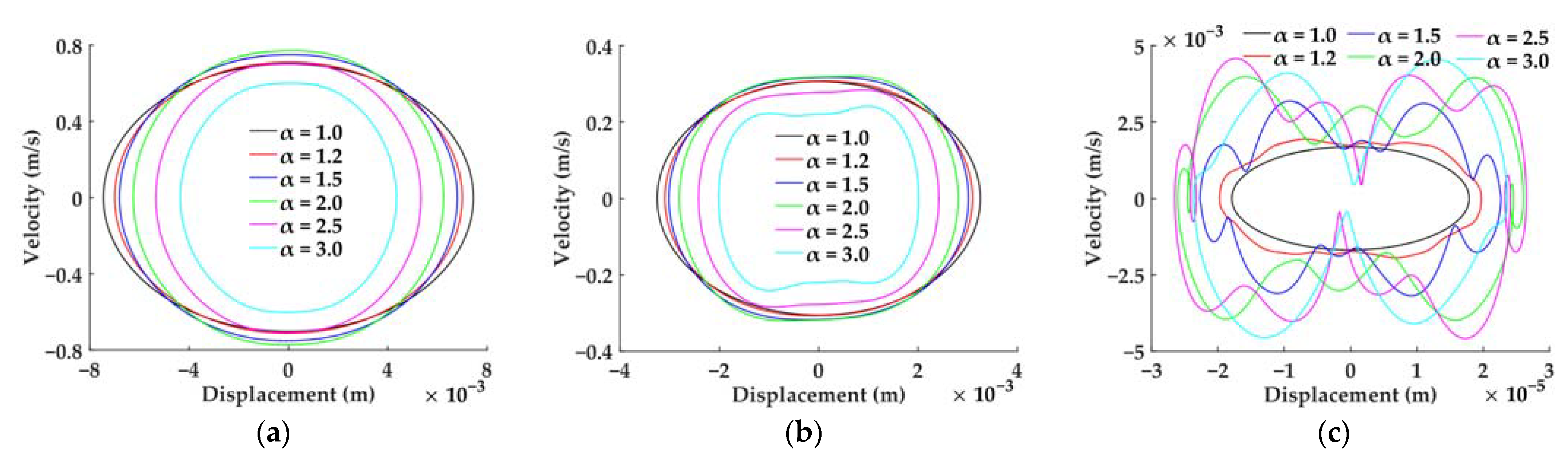
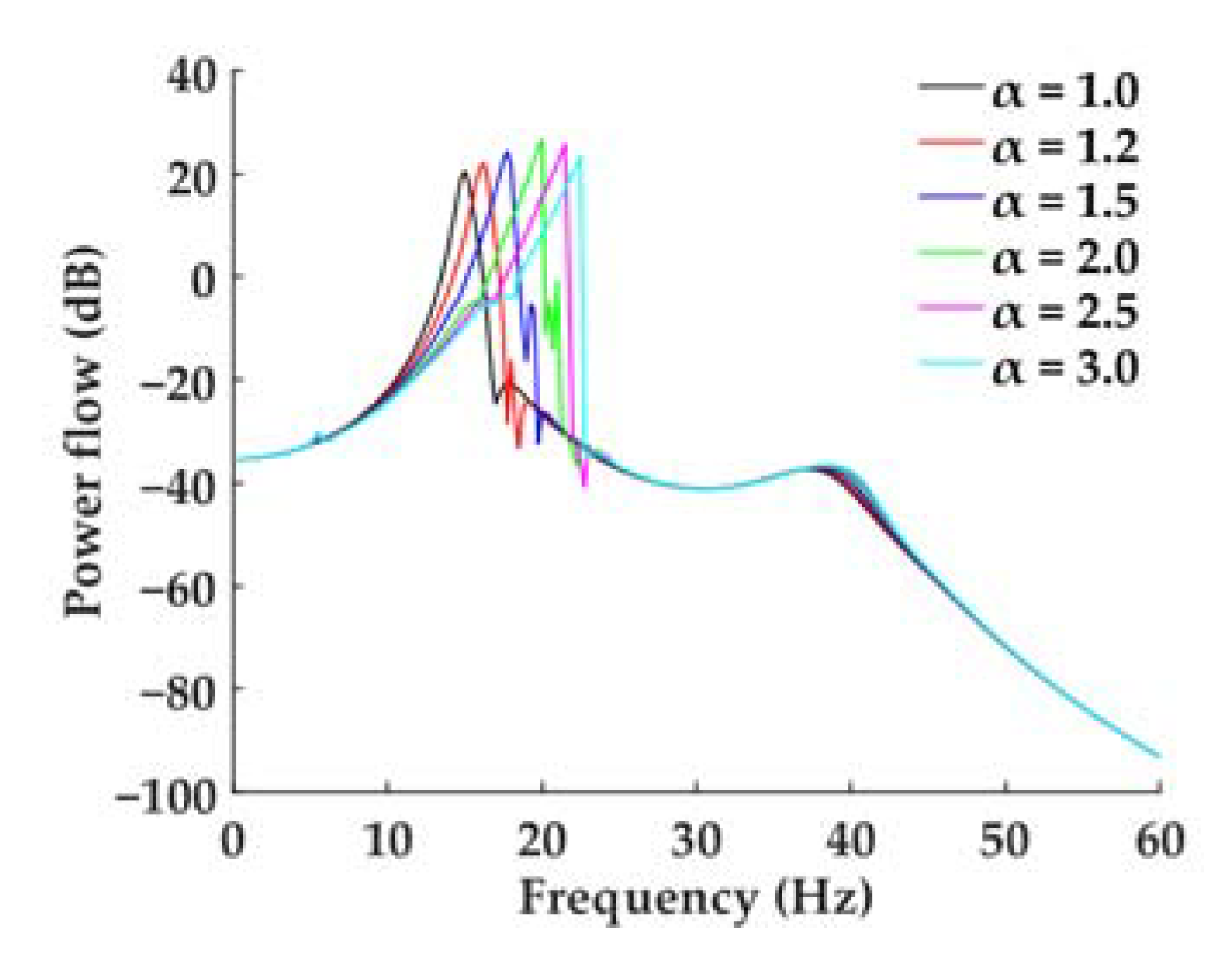
3.2.1. Effect of Stiffness Ratio α
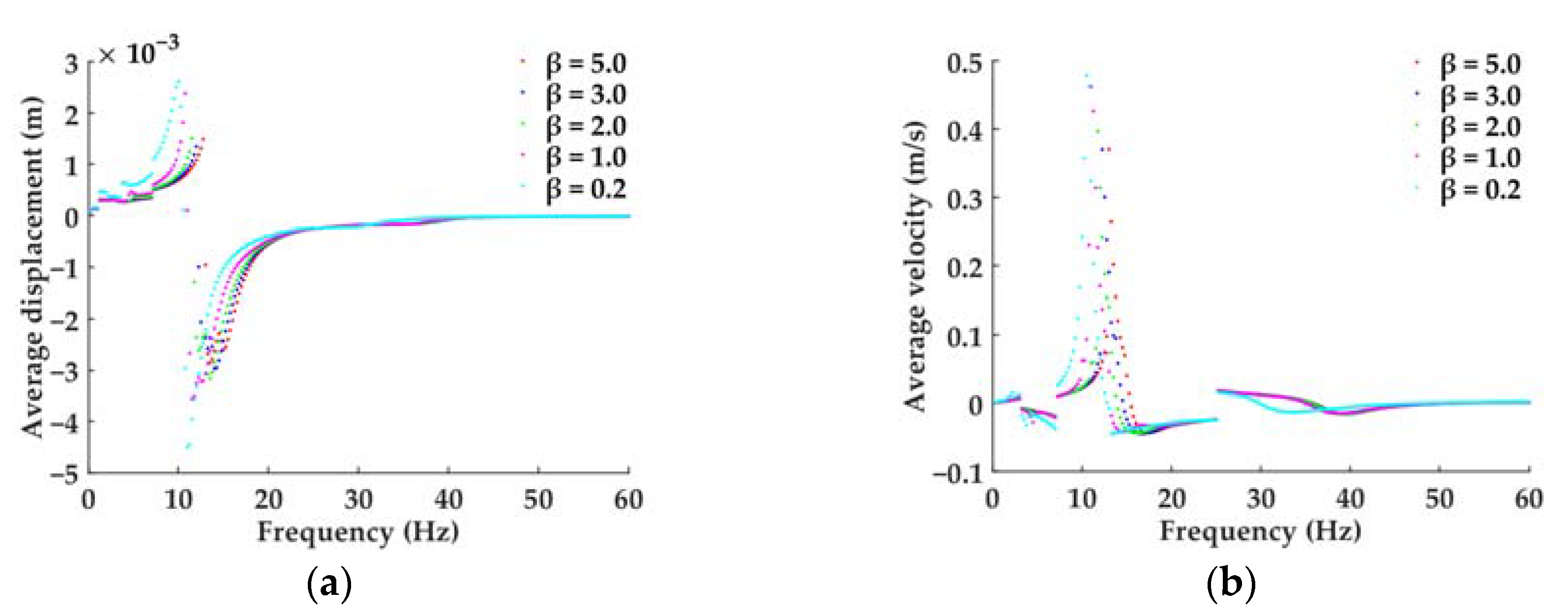
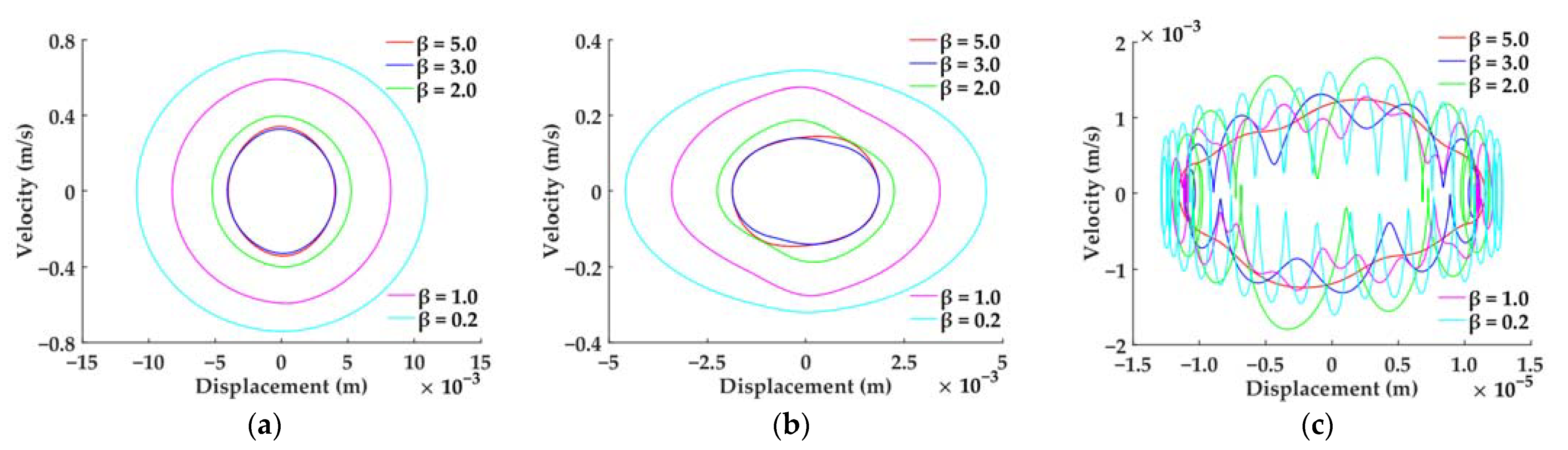
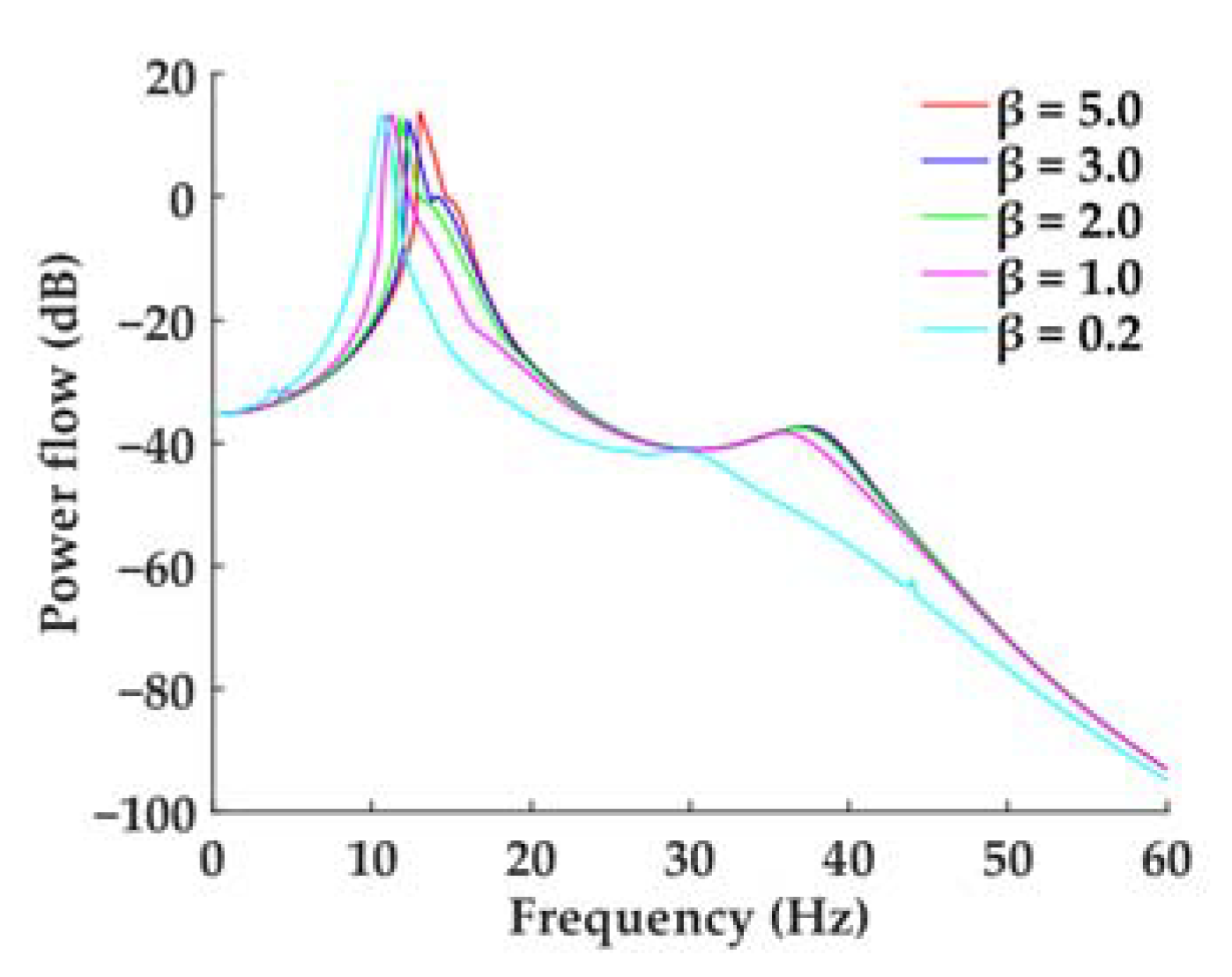
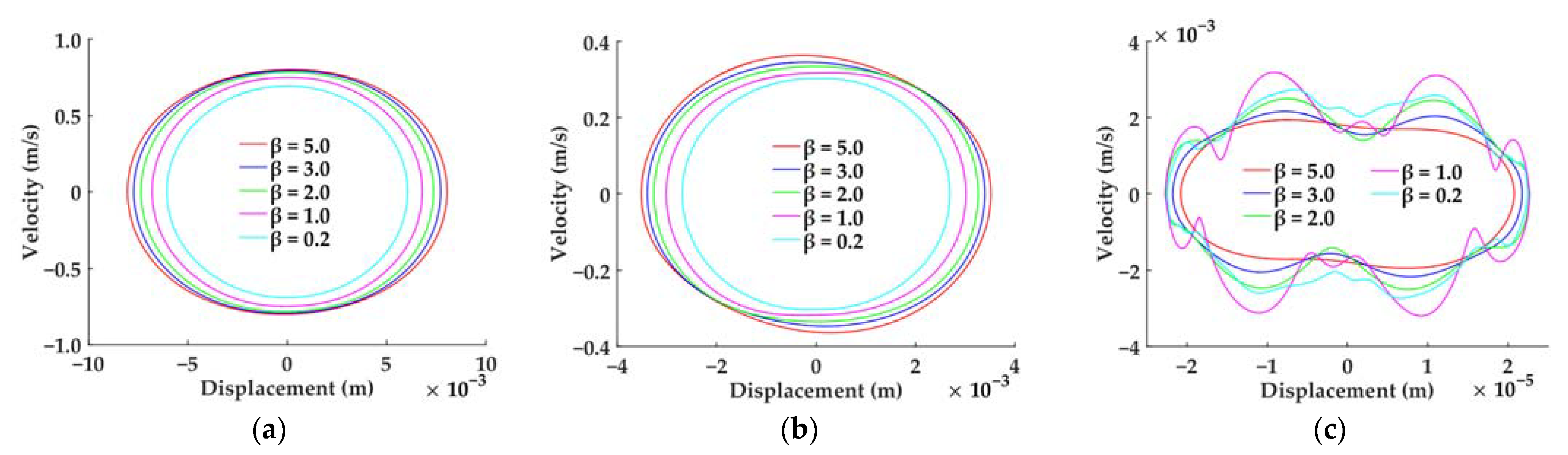
3.2.2. Effect of Approximate Linear Working Interval Length Control Parameter β
3.3. Effect of Nonlinear Stiffness with Hardening Characteristic on the Low-Frequency Vibro-Acoustic Characteristics for the Coupled System
3.3.1. Effect of Stiffness Ratio α
3.3.2. Effect of Approximate Linear Working Interval Length Control Parameter β
4. Conclusions
- For the coupled system with a nonlinear softening stiffness characteristic of the vibration isolation supports, the steady state vibration mode under harmonic excitation is single-periodic motion, but the superharmonic phenomenon is common. And the smaller the stiffness ratio is, the more superharmonic components are and the more complex the vibration form is. Compared with the linear stiffness case, the softening nonlinear stiffness can reduce the fundamental frequency and decrease the low-frequency vibro-acoustic transmission level within and after the resonance region for the vibration isolation system. And the stronger the softening characteristic is, the more significant the above effect is;
- For the coupled system with a nonlinear hardening stiffness characteristic of the vibration isolation supports, the vibration mode under harmonic excitation is single-periodic motion at most frequencies, and the existence of superharmonics is its main vibration feature. And the larger the stiffness ratio is, the more superharmonic components are and the more complex the vibration form is. In a frequency band slightly higher than the fundamental frequency, it may show a nonperiodic motion mode (quasi-periodic or chaotic). Compared with the linear stiffness case, the hardening nonlinear stiffness can increase the fundamental frequency, broaden the resonance band and slightly raise the low-frequency vibro-acoustic transmission level within and after the resonance region for the vibration isolation system. And the stronger the hardening characteristic is, the more significant the above effect is;
- For the determined nonlinear stiffness ratio, the variation of the length of the approximate linear working interval has no effect on the periodicity of the vibration of the coupled system. For the coupled system with a nonlinear softening stiffness characteristic of the vibration isolation supports, the smaller the length of the approximately linear working interval is, the lower the low-frequency vibro-acoustic transfer level after the fundamental frequency resonance region is; while for the coupled system with a nonlinear hardening stiffness characteristic of the vibration isolation supports, the smaller the length of the approximately linear working interval is, the higher the low-frequency vibro-acoustic transfer level after the fundamental frequency resonance region is.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A. State Space Model of the Cylindrical Shell—Underwater Acoustic Field Coupled Subsystem
Appendix A.1. Solutions of the Admittance Functions for the Coupled Subsystem
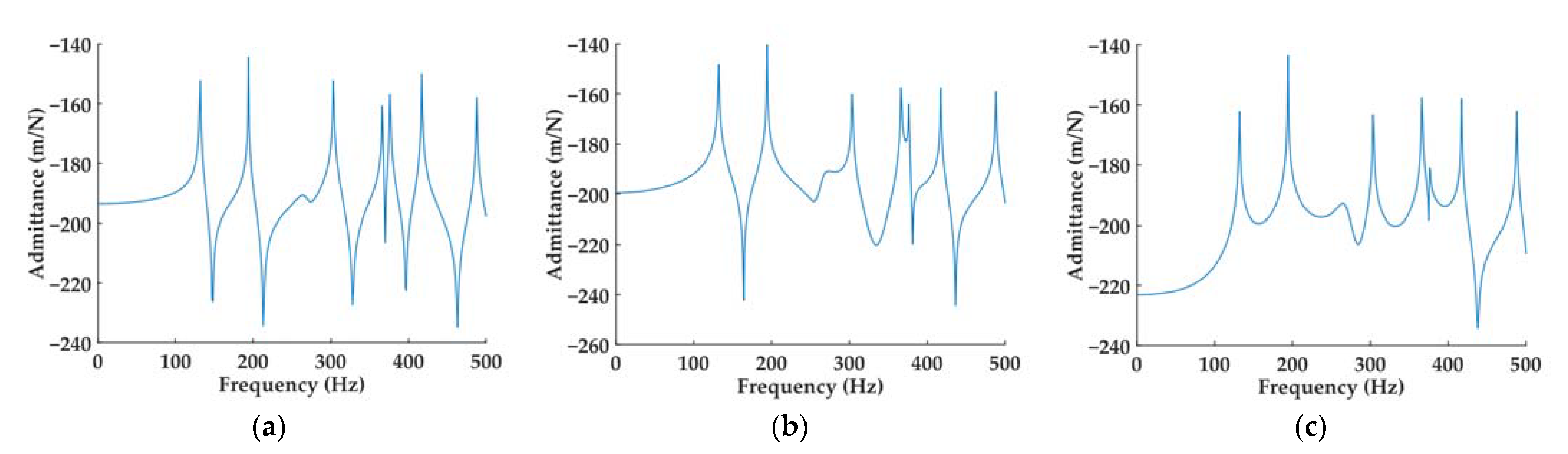
Appendix A.2. Modal Parameter Identification of the Coupled Subsystem
Appendix A.3. Establishment of State Space Equation for the Coupled Subsystem
Appendix B. Control Parameters in Simulation Calculation

References
- Smirnov, V.; Mondrus, V. Comparison of linear and nonlinear vibration isolation system under random excitation. Procedia Eng. 2016, 153, 673–678. [Google Scholar] [CrossRef]
- Santhosh, B. Dynamics and performance evaluation of an asymmetric nonlinear vibration isolation mechanism. J. Braz. Soc. Mech. Sci. Eng. 2018, 40, 169. [Google Scholar] [CrossRef]
- Dutta, S.; Chakraborty, G. Performance analysis of nonlinear vibration isolator with magneto-rheological damper. J. Sound Vib. 2014, 333, 5097–5114. [Google Scholar] [CrossRef]
- Suman, S.; Balaji, P.S.; Selvakumar, K.; Kumaraswamidhas, L.A. Nonlinear vibration control device for a vehicle suspension using negative stiffness mechanism. J. Vib. Eng. Technol. 2021, 9, 957–966. [Google Scholar] [CrossRef]
- Araki, Y.; Asai, T.; Kimura, K.; Maezawa, K.; Masui, T. Nonlinear vibration isolator with adjustable restoring force. J. Sound Vib. 2013, 332, 6063–6077. [Google Scholar] [CrossRef]
- Zhao, F.; Ji, J.C.; Ye, K.; Luo, Q. Increase of quasi-zero stiffness region using two pairs of oblique springs. Mech. Syst. Signal Process. 2020, 144, 106975. [Google Scholar] [CrossRef]
- Zhao, F.; Ji, J.C.; Ye, K.; Luo, Q. An innovative quasi-zero stiffness isolator with three pairs of oblique springs. Int. J. Mech. Sci. 2021, 192, 106093. [Google Scholar] [CrossRef]
- Ye, K.; Ji, J.C.; Brown, T. Design of a quasi-zero stiffness isolation system for supporting different loads. J. Sound Vib. 2020, 471, 115198. [Google Scholar] [CrossRef]
- Chang, Y.; Zhou, J.; Wang, K.; Xu, D. A quasi-zero-stiffness dynamic vibration absorber. J. Sound Vib. 2021, 494, 115859. [Google Scholar] [CrossRef]
- Bouna, H.S.; Nbendjo, B.R.N.; Woafo, P. Isolation performance of a quasi-zero stiffness isolator in vibration isolation of a multi-span continuous beam bridge under pier base vibrating excitation. Nonlinear Dyn. 2020, 100, 1125–1141. [Google Scholar] [CrossRef]
- Drezet, C.; Kacem, N.; Bouhaddi, N. Design of a nonlinear energy harvester based on high static low dynamic stiffness for low frequency random vibrations. Sens. Actuators A Phys. 2018, 283, 54–64. [Google Scholar] [CrossRef]
- Lu, Z.; Wu, D.; Ding, H.; Chen, L. Vibration isolation and energy harvesting integrated in a Stewart platform with high static and low dynamic stiffness. Appl. Math. Model. 2021, 89, 249–267. [Google Scholar] [CrossRef]
- Hao, R.; Lu, Z.; Ding, H.; Chen, L. Orthogonal six-DOFs vibration isolation with tunable high-static-low-dynamic stiffness: Experiment and analysis. Int. J. Mech. Sci. 2022, 222, 107237. [Google Scholar] [CrossRef]
- Hao, R.; Lu, Z.; Ding, H.; Chen, L. A nonlinear vibration isolator supported on a flexible plate: Analysis and experiment. Nonlinear Dyn. 2022, 108, 941–958. [Google Scholar] [CrossRef]
- Sun, Y.; Zhou, J.; Thompson, D.; Yuan, T.; Gong, D.; You, T. Design, analysis and experimental validation of high static and low dynamic stiffness mounts based on target force curves. Int. J. Non-Linear Mech. 2020, 126, 103559. [Google Scholar] [CrossRef]
- Yao, Y.; Li, H.; Li, Y.; Wang, X. Analytical and experimental investigation of a high-static-low-dynamic stiffness isolator with cam-roller-spring mechanism. Int. J. Mech. Sci. 2020, 186, 105888. [Google Scholar] [CrossRef]
- Chong, X.; Wu, Z.; Li, F. Vibration isolation properties of the nonlinear X-combined structure with a high-static and low-dynamic stiffness: Theory and experiment. Mech. Syst. Signal Process. 2022, 179, 109352. [Google Scholar] [CrossRef]
- Leutcho, G.D.; Kengne, J. A unique chaotic snap system with a smoothly adjustable symmetry and nonlinearity: Chaos, offset-boosting, antimonotonicity, and coexisting multiple attractors. Chaos Solitons Fractals 2018, 113, 275–293. [Google Scholar] [CrossRef]
- Yan, B.; Ma, H.; Jian, B.; Wang, K.; Wu, C. Nonlinear dynamics analysis of a bi-state nonlinear vibration isolator with symmetric permanent magnets. Nonlinear Dyn. 2019, 97, 2499–2519. [Google Scholar] [CrossRef]
- Rahman, Z.A.S.A.; Jasim, B.H. Hidden Dynamics Investigation, Fast Adaptive Synchronization, and Chaos-Based Secure Communication Scheme of a New 3D Fractional-Order Chaotic System. Inventions 2022, 7, 108. [Google Scholar] [CrossRef]
- Li, Y.; Yi, Y.; Wu, J.; Gu, Y. A novel feature extraction method for ship-radiated noise based on hierarchical refined composite multi-scale dispersion entropy-based Lempel-Ziv complexity. Deep Sea Res. Part I Oceanogr. Res. Pap. 2023, 199, 104111. [Google Scholar] [CrossRef]
- Karimov, A.; Rybin, V.; Dautov, A.; Karimov, T.; Bobrova, Y.; Butusov, D. Mechanical Chaotic Duffing System with Magnetic Springs. Inventions 2023, 8, 19. [Google Scholar] [CrossRef]
- Dreau, J.; Magnain, B.; Batailly, A. Multi-element polynomial chaos expansion based on automatic discontinuity detection for nonlinear systems. J. Sound Vib. 2023, 567, 117920. [Google Scholar] [CrossRef]
- Cassidy, I.L.; Scruggs, J.T. Nonlinear stochastic controllers for power-flow-constrained vibratory energy harvesters. J. Sound Vib. 2013, 332, 3134–3147. [Google Scholar] [CrossRef]
- Silva, O.M.; Neves, M.M.; Jordan, R.; Lenzi, A. An FEM-based method to evaluate and optimize vibration power flow through a beam-to-plate connection. J. Braz. Soc. Mech. Sci. Eng. 2017, 39, 413–426. [Google Scholar] [CrossRef]
- Varghese, C.K.; Shankar, K.K. Damage identification using combined transient power flow balance and acceleration matching technique. Struct. Control. Health Monit. 2014, 21, 135–155. [Google Scholar] [CrossRef]
- Yang, J.; Xiong, Y.; Xing, J. Vibration power flow and force transmission behaviour of a nonlinear isolator mounted on a nonlinear base. Int. J. Mech. Sci. 2016, 115, 238–252. [Google Scholar] [CrossRef]
- Shi, B.; Yang, J. Quantification of vibration force and power flow transmission between coupled nonlinear oscillators. Int. J. Dyn. Control. 2020, 8, 418–435. [Google Scholar] [CrossRef]
- Ren, Y.; Chang, S.; Liu, G.; Wu, L.; Wang, H. Vibratory power flow analysis of a gear-housing-foundation coupled system. Shock. Vib. 2018, 2018, 5974759. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhou, L.; Wang, S.; Yang, T.; Chen, L. Vibration power flow characteristics of the whole-spacecraft with a nonlinear energy sink. J. Low Freq. Noise Vib. Act. Control 2019, 38, 341–351. [Google Scholar] [CrossRef]
- Xu, D.; Du, J.; Zhao, Y. Flexural vibration and power flow analyses of axially loaded beams with general boundary and non-uniform elastic foundations. Adv. Mech. Eng. 2020, 12, 1687814020921719. [Google Scholar] [CrossRef]
- Mahapatra, K.; Panigrahi, S.K. Effect of general coupling conditions on the vibration and power flow characteristics of a two-plate built-up plate structure. Mech. Based Des. Struct. Mach. 2021, 49, 841–861. [Google Scholar] [CrossRef]
- Liu, S.; Huo, R.; Wang, L. Vibroacoustic Transfer Characteristics of Underwater Cylindrical Shells Containing Complex Internal Elastic Coupled Systems. Appl. Sci. 2023, 13, 3994. [Google Scholar] [CrossRef]
- More, S.; Padmanabhan, C. Power flow based analysis of a floating raft vibration isolation system. Am. Phys. Soc. 2014, 72, 691–708. [Google Scholar]

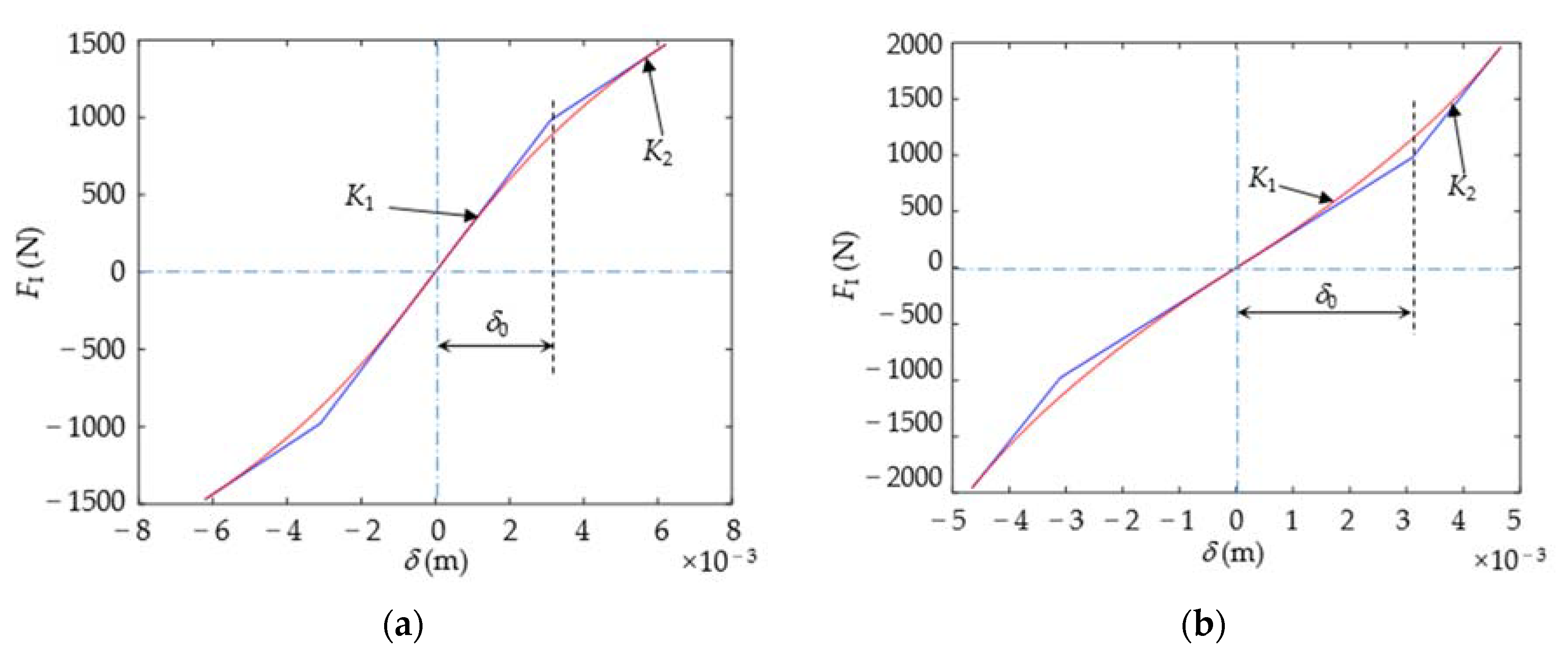



| Control Parameters | Symbols | Units | Values | Remarks |
|---|---|---|---|---|
| Stiffness control parameters in the approximate linear working interval | KA11 | N/m | 2.0 × 105 | |
| KA21 | N/m | 2.7 × 105 | ||
| KR1 | N/m | 8.6 × 105 | ||
| Stiffness control parameters outside the linear working interval | KA12 | N/m | α⋅2.0 × 105 | α ∈ [0.1, 3.0] α < 1, softening characteristic α > 1, hardening characteristic |
| KA22 | N/m | α⋅2.7 × 105 | ||
| KR2 | N/m | α⋅8.6 × 105 | ||
| Linear working interval length control parameters | δ10 | m | β⋅6.125 × 10−4 | β ∈ [0.2, 5.0] |
| δ20 | m | β⋅6.352 × 10−4 | ||
| δR0 | m | β⋅6.267 × 10−4 | ||
| Damping coefficients | c1 | kg/s | 159.155 | |
| c2 | kg/s | 214.859 | ||
| cR | kg/s | 684.366 |
| System Structure Parameters | Symbols | Units | Values | |
|---|---|---|---|---|
| Characteristic parameters of the cylindrical shell | Elastic modulus | E | N/m2 | 2.1 × 1011 |
| Density | ρ | kg/m3 | 7.8 × 103 | |
| Poisson’s ratio | μ | 0.28 | ||
| Dissipation factor | ξ | 0.01 | ||
| Length, radius, thickness | L, a, d | m | 2, 0.4, 0.02 | |
| Structure parameters of the units and the raft frame | Mass of the unit A1 | m1 | kg | 50 |
| Mass of the unit A2 | m2 | kg | 70 | |
| Mass of the raft frame R | mR | kg | 100 | |
| Moment of inertia of the unit A1 | J1x, J1z | Kg·m2 | 1, 0.5 | |
| Moment of inertia of the unit A2 | J2x, J2z | Kg·m2 | 1.5, 1 | |
| Moment of inertia of the raft frame R | JRx, JRz | Kg·m2 | 8.5, 2.5 | |
| Linear complex stiffness of the vibration isolation supports | Support stiffness of the unit A1 | k1 | N/m | 2.0 × 105 × (1 + 0.1 j) |
| Support stiffness of the unit A2 | k2 | N/m | 2.7 × 105 × (1 + 0.1 j) | |
| Support stiffness of the raft frame R | kR | N/m | 8.6 × 105 × (1 + 0.1 j) | |
| Installation position parameters | Centroid positioning of the unit A1 | b1 | m | 0.25 |
| Centroid positioning of the unit A2 | b2 | m | 0.25 | |
| Centroid positioning of the raft frame R | LR | m | 0.8 | |
| Support spacing of the unit A1 | h1x, h1z | m | 0.2, 0.3 | |
| Support spacing of the unit A2 | h2x, h2z | m | 0.2, 0.3 | |
| Support spacing of the raft frame R | Hx, Hz | m | 0.35, 0.7 | |
| Orders i | Poles sCi, sCi* * | Modal Frequencies fCi (Hz) |
|---|---|---|
| 1 | −0.366 ± 8.277 × 102 j | 131.73 |
| 2 | −0.574 ± 1.219 × 103 j | 194.95 |
| 3 | −0.660 ± 1.672 × 103 j | 266.24 |
| 4 | −0.924 ± 1.906 × 103 j | 303.33 |
| 5 | −1.246 ± 2.302 × 103 j | 366.30 |
| 6 | −1.753 ± 2.364 × 103 j | 376.21 |
| 7 | −1.307 ± 2.621 × 103 j | 417.11 |
| 8 | −1.586 ± 3.065 × 103 j | 487.86 |
| 9 | −3.624 ± 3.304 × 103 j | 525.88 |
| 10 | −2.863 × 103 ± 1.973 × 103 j | 553.34 |
| Orders i | j = 1 | j = 2 | j = 3 | j = 4 |
|---|---|---|---|---|
| i = 1 | 0.475 − 4.630 j | 0.686 − 0.703 j | 0.104 − 0.117 j | 0.163 − 0.172 j |
| i = 2 | 0.462 − 0.429 j | 0.601 − 0.647 j | −0.317 + 0.312 j | −0.448 + 0.469 j |
| i = 3 | 0.436 − 0.208 j | 0.271 − 0.328 j | 0.068 − 0.226 j | 0.214 − 0.335 j |
| i = 4 | 0.510 − 0.529 j | −0.274 + 0.240 j | −0.398 + 0.384 j | 0.170 − 0.169 j |
| i = 5 | 0.367 − 0.352 j | 0.402 − 0.441 j | −0.347 + 0.339 j | −0.453 + 0.479 j |
| i = 6 | 0.159 − 0.407 j | −0.342 + 0.117 j | 0.412 − 0.031 j | 0.006 + 0.032 j |
| i = 7 | 0.473 − 0.475 j | −0.218 + 0.218 j | −0.464 + 0.464 j | 0.215 − 0.215 j |
| i = 8 | 0.357 − 0.356 j | −0.317 + 0.318 j | −0.261 + 0.261 j | 0.233 − 0.234 j |
| i = 9 | 0.470 − 0.434 j | −0.254 + 0.271 j | −0.361 + 0.357 j | 0.248 − 0.270 j |
| i = 10 | 0.716 − 0.762 j | 0.082 − 0.105 j | −0.244 + 0.233 j | −0.053 + 0.012 j |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, L.; Huo, R. Effect of Support Stiffness Nonlinearity on the Low-Frequency Vibro-Acoustic Characteristics for a Mechanical Equipment—Floating Raft—Underwater Cylindrical Shell Coupled System. Inventions 2023, 8, 118. https://doi.org/10.3390/inventions8050118
Wang L, Huo R. Effect of Support Stiffness Nonlinearity on the Low-Frequency Vibro-Acoustic Characteristics for a Mechanical Equipment—Floating Raft—Underwater Cylindrical Shell Coupled System. Inventions. 2023; 8(5):118. https://doi.org/10.3390/inventions8050118
Chicago/Turabian StyleWang, Likang, and Rui Huo. 2023. "Effect of Support Stiffness Nonlinearity on the Low-Frequency Vibro-Acoustic Characteristics for a Mechanical Equipment—Floating Raft—Underwater Cylindrical Shell Coupled System" Inventions 8, no. 5: 118. https://doi.org/10.3390/inventions8050118





