13C Isotope Labelling to Follow the Flux of Photorespiratory Intermediates
Abstract
:1. Introduction
2. Results
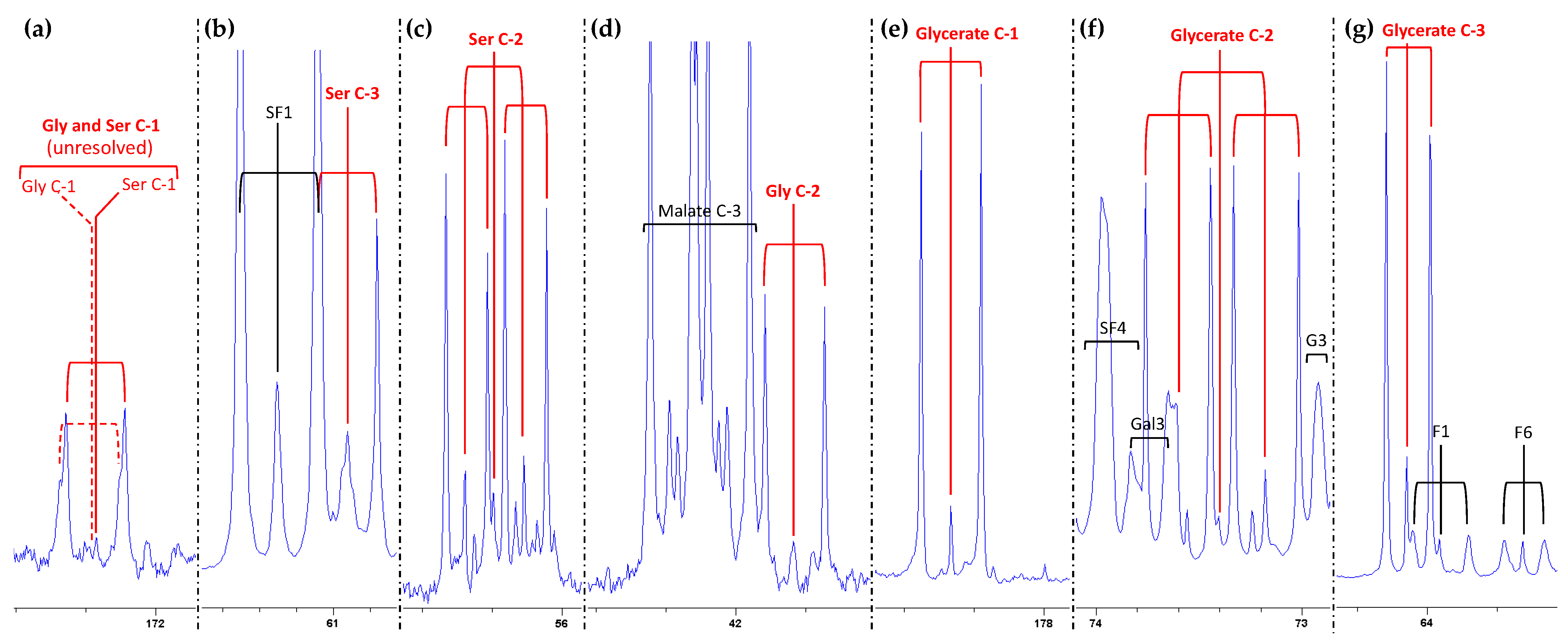
2.1. Isotopic Signals in Photorespiratory Intermediates
2.2. Isotopologue Distribution
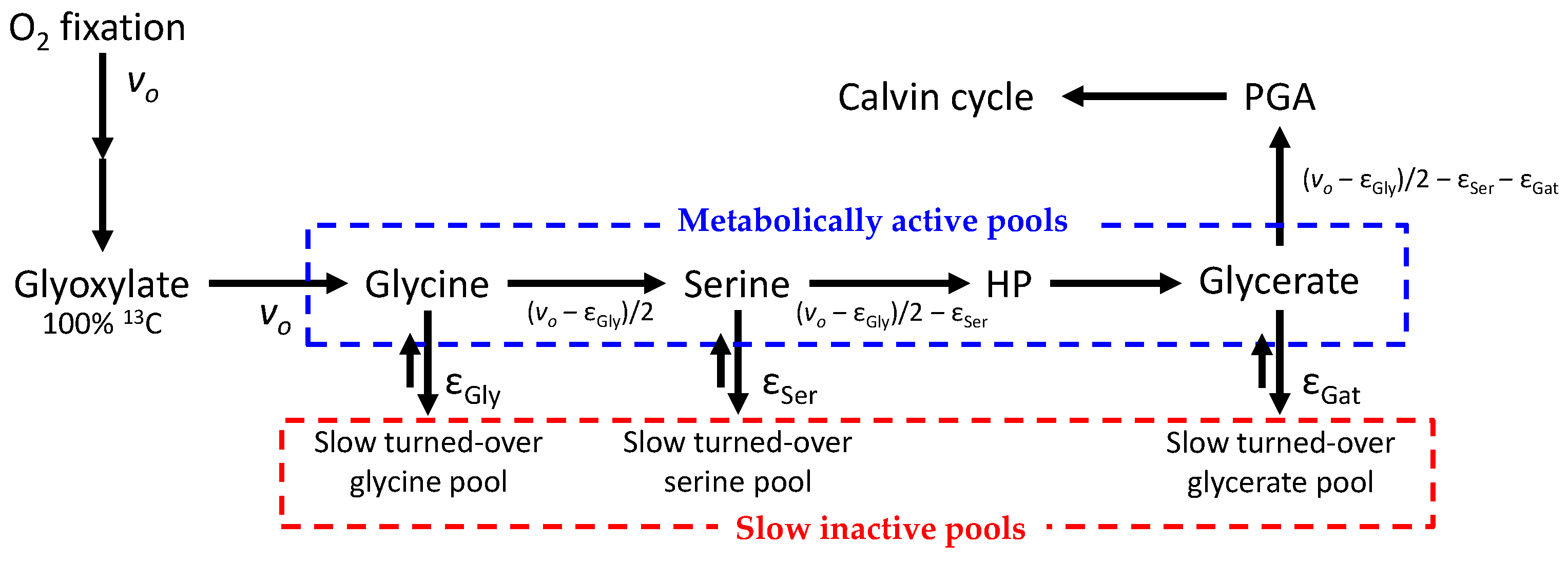
2.3. Metabolic Fluxes
3. Discussion
3.1. Pros and Cons of the 13C-Based Isotopic Method
3.2. Possible Consequences of Accumulation of Photorespiratory Intermediates
4. Materials and Methods
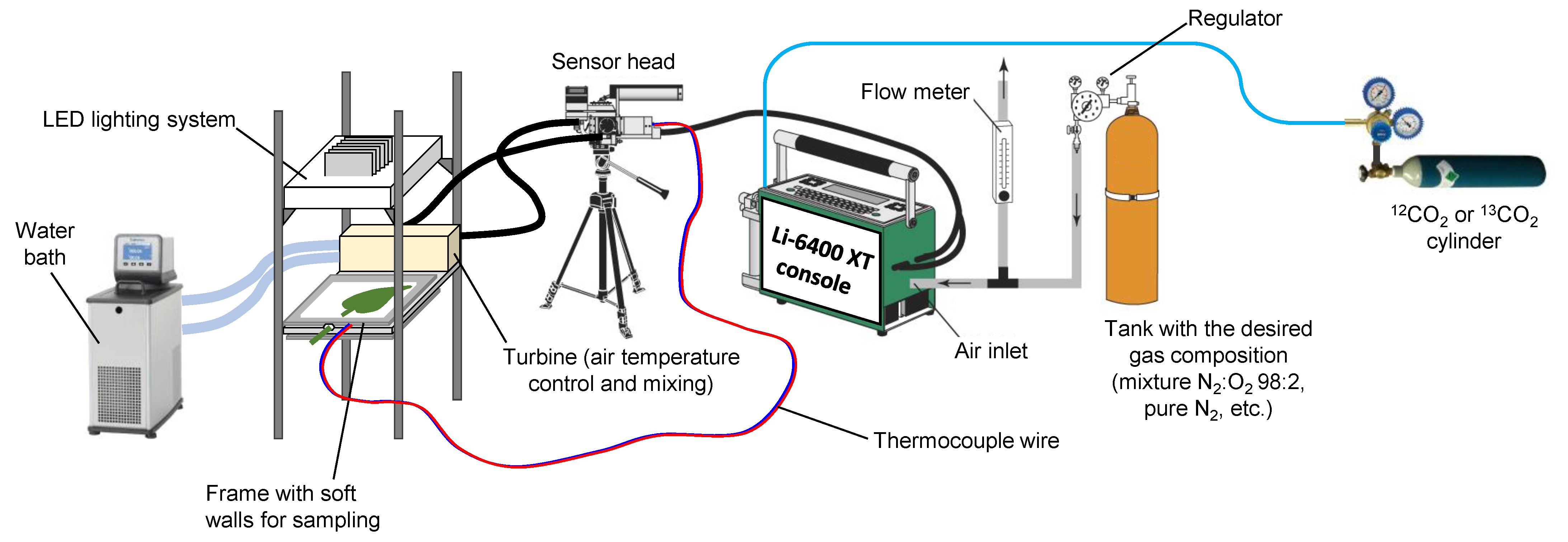
4.1. Gas Exchange System
4.2. NMR Analysis
4.3. LC–MS Analyses
4.4. Calculations
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Busch, F.A. Current methods for estimating the rate of photorespiration in leaves. Plant Biol. 2013, 15, 648–655. [Google Scholar] [CrossRef]
- Von Caemmerer, S. Steady-state models of photosynthesis. Plant Cell Environ. 2013, 36, 1617–1630. [Google Scholar] [CrossRef] [PubMed]
- Douce, R.; Bourguignon, J.; Neuburger, M.; Rébeillé, F. The glycine decarboxylase system: A fascinating complex. Trends Plant Sci. 2001, 6, 167–176. [Google Scholar] [CrossRef]
- Tcherkez, G. Is the recovery of (photo)respiratory CO2 and intermediates minimal? New Phytol. 2013, 198, 334–338. [Google Scholar] [CrossRef] [PubMed]
- Dirks, R.C.; Singh, M.; Potter, G.S.; Sobotka, L.G.; Schaefer, J. Carbon partitioning in soybean (Glycine max) leaves by combined 11C and 13C labeling. New Phytol. 2012, 196, 1109–1121. [Google Scholar] [CrossRef] [PubMed]
- Busch, F.A.; Sage, R.F.; Farquhar, G.D. Plants increase CO2 uptake by assimilating nitrogen via the photorespiratory pathway. Nat. Plants 2018, 4, 46–54. [Google Scholar] [CrossRef]
- Mahon, J.D.; Fock, H.; Canvin, D.T. Changes in specific radioactivity of sunflower leaf metabolites during photosynthesis in 14CO2 and 12CO2 at three concentrations of CO2. Planta 1974, 120, 245–254. [Google Scholar] [CrossRef]
- Abadie, C.; Boex-Fontvieille, E.R.; Carroll, A.J.; Tcherkez, G. In vivo stoichiometry of photorespiratory metabolism. Nat. Plants 2016, 2, 1–4. [Google Scholar] [CrossRef]
- Gauthier, P.P.; Battle, M.O.; Griffin, K.L.; Bender, M.L. Measurement of gross photosynthesis, respiration in the light, and mesophyll conductance using H218O labeling. Plant Physiol. 2018, 177, 62–74. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Canvin, D.T.; Berry, J.A.; Badger, M.R.; Fock, H.; Osmond, C.B. Oxygen exchange in leaves in the light. Plant Physiol. 1980, 66, 302–307. [Google Scholar] [CrossRef]
- Pick, T.R.; Bräutigam, A.; Schulz, M.A.; Obata, T.; Fernie, A.R.; Weber, A.P.M. PLGG1, a plastidic glycolate glycerate transporter, is required for photorespiration and defines a unique class of metabolite transporters. Proc. Natl. Acad. Sci. USA 2013, 110, 3185–3189. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Berry, J.A.; Osmond, C.B.; Lorimer, G.H. Fixation of 18O2 during photorespiration: Kinetic and steady-state studies of the photorespiratory carbon oxidation cycle with intact leaves and isolated chloroplasts of C3 Plants. Plant Physiol. 1978, 62, 954–964. [Google Scholar] [CrossRef] [Green Version]
- Szecowka, M.; Heise, R.; Tohge, T.; Nunes-Nesi, A.; Vosloh, D.; Huege, J.; Feil, R.; Lunn, J.; Nikoloski, Z.; Stitt, M. Metabolic fluxes in an illuminated Arabidopsis rosette. Plant Cell 2013, 25, 694–714. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Cegelski, L.; Schaefer, J. NMR determination of photorespiration in intact leaves using in vivo 13CO2 labeling. J. Magn. Reson. 2006, 178, 1–10. [Google Scholar] [CrossRef] [PubMed]
- De Souza, L.P.; Szecówka, M.; Fernie, A.R.; Tohge, T. 13CO2 labeling and mass spectral analysis of photorespiration. In Photorespiration: Methods and Protocols; Fernie, A.R., Bauwe, H., Weber, A.P.M., Eds.; Springer: New York, NY, USA, 2017; pp. 157–166. [Google Scholar] [CrossRef]
- Abadie, C.; Bathellier, C.; Tcherkez, G. Carbon allocation to major metabolites in illuminated leaves is not just proportional to photosynthesis when gaseous conditions (CO2 and O2) vary. New Phytol. 2018, 218, 94–106. [Google Scholar] [CrossRef] [Green Version]
- Abadie, C.; Lalande, J.; Limami, A.M.; Tcherkez, G. Non-targeted 13C metabolite analysis demonstrates broad re-orchestration of leaf metabolism when gas exchange conditions vary. Plant Cell Environ. 2021, in press. [Google Scholar] [CrossRef]
- Abadie, C.; Lothier, J.; Boex-Fontvieille, E.; Carroll, A.; Tcherkez, G. Direct assessment of the metabolic origin of carbon atoms in glutamate from illuminated leaves using 13C-NMR. New Phytol. 2017, 216, 1079–1089. [Google Scholar] [CrossRef] [Green Version]
- Abadie, C.; Tcherkez, G. In vivo phosphoenolpyruvate carboxylase activity is controlled by CO2 and O2 mole fractions and represents a major flux at high photorespiration rates. New Phytol. 2019, 221, 1843–1852. [Google Scholar] [CrossRef]
- Abadie, C.; Tcherkez, G. Plant sulphur metabolism is stimulated by photorespiration. Nat. Commun. Biol. 2019, 2, 1–7. [Google Scholar] [CrossRef]
- Jacob, J.; Lawlor, D.W. Dependence of photosynthesis of sunflower and maize leaves on phosphate supply, ribulose 1,5-bisphosphate carboxylase/oxygenase activity, and ribulose 1,5-bisphosphate pool size. Plant Physiol. 1992, 98, 801–807. [Google Scholar] [CrossRef] [Green Version]
- Abadie, C.; Blanchet, S.; Carroll, A.; Tcherkez, G. Metabolomics analysis of post-photosynthetic effects of gaseous O2 on primary metabolism in illuminated leaves. Funct. Plant Biol. 2017, 44, 929–940. [Google Scholar] [CrossRef]
- Tcherkez, G.; Carroll, A.; Abadie, C.; Mainguet, S.; Davanture, M.; Zivy, M. Protein synthesis increases with photosynthesis via the stimulation of translation initiation. Plant Sci. 2020, 291, 110352. [Google Scholar] [CrossRef] [PubMed]
- Ishihara, H.; Obata, T.; Sulpice, R.; Fernie, A.R.; Stitt, M. Quantifying protein synthesis and degradation in Arabidopsis by dynamic 13C labeling and analysis of enrichment in individual amino acids in their free pools and in protein. Plant Physiol. 2015, 168, 74–84. [Google Scholar] [CrossRef] [Green Version]
- Tcherkez, G.; Mahe, A.; Guerard, F.; Boex-Fontvieille, E.R.A.; Gout, E.; Lamothe, M.; Barbour, M.M.; Bligny, R. Short-term effects of CO2 and O2 on citrate metabolism in illuminated leaves. Plant Cell Environ. 2012, 35, 2208–2220. [Google Scholar] [CrossRef] [PubMed]
- Tcherkez, G.; Limami, A.M. Net photosynthetic CO2 assimilation: More than just CO2 and O2 reduction cycles. New Phytol. 2019, 223, 520–529. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Peoples, T.R.; Koch, D.W. Role of potassium in carbon dioxide assimilation in Medicago sativa L. Plant Physiol. 1979, 63, 878–888. [Google Scholar] [CrossRef] [Green Version]
- Bednarz, C.; Oosterhuis, D.; Evans, R. Leaf photosynthesis and carbon isotope discrimination of cotton in response to potassium deficiency. Environ. Exp. Bot. 1998, 39, 131–139. [Google Scholar] [CrossRef]
- Singh, S.K.; Reddy, V.R. Co-regulation of photosynthetic processes under potassium deficiency across CO2 levels in soybean: Mechanisms of limitations and adaptations. Photosynth. Res. 2018, 137, 183–200. [Google Scholar] [CrossRef]
- Tezara, W.; Mitchell, V.; Driscoll, S.; Lawlor, D. Effects of water deficit and its interaction with CO2 supply on the biochemistry and physiology of photosynthesis in sunflower. J. Exp. Bot. 2002, 53, 1781–1791. [Google Scholar] [CrossRef] [Green Version]
- Tezara, W.; Driscoll, S.; Lawlor, D.W. Partitioning of photosynthetic electron flow between CO2 assimilation and O2 reduction in sunflower plants under water deficit. Photosynthetica 2008, 46, 127–137. [Google Scholar] [CrossRef]
- Li, P.; Ainsworth, E.A.; Leakey, A.D.B.; Ulanov, A.; Lozovaya, V.; Ort, D.R.; Bohnert, H.J. Arabidopsis transcript and metabolite profiles: Ecotype-specific responses to open-air elevated [CO2]. Plant Cell Environ. 2008, 31, 1673–1687. [Google Scholar] [CrossRef] [PubMed]


| Condition | O2/CO2 | Photorespiration |
|---|---|---|
| 1 | 100/380 | Very high |
| 2 | 21/140 | High |
| 3 | 21/380 | Normal |
| 4 | 21/800 | Low |
| 5 | 2/380 | Very low |
| 6 | 0/380 | Negligible |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abadie, C.; Tcherkez, G. 13C Isotope Labelling to Follow the Flux of Photorespiratory Intermediates. Plants 2021, 10, 427. https://doi.org/10.3390/plants10030427
Abadie C, Tcherkez G. 13C Isotope Labelling to Follow the Flux of Photorespiratory Intermediates. Plants. 2021; 10(3):427. https://doi.org/10.3390/plants10030427
Chicago/Turabian StyleAbadie, Cyril, and Guillaume Tcherkez. 2021. "13C Isotope Labelling to Follow the Flux of Photorespiratory Intermediates" Plants 10, no. 3: 427. https://doi.org/10.3390/plants10030427
APA StyleAbadie, C., & Tcherkez, G. (2021). 13C Isotope Labelling to Follow the Flux of Photorespiratory Intermediates. Plants, 10(3), 427. https://doi.org/10.3390/plants10030427






