Large Deviations for Continuous Time Random Walks
Abstract
:1. Introduction
2. Appetizer for Exponential Tails
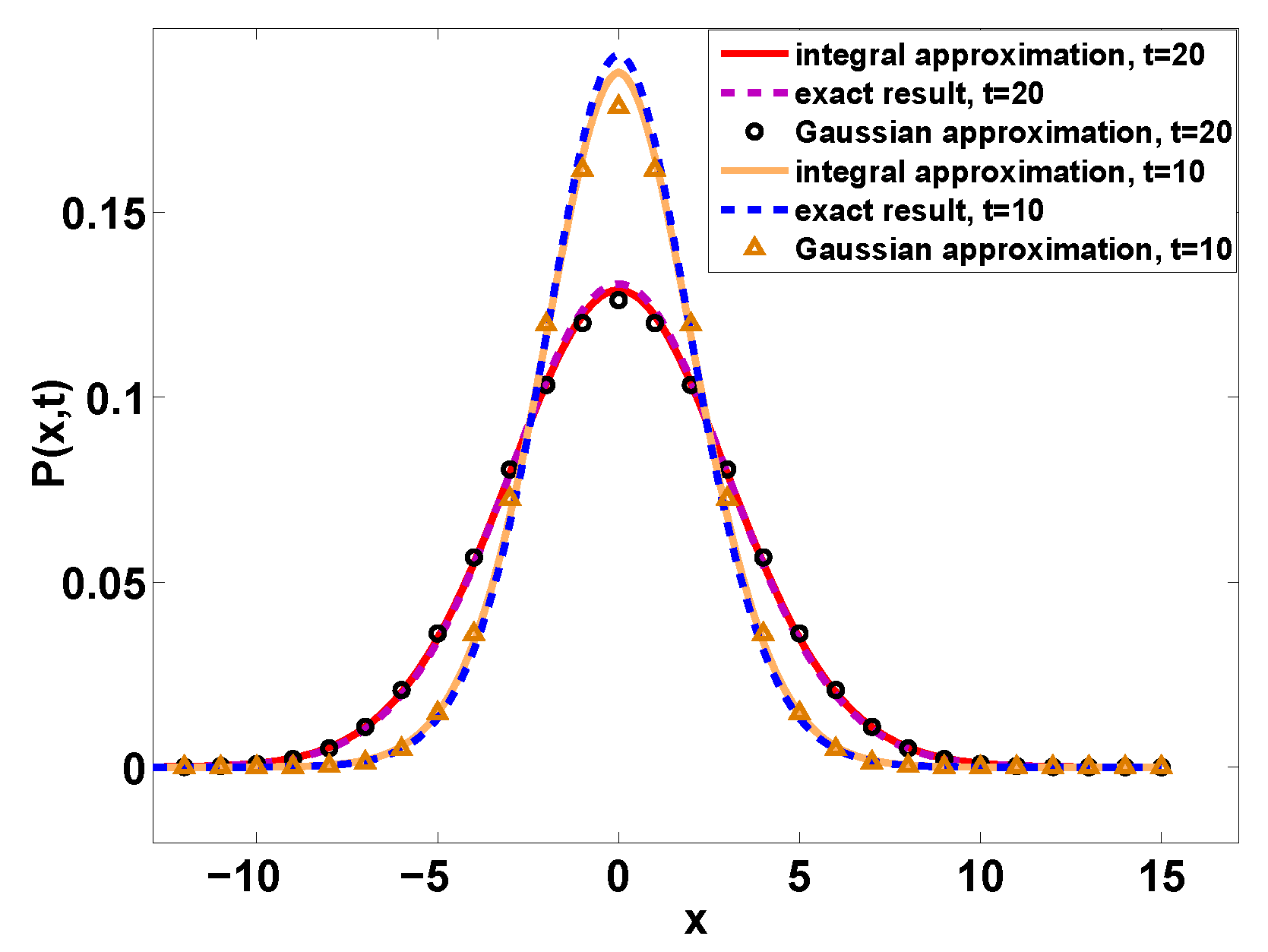
2.1. Displacement Follows Gaussian Distribution
2.2. Displacement Drawn from the Discrete PDF
3. The Exponential Tails for the Erlang Waiting Time PDF
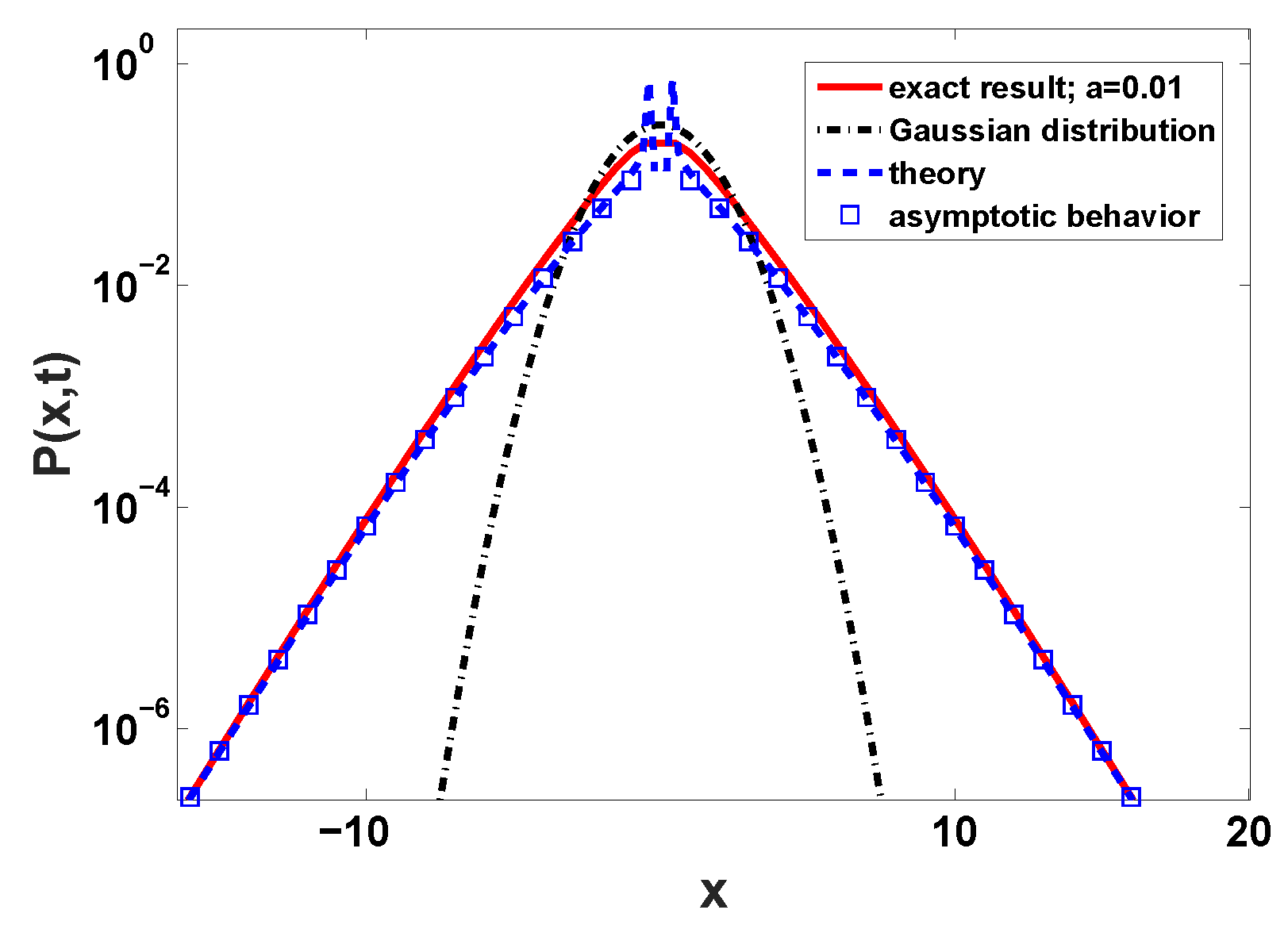
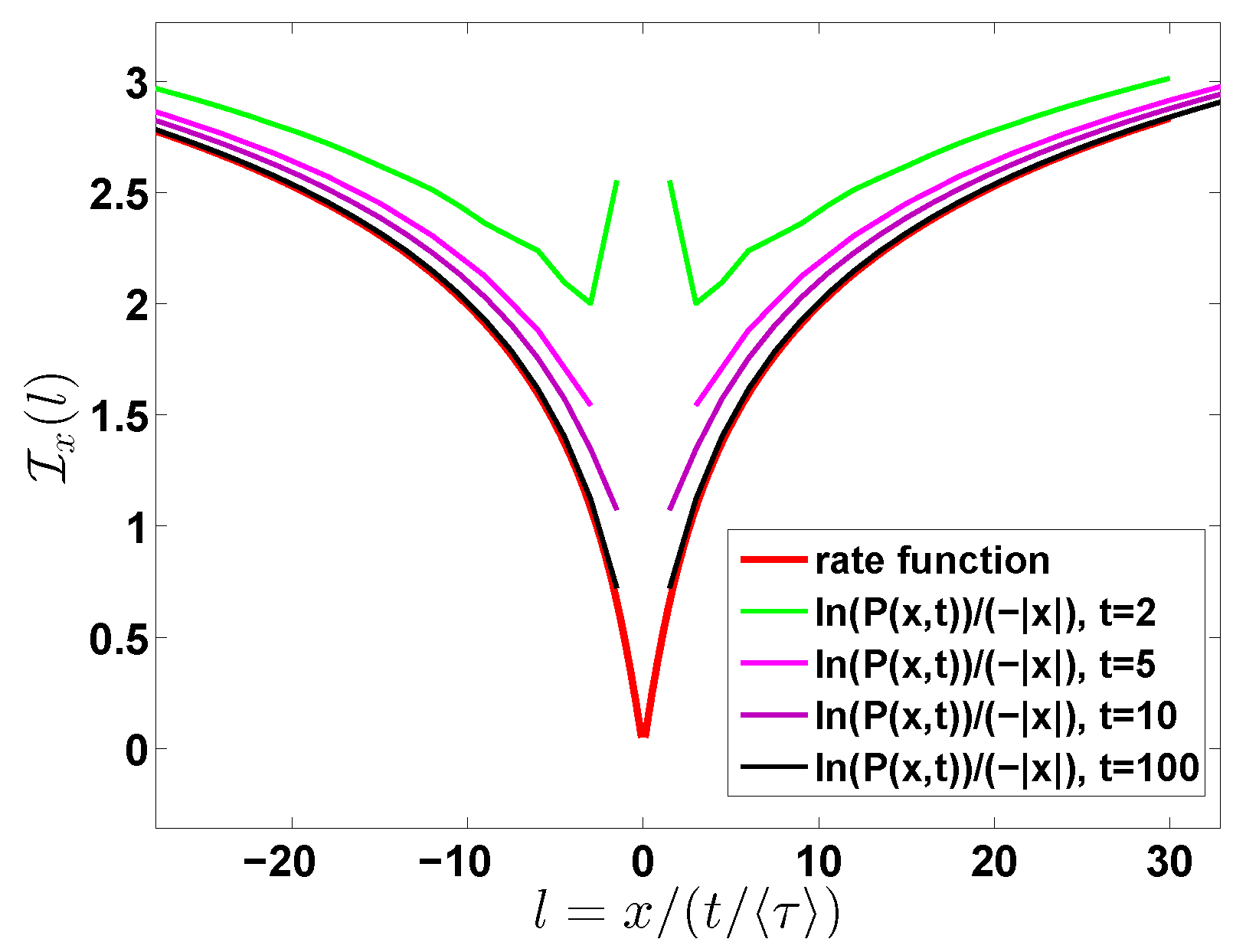
3.1. The Exponential Tail of the Number of Events
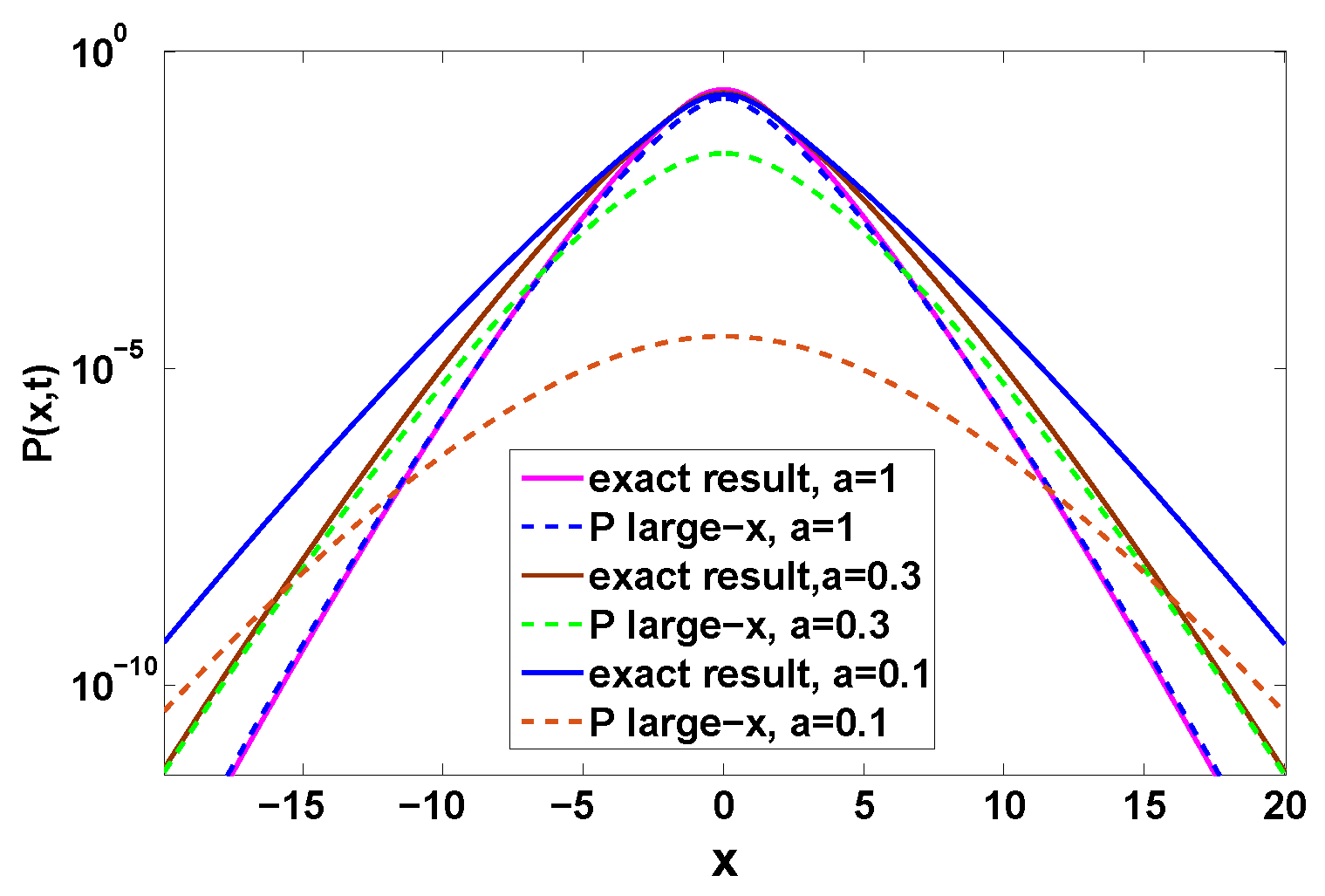
3.2. The Far Tails of the Positional PDF
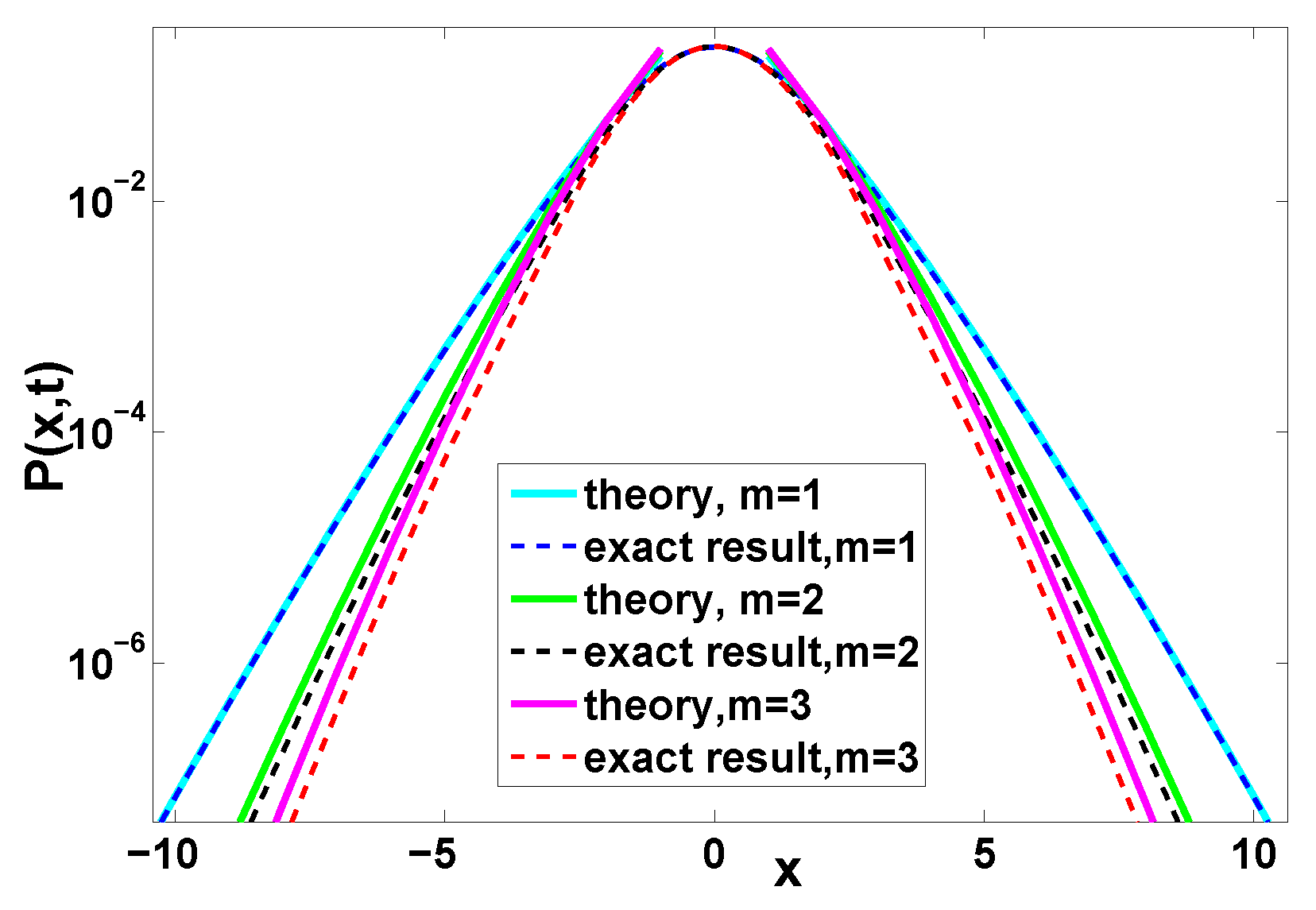
4. Bunching Case of Waiting Time PDF
5. Conclusions
- In the present case we assumed that the jump process starts at time . This is called an ordinary renewal process. If the processes started long before the measurement, we will have a modification of the PDF of the first waiting time [3,58]. How does this effect the large x behavior of ? This issue seems important since the phenomenon can be found for relatively short times.
- What happens in dimension ?
- What are ideal waiting time PDFs and jump length distributions, where exponential tails are pronounce and if possible maintained for longer times. We showed how this is related to bunching and anti-bunching, however more refined work can help to clarify a better the widely observed behavior.
- We used CTRW, instead one could use the noisy CTRW model [61]. This adds to the jumps also noise when the particle is waiting for its next jump. Thus noisy CTRW is much more similar to real experiments.
- Recently Dechant et al. showed how the CTRW picture emerges from an under-damped Langevin description of a particle in a periodic potential [29]. And then showed how this model can be used to analyse dynamics of Cesium atoms in optical lattices. Thus we expect to find also here exponential tails of packets, however influence of the control parameters of this phenomenon such as the depth of the optical potential, the noise etc, are left unknown to us. Similarly, over damped Brownian motion in corrugated channels, a model of biophysical transport, is likely related to CTRW as a coarse grained description. In the former system exponential decay was already explored in Reference [67]. Thus exponential tails are found both via Langevin dynamics and within CTRW, the two approaches are related in some limits.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
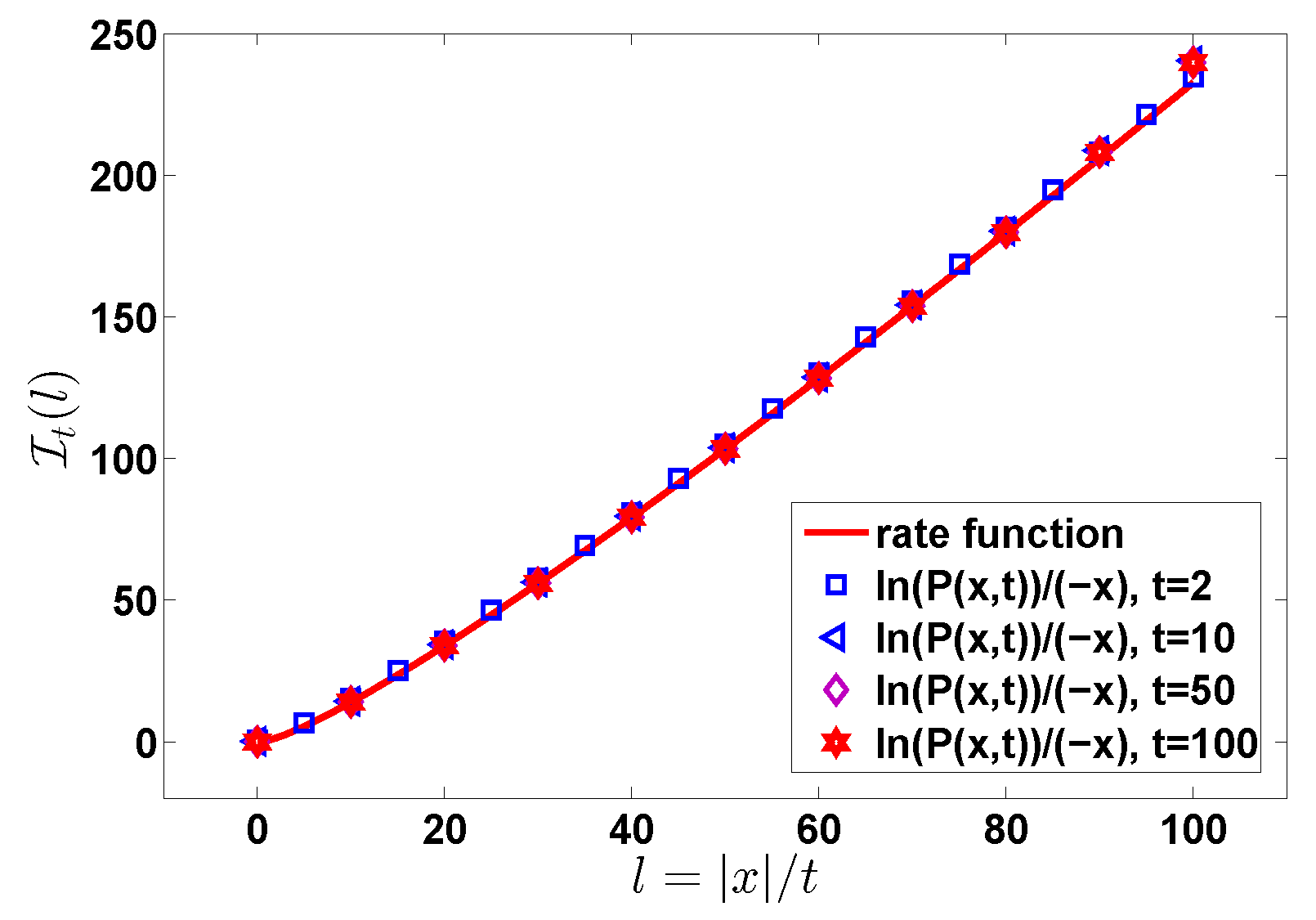
Appendix A. PDFs of n and x in the Long Time Limit
Appendix B. Calculation of Q t (n) and P(x,t) with Waiting Time Following Equation (55)

References and Note
- Majumdar, S.N. Brownian functionals in physics and computer science. In The Legacy Of Albert Einstein: A Collection of Essays in Celebration of the Year of Physics; World Scientific: Singapore, 2007; pp. 93–129. [Google Scholar]
- Montroll, E.W.; Weiss, G.H. Random walks on lattices. II. J. Math. Phys. 1965, 6, 167–181. [Google Scholar] [CrossRef]
- Haus, J.; Kehr, K. Diffusion in regular and disordered lattices. Phys. Rep. 1987, 150, 263–406. [Google Scholar] [CrossRef]
- Chaudhuri, P.; Berthier, L.; Kob, W. Universal nature of particle displacements close to glass and jamming transitions. Phys. Rev. Lett. 2007, 99, 060604. [Google Scholar] [CrossRef] [Green Version]
- Kegel, W.K.; van Blaaderen, A. Direct observation of dynamical heterogeneities in colloidal hard-sphere suspensions. Science 2000, 287, 290–293. [Google Scholar] [CrossRef] [Green Version]
- Masoliver, J.; Montero, M.; Porrà, J.M. A dynamical model describing stock market price distributions. Phys. A 2000, 283, 559–567. [Google Scholar] [CrossRef] [Green Version]
- Weeks, E.R.; Crocker, J.C.; Levitt, A.C.; Schofield, A.; Weitz, D.A. Three-dimensional direct imaging of structural relaxation near the colloidal glass transition. Science 2000, 287, 627–631. [Google Scholar] [CrossRef] [Green Version]
- Wang, B.; Anthony, S.M.; Bae, S.C.; Granick, S. Anomalous yet Brownian. Proc. Natl. Acad. Sci. USA 2009, 106, 15160–15164. [Google Scholar] [CrossRef] [Green Version]
- Hapca, S.; Crawford, J.W.; Young, I.M. Anomalous diffusion of heterogeneous populations characterized by normal diffusion at the individual level. J. R. Soc. Interface 2009, 6, 111–122. [Google Scholar] [CrossRef] [Green Version]
- Leptos, K.C.; Guasto, J.S.; Gollub, J.P.; Pesci, A.I.; Goldstein, R.E. Dynamics of enhanced tracer diffusion in suspensions of swimming eukaryotic microorganisms. Phys. Rev. Lett. 2009, 103, 198103. [Google Scholar] [CrossRef] [Green Version]
- Eisenmann, C.; Kim, C.; Mattsson, J.; Weitz, D.A. Shear melting of a colloidal glass. Phys. Rev. Lett. 2010, 104, 035502. [Google Scholar] [CrossRef] [PubMed]
- Toyota, T.; Head, D.A.; Schmidt, C.F.; Mizuno, D. Non-Gaussian athermal fluctuations in active gels. Soft Matter 2011, 7, 3234–3239. [Google Scholar] [CrossRef] [Green Version]
- Skaug, M.J.; Mabry, J.; Schwartz, D.K. Intermittent molecular hopping at the solid-liquid interface. Phys. Rev. Lett. 2013, 110, 256101. [Google Scholar] [CrossRef] [Green Version]
- Xue, C.; Zheng, X.; Chen, K.; Tian, Y.; Hu, G. Probing non-Gaussianity in confined diffusion of nanoparticles. J. Phys. Chem. 2016, 7, 514–519. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Wang, D.; Wu, H.; Schwartz, D.K. Three-dimensional tracking of interfacial hopping diffusion. Phys. Rev. Lett. 2017, 119, 268001. [Google Scholar] [CrossRef] [Green Version]
- Jeanneret, R.; Pushkin, D.O.; Kantsler, V.; Polin, M. Entrainment dominates the interaction of microalgae with micron-sized objects. Nat. Commun. 2016, 7, 1–7. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chechkin, A.V.; Seno, F.; Metzler, R.; Sokolov, I.M. Brownian yet non-Gaussian diffusion: From superstatistics to subordination of diffusing diffusivities. Phys. Rev. X 2017, 7, 021002. [Google Scholar] [CrossRef] [Green Version]
- Lampo, T.J.; Stylianidou, S.; Backlund, M.P.; Wiggins, P.A.; Spakowitz, A.J. Cytoplasmic RNA-protein particles exhibit non-Gaussian subdiffusive behavior. Biophys. J. 2017, 112, 532–542. [Google Scholar] [CrossRef] [Green Version]
- Cherstvy, A.G.; Thapa, S.; Wagner, C.E.; Metzler, R. Non-Gaussian, non-ergodic, and non-Fickian diffusion of tracers in mucin hydrogels. Soft Matter 2019, 15, 2526–2551. [Google Scholar] [CrossRef]
- Witzel, P.; Götz, M.; Lanoiselée, Y.; Franosch, T.; Grebenkov, D.S.; Heinrich, D. Heterogeneities shape passive intracellular transport. Biophys. J. 2019, 117, 203–213. [Google Scholar] [CrossRef] [Green Version]
- Shin, K.; Song, S.; Song, Y.H.; Hahn, S.; Kim, J.H.; Lee, G.; Jeong, I.C.; Sung, J.; Lee, K.T. Anomalous dynamics of in vivo cargo delivery by motor protein multiplexes. J. Phys. Chem. 2019, 10, 3071–3079. [Google Scholar] [CrossRef]
- Singh, R.K.; Mahato, J.; Chowdhury, A.; Sain, A.; Nandi, A. Non-Gaussian subdiffusion of single-molecule tracers in a hydrated polymer network. J. Chem. Phys. 2020, 152, 024903. [Google Scholar] [CrossRef] [PubMed]
- Mejia-Monasterio, C.; Nechaev, S.; Oshanin, G.; Vasilyev, O. Tracer diffusion on a crowded random Manhattan lattice. New J. Phys. 2020, 22, 033024. [Google Scholar] [CrossRef]
- Xue, C.; Shi, X.; Tian, Y.; Zheng, X.; Hu, G. Diffusion of nanoparticles with activated hopping in crowded polymer solutions. Nano Lett. 2020. [Google Scholar] [CrossRef] [PubMed]
- Chakraborty, I.; Roichman, Y. Disorder-induced Fickian, yet non-Gaussian diffusion in heterogeneous media. Phys. Rev. Res. 2020. [Google Scholar] [CrossRef]
- Wang, B.; Kuo, J.; Bae, S.C.; Granick, S. When Brownian diffusion is not Gaussian. Nat. Mater. 2012, 11, 481–485. [Google Scholar] [CrossRef]
- Munder, M.C.; Midtvedt, D.; Franzmann, T.; Nüske, E.; Otto, O.; Herbig, M.; Ulbricht, E.; Müller, P.; Taubenberger, A.; Maharana, S.; et al. A pH-driven transition of the cytoplasm from a fluid- to a solid-like state promotes entry into dormancy. eLife 2016, 5, e09347. [Google Scholar] [CrossRef]
- Barkai, E.; Burov, S. Packets of diffusing particles exhibit universal exponential tails. Phys. Rev. Lett. 2020, 124, 060603. [Google Scholar] [CrossRef] [Green Version]
- Kindermann, F.; Dechant, A.; Hohmann, M.; Lausch, T.; Mayer, D.; Schmidt, F.; Lutz, E.; Widera, A. Nonergodic diffusion of single atoms in a periodic potential. Nat. Phys. 2017, 13, 137–141. [Google Scholar] [CrossRef] [Green Version]
- Kutner, R.; Masoliver, J. The continuous time random walk, still trendy: Fifty-year history, state of art and outlook. Eur. Phys. J. B 2017, 90, 50. [Google Scholar] [CrossRef] [Green Version]
- Godrèche, C.; Luck, J.M. Statistics of the occupation time of renewal processes. J. Stat. Phys. 2001, 104, 489–524. [Google Scholar] [CrossRef]
- Derrida, B. Non-equilibrium steady states: Fluctuations and large deviations of the density and of the current. J. Stat. Mech. Theory Exp. 2007, 2007. [Google Scholar] [CrossRef]
- Chi, Z. Uniform convergence of exact large deviations for renewal reward processes. Ann. Appl. Probab. 2007, 17, 1019–1048. [Google Scholar] [CrossRef] [Green Version]
- Touchette, H. The large deviation approach to statistical mechanics. Phys. Rep. 2009, 478, 1–69. [Google Scholar] [CrossRef] [Green Version]
- Majumdar, S.N.; Vergassola, M. Large deviations of the maximum eigenvalue for wishart and Gaussian random matrices. Phys. Rev. Lett. 2009, 102, 060601. [Google Scholar] [CrossRef] [Green Version]
- Lefevere, R.; Mariani, M.; Zambotti, L. Large deviations for renewal processes. Stoch. Process. Their Appl. 2011, 121, 2243–2271. [Google Scholar] [CrossRef] [Green Version]
- Tsirelson, B. From uniform renewal theorem to uniform large and moderate deviations for renewal-reward processes. Electron. Comm. Probab. 2013, 18, 1–13. [Google Scholar] [CrossRef]
- Krapivsky, P.L.; Mallick, K.; Sadhu, T. Large deviations in single-file diffusion. Phys. Rev. Lett. 2014, 113, 078101. [Google Scholar] [CrossRef] [Green Version]
- Hegde, C.; Sabhapandit, S.; Dhar, A. Universal large deviations for the tagged particle in single-file motion. Phys. Rev. Lett. 2014, 113, 120601. [Google Scholar] [CrossRef] [Green Version]
- Mariani, M.; Zambotti, L. Large deviations for the empirical measure of heavy-tailed Markov renewal processes. Adv. Appl. Probab. 2016, 48, 648–671. [Google Scholar] [CrossRef] [Green Version]
- Lapolla, A.; Godec, A. Unfolding tagged particle histories in single-file diffusion: Exact single-and two-tag local times beyond large deviation theory. New J. Phys. 2018, 20, 113021. [Google Scholar] [CrossRef]
- Hartich, D.; Godec, A. Extreme value statistics of ergodic Markov processes from first passage times in the large deviation limit. J. Phys. A 2019, 52, 244001. [Google Scholar] [CrossRef]
- Daniels, H.E. Saddlepoint approximations in statistics. Ann. Math. Statist. 1954, 25, 631–650. [Google Scholar] [CrossRef]
- For standard random walks, namely the case where the number of jumps n is fixed, and when the PDF of jump length is exponential, one can show that P(x|n) has exponential tails for any n. In the manuscript, we wish to avoid this nearly trivial case, namely we want to show that starting with say a Gaussian PDF of jump lengths, we get an exponential tail for P(x,t). We need the effect of bunching, many jumps close one to another, then maybe some inactive phase.
- Dence, T.P. A brief look into the Lambert W function. Appl. Math. 2013, 4, 887–892. [Google Scholar] [CrossRef] [Green Version]
- Nickelsen, D.; Touchette, H. Anomalous scaling of dynamical large deviations. Phys. Rev. Lett. 2018, 121, 090602. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Touchette, H. Introduction to dynamical large deviations of Markov processes. Physica A 2018, 504, 5–19, Lecture Notes of the 14th International Summer School on Fundamental Problems in Statistical Physics. [Google Scholar] [CrossRef] [Green Version]
- Cox, D.R.; Miller, H.D. The Theory of Stochastic Processes; CRC Press: Boca Raton, FL, USA, 1977; Volume 134. [Google Scholar]
- Forbes, C.; Evans, M.; Hastings, N.; Peacock, B. Statistical Distributions; John Wiley & Sons: Hoboken, NJ, USA, 2011. [Google Scholar]
- Abramowitz, M.; Stegun, I.A. (Eds.) Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables; John Wiley & Sons, Inc.: New York, NY, USA, 1984. [Google Scholar]
- Haubold, H.J.; Mathai, A.M.; Saxena, R.K. Mittag-Leffler functions and their applications. J. Appl. Math. 2011. [Google Scholar] [CrossRef] [Green Version]
- Mandel, L. Sub-Poissonian photon statistics in resonance fluorescence. Opt. Lett. 1979, 4, 205–207. [Google Scholar] [CrossRef]
- Chubynsky, M.V.; Slater, G.W. Diffusing diffusivity: A model for anomalous, yet Brownian, diffusion. Phys. Rev. Lett. 2014, 113, 098302. [Google Scholar] [CrossRef] [Green Version]
- Jain, R.; Sebastian, K.L. Diffusion in a crowded, rearranging environment. J. Phys. Chem. B 2016, 120, 3988–3992. [Google Scholar] [CrossRef]
- Grebenkov, D.S. A unifying approach to first-passage time distributions in diffusing diffusivity and switching diffusion models. J. Phys. A Math. Theor. 2019, 52, 174001. [Google Scholar] [CrossRef] [Green Version]
- Metzler, R. Superstatistics and non-Gaussian diffusion. Eur. Phys. J. Spec. Top. 2020, 229, 711–728. [Google Scholar] [CrossRef] [Green Version]
- Hidalgo-Soria, M.; Barkai, E. The Hitchhiker model for Laplace diffusion processes in the cell environment. arXiv 2019, arXiv:1909.07189. [Google Scholar]
- Hou, R.; Cherstvy, A.G.; Metzler, R.; Akimoto, T. Biased continuous-time random walks for ordinary and equilibrium cases: Facilitation of diffusion, ergodicity breaking and ageing. Phys. Chem. Chem. Phys. 2018, 20, 20827–20848. [Google Scholar] [CrossRef] [PubMed]
- Bouchaud, J.P.; Georges, A. Anomalous diffusion in disordered media: Statistical mechanisms, models and physical applications. Phys. Rep. 1990, 195, 127–293. [Google Scholar] [CrossRef]
- Metzler, R.; Klafter, J. The random walk’s guide to anomalous diffusion: A fractional dynamics approach. Phys. Rep. 2000, 339, 1–77. [Google Scholar] [CrossRef]
- Jeon, J.H.; Barkai, E.; Metzler, R. Noisy continuous time random walks. J. Chem. Phys. 2013, 139, 121916. [Google Scholar] [CrossRef] [Green Version]
- Klafter, J.; Zumofen, G. Lévy statistics in a Hamiltonian system. Phys. Rev. E 1994, 49, 4873–4877. [Google Scholar] [CrossRef]
- Magdziarz, M.; Zorawik, T. Aging ballistic Lévy walks. Phys. Rev. E 2017, 95, 022126. [Google Scholar] [CrossRef]
- Chistjakov, V.P. A theorem on sums of independent positive random variables and its applications to branching random processes. Theory Probab. Appl. 1964, 9, 640–648. [Google Scholar] [CrossRef]
- Vezzani, A.; Barkai, E.; Burioni, R. Single-big-jump principle in physical modeling. Phys. Rev. E 2019, 100, 012108. [Google Scholar] [CrossRef] [Green Version]
- Wang, W.; Vezzani, A.; Burioni, R.; Barkai, E. Transport in disordered systems: The single big jump approach. Phys. Rev. Res. 2019, 1, 033172. [Google Scholar] [CrossRef] [Green Version]
- Li, Y.; Marchesoni, F.; Debnath, D.; Ghosh, P.K. Non-Gaussian normal diffusion in a fluctuating corrugated channel. Phys. Rev. Res. 2019, 1, 033003. [Google Scholar] [CrossRef] [Green Version]






© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, W.; Barkai, E.; Burov, S. Large Deviations for Continuous Time Random Walks. Entropy 2020, 22, 697. https://doi.org/10.3390/e22060697
Wang W, Barkai E, Burov S. Large Deviations for Continuous Time Random Walks. Entropy. 2020; 22(6):697. https://doi.org/10.3390/e22060697
Chicago/Turabian StyleWang, Wanli, Eli Barkai, and Stanislav Burov. 2020. "Large Deviations for Continuous Time Random Walks" Entropy 22, no. 6: 697. https://doi.org/10.3390/e22060697
APA StyleWang, W., Barkai, E., & Burov, S. (2020). Large Deviations for Continuous Time Random Walks. Entropy, 22(6), 697. https://doi.org/10.3390/e22060697