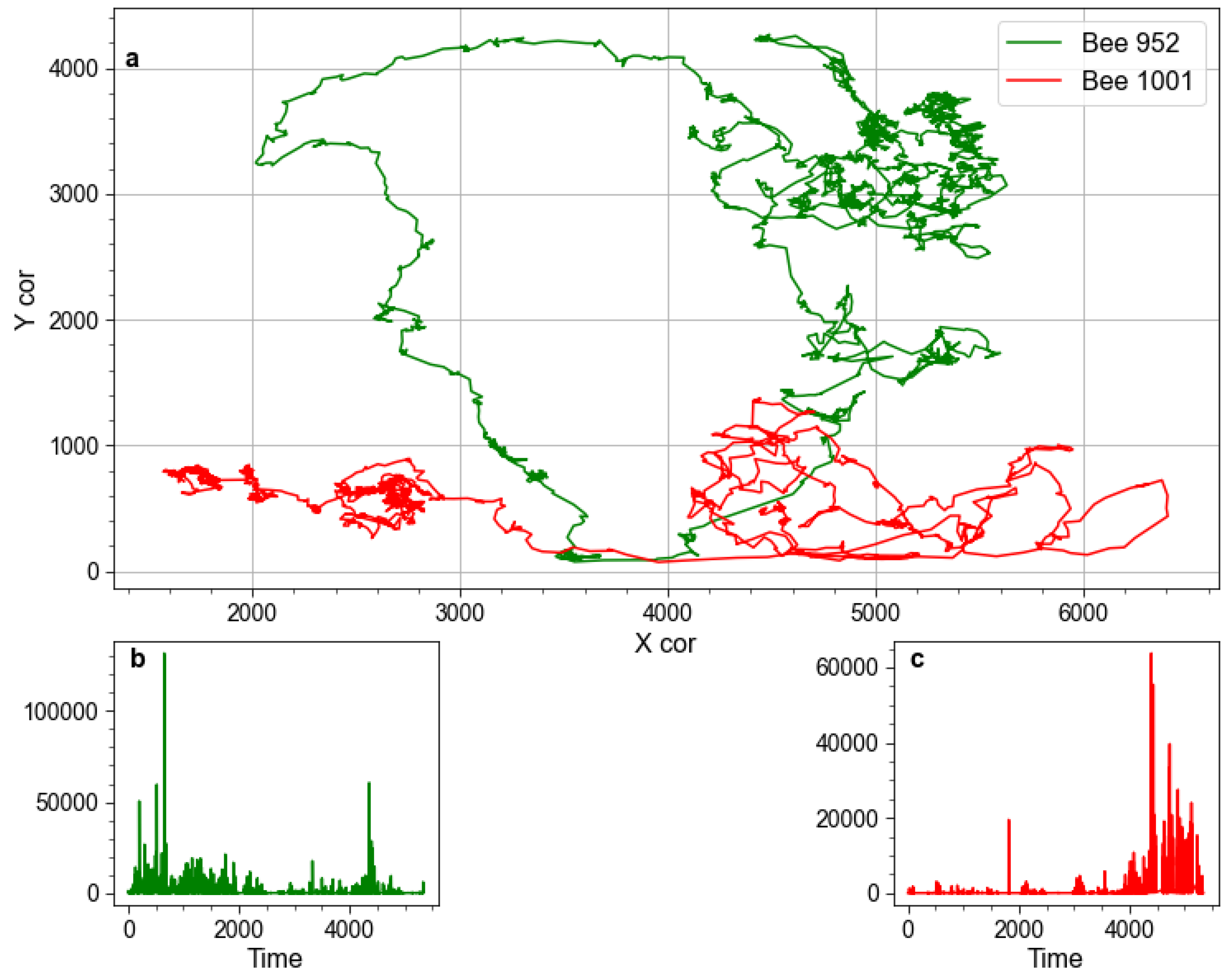
4.1. Key Finding and Their Implications
In our work, we analyzed a dataset of bee trajectories in a way that allowed us to compare empirical data to a well-studied model. Such an approach has been previously used (e.g., [
19]) to show that key dynamical characteristics of the human brain at its resting state exhibit a notable resemblance to the Ising model when the latter is in the vicinity of the critical state. Long-range temporal autocorrelations are also considered a hallmark of criticality in the Ising model and the neural time-series [
43]. With some minor differences, which are to be expected given the different nature of the data, our analysis of bee correlation networks yielded remarkably similar results, namely similarity to the Ising model at
and lack thereof at low and high temperatures.
First, our observations are confirmed by the rigorous statistical analysis of the degree distributions. Second, the analysis of the correlation networks showed that certain characteristics, most notably clustering and path length, are comparable between the network of bee correlations and critical state of the model, but are drastically different otherwise. Third, analysis of the mean parameters and M showed that recordings of the average activity of both the bee hive and the Ising model at critical states are marked with considerable autocorrelations within the time-series.
These similarities in the dynamics of the systems become even more noteworthy when we consider that in structural terms, both the Ising model and the human brain are drastically different from the bee hive. In the context of our analyses, differences in spatial structure are particularly important. The two-dimensional Ising model possesses only nearest-neighbor interactions, while the human brain is characterized by intricate connection patterns on the macro and micro levels. Although correlation networks are obtained solely from dynamics, one would expect them to be strongly influenced by the underlying spatial structure. The bee hive is quite different because the nodes of its correlation matrix represent agents largely unhindered by constraints in movement and interaction. Despite these dissimilarities, functional constraints on behavior are shared by the systems in question to a degree that precludes the appearance of dynamical similarities by mere chance. We believe that they signal a shared systemic property—the critical state, whose influence on the systems’ dynamics goes beyond specific structural constraints.
One potential concern with our comparative analysis is the choice of running the Ising model on a 2D grid, thereby imposing a local interaction constraint on the system. Alternatively, we could have implemented the model on a complete graph, in which all lattice sites are connected. Intuitively, that might seem a better approximation of the bee hive, with highly mobile agents. Empirical research and modeling studies of flocks and schools have demonstrated that in such systems, an ubiquitous strategy of the individual agents is to align their behavior with that of the nearby neighbors [
44,
45,
46]. Information, however, could propagate through the whole system seemingly unencumbered by the prevalence of local connections. Such behavior is a clear mark of critical state. We do not have definitive information that bees follow such an alignment pattern inside the hive, yet the remarkable ability of the 2D Ising model with only local interaction to emulate a high-level description of bee hive dynamics, implies that a similar mechanism could be in play.
4.2. Critical Brains and Critical Swarms
Our results provide evidence in support of what we could call a “critical colony” hypothesis. As we had summarized earlier in
Section 1.2, being in a critical state provides benefits to a system’s cognitive ability, to an extent that it can be argued that such a state is not simply advantageous, but even necessary. At the same time, there are convincing arguments in
Section 1.1 for the claim that social insects are endowed with a sort of collective cognitive capacity, which, despite their entirely different mode of organization, shares key similarities with the capacities of vertebrate brains. Thus, we suggest that the benefits that the critical state brings would be desirable characteristic for both of these systems.
Previous research showed that hallmarks of the critical state are observable in swarming midges [
3] and flocking birds [
2]. Moreover, previous research on the same bee dataset found that the time-series of the
of the bee hive exhibits bursts of activity, significantly exceeding the average level, which is interspersed with quiescent periods [
30]. The distribution of waiting times between these bursts abides by the power-law distribution. The authors did not discuss criticality as such, but it is noteworthy that this kind of distribution is also found to be the case for “avalanches” in the Back–Wisenfield sandpile model, a famous model of self-organized criticality [
47], and for the patterns of the neural activity recorded in the cortical slices of mice [
18] in vitro.
One of the benefits of the critical state in neural networks is that it enables an easier spread and integration of information. Our findings show that the correlation graphs of bee activity exhibit distinct small-world structures and contain hubs—nodes with exceptionally high degrees. A similar organization emerges in the Ising model when
. Such network properties are also known to enhance the integration of information and facilitate the spreading of signals. In the human brain, there is a similar structural organization of cortical wiring [
48,
49]. The bee hive and the critical Ising model lack such structural constraints, yet their functional connectivity is remarkably similar. Uncovering such network structures in the functional correlations of the bees suggests that this type of organization is not only ubiquitous in the brain but is fundamental to the functionality of any cognitive system.
It is worth highlighting important differences between our work and the corpus of research that concerns hallmarks of criticality in the swarm dynamics, as well as with the studies employing tools of network science to study eusocial insects [
5,
50]. The most notable dissimilarity with the latter is that most studies focused on social networks, constructed by observing species-specific means of communication, such as trophallaxis in bees and attenuation in ants. Such contacts, important as they are, only constitute a fraction of individuals’ total activity, and they arguably account only for a portion of the total informational exchange that takes place inside the colony. Significant correlations between the kinetic energy time-series of individuals and highly specific degree distributions of the correlation graph, in our view, reflect features of the social system underlying collective cognition in the hive, aided by its critical state.
Hallmarks of critical behavior in collective entities, when studied empirically, were investigated in freely-moving agents: flocking birds or swarming midges. Thus, it is possible to argue that observed features, such as long-range velocity correlations, were begotten by the spatial order the organisms maintained and are transient in nature.
The movement data we analyzed were acquired when bees were locked inside the hive and provided with sustenance. They were under no pressure to maintain a specific movement order, as they would have been during swarming, or to move at all, in fact. Given these conditions, it is plausible that the hallmarks of the critical state revealed by our analysis would be observed only if such a state is inherent to the system, much like it is to the human brain at its resting state. Indeed, brain resting-state networks have been proposed to reflect a process of “constant inner state of exploration” that optimizes the system for a given impending input, thus influencing perception and cognitive processing [
51,
52]. It is exciting to consider the possibility that the colonies of eusocial insects exhibit a similar process.