Network Higher-Order Structure Dismantling
Abstract
1. Introduction
2. Network Higher-Order Structure Dismantling
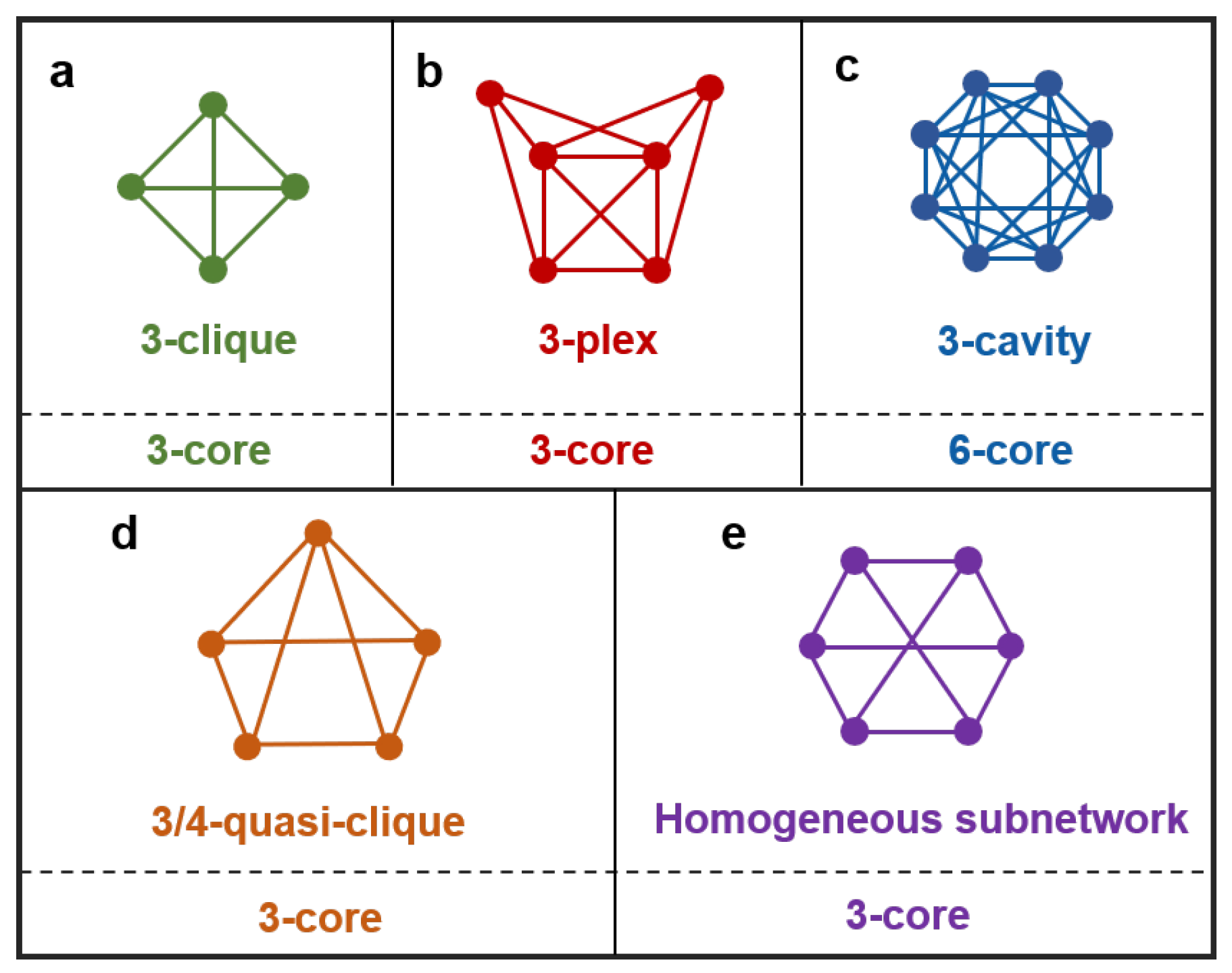
2.1. Definition
2.2. Evaluation Metrics
3. Belief Propagation-Guided Higher-Order Dismantling
4. Results
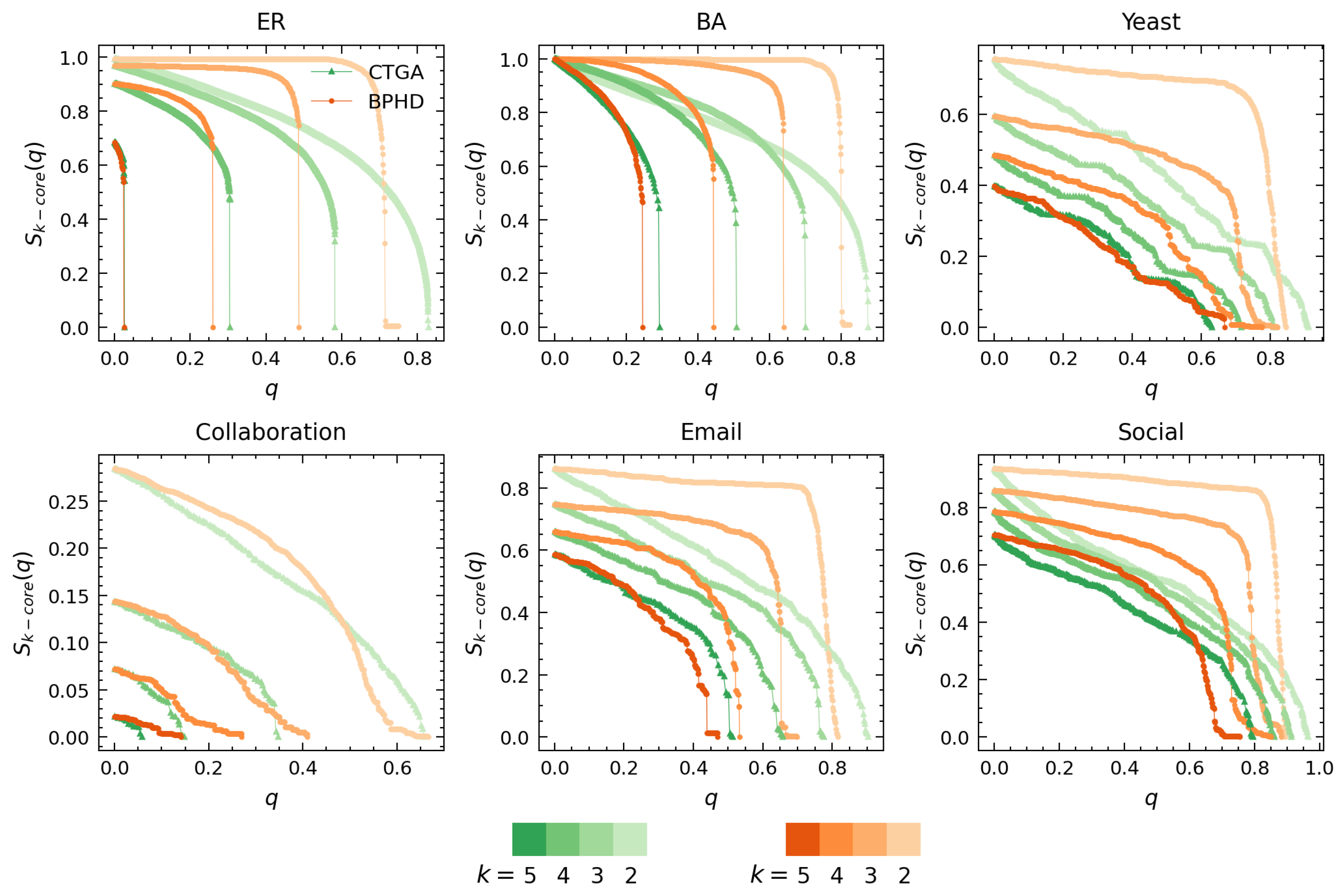
4.1. Connectivity Dismantling
4.2. Higher-Order Structure Dismantling
5. Conclusions and Discussion
6. Methods
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Albert, R.; Jeong, H.; Barabási, A.L. Error and attack tolerance of complex networks. Nature 2000, 406, 378–382. [Google Scholar] [CrossRef]
- Morone, F.; Makse, H.A. Influence Maximization in Complex Networks through Optimal Percolation. Nature 2015, 524, 65–68. [Google Scholar] [CrossRef]
- Lü, L.; Zhou, T. Link prediction in complex networks: A survey. Physica A Stat. Mech. Appl. 2011, 390, 1150–1170. [Google Scholar] [CrossRef]
- Clusella, P.; Grassberger, P.; Pérez-Reche, F.J.; Politi, A. Immunization and targeted destruction of networks using explosive percolation. Phys. Rev. Lett. 2016, 117, 208301. [Google Scholar] [CrossRef]
- Lü, L.; Chen, D.; Ren, X.L.; Zhang, Q.M.; Zhang, Y.C.; Zhou, T. Vital nodes identification in complex networks. Phys. Rep. 2016, 650, 1–63. [Google Scholar] [CrossRef]
- Cohen, R.; Erez, K.; Ben-Avraham, D.; Havlin, S. Breakdown of the internet under intentional attack. Phys. Rev. Lett. 2001, 86, 3682. [Google Scholar] [CrossRef] [PubMed]
- Braunstein, A.; Dall’Asta, L.; Semerjian, G.; Zdeborová, L. Network Dismantling. Proc. Natl. Acad. Sci. USA 2016, 113, 12368–12373. [Google Scholar] [CrossRef] [PubMed]
- Schneider, C.M.; Moreira, A.A.; Andrade, J.S.; Havlin, S.; Herrmann, H.J. Mitigation of Malicious Attacks on Networks. Proc. Natl. Acad. Sci. USA 2011, 108, 3838–3841. [Google Scholar] [CrossRef] [PubMed]
- Ren, X.L.; Gleinig, N.; Helbing, D.; Antulov-Fantulin, N. Generalized Network Dismantling. Proc. Natl. Acad. Sci. USA 2019, 116, 6554–6559. [Google Scholar] [CrossRef] [PubMed]
- Krebs, V.E. Mapping networks of terrorist cells. Connections 2002, 24, 43–52. [Google Scholar]
- Yan, G.; Vértes, P.E.; Towlson, E.K.; Chew, Y.L.; Walker, D.S.; Schafer, W.R.; Barabási, A.L. Network control principles predict neuron function in the Caenorhabditis elegans connectome. Nature 2017, 550, 519–523. [Google Scholar] [CrossRef] [PubMed]
- Lehár, J.; Krueger, A.; Zimmermann, G.; Borisy, A. High-order combination effects and biological robustness. Mol. Syst. Biol. 2008, 4, 215. [Google Scholar] [CrossRef] [PubMed]
- Morone, F.; Del Ferraro, G.; Makse, H.A. The K-Core as a Predictor of Structural Collapse in Mutualistic Ecosystems. Nat. Phys. 2019, 15, 95–102. [Google Scholar] [CrossRef]
- Wu, A.K.; Tian, L.; Liu, Y.Y. Bridges in complex networks. Phys. Rev. E 2018, 97, 012307. [Google Scholar] [CrossRef] [PubMed]
- Requião da Cunha, B.; González-Avella, J.C.; Gonçalves, S. Fast fragmentation of networks using module-based attacks. PLoS ONE 2015, 10, e0142824. [Google Scholar] [CrossRef] [PubMed]
- Musciotto, F.; Miccichè, S. Exploring the landscape of dismantling strategies based on the community structure of networks. Sci. Rep. 2023, 13, 14448. [Google Scholar] [CrossRef]
- Peng, P.; Fan, T.; Ren, X.L.; Lü, L. Unveiling Explosive Vulnerability of Networks through Edge Collective Behavior. arXiv 2023, arXiv:2310.06407. [Google Scholar]
- Lambiotte, R.; Rosvall, M.; Scholtes, I. From Networks to Optimal Higher-Order Models of Complex Systems. Nat. Phys. 2019, 15, 313–320. [Google Scholar] [CrossRef]
- Battiston, F.; Cencetti, G.; Iacopini, I.; Latora, V.; Lucas, M.; Patania, A.; Young, J.G.; Petri, G. Networks beyond Pairwise Interactions: Structure and Dynamics. Phys. Rep. 2020, 874, 1–92. [Google Scholar] [CrossRef]
- Sanchez-Gorostiaga, A.; Bajić, D.; Osborne, M.L.; Poyatos, J.F.; Sanchez, A. High-order interactions distort the functional landscape of microbial consortia. PLoS Biol. 2019, 17, e3000550. [Google Scholar] [CrossRef]
- Yu, S.; Yang, H.; Nakahara, H.; Santos, G.S.; Nikolić, D.; Plenz, D. Higher-Order Interactions Characterized in Cortical Activity. J. Neurosci. 2011, 31, 17514–17526. [Google Scholar] [CrossRef]
- Alvarez-Rodriguez, U.; Battiston, F.; de Arruda, G.F.; Moreno, Y.; Perc, M.; Latora, V. Evolutionary dynamics of higher-order interactions in social networks. Nat. Hum. Behav. 2021, 5, 586–595. [Google Scholar] [CrossRef]
- Grilli, J.; Barabás, G.; Michalska-Smith, M.J.; Allesina, S. Higher-Order Interactions Stabilize Dynamics in Competitive Network Models. Nature 2017, 548, 210–213. [Google Scholar] [CrossRef] [PubMed]
- Myers, C.R. Software Systems as Complex Networks: Structure, Function, and Evolvability of Software Collaboration Graphs. Phys. Rev. E 2003, 68, 046116. [Google Scholar] [CrossRef]
- Battiston, F.; Amico, E.; Barrat, A.; Bianconi, G.; Ferraz de Arruda, G.; Franceschiello, B.; Iacopini, I.; Kéfi, S.; Latora, V.; Moreno, Y.; et al. The Physics of Higher-Order Interactions in Complex Systems. Nat. Phys. 2021, 17, 1093–1098. [Google Scholar] [CrossRef]
- Zhang, Y.; Lucas, M.; Battiston, F. Higher-order interactions shape collective dynamics differently in hypergraphs and simplicial complexes. Nat. Commun. 2023, 14, 1605. [Google Scholar] [CrossRef]
- Milo, R.; Shen-Orr, S.; Itzkovitz, S.; Kashtan, N.; Chklovskii, D.; Alon, U. Network motifs: Simple building blocks of complex networks. Science 2002, 298, 824–827. [Google Scholar] [CrossRef] [PubMed]
- Spirin, V.; Mirny, L.A. Protein complexes and functional modules in molecular networks. Proc. Natl. Acad. Sci. USA 2003, 100, 12123–12128. [Google Scholar] [CrossRef]
- Benson, A.R.; Gleich, D.F.; Leskovec, J. Higher-Order Organization of Complex Networks. Science 2016, 353, 163–166. [Google Scholar] [CrossRef]
- Sizemore, A.E.; Giusti, C.; Kahn, A.; Vettel, J.M.; Betzel, R.F.; Bassett, D.S. Cliques and cavities in the human connectome. J. Comput. Neurosci. 2018, 44, 115–145. [Google Scholar] [CrossRef]
- Giusti, C.; Pastalkova, E.; Curto, C.; Itskov, V. Clique Topology Reveals Intrinsic Geometric Structure in Neural Correlations. Proc. Natl. Acad. Sci. USA 2015, 112, 13455–13460. [Google Scholar] [CrossRef]
- Lu, Y.; Huang, Y.; Li, T. More is Different: Constructing the Most Comprehensive Human Protein High-Order Interaction Dataset. bioRxiv 2023. [Google Scholar] [CrossRef]
- Marsh, J.A.; Teichmann, S.A. Structure, dynamics, assembly, and evolution of protein complexes. Annu. Rev. Biochem. 2015, 84, 551–575. [Google Scholar] [CrossRef]
- Bairey, E.; Kelsic, E.D.; Kishony, R. High-Order Species Interactions Shape Ecosystem Diversity. Nat. Commun. 2016, 7, 12285. [Google Scholar] [CrossRef]
- Mayfield, M.M.; Stouffer, D.B. Higher-Order Interactions Capture Unexplained Complexity in Diverse Communities. Nat. Ecol. Evol. 2017, 1, 0062. [Google Scholar] [CrossRef]
- Pattison, P.; Robins, G. Neighborhood–based models for social networks. Sociol. Methodol. 2002, 32, 301–337. [Google Scholar] [CrossRef]
- Majhi, S.; Perc, M.; Ghosh, D. Dynamics on higher-order networks: A review. J. R. Soc. Interface 2022, 19, 20220043. [Google Scholar] [CrossRef]
- Kavitha, T.; Liebchen, C.; Mehlhorn, K.; Michail, D.; Rizzi, R.; Ueckerdt, T.; Zweig, K.A. Cycle bases in graphs characterization, algorithms, complexity, and applications. Comput. Sci. Rev. 2009, 3, 199–243. [Google Scholar] [CrossRef]
- Fan, T.; Lü, L.; Shi, D.; Zhou, T. Characterizing cycle structure in complex networks. Commun. Phys. 2021, 4, 272. [Google Scholar] [CrossRef]
- Zhang, W.; Li, W.; Deng, W. The characteristics of cycle-nodes-ratio and its application to network classification. Commun. Nonlinear Sci. Numer. Simul. 2021, 99, 105804. [Google Scholar] [CrossRef]
- Zdeborová, L.; Zhang, P.; Zhou, H.J. Fast and simple decycling and dismantling of networks. Sci. Rep. 2016, 6, 37954. [Google Scholar] [CrossRef]
- Goltsev, A.V.; Dorogovtsev, S.N.; Mendes, J.F.F. k-core (bootstrap) percolation on complex networks: Critical phenomena and nonlocal effects. Phys. Rev. E 2006, 73, 056101. [Google Scholar] [CrossRef]
- Dorogovtsev, S.N.; Goltsev, A.V.; Mendes, J.F.F. K-core organization of complex networks. Phys. Rev. Lett. 2006, 96, 040601. [Google Scholar] [CrossRef]
- Schaub, M.T.; Benson, A.R.; Horn, P.; Lippner, G.; Jadbabaie, A. Random walks on simplicial complexes and the normalized Hodge 1-Laplacian. SIAM Rev. 2020, 62, 353–391. [Google Scholar] [CrossRef]
- Vega, Y.M.; Vázquez-Prada, M.; Pacheco, A.F. Fitness for synchronization of network motifs. Phys. A: Stat. Mech. Its Appl. 2004, 343, 279–287. [Google Scholar] [CrossRef]
- Iacopini, I.; Petri, G.; Barrat, A.; Latora, V. Simplicial models of social contagion. Nat. Commun. 2019, 10, 2485. [Google Scholar] [CrossRef] [PubMed]
- Derényi, I.; Palla, G.; Vicsek, T. Clique percolation in random networks. Phys. Rev. Lett. 2005, 94, 160202. [Google Scholar] [CrossRef] [PubMed]
- Zhou, H.J. Spin Glass Approach to the Feedback Vertex Set Problem. Eur. Phys. J. B 2013, 86, 455. [Google Scholar] [CrossRef]
- Mugisha, S.; Zhou, H.J. Identifying Optimal Targets of Network Attack by Belief Propagation. Phys. Rev. E 2016, 94, 012305. [Google Scholar] [CrossRef] [PubMed]
- Shi, D.; Chen, Z.; Sun, X.; Chen, Q.; Ma, C.; Lou, Y.; Chen, G. Computing cliques and cavities in networks. Commun. Phys. 2021, 4, 249. [Google Scholar] [CrossRef]
- Sanei-Mehri, S.V.; Das, A.; Tirthapura, S. Enumerating top-k quasi-cliques. In Proceedings of the 2018 IEEE International Conference on Big Data (Big Data), Seattle, WA, USA, 10–13 December 2018; pp. 1107–1112. [Google Scholar]
- Balasundaram, B.; Butenko, S.; Hicks, I.V. Clique relaxations in social network analysis: The maximum k-plex problem. Oper. Res. 2011, 59, 133–142. [Google Scholar] [CrossRef]
- Shi, D.; Chen, G.; Thong, W.W.K.; Yan, X. Searching for optimal network topology with best possible synchronizability. IEEE Circuits Syst. Mag. 2013, 13, 66–75. [Google Scholar] [CrossRef]
- Yeger-Lotem, E.; Sattath, S.; Kashtan, N.; Itzkovitz, S.; Milo, R.; Pinter, R.Y.; Alon, U.; Margalit, H. Network motifs in integrated cellular networks of transcription–regulation and protein–protein interaction. Proc. Natl. Acad. Sci. USA 2004, 101, 5934–5939. [Google Scholar] [CrossRef] [PubMed]
- Alon, U. Network motifs: Theory and experimental approaches. Nat. Rev. Genet. 2007, 8, 450–461. [Google Scholar] [CrossRef] [PubMed]
- Rives, A.W.; Galitski, T. Modular organization of cellular networks. Proc. Natl. Acad. Sci. USA 2003, 100, 1128–1133. [Google Scholar] [CrossRef] [PubMed]
- Bettencourt, L.M.; Gintautas, V.; Ham, M.I. Identification of functional information subgraphs in complex networks. Phys. Rev. Lett. 2008, 100, 238701. [Google Scholar] [CrossRef] [PubMed]
- Itzkovitz, S.; Alon, U. Subgraphs and network motifs in geometric networks. Phys. Rev. E 2005, 71, 026117. [Google Scholar] [CrossRef] [PubMed]
- Newman, M. Networks; Oxford University Press: Oxford, UK, 2018. [Google Scholar]
- Barabasi, A.L.; Albert, R. Emergence of Scaling in Random Networks. Science 1999, 286, 509–512. [Google Scholar] [CrossRef] [PubMed]
- Jeong, H.; Mason, S.P.; Barabási, A.L.; Oltvai, Z.N. Lethality and centrality in protein networks. Nature 2001, 411, 41–42. [Google Scholar] [CrossRef]
- Rossi, R.; Ahmed, N. The Network Data Repository with Interactive Graph Analytics and Visualization. Proc. AAAI Conf. Artif. Intell. 2015, 29, 2492–2493. [Google Scholar] [CrossRef]
- Cheng, X.Q.; Ren, F.X.; Shen, H.W.; Zhang, Z.K.; Zhou, T. Bridgeness: A Local Index on Edge Significance in Maintaining Global Connectivity. J. Stat. Mech. Theory Exp. 2010, 2010, P10011. [Google Scholar] [CrossRef]
- Girvan, M.; Newman, M.E. Community Structure in Social and Biological Networks. Proc. Natl. Acad. Sci. 2002, 99, 7821–7826. [Google Scholar] [CrossRef]
- Zhou, H.J. Cycle-Tree Guided Attack of Random K-core: Spin Glass Model and Efficient Message-Passing Algorithm. Sci. China Physics. Mech. Astron. 2022, 65, 230511. [Google Scholar] [CrossRef]
- Zhao, D.; Li, R.; Peng, H.; Zhong, M.; Wang, W. Higher-order percolation in simplicial complexes. Chaos Solitons Fractals 2022, 155, 111701. [Google Scholar] [CrossRef]
- Sharma, A.; Vanjani, P.; Paliwal, N.; Basnayaka, C.M.W.; Jayakody, D.N.K.; Wang, H.C.; Muthuchidambaranathan, P. Communication and networking technologies for UAVs: A survey. J. Netw. Comput. Appl. 2020, 168, 102739. [Google Scholar] [CrossRef]
- Xu, J.J.; Chen, H. Fighting organized crimes: Using shortest-path algorithms to identify associations in criminal networks. Decis. Support Syst. 2004, 38, 473–487. [Google Scholar] [CrossRef]
- Holland, P.W.; Laskey, K.B.; Leinhardt, S. Stochastic blockmodels: First steps. Soc. Netw. 1983, 5, 109–137. [Google Scholar] [CrossRef]



| Dismantling Cost | ||||||
|---|---|---|---|---|---|---|
| Network | N | M | kmax | BPHD | Suboptimal | |
| ER | 10,000 | 35,000 | 7.00 | 5 | 0.74 | 0.84 |
| BA | 10,000 | 49,985 | 10.00 | 5 | 0.82 | 0.88 |
| Yeast | 2375 | 11,693 | 9.85 | 40 | 0.79 | 0.84 |
| Collaboration | 5094 | 7515 | 2.95 | 7 | 0.51 | 0.75 |
| 1134 | 5451 | 9.61 | 11 | 0.80 | 0.93 | |
| Social | 2000 | 16,098 | 16.10 | 24 | 0.85 | 0.88 |
| Dismantling Cost | ||||||||
|---|---|---|---|---|---|---|---|---|
| 2-Core | 3-Core | 4-Core | 5-Core | |||||
| Network | BPHD | CTGA | BPHD | CTGA | BPHD | CTGA | BPHD | CTGA |
| ER | 0.75 | 0.83 | 0.49 | 0.58 | 0.26 | 0.30 | 0.02 | 0.03 |
| BA | 0.82 | 0.76 | 0.64 | 0.70 | 0.44 | 0.51 | 0.25 | 0.29 |
| Yeast | 0.84 | 0.91 | 0.82 | 0.81 | 0.77 | 0.71 | 0.67 | 0.63 |
| Collaboration | 0.78 | 0.66 | 0.39 | 0.35 | 0.25 | 0.15 | 0.13 | 0.06 |
| 0.82 | 0.90 | 0.70 | 0.78 | 0.53 | 0.66 | 0.47 | 0.51 | |
| Social | 0.89 | 0.96 | 0.88 | 0.91 | 0.85 | 0.86 | 0.75 | 0.80 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Peng, P.; Fan, T.; Lü, L. Network Higher-Order Structure Dismantling. Entropy 2024, 26, 248. https://doi.org/10.3390/e26030248
Peng P, Fan T, Lü L. Network Higher-Order Structure Dismantling. Entropy. 2024; 26(3):248. https://doi.org/10.3390/e26030248
Chicago/Turabian StylePeng, Peng, Tianlong Fan, and Linyuan Lü. 2024. "Network Higher-Order Structure Dismantling" Entropy 26, no. 3: 248. https://doi.org/10.3390/e26030248
APA StylePeng, P., Fan, T., & Lü, L. (2024). Network Higher-Order Structure Dismantling. Entropy, 26(3), 248. https://doi.org/10.3390/e26030248







