Density Distribution of Strongly Quantum Degenerate Fermi Systems Simulated by Fictitious Identical Particle Thermodynamics
Abstract
:1. Introduction
2. Fictitious Identical Particle PIMD and Constant Density Semi-Extrapolation Method
2.1. Fictitious Identical Particle PIMD
2.2. The Extrapolation Method for Strongly Quantum Degenerate Fermi Gases and Its Application in Density Distribution
- (1)
- Obtain the p-values (energy E or density ) at 13 points in at different temperatures through PIMD simulations;
- (2)
- Fit the above data to obtain the function for p as a function of temperature at different ;
- (3)
- Given a p-value, solve for the temperature T at different where ;
- (4)
- Use the corresponding , T as input data into Equation (19) for fitting, obtaining the corresponding coefficients , , , and ;
- (5)
- (6)
- Select a series of different p-values and repeat steps (3) to (5) to obtain the p-values of fermions at different temperatures.
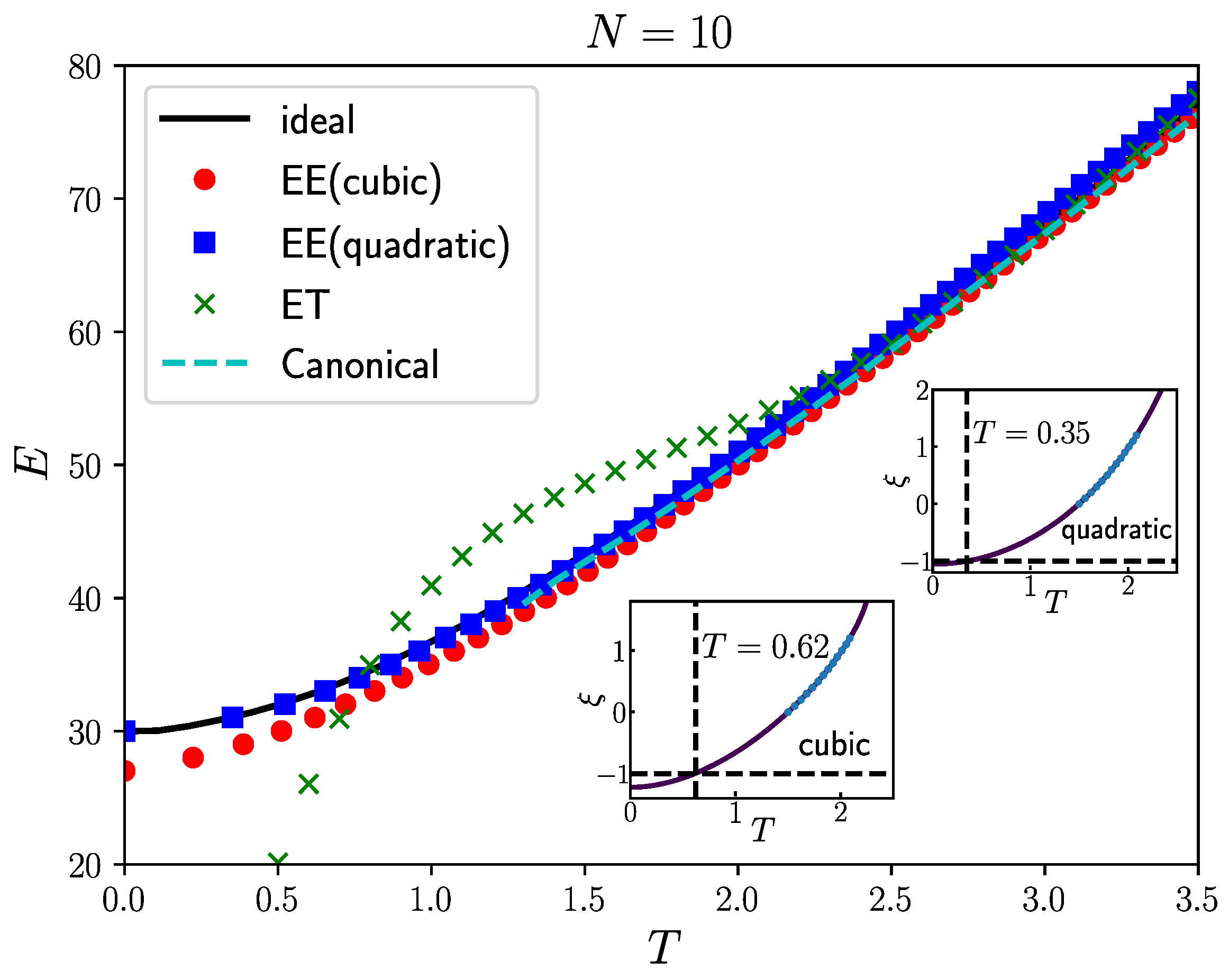
3. Results
3.1. Non-Interacting Case
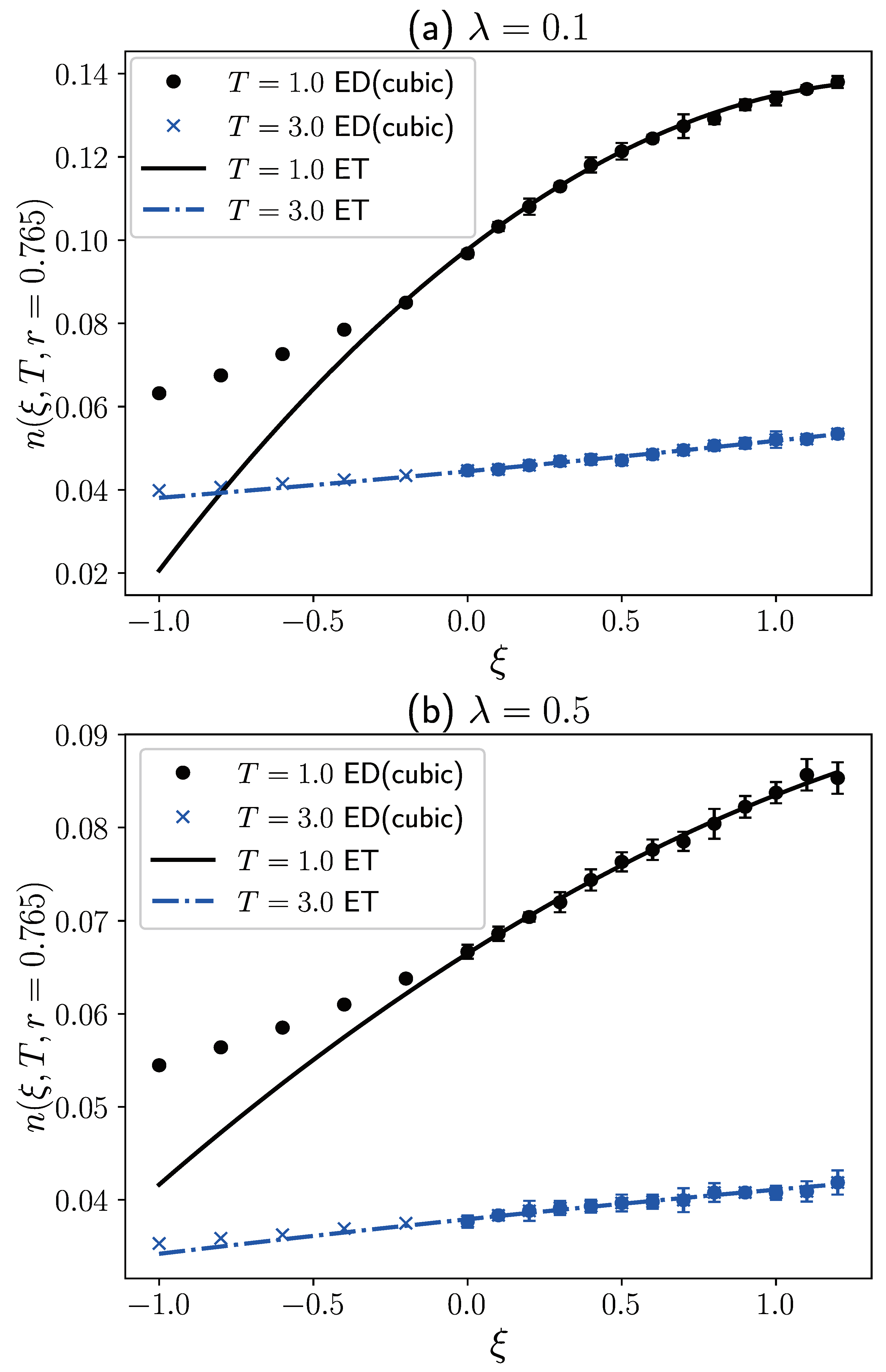
3.2. Interacting Case
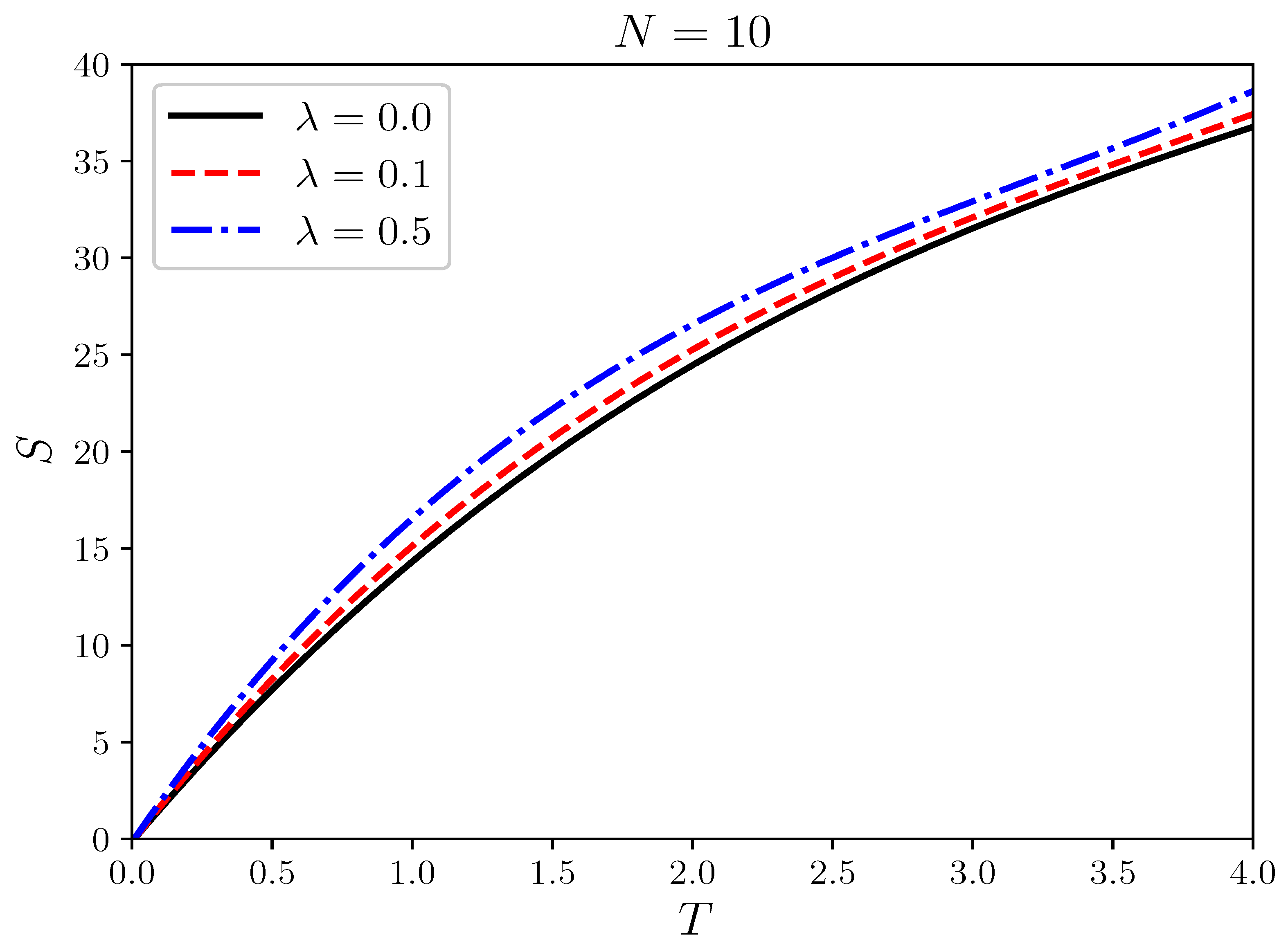
3.3. Ab Initio Simulation of Entropy in Fermi Systems
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ceperley, D.M. Path Integral Monte Carlo Methods for Fermions. In Monte Carlo and Molecular Dynamics of Condensed Matter Systems; Binder, K., Ciccotti, G., Eds.; Editrice Compositori: Bologna, Italy, 1996. [Google Scholar]
- Troyer, M.; Wiese, U.J. Computational Complexity and Fundamental Limitations to Fermionic Quantum Monte Carlo Simulations. Phys. Rev. Lett. 2005, 94, 170201. [Google Scholar] [CrossRef] [PubMed]
- Dornheim, T.; Groth, S.; Bonitz, M. The uniform electron gas at warm dense matter conditions. Phys. Rep. 2018, 744, 1. [Google Scholar] [CrossRef]
- Alexandru, A.; Basar, G.; Bedaque, P.F.; Warrington, N.C. Complex paths around the sign problem. Rev. Mod. Phys. 2022, 94, 015006. [Google Scholar] [CrossRef]
- Bonitz, M.; Vorberger, J.; Bethkenhagen, M.; Böhme, M.; Ceperley, D.; Filinov, A.; Gawne, T.; Graziani, F.; Gregori, G.; Hamann, P.; et al. Toward First Principle Simulations of Dense Hydrogen. Phys. Plasmas 2024, 31, 110501. [Google Scholar] [CrossRef]
- Martin, R.M.; Reining, L.; Ceperley, D.M. Interacting Electrons: Theory and Computational Approaches; Cambridge University Press: Cambridge, UK, 2016. [Google Scholar]
- Feynman, R.P.; Albert, R.H. Quantum Mechanics and Path Integrals; McGraw-Hill: New York, NY, USA, 1965. [Google Scholar]
- Tuckerman, M.E. Statistical Mechanics: Theory and Molecular Simulation; Oxford University: New York, NY, USA, 2010. [Google Scholar]
- Fosdick, L.D.; Jordan, H.F. Path-integral calculation of the two-particle Slater sum for He4. Phys. Rev. 1966, 143, 58–66. [Google Scholar] [CrossRef]
- Herman, M.F.; Bruskin, E.J.; Berne, B.J. On path integral Monte Carlo simulations. J. Chem. Phys. 1982, 76, 5150–5155. [Google Scholar] [CrossRef]
- Ceperley, D.M. Path integrals in the theory of condensed helium. Rev. Mod. Phys. 1995, 67, 279. [Google Scholar] [CrossRef]
- Boninsegni, M.; Prokof’ev, N.V.; Svistunov, B.V. Worm Algorithm for Continuous-Space Path Integral Monte Carlo Simulations. Phys. Rev. Lett. 2006, 96, 070601. [Google Scholar] [CrossRef]
- Boninsegni, M.; Prokof’ev, N.V.; Svistunov, B.V. Worm algorithm and diagrammatic Monte Carlo: A new approach to continuous-space path integral Monte Carlo simulations. Phys. Rev. E 2006, 74, 036701. [Google Scholar] [CrossRef]
- Spada, G.; Giorgini, S.; Pilati, S. Path-integral Monte Carlo worm algorithm for Bose systems with periodic boundary conditions. Condens. Matter 2022, 7, 30. [Google Scholar] [CrossRef]
- Morresi, T.; Garberoglio, G. Normal liquid 3He studied by path-integral Monte Carlo with a parametrized partition function. Phys. Rev. B 2025, 111, 014521. [Google Scholar] [CrossRef]
- Hirshberg, B.; Rizzi, V.; Parrinello, M. Path integral molecular dynamics for bosons. Proc. Natl. Acad. Sci. USA 2019, 116, 21445. [Google Scholar] [CrossRef]
- Hirshberg, B.; Invernizzi, M.; Parrinello, M. Path Integral Molecular Dynamics for Fermions: Alleviating the Sign Problem with the Bogoliubov Inequality. J. Chem. Phys. 2020, 152, 171102. [Google Scholar] [CrossRef] [PubMed]
- Feldman, Y.M.Y.; Hirshberg, B. Quadratic Scaling Bosonic Path Integral Molecular Dynamics. J. Chem. Phys. 2023, 159, 154107. [Google Scholar] [CrossRef]
- Myung, C.W.; Hirshberg, B.; Parrinello, M. Prediction of a supersolid phase in high-pressure deuterium. Phys. Rev. Lett. 2022, 128, 045301. [Google Scholar] [CrossRef] [PubMed]
- Yu, Y.; Liu, S.; Xiong, H.; Xiong, Y. Path integral molecular dynamics for thermodynamics and Green’s function of ultracold spinor bosons. J. Chem. Phys. 2022, 157, 064110. [Google Scholar] [CrossRef]
- Xiong, Y.; Xiong, H. Path integral molecular dynamics simulations for Green’s function in a system of identical bosons. J. Chem. Phys. 2022, 156, 134112. [Google Scholar] [CrossRef]
- Xiong, Y.; Liu, S.; Xiong, H. Quadratic scaling path integral molecular dynamics for fictitious identical particles and its application to fermion systems. Phys. Rev. E 2024, 110, 065303. [Google Scholar] [CrossRef]
- Higer, J.; Feldman, Y.M.Y.; Hirshberg, B. Periodic Boundary Conditions for Bosonic Path Integral Molecular Dynamics. arXiv 2025, arXiv:2501.17618. [Google Scholar]
- Ceperley, D.M. Fermion nodes. J. Stat. Phys. 1991, 63, 1237. [Google Scholar] [CrossRef]
- Schoof, T.; Groth, S.; Vorberger, J.; Bonitz, M. Ab Initio Thermodynamic Results for the Degenerate Electron Gas at Finite Temperature. Phys. Rev. Lett. 2015, 115, 130402. [Google Scholar] [CrossRef]
- Ceperley, D.M. Path-integral calculations of normal liquid 3He. Phys. Rev. Lett. 1992, 69, 331. [Google Scholar] [CrossRef]
- Militzer, B.; Pollock, E.L.; Ceperley, D.M. Path integral Monte Carlo calculation of the momentum distribution of the homogeneous electron gas at finite temperature. High Energy Dens. Phys. 2019, 30, 13. [Google Scholar] [CrossRef]
- Mak, C.H.; Egger, R.; Weber-Gottschick, H. Multilevel blocking approach to the fermion sign problem in path-integral Monte Carlo simulations. Phys. Rev. Lett. 1998, 81, 4533. [Google Scholar] [CrossRef]
- Blunt, N.S.; Rogers, T.W.; Spencer, J.S.; Foulkes, W.M. Density-matrix quantum Monte Carlo method. Phys. Rev. B 2014, 89, 245124. [Google Scholar] [CrossRef]
- Schoof, T.; Bonitz, M.; Filinov, A.V.; Hochstuhl, D.; Dufty, J.W. Configuration Path Integral Monte Carlo. Contrib. Plasma Phys. 2011, 51, 687. [Google Scholar] [CrossRef]
- Groth, S.; Dornheim, T.; Sjostrom, T.; Malone, F.D.; Foulkes, W.M.C.; Bonitz, M. Ab initio exchange-correlation free energy of the uniform electron gas at warm dense matter conditions. Phys. Rev. Lett. 2017, 119, 135001. [Google Scholar] [CrossRef] [PubMed]
- Carlson, J.; Gandolfi, S.; Schmidt, K.E.; Zhang, S. Auxiliary-field quantum Monte Carlo method for strongly paired fermions. Phys. Rev. A 2011, 84, 061602. [Google Scholar] [CrossRef]
- Qin, M.; Shi, H.; Zhang, S. Benchmark study of the two-dimensional Hubbard model with auxiliary-field quantum Monte Carlo method. Phys. Rev. B 2016, 94, 085103. [Google Scholar] [CrossRef]
- Prokof’ev, N.V.; Svistunov, B.V. Bold diagrammatic Monte Carlo technique: When the sign problem is welcome. Phys. Rev. Lett. 2007, 99, 250201. [Google Scholar] [CrossRef]
- Hou, P.C.; Wang, B.Z.; Haule, K.; Deng, Y.; Chen, K. Exchange-correlation effect in the charge response of a warm dense electron gas. Phys. Rev. B 2022, 106, L081126. [Google Scholar] [CrossRef]
- Xiong, Y.; Xiong, H. On the thermodynamic properties of fictitious identical particles and the application to fermion sign problem. J. Chem. Phys. 2022, 157, 094112. [Google Scholar] [CrossRef] [PubMed]
- Xiong, Y.; Xiong, H. On the thermodynamics of fermions at any temperature based on parametrized partition function. Phys. Rev. E 2023, 107, 055308. [Google Scholar] [CrossRef]
- Dornheim, T.; Tolias, P.; Groth, S.; Moldabekov, Z.A.; Vorberger, J.; Hirshberg, B. Fermionic physics from ab initio path integral Monte Carlo simulations of fictitious identical particles. J. Chem. Phys. 2023, 159, 164113. [Google Scholar] [CrossRef]
- Dornheim, T.; Schwalbe, S.; Moldabekov, Z.A.; Vorberger, J.; Tolias, P. Ab initio path integral Monte Carlo simulations of the uniform electron gas on large length scales. J. Phys. Chem. Lett. 2024, 15, 1305. [Google Scholar] [CrossRef]
- Dornheim, T.; Döppner, T.; Tolias, P.; Böhme, M.; Fletcher, L.; Gawne, T.; Graziani, F.; Kraus, D.; MacDonald, M.; Moldabekov, Z.; et al. Unraveling electronic correlations in warm dense quantum plasmas. arXiv 2024, arXiv:2402.19113. [Google Scholar]
- Dornheim, T.; Schwalbe, S.; Böhme, M.P.; Moldabekov, Z.A.; Vorberger, J.; Tolias, P. Ab initio path integral Monte Carlo simulations of warm dense two-component systems without fixed nodes: Structural properties. J. Chem. Phys. 2024, 160, 164111. [Google Scholar] [CrossRef]
- Dornheim, T.; Schwalbe, S.; Tolias, P.; Moldabekov, M.P.B.Z.A.; Vorberger, J. Ab initio Density Response and Local Field Factor of Warm Dense Hydrogen. Matter Radiat. Extremes 2024, 9, 057401. [Google Scholar] [CrossRef]
- Xiong, Y.; Xiong, H. Ab initio simulation of the universal properties of unitary Fermi gas in a harmonic trap. arXiv 2024, arXiv:2403.02961. [Google Scholar]
- Nosé, S. A molecular dynamics method for simulations in the canonical ensemble. Mol. Phys. 1984, 52, 255. [Google Scholar] [CrossRef]
- Nosé, S. A unified formulation of the constant temperature molecular dynamics methods. J. Chem. Phys. 1984, 81, 511. [Google Scholar] [CrossRef]
- Hoover, W.G. Canonical dynamics: Equilibrium phase-space distributions. Phys. Rev. A 1985, 31, 1695. [Google Scholar] [CrossRef] [PubMed]
- Martyna, G.J.; Klein, M.L.; Tuckerman, M. Nosé-Hoover chains: The canonical ensemble via continuous dynamics. J. Chem. Phys. 1992, 97, 2635. [Google Scholar] [CrossRef]
- Jang, S.; Voth, G.A. Simple reversible molecular dynamics algorithms for Nosé-Hoover chain dynamics. J. Chem. Phys. 1997, 107, 9514. [Google Scholar] [CrossRef]
- Xiong, Y.; Xiong, H. Ab initio simulations of the thermodynamic properties and phase transition of Fermi systems based on fictitious identical particles and physics-informed neural networks. arXiv 2024, arXiv:2402.07231. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, B.; Yu, H.; Liu, S.; Zhu, F. Density Distribution of Strongly Quantum Degenerate Fermi Systems Simulated by Fictitious Identical Particle Thermodynamics. Entropy 2025, 27, 458. https://doi.org/10.3390/e27050458
Yang B, Yu H, Liu S, Zhu F. Density Distribution of Strongly Quantum Degenerate Fermi Systems Simulated by Fictitious Identical Particle Thermodynamics. Entropy. 2025; 27(5):458. https://doi.org/10.3390/e27050458
Chicago/Turabian StyleYang, Bo, Hongsheng Yu, Shujuan Liu, and Fengzheng Zhu. 2025. "Density Distribution of Strongly Quantum Degenerate Fermi Systems Simulated by Fictitious Identical Particle Thermodynamics" Entropy 27, no. 5: 458. https://doi.org/10.3390/e27050458
APA StyleYang, B., Yu, H., Liu, S., & Zhu, F. (2025). Density Distribution of Strongly Quantum Degenerate Fermi Systems Simulated by Fictitious Identical Particle Thermodynamics. Entropy, 27(5), 458. https://doi.org/10.3390/e27050458



