The Consistency Factor and the Viscosity Exponent of Soybean-Protein-Isolate/Wheat-Gluten/Corn-Starch Blends by Using a Capillary Rheometry
Abstract
:1. Introduction
2. Results
2.1. DSC of Soybean-Protein-Isolate, Corn-Starch, and Wheat-Gluten
2.2. Rheological Characterization of Soybean-Protein-Isolate/Corn-Starch Blends
2.3. Rheological Characterization of Soybean-Protein-Isolate/Wheat-Gluten Blends
2.4. Rheological Characterization of WG/CS Blends
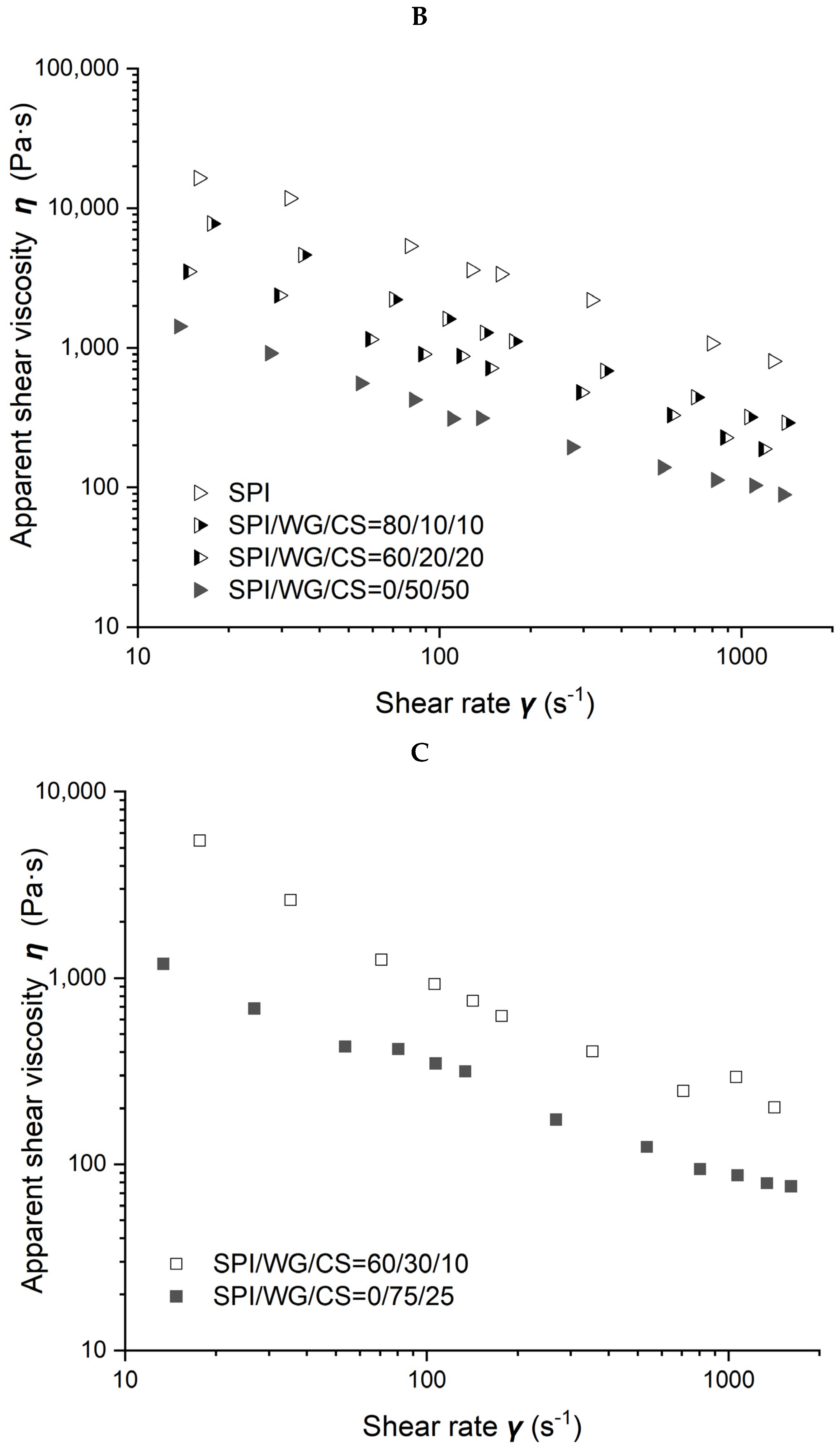
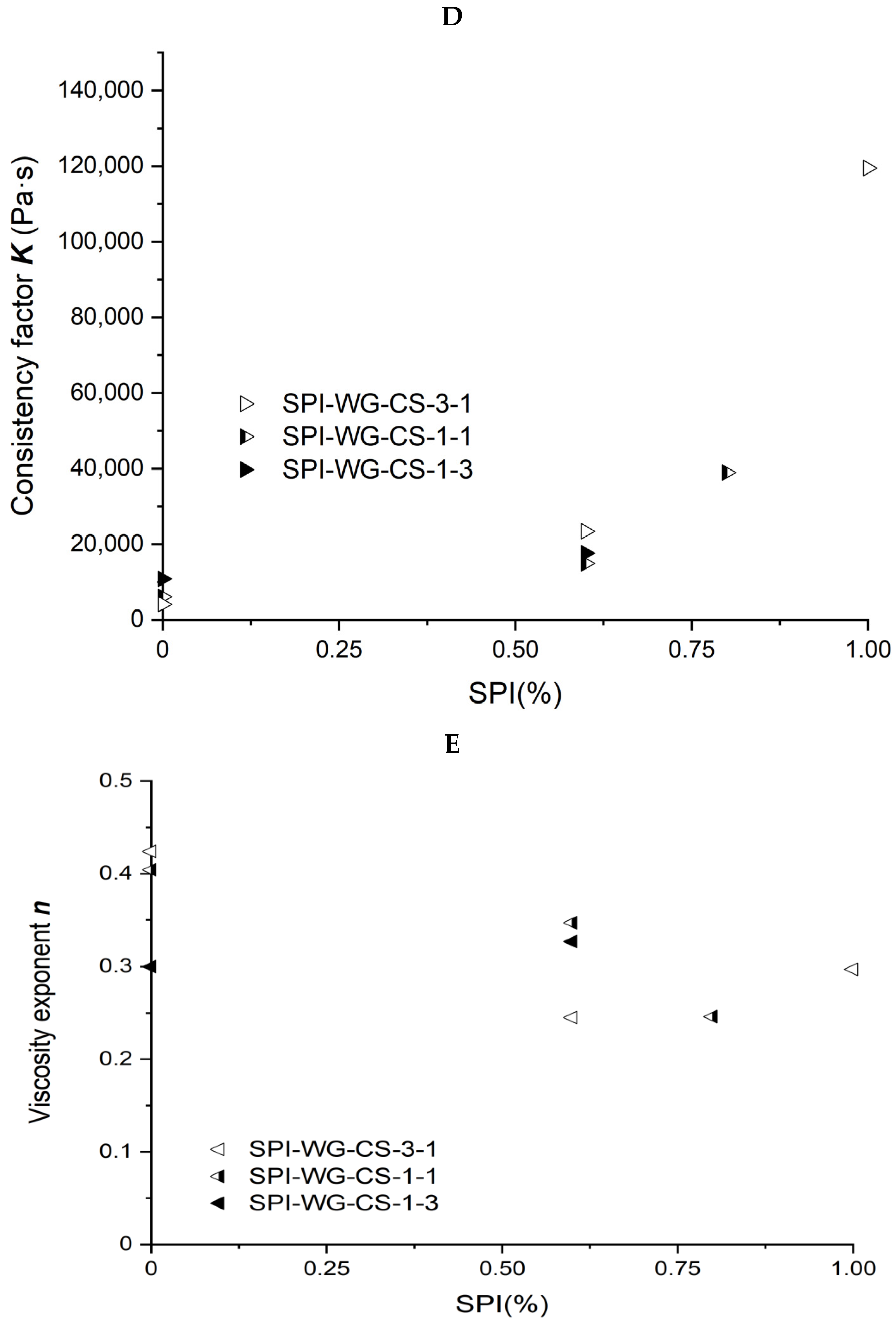
2.5. Rheological Characterization of the Blends of SPI/WG/CS
2.6. Fitted Equations between Mass Fraction and nno-inter or Kno-inter of a Non-Interactive Model
3. Discussion
4. Materials and Methods
4.1. Materials
4.2. Differential Scanning Calorimetry
4.3. Samples Preparation
4.4. Rheology Measurements
4.5. A Non-Interactive Model
4.6. Statistical Analysis
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Sample Availability
References
- Zhang, B.; Zhang, J.C.; Zhang, W.; Wei, Y.M. The effect of raw physicochemical properties on the quality of extruded texturized vegetable protein. J. Chin. Inst. Food Sci. Technol. 2017, 17, 155–163. [Google Scholar]
- Murillo, J.L.S.; Osen, R.; Hiermaier, S.; Ganzenmüller, G. Towards understanding the mechanism of fibrous texture formation during high-moisture extrusion of meat substitutes. J. Food Eng. 2019, 242, 8–20. [Google Scholar] [CrossRef]
- Michaeli, W. Extrusion Dies for Plastics and Rubber: Design and Engineering Computations; Hanser Publications: Cincinnati, OH, USA, 2016; pp. 12–60. [Google Scholar]
- Li, L.W.; Jin, F.; He, W.D.; Wu, C. How do polymer chains with different topologies pass through a cylindrical pore under an elongational flow field? Acta Polym. Sin. 2014, 1, 1–21. [Google Scholar]
- Luxenburg, L.A.; Baird, D.G.; Joseph, E.G. Background studies in the modeling of extrusion cooking processes for soy flour doughs. Biotechnol. Prog. 1985, 1, 33–38. [Google Scholar] [CrossRef]
- Li, P.X.; Campanella, O.H.; Hardacre, A.K. Using an in-line slit-die viscometer to study the effects of extrusion parameters on corn melt rheology. Cereal Chem. 2004, 81, 70–76. [Google Scholar] [CrossRef]
- Chen, Q.; Zhang, J.; Zhang, Y.; Meng, S.; Wang, Q. Rheological properties of pea protein isolate-amylose/amylopectin mixtures and the application in the high-moisture extruded meat substitutes. Food Hydrocoll. 2021, 117, 106732. [Google Scholar] [CrossRef]
- Ravindra, P.; Genovese, D.B.; Foegeding, E.A.; Rao, M.A. Rheology of heated mixed whey protein isolate/cross-linked waxy maize starch dispersions. Food Hydrocoll. 2004, 18, 775–781. [Google Scholar] [CrossRef]
- Willett, J.L.; Millard, M.M.; Jasberg, B.K. Extrusion of waxy maize starch: Melt rheology and molecular weight degradation of amylopectin. Polymer 1997, 38, 5983–5989. [Google Scholar] [CrossRef]
- Ralston, B.E.; Osswald, T.A. Viscosity of soy protein plastics determined by screw-driven capillary rheometry. J. Polym. Environ. 2008, 16, 169–176. [Google Scholar] [CrossRef]
- Roccia, P.; Ribotta, P.D.; Pérez, G.T.; León, A.E. Influence of soy protein on rheological properties and water retention capacity of wheat gluten. LWT Food Sci. Technol. 2009, 42, 358–362. [Google Scholar] [CrossRef]
- Chanvrier, H.; Uthayakumaran, S.; Lillford, P. Rheological properties of wheat flour processed at low levels of hydration: Influence of starch and gluten. J. Cereal Sci. 2007, 45, 263–274. [Google Scholar] [CrossRef]
- Attenburrow, G.; Barnes, D.J.; Davies, A.P.; Ingman, S.J. Rheological properties of wheat gluten. J. Cereal Sci. 1990, 12, 1–14. [Google Scholar] [CrossRef]
- Rao, M.A. Rheological Behavior of Processed Fluid and Semisolid Foods. In Rheology of Fluid and Semisolid Foods: Principles and Applications; Springer: Boston, MA, USA, 2007; pp. 223–357. [Google Scholar]
- Shim, J.; Mulvaney, S.J. Effect of heating temperature, pH, concentration and starch/whey protein ratio on the viscoelastic properties of corn starch/whey protein mixed gels. J. Sci. Food Agric. 2001, 81, 706–717. [Google Scholar] [CrossRef]
- Li, S.; Wei, Y.; Fang, Y.; Zhang, W.; Zhang, B. DSC study on the thermal properties of soybean protein isolates/corn starch mixture. J. Therm. Anal. Calorim. 2014, 115, 1633–1638. [Google Scholar] [CrossRef]
- Nishinari, K.; Fang, Y.; Guo, S.; Phillips, G.O. Soy proteins: A review on composition, aggregation and emulsification. Food Hydrocoll. 2014, 39, 301–318. [Google Scholar] [CrossRef]
- Amend, T.; Belitz, H.-D. Microscopical studies of water/flour systems. Z. Lebensm.-Unters. Forsch. 1989, 189, 103–109. [Google Scholar] [CrossRef]
- Zhang, H.-H.; Li, Q.; Claver, I.P.; Zhu, K.-X.; Peng, W.; Zhou, H.-M. Effect of cysteine on structural, rheological properties and solubility of wheat gluten by enzymatic hydrolysis. Int. J. Food Sci. Technol. 2010, 45, 2155–2161. [Google Scholar] [CrossRef]
- Wieser, H. Chemistry of gluten proteins. Food Microbiol. 2007, 24, 115–119. [Google Scholar] [CrossRef] [PubMed]
- Lindsay, M.P.; Skerritt, J.H. Immunocytochemical Localization of Gluten Proteins Uncovers Structural Organization of Glutenin Macropolymer. Cereal Chem. 2000, 77, 360–369. [Google Scholar] [CrossRef]
- Moore, M.M.; Schober, T.J.; Dockery, P.; Arendt, E.K. Textural comparisons of gluten-free and wheat-based doughs, batters, and breads. Cereal Chem. 2004, 81, 567–575. [Google Scholar] [CrossRef]
- Purna, S.K.G.; Miller, R.A.; Seib, P.A.; Graybosch, R.A.; Shi, Y.-C. Volume, texture, and molecular mechanism behind the collapse of bread made with different levels of hard waxy wheat flours. J. Cereal Sci. 2011, 54, 37–43. [Google Scholar] [CrossRef] [Green Version]
- Bengoechea, C.; Arrachid, A.; Guerrero, A.; Hill, S.E.; Mitchell, J.R. Relationship between the glass transition temperature and the melt flow behavior for gluten, casein and soya. J. Cereal Sci. 2007, 45, 275–284. [Google Scholar] [CrossRef]
- Basiak, E.; Galus, S.; Lenart, A. Characterisation of composite edible films based on wheat starch and whey-protein isolate. Int. J. Food Sci. Technol. 2015, 50, 372–380. [Google Scholar] [CrossRef]
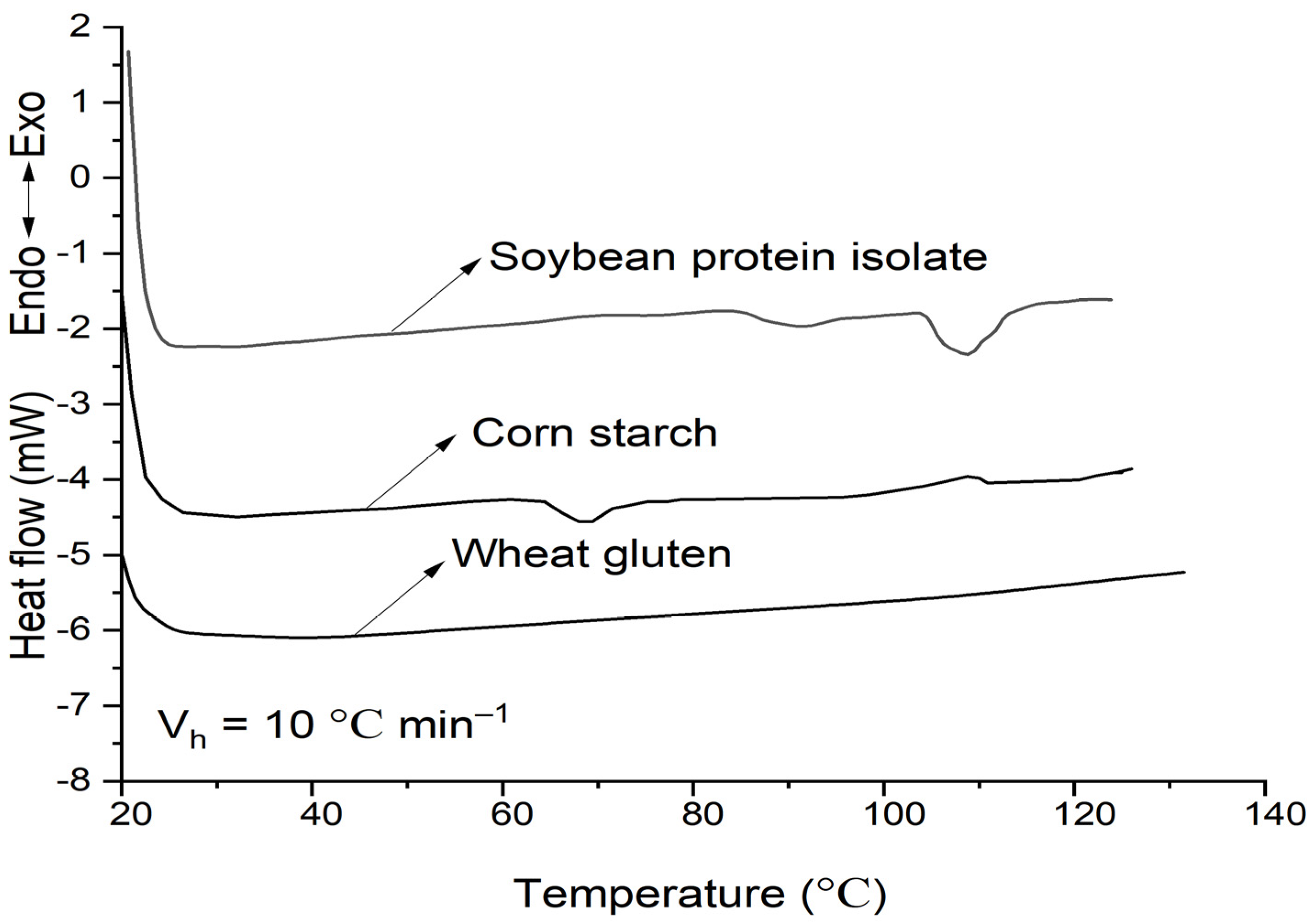

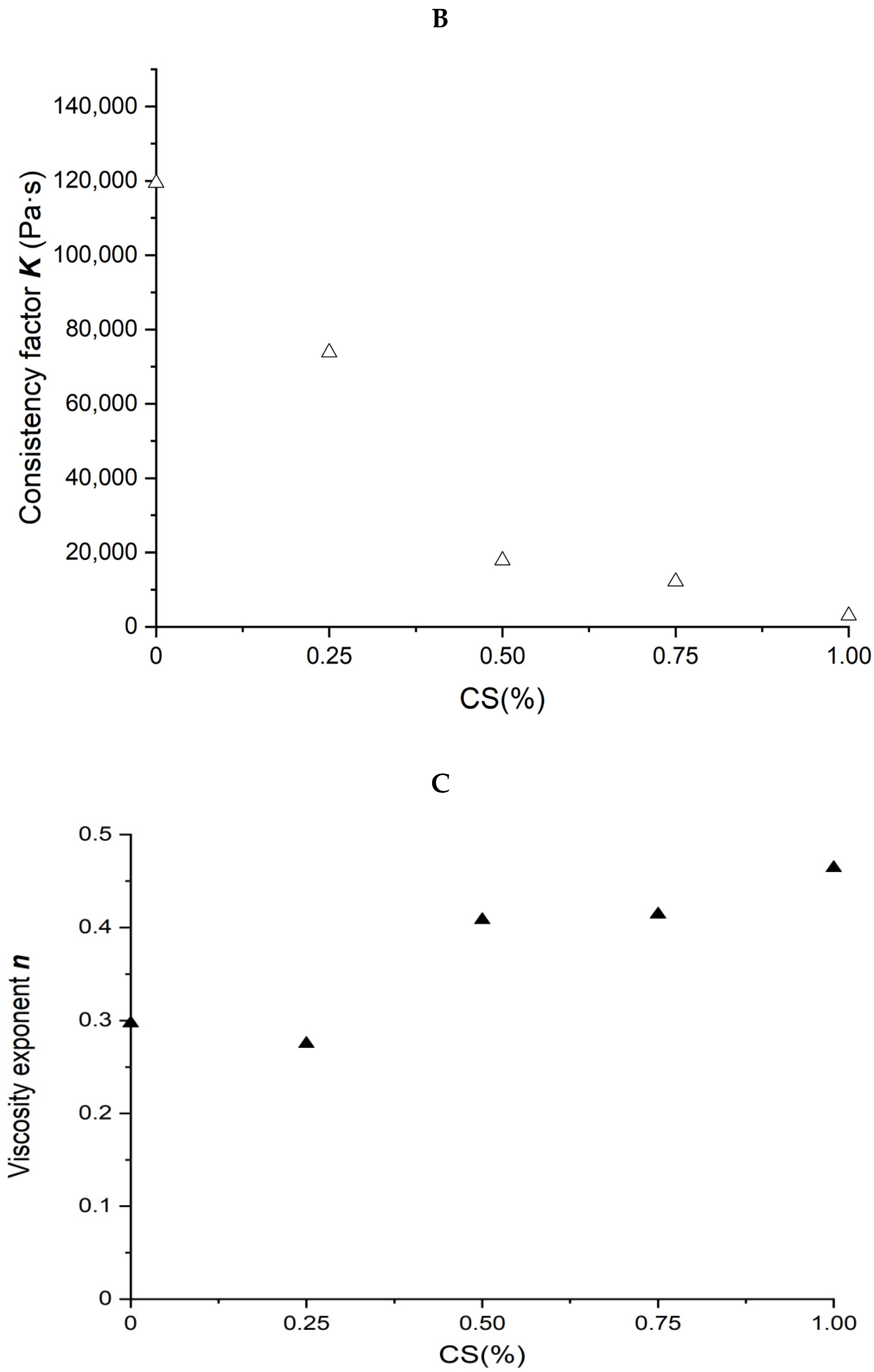


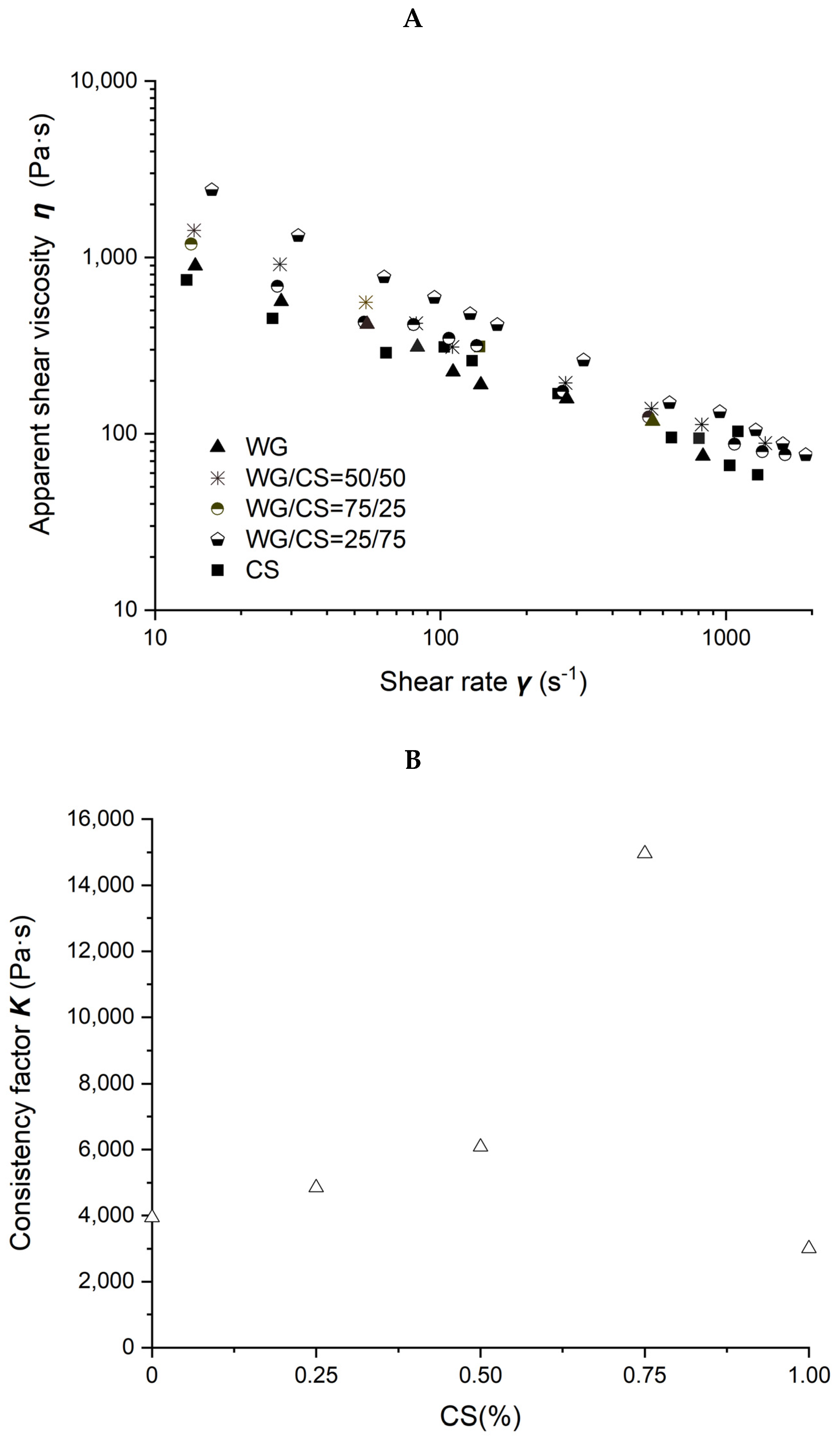
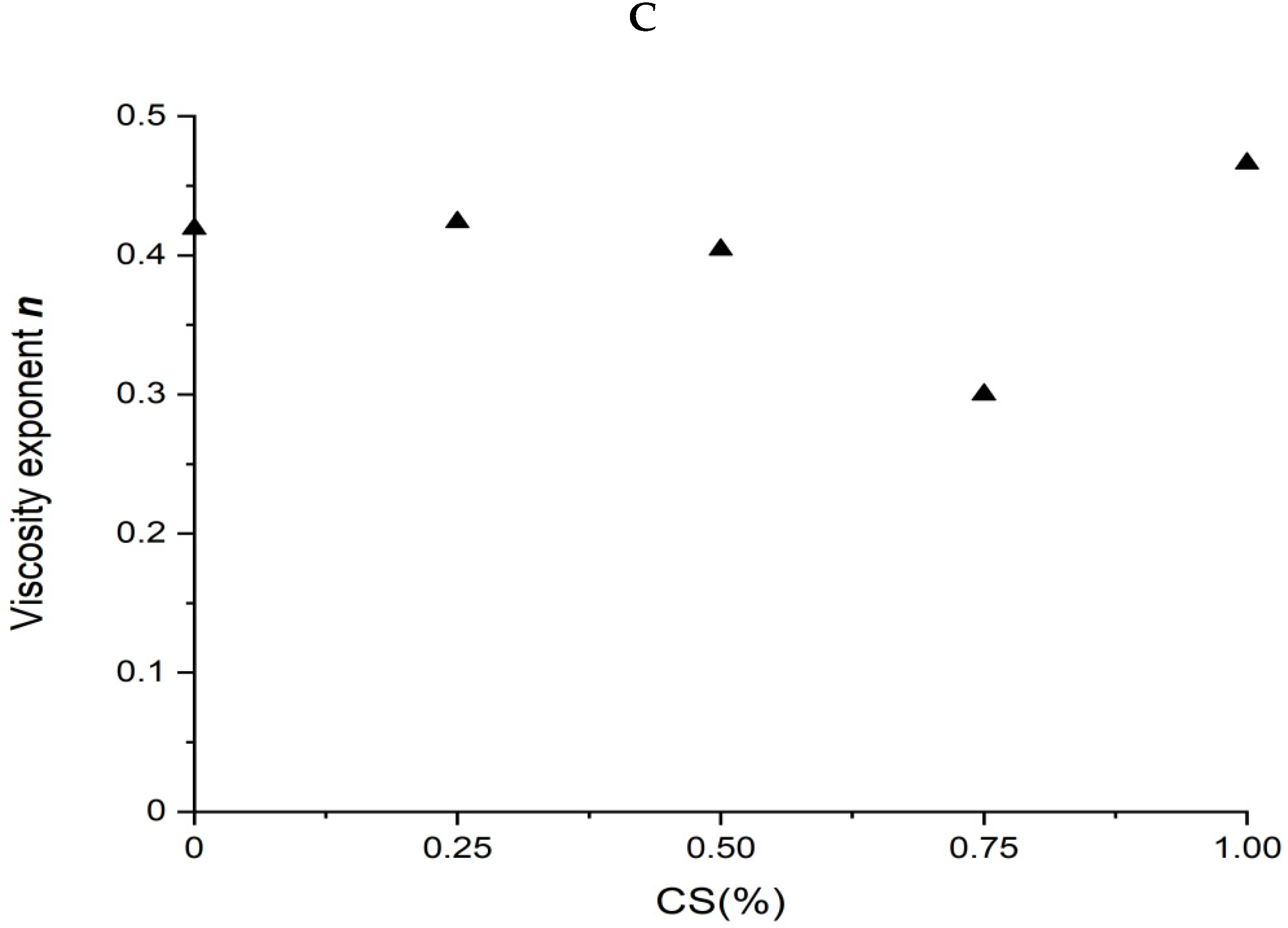

| Materials | To/°C | Tp/°C | ΔH/J g−1 |
|---|---|---|---|
| SPI | 103.11 ± 0.92 | 108.25 ± 0.86 | 4.45 ± 0.37 |
| CS | 63.54 ± 0.61 | 68.56 ± 0.40 | 3.62 ± 0.61 |
| WG | -- | -- | -- |
| Samples | X | Linear Fitting Equation | R2 | Exponential Fitting Equation | R2 | Power Function Fitting Equation | Logarithm Fitting Equation |
|---|---|---|---|---|---|---|---|
| SPI/CS | CS% | n = 0.1424x + 0.2666 | 0.625 | n = 0.2744e0.2852x | 0.652 | \ | \ |
| SPI/WG | WG% | n = 0.1044x + 0.2748 | 0.631 | n = 0.2793e0.2974x | 0.652 | \ | \ |
| WG/CS | CS% | n = 0.0464x + 0.4184 | 0.997 | n = 0.4187e0.105x | 0.999 | \ | \ |
| SPI-WG/CS = 1/3 | SPI% | n = −0.1625x + 0.437 | 0.862 | n = 0.4344e−0.440x | 0.867 | \ | \ |
| SPI-WG/CS = 1/1 | SPI% | n = −0.1536x + 0.4271 | 0.881 | n = 0.4249e−0.421x | 0.885 | \ | \ |
| SPI-WG/CS = 3/1 | SPI% | n = −0.1393x + 0.4173 | 0.866 | n = 0.4154e−0.387x | 0.870 | \ | \ |
| Samples | X | Linear Fitting Equation | R2 | Exponential Fitting Equation | R2 | Power Function Fitting Equation | Logarithm Fitting Equation |
|---|---|---|---|---|---|---|---|
| SPI/CS | CS% | K = −116720x + 119148 | 0.9999 | K = 194371e−3.371x | 0.813 | \ | \ |
| SPI/WG | WG% | K = −115708x + 119287 | 1.0000 | K = 184951e−3.143x | 0.828 | \ | \ |
| WG/CS | CS% | K = −934.88x + 3928.5 | 0.9993 | K = 3945e−0.271x | 1.000 | \ | \ |
| SPI-WG/CS = 1/1 | SPI% | K = 115934x + 3309.6 | 1.0000 | K = 4419.1e3.7195x | 0.931 | \ | \ |
| SPI-WG/CS = 1/3 | SPI% | K = 116226x + 3132.7 | 1.0000 | K = 4133.8e3.7382x | 0.923 | \ | \ |
| SPI-WG/CS = 3/1 | SPI% | K = 115736x + 3556.7 | 1.000 | K = 4667.1e3.5954x | 0.926 | \ | \ |
| Samples | SPI(%) | WG(%) | CS(%) |
|---|---|---|---|
| 1 | 100 | 0 | 0 |
| 2 | 0 | 100 | 0 |
| 3 | 0 | 0 | 100 |
| 4 | 75 | 25 | 0 |
| 5 | 50 | 50 | 0 |
| 6 | 25 | 75 | 0 |
| 7 | 75 | 0 | 25 |
| 8 | 50 | 0 | 50 |
| 9 | 25 | 0 | 75 |
| 10 | 0 | 75 | 25 |
| 11 | 0 | 50 | 50 |
| 12 | 0 | 25 | 75 |
| 13 | 60 | 10 | 30 |
| 14 | 60 | 20 | 20 |
| 15 | 60 | 30 | 10 |
| 16 | 80 | 10 | 10 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, W.; Zhao, D.; Dong, Z.; Li, J.; Zhang, B.; Yu, W. The Consistency Factor and the Viscosity Exponent of Soybean-Protein-Isolate/Wheat-Gluten/Corn-Starch Blends by Using a Capillary Rheometry. Molecules 2022, 27, 6693. https://doi.org/10.3390/molecules27196693
Zhang W, Zhao D, Dong Z, Li J, Zhang B, Yu W. The Consistency Factor and the Viscosity Exponent of Soybean-Protein-Isolate/Wheat-Gluten/Corn-Starch Blends by Using a Capillary Rheometry. Molecules. 2022; 27(19):6693. https://doi.org/10.3390/molecules27196693
Chicago/Turabian StyleZhang, Wei, Donglin Zhao, Ziyan Dong, Jian Li, Bo Zhang, and Wenhua Yu. 2022. "The Consistency Factor and the Viscosity Exponent of Soybean-Protein-Isolate/Wheat-Gluten/Corn-Starch Blends by Using a Capillary Rheometry" Molecules 27, no. 19: 6693. https://doi.org/10.3390/molecules27196693
APA StyleZhang, W., Zhao, D., Dong, Z., Li, J., Zhang, B., & Yu, W. (2022). The Consistency Factor and the Viscosity Exponent of Soybean-Protein-Isolate/Wheat-Gluten/Corn-Starch Blends by Using a Capillary Rheometry. Molecules, 27(19), 6693. https://doi.org/10.3390/molecules27196693








