Detecting the Hydrogen Bond Cooperativity in a Protein β-Sheet by H/D Exchange
Abstract
1. Introduction
2. Results
2.1. H/D Exchange Process in β-Sheet
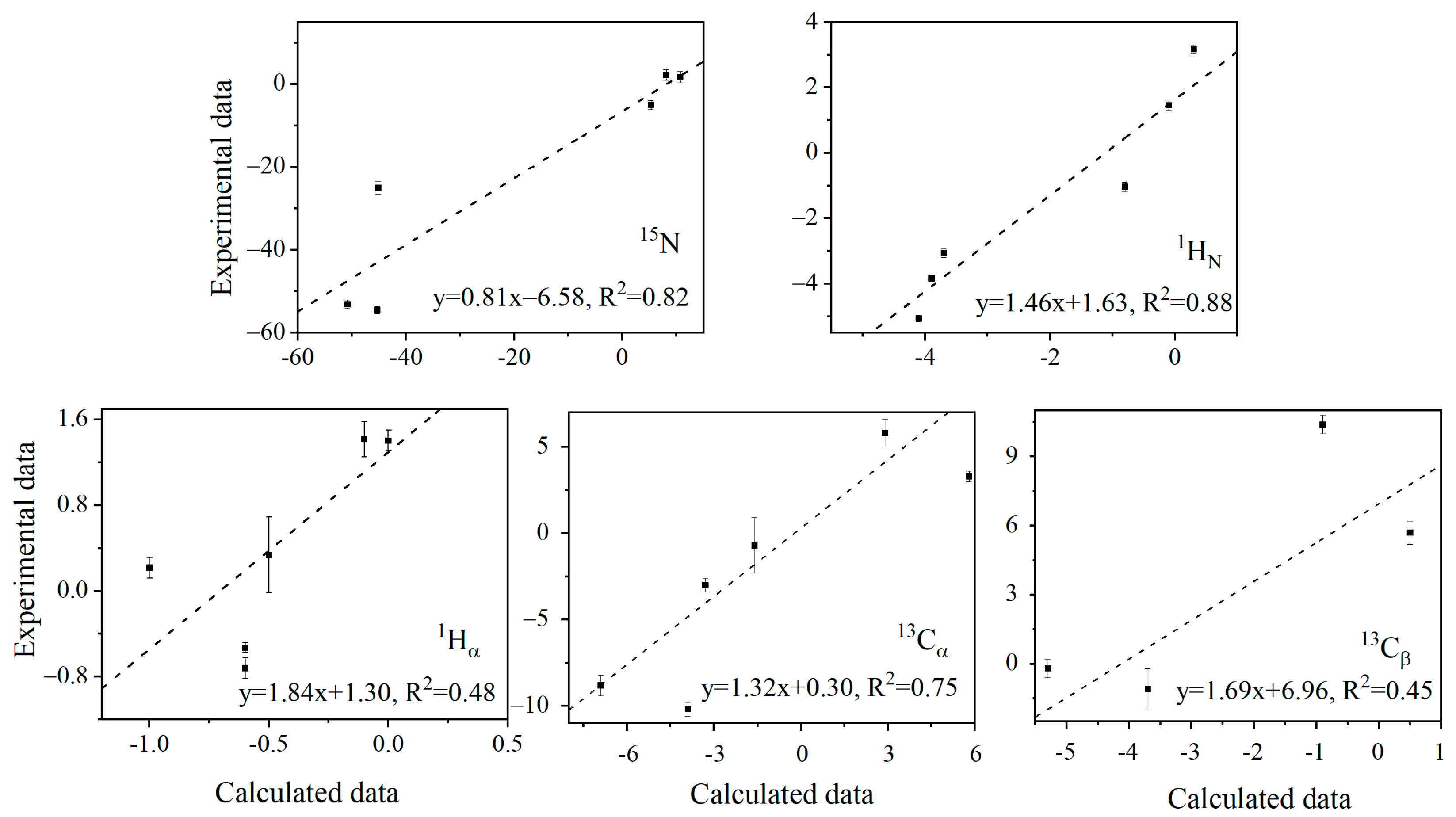
2.2. The Isotope Effect on Chemical Shifts Obtained from the NMR H/D Exchange Measurement
3. Discussion
4. Materials and Methods
4.1. NMR Spectroscopy and Exchange Rate Fitting
4.2. ONIOM Calculations
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Huma, Z.E.; Ludeman, J.P.; Wilkinson, B.L.; Payne, R.J.; Stone, M.J. NMR characterization of cooperativity: Fast ligand binding coupled to slow protein dimerization. Chem. Sci. 2014, 5, 2783–2788. [Google Scholar] [CrossRef]
- Dellarole, M.; Caro, J.A.; Roche, J.; Fossat, M.; Barthe, P.; Garcia-Moreno, E.B.; Royer, C.A.; Roumestand, C. Evolutionarily Conserved Pattern of Interactions in a Protein Revealed by Local Thermal Expansion Properties. J. Am. Chem. Soc. 2015, 137, 9354–9362. [Google Scholar] [CrossRef] [PubMed]
- Kung, V.M.; Cornilescu, G.; Gellman, S.H. Impact of Strand Number on Parallel beta-Sheet Stability. Angew. Chem. Int. Ed. Engl. 2015, 54, 14336–14339. [Google Scholar] [CrossRef] [PubMed]
- Li, G.C.; Srivastava, A.K.; Kim, J.; Taylor, S.S.; Veglia, G. Mapping the Hydrogen Bond Networks in the Catalytic Subunit of Protein Kinase A Using H/D Fractionation Factors. Biochemistry 2015, 54, 4042–4049. [Google Scholar] [CrossRef]
- Wang, Y.; Manu, V.S.; Kim, J.; Li, G.; Ahuja, L.G.; Aoto, P.; Taylor, S.S.; Veglia, G. Globally correlated conformational entropy underlies positive and negative cooperativity in a kinase’s enzymatic cycle. Nat. Commun. 2019, 10, 799. [Google Scholar] [CrossRef]
- Nisius, L.; Grzesiek, S. Key stabilizing elements of protein structure identified through pressure and temperature perturbation of its hydrogen bond network. Nat. Chem. 2012, 4, 711–717. [Google Scholar] [CrossRef]
- Zhou, S.; Wang, L. Unraveling the structural and chemical features of biological short hydrogen bonds. Chem. Sci. 2019, 10, 7734–7745. [Google Scholar] [CrossRef]
- Wieczorek, R.; Dannenberg, J.J. H-bonding cooperativity and energetics of alpha-helix formation of five 17-amino acid peptides. J. Am. Chem. Soc. 2003, 125, 8124–8129. [Google Scholar] [CrossRef]
- Morozov, A.V.; Kortemme, T.; Tsemekhman, K.; Baker, D. Close agreement between the orientation dependence of hydrogen bonds observed in protein structures and quantum mechanical calculations. Proc. Natl. Acad. Sci. USA 2004, 101, 6946–6951. [Google Scholar] [CrossRef]
- Li, J.; Wang, Y.; Chen, J.; Liu, Z.; Bax, A.; Yao, L. Observation of alpha-Helical Hydrogen-Bond Cooperativity in an Intact Protein. J. Am. Chem. Soc. 2016, 138, 1824–1827. [Google Scholar] [CrossRef]
- Zhao, Y.L.; Wu, Y.D. A theoretical study of beta-sheet models: Is the formation of hydrogen-bond networks cooperative? J. Am. Chem. Soc. 2002, 124, 1570–1571. [Google Scholar] [CrossRef]
- Rossmeisl, J.; Norskov, J.K.; Jacobsen, K.W. Elastic effects behind cooperative bonding in beta-sheets. J. Am. Chem. Soc. 2004, 126, 13140–13143. [Google Scholar] [CrossRef] [PubMed]
- Viswanathan, R.; Asensio, A.; Dannenberg, J.J. Cooperative hydrogen-bonding in models of antiparallel beta-sheets. J. Phys. Chem. A 2004, 108, 9205–9212. [Google Scholar] [CrossRef]
- Li, Y.; Wang, X.; Ren, L.; Cao, X.; Ji, C.; Xia, F.; Zhang, J.Z. Electrostatic Polarization Effect on Cooperative Aggregation of Full Length Human Islet Amyloid. J. Chem. Inf. Model. 2018, 58, 1587–1595. [Google Scholar] [CrossRef] [PubMed]
- Ruggeri, F.S.; Longo, G.; Faggiano, S.; Lipiec, E.; Pastore, A.; Dietler, G. Infrared nanospectroscopy characterization of oligomeric and fibrillar aggregates during amyloid formation. Nat. Commun. 2015, 6, 7831. [Google Scholar] [CrossRef]
- Ganesan, M.; Paranthaman, S. Studies on the structure and conformational flexibility of secondary structures in amyloid beta—A quantum chemical study. J. Theor. Comput. Chem. 2020, 19, 2050014. [Google Scholar] [CrossRef]
- Zhou, M.; Wen, H.; Lei, H.; Zhang, T. Molecular dynamics study of conformation transition from helix to sheet of A beta 42 peptide. J. Mol. Graph. Model. 2021, 109, 108027. [Google Scholar] [CrossRef]
- Han, S.; Cao, S.; Wang, Y.; Wang, J.; Xia, D.; Xu, H.; Zhao, X.; Lu, J.R. Self-Assembly of Short Peptide Amphiphiles: The Cooperative Effect of Hydrophobic Interaction and Hydrogen Bonding. Chem. Eur. J. 2011, 17, 13095–13102. [Google Scholar] [CrossRef]
- Wang, M.; Wang, J.; Zhou, P.; Deng, J.; Zhao, Y.; Sun, Y.; Yang, W.; Wang, D.; Li, Z.; Hu, X.; et al. Nanoribbons self-assembled from short peptides demonstrate the formation of polar zippers between β-sheets. Nat. Commun. 2018, 9, 5118. [Google Scholar] [CrossRef]
- Mahadevi, A.S.; Sastry, G.N. Cooperativity in Noncovalent Interactions. Chem. Rev. 2016, 116, 2775–2825. [Google Scholar] [CrossRef]
- Zhou, Y.; Deng, G.; Zheng, Y.Z.; Xu, J.; Ashraf, H.; Yu, Z.W. Evidences for Cooperative Resonance-Assisted Hydrogen Bonds in Protein Secondary Structure Analogs. Sci. Rep. 2016, 6, 36932. [Google Scholar] [PubMed]
- Cordier, F.; Barfield, M.; Grzesiek, S. Direct observation of C-alpha-H-alpha center dot center dot center dot O=C hydrogen bonds in proteins by interresidue (h3)J(C alpha C′) scalar couplings. J. Am. Chem. Soc. 2003, 125, 15750–15751. [Google Scholar] [CrossRef] [PubMed]
- Vakonakis, I.; LiWang, A.C. Trans-hydrogen bond deuterium isotope effects of A: T base pairs in DNA. J. Biomol. NMR 2004, 29, 65–72. [Google Scholar] [PubMed]
- Vakonakis, I.; Salazar, M.; Kang, M.J.; Dunbar, K.R.; LiWang, A.C. Deuterium isotope effects and fractionation factors of hydrogen-bonded A: T base pairs of DNA. J. Biomol. NMR 2003, 25, 105–112. [Google Scholar] [CrossRef]
- Coman, D.; Russu, I.M. Probing hydrogen bonding in a DNA triple helix using protium-deuterium fractionation factors. J. Am. Chem. Soc. 2003, 125, 6626–6627. [Google Scholar] [CrossRef] [PubMed]
- Sass, H.-J.; Schmid, F.F.-F.; Grzesiek, S. Correlation of protein structure and dynamics to scalar couplings across hydrogen bonds. J. Am. Chem. Soc. 2007, 129, 5898–5903. [Google Scholar] [CrossRef]
- Manalo, M.N.; Perez, L.M.; LiWang, A. Hydrogen-bonding and pi-pi base-stacking interactions are coupled in DNA, as suggested by calculated and experimental trans-Hbond deuterium isotope shifts. J. Am. Chem. Soc. 2007, 129, 11298–11299. [Google Scholar]
- Hansen, P.E. Isotope effects on chemical shifts in the study of hydrogen bonded biological systems. Prog. Nucl. Magn. Reson. Spectrosc. 2020, 120–121, 109–117. [Google Scholar] [CrossRef]
- McKercher, M.A.; Wuttke, D.S. NMR Chemical Shift Mapping of SH2 Peptide Interactions. Methods Mol. Biol. 2017, 1555, 269–290. [Google Scholar]
- Dingley, A.J.; Cordier, F.; Grzesiek, S. An introduction to hydrogen bond scalar couplings. Concepts Magn. Reson. 2001, 13, 103–127. [Google Scholar] [CrossRef]
- Preimesberger, M.; Majumdar, A.; Rice, S.; Que, L.; Lecomte, J. Helix-Capping Histidines: Diversity of N-H···N Hydrogen Bond Strength Revealed by (2h)JNN Scalar Couplings. Biochemistry 2015, 54, 6896–6908. [Google Scholar] [CrossRef] [PubMed]
- Li, J.; Chen, J.; An, L.; Yuan, X.; Yao, L. Polyol and sugar osmolytes can shorten protein hydrogen bonds to modulate function. Commun. Biol. 2020, 3, 528–536. [Google Scholar] [CrossRef]
- Takeda, M.; Miyanoiri, Y.; Terauchi, T.; Yang, C.-J.; Kainosho, M. Use of H/D isotope effects to gather information about hydrogen bonding and hydrogen exchange rates. J. Magn. Reson. 2014, 241, 148–154. [Google Scholar] [CrossRef] [PubMed]
- Yu, B.; Pletka, C.C.; Iwahara, J. NMR Observation of Intermolecular Hydrogen Bonds between Protein Tyrosine Side-Chain OH and DNA Phosphate Groups. J. Phys. Chem. B 2020, 124, 1065–1070. [Google Scholar] [CrossRef] [PubMed]
- Pinney, M.M.; Natarajan, A.; Yabukarski, F.; Sanchez, D.M.; Liu, F.; Liang, R.; Doukov, T.; Schwans, J.P.; Martinez, T.J.; Herschlag, D. Structural Coupling Throughout the Active Site Hydrogen Bond Networks of Ketosteroid Isomerase and Photoactive Yellow Protein. J. Am. Chem. Soc. 2018, 140, 9827–9843. [Google Scholar] [CrossRef] [PubMed]
- Sigala, P.A.; Caaveiro, J.M.; Ringe, D.; Petsko, G.A.; Herschlag, D. Hydrogen bond coupling in the ketosteroid isomerase active site. Biochemistry 2009, 48, 6932–6939. [Google Scholar] [CrossRef][Green Version]
- Juranic, N.; Macura, S. Correlations among (1)J(NC′) and (h3)J(NC′) coupling constants in the hydrogen-bonding network of human ubiquitin. J. Am. Chem. Soc. 2001, 123, 4099–4100. [Google Scholar] [CrossRef] [PubMed]
- Bai, Y.; Milne, J.S.; Mayne, L.; Englander, S.W. Primary structure effects on peptide group hydrogen exchange. Proteins 1993, 17, 75–86. [Google Scholar] [CrossRef]
- Bai, Y.W.; Milne, J.S.; Mayne, L.; Englander, S.W. Protein Stability Parameters Measured by Hydrogen-Exchange. Proteins 1994, 20, 4–14. [Google Scholar] [CrossRef]
- Derrick, J.P.; Wigley, D.B. The third IgG-binding domain from streptococcal protein G. An analysis by X-ray crystallography of the structure alone and in a complex with Fab. J. Mol. Biol. 1994, 243, 906–918. [Google Scholar] [CrossRef]
- Englander, S.W.; Kallenbach, N.R. Hydrogen exchange and structural dynamics of proteins and nucleic acids. Q. Rev. Biophys. 1983, 16, 521–655. [Google Scholar] [CrossRef] [PubMed]
- Ubbelohde, A.R.; Gallagher, K.J. Acid-Base Effects in Hydrogen Bonds in Crystals. Acta Crystallogr. 1955, 8, 71–83. [Google Scholar] [CrossRef]
- Jaravine, V.A.; Cordier, F.; Grzesiek, S. Quantification of H/D isotope effects on protein hydrogen-bonds by (h3)J(NC′) and (1)J(NC′) couplings and peptide group N-15 and C-13′ chemical shifts. J. Biomol. NMR 2004, 29, 309–318. [Google Scholar] [CrossRef] [PubMed]
- Grzesiek, S.; Cordier, F.; Jaravine, V.; Barfield, M. Insights into biomolecular hydrogen bonds from hydrogen bond scalar couplings. Prog. Nucl. Magn. Reson. Spectrosc. 2004, 45, 275–300. [Google Scholar] [CrossRef]
- Krantz, B.A.; Srivastava, A.K.; Nauli, S.; Baker, D.; Sauer, R.T.; Sosnick, T.R. Understanding protein hydrogen bond formation with kinetic H/D amide isotope effects. Nat. Struct. Biol. 2002, 9, 458–463. [Google Scholar] [CrossRef]
- Wishart, D.S. Interpreting protein chemical shift data. Prog. Nucl. Magn. Reson. Spectrosc. 2011, 58, 62–87. [Google Scholar] [CrossRef]
- Chung, L.W.; Hirao, H.; Li, X.; Morokuma, K. The ONIOM method: Its foundation and applications to metalloenzymes and photobiology. Wires Comput. Mol. Sci. 2012, 2, 327–350. [Google Scholar] [CrossRef]
- Benedict, H.; Limbach, H.H.; Wehlan, M.; Fehlhammer, W.P.; Golubev, N.S.; Janoschek, R. Solid State15N NMR and Theoretical Studies of Primary and Secondary Geometric H/D Isotope Effects on Low-Barrier NHN-Hydrogen Bonds. J. Am. Chem. Soc. 1998, 120, 2939–2950. [Google Scholar] [CrossRef]
- Rossetti, G.; Magistrato, A.; Pastore, A.; Carloni, P. Hydrogen Bonding Cooperativity in polyQ beta-Sheets from First Principle Calculations. J. Chem. Theory. Comput. 2010, 6, 1777–1782. [Google Scholar] [CrossRef] [PubMed]
- Plumley, J.A.; Dannenberg, J.J. The Importance of Hydrogen Bonding between the Glutamine Side Chains to the Formation of Amyloid VQIVYK Parallel beta-Sheets: An ONIOM DFT/AM1 Study. J. Am. Chem. Soc. 2010, 132, 1758–1759. [Google Scholar] [CrossRef]
- Reed, A.E. Intermolecular Interactions from a Natural Bond Orbital, Donor-Acceptor Viewpoint. Chem. Rev. 1988, 88, 899–926. [Google Scholar] [CrossRef]
- Newberry, R.W.; Raines, R.T. A prevalent intraresidue hydrogen bond stabilizes proteins. Nat. Chem. Biol. 2016, 12, 1084–1088. [Google Scholar] [CrossRef] [PubMed]
- Mundlapati, V.R.; Imani, Z.; D’Mello, V.C.; Brenner, V.; Gloaguen, E.; Baltaze, J.P.; Robin, S.; Mons, M.; Aitken, D.J. N-H⋯X interactions stabilize intra-residue C5 hydrogen bonded conformations in heterocyclic α-amino acid derivatives. Chem. Sci. 2021, 12, 14826–14832. [Google Scholar] [CrossRef] [PubMed]
- Parker, L.L.; Houk, A.R.; Jensen, J.H. Cooperative hydrogen bonding effects are key determinants of backbone amide proton chemical shifts in proteins. J. Am. Chem. Soc. 2006, 128, 9863–9872. [Google Scholar] [CrossRef]
- Bouvignies, G.; Bernado, P.; Meier, S.; Cho, K.; Grzesiek, S.; Bruschweiler, R.; Blackledge, M. Identification of slow correlated motions in proteins using residual dipolar and hydrogen-bond scalar couplings. Proc. Natl. Acad. Sci. USA 2005, 102, 13885–13890. [Google Scholar] [CrossRef]
- Schenck, H.L.; Gellman, S.H. Use of a Designed Triple-Stranded Antiparallel beta-Sheet To Probe beta-Sheet Cooperativity in Aqueous Solution. J. Am. Chem. Soc. 1998, 120, 4869–4870. [Google Scholar] [CrossRef]
- Stanger, H.E.; Syud, F.A.; Espinosa, J.F.; Giriat, I.; Muir, T.; Gellman, S.H. Length-dependent stability and strand length limits in antiparallel beta-sheet secondary structure. Proc. Natl. Acad. Sci. USA 2001, 98, 12015–12020. [Google Scholar] [CrossRef]
- Syud, F.A.; Stanger, H.E.; Mortell, H.S.; Espinosa, J.F.; Fisk, J.D.; Fry, C.G.; Gellman, S.H. Influence of Strand Number on Antiparallel β-Sheet Stability in Designed Three- and Four-stranded β-Sheets. J. Magn. Reson. 2003, 326, 553–568. [Google Scholar]
- Fenwick, R.B.; Orellana, L.; Esteban-Martin, S.; Orozco, M.; Salvatella, X. Correlated motions are a fundamental property of beta-sheets. Nat. Commun. 2014, 5, 5070. [Google Scholar] [CrossRef]
- Yao, L.; Ying, J.; Bax, A. Improved accuracy of N-15-H-1 scalar and residual dipolar couplings from gradient-enhanced IPAP-HSQC experiments on protonated proteins. J. Biomol. NMR 2009, 43, 161–170. [Google Scholar] [CrossRef]
- Kay, E.L.; Keifer, P.A.; Saarinen, T. Pure absorption gradient enhanced heteronuclear single quantum correlation spectroscopy with improved sensitivity. J. Am. Chem. Soc. 1992, 114, 10663–10665. [Google Scholar] [CrossRef]
- Palmer, A.G.; Cavanagh, J.; Wright, P.E.; Rance, M. Sensitivity improvement in proton-detected two-dimensional heteronuclear correlation NMR spectroscopy. J. Magn. Reson. 1991, 93, 151–170. [Google Scholar] [CrossRef]
- Delaglio, F.; Grzesiek, S.; Vuister, G.W.; Zhu, G.; Pfeifer, J.; Bax, A. NMRPipe: A multidimensional spectral processing system based on UNIX pipes. J. Biomol. NMR 1995, 6, 277–293. [Google Scholar] [CrossRef] [PubMed]
- Dapprich, S.; Komáromi, I.; Byun, K.S.; Morokuma, K.; Frisch, M.J. A new ONIOM implementation in Gaussian98. Part I. The calculation of energies, gradients, vibrational frequencies and electric field derivatives. J. Mol. Struct. Theochem. 1999, 461–462, 1–21. [Google Scholar] [CrossRef]
- Malloum, A.; Fifen, J.J.; Dhaouadi, Z.; Engo, S.G.N.; Jaidane, N.-E. Structures and relative stabilities of ammonia clusters at different temperatures: DFT vs. ab initio. Phys. Chem. Chem. Phys. 2015, 17, 29226–29242. [Google Scholar] [CrossRef]
- Moon, S.; Case, D.A. A new model for chemical shifts of amide hydrogens in proteins. J. Biomol. NMR 2007, 38, 139–150. [Google Scholar] [CrossRef]
- Case, D.A. Calculations of NMR dipolar coupling strengths in model peptides. J. Biomol. NMR 1999, 15, 95–102. [Google Scholar] [CrossRef]
- Moon, S.; Case, D.A. A comparison of quantum chemical models for calculating NMR shielding parameters in peptides: Mixed basis set and ONIOM methods combined with a complete basis set extrapolation. J. Comput. Chem. 2006, 27, 825–836. [Google Scholar] [CrossRef]
- Frisch M., J.; Trucks G., W.; Schlegel H., B.; Scuseria G., E.; Robb M., A.; Cheeseman J., R.; Scalmani, G.; Barone, V.; Petersson G., A.; Nakatsuji, H.; et al. Gaussian 09; Gaussian, Inc.: Oxfordshire, UK.



| N–H (Å) | N–D (Å) | |
|---|---|---|
| Y3 | 1.03398 | 1.02978 |
| L5 | 1.03754 | 1.03324 |
| I7 | 1.03473 | 1.03038 |
| Exchange Site | ∆φia (°) | ∆ψi (°) | ∆φi+1 (°) | ∆ψi+1 (°) |
|---|---|---|---|---|
| Y3→T18 | −0.017 | −0.026 | 0.044 | 0.008 |
| L5→T16 | −0.018 | −0.026 | 0.026 | 0.001 |
| I7→G14 | −0.014 | −0.020 | 0.019 | 0.004 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Chen, J.; Wang, Y.; Yao, L. Detecting the Hydrogen Bond Cooperativity in a Protein β-Sheet by H/D Exchange. Int. J. Mol. Sci. 2022, 23, 14821. https://doi.org/10.3390/ijms232314821
Li J, Chen J, Wang Y, Yao L. Detecting the Hydrogen Bond Cooperativity in a Protein β-Sheet by H/D Exchange. International Journal of Molecular Sciences. 2022; 23(23):14821. https://doi.org/10.3390/ijms232314821
Chicago/Turabian StyleLi, Jingwen, Jingfei Chen, Yefei Wang, and Lishan Yao. 2022. "Detecting the Hydrogen Bond Cooperativity in a Protein β-Sheet by H/D Exchange" International Journal of Molecular Sciences 23, no. 23: 14821. https://doi.org/10.3390/ijms232314821
APA StyleLi, J., Chen, J., Wang, Y., & Yao, L. (2022). Detecting the Hydrogen Bond Cooperativity in a Protein β-Sheet by H/D Exchange. International Journal of Molecular Sciences, 23(23), 14821. https://doi.org/10.3390/ijms232314821





