Model Design and Study of a U-Channel Photonic Crystal Fib Optic Sensor for Measuring Glucose Concentration in Blood
Abstract
1. Introduction
2. Structural Parameters
3. Results and Analyses
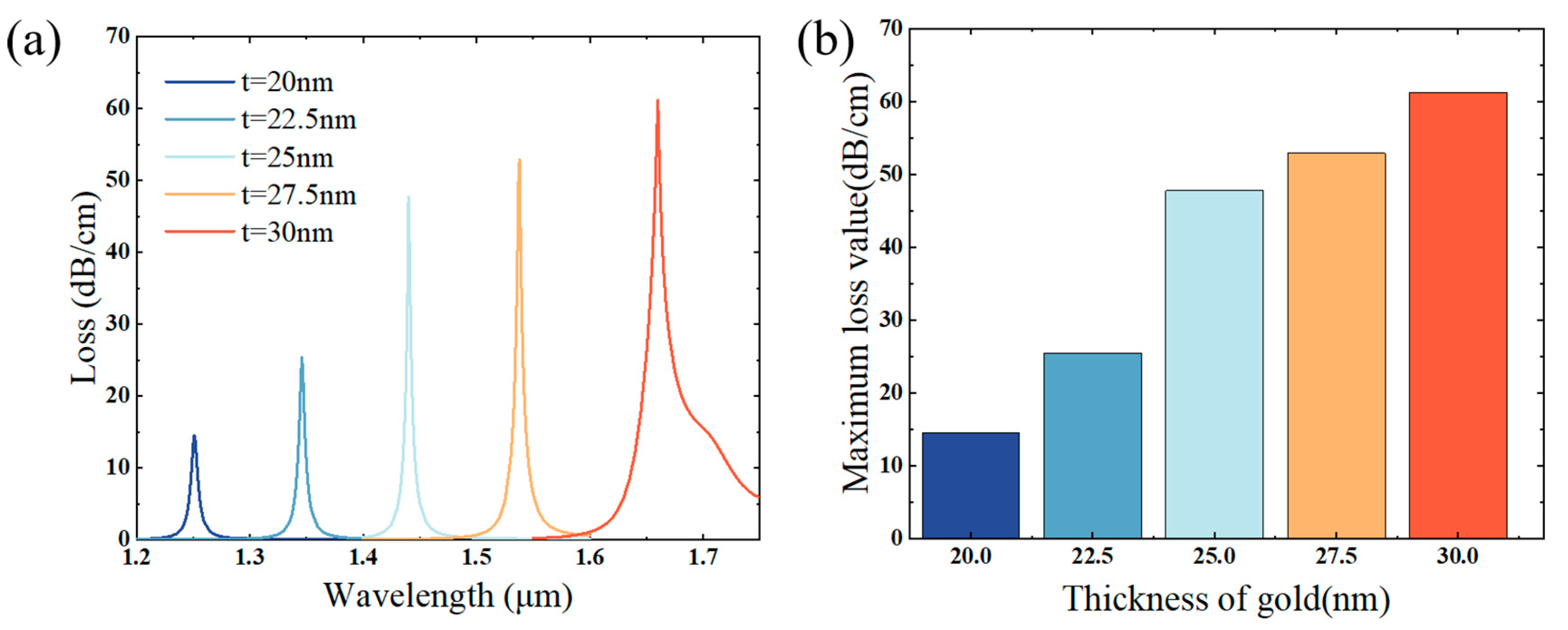
3.1. Au Film Thickness
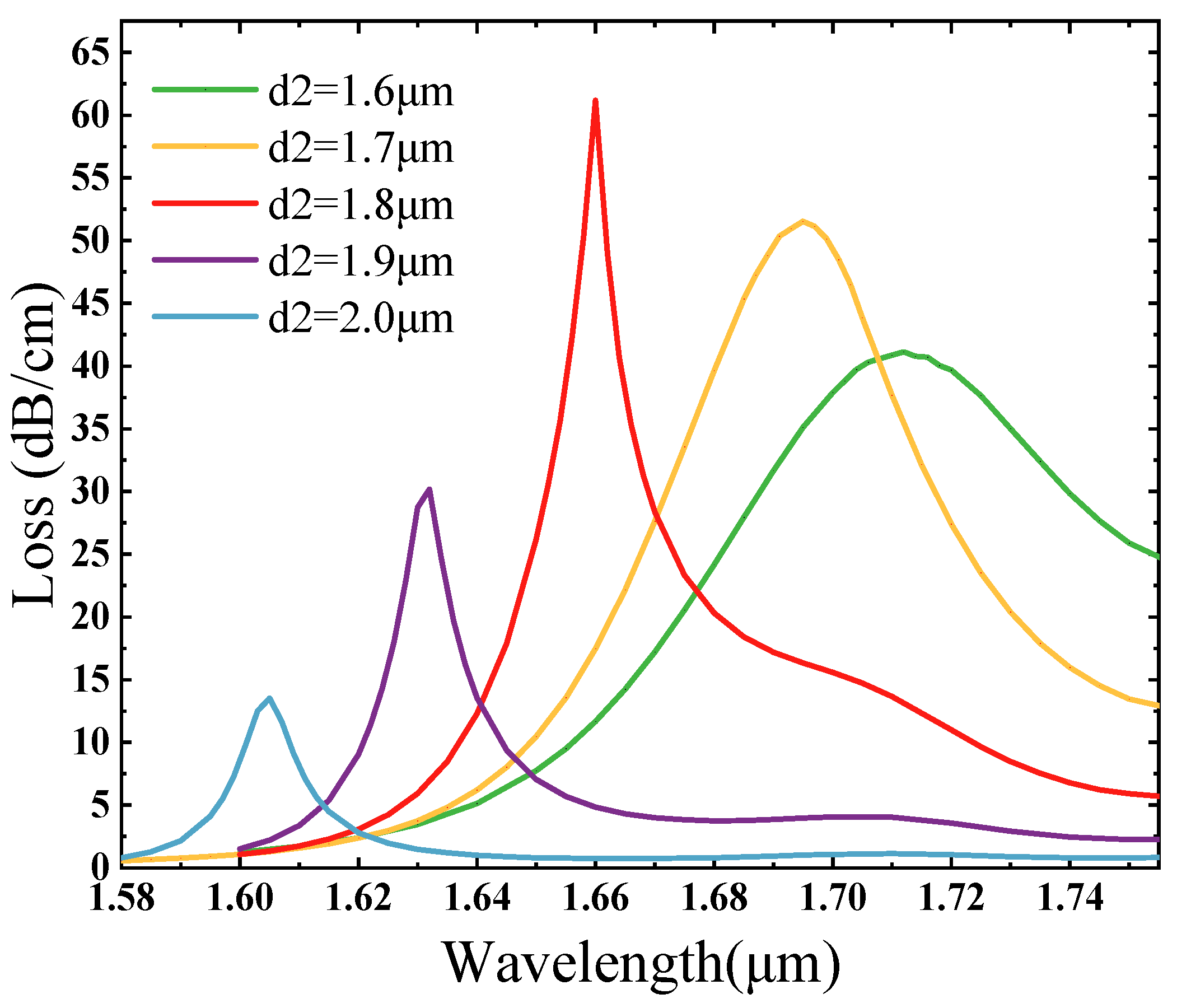
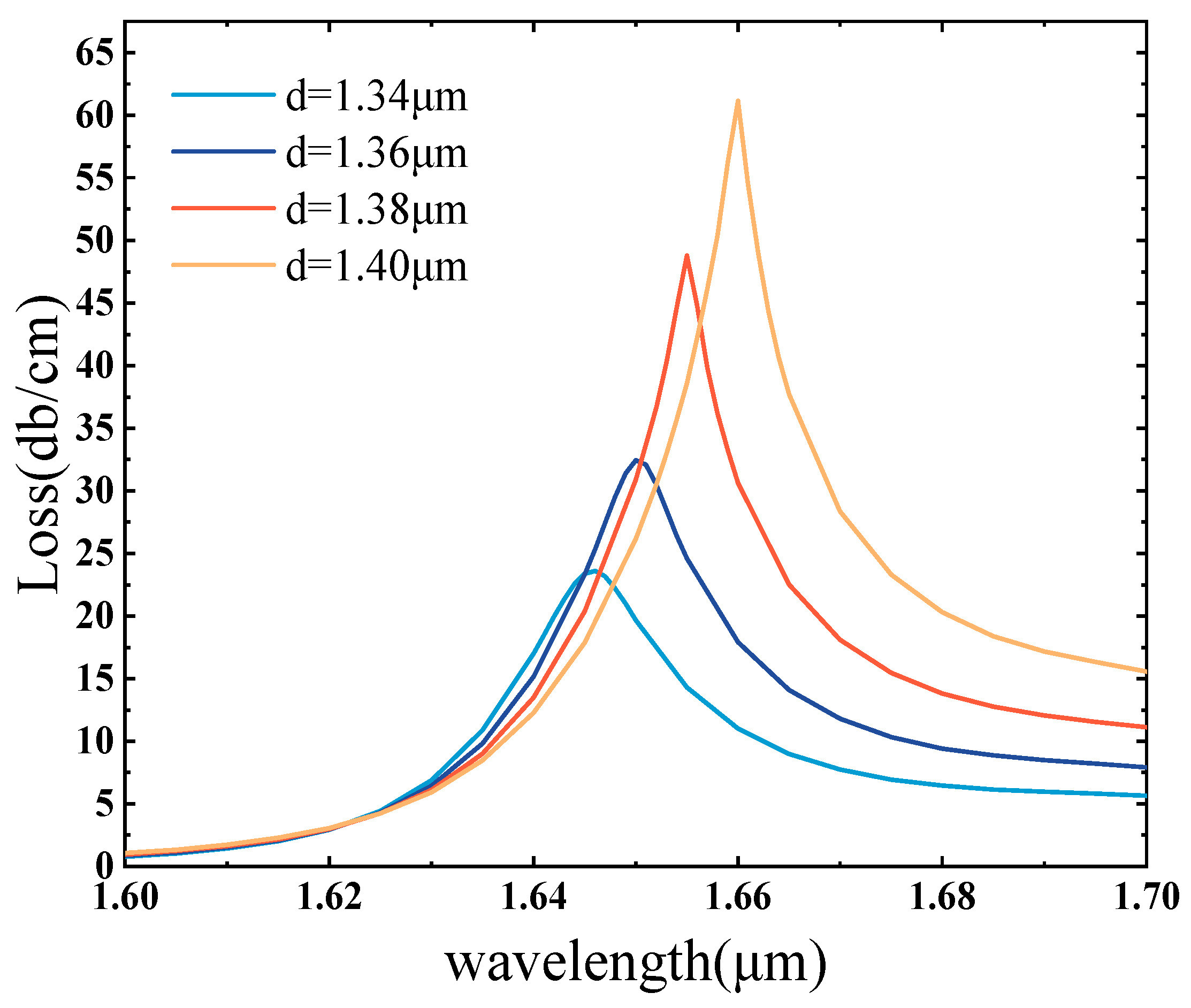
3.2. Channel Circle Diameter
3.3. Distance Between Channel Circles
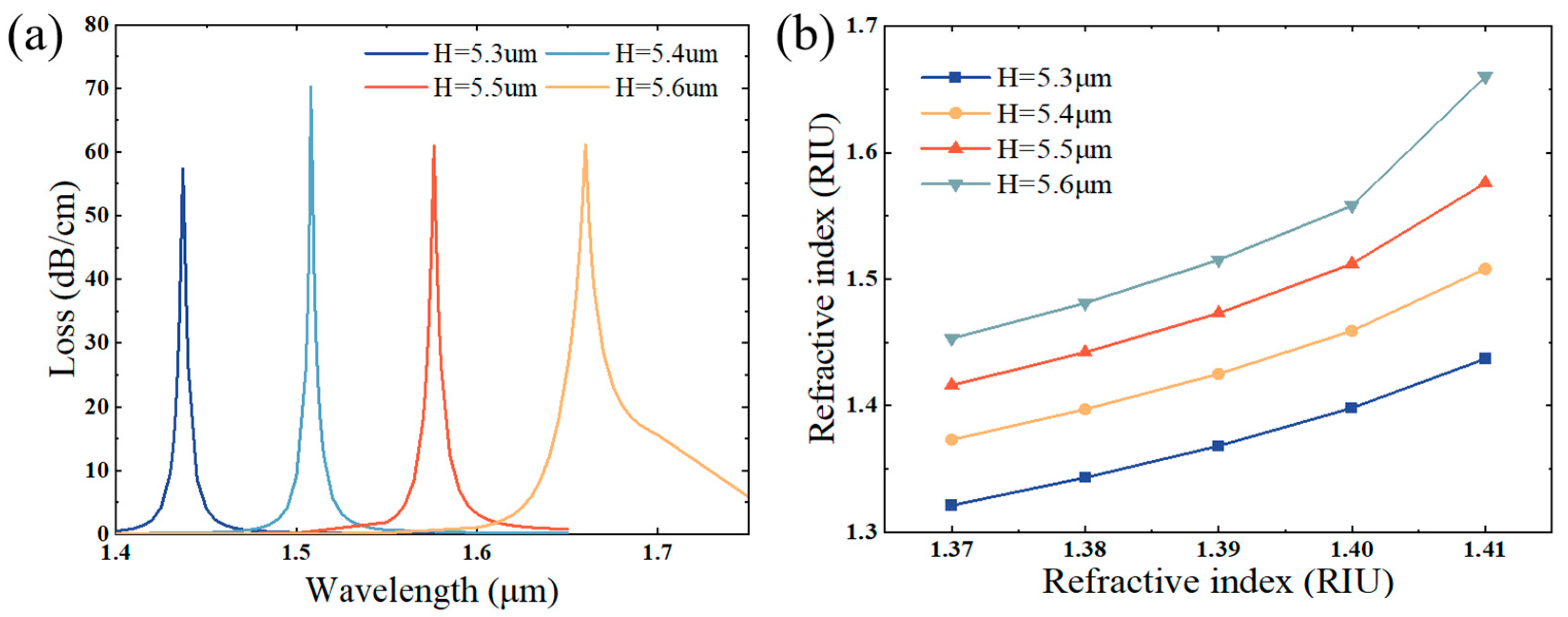
3.4. Distance of Fiber Core from D-Section H
3.5. Analysing Performance by Changing Refractive Index
4. Performances
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Zeng, L.L.; Li, B.X.; Wen, R.Q.; Zhang, X.J. Plasmonic Sensor Based on Multi Fano Resonance in Inverse T Shape Structure for Detection of CO2 Concentration. IEEE Photonics J. 2023, 15, 2201805. [Google Scholar] [CrossRef]
- Zhang, S.W.; Yang, H.; Tang, C.J.; Yi, Z.; Zhang, J.G.; Wang, J.Q.; Li, B.X. Multiple tunable six-peak graphene absorber for high-performance refractive index sensing. Phys. B Condens. Matter 2025, 708, 417225. [Google Scholar] [CrossRef]
- Zhang, B.W.; Luo, Y.N. Dynamic optical tuning and sensing in L-shaped dirac semimetal-based terahertz metasurfaces. Phys. Lett. A 2025, 541, 130419. [Google Scholar] [CrossRef]
- Hu, J.Y.; Tan, C.X.; Bai, W.D.; Li, Y.M.; Lin, Q.; Wang, L.L. Dielectric nanocavity-coupled surface lattice resonances for high-efficiency plasmonic sensing. J. Phys. D Appl. Phys. 2022, 55, 075105. [Google Scholar] [CrossRef]
- Pervadchuk, V.; Vladimirova, D.; Derevyankina, A. Mathematical Modeling of Capillary Drawing Stability for Hollow Optical Fibers. Algorithms 2023, 16, 83. [Google Scholar] [CrossRef]
- Jiang, B.; Hou, Y.; Wu, J.; Ma, Y.; Gan, X.; Zhao, J. In-fiber photoelectric device based on graphene-coated tilted fiber grating. Opto-Electron Sci 2023, 2, 230012. [Google Scholar] [CrossRef]
- Fano, U. The theory of anomalous diffraction gratings and of quasi-stationary waves on metallic surfaces (Sommerfeld’s waves). J. Opt. Soc. Am. 1941, 31, 213–222. [Google Scholar] [CrossRef]
- Kao, K.; Hockham, G. Dielectric-fibre surface waveguides for optical frequencies. Proc. IEEE 1966, 113, 115–158. [Google Scholar] [CrossRef]
- Safaei, A.; Chandra, S.; Shabbir, M.W.; Leuenberger, M.N.; Chanda, D. Dirac plasmon-assisted asymmetric hot carrier generation for room-temperature infrared detection. Nat. Commun. 2019, 10, 3498. [Google Scholar] [CrossRef]
- Li, B.X.; Zeng, L.L.; Wen, R.Q.; Zhang, X.J. Dynamic Regulation of Multiple Fano Resonances Based on Liquid Crystal. IEEE Photonics J. 2023, 15, 2200506. [Google Scholar] [CrossRef]
- Ma, R.; Zhang, L.; Liu, G.; Wang, L.; Lin, Q. The total optical force exerted on black phosphorus coated dielectric cylinder pairs enhanced by localized surface plasmon. J. Appl. Phys. 2021, 130, 113103. [Google Scholar] [CrossRef]
- Yan, X.; Lin, Q.; Wang, L.; Liu, G.D. Active absorption modulation by employing strong coupling between magnetic plasmons and borophene surface plasmons in the telecommunication band. J. Appl. Phys. 2022, 132, 063101. [Google Scholar] [CrossRef]
- Wang, H.Y.; Ma, R.; Liu, G.D.; Wang, L.L.; Lin, Q. Optical force conversion and conveyor belt effect with coupled graphene plasmon waveguide modes. Opt. Express 2023, 31, 32422. [Google Scholar] [CrossRef] [PubMed]
- Ma, R.; Zhang, L.G.; Zeng, Y.; Liu, G.D.; Wang, L.L.; Lin, Q. Extreme enhancement of optical force via the acoustic graphene plasmon mode. Opt. Express 2023, 31, 482723. [Google Scholar] [CrossRef]
- Ai, Z.; Liu, H.F.; Cheng, S.B.; Zhang, H.F.; Yi, Z.; Zeng, Q.D.; Wu, P.H.; Zhang, J.G.; Tang, C.J.; Hao, Z.Q. Four peak and high angle tilted insensitive surface plasmon resonance graphene absorber based on circular etching square window. J. Phys. D Appl. Phys. 2025. [Google Scholar] [CrossRef]
- Zeng, Z.L.; Liu, H.F.; Zhang, H.F.; Cheng, S.B.; Yi, Y.G.; Yi, Z.; Wang, J.Q.; Zhang, J.G. Tunable ultra-sensitive four-band terahertz sensors based on Dirac semimetals. Photonics Nanostruct.-Fundam. Appl. 2025, 63, 101347. [Google Scholar] [CrossRef]
- Rao, X.J.; Zhu, H.R.; Wang, X.X.; Chen, Y.Z.; Qi, Y.P.; Yang, H. High-sensitivity plasmonic refractive index sensor based on embedded gratings with triple self-reference characteristics. J. Opt. Soc. Am. B 2025, 42, 431–437. [Google Scholar] [CrossRef]
- Cheng, S.B.; Li, W.X.; Zhang, H.F.; Akhtar, M.N.; Yi, Z.; Zeng, Q.D.; Ma, C.; Sun, T.Y.; Wu, P.H.; Ahmad, S. High sensitivity five band tunable metamaterial absorption device based on block like Dirac semimetals. Opt. Commun. 2024, 569, 130816. [Google Scholar] [CrossRef]
- Wu, G.X.; Zhu, R.Z.; Lu, Y.Q.; Hong, M.; Xu, F. Optical scanning endoscope via a single multimode optical fiber. Opto-Electron. Sci. 2024, 3, 230041. [Google Scholar] [CrossRef]
- Wang, H.; Liu, S.; Liu, W.; Wang, S. Dual-Parameter Surface Plasmon Resonance Photonic Crystal Fiber Sensor for Simultaneous Magnetic Field and Temperature Detection with Potential SERS Applications. Photonics 2025, 12, 355. [Google Scholar] [CrossRef]
- Chauhan, J.; Kalra, Y.; Sinha, R.K. Chromatic Dispersion of Chalcogenide Glass-Based Photonic Crystal Fiber with Ultra-High Numerical Aperture. Phys. Sci. Forum 2024, 10, 8. [Google Scholar] [CrossRef]
- Lv, J.; Ren, Y.; Wang, D.; Wang, J.; Lu, X.; Yu, Y.; Li, W.; Liu, Q.; Xu, X.; Liu, W.; et al. Optical switching with high-Q Fano resonance of all-dielectric metasurface governed by bound states in the continuum. Opt. Express 2024, 32, 28334–28347. [Google Scholar] [CrossRef] [PubMed]
- Liu, M.L.; Li, B.X.; Zeng, L.L.; Wei, Y.; Wen, R.Q.; Zhang, X.J.; Deng, C.S. Dynamic tunable narrow-band perfect absorber for fiber -optic communication band based on liquid crystal. J. Phys. D Appl. Phys. 2023, 56, 505102. [Google Scholar] [CrossRef]
- Denisov, A.N.; Dvoyrin, V.V.; Semjonov, S.L. Crossing and Anticrossing in Bent All-Glass Leakage Channel Microstructured Optical Fibers: The Effect of Polymer Coating. Photonics 2024, 11, 1204. [Google Scholar] [CrossRef]
- Zhang, L.; Zhen, Y.; Tong, L. Optical Micro/Nanofiber Enabled Tactile Sensors and Soft Actuators: A Review. Opto-Electron. Sci. 2024, 3, 24005–240017. [Google Scholar] [CrossRef]
- Yang, C.; Luo, M.H.; Ju, X.W.; Hu, J.Y. Ultra-narrow dual-band perfect absorber based on double-slotted silicon nanodisk arrays. J. Phys. D Appl. Phys. 2024, 57, 345104. [Google Scholar] [CrossRef]
- Jarnda, K.V.; Dai, H.; Ali, A.; Bestman, P.L.; Trafialek, J.; Roberts-Jarnda, G.P.; Anaman, R.; Kamara, M.G.; Wu, P.; Ding, P. A Review on Optical Biosensors for Monitoring of Uric Acid and Blood Glucose Using Portable POCT Devices: Status, Challenges, and Future Horizons. Biosensors 2025, 15, 222. [Google Scholar] [CrossRef]
- Hidalgo, J.I.; Colmenar, J.M.; Kronberger, G.; Winkler, S.M.; Garnica, O.; Lanchares, J. Data based prediction of blood glucose concentrations using evolutionary methods. J. Med. Syst. 2017, 41, 142. [Google Scholar] [CrossRef]
- Abdelghaffar, M.; Gamal, Y.; El-Khoribi, R.A.; Soliman, W.; Badr, Y.; Hameed, M.F.O.; Obayya, S.S.A. Highly sensitive V-shaped SPR PCF biosensor for cancer detection. Opt. Quant. Electron. 2023, 55, 472. [Google Scholar] [CrossRef]
- Islam, M.R.; Jamil, M.A.; Zaman, S.U.; Ahsan, S.A.H.; Islam, M. Design and Analysis of Birefringent SPR Based PCF Biosensor with Ultra-High Sensitivity and Low Loss. Optik 2020, 221, 165311. [Google Scholar] [CrossRef]
- Li, Y.M.; Tan, C.X.; Hu, J.Y.; Bai, W.D.; Zhang, R.L.; Lin, Q.; Zhang, Y.; Wang, L.L. Ultra-narrow band perfect absorbance induced by magnetic lattice resonances in dielectric dimer metamaterials. Results Phys. 2022, 39, 105730. [Google Scholar] [CrossRef]
- Chiang, J.S.; Wu, T.L. Analysis of propagation characteristics for an octagonal photonic crystal fiber (O-PCF). Opt. Commun. 2006, 258, 170–176. [Google Scholar] [CrossRef]
- Ling, Z.X.; Zeng, Y.; Liu, G.D.; Wang, L.L.; Lin, Q. Unified model for plasmon-induced transparency with direct and indirect coupling in borophene-integrated metamaterials. Opt. Express 2022, 30, 21966. [Google Scholar] [CrossRef] [PubMed]
- Li, W.X.; Cheng, S.B.; Yi, Z.; Zhang, H.F.; Song, Q.J.; Hao, Z.Q.; Sun, T.Y.; Wu, P.H.; Zeng, Q.D.; Raza, R. Advanced optical reinforcement materials based on three-dimensional four-way weaving structure and metasurface technology. Appl. Phys. Lett. 2025, 126, 033503. [Google Scholar] [CrossRef]
- Li, Z.T.; Cheng, S.B.; Zhang, H.F.; Yang, W.X.; Yi, Z.; Yi, Y.G.; Wang, J.Q.; Ahmad, S.; Raza, R. Ultrathin broadband terahertz metamaterial based on single-layer nested patterned graphene. Phys. Lett. A 2025, 534, 130262. [Google Scholar] [CrossRef]
- Li, B.X.; Liu, M.L.; Wen, R.Q.; Wei, Y.; Zeng, L.L.; Deng, C.S. Dynamic control of Fano-like interference in the graphene periodic structure. J. Phys. D Appl. Phys. 2023, 56, 115104. [Google Scholar] [CrossRef]
- Hu, J.Y.; Bai, W.D.; Tan, C.X.; Li, Y.M.; Lin, Q.; Wang, L.L. Highly electric field enhancement induced by anapole modes coupling in the hybrid dielectric-metal nanoantenna. Opt. Commun. 2022, 511, 127987. [Google Scholar] [CrossRef]
- Wang, J.Q.; Sun, J.Y.; Sun, S.; Zhang, H.; Wang, Q.Q.; Yang, J.Y.; Mei, Y.W. Numerical simulation of electromagnetically induced transparency in composite metamaterial. Phys. Scr. 2025, 100, 025512. [Google Scholar] [CrossRef]
- Zhou, H.Y.; Zhang, C.; Nong, H.C.; Weng, J.; Wang, D.; Yu, Y.; Zhang, J.; Zhang, C.; Yu, J.; Zhang, Z.; et al. Multi-photon neuron embedded bionic skin for high-precision complex texture and object reconstruction perception research. Opto-Electron. Adv. 2025, 8, 240152. [Google Scholar] [CrossRef]
- Chen, Z.Y.; Cheng, S.B.; Zhang, H.F.; Yi, Z.; Tang, B.; Chen, J.; Zhang, J.G.; Tang, C.J. Ultra wideband absorption absorber based on Dirac semimetallic and graphene metamaterials. Phys. Lett. A 2024, 517, 129675. [Google Scholar] [CrossRef]
- Denisov, A.N.; Dvoyrin, V.V.; Semjonov, S.L. Anticrossing and Mode Coupling in Bent All-Glass Leakage Channel Microstructured Optical Fibers with Large Mode Area. Photonics 2024, 11, 985. [Google Scholar] [CrossRef]
- Yin, S.Y.; Guo, Q.; Liu, S.R.; He, J.W.; Yu, Y.S.; Tian, Z.N.; Chen, Q.D. Three-dimensional multichannel waveguide grating filters. Opto-Electron. Sci. 2024, 3, 240003. [Google Scholar] [CrossRef]
- Li, W.; Cheng, S.; Zhang, H.; Yi, Z.; Tang, B.; Ma, C.; Wu, P.; Zeng, Q.; Raza, R. Multi-functional metasurface: Ultra-wideband/multi-band absorption switching by adjusting guided mode resonance and local surface plasmon resonance effects. Commun. Theor. Phys. 2024, 76, 065701. [Google Scholar] [CrossRef]
- Luo, M.H.; Hu, J.Y.; Li, Y.M.; Bai, W.D.; Zhang, R.L.; Lin, Q.; Wang, L.L. Anapole-assisted ultra-narrow-band lattice resonance in slotted silicon nanodisk arrays. J. Phys. D Appl. Phys. 2023, 56, 375102. [Google Scholar] [CrossRef]
- Gao, H.; Hu, H.F.; Zhan, Q.W. Tailoring temperature response for a multimode fiber. Opto-Electron. Sci. 2025, 4, 240004. [Google Scholar] [CrossRef]
- Li, Z.T.; Li, X.; Liu, G.D.; Wang, L.L.; Lin, Q. Analytical investigation of unidirectional reflectionless phenomenon near the exceptional points in graphene plasmonic system. Opt. Express 2023, 31, 30458. [Google Scholar] [CrossRef]
- Li, W.; Yi, Y.; Yang, H.; Cheng, S.; Yang, W.X.; Zhang, H.; Yi, Z.; Yi, Y.; Li, H. Active Tunable Terahertz Band-width Absorber Based on single layer Graphene. Commun. Theor. Phys. 2023, 75, 045503. [Google Scholar] [CrossRef]
- Zeng, Y.; Ling, Z.X.; Liu, G.D.; Wang, L.L.; Lin, Q. Tunable plasmonically induced transparency with giant group delay in gain-assisted graphene metamaterials. Opt. Express 2022, 30, 455954. [Google Scholar] [CrossRef]
- Imas, J.J.; Matías, I.R.; Del Villar, I.; Ozcáriz, A.; Zamarreño, C.R.; Albert, J. All-fiber ellipsometer for nanoscale dielectric coatings. Opto-Electron. Adv. 2023, 6, 230048. [Google Scholar] [CrossRef]
- Li, Z.; Chen, J.X.; Li, L.Z.; Zhang, J.J.; Yao, J.P. Exceptional-point-enhanced sensing in an all-fiber bending sensor. Opto-Electron. Adv. 2023, 6, 230019. [Google Scholar] [CrossRef]
- Yang, C.; Lin, Q.; Du, W.J.; Wang, L.L.; Liu, G.D. Bi-tunable absorber based on borophene and VO2 in the optical telecom-munication band. J. Opt. Soc. Am. B 2022, 39, 2969–2974. [Google Scholar] [CrossRef]
- Ding, X.; Lin, Q.; Wang, M.; Liu, S.; Zhang, W.; Chen, N.; Wang, Y. Design and Simulation of High-Performance D-Type Dual-Mode PCF-SPR Refractive Index Sensor Coated with Au-TiO2 Layer. Sensors 2024, 24, 6118. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Li, Z.T.; Wang, L.L.; Lin, Q. Unidirectional reflectionless propagation in borophene plasmonic metamaterials. J. Phys. D Appl. Phys. 2023, 57, 015103. [Google Scholar] [CrossRef]
- Li, Z.; Song, Q.J.; Jia, L.B.; Yi, Z.; Cheng, S.B.; Wang, J.Q.; Li, B.X. Actively tunable multi-frequency narrowband terahertz absorber using graphene metamaterials. Opt. Commun. 2025, 583, 131768. [Google Scholar] [CrossRef]
- Sherburne, M.; Harjes, C.; Klitsner, B.; Gigax, J.; Ivanov, S.; Schamiloglu, E.; Lehr, J. Rapid Prototyping for Nanoparticle-Based Photonic Crystal Fiber Sensors. Sensors 2024, 24, 3707. [Google Scholar] [CrossRef]
- Feng, H.; Chen, X.; Zhu, R.; Xiong, Y.; Chen, Y.; Lu, Y.; Xu, F. Seeing at a distance with multicore fibers. Opto-Electron. Adv. 2024, 7, 230202. [Google Scholar] [CrossRef]
- Vladimirova, D.; Pervadchuk, V.; Konstantinov, Y. Manufacture of Microstructured Optical Fibers: Problem of Optimal Control of Silica Capillary Drawing Process. Computation 2024, 12, 86. [Google Scholar] [CrossRef]
- Zhu, H.T.; Luo, J.X.; Dai, Q.; Zhu, S.G.; Yang, H.; Zhou, K.; Zhan, L.; Xu, B.; Chen, Y.; Lu, Y.; et al. Spatiotemporal hemodynamic monitoring via configurable skin-like microfiber Bragg grating group. Opto-Electron. Adv. 2023, 6, 230018. [Google Scholar] [CrossRef]
- Yan, D.Y.; Tang, C.J.; Yi, Z.; Wang, J.Q.; Li, B.X. A fully symmetric solar absorber for thermophotovoltaic power generation. Phys. Lett. A 2025, 542, 130461. [Google Scholar] [CrossRef]
- Tan, Z.Q.; Lin, Q.; Du, W.J.; Wang, L.L.; Liu, G.D. Simultaneously enhance electric and magnetic Purcell factor by strong coupling between toroidal dipole quasi-BIC and electric dipole. J. Appl. Phys. 2025, 137, 033103. [Google Scholar] [CrossRef]
- Zhang, Y.X.; Lin, Q.; Yan, X.Q.; Wang, L.L.; Liu, G.D. Flat-band Friedrich-Wintgen bound states in the continuum based on borophene metamaterials. Opt. Express 2024, 32, 10669–10678. [Google Scholar] [CrossRef] [PubMed]
- Cano-Velázquez, M.S.; Hendriks, A.L.; Picelli, L.; van Veldhoven, R.P.J.; Fiore, A. Temperature-Compensated Solution Concentration Measurements Using Photonic Crystal Fiber-Tip Sensors. Sensors 2023, 23, 7703. [Google Scholar] [CrossRef] [PubMed]
- Xiang, T.; Sun, Z.; Wang, L.L.; Lin, Q.; Liu, G.D. Polarization independent perfect absorption of borophene metamaterials operating in the communication band. Phys. Scr. 2024, 99, 085519. [Google Scholar] [CrossRef]
- Chen, T.S.; Gao, J.Y.; Wang, X.X.; Chen, Y.Z.; Yang, H.; Qi, Y.P. High FOM fano resonance refractive-index sensor based on a baffled MIM waveguide coupled with an inverted L-Shaped resonator. Phys. Scr. 2025, 100, 015533. [Google Scholar] [CrossRef]
- Panda, A.; Pukhrambam, P.D.; Keiser, G. Performance analysis of graphene-based surface plasmon resonance biosensor for blood glucose and gas detection. Appl. Phys. A 2020, 126, 153. [Google Scholar] [CrossRef]
- Jiang, B.; Zhou, K.; Wang, C.; Sun, Q.; Yin, G.; Tai, Z.; Wilson, K.; Zhao, J.; Zhang, L. Label-free glucose biosensor based on enzymatic graphene oxide-functionalized tilted fiber grating. Sens. Actuators B Chem. 2018, 254, 1033–1039. [Google Scholar] [CrossRef]
- Wang, X.Y.; Lin, Q.; Wang, L.L.; Liu, G.D. Dynamic control of polarization conversion based on borophene nanostructures in optical communication bands. Phys. Scr. 2024, 99, 085531. [Google Scholar] [CrossRef]
- Zeng, T.Y.; Liu, G.D.; Wang, L.L.; Lin, Q. Light-matter interactions enhanced by quasi-bound states in the continuum in a graphene-dielectric metasurface. Opt. Express 2021, 29, 40177–40186. [Google Scholar] [CrossRef]
- Wang, Y.J.; Liu, Z.M.; Zhou, F.Q.; Yi, Z.; Wang, J.Q. Perfect absorption properties of a near-infrared super-surface perfect absorber based on a multilayer subwavelength array structure. Phys. Lett. A 2025, 540, 130395. [Google Scholar] [CrossRef]
- Wang, J.Q.; Yang, J.Y.; Mei, Y.W. Non-radiating anapole state in dielectric nanostructures and metamaterials. J. Phys. D Appl. Phys. 2025. [Google Scholar] [CrossRef]
- Gu, X.; Liu, X.; Yan, X.F.; Du, W.J.; Lin, Q.; Wang, L.L.; Liu, G.D. Polaritonic coherent perfect absorption based on self-hybridization of a quasi-bound state in the continuum and exciton. Opt. Express 2023, 31, 4691–4700. [Google Scholar] [CrossRef] [PubMed]
- Denisov, A.; Dvoyrin, V.; Kosolapov, A.; Likhachev, M.; Velmiskin, V.; Zhuravlev, S.; Semjonov, S. All-Glass Single-Mode Leakage Channel Microstructured Optical Fibers with Large Mode Area and Low Bending Loss. Photonics 2023, 10, 465. [Google Scholar] [CrossRef]
- Vafapour, Z. Polarization-Independent Perfect Optical Metamaterial Absorber as a Glucose Sensor in Food Industry Applications. IEEE Trans. Nanobiosci. 2019, 18, 622–627. [Google Scholar] [CrossRef] [PubMed]
- Sharifi, H.; Eskandari, S. Sensing blood components and cancer cells with photonic crystal resonator biosensor. Results Opt. 2024, 14, 100593. [Google Scholar] [CrossRef]
- Yashaswini, P.; Gayathri, H.; Srikanth, P. Performance analysis of photonic crystal based biosensor for the detec-tion of bio-molecules in urine and blood. Mater. Today Proc. 2023, 80, 2247–2254. [Google Scholar] [CrossRef]
- Parandin, F. High contrast ratio all-optical 4 × 2 encoder based on two-dimensional photonic crystals. Opt. Laser Technol. 2019, 113, 447–452. [Google Scholar] [CrossRef]
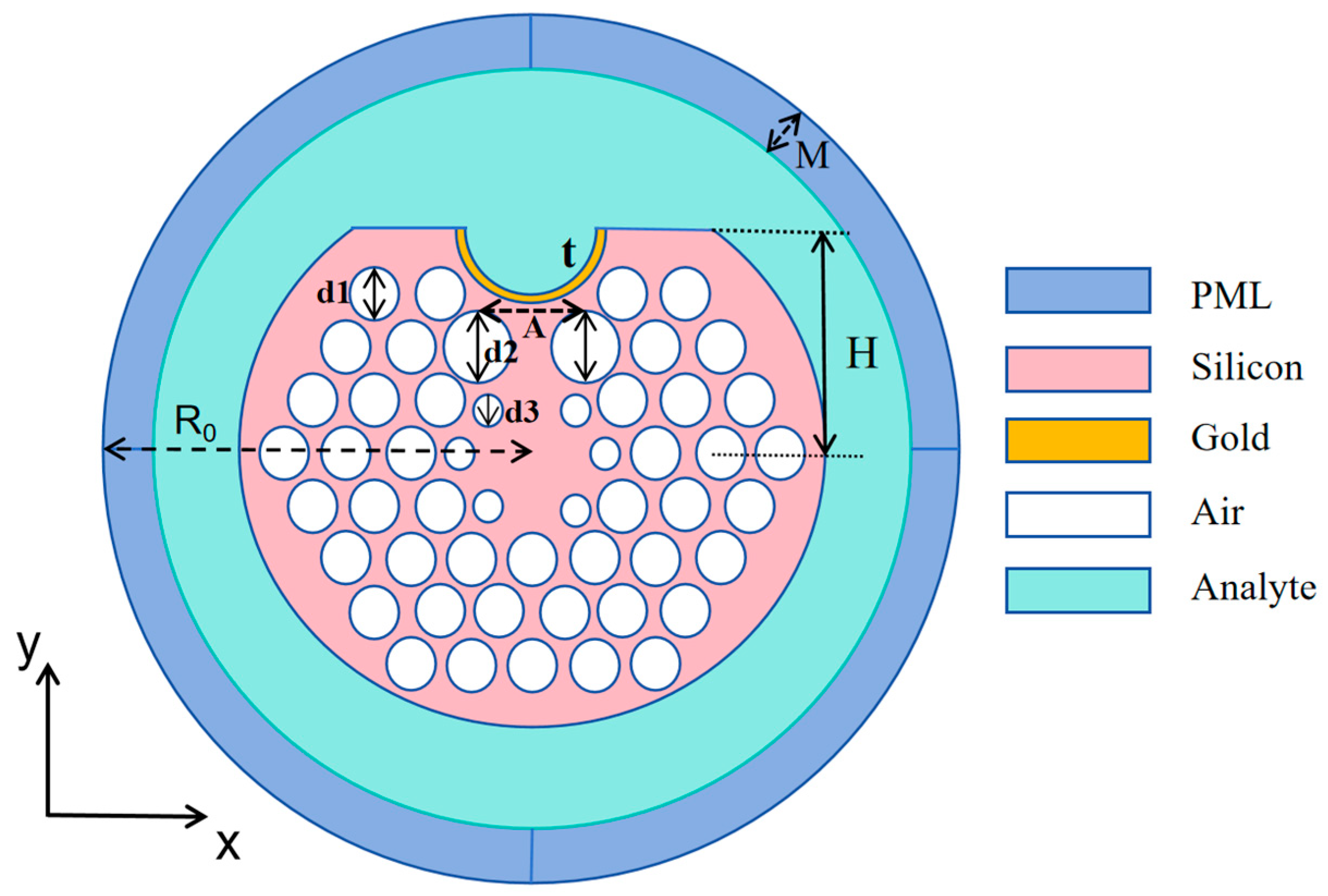




| Notation | Parametric | Numerical Value |
|---|---|---|
| R0 | PCF radius | 11 μm |
| d1 | Diameter of No. 1 air hole | 1.4 μm |
| d2 | Diameter of air holes No. 2 | 1.8 μm |
| d3 | Hole 3 diameter | 0.8 μm |
| t | Metal film thickness | 30 nm |
| A | Distance between two channel circles | 2.8 μm |
| H | Core distance from D-section | 5.6 μm |
| M | PML layer thickness | 1 μm |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, L.; Yang, H.; Sun, T.; Song, Q.; Yi, Z.; Yi, Y. Model Design and Study of a U-Channel Photonic Crystal Fib Optic Sensor for Measuring Glucose Concentration in Blood. Sensors 2025, 25, 2647. https://doi.org/10.3390/s25092647
Zhao L, Yang H, Sun T, Song Q, Yi Z, Yi Y. Model Design and Study of a U-Channel Photonic Crystal Fib Optic Sensor for Measuring Glucose Concentration in Blood. Sensors. 2025; 25(9):2647. https://doi.org/10.3390/s25092647
Chicago/Turabian StyleZhao, Lei, Hua Yang, Tangyou Sun, Qianju Song, Zao Yi, and Yougen Yi. 2025. "Model Design and Study of a U-Channel Photonic Crystal Fib Optic Sensor for Measuring Glucose Concentration in Blood" Sensors 25, no. 9: 2647. https://doi.org/10.3390/s25092647
APA StyleZhao, L., Yang, H., Sun, T., Song, Q., Yi, Z., & Yi, Y. (2025). Model Design and Study of a U-Channel Photonic Crystal Fib Optic Sensor for Measuring Glucose Concentration in Blood. Sensors, 25(9), 2647. https://doi.org/10.3390/s25092647







