Sensitivity of Numerical Predictions to the Permeability Coefficient in Simulations of Melting and Solidification Using the Enthalpy-Porosity Method †
Abstract
:1. Introduction
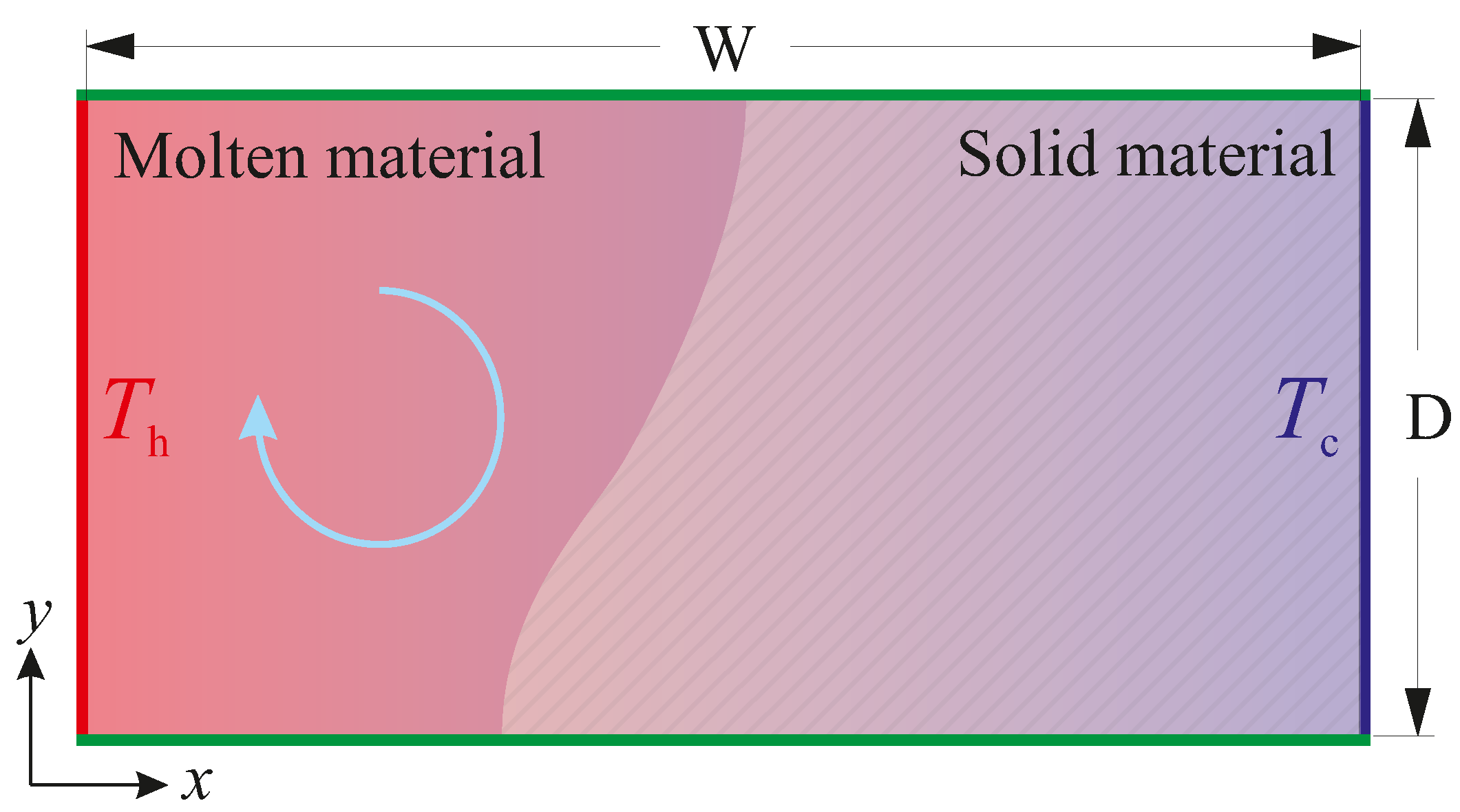
2. Problem Description
3. Mathematical Formulation
4. Numerical Procedure
5. Results
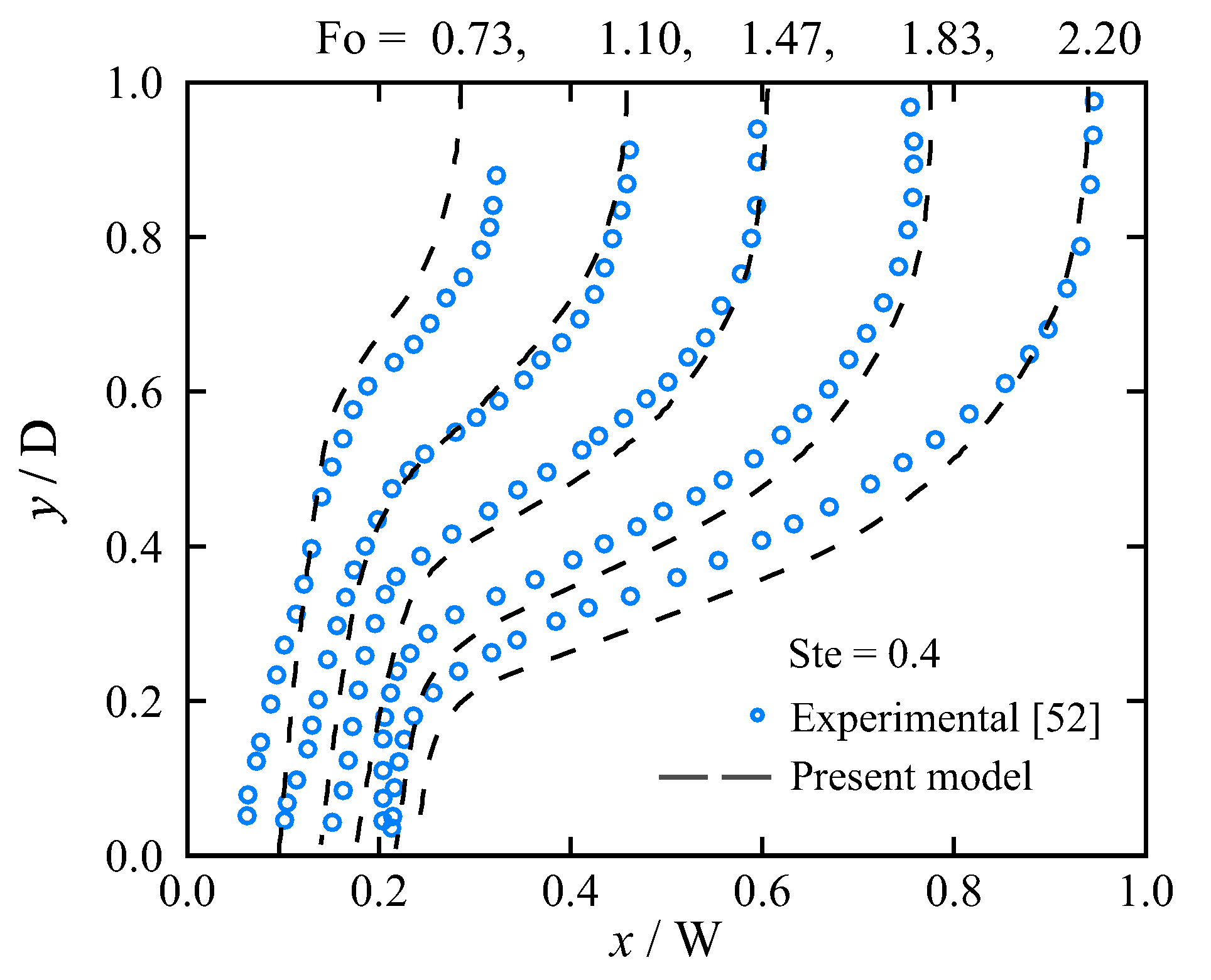
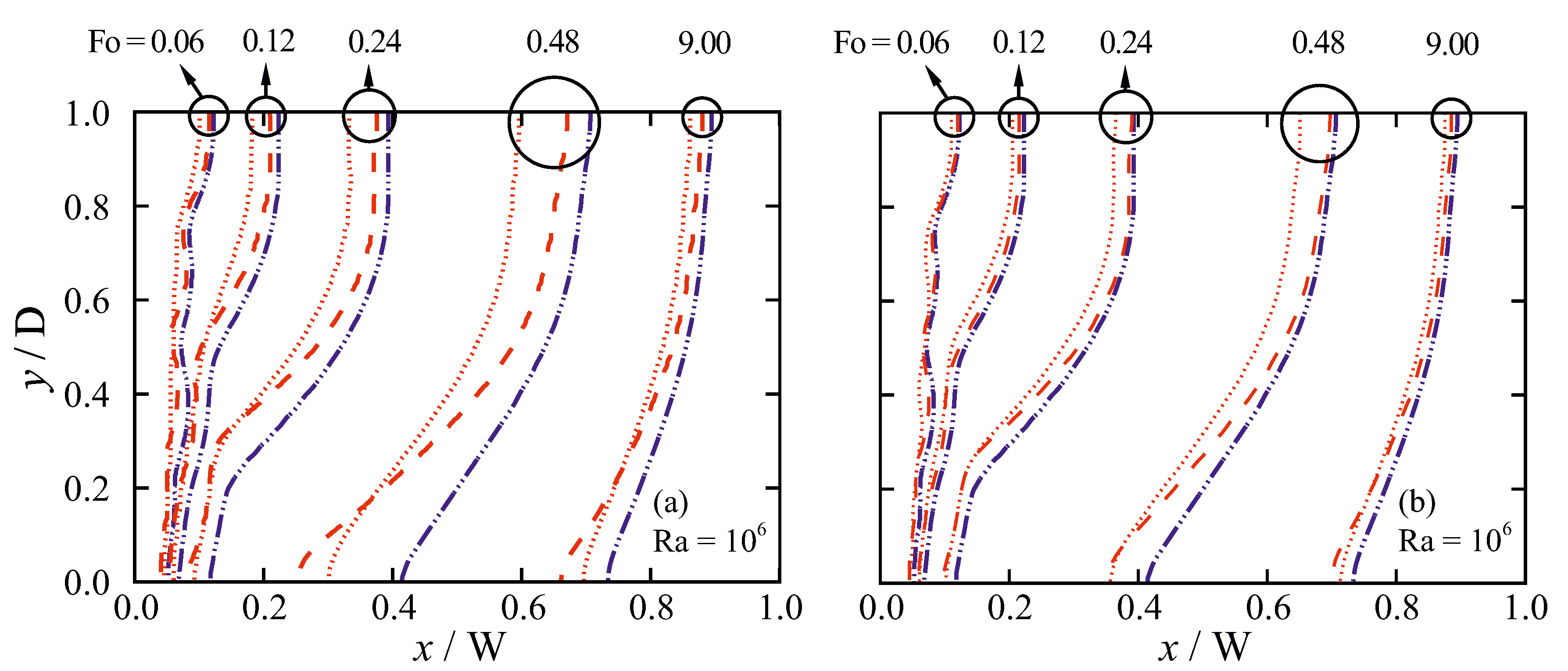
5.1. Model Verification
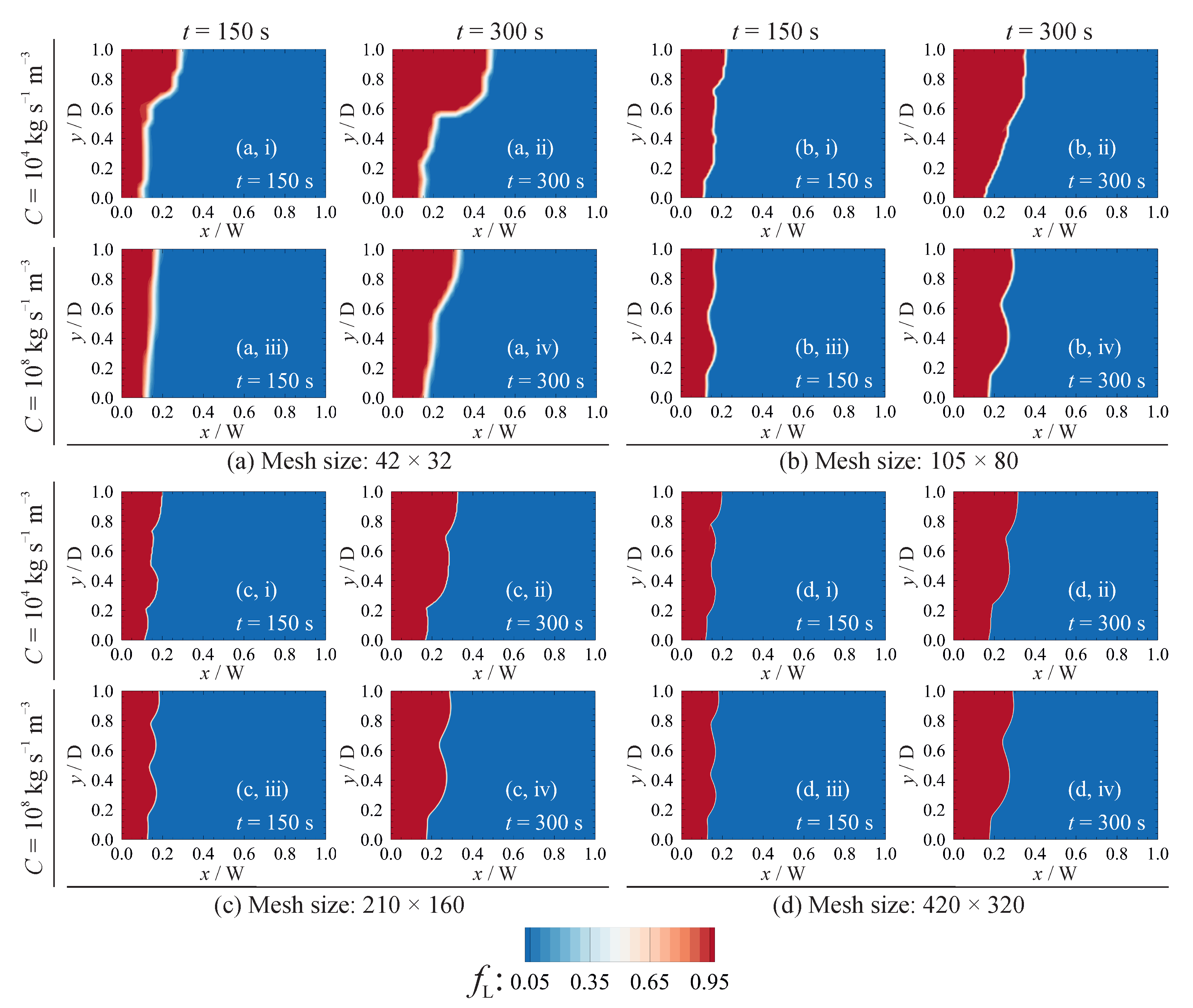
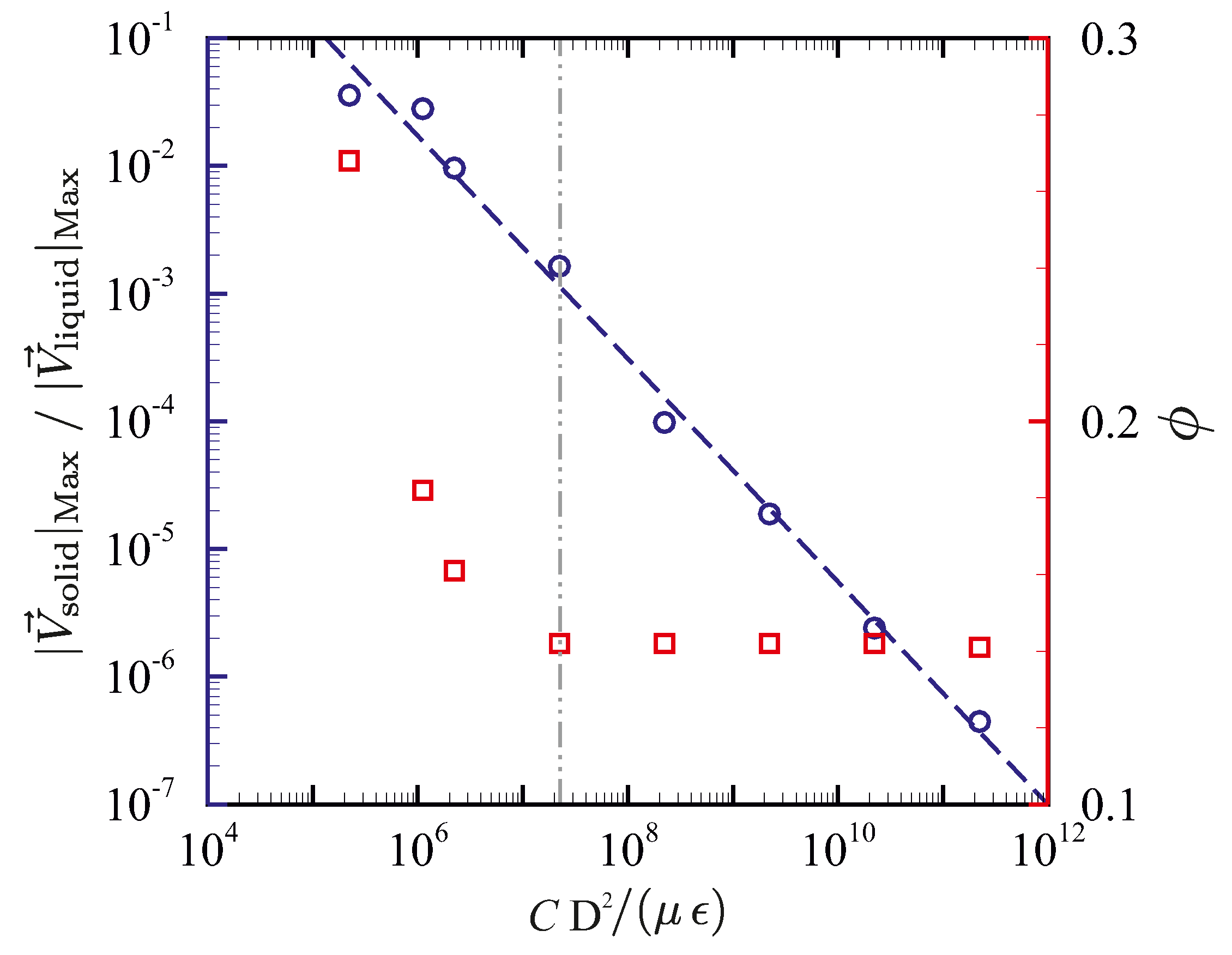
5.2. Grid Size and Sensitivity to the Permeability Coefficient for Isothermal Phase Change
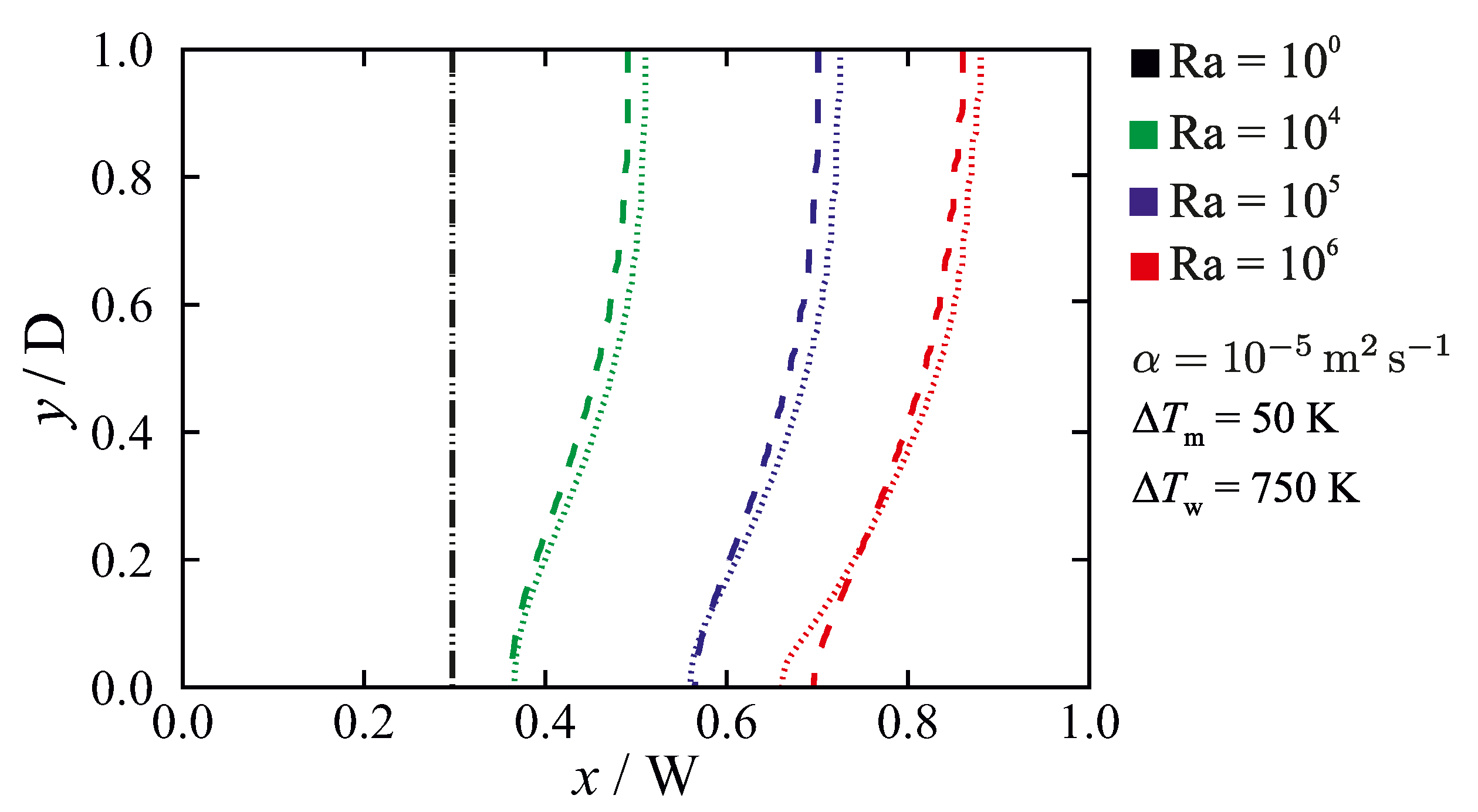
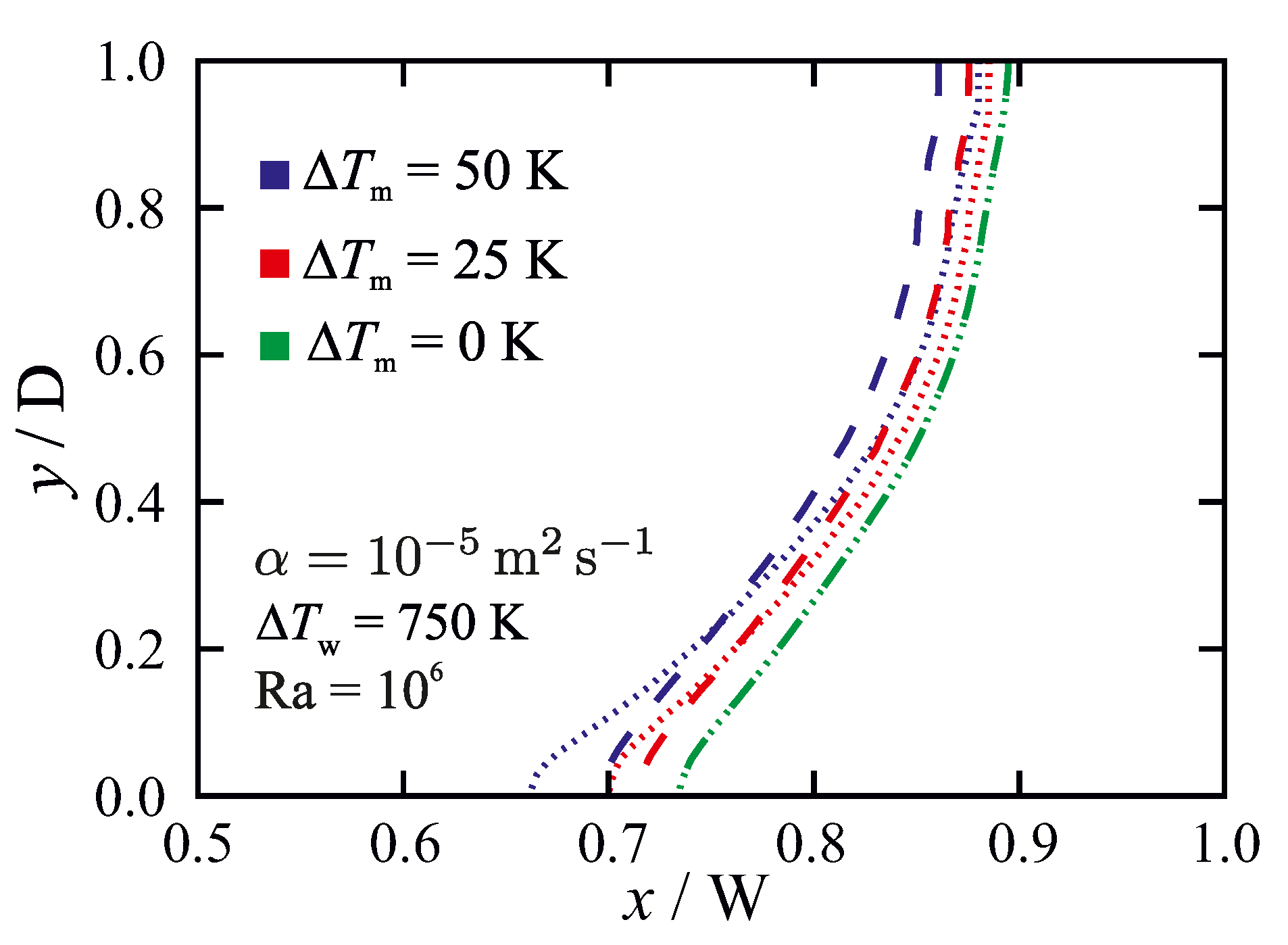
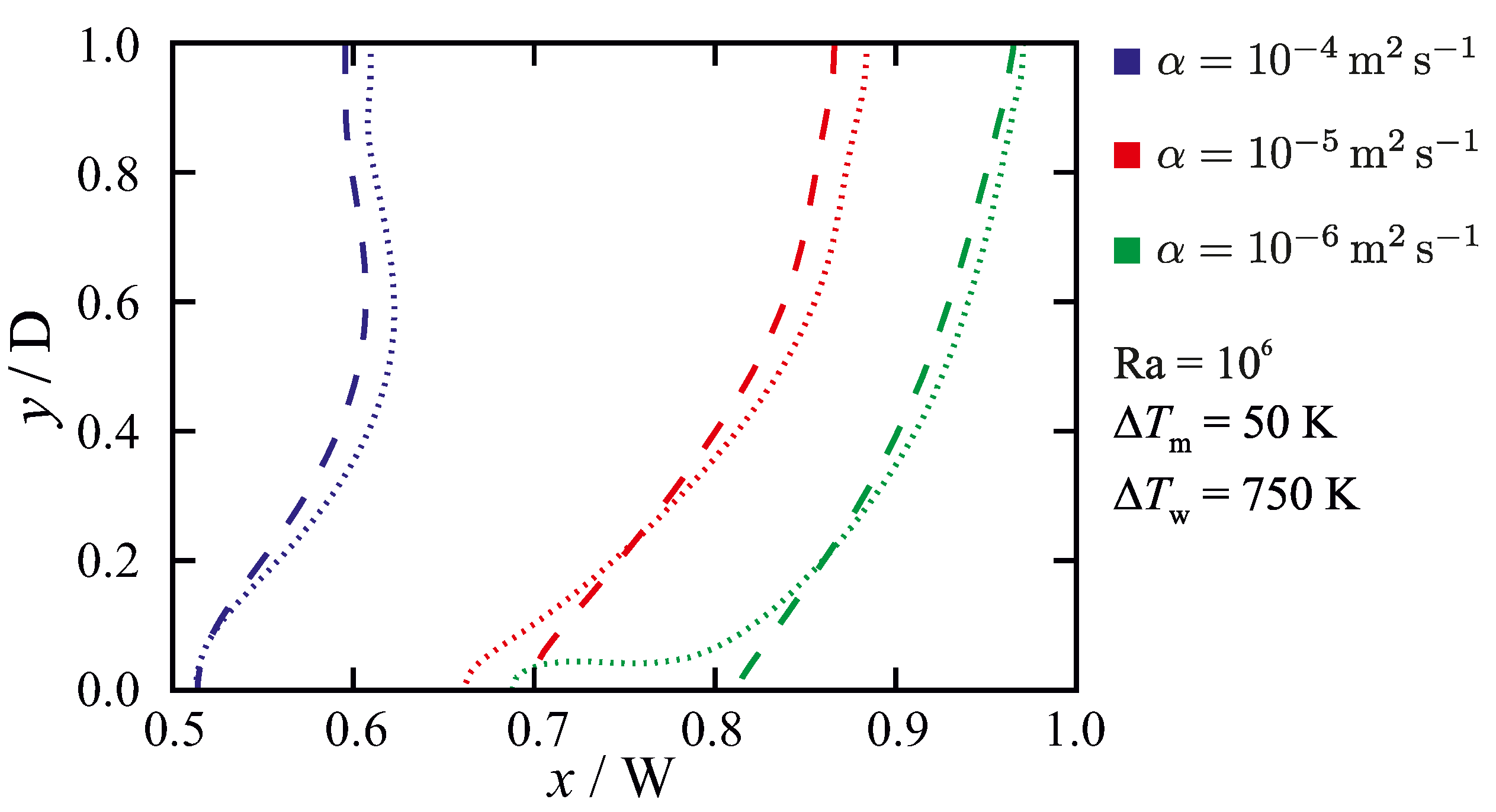
5.3. Non-Isothermal Phase-Change
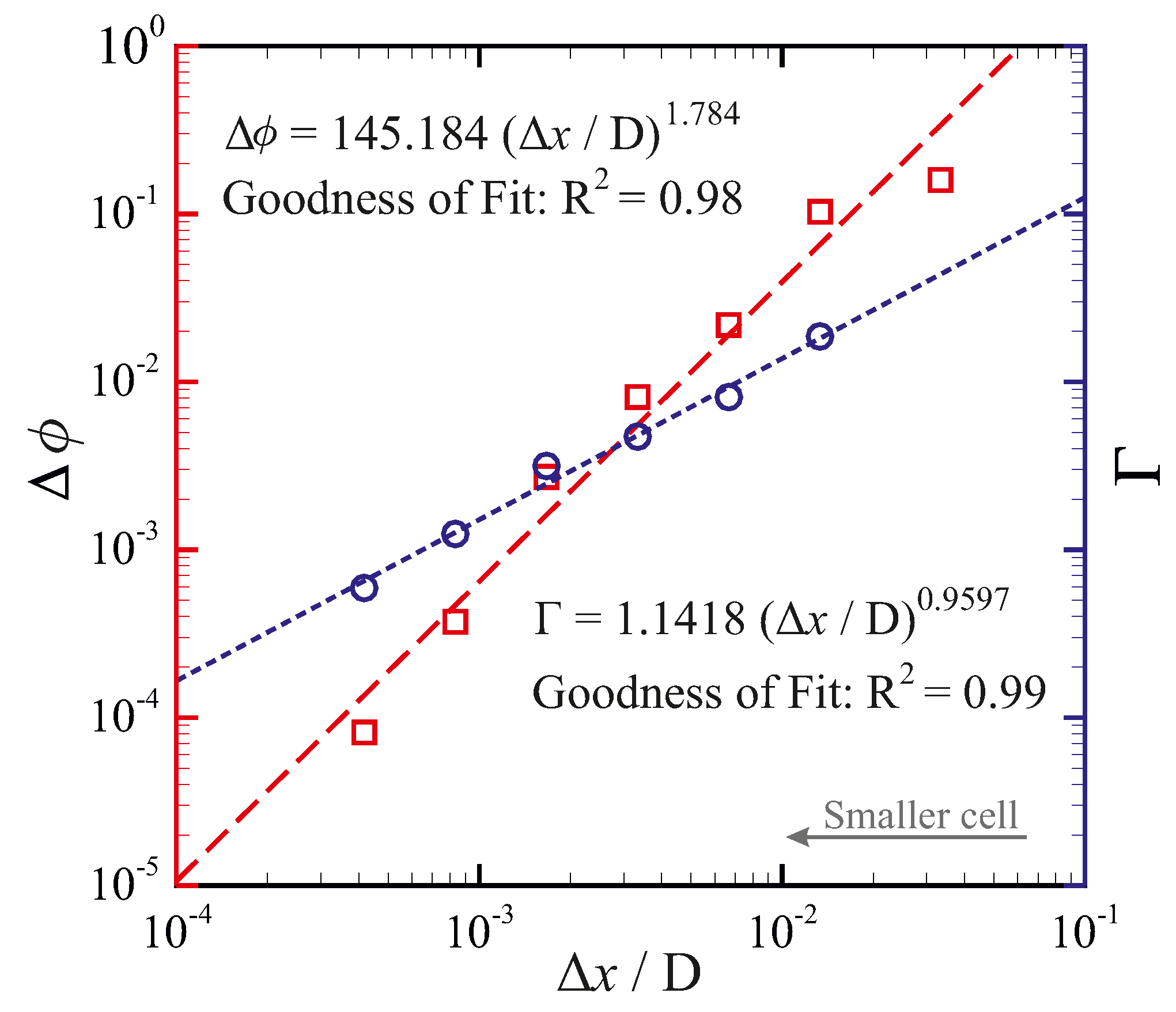
5.3.1. Grid Sensitivity
5.3.2. Influence of the Permeability Coefficient on Predicted Results
6. Discussion
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Crank, J. Free and Moving Boundary Problems; Clarendon Press: Oxford, UK, 1984. [Google Scholar]
- Shyy, W. Multi-scale computational heat transfer with moving solidification boundaries. Int. J. Heat Fluid Flow 2002, 23, 278–287. [Google Scholar] [CrossRef]
- Rappaz, M. Modelling of microstructure formation in solidification processes. Int. Mater. Rev. 1989, 34, 93–124. [Google Scholar] [CrossRef]
- Dutil, Y.; Rousse, D.R.; Salah, N.B.; Lassue, S.; Zalewski, L. A review on phase-change materials: Mathematical modeling and simulations. Renew. Sustain. Energy Rev. 2011, 15, 112–130. [Google Scholar] [CrossRef]
- Verma, S.; Dewan, A. Solidification Modeling: Evolution, Benchmarks, Trends in Handling Turbulence, and Future Directions. Metall. Mater. Trans. B 2014, 45, 1456–1471. [Google Scholar] [CrossRef]
- Jaafar, M.A.; Rousse, D.R.; Gibout, S.; Bédécarrats, J.P. A review of dendritic growth during solidification: Mathematical modeling and numerical simulations. Renew. Sustain. Energy Rev. 2017, 74, 1064–1079. [Google Scholar] [CrossRef]
- Basu, B.; Date, A.W. Numerical modelling of melting and solidification problems—A review. Sadhana 1988, 13, 169–213. [Google Scholar] [CrossRef]
- Lacroix, M.; Voller, V.R. Finite difference solutions of solidification phase change problems: transformed versus fixed grids. Numer. Heat Transf. Part B Fundam. 1990, 17, 25–41. [Google Scholar] [CrossRef]
- Voller, V.R. Numerical Methods for Phase-Change Problems. In Handbook of Numerical Heat Transfer; Wiley-Blackwell: Hoboken, NJ, USA, 2009; Chapter 19; pp. 593–622. [Google Scholar] [CrossRef]
- Mencinger, J. Numerical simulation of melting in two-dimensional cavity using adaptive grid. J. Comput. Phys. 2004, 198, 243–264. [Google Scholar] [CrossRef]
- Hannoun, N.; Alexiades, V.; Mai, T.Z. A reference solution for phase change with convection. Int. J. Numer. Methods Fluids 2005, 48, 1283–1308. [Google Scholar] [CrossRef]
- Lan, C.; Liu, C.; Hsu, C. An Adaptive Finite Volume Method for Incompressible Heat Flow Problems in Solidification. J. Comput. Phys. 2002, 178, 464–497. [Google Scholar] [CrossRef]
- Worster, M.G. Convection in mushy layers. Annu. Rev. Fluid Mech. 1997, 29, 91–122. [Google Scholar] [CrossRef]
- Quéré, P.L.; Gobin, D. A note on possible flow instabilities in melting from the side. Int. J. Therm. Sci. 1999, 38, 595–600. [Google Scholar] [CrossRef]
- Morgan, K. A numerical analysis of freezing and melting with convection. Comput. Methods Appl. Mech. Eng. 1981, 28, 275–284. [Google Scholar] [CrossRef]
- Voller, V.; Prakash, C. A fixed grid numerical modelling methodology for convection-diffusion mushy region phase-change problems. Int. J. Heat Mass Transf. 1987, 30, 1709–1719. [Google Scholar] [CrossRef]
- Voller, V.R.; Cross, M.; Markatos, N.C. An enthalpy method for convection/diffusion phase change. Int. J. Numer. Methods Eng. 1987, 24, 271–284. [Google Scholar] [CrossRef]
- Brinkman, H.C. A calculation of the viscous force exerted by a flowing fluid on a dense swarm of particles. Flow Turbul. Combust. 1949, 1. [Google Scholar] [CrossRef]
- Poirier, D.R. Permeability for flow of interdendritic liquid in columnar-dendritic alloys. Metall. Trans. B 1987, 18, 245–255. [Google Scholar] [CrossRef]
- Singh, A.K.; Pardeshi, R.; Basu, B. Modelling of convection during solidification of metal and alloys. Sadhana 2001, 26, 139–162. [Google Scholar] [CrossRef]
- Fadl, M.; Eames, P.C. Numerical investigation of the influence of mushy zone parameter Amush on heat transfer characteristics in vertically and horizontally oriented thermal energy storage systems. Appl. Therm. Eng. 2019, 151, 90–99. [Google Scholar] [CrossRef]
- Hong, Y.; Ye, W.B.; Du, J.; Huang, S.M. Solid-liquid phase-change thermal storage and release behaviors in a rectangular cavity under the impacts of mushy region and low gravity. Int. J. Heat Mass Transf. 2019, 130, 1120–1132. [Google Scholar] [CrossRef]
- Rai, R.; Roy, G.G.; DebRoy, T. A computationally efficient model of convective heat transfer and solidification characteristics during keyhole mode laser welding. J. Appl. Phys. 2007, 101, 054909. [Google Scholar] [CrossRef]
- Zheng, Y.; Li, Q.; Zheng, Z.; Zhu, J.; Cao, P. Modeling the impact, flattening and solidification of a molten droplet on a solid substrate during plasma spraying. Appl. Surf. Sci. 2014, 317, 526–533. [Google Scholar] [CrossRef]
- Yang, X.H.; Tan, S.C.; Liu, J. Numerical investigation of the phase change process of low melting point metal. Int. J. Heat Mass Transf. 2016, 100, 899–907. [Google Scholar] [CrossRef]
- Kousksou, T.; Mahdaoui, M.; Ahmed, A.; Msaad, A.A. Melting over a wavy surface in a rectangular cavity heated from below. Energy 2014, 64, 212–219. [Google Scholar] [CrossRef]
- Karami, R.; Kamkari, B. Investigation of the effect of inclination angle on the melting enhancement of phase change material in finned latent heat thermal storage units. Appl. Therm. Eng. 2019, 146, 45–60. [Google Scholar] [CrossRef]
- Pan, C.; Charles, J.; Vermaak, N.; Romero, C.; Neti, S.; Zheng, Y.; Chen, C.H.; Bonner, R. Experimental, numerical and analytic study of unconstrained melting in a vertical cylinder with a focus on mushy region effects. Int. J. Heat Mass Transf. 2018, 124, 1015–1024. [Google Scholar] [CrossRef]
- Prieto, M.; González, B. Fluid flow and heat transfer in PCM panels arranged vertically and horizontally for application in heating systems. Renew. Energy 2016, 97, 331–343. [Google Scholar] [CrossRef]
- Kheirabadi, A.C.; Groulx, D. Simulating phase change heat transfer using COMSOL and Fluent: Effect of the mushy-zone constant. Comput. Therm. Sci. Int. J. 2015, 7, 427–440. [Google Scholar] [CrossRef]
- Hosseinizadeh, S.; Darzi, A.R.; Tan, F.; Khodadadi, J. Unconstrained melting inside a sphere. Int. J. Therm. Sci. 2013, 63, 55–64. [Google Scholar] [CrossRef]
- Shmueli, H.; Ziskind, G.; Letan, R. Melting in a vertical cylindrical tube: Numerical investigation and comparison with experiments. Int. J. Heat Mass Transf. 2010, 53, 4082–4091. [Google Scholar] [CrossRef]
- Arena, S.; Casti, E.; Gasia, J.; Cabeza, L.F.; Cau, G. Numerical simulation of a finned-tube LHTES system: Influence of the mushy zone constant on the phase change behaviour. Energy Procedia 2017, 126, 517–524. [Google Scholar] [CrossRef]
- Kumar, M.; Krishna, D.J. Influence of Mushy Zone Constant on Thermohydraulics of a PCM. Energy Procedia 2017, 109, 314–321. [Google Scholar] [CrossRef]
- Sattari, H.; Mohebbi, A.; Afsahi, M.; Yancheshme, A.A. CFD simulation of melting process of phase change materials (PCMs) in a spherical capsule. Int. J. Refrig. 2017, 73, 209–218. [Google Scholar] [CrossRef]
- Hu, Z.; Li, A.; Gao, R.; Yin, H. Effect of the length ratio on thermal energy storage in wedge-shaped enclosures. J. Therm. Anal. Calorim. 2014, 117, 807–816. [Google Scholar] [CrossRef]
- Vogel, J.; Felbinger, J.; Johnson, M. Natural convection in high temperature flat plate latent heat thermal energy storage systems. Appl. Energy 2016, 184, 184–196. [Google Scholar] [CrossRef] [Green Version]
- Hameter, M.; Walter, H. Influence of the Mushy Zone Constant on the Numerical Simulation of the Melting and Solidification Process of Phase Change Materials. In Computer Aided Chemical Engineering; Elsevier: Amsterdam, The Netherlands, 2016; pp. 439–444. [Google Scholar] [CrossRef]
- Tritton, D.J. Physical Fluid Dynamics, 1st ed.; Springer: Dordrecht, The Netherlands, 1977. [Google Scholar] [CrossRef]
- Voller, V.R.; Swaminathan, C.R.; Thomas, B.G. Fixed grid techniques for phase change problems: A review. Int. J. Numer. Methods Eng. 1990, 30, 875–898. [Google Scholar] [CrossRef]
- Voller, V.R.; Swaminathan, C.R. General source-based method for solidification phase change. Numer. Heat Transf. Part B Fundam. 1991, 19, 175–189. [Google Scholar] [CrossRef]
- Swaminathan, C.R.; Voller, V.R. A general enthalpy method for modeling solidification processes. Metall. Trans. B 1992, 23, 651–664. [Google Scholar] [CrossRef]
- Ferreira, I.L.; Voller, V.R.; Nestler, B.; Garcia, A. Two-dimensional numerical model for the analysis of macrosegregation during solidification. Comput. Mater. Sci. 2009, 46, 358–366. [Google Scholar] [CrossRef]
- Faraji, M.; Qarnia, H.E. Numerical study of melting in an enclosure with discrete protruding heat sources. Appl. Math. Model. 2010, 34, 1258–1275. [Google Scholar] [CrossRef]
- Bouabdallah, S.; Bessaih, R. Effect of magnetic field on 3D flow and heat transfer during solidification from a melt. Int. J. Heat Fluid Flow 2012, 37, 154–166. [Google Scholar] [CrossRef]
- Mahdaoui, M.; Kousksou, T.; Blancher, S.; Msaad, A.A.; Rhafiki, T.E.; Mouqallid, M. A numerical analysis of solid–liquid phase change heat transfer around a horizontal cylinder. Appl. Math. Model. 2014, 38, 1101–1110. [Google Scholar] [CrossRef]
- Farsani, R.Y.; Raisi, A.; Nadooshan, A.A.; Vanapalli, S. Does nanoparticles dispersed in a phase change material improve melting characteristics? Int. Commun. Heat Mass Transf. 2017, 89, 219–229. [Google Scholar] [CrossRef]
- Dannenhoffer, J., III; Baron, J. Grid adaptation for the 2-D Euler equations. In Proceedings of the 23rd Aerospace Sciences Meeting. American Institute of Aeronautics and Astronautics, Reno, NV, USA, 14–17 January 1985. [Google Scholar] [CrossRef]
- Issa, R. Solution of the implicitly discretised fluid flow equations by operator-splitting. J. Comput. Phys. 1986, 62, 40–65. [Google Scholar] [CrossRef]
- Patankar, S.V. Numerical Heat Transfer and Fluid Flow, 1st ed.; Taylor & Francis Inc.: Leiden, The Netherlands, 1980. [Google Scholar]
- Voller, V. Development and application of a heat balance integral method for analysis of metallurgical solidification. Appl. Math. Model. 1989, 13, 3–11. [Google Scholar] [CrossRef]
- Kumar, L.; Manjunath, B.; Patel, R.; Markandeya, S.; Agrawal, R.; Agrawal, A.; Kashyap, Y.; Sarkar, P.; Sinha, A.; Iyer, K.; et al. Experimental investigations on melting of lead in a cuboid with constant heat flux boundary condition using thermal neutron radiography. Int. J. Therm. Sci. 2012, 61, 15–27. [Google Scholar] [CrossRef]
- Gau, C.; Viskanta, R. Melting and Solidification of a Pure Metal on a Vertical Wall. J. Heat Transf. 1986, 108, 174. [Google Scholar] [CrossRef]
- Hannoun, N.; Alexiades, V.; Mai, T.Z. Resolving the controversy over tin and gallium melting in a rectangular cavity heated from the side. Numer. Heat Transf. Part B Fundam. 2003, 44, 253–276. [Google Scholar] [CrossRef] [Green Version]
- Lee, Y.; Korpela, S.A. Multicellular natural convection in a vertical slot. J. Fluid Mech. 1983, 126, 91. [Google Scholar] [CrossRef]
- Dantzig, J.A. Modelling liquid-solid phase changes with melt convection. Int. J. Numer. Methods Eng. 1989, 28, 1769–1785. [Google Scholar] [CrossRef]
- Cerimele, M.; Mansutti, D.; Pistella, F. Numerical modelling of liquid/solid phase transitions: Analysis of a gallium melting test. Comput. Fluids 2002, 31, 437–451. [Google Scholar] [CrossRef]
- Schroeder, P.W.; Lube, G. Stabilised dG-FEM for incompressible natural convection flows with boundary and moving interior layers on non-adapted meshes. J. Comput. Phys. 2017, 335, 760–779. [Google Scholar] [CrossRef] [Green Version]
- Vogel, J.; Thess, A. Validation of a numerical model with a benchmark experiment for melting governed by natural convection in latent thermal energy storage. Appl. Therm. Eng. 2019, 148, 147–159. [Google Scholar] [CrossRef]
- Pitscheneder, W.; Debroy, T.; Mundra, K.; Ebner, R. Role of sulfur and processing variables on the temporal evolution of weld pool geometry during multikilowatt laser beam welding of steels. Weld. J. 1996, 75, 71–80. [Google Scholar]
- Yan, J.; Yan, W.; Lin, S.; Wagner, G. A fully coupled finite element formulation for liquid–solid–gas thermo-fluid flow with melting and solidification. Comput. Methods Appl. Mech. Eng. 2018, 336, 444–470. [Google Scholar] [CrossRef]
- Saldi, Z.; Kidess, A.; Kenjereš, S.; Zhao, C.; Richardson, I.; Kleijn, C. Effect of enhanced heat and mass transport and flow reversal during cool down on weld pool shapes in laser spot welding of steel. Int. J. Heat Mass Transf. 2013, 66, 879–888. [Google Scholar] [CrossRef]
- Sahoo, P.; Debroy, T.; McNallan, M.J. Surface tension of binary metal—Surface active solute systems under conditions relevant to welding metallurgy. Metall. Trans. B 1988, 19, 483–491. [Google Scholar] [CrossRef]





| Property | Value | Unit |
|---|---|---|
| Density | ||
| Specific heat capacity | ||
| Thermal conductivity k | 1, 10 and 100 | |
| Dynamic viscosity | ||
| Latent heat of fusion | ||
| Thermal expansion coefficient | ||
| Melting temperature | ||
| Melting-temperature range | 0, 5, 10, 25 and 50 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ebrahimi, A.; Kleijn, C.R.; Richardson, I.M. Sensitivity of Numerical Predictions to the Permeability Coefficient in Simulations of Melting and Solidification Using the Enthalpy-Porosity Method. Energies 2019, 12, 4360. https://doi.org/10.3390/en12224360
Ebrahimi A, Kleijn CR, Richardson IM. Sensitivity of Numerical Predictions to the Permeability Coefficient in Simulations of Melting and Solidification Using the Enthalpy-Porosity Method. Energies. 2019; 12(22):4360. https://doi.org/10.3390/en12224360
Chicago/Turabian StyleEbrahimi, Amin, Chris R. Kleijn, and Ian M. Richardson. 2019. "Sensitivity of Numerical Predictions to the Permeability Coefficient in Simulations of Melting and Solidification Using the Enthalpy-Porosity Method" Energies 12, no. 22: 4360. https://doi.org/10.3390/en12224360
APA StyleEbrahimi, A., Kleijn, C. R., & Richardson, I. M. (2019). Sensitivity of Numerical Predictions to the Permeability Coefficient in Simulations of Melting and Solidification Using the Enthalpy-Porosity Method. Energies, 12(22), 4360. https://doi.org/10.3390/en12224360






