An Advanced Exergoeconomic Comparison of CO2-Based Transcritical Refrigeration Cycles
Abstract
:1. Introduction
2. System Configurations and Assumptions
2.1. Description of the Configurations
- (1)
- The compressor, ejector, and turbine have fixed isentropic efficiencies;
- (2)
- The refrigerant at the evaporator outlet is saturated vapor;
- (3)
- The pressure drops and heat losses in the gas cooler and evaporator, as well as in the piping systems, are negligible;
- (4)
- A constant cooling capacity for the system.
2.2. Mathematical Models
2.2.1. Exergy Model
2.2.2. Exergoeconomic Model
2.2.3. Environmental Model
3. Results
3.1. Exergy Analysis
3.2. Exergoeconomic Analysis
4. Conclusions
- The highest exergy efficiency was achieved for the ejector cycle, reaching an average value of 33%. As expected, the basic cycle had the lowest exergy efficiency. In the exergy efficiency behavior, it was observed that the evaporation temperature was the variable that least influenced the measurements;
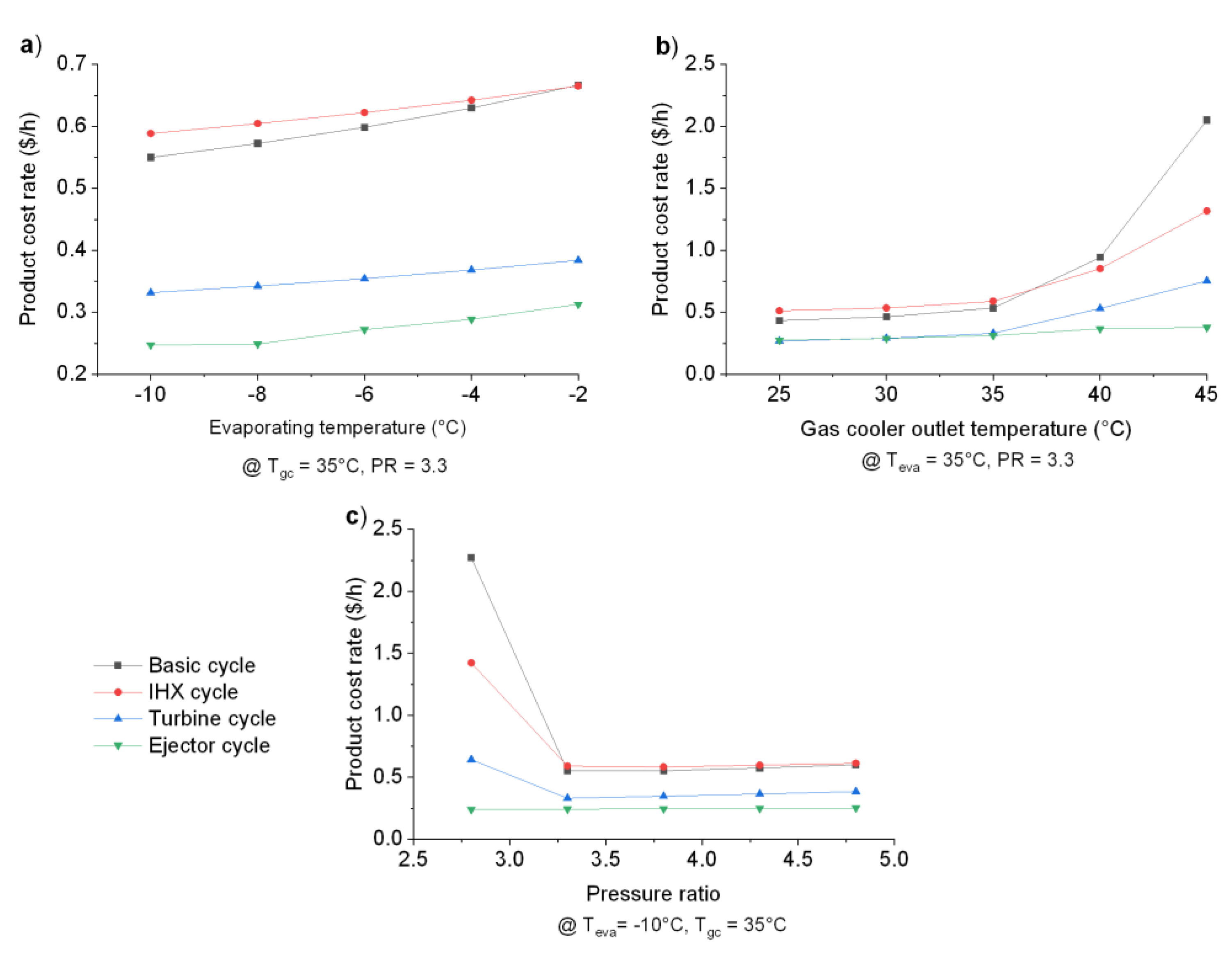
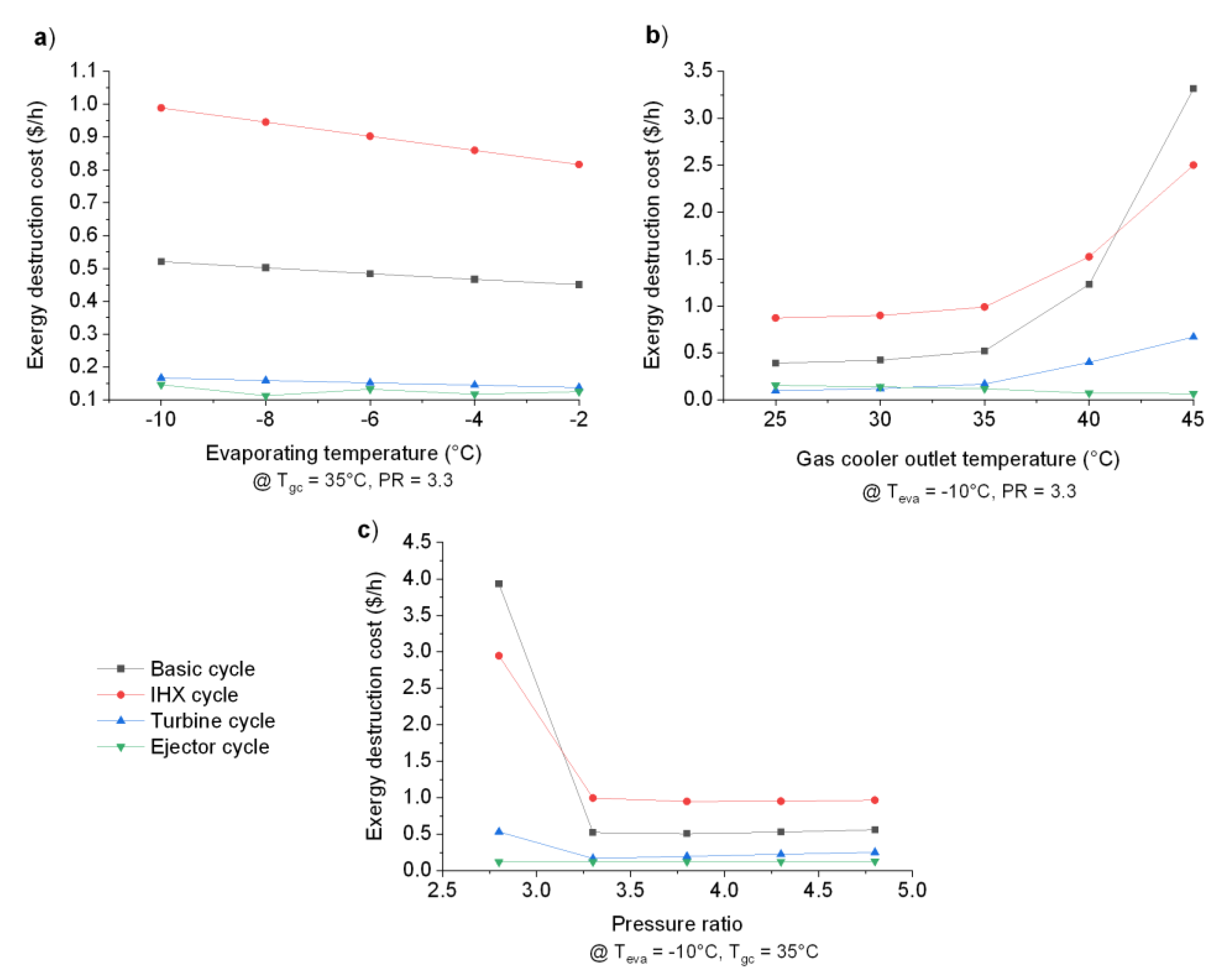
- Regarding the cost rate product variation, the basic and IHX cycles were the configurations that presented the highest cooling costs. For example, for an evaporation temperature of −2 °C, costs of 0.65 $/h were observed. This same behavior was reflected in the exergy destruction cost;
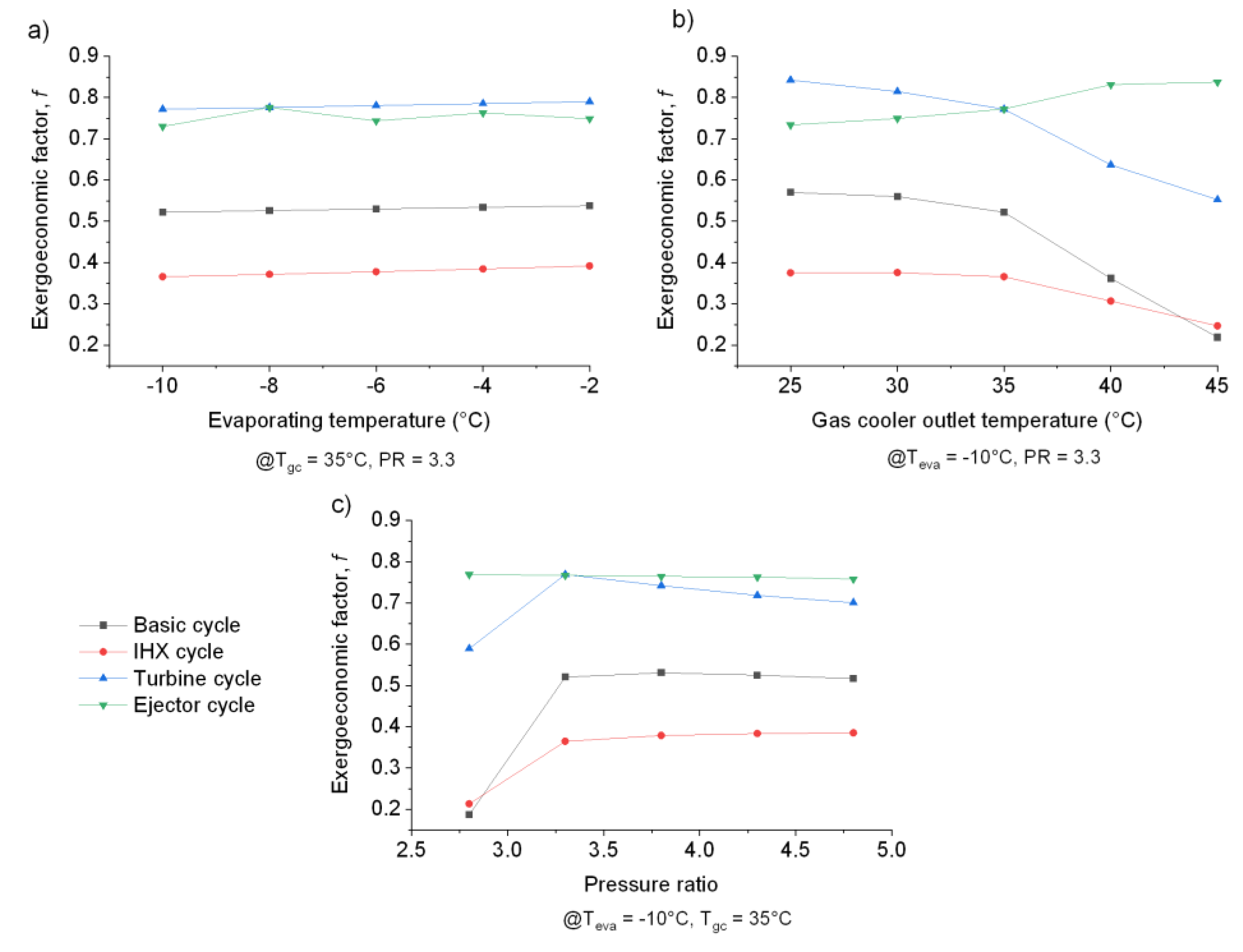
- With respect to the exergoeconomic factor, the cycles with the ejector and turbine were those that showed the greatest factor values, ranging from 0.7 to 0.8. The lowest average value of 0.53 on was shown by the IHX cycle;
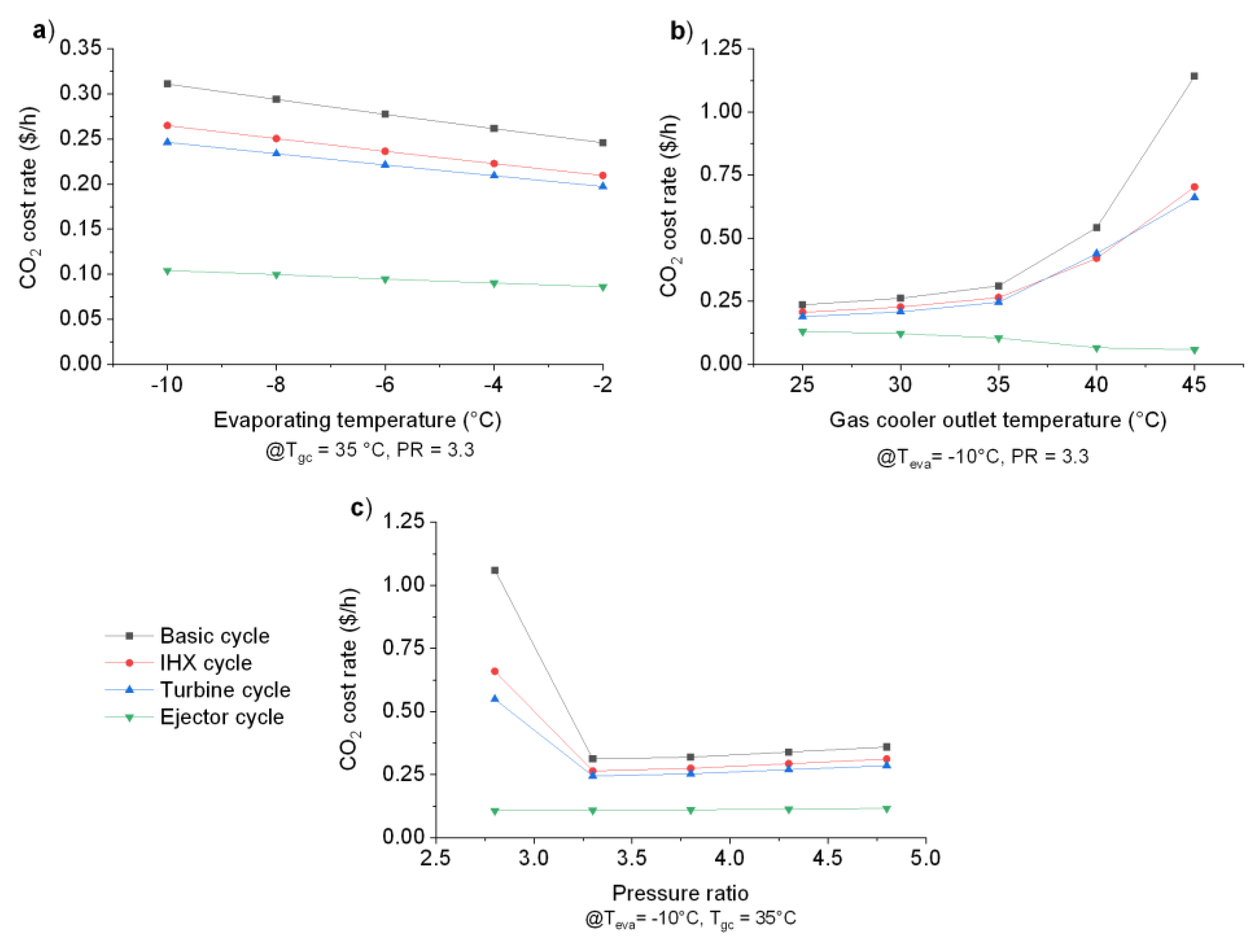
- The variation in the penalty cost was another of the behaviors analyzed; in this case, the basic cycle was the configuration with the most CO2 emissions emitted due to energy consumption. For high gas cooler outlet temperature conditions (45 °C), a value of 1.12 $/h was obtained, whereas for the same conditions, the cycle with an ejector gave a value of 0.05 $/h;
- Finally, it can be concluded that according to the results discussed here, the transcritical cooling cycle with the ejector turned out to be the most efficient method from an exergo-economic point of view. However, it still requires further technological development to decrease its production cost.
Author Contributions
Funding
Conflicts of Interest
References
- Pérez-García, V.; Rodríguez-Muñoz, J.L.; Ramírez-Minguela, J.J.; Belman-Flores, J.M.; Méndez-Díaz, S. Comparative analysis of energy improvements in single transcritical cycle in refrigeration mode. Appl. Therm. Eng. 2016, 99, 866–872. [Google Scholar] [CrossRef]
- Llopis, R.; Cabello, R.; Sánchez, D.; Torrella, E. Energy improvements of CO2 transcritical refrigeration cycles using dedicated mechanical subcooling. Int. J. Refrig. 2015, 55, 129–141. [Google Scholar] [CrossRef] [Green Version]
- Fazelpour, F. Energetic and exergetic analysis of carbon dioxide transcritical refrigeration systems for hot climates. Thermal Science 2015, 19, 905–914. [Google Scholar] [CrossRef]
- Elbel, S.; Hrnjak, P. Experimental validation of a prototype ejector designed to reduce throttling losses encountered in transcritical R744 system operation. Int. J. Refrig. 2008, 31, 411–422. [Google Scholar] [CrossRef]
- Kursad, E.H.; Nagihan, B. Performance characteristics of ejector expander transcritical CO2 refrigeration cycle. Proc. Inst. Mech. Eng. Part A J. Power Energy 2014, 226, 623–635. [Google Scholar]
- Brian, T.A.; Sumathy, K. Transcritical carbon dioxide heat pump systems: A Review. Renew. Sustain. Energy Rev. 2011, 15, 4013–4029. [Google Scholar]
- Elbel, S.; Lawrence, N. Review of recent developments in advanced ejector technology. Int. J. Refrig. 2016, 62, 1–18. [Google Scholar] [CrossRef]
- Yang, J.L.; Ma, Y.T.; Li, M.X.; Guan, H.Q. Exergy analysis of transcritical carbon dioxide refrigeration cycle with an expander. Energy 2005, 30, 1162–1175. [Google Scholar] [CrossRef]
- Murthy, A.A.; Subiantoro, A.; Norris, S.; Fukuta, M. A review of expanders and their performance in vapour compression refrigeration systems. Int. J. Refrig. 2019, 106, 427–446. [Google Scholar] [CrossRef]
- Boewe, D.E.; Bullard, C.W.; Yin, J.M.; Hrnjak, H.S. Contribution of Internal Heat Exchanger to Transcritical R-744 Cycle Performance. HVAC&R Res. 2011, 7, 155–168. [Google Scholar]
- Wang, Z.; Gong, Y.; Wu, X.H.; Zhang, W.H.; Lu, Y.L. Thermodynamic analysis and experimental research of transcritical CO2 cycle with internal heat exchanger and dual expansion. Int. Air Cond. Refrig. 2013, 21, 1350005. [Google Scholar] [CrossRef]
- Nilesh, P.; Dileep, K.G.; Mani, S.D. Experimental investigation of a CO2 trans-critical cycle with IHX for chiller application and its energetic and exergetic evaluation in warm climate. Appl. Therm. Eng. 2018, 136, 617–632. [Google Scholar]
- Mehrdad, S.; Dadsetani, R.; Amiriyoon, A.; Leon, A.S.; Safaei, M.R.; Goodarzi, M. Exergo-economic optimization of organic rankine cycle for saving of thermal energy in a sample power plant by using of strength pareto evolutionary algorithm II. Processes 2020, 8, 264. [Google Scholar] [CrossRef] [Green Version]
- Dadsetani, R.; Sheikhzadeh, G.A.; Safaei, M.R.; Alnaqi, A.A.; Amiriyoon, A. Exergoeconomic optimization of liquefying cycle for noble gas argon. Heat Mass Transf. 2019, 55, 1995–2007. [Google Scholar] [CrossRef]
- Rangel-Hernández, V.H.; Belman-Flores, J.M.; Rodríguez-Valderrama, D.A.; Pardo-Cely, D.; Rodríguez-Muñoz, A.P.; Ramírez-Minguela, J.J. Exergoeconomic performance comparison of R1234yf as a drop-in replacement for R134a in a domestic refrigerator. Int. J. Refrig. 2019, 100, 113–123. [Google Scholar] [CrossRef]
- Fazelpour, F.; Morosuk, T. Exergoeconomic analysis of carbon dioxide transcritical refrigeration machines. Int. J. Refrig. 2014, 38, 128–139. [Google Scholar] [CrossRef]
- Nemati, A.; Nami, H.; Yari, M. A comparison of refrigerants in a two-stage ejector-expansion transcritical refrigeration cycle based on exergoeconomic and environmental analysis. Int. J. Refrig. 2017, 84, 139–150. [Google Scholar] [CrossRef]
- Megdouli, K.; Sahli, H.; Tashtoush, B.M.; Nahdi, E.; Kairouani, L. Theoretical research of the performance of a novel enhanced transcritical CO2 refrigeration cycle for power and cold generation. Energy Convers. Manag. 2019, 201, 112139. [Google Scholar] [CrossRef]
- Gullo, P.; Cortella, G. Comparative exergoeconomic analysis of various transcritical R744 commercial refrigeration systems. In Proceedings of the ECOS 2016, Portorož, Slovenia, 19–23 June 2016. [Google Scholar]
- Nilesh, P.; Dileep, K.G.; Mani, S.D. Energetic and economic analysis of trans-critical CO2 booster system for refrigeration in warm climatic condition. Int. J. Refrig. 2017, 80, 182–196. [Google Scholar]
- Liao, S.M.; Zhao, T.S.; Jakobsen, A. A correlation of optimal rejection pressures in transcritical carbon dioxide cycles. Appl. Therm. Eng. 2000, 20, 831–841. [Google Scholar] [CrossRef]
- Li, D.; Groll, E.A. Transcritical CO2 refrigeration cycle with ejector expansion device. Int. J. Refrig. 2005, 28, 766–773. [Google Scholar] [CrossRef] [Green Version]
- Sarkar, J.; Bhattacharyya, S.; Ram Gopal, M. Optimization of a transcritical CO2 heat pump cycle for simultaneous cooling and heating applications. Int. J. Refrig. 2004, 27, 830–838. [Google Scholar] [CrossRef]
- Huashan, L.; Fei, C.; Xianbiao, B.; Lingbao, W.; Xianlong, W. Performance characteristics of R1234yf ejector-expansion refrigeration cycle. Appl. Energy 2014, 121, 96–103. [Google Scholar]
- Bejan, A.; Tsatsaronis, G.; Moran, M. Thermal Design and Optimization; John Wiley and Sons: New York, NY, USA, 1996. [Google Scholar]
- Nemati, A.; Nami, H.; Yari, M.; Ranjbar, F.; Kolvir, H.R. Development of an exergoeconomic model for analysis and multi-objective optimization of a thermoelectric heat-pump. Energy Convers. Manag. 2016, 130, 1–3. [Google Scholar] [CrossRef]
- Ghaebi, H.; Parikhani, T.; Rostamzadeh, H. A novel trigeneration system using geothermal heat source and liquiefied natural gas cold energy recovery: Energy, exergy and exergoeconomic analysis. Renew. Energy 2018, 119, 513–527. [Google Scholar] [CrossRef]
- Ghaebi, H.; Parikhani, T.; Rostamzadeh, H. Energy, exergy and thermoeconomic analysis of a novel combined cooling and power system using low-temperature heat source and LNG cold energy recovery. Energy Convers. Manag. 2017, 50, 678–692. [Google Scholar] [CrossRef]
- Parikhani, T.; Gholizadeh, T.; Ghaebi, H.; Sadat, S.M.S.; Sarabi, M. Exergoeconomic optimization of a novel multigeneration system driven by geothermal heat source and liquefied natural gas cold energy recovery. J. Clean. Prod. 2019, 209, 550–571. [Google Scholar] [CrossRef]
- Tsatsaronis, G. Thermoeconomic analysis and optimization of energy systems. Prog. Energy Combust. Sci. 1993, 19, 227–257. [Google Scholar] [CrossRef]
- Misra, R.D.; Sahoo, P.K.; Sahoo, S.; Gupta, A. Thermoeconomic optimization of a single effect water/LiBr vapour absorption refrigeration system. Int. J. Refrig. 2003, 26, 158–169. [Google Scholar] [CrossRef]
- Farschi, L.G.; Mahmoudi, S.S.; Rosen, M.A. Exergoeconomic comparison of double effect and combined ejector-double effect absorption refrigeration systems. Appl. Energy 2013, 103, 700–711. [Google Scholar] [CrossRef]
- Mosafia, A.H.; Farschi, L.G.; Ferreira, C.I.; Rosen, M.A. Exergoeconomic and environmental analysis of CO2/NH3 cascade refrigeration systems equipped with different types of flash tank intercoolers. Energy Convers. Manag. 2017, 117, 442–453. [Google Scholar] [CrossRef] [Green Version]
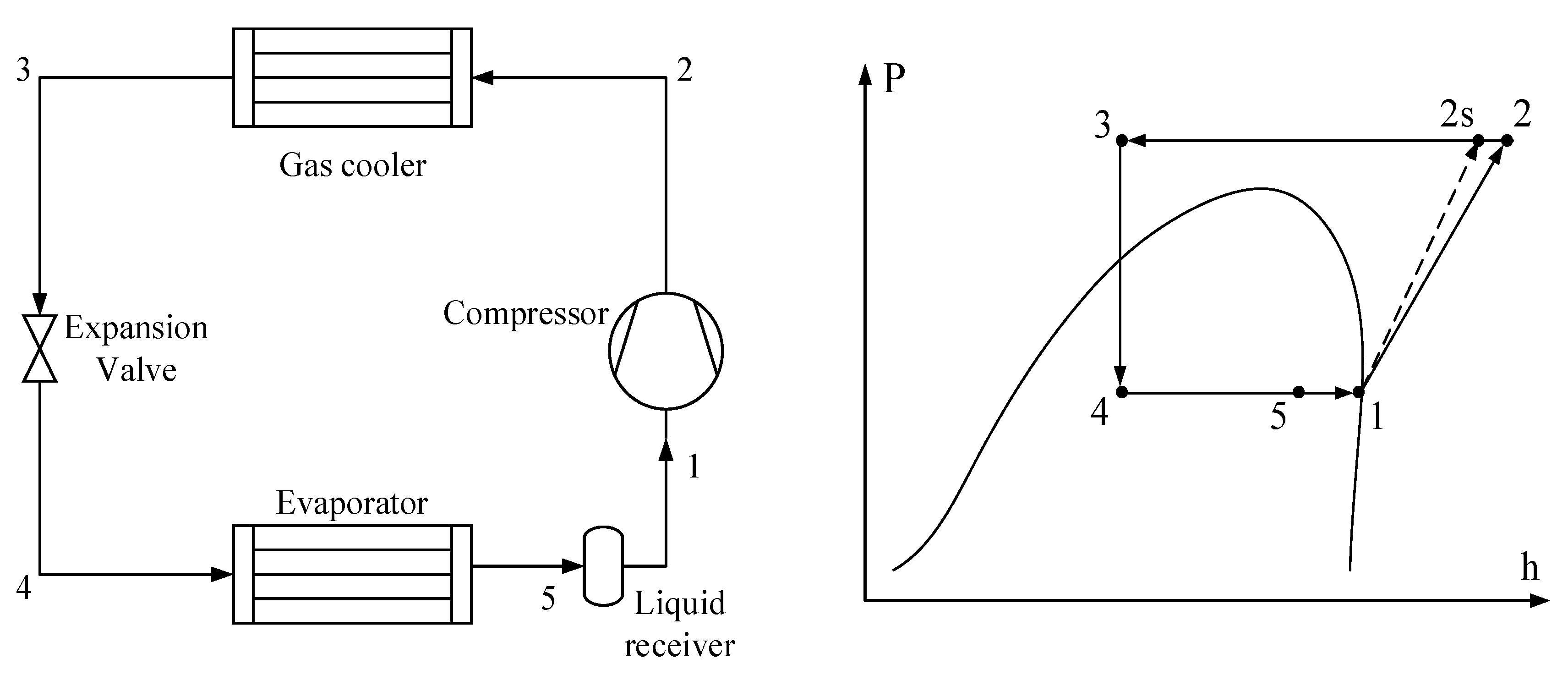
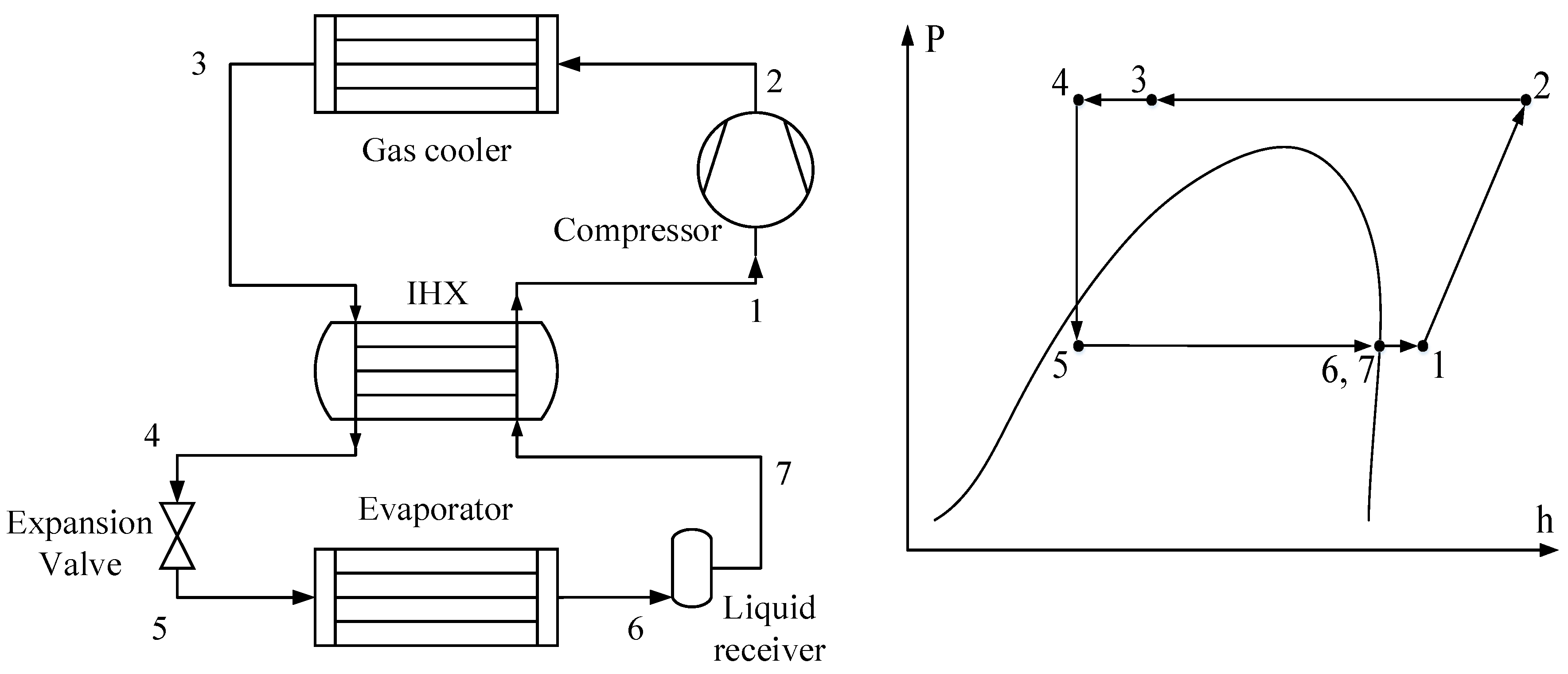
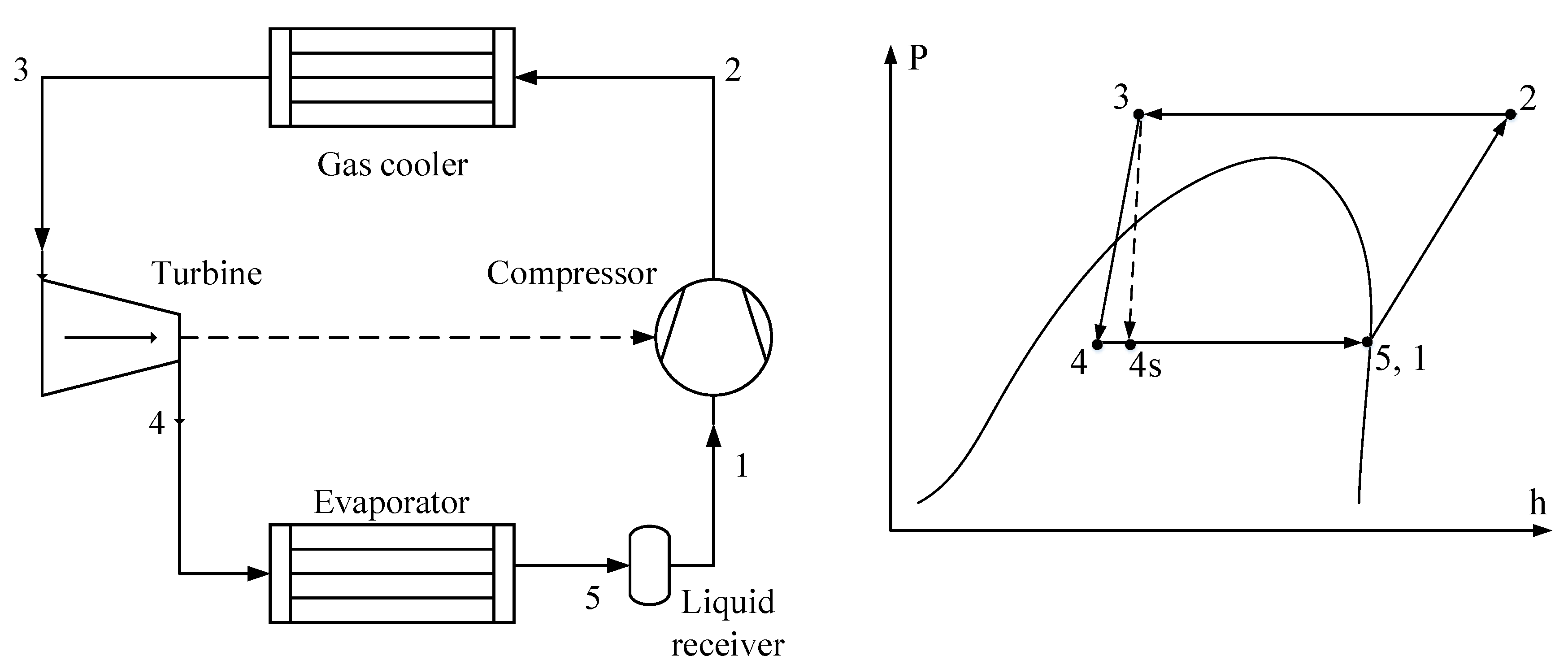
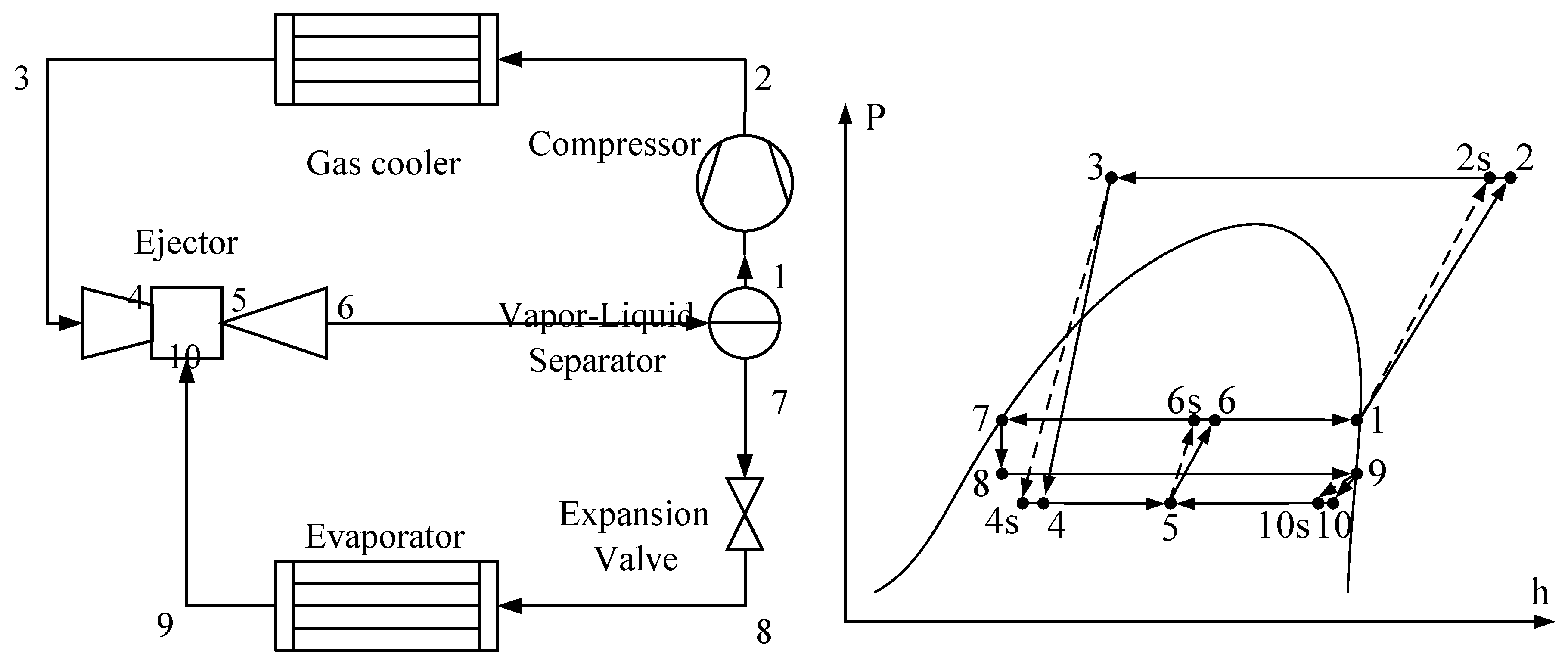

| Parameter | Value |
|---|---|
| Evaporating temperature | 263 K |
| Gas cooler temperature | 308 K |
| Isentropic efficiency of the compressor | [21] |
| Isentropic efficiency of the turbine | 0.75 |
| Internal heat exchanger effectiveness | 0.5 |
| Nozzle efficiency | 0.85 |
| Diffuser efficiency | 0.85 |
| Cooling capacity | 7 kW |
| Pressure drop in the suction nozzle | 0.03 MPa [22] |
| Environmental state | 298 K 1.01 MPa |
| Equipment | Cost Function Equations | Reference Values | Ref. |
|---|---|---|---|
| Compressor | [31] | ||
| Evaporator | ICCref = 16000 Aref = 100 m2 | [32] | |
| Gas cooler | ICCref = 8000 Aref = 100 m2 | [32] | |
| Internal heat exchanger | ICCref = 12000 Aref = 100 m2 | [32] | |
| Ejector | |||
| Expansion valve |
| Component | Exergy Analysis | Exergoeconomic Analysis |
|---|---|---|
| Compressor | ||
| Gas cooler | ||
| Expansion valve | , | |
| Evaporator | ||
| Ejector | ||
| aTurbine | ||
| Internal heat exchanger | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Belman-Flores, J.M.; Rangel-Hernández, V.H.; Pérez-García, V.; Zaleta-Aguilar, A.; Fang, Q.; Méndez-Méndez, D. An Advanced Exergoeconomic Comparison of CO2-Based Transcritical Refrigeration Cycles. Energies 2020, 13, 6454. https://doi.org/10.3390/en13236454
Belman-Flores JM, Rangel-Hernández VH, Pérez-García V, Zaleta-Aguilar A, Fang Q, Méndez-Méndez D. An Advanced Exergoeconomic Comparison of CO2-Based Transcritical Refrigeration Cycles. Energies. 2020; 13(23):6454. https://doi.org/10.3390/en13236454
Chicago/Turabian StyleBelman-Flores, J. M., V. H. Rangel-Hernández, V. Pérez-García, A. Zaleta-Aguilar, Qingping Fang, and D. Méndez-Méndez. 2020. "An Advanced Exergoeconomic Comparison of CO2-Based Transcritical Refrigeration Cycles" Energies 13, no. 23: 6454. https://doi.org/10.3390/en13236454
APA StyleBelman-Flores, J. M., Rangel-Hernández, V. H., Pérez-García, V., Zaleta-Aguilar, A., Fang, Q., & Méndez-Méndez, D. (2020). An Advanced Exergoeconomic Comparison of CO2-Based Transcritical Refrigeration Cycles. Energies, 13(23), 6454. https://doi.org/10.3390/en13236454









