Comparison of Optimized and Conventional Models of Passive Solar Greenhouse—Case Study: The Indoor Air Temperature, Irradiation, and Energy Demand
Abstract
:1. Introduction
2. Materials and Methods
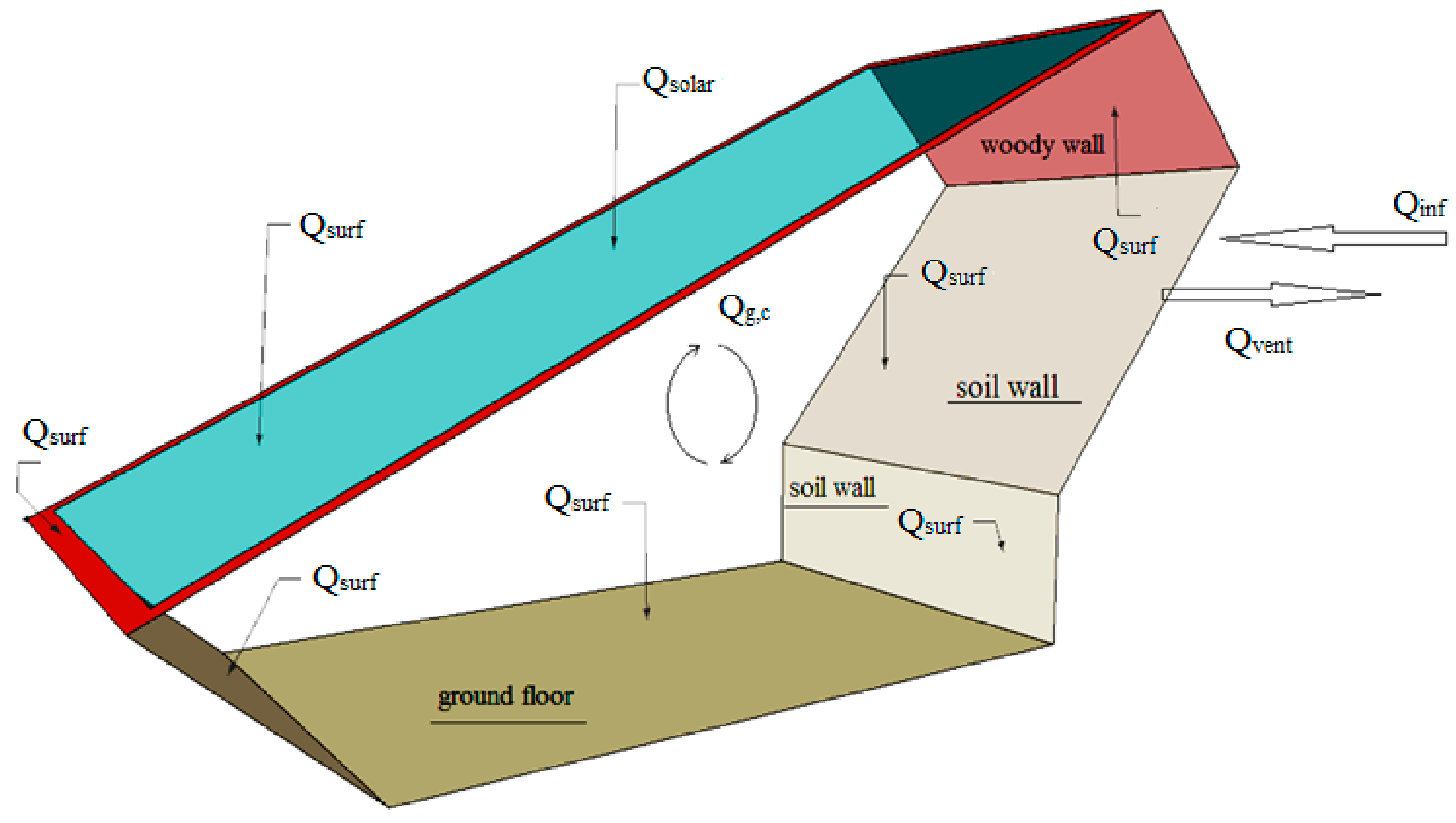
2.1. Design and Modeling
2.2. Types of Wall
2.3. Math Calculation
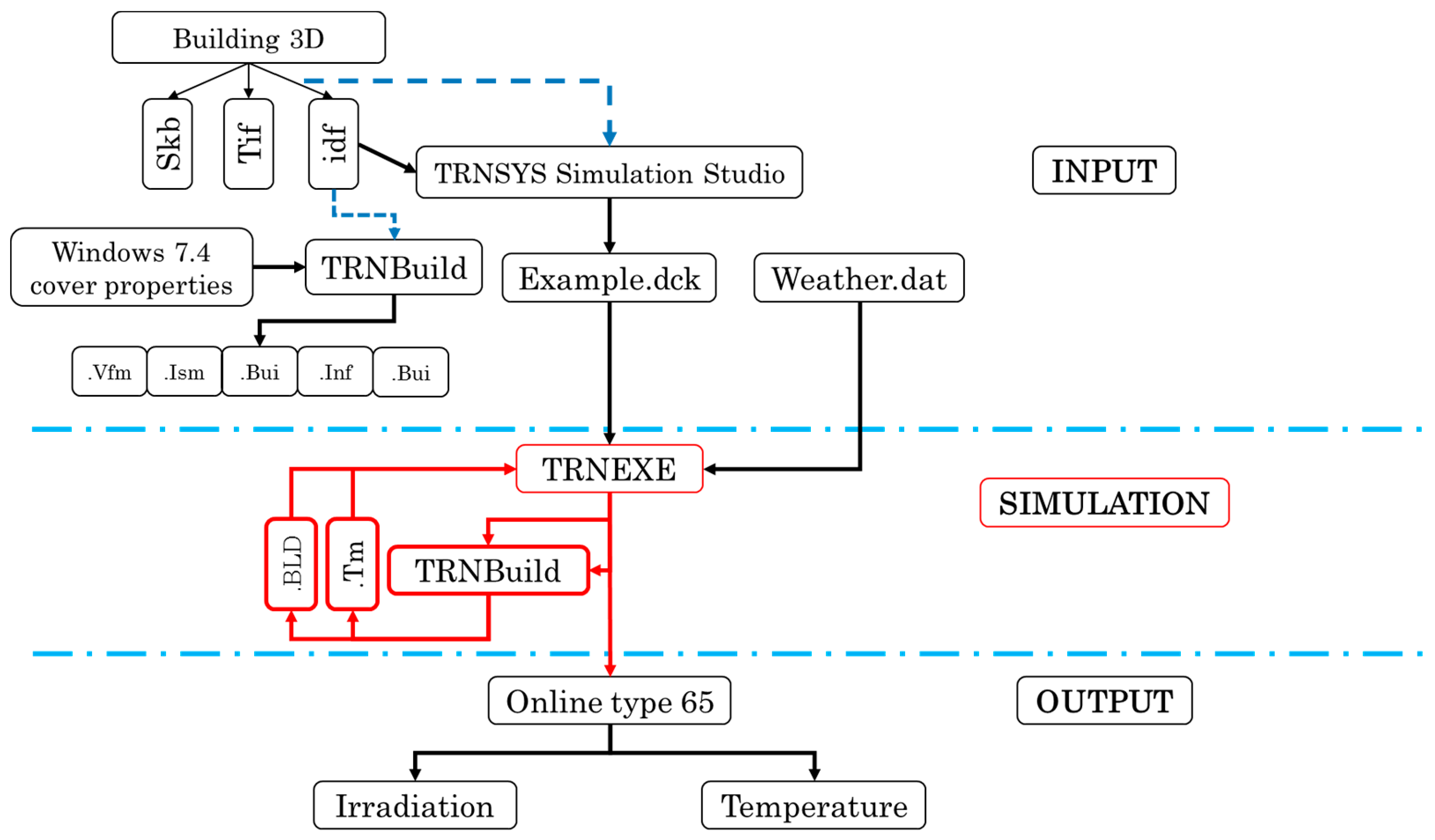
2.4. Model of Energy Demand, Temperature, and Radiation Calculation
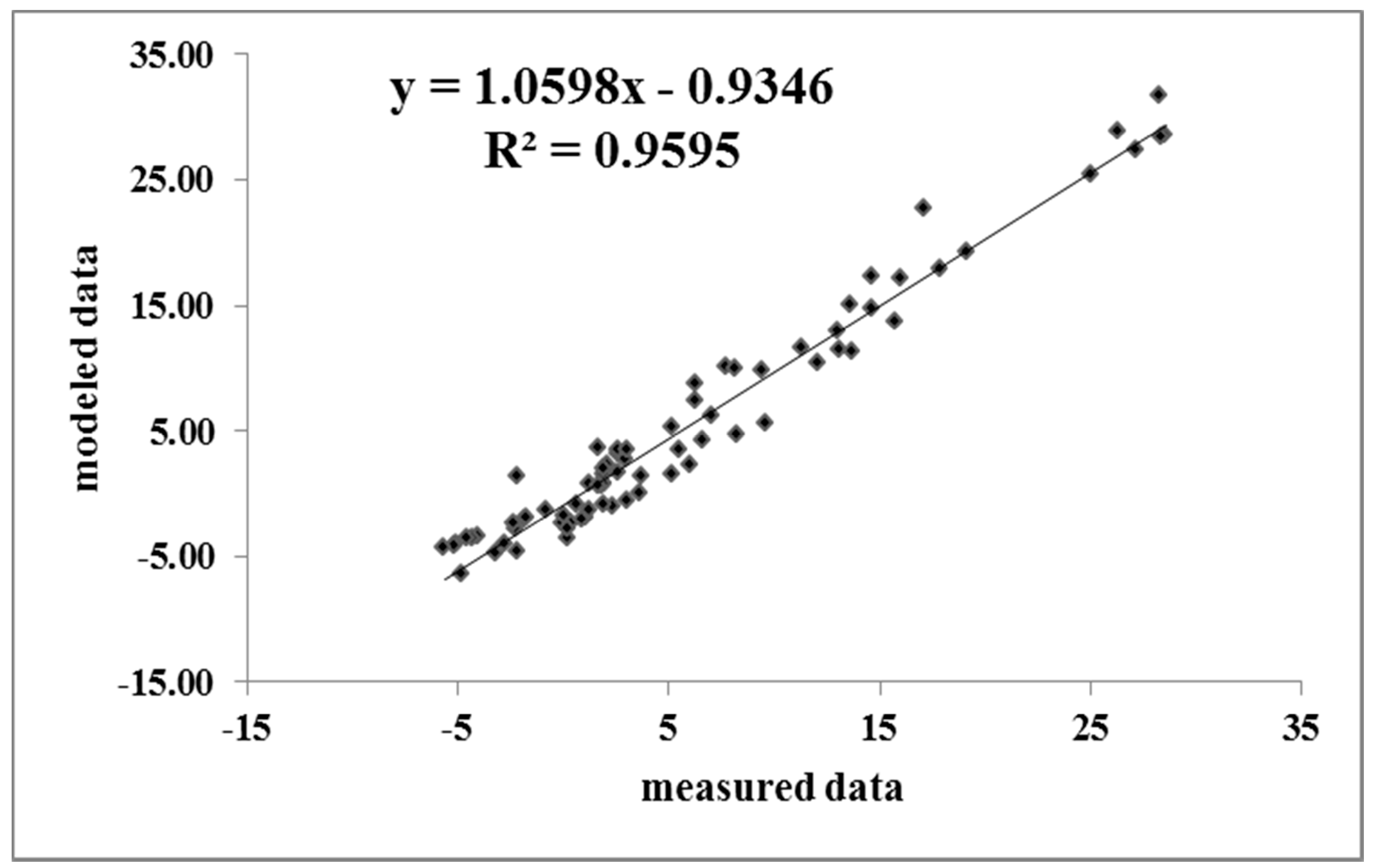
2.5. Validation
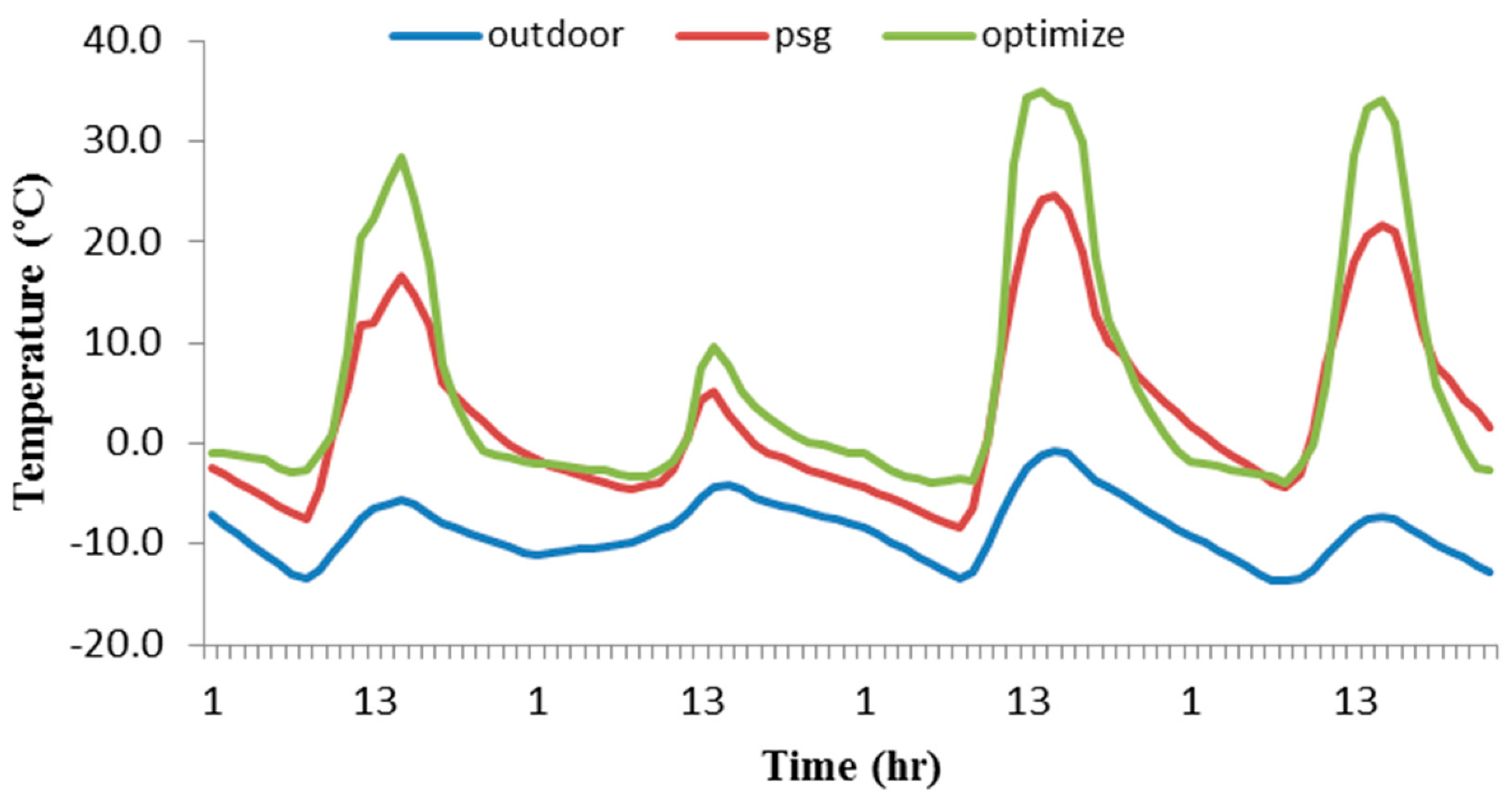
3. Results and Discussion
4. Conclusions
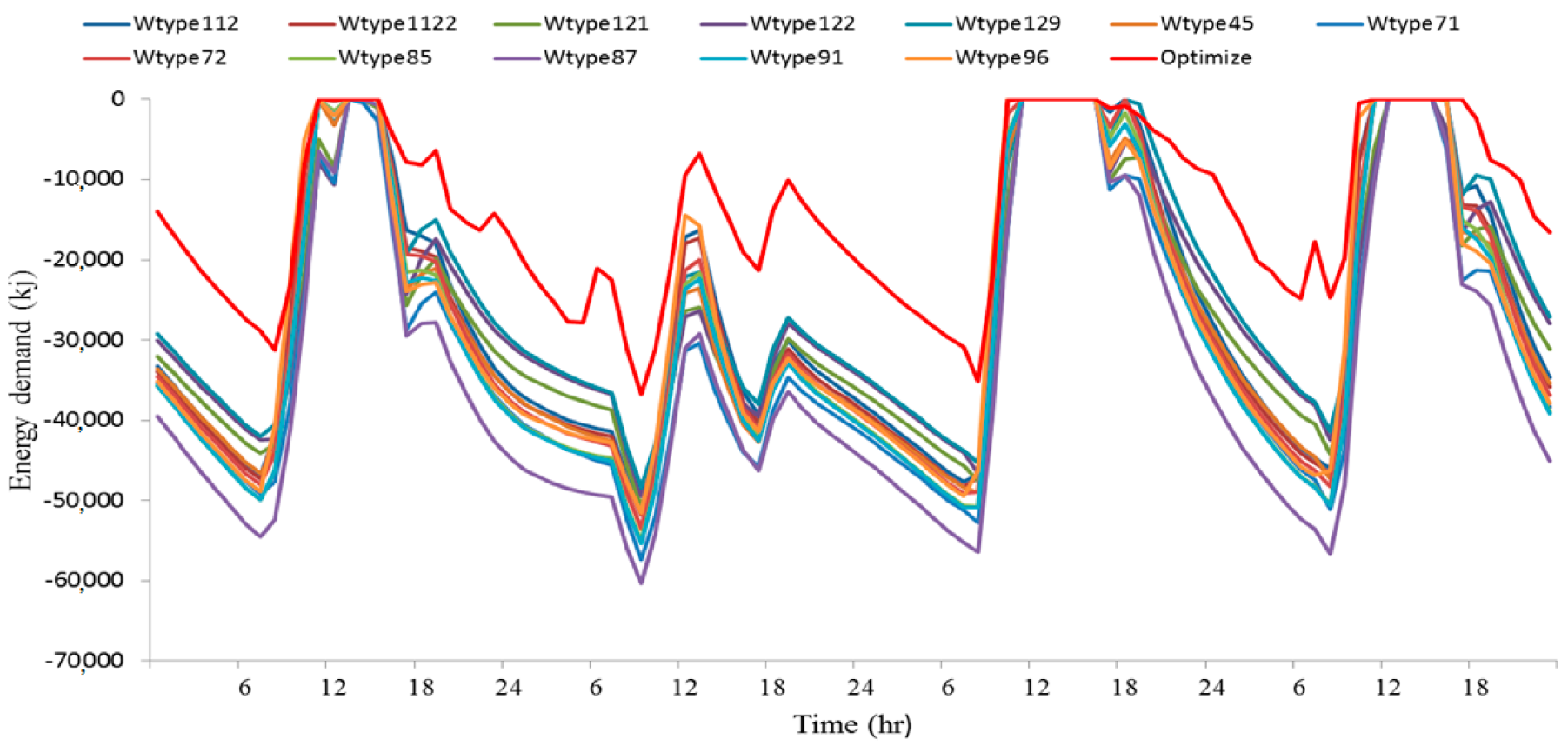
- In conventional passive solar greenhouses, the greenhouse heating demand is seen as minimum in walls of Wtype45 (50-mm insulation with 200-mm clay tile) and maximum in Wtype87 (100-mm lightweight concrete block).
- In the optimized model, the north wall and parts of the south, east, and west walls were constructed with soil that decreased heat demand in comparison with Wtype45 (50-mm insulation with 200-mm clay tile).
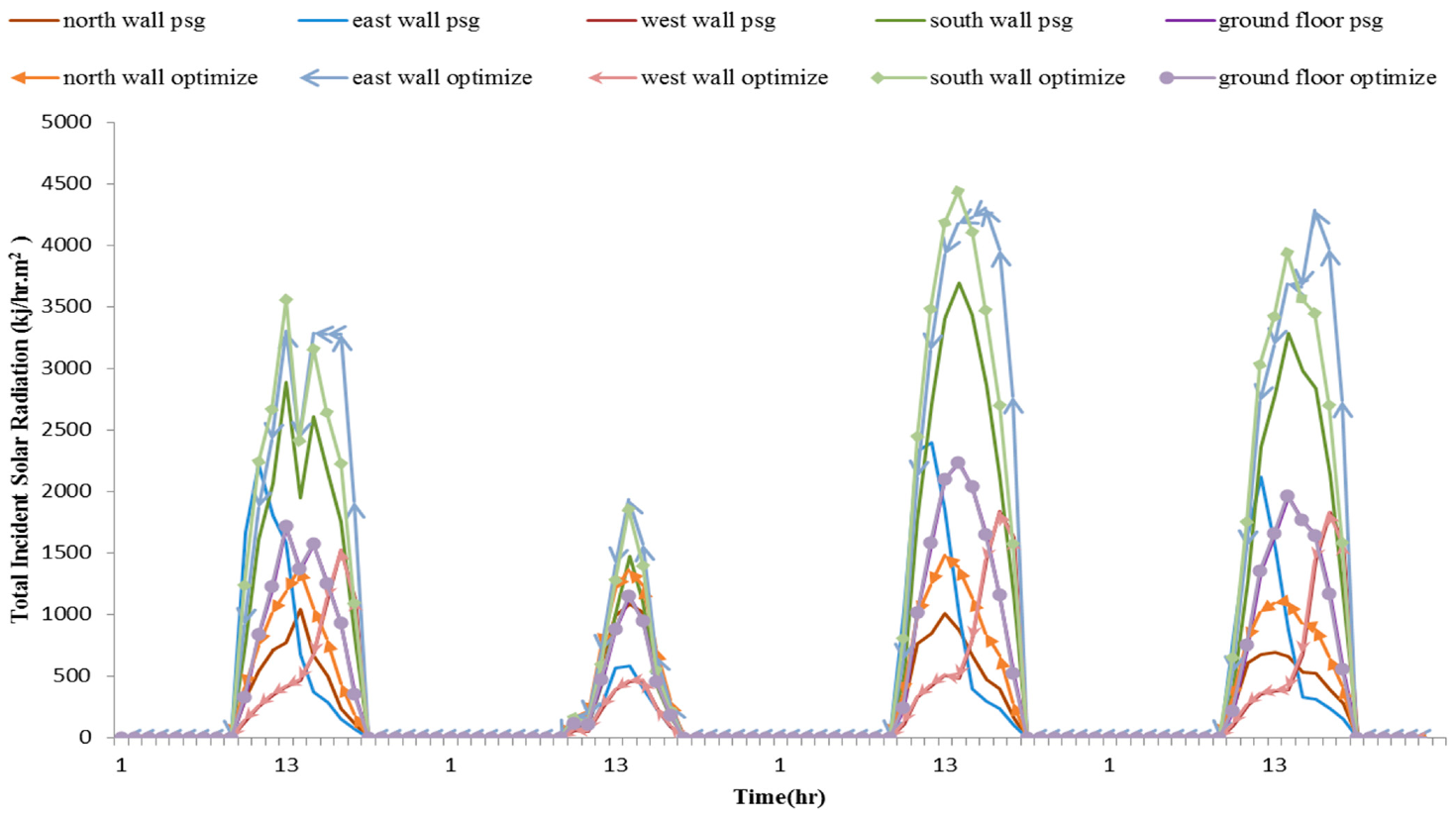
- In the optimized model, irradiation in the east and south walls was more than conventional passive solar greenhouses. In addition, Indoor air temperature in the optimized model was more than the conventional models on sunny days in the winter.
- One of the biggest sources of global warming comes from huge energy consumption in agriculture field. This provides a challenge for researchers around the world to find new ways for energy consumption reduction.
- A current study revealed that the present optimized greenhouse model has lower energy consumption compared to the other types of greenhouses.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Nomenclature
| TRNSYS | TRaNsient System Simulation |
| 3-D | three dimensions |
| Wtype112 | 150 mm insulation with 50 mm wood |
| Wtype121 | 85 mm insulation with 200 mm common brick |
| Wtype122 | 200 mm common brick with 50 mm insulation |
| Wtype129 | Face brick and 200 clay tile with 50 mm insulation |
| Wtype45 | 50 mm insulation with 200 mm clay tile |
| Wtype71 | 200 mm clay tile with air space |
| Wtype72 | 200 mm clay |
| Wtype85 | 100 mm lightweight concrete block and 25 mm insulation |
| Wtype87 | 100 mm lightweight concrete block |
| Wtype91 | 100 mm clay tile and 25 mm insulation |
| Wtype96 | Sheet metal with 75 mm insulation |
| i | net heat gain |
| surf,i | total heat loss from the surface |
| inf,i | infiltration gains |
| vent,i | ventilation heat gain |
| gc,i | internal convective heat gain |
| cplg,i | convective heat gain |
| rate of attic infiltration | |
| density | |
| Cp | fluid specific heat |
| Toutside,i | Outside temperature |
| Tair | Air temperature |
| Tventilation,i | temperature of ventilation |
| TZone,i | zone temperature |
| r,w | radiative gains for the wall surface temperature node |
| g,r,w | radiative air node internal gains received by wall |
| sol,w | solar gains through zone windows received by walls |
| long,w | longwave radiation exchange between the wall and all other walls and windows |
| wall-gain | user-specified heat flow to the wall or window surface |
| R2 | coefficient of determination |
References
- Grange, R.; Hurd, R. Thermal screens—Environmental and plant studies. Sci. Hortic. 1983, 19, 201–211. [Google Scholar] [CrossRef]
- De Koning, A. Long-term temperature integration of tomato. Growth and development under alternating temperature regimes. Sci. Hortic. 1990, 45, 117–127. [Google Scholar] [CrossRef]
- Asdrubali, F.; Cotana, F.; Messineo, A. On the evaluation of solar greenhouse efficiency in building simulation during the heating period. Energies 2012, 5, 1864–1880. [Google Scholar] [CrossRef] [Green Version]
- Chung, M.; Park, J.-U.; Yoon, H.-K. Simulation of a central solar heating system with seasonal storage in Korea. Sol. Energy 1998, 64, 163–178. [Google Scholar] [CrossRef]
- Opdam, J.; Schoonderbeek, G.; Heller, E.; De Gelder, A. Closed greenhouse: A starting point for sustainable entrepreneurship in horticulture. In Proceedings of the International Conference on Sustainable Greenhouse Systems-Greensys 2004, Leuven, Belgium, 31 October 2005; pp. 517–524. [Google Scholar]
- Sturm, B.; Maier, M.; Royapoor, M.; Joyce, S. Dependency of production planning on availability of thermal energy in commercial greenhouses–A case study in Germany. Appl. Therm. Eng. 2014, 71, 239–247. [Google Scholar] [CrossRef]
- Kaukoranta, T.; Näkkilä, J.; Särkkä, L.; Jokinen, K. Effects of lighting, semi-closed greenhouse and split-root fertigation on energy use and CO2 emissions in high latitude cucumber growing. Agric. Food Sci. 2014, 23, 220–235. [Google Scholar] [CrossRef]
- Wong, B.; McClung, L.; Snijders, A.; McClenahan, D.; Thornton, J. The application of aquifer thermal energy storage in the Canadian greenhouse industry. In Proceedings of the International Symposium on High Technology for Greenhouse Systems: GreenSys2009, Quebec, QC, Canada, 14–19 June 2009; pp. 437–444. [Google Scholar]
- Yıldız, İ.; Yue, J.; Yıldız, A.C. Air Source Heat Pump Performance in Open, Semi-closed, and Closed Greenhouse Systems in the Canadian Maritimes. In Progress in Clean Energy; Springer: Berlin/Heidelberg, Germany, 2015; Volume 1, pp. 183–191. [Google Scholar]
- Vadiee, A.; Martin, V. Thermal energy storage strategies for effective closed greenhouse design. Appl. Energy 2013, 109, 337–343. [Google Scholar] [CrossRef]
- Ahamed, M.S.; Guo, H.; Taylor, L.; Tanino, K. Heating demand and economic feasibility analysis for year-round vegetable production in Canadian Prairies greenhouses. Inf. Process. Agric. 2019, 6, 81–90. [Google Scholar] [CrossRef]
- Ahamed, M.S. Thermal Environment Modeling and Optimization of Greenhouse in Cold Regions. Ph.D. Thesis, University of Saskatchewan, Saskatoon, SK, Canada, 2018. [Google Scholar]
- Santamouris, M.; Argiriou, A.; Vallindras, M. Design and operation of a low energy consumption passive solar agricultural greenhouse. Sol. Energy 1994, 52, 371–378. [Google Scholar] [CrossRef]
- Gupta, R.; Tiwari, G. Thermal modellina of a greenhouse having a north wall using periodic analysis and its experimental validation. Int. J. Ambient. Energy 2005, 26, 115–128. [Google Scholar] [CrossRef]
- Mobtaker, H.G.; Ajabshirchi, Y.; Ranjbar, S.F.; Matloobi, M. Solar energy conservation in greenhouse: Thermal analysis and experimental validation. Renew. Energy 2016, 96, 509–519. [Google Scholar] [CrossRef]
- TRNSYS. Multizone Building Modeling with Type56 and TRNBuild; Solar Energy Laboratory, University of Wisconsin: Madison, WI, USA, 2017. [Google Scholar]
- Carlini, M.; Honorati, T.; Castellucci, S. Photovoltaic greenhouses: Comparison of optical and thermal behaviour for energy savings. Math. Probl. Eng. 2012, 2012, 1–10. [Google Scholar] [CrossRef]
- Marucci, A.; Carlini, M.; Castellucci, S.; Cappuccini, A. Energy efficiency of a greenhouse for the conservation of forestry biodiversity. Math. Probl. Eng. 2013, 2013, 1–7. [Google Scholar] [CrossRef]
- Vadiee, A.; Martin, V. Energy management in horticultural applications through the closed greenhouse concept, state of the art. Renew. Sustain. Energy Rev. 2012, 16, 5087–5100. [Google Scholar] [CrossRef]
- Zhang, L.; Xu, P.; Mao, J.; Tang, X.; Li, Z.; Shi, J. A low cost seasonal solar soil heat storage system for greenhouse heating: Design and pilot study. Appl. Energy 2015, 156, 213–222. [Google Scholar] [CrossRef]
- Patil, R.; Atre, U.; Nicklas, M.; Bailey, G.; Power, G. An Integrated Sustainable Food Production and Renewable Energy System with Solar & Biomass CHP; American Solar Energy Society: Austin, TX, USA, 2013. [Google Scholar]
- Mashonjowa, E.; Ronsse, F.; Milford, J.R.; Pieters, J. Modelling the thermal performance of a naturally ventilated greenhouse in Zimbabwe using a dynamic greenhouse climate model. Sol. Energy 2013, 91, 381–393. [Google Scholar] [CrossRef]
- Chargui, R.; Sammouda, H.; Farhat, A. Geothermal heat pump in heating mode: Modeling and simulation on TRNSYS. Int. J. Refrig. 2012, 35, 1824–1832. [Google Scholar] [CrossRef]
- Candy, S.; Moore, G.; Freere, P. Design and modeling of a greenhouse for a remote region in Nepal. Procedia Eng. 2012, 49, 152–160. [Google Scholar] [CrossRef] [Green Version]
- Beshada, E.; Zhang, Q.; Boris, R. Winter performance of a solar energy greenhouse in southern Manitoba. Can. Biosyst. Eng. 2006, 48, 1–8. [Google Scholar]
- Lu, W.; Zhang, Y.; Fang, H.; Ke, X.; Yang, Q. Modelling and experimental verification of the thermal performance of an active solar heat storage-release system in a Chinese solar greenhouse. Biosyst. Eng. 2017, 160, 12–24. [Google Scholar] [CrossRef]
- Joudi, K.A.; Farhan, A.A. A dynamic model and an experimental study for the internal air and soil temperatures in an innovative greenhouse. Energy Convers. Manag. 2015, 91, 76–82. [Google Scholar] [CrossRef]
- Chen, W.; Liu, W. Numerical simulation of the airflow and temperature distribution in a lean-to greenhouse. Renew. Energy 2006, 31, 517–535. [Google Scholar] [CrossRef]
- Sayyah, A.H.A.; Mohammadi, S.; Nikbakht, A.M.; Khalife, E. Modeling and Design a Special Type of Passive Solar Greenhouse in Cold Climate by TRNSYS. J. Agric. Sci. 2020, 26, 488–498. [Google Scholar]
- Wei, B.; Guo, S.; Wang, J.; Li, J.; Wang, J.; Zhang, J.; Qian, C.; Sun, J. Thermal performance of single span greenhouses with removable back walls. Biosyst. Eng. 2016, 141, 48–57. [Google Scholar] [CrossRef]
- Li, J.; Li, L.; Wang, H.; Ferentinos, K.P.; Li, M.; Sigrimis, N. Proactive energy management of solar greenhouses with risk assessment to enhance smart specialisation in China. Biosyst. Eng. 2017, 158, 10–22. [Google Scholar] [CrossRef]
- Rasheed, A.; Lee, J.W.; Lee, H.W. Development and optimization of a building energy simulation model to study the effect of greenhouse design parameters. Energies 2018, 11, 2001. [Google Scholar] [CrossRef] [Green Version]





| Name | Type | Properties |
|---|---|---|
| 22 | Wtype112 | 150-mm insulation with 50-mm wood |
| 32 | Wtype121 | 85-mm insulation with 200-mm common brick |
| 33 | Wtype122 | 200-mm common brick with 50-mm insulation |
| 40 | Wtype129 | Face brick and 200-mm clay tile with 50-mm insulation |
| 91 | Wtype45 | 50-mm insulation with 200-mm clay tile |
| 120 | Wtype71 | 200-mm clay tile with air space |
| 121 | Wtype72 | 200-mm clay |
| 135 | Wtype85 | 100-mm lightweight concrete block and 25-mm insulation |
| 137 | Wtype87 | 100-mm lightweight concrete block |
| 142 | Wtype91 | 100-mm clay tile and 25-mm insulation |
| 147 | Wtype96 | Sheet metal with 75-mm insulation |
| Time | Wtype112 | Wtype1122 | Wtype121 | Wtype122 | Wtype129 | Wtype45 | Wtype71 | Wtype72 | Wtype85 | Wtype87 | Wtype91 | Wtype96 | Optimize |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| January | 19,443.4 | 19,901.9 | 20,013.2 | 19,270.9 | 18,318.0 | 20,841.9 | 22,778.4 | 20,532.5 | 21,321.1 | 24,269.8 | 21,525.3 | 20,314.2 | 12,173.9 |
| February | 11,436.6 | 11,812.7 | 11,244.1 | 10,467.8 | 9946.9 | 12,297.6 | 13,734.7 | 12,282.4 | 12,927.5 | 15,289.4 | 13,069.8 | 12,219.3 | 6039.9 |
| March | 6814.9 | 7072.6 | 6081.4 | 5492.5 | 5344.3 | 7211.4 | 8216.7 | 7370.5 | 7888.8 | 9677.7 | 7973.1 | 7488.6 | 2975.8 |
| April | 924.2 | 999.4 | 181.2 | 81.4 | 143.2 | 704.9 | 834.6 | 1042.1 | 1276.6 | 1941.4 | 1271.8 | 1303.6 | 98.6 |
| May | 131.5 | 143.6 | 2.6 | 0.0 | 3.8 | 74 | 86.8 | 147.7 | 201.5 | 352.5 | 197.8 | 224.9 | 3.4 |
| June | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| July | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| August | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| September | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 | 0.0 |
| October | 875.2 | 930.5 | 244.9 | 146.5 | 228.9 | 702.1 | 817.5 | 963.0 | 1143.3 | 1652.8 | 1141.5 | 1169.6 | 212.0 |
| November | 5520.0 | 5752.5 | 4446.6 | 3838.8 | 3846.7 | 5752.3 | 6587.2 | 6007.0 | 6463.4 | 8027.1 | 6532.8 | 6145.5 | 2381.5 |
| December | 11,169.9 | 11,579.1 | 10,811.9 | 9,943.0 | 9,434.4 | 12,019.2 | 13,517.3 | 12,048.7 | 12,724.1 | 15,218.4 | 12,875.2 | 12,056.1 | 6090.5 |
| Summary | 56,315.7 | 58,192.4 | 53,026.0 | 49,240.9 | 47,266.3 | 59,576.4 | 66,573.1 | 60,393.9 | 63,947.7 | 76,445.4 | 64,588.5 | 60,926.6 | 29,975.7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mohammadi, S.; Khalife, E.; Kaveh, M.; Sayyah, A.H.A.; Nikbakht, A.M.; Szymanek, M.; Dziwulski, J. Comparison of Optimized and Conventional Models of Passive Solar Greenhouse—Case Study: The Indoor Air Temperature, Irradiation, and Energy Demand. Energies 2021, 14, 5369. https://doi.org/10.3390/en14175369
Mohammadi S, Khalife E, Kaveh M, Sayyah AHA, Nikbakht AM, Szymanek M, Dziwulski J. Comparison of Optimized and Conventional Models of Passive Solar Greenhouse—Case Study: The Indoor Air Temperature, Irradiation, and Energy Demand. Energies. 2021; 14(17):5369. https://doi.org/10.3390/en14175369
Chicago/Turabian StyleMohammadi, Saleh, Esmail Khalife, Mohammad Kaveh, Amir Hosein Afkari Sayyah, Ali Mohammad Nikbakht, Mariusz Szymanek, and Jacek Dziwulski. 2021. "Comparison of Optimized and Conventional Models of Passive Solar Greenhouse—Case Study: The Indoor Air Temperature, Irradiation, and Energy Demand" Energies 14, no. 17: 5369. https://doi.org/10.3390/en14175369
APA StyleMohammadi, S., Khalife, E., Kaveh, M., Sayyah, A. H. A., Nikbakht, A. M., Szymanek, M., & Dziwulski, J. (2021). Comparison of Optimized and Conventional Models of Passive Solar Greenhouse—Case Study: The Indoor Air Temperature, Irradiation, and Energy Demand. Energies, 14(17), 5369. https://doi.org/10.3390/en14175369







