A Potential Method to Predict Performance of Positive Stirling Cycles Based on Reverse Ones
Abstract
:Highlights:
- An analysis model is proposed for positive and reverse Stirling cycles.
- Positive and reverse cycles are analyzed at different gases and rotary speeds.
- Maximum relative error is <15.3% for both the Stirling engine and the refrigerator.
- A method to predict performance of positive cycles is based on reverse ones.
1. Introduction
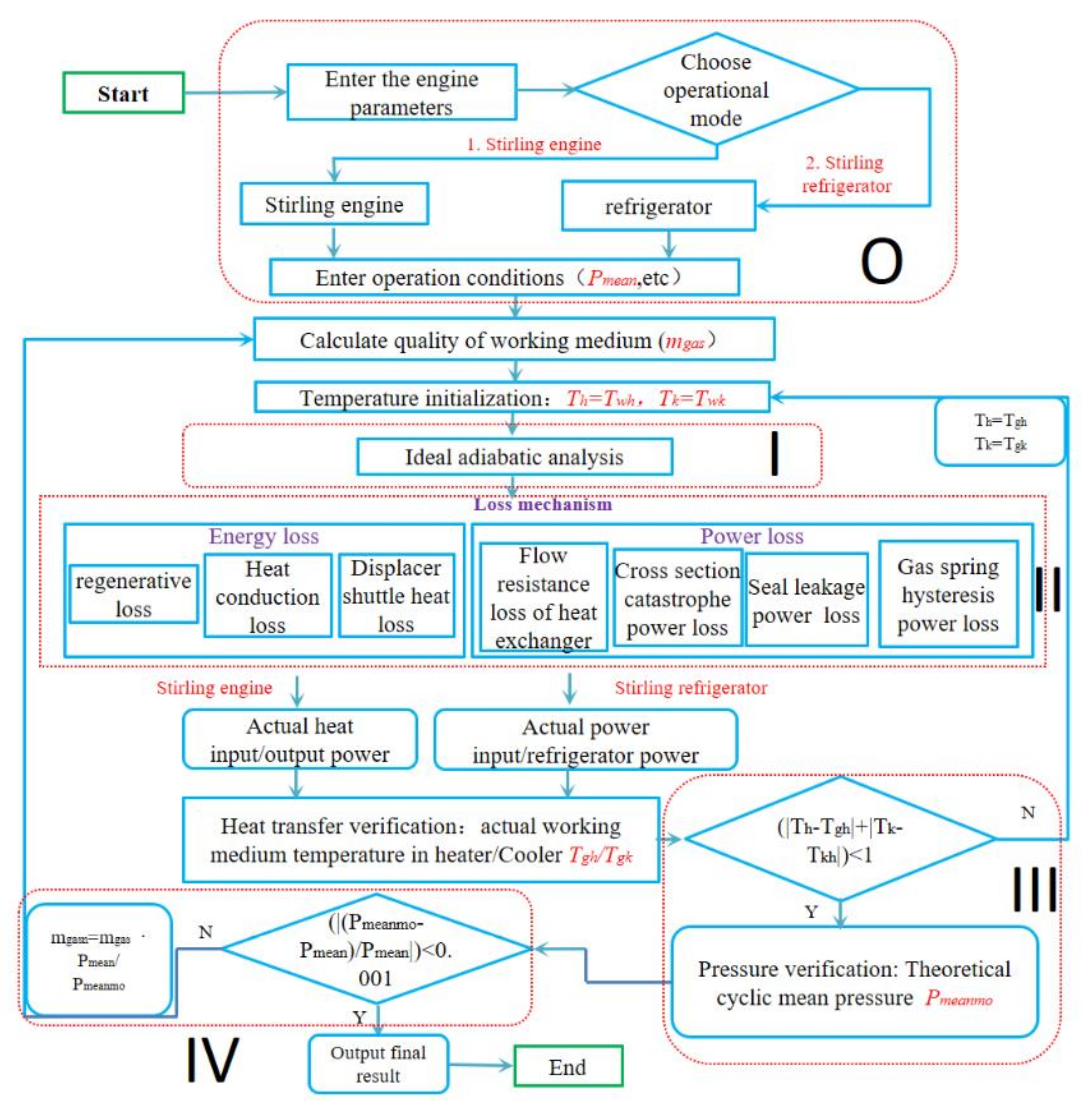
2. Modeling and Experimental System of Positive and Reverse Stirling Cycles
2.1. Improved Simple Model Combined with Various Heat and Power Losses
2.2. Experimental System
3. Verification of the Model with GPU-3 Stirling Engine
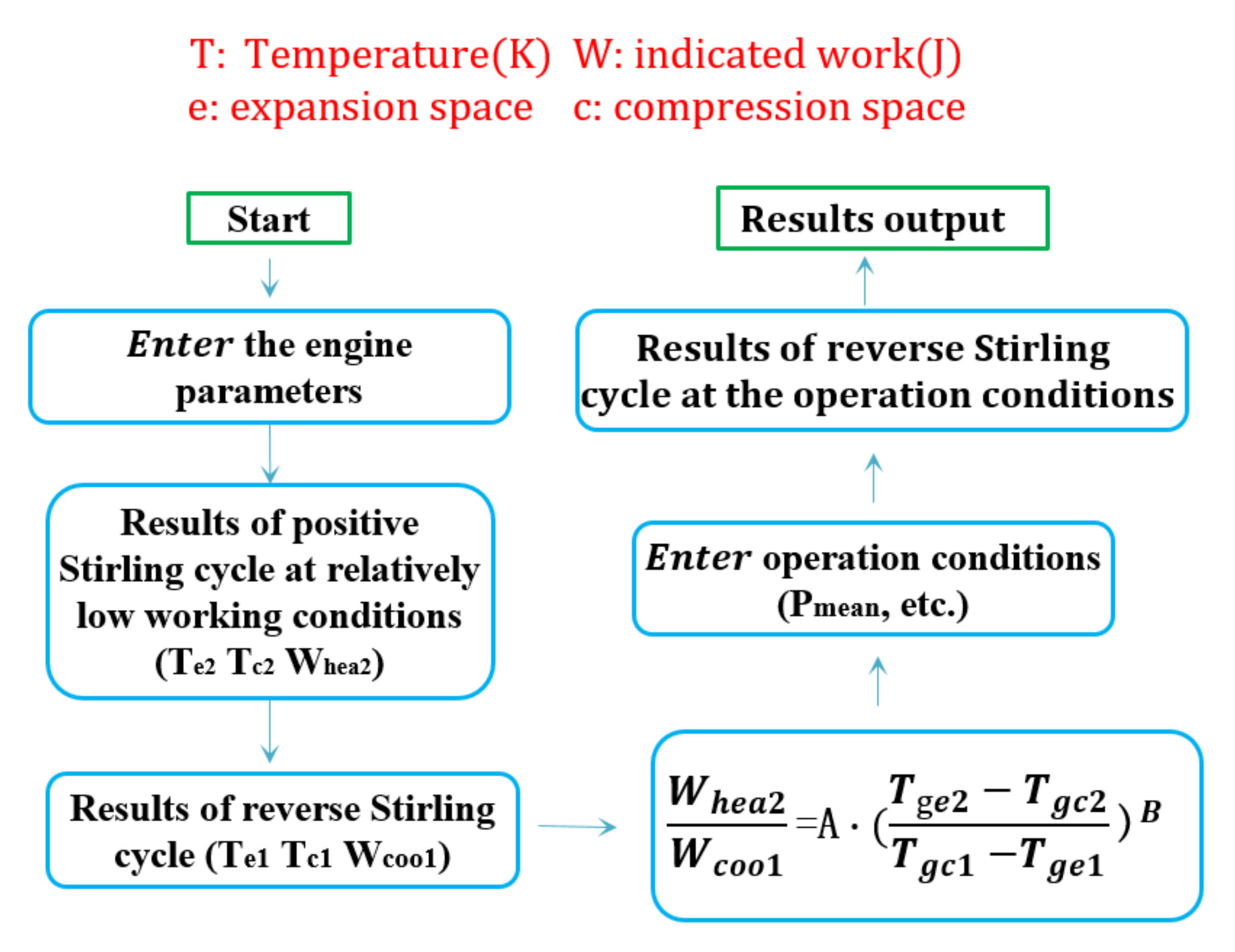
4. A Method to Predict the Performance of Positive Stirling Cycles Based on Reverse Stirling Cycles
- I.
- Carry out the positive and reverse Stirling cycles at the same low conditions according to the same Stirling engine working as both a prime mover and a refrigerator.
- II.
- Establish a mathematical model based on the positive and reverse Stirling cycles in part I.
- III.
- Carry out reverse Stirling cycles at high conditions and predict the performance of the positive Stirling cycles based on the mathematical model in part II.
5. Results and Discussion
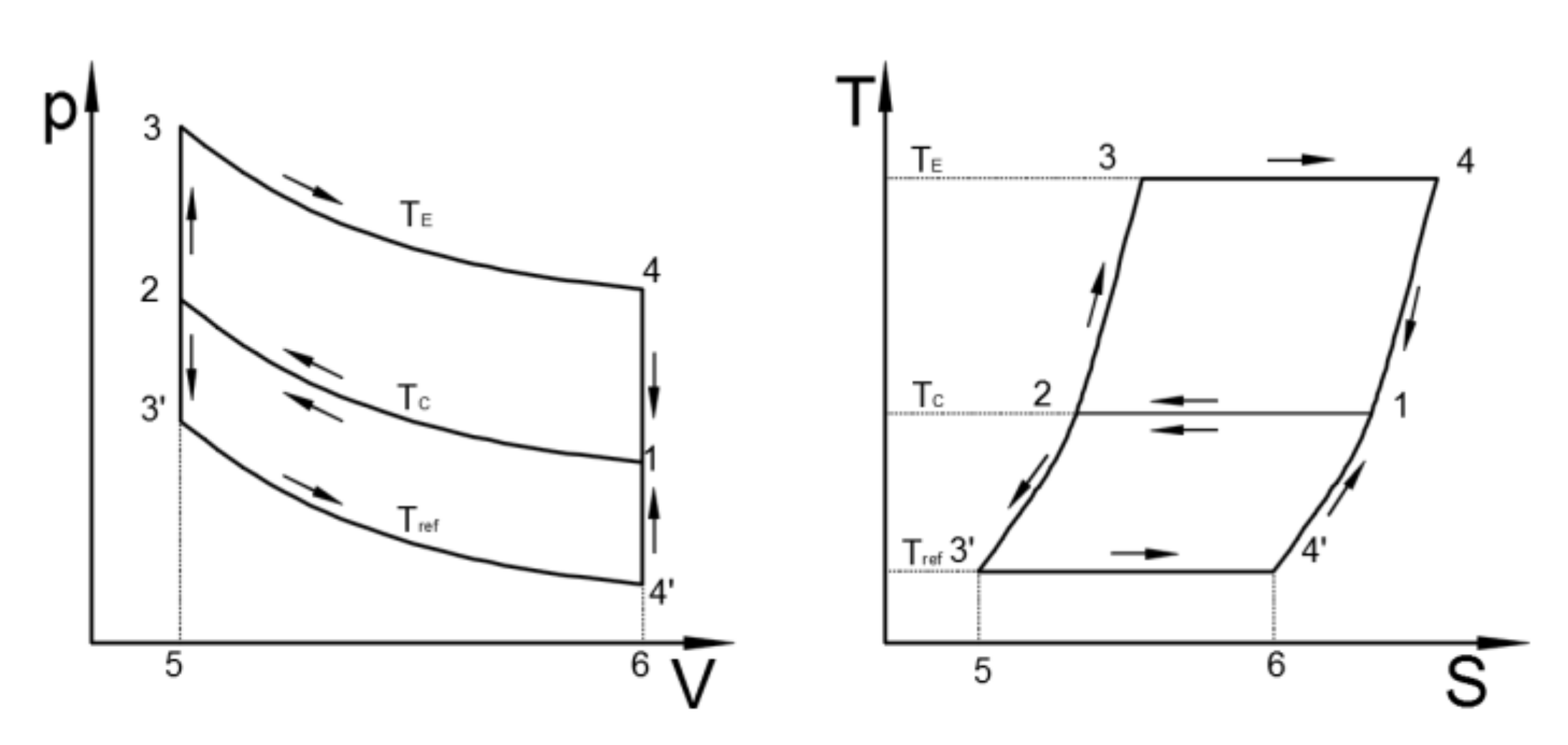
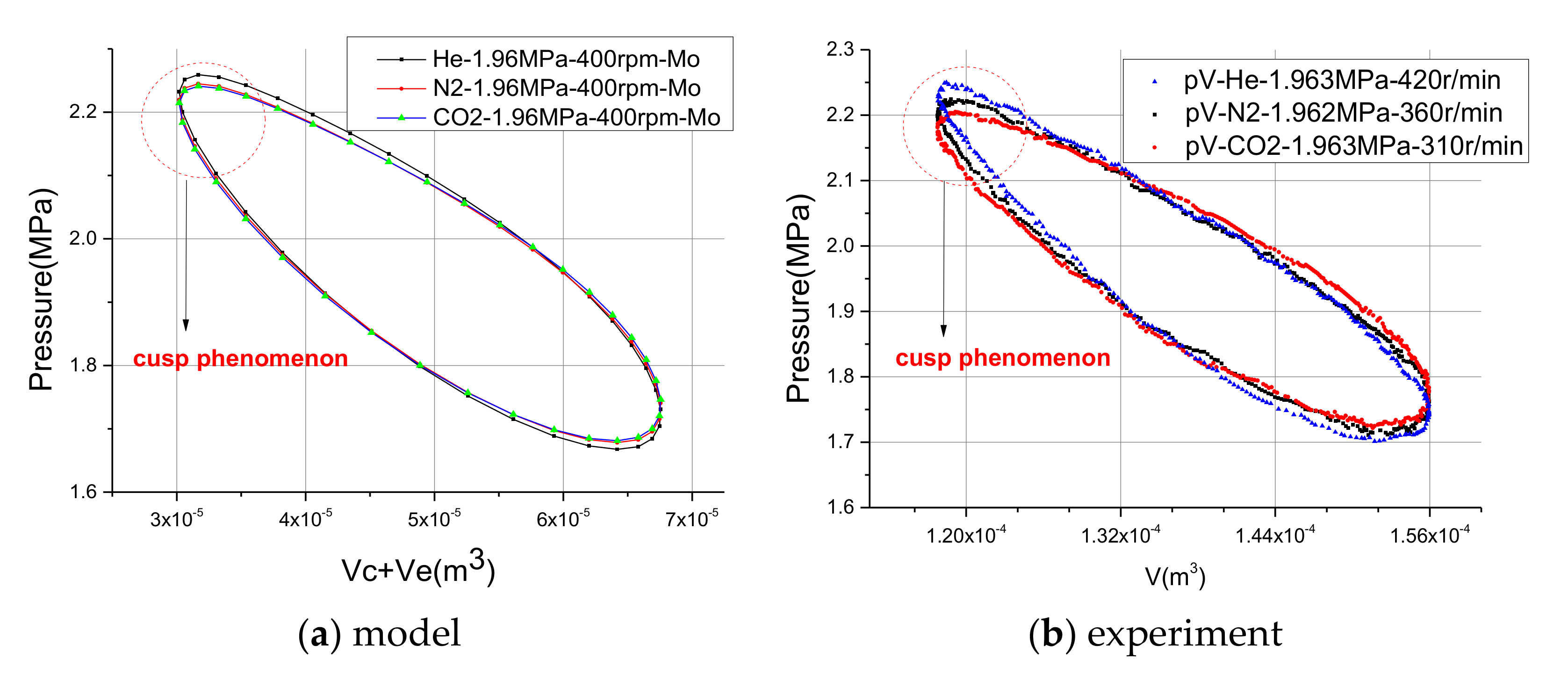
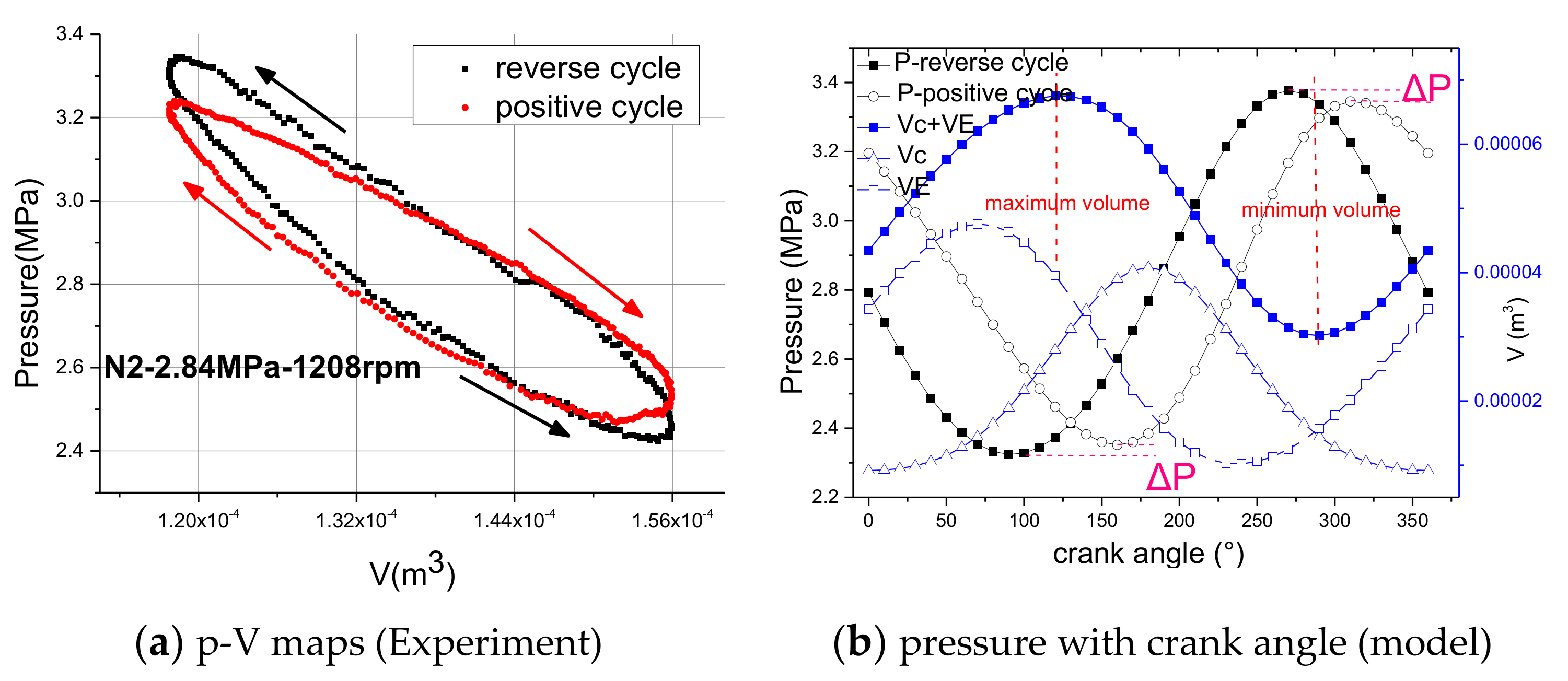
5.1. Positive and Reverse Stirling Cycles’ p-V Maps
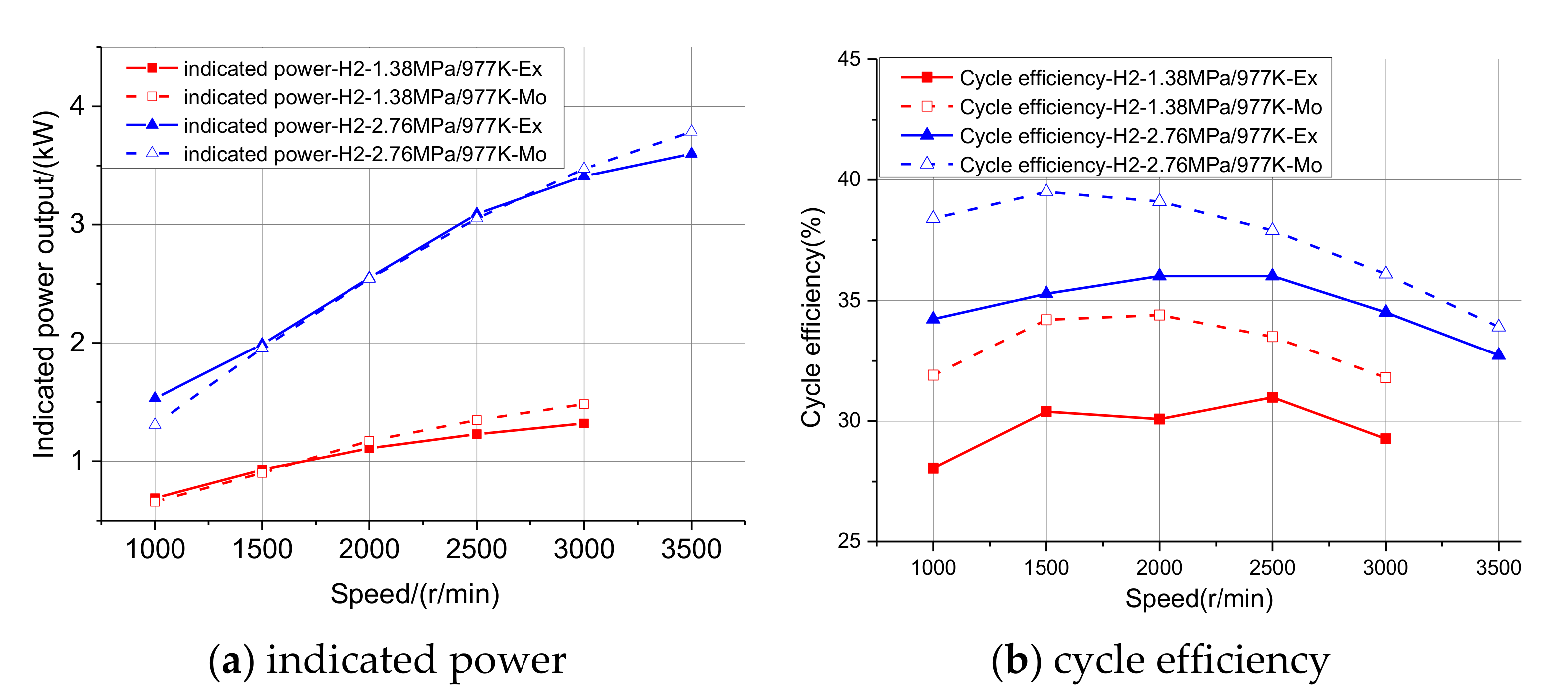
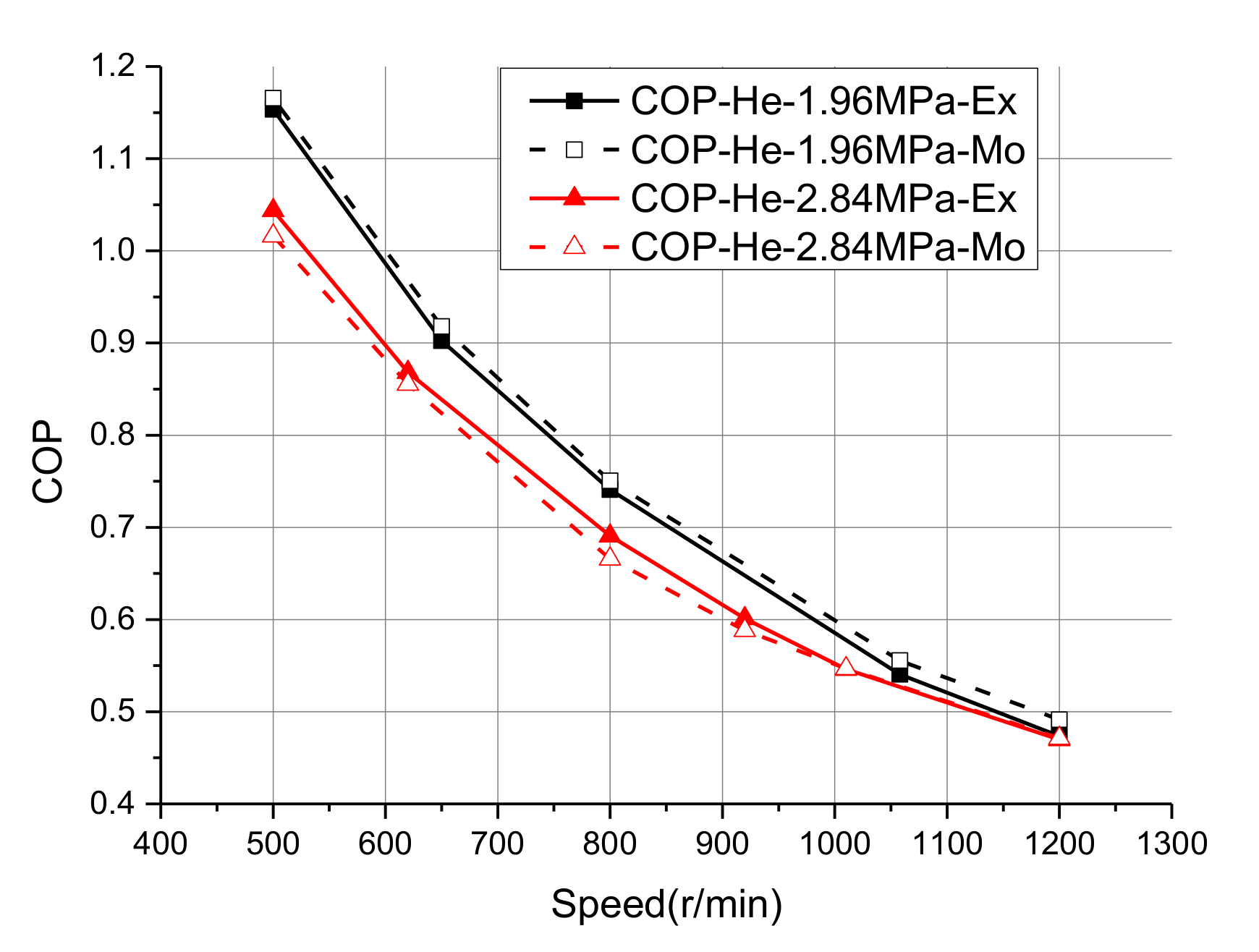
5.2. Performance of Stirling Engine
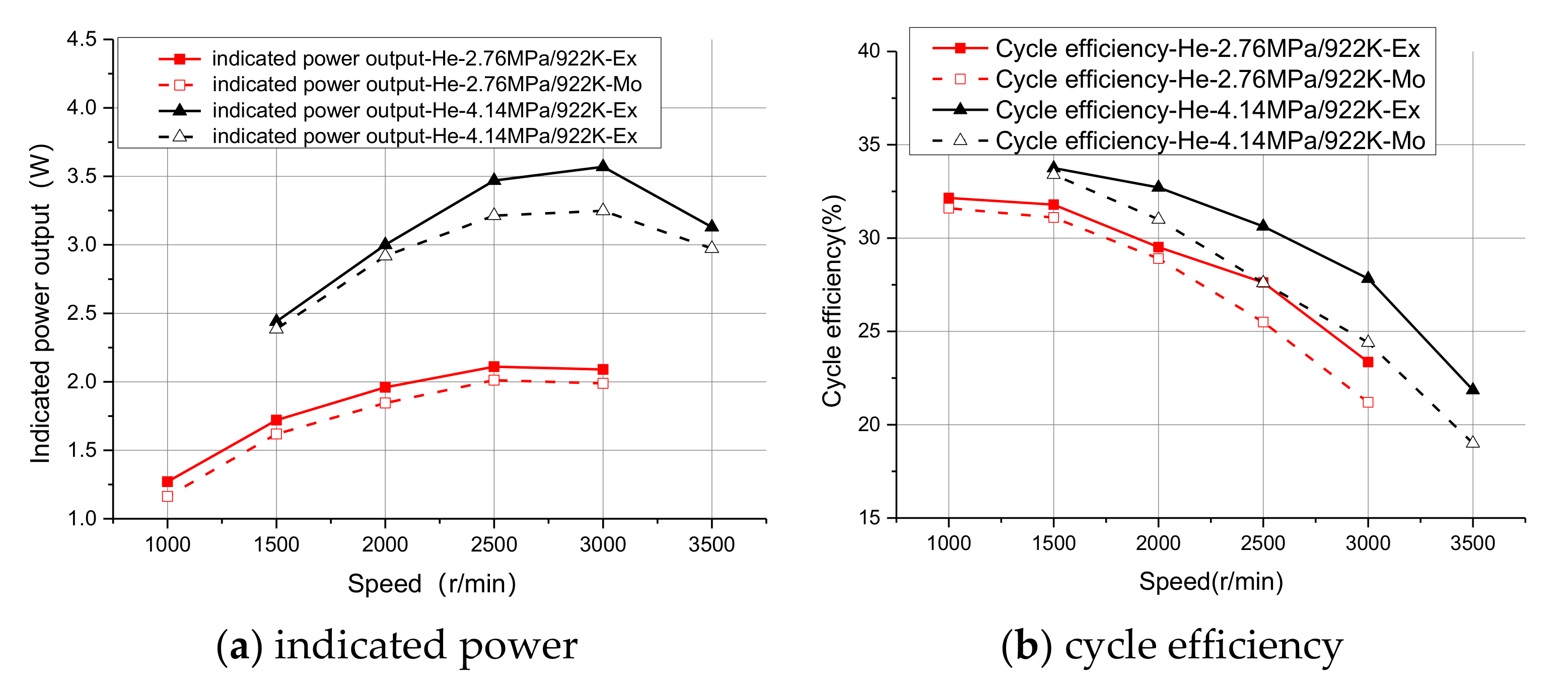
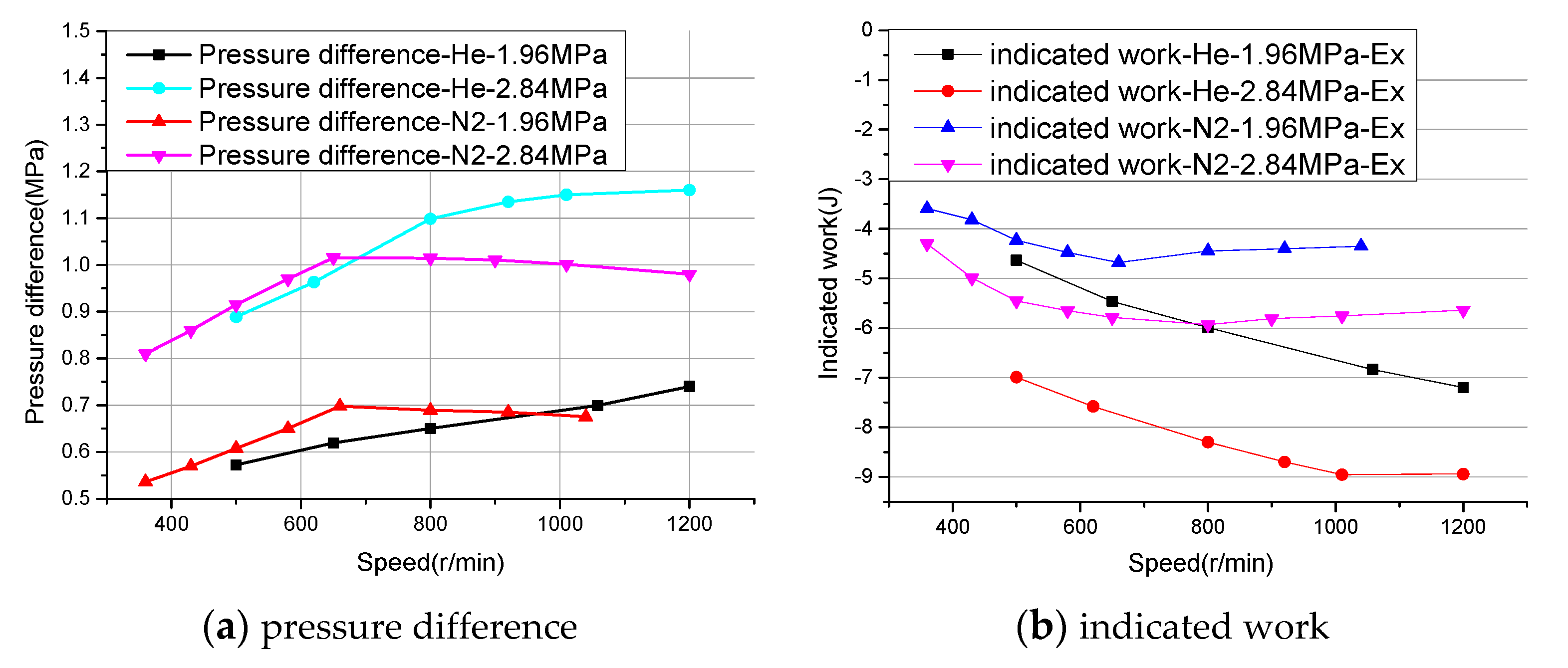
5.3. Performance of Stirling Refrigerator
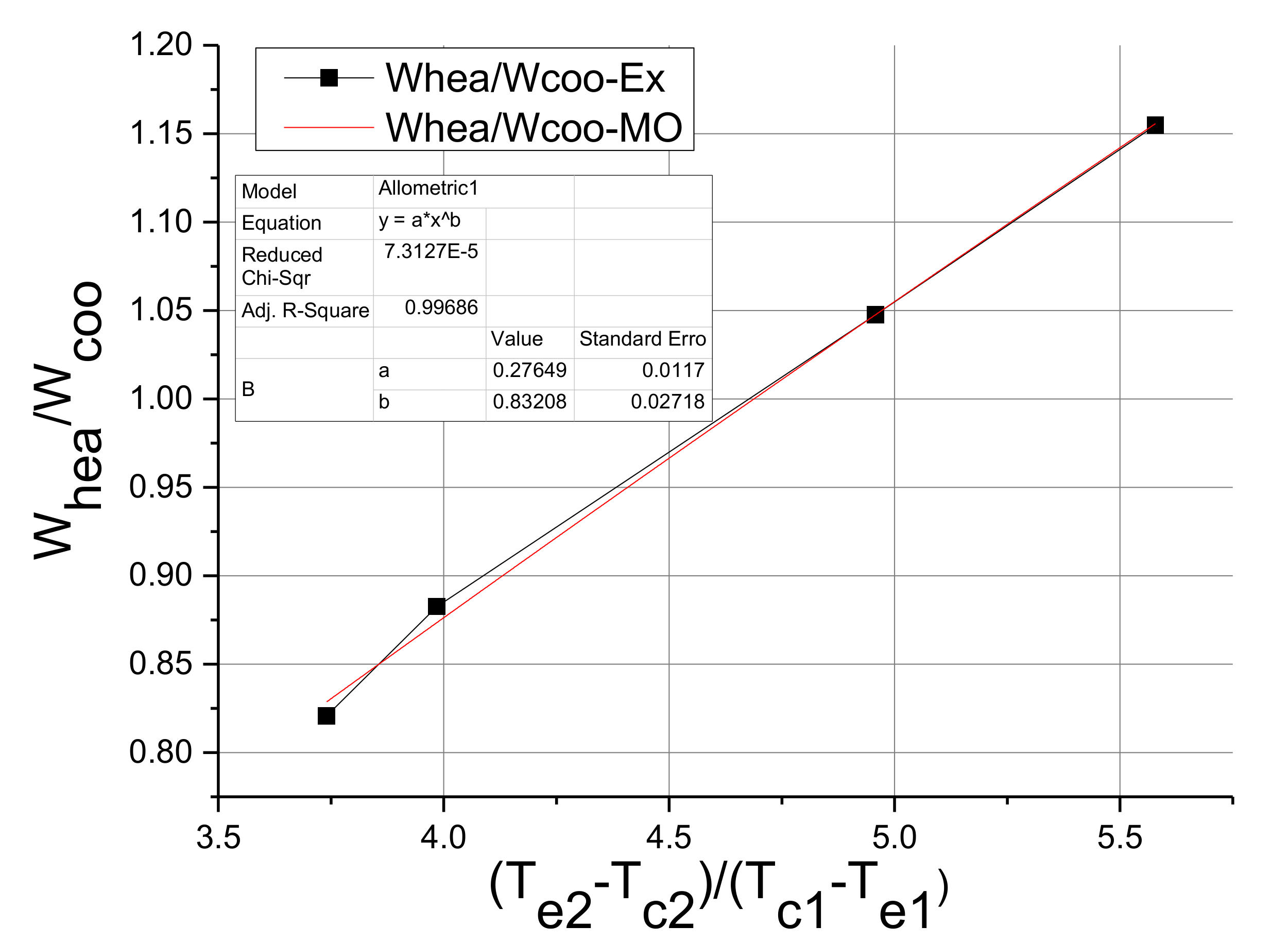
5.4. Relationship between the Indicated Work of Stirling Engine and Refrigerator of the 100 W Stirling Engine
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | pressure term coefficient |
| Ah/Aco | heat transfer area of the heater/cooler (m2) |
| Aw | cross-sectional area (m2) |
| a | ank angle (°) |
| B | temperature term coefficient |
| Bd | displacer piston rod length (m) |
| Bp | power piston rod length (m) |
| cco | heat capacity of water (J/(kg·K)) |
| Cref | Reynolds friction factor |
| Dc | cylinder diameter (m) |
| Dro | displacer piston rod diameter (m) |
| Dresh | regenerator shell diameter (m) |
| e | eccentricity (m) |
| hj | cross-sectional loss coefficient |
| km | material thermal conductivity (WK−3) |
| L | connecting rod length (m) |
| lresh | regenerator shell height (m) |
| Lt | thermal wavelength (m) |
| km | material thermal conductivity (Wm−1K−1) |
| n | rotational speed (r/min) |
| P | pressure (MPa) |
| Qacc | actual cooling power (W) of SR |
| Qacco | actual cooling power (W) of SE |
| Qach | actual heat input (W) of SE |
| Qadh | adiabatic analysis heat input (W) |
| Qacco | actual cooling power (W) of SE |
| Qadco | adiabatic analysis cooling power (W) |
| Qresh | regenerator shell natural convective heat loss (W) |
| Qw | heat conduction loss (W) |
| Qrloss | regenerative heat loss (W) |
| Qsh | shuttle heat loss (W) |
| qco | cooling water flow (kg/s) |
| qmleak | leakage mass flow (kg·s−1) |
| qco | cooling water flow (kg/s) |
| R | the universal gas constant (J/(mol·K)) |
| Re | Reynolds number |
| sd | expansion space height (m) |
| SE | Stirling engine |
| SR | Stirling refrigerator |
| sp | compression space height (m) |
| St | Stanton number |
| Tgh/Tgk | gas temperature in the heater/cooler (°C) |
| Tge/Tgc | gas temperature in expansion/compression space (°C) |
| Twh/Twk | wall temperature of the heater/cooler (°C) |
| Th/Tk | adiabatic analysis gas temperature in heater/cooler (°C) |
| Tleak | leakage gas temperature (K) |
| Tresh | regenerator shell average temperature (°C) |
| u | velocity of working gas (m/s) |
| Ve/Vc | volumes of expansion/compression space (m3) |
| Wacip | actual indicated power output (W) |
| Wacipi | actual cycle input power of SR (W) |
| Wacipo | actual cycle output power (W) of SE |
| Wadip | adiabatic analysis indicated power (W) |
| Wcy | indicated work (J) |
| Wfj | minor power loss (W) |
| Wfr | flow resistance power loss (W) |
| Wgp | gas spring hysteresis power losses (W) |
| Wleak | seal leakage power loss (W) |
| Wsh | shaft power (W) |
| λ | heat conduction coefficient (W/(m·K) |
| γ | insulation factor |
| ε | regenerator effectiveness |
| δ | gap between displacer and cylinder wall (m) |
Appendix A
| pressure | |
| masses | |
| mass accumulations | |
| mass flow | |
| if then , else | conditional temperature |
| if then , else | |
| temperatures | |
| energy | |

| Basic Working Condition | Transmission Parameters | Heater | Regenerator | Cooler | |||||
|---|---|---|---|---|---|---|---|---|---|
| Heat source temperature (°C) | 535 | Diameter of displacer/power piston cylinder (mm) | 45 | Internal diameter of heating tube (mm) | 3 | Outer diameter (mm) | 72 | Internal diameter of cooling tube (mm) | 3 |
| Cooling water temperature (°C) | 13 | connecting rod length of displacer/power piston (mm) | 42 | Outer diameter of heating tube (mm) | 4 | Internal diameter (mm) | 51 | Outer diameter of cooling tube (mm) | 4 |
| Pressure (Mpa) | 1—3 | Eccentricity (mm) | 17 | Number of heating tube | 30 | Axial length (mm) | 29 | Number of cooling tube | 40 |
| Rotation speed (r/min) | 300–1500 | Crank radius (mm) | 11 | Length of heating tube (mm) | 140 | Matrix material | Stainless steel wire mesh | Length of cooling tube (mm) | 49 |
| Ambient temperature (°C) | 5—10 | Piston stroke (mm) | 24.3 | Material heating tube | Stainless steel | Porosity | 0.71 | Material cooling tube | Chromium material |
| Cooling water flow (L/min) | 3.8–4.2 | Phase difference (°) | 109 | Number | 1 | Mesh number | 200 | Number | 1 |
| Acquisition Signal | Acquisition Instrument | Model | Measurement Accuracy and Range | Picture |
|---|---|---|---|---|
| Temperature | Thermocouple | TJ120-CAXL-116U-18 Omega K-type | 0 °C−1200 °C, 0.1° |  |
| Pressure | Pressure transducer | HM90C2-1-A2-F1-W1 Nanjing HongMu | 0–5 MPa, 0–5 VDC, 0.5% FS |  |
| Flow | Turbine flowmeter | DN10 | Accuracy: 0.5% Signal output: 4–20 mA |  |
| Torque | The torque sensor | KR-803 | ±5 N·m 0–5–10 V 24 VDC |  |
| Electric power | Power meter | HY194E-9S1 Shanghai HongYing | 0–220 V, 0–20 A, AC·0.5% |  |
| Speed and phase angle | Hall sensor | CL12-3005NA | Maximum current 200 mA 1–20,000 rpm |  |
| Data acquisition instrument | Agilent data acquisition Instrument | 34972A | 20 channels eleven different signals are available |  |
| NI data acquisition Card | NI 9215 | 4 channels, +10 V Resolution: 16 bit Acquisition frequency:100 KHz |  | |
| NI acquisition instrument cabinet | NI cDAQ-9174 | Four universal 32-bit counters/timers |  |

References
- Awan, A.B.; Zubair, M.; Memon, Z.A.; Ghalleb, N.; Tlili, I. Comparative analysis of dish Stirling engine and photovoltaic technologies: Energy and economic perspective. Sustain. Energy Technol. Assess. 2021, 44, 101028. [Google Scholar]
- William R, M.; Martini Engineering. Stirling engine design manual. 1983. Available online: https://ntrs.nasa.gov/api/citations/19830022057/downloads/19830022057.pdf (accessed on 25 October 2021).
- Ferreira, A.C.; Silva, J.; Teixeira, S.; Carlos Teixeira, J.; Azucena Nebra, S. Assessment of the Stirling engine performance comparing two renewable energy sources: Solar energy and biomass. Renew. Energy 2020, 154, 581–597. [Google Scholar] [CrossRef]
- Urieli, I.; David, M. Berchowitz, Stirling Cycle Engine Analysis; A Hilger: Hilger, MT, USA, 1984. [Google Scholar]
- Ahmed, F.; Huang, H.L.; Khan, A.M. Numerical modeling and optimization of beta-type Stirling engine. Appl. Therm. Eng. 2019, 149, 385–400. [Google Scholar] [CrossRef]
- Tlili, I. Finite time thermodynamic evaluation of endoreversible Stirling heat engine at maximum power conditions—ScienceDirect. Renew. Sustain. Energy Rev. 2012, 16, 2234–2241. [Google Scholar] [CrossRef]
- Hosseinzade, H.; Sayyaadi, H. CAFS: The Combined Adiabatic-Finite Speed thermal model for simulation and optimization of Stirling engines. Energy Convers. Manag. 2015, 91, 32–53. [Google Scholar] [CrossRef]
- Crowley, J.L. Standardized efficiency terms: Comparison of some Stirling engine performance figures. In Proceedings Intersociety Energy Conversion Engineering Conference; Engineering Technology Division, Oak Ridge National Laboratory: Oak Ridge, TN, USA, 1984; Volume 3. Available online: https://www.osti.gov/biblio/5854341 (accessed on 25 October 2021).
- Stine, W.B.; Diver, R.B. A Compendium of Solar Dish/Stirling Technology. 1994. Available online: https://www.researchgate.net/publication/235087117_A_Compendium_of_Solar_DishStirling_Technology (accessed on 25 October 2021).
- Michels, A.P.J. The Philips Stirling Engine: A Study of Its Efficiency as a Function of Operating Temperatures and Working Fluids. In Proceedings of the Intersociety Energy Conversion Engineering Conference, 11th, Proceedings 1976 (SAE 769258 Proceeding), 12–17 September 1976; Available online: https://trid.trb.org/view/51882 (accessed on 25 October 2021).
- Percival, W.H. History and Overview of Stirling Engines. In Proceedings of the 8th Energy Technology Conference; 1981. Available online: https://pascal-francis.inist.fr/vibad/index.php?action=getRecordDetail&idt=PASCAL82X0308649 (accessed on 25 October 2021).
- Çınar, C.; Aksoy, F.; Solmaz, H.; Yılmaz, E.; Uyumaz, A. Manufacturing and testing of an α-type Stirling engine. Appl. Therm. Eng. 2018, 130, 1373–1379. [Google Scholar] [CrossRef]
- Cheng, C.H.; Yang, H.-S.; Keong, L. Theoretical and experimental study of a 300-W beta-type Stirling engine. Energy-Oxford 2013, 59, 590–599. [Google Scholar] [CrossRef]
- Yang, H.S.; Cheng, C.H.; Huang, S.T. A complete model for dynamic simulation of a 1-kW class beta-type Stirling engine with rhombic-drive mechanism. Energy 2018, 161, 892–906. [Google Scholar] [CrossRef]
- Kato, Y. Indicated diagrams of low temperature differential Stirling engines with channel-shaped heat exchangers. Renew. Energy 2017, 103, 30–37. [Google Scholar] [CrossRef]
- Tavakolpour-Saleh, A.R.; Zare, S.H.; Bahreman, H. A novel active free piston Stirling engine: Modeling, development, and experiment. Appl. Energy 2017, 199, 400–415. [Google Scholar] [CrossRef]
- Romanelli, A. Stirling engine operating at low temperature difference. Am. J. Phys. 2020, 88, 319–324. [Google Scholar] [CrossRef] [Green Version]
- Takeuchi, M.; Suzuki, S.; Abe, Y. Development of a Low-temperature-difference Indirect-heating Kinematic Stirling Engine. Energy 2021, 229, 120577. [Google Scholar] [CrossRef]
- Alireza, B.; Ali, K. A Gamma type Stirling refrigerator optimization: An experimental and analytical investigation. Int. J. Refrig. 2018, 91, 89–100. [Google Scholar]
- Guo, Y.; Chao, Y.; Wang, B.; Wang, Y.; Gan, Z. A general model of Stirling refrigerators and its verification. Energy Convers. Manag. 2019, 188, 54–65. [Google Scholar] [CrossRef]
- Hachem, H.; Gheith, R.; Aloui, F.; Ben Nasrallah, S. Optimization of an air-filled Beta type Stirling refrigerator. Int. J. Refrig. 2017, 76, 296–312. [Google Scholar] [CrossRef]
- Tekin, Y.; Ataer, O.E. Performance of V-type Stirling-cycle refrigerator for different working fluids. Int. J. Refrig. 2010, 33, 12–18. [Google Scholar] [CrossRef]
- Cheng, C.H.; Huang, C.Y.; Yang, H.S. Development of a 90-K beta type Stirling cooler with rhombic drive mechanism. Int. J. Refrig. 2019, 98, 388–398. [Google Scholar] [CrossRef]
- Li, X.; Dai, W.; Zhang, W.; Zhou, R.; Xiao, Q.; Yu, G.; Luo, E.; Zhu, S. A high-efficiency free-piston Stirling cooler with 350 W cooling capacity at 80 K. Energy Procedia 2019, 158, 4416–4422. [Google Scholar] [CrossRef]
- Smirnov, D.; Dvortsov, V.; Saichenko, A.; Tkachenko, M.; Kukolev, M.; Bischi, A.; Ouerdane, H. Experimental study of a high-tolerance piston-cylinder pair in the alpha Ross-yoke Stirling refrigerator. Int. J. Refrig. 2019, 100, 235–245. [Google Scholar] [CrossRef]
- Ni, M.J.; Shi, B.; Xiao, G.; Peng, H.; Sultan, U.; Wang, S.; Luo, Z.; Cen, K. Improved Simple Analytical Model and experimental study of a 100 W beta-type Stirling engine. Appl. Energy 2016, 169, 768–787. [Google Scholar] [CrossRef]
- Xiao, G.; Huang, Y.; Wang, S.; Peng, H.; Ni, M.; Gan, Z.; Luo, Z.; Cen, K. An approach to combine the second-order and third-order analysis methods for optimization of a Stirling engine. Energy Convers. Manag. 2018, 165, 447–458. [Google Scholar] [CrossRef]
- Holman, J.P. Heat Transfer, 10th ed.; China Machine Press: Beijing, China, 2011. [Google Scholar]
- Gedeon, D.; Wood, J.G. Oscillating-flow regenerator test rig: Hardware and theory with derived correlations for screens and felts. NASA CR-198442; 1996. Available online: https://www.researchgate.net/publication/24300665_Oscillating-Flow_Regenerator_Test_Rig_Hardware_and_Theory_With_Derived_Correlations_for_Screens_and_Felts (accessed on 25 October 2021).
- Timoumi, Y.; Tlili, I.; Nasrallah, S.B. Design and performance optimization of GPU-3 Stirling engines. Energy 2008, 33, 1100–1114. [Google Scholar] [CrossRef]
- Kays, W.M.; London, A.L. Compact Heat Exchangers; Krieger Pub Co: Malabar, FL, USA, 1998. [Google Scholar]
- Wang, K.; Dubey, S.; Choo, F.H.; Duan, F. A transient one-dimensional numerical model for kinetic Stirling engine. Appl. Energy 2016, 183, 775–790. [Google Scholar] [CrossRef] [Green Version]
- Babaelahi, M.; Sayyaadi, H. Simple-II: A new numerical thermal model for predicting thermal performance of Stirling engines. Energy 2014, 69, 873–890. [Google Scholar] [CrossRef]
- Muller, H.K.; Nau, B.S. Fluid Sealing Technology: Principles and Applications; Marcel Dekker: New York, NY, USA, 1998. [Google Scholar]
- Szczygiel, I.; Stanek, W.; Szargut, J. Application of the Stirling engine driven with cryogenic exergy of LNG (liquefied natural gas) for the production of electricity. Energy 2016, 105, 25–31. [Google Scholar] [CrossRef]
- Gheith, R.; Hachem, H.; Aloui, F.; Ben Nasrallah, S. Experimental and Theoretical Investigation of Flows Inside a Gamma Stirling Engine Regenerator; Springer: Cham, Switzerland, 2018; pp. 383–395. [Google Scholar]
- Ma, J.; Lv, P.; Luo, X.; Liu, Y.; Li, H.; Wen, J. Experimental investigation of flow and heat transfer characteristics in double-laminated sintered woven wire mesh. Appl. Therm. Eng. 2016, 95, 53–61. [Google Scholar] [CrossRef]
- Amel, A.N.; Kouravand, S.; Zarafshan, P.; Kermani, A.M.; Khashehchi, M. Study the Heat Recovery Performance of Micro and Nano Metfoam Regenerators in Alpha Type Stirling Engine Conditions. Nanoscale Microscale Thermophys. Eng. 2018, 22, 137–151. [Google Scholar] [CrossRef]










| Category | Equation | Description | |
|---|---|---|---|
| Heat losses | heat conduction loss (W) [28] | Qw: heat conduction loss (W) km: material thermal conductivity (WK−3) Aw: cross-sectional area (m2) l: component length (m) | |
| regenerative heat loss (W) [29] | Qrloss: regenerative heat loss (W) Qr: heat transferred to the regenerator (W) ε: regenerator effectiveness St: Stanton number Awg: regenerator internal wetted area (m2) Ar: regenerator internal free-flow area (m2) | ||
| displacer shuttle heat loss (W) [30] | Qsh: shuttle heat loss (W) Sp: displacer stroke (m) Kg: gas heat conductivity coefficient (Wm−1K−1) Lp: displacer length (m) δ: gap between displacer and cylinder wall (m) Lt: thermal wavelength (m) Ltc: thermal wavelength of cylinder wall (m) Ltp: thermal wavelength of displacer wall (m) Kmc: material thermal conductivity of cylinder (Wm−1K−1) kmp: material thermal conductivity of displacer (Wm−1K−1) | ||
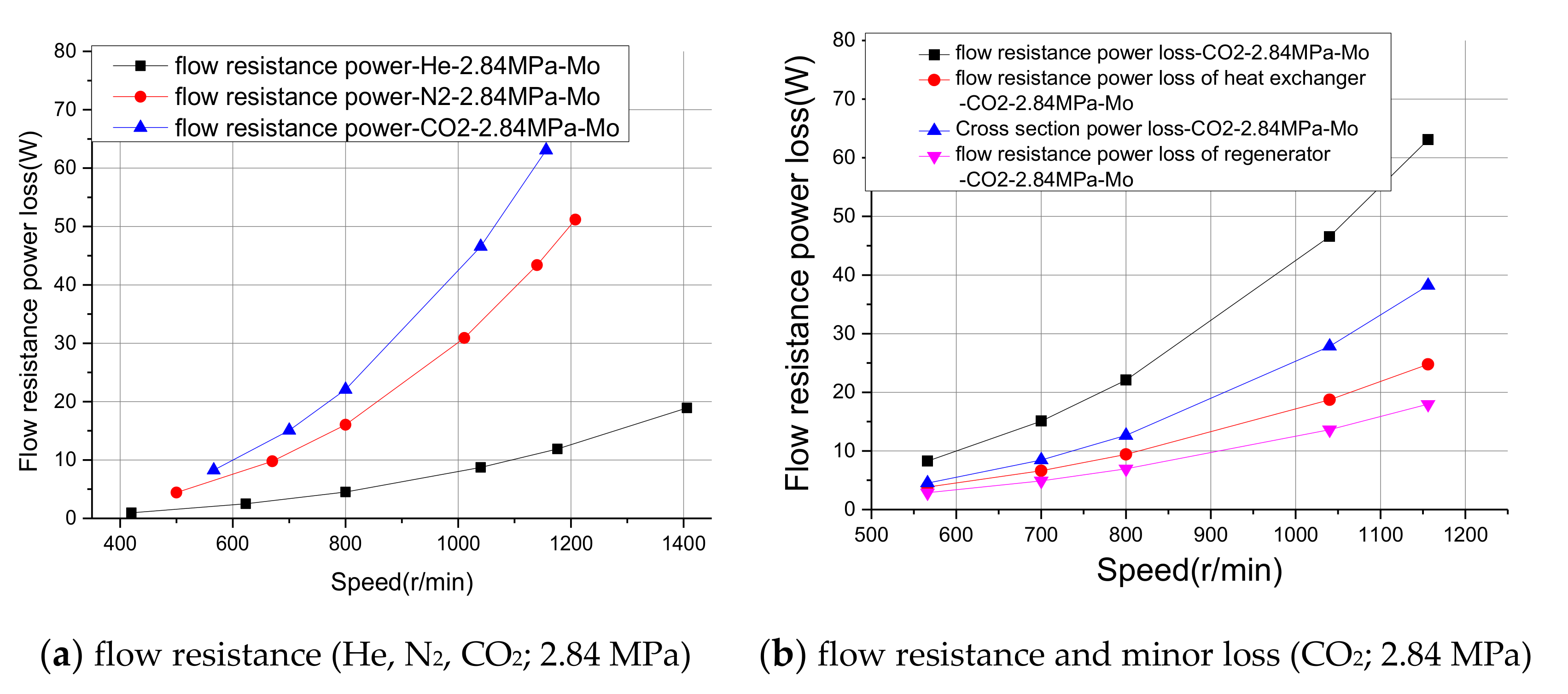
| Power losses | flow resistance loss of heat exchanger (W) [31] | Wfr: flow resistance loss of heat exchanger (W) Cref: Reynolds friction factor V: void volume (m3) A0: cross-sectional (free flow) area (m2) | |
| cross-section mutation power loss (W) [28,32] | hj: cross-sectional loss coefficient A1: small free flow area (m2) A2: large free flow area(m2) | ||
| gas spring hysteresis power loss (W) [33] | Wgp: gas spring hysteresis power loss (W) γ: insulation factor Tw: average wall temperature (K) Pmean: average pressure (MPa) ΔV: volume amplitude (m3) VB: mean volume of the gap spring cavity (m3) Aw: mean wetted area (m2) | ||
| seal leakage power loss (W) [33,34] | Wleak: seal leakage power loss (W) qmleak: leakage mass flow (kg·s−1) Tleak: leakage gas temperature (K) ΔP: pressure difference (MPa) Dc: cylinder diameter (m) h0: cylinder wall roughness up: piston velocity (ms−1) L: piston ring axial length (m) | ||
| Type of model | Cycle Power (W) | Error of Cycle Power | Cycle Efficiency (%) | Error of Cycle Efficiency |
|---|---|---|---|---|
| Experiment [2] | 3958 | 35 | ||
| Adiabatic model (Urieli and Berchowitz [4]) | 8300 | 109.7% | 62.5 | 78.6% |
| Simple model (Urieli and Berchowitz [4]) | 6700 | 69.3% | 52.5 | 50.0% |
| Dynamic best model (Timoumi [30]) | 4273 | 8.3% | 38.49 | 10.0% |
| CAFS model (Hosseinzade [7]) | 4107 | 3.8% | 36.2 | 3.4% |
| Fawad’s optimization model [5] | 4507 | 13.9% | 36.56 | 4.5% |
| Improved Simple analysis model in this paper | 4256 | 7.5% | 35.3 | 0.9% |
| Experimental Value | Mean Pressure (MPa) | Speed (r/min) | Cycle Power (W) | Cycle Efficiency (%) | Indicated Work (J) | Shaft Power (W) | Electric Power (W) | Pressure Difference (MPa) | tge (°C) | tgc (°C) |
|---|---|---|---|---|---|---|---|---|---|---|
| He | 2.84 | 1040 | 148.02 | 13.98 | 8.54 | 82.65 | 51 | 0.77 | 469 | 53.8 |
| N2 | 2.84 | 1011 | 138.06 | 12.08 | 8.19 | 53.5 | 24 | 0.73 | 472.2 | 48.2 |
| CO2 | 2.84 | 1040 | 140 | 11.26 | 8.1 | 46.37 | 12 | 0.71 | 473.9 | 40.7 |
| Numerical Value | Cycle Power (W) | Indicated Work (J) | Cycle Efficiency (%) | Heat Conduction Loss (W) | Regenerative Heat Loss (W) | Shuttle Heat Loss (W) | Flow Resistance Loss (W) | Leakage Loss (W) | Spring Hysteresis Loss (W) | Flow Resistance Loss of Regenerator (W) |
| He | 161.34 | 9.31 | 15.22 | 407.5 | 41.6 | 62.5 | 8.7 | 42.4 | 4 | 5.3 |
| N2 | 150.17 | 8.91 | 12.53 | 407.1 | 132.5 | 10.8 | 30.9 | 27.4 | 1 | 10.6 |
| CO2 | 157.1 | 9.06 | 12.96 | 407.3 | 182.4 | 11.4 | 46.6 | 16.6 | 0.9 | 13.6 |
| Working Gas | Mean Pressure (MPa) | Speed (r/min) | Tc-Co (K) | Te-Co (K) | Indicated Work-Coo (J) | Cycle Power-Coo (W) | Te-He (K) | Tc-He (K) | Indicated Work-He (J) | Cycle Power-He (W) |
|---|---|---|---|---|---|---|---|---|---|---|
| He | 1.96 | 650 | 299.30 | 222.11 | 5.46 | 59.19 | 746.39 | 315.74 | 6.31 | 68.35 |
| He | 1.96 | 800 | 301.02 | 214.73 | 5.99 | 79.89 | 746.42 | 318.62 | 6.28 | 83.69 |
| He | 1.96 | 1058 | 304.26 | 198.33 | 6.84 | 120.55 | 744.32 | 322.20 | 6.03 | 106.50 |
| He | 1.96 | 1200 | 306.50 | 194.20 | 7.20 | 144.00 | 743.43 | 323.36 | 5.91 | 117.30 |
| Working Gas | Mean Pressure (MPa) | Speed (r/min) | Tc-Co (K) | Te-Co (K) | Indicated Work-Coo (J) | Cycle Power-Coo (W) | Te-He (K) | Tc-He (K) | Indicated Work-He (J) | Cycle Power-He (W) |
|---|---|---|---|---|---|---|---|---|---|---|
| He | 1.42 | 800 | 298.61 | 224.00 | 4.32 | 57.66 | 747.25 | 316.05 | 3.93 | 60.40 |
| He | 1.96 | 800 | 301.02 | 214.73 | 5.99 | 79.89 | 746.42 | 318.62 | 6.28 | 83.69 |
| He | 2.15 | 800 | 301.39 | 205.64 | 6.52 | 86.96 | 745.52 | 319.63 | 6.70 | 89.36 |
| He | 2.41 | 800 | 302.30 | 197.80 | 7.28 | 97.07 | 745.47 | 320.02 | 7.39 | 98.53 |
| He | 2.84 | 800 | 304.10 | 191.00 | 8.30 | 110.67 | 743.58 | 322.27 | 8.70 | 116.03 |
| Mean Pressure (MPa) | A |
|---|---|
| 1.42 | 0.211 |
| 1.96 | 0.276 |
| 2.15 | 0.297 |
| 2.41 | 0.316 |
| 2.84 | 0.351 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, S.; Liu, B.; Xiao, G.; Ni, M. A Potential Method to Predict Performance of Positive Stirling Cycles Based on Reverse Ones. Energies 2021, 14, 7040. https://doi.org/10.3390/en14217040
Wang S, Liu B, Xiao G, Ni M. A Potential Method to Predict Performance of Positive Stirling Cycles Based on Reverse Ones. Energies. 2021; 14(21):7040. https://doi.org/10.3390/en14217040
Chicago/Turabian StyleWang, Shulin, Baiao Liu, Gang Xiao, and Mingjiang Ni. 2021. "A Potential Method to Predict Performance of Positive Stirling Cycles Based on Reverse Ones" Energies 14, no. 21: 7040. https://doi.org/10.3390/en14217040
APA StyleWang, S., Liu, B., Xiao, G., & Ni, M. (2021). A Potential Method to Predict Performance of Positive Stirling Cycles Based on Reverse Ones. Energies, 14(21), 7040. https://doi.org/10.3390/en14217040






