Modeling of Tractor Fuel Consumption
Abstract
1. Introduction
2. Materials and Methods
2.1. Machine Unit
- Instantaneous fuel consumption Gt—with a fuel gauge Flowtronic 215.
- Actual linear velocity of the tractor v—with a radar II DICKEY-JOHN type DJRVSII.
- Oil pressure ph in the hydraulic system of the implement linkage system—P8AP pressure sensor (20 MPa) by HBM.
- Implement position with reference to the tractor hp—mutual inductance sensor (LVDT) Bosch EHR system sensor.
- Force in the upper linkage bar Flg—custom made, calibrated tensometer.
- Force in linkage lower bars, left Fll and right Flp—elastomagnetic sensors, Bosch EHR system sensor.
- Force in hangers, left Fwl and right Fwp—custom made, calibrated tensometers;
- Angular velocity of the tractor’s rear wheels ωkr—induction sensors PCID-4 ZN (2 pcs).
- Torques of rear wheels, left Twl and right Twp—custom made, calibrated tensometers.
2.2. Tractor Performance Indicators
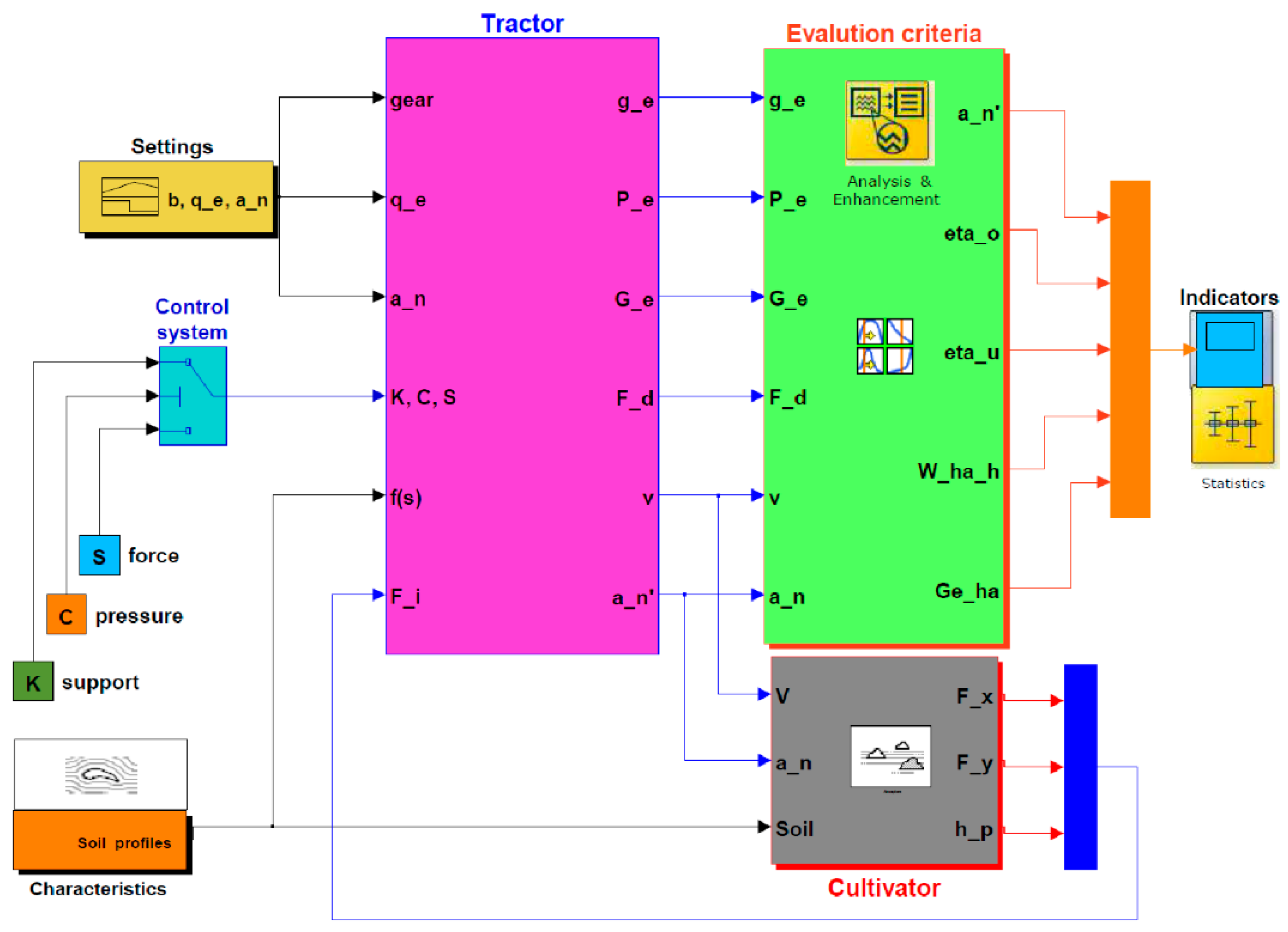
2.3. Simulation Model
- Settings—to introduce the setting for the soil cutting depth, a gear choice, accelerator level position (to maximum setting);
- Controlling system—to select one of the three implement linkage systems, i.e., K—support, C—pressure, or S—force;
- Soil profile characteristics—values describing physical and mechanical properties of individual soil types;
- Cultivator—to determine resistance forces for soil cutting as a function of the three-point linkage position;
- Evaluation criteria—to determine performance indicators according to the assumed criteria;
- Tractor—contains five subsystems. The diagram of the tractor’s power unit is shown in Figure 6.
- Perkins AD 3.152 engine of the MF 235 tractor,
- Drive train,
- Tractor hull—rear drive wheels,
- Hydraulic and automatic system,
- Implement linkage system (TUZ).
3. Results
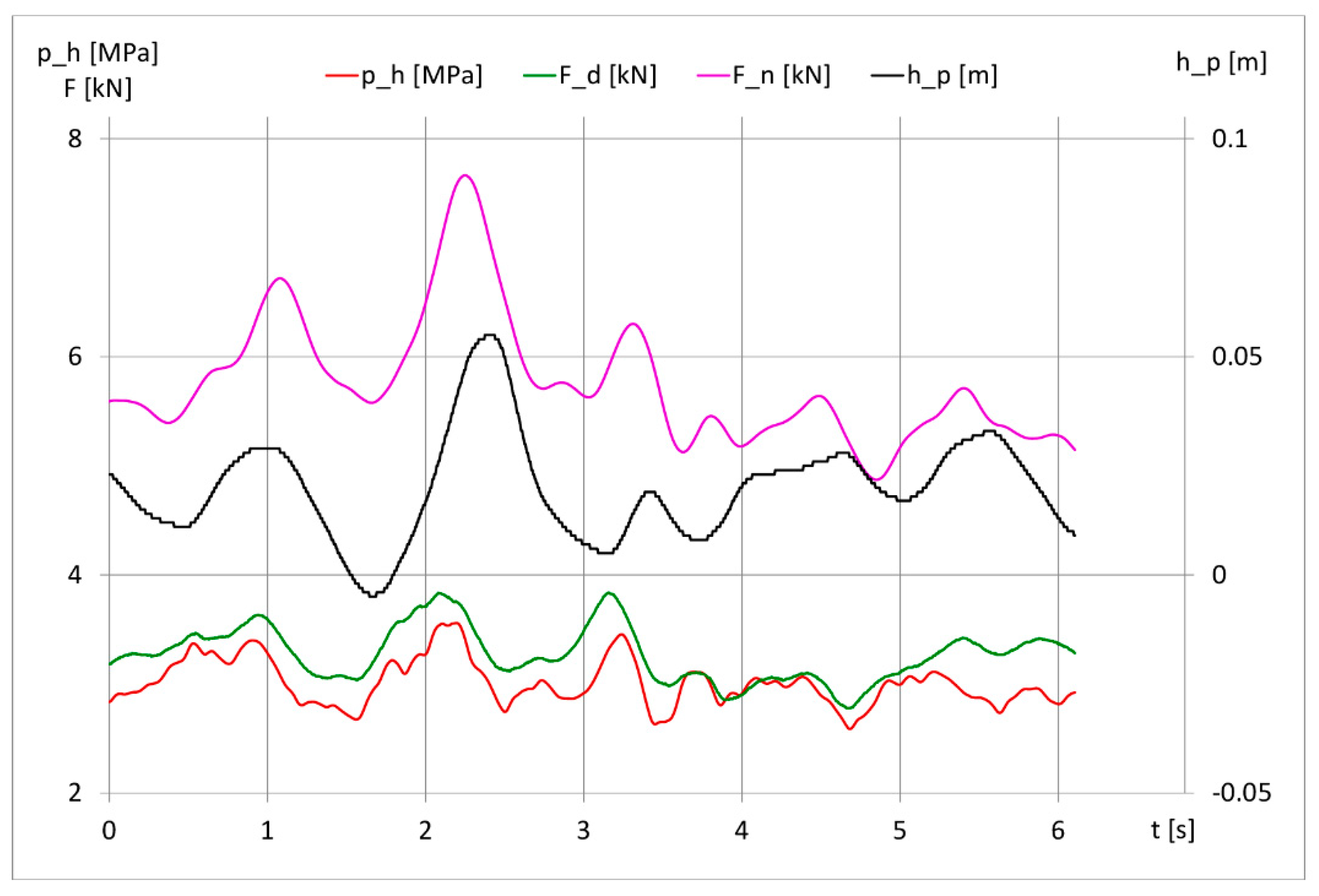
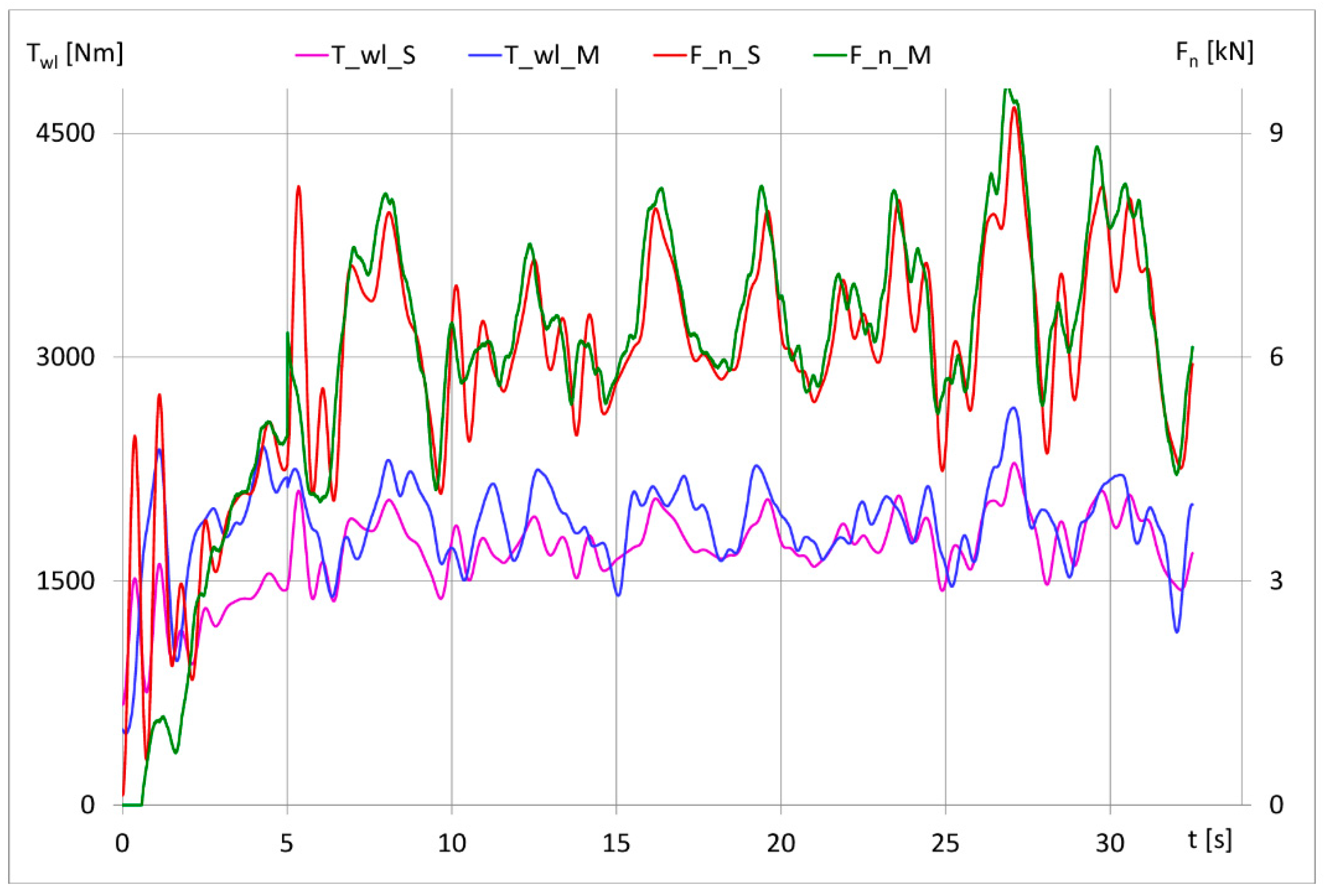
3.1. Validation of Simulation Model
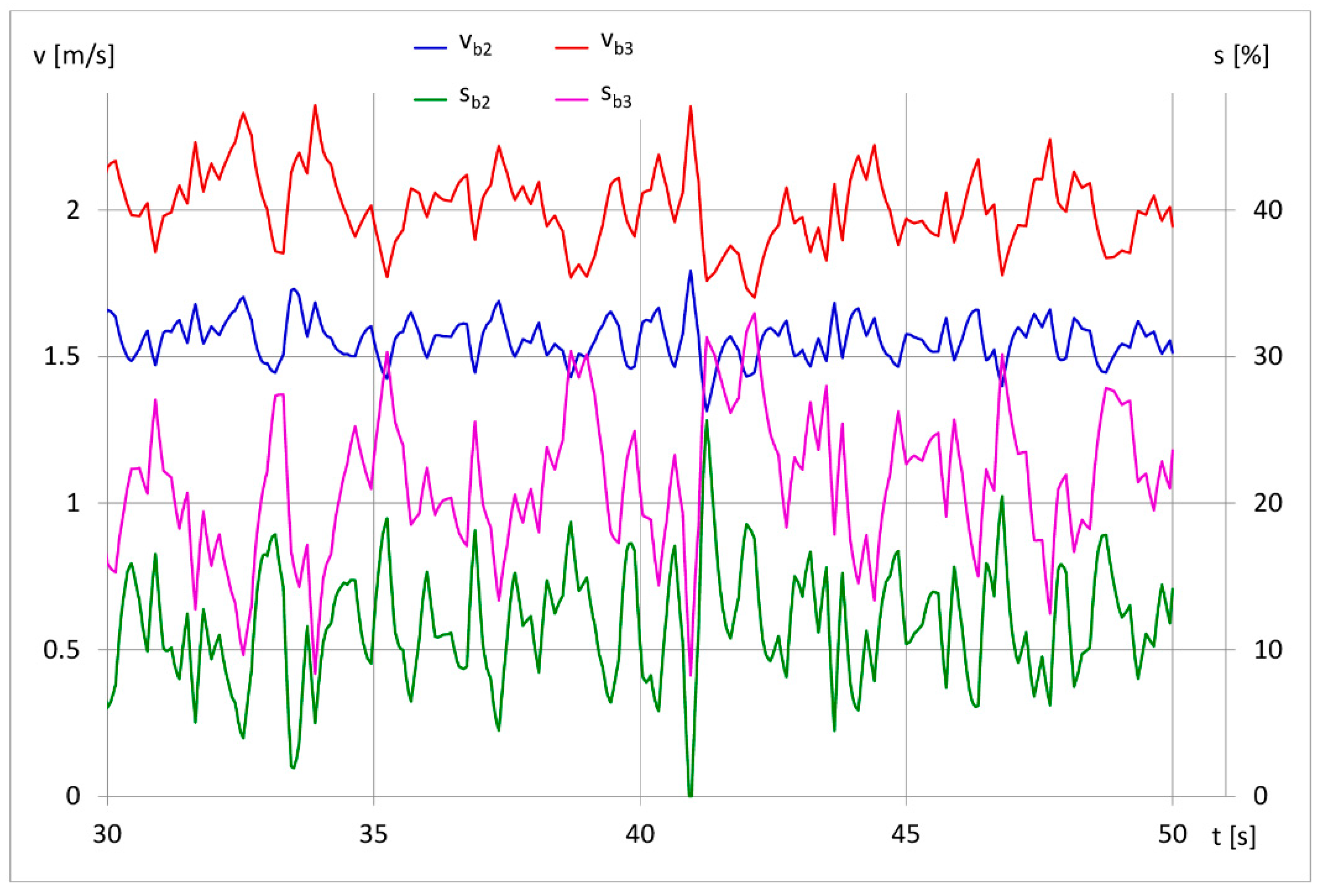
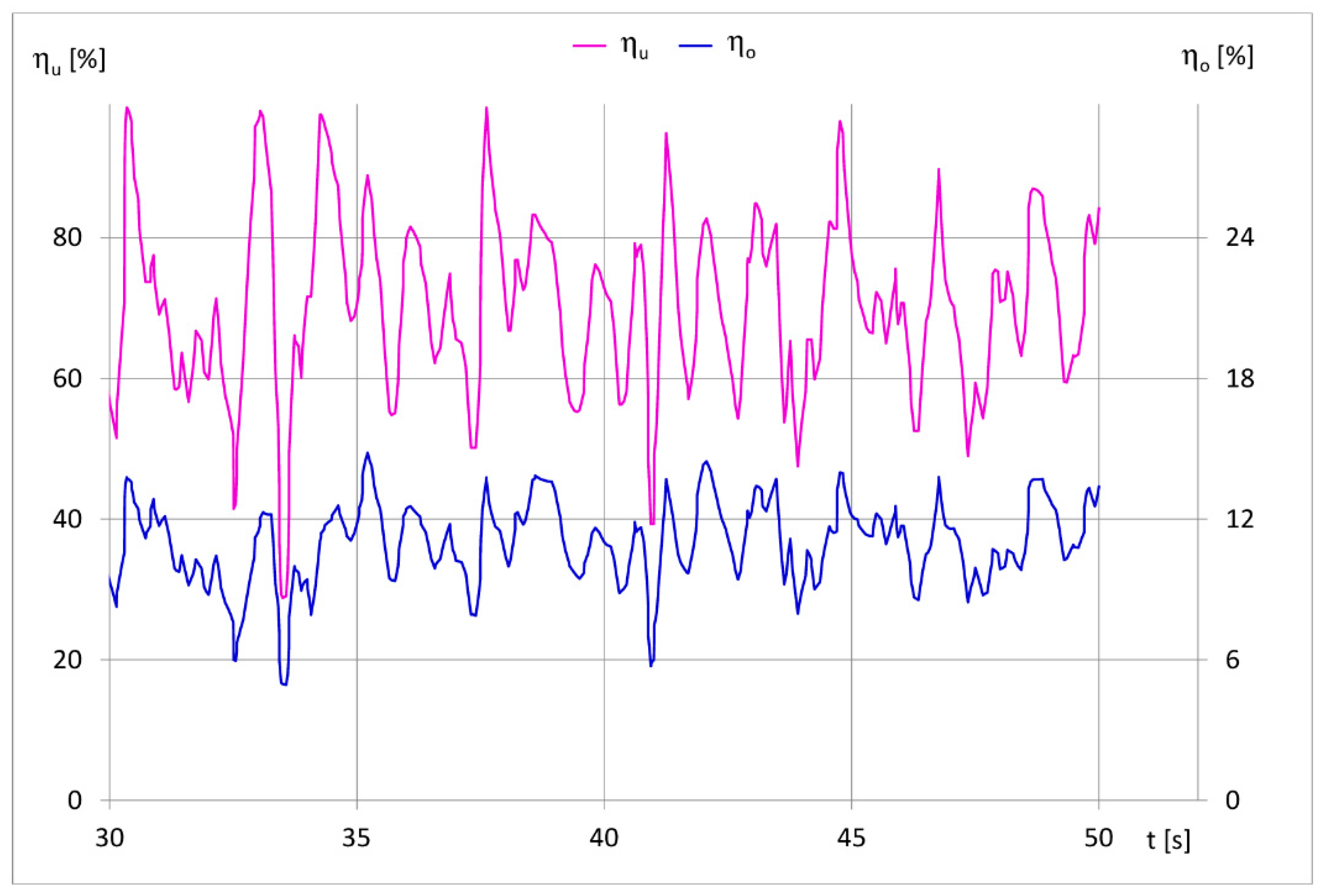
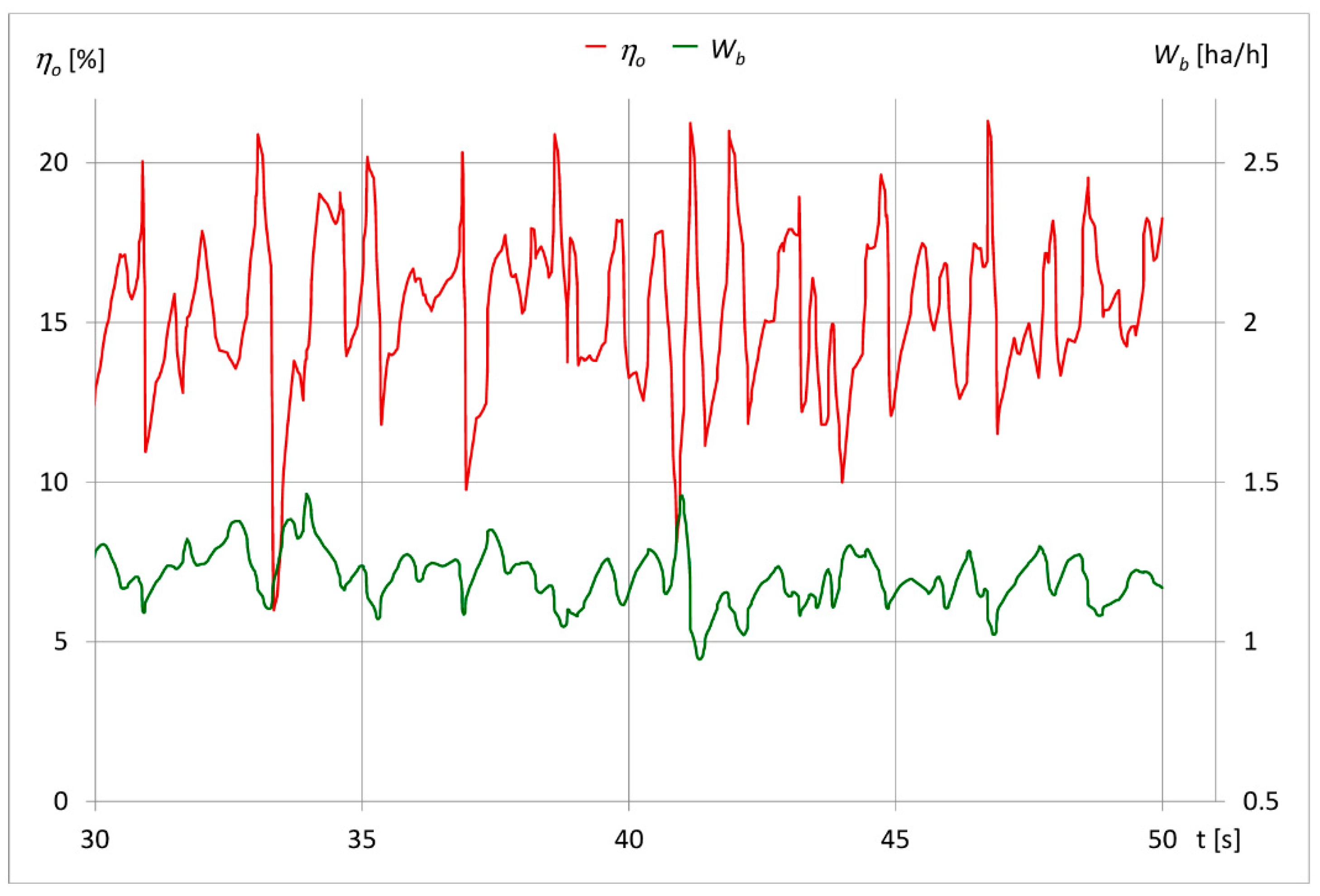
3.2. Simulation Results and Their Analysis
4. Conclusions
Funding
Conflicts of Interest
Nomenclature
| Parameter | Explanation |
| an | soil cutting depth (m) |
| B1, B2 | empirical coefficients of implement resistance |
| Flg | force in upper linkage bar (N) |
| Fwl | force in left hanger (N) |
| Fwp | force in right hanger (N) |
| hp | height of the implement position (m) |
| ge | specific fuel consumption (g kWh−1) |
| Gt | instantaneous fuel consumption (dm3 s−1) |
| Pe | power of engine (kW), |
| PW | power delivered to drive wheels (kW) |
| Te | torque of engine (Nm) |
| Th | torque on the hydraulic pump shaft (Nm) |
| ωe | angular velocity of the engine shaft (rad s−1) |
| m | tractor weight (kg) |
| Oi | experimental values |
| Si | simulated values |
| s | drive wheel slip |
| µ | coefficient of wheel traction |
| Wd | calorific value of fuel (kJ kg−1), |
| Fi | resultant of the resistance force of the tool affecting the tractor (N) |
| bn | soil cutting width (m) |
| Fn | force driving tractor’s rear wheels (N) |
| Fd | drawbar pull of the tractor (N) |
| Fll | force in left lower linkage bar (N) |
| Flp | force in right lower linkage bar (N) |
| Ge | fuel consumption per hour (dm3 h−1) |
| Gp | fuel consumption per hectare (dm3 ha−1) |
| ph | oil pressure in the hydraulic system (MPa) |
| Pu | pulling power (kW) |
| Rr | resultant ground reaction forces on rear wheels (N) |
| ηm | power transmission efficiency |
| Tkl, Tkp | torque on the half-shaft of the right and left drive wheel (Nm) |
| ωw | angular velocity of the drive wheel (rad s−1) |
| rdr | dynamic radius of rear wheel (m) |
| Omean | mean of experimental values |
| vt | theoretical tractor velocity (m s−1) |
| v | actual tractor velocity (m s−1) |
| ηu, ηo | tractive and overall tractor efficiency |
| Wb | area productivity (ha h−1) |
| S, K, C | control system of the implement linkage system (force, support, pressure) |
References
- Zoz, F.M. Predicting Tractor Field Performance (Updated); Paper 87-1623; ASAE: St. Joseph, MI, USA, 1987; p. 12. [Google Scholar]
- Sharma, A.K.; Pandey, K.P. Modelling power requirement for traction tyres with zero sinkage. J. Terramech. 1997, 34, 13–21. [Google Scholar] [CrossRef]
- Shmulevich, I.; Osetinsky, A. Traction performance of a pushed/pulled drive wheel. Trans. ASAE 2004, 47, 981–994. [Google Scholar] [CrossRef]
- Elwaleed, A.K.; Yahya, A.; Zohadie, M.; Ahmad, D.; Kheiralla, A.F. Net traction ratio prediction for high-lug agricultural tyre. J. Terramech. 2006, 43, 119–139. [Google Scholar] [CrossRef]
- Schreiber, M.; Kutzbach, H.D. Comparison of different zero-slip definitions and a proposal to standardize tire traction performance. J. Terramech. 2007, 44, 75–79. [Google Scholar] [CrossRef]
- Simikić, M.; Dedović, N.; Savin, L.; Tomić, M.; Ponjičan, O. Power delivery efficiency of a wheeled tractor at oblique drawbar force. Soil Tillage Res. 2014, 141, 32–43. [Google Scholar] [CrossRef]
- Battiato, A.; Diserens, E. Tractor traction performance simulation on differently textured soils and validation: A basic study to make traction and energy requirements accessible to the practice. Soil Tillage Res. 2017, 166, 18–32. [Google Scholar] [CrossRef]
- Jenane, C.; Bashford, L.L.; Monroe, G. Reduction of fuel consumption through improved tractive performance. J. Agric. Eng. Res. 1996, 64, 131–137. [Google Scholar] [CrossRef]
- Sahay, C.S.; Tewari, V.K. Computer simulation of tractor single-point drawbar performance. Biosyst. Eng. 2004, 88, 419–428. [Google Scholar] [CrossRef]
- Portes, P.; Bauer, F.; Ćupera, J. Laboratory-experimental verification of calculation of force effects in tractor’s three-point hitch acting on driving wheels. Soil Tillage Res. 2013, 128, 81–90. [Google Scholar] [CrossRef]
- Kazemi, N.; Almassi, M.; Bahrami, H.; Shaykhdavoodi, M.J.; Mesgarbashi, M. Analysis of Factors Affecting the Management of Overall Energy Efficiency of Tractor-Implement by Real-Time Performance Monitoring. J. Agric. Mach. 2014, 4, 214–225. [Google Scholar]
- Lee, J.W.; Kim, J.S.; Kim, K.U. Computer simulations to maximise fuel efficiency and work performance of agricultural tractors in rotovating and ploughing operations. Biosyst. Eng. 2016, 142, 1–11. [Google Scholar] [CrossRef]
- Keen, A.; Ward, J.; Godwin, R.; Cooper, S.; Hall, N. Improvements to the Tractive Efficiency of Agricultural Tractors Carrying out Cultivations; Paper Number: 096639; ASABE: St. Joseph, MI, USA, 2009. [Google Scholar]
- Al-Janobi, A. A data-acquisition system to monitor performance of fully mounted implements. J. Agric. Eng. Res. 2000, 75, 167–175. [Google Scholar] [CrossRef]
- Kim, Y.-S.; Kim, W.-S.; Siddique, M.A.A.; Baek, S.-Y.; Baek, S.-M.; Cheon, S.-H.; Lee, S.-D.; Lee, K.-H.; Hong, D.-H.; Park, S.-U.; et al. Power Transmission Efficiency Analysis of 42 kW Power Agricultural Tractor According to Tillage Depth during Moldboard Plowing. Agronomy 2020, 10, 1263. [Google Scholar] [CrossRef]
- Ashok Kumar, A.; Tewari, V.K.; Nare, B. Embedded digital draft force and wheel slip indicator for tillage research. Comput. Electron. Agric. 2016, 127, 38–49. [Google Scholar] [CrossRef]
- Čupera, J.; Bauer, F.; Severa, L.; Tatíček, M. Analysis of force effects measured in the tractor three-point linkage. Res. Agric. Eng. 2011, 57, 79–87. [Google Scholar] [CrossRef]
- Md-Tahir, H.; Zhang, J.; Xia, J.; Zhou, Y.; Zhou, H.; Du, J.; Sultan, M.; Mamona, H. Experimental Investigation of Traction Power Transfer Indices of Farm-Tractors for Efficient Energy Utilization in Soil Tillage and Cultivation Operations. Agronomy 2021, 11, 168. [Google Scholar] [CrossRef]
- Al-Hamed, S.A.; Al-Janobi, A.A. A program for predicting tractor performance in Visual C++. Comput. Electron. Agric. 2001, 31, 137–149. [Google Scholar] [CrossRef]
- Grisso, R.D.; Kocher, M.F.; Vaughan, D.H. Predicting Tractor Fuel Consumption. Appl. Eng. Agric. 2004, 20, 553–561. [Google Scholar] [CrossRef]
- Kolator, B.; Białobrzewski, I. A simulation model of 2WD tractor performance. Comput. Electron. Agric. 2011, 76, 231–239. [Google Scholar] [CrossRef]
- Kim, W.S.; Kim, Y.J.; Chung, S.O.; Lee, D.H.; Choi, C.H.; Yoon, Y.H. Development of simulation model for fuel efficiency of agricultural tractor. Korean J. Agric. Sci. 2016, 43, 116–126. [Google Scholar] [CrossRef][Green Version]
- Li, B.; Sun, D.; Hu, M.; Liu, J. Research on Economic Comprehensive Control Strategies of Tractor-Planter Combinations in Planting, Including Gear-Shift and Cruise Control. Energies 2018, 11, 686. [Google Scholar] [CrossRef]
- Li, T.; Xie, B.; Li, Z.; Li, J. Design and Optimization of a Dual-Input Coupling Powertrain System: A Case Study for Electric Tractors. Appl. Sci. 2020, 10, 1608. [Google Scholar] [CrossRef]
- McBratney, A.; Whelan, B.; Ancev, T.; Bouma, J. Future directions of precision agriculture. Precis. Agric. 2005, 6, 7–23. [Google Scholar] [CrossRef]
- Heywood, J.B. Internal Combustion Engine Fundamentals; McGraw-Hill Book Company: New York, NY, USA, 1988. [Google Scholar]
- Olszewski, A. Wpływ Sterowania Trzypunktowym Układem Zawieszenia Narzędzia na Efektywność Funkcjonowania Agregatu Ciągnikowego. Ph.D. Thesis, Uniwersytet Warmińsko-Mazurski, Olsztyn, Poland, 2015. [Google Scholar]
- Muleta, M.K. Model performance sensitivity to objective function during automated calibrations. J. Hydrol. Eng. 2011, 17, 756–767. [Google Scholar] [CrossRef]
- Barbosa, R.N.; Wilkerson, J.B.; Denton, H.P.; Yoder, D.C. Slope Gradient and Vehicle Attitude Definition Based on Pitch and Roll Angle Measurements: A Simplified Approach. Open Agric. J. 2012, 6, 36–40. [Google Scholar] [CrossRef]







| Values Assumed for Validation | Values of Modified NS Coefficient | |
|---|---|---|
| Full Range Time | 5–32 s Range | |
| angular velocity of the drive wheel ωw | 0.50 | 0.50 |
| linear velocity of the unit vp | 0.65 | 0.55 |
| torque of the drive wheel axle shaft Tw | −0.15 | 0.05 |
| driving force of the tractor Fn | −1.31 | −1.18 |
| Soil Profile | Setting an | Gear | Mean Indicator Values | |||||
|---|---|---|---|---|---|---|---|---|
| anS | s | ηu | ηo | Wb | Gp | |||
| m | m | % | % | % | ha h−1 | dm3 ha−1 | ||
| Loamy sand | 0.130 | I | 0.127 | 6.4 | 65.81 | 5.94 | 0.977 | 3.554 |
| II | 0.126 | 8.5 | 69.22 | 9.15 | 1.274 | 3.160 | ||
| III | 0.123 | 13.8 | 69.09 | 12.92 | 1.754 | 3.470 | ||
| 0.190 | I | 0.187 | 8.7 | 70.26 | 7.87 | 0.950 | 3.894 | |
| II | 0.186 | 11.7 | 73.20 | 11.42 | 1.224 | 3.676 | ||
| III | 0.185 | 22.9 | 64.95 | 12.73 | 1.550 | 4.646 | ||
| Silty loam | 0.130 | I | 0.125 | 9.2 | 68.73 | 7.72 | 0.947 | 3.945 |
| II | 0.127 | 13.1 | 71.28 | 11.02 | 1.205 | 3.784 | ||
| III | 0.129 | 24.2 | 62.87 | 12.32 | 1.521 | 4.922 | ||
| 0.190 | I | 0.188 | 13.1 | 73.15 | 9.99 | 0.900 | 4.529 | |
| II | 0.188 | 19.8 | 69.88 | 12.36 | 1.107 | 4.774 | ||
| III | 0.185 | 33.8 | 60.03 | 11.94 | 1.305 | 6.123 | ||
| Medium clay | 0.130 | I | 0.126 | 7.7 | 74.11 | 9.59 | 0.967 | 4.208 |
| II | 0.128 | 10.3 | 74.75 | 12.93 | 1.241 | 4.332 | ||
| III | 0.132 | 16.7 | 73.65 | 15.44 | 1.449 | 5.040 | ||
| 0.190 | I | 0.189 | 10.9 | 77.92 | 12.42 | 0.921 | 5.069 | |
| II | 0.192 | 17.0 | 74.33 | 14.30 | 1.132 | 5.844 | ||
| III | 0.194 | 19.8 | 73.22 | 15.83 | 1.176 | 5.651 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kolator, B.A. Modeling of Tractor Fuel Consumption. Energies 2021, 14, 2300. https://doi.org/10.3390/en14082300
Kolator BA. Modeling of Tractor Fuel Consumption. Energies. 2021; 14(8):2300. https://doi.org/10.3390/en14082300
Chicago/Turabian StyleKolator, Bronisław Andrzej. 2021. "Modeling of Tractor Fuel Consumption" Energies 14, no. 8: 2300. https://doi.org/10.3390/en14082300
APA StyleKolator, B. A. (2021). Modeling of Tractor Fuel Consumption. Energies, 14(8), 2300. https://doi.org/10.3390/en14082300






