Determination and Analysis of Joule’s Heat and Temperature in an Electrically Conductive Plate Element Subject to Short-Term Induction Heating by a Non-Stationary Electromagnetic Field
Abstract
1. Introduction
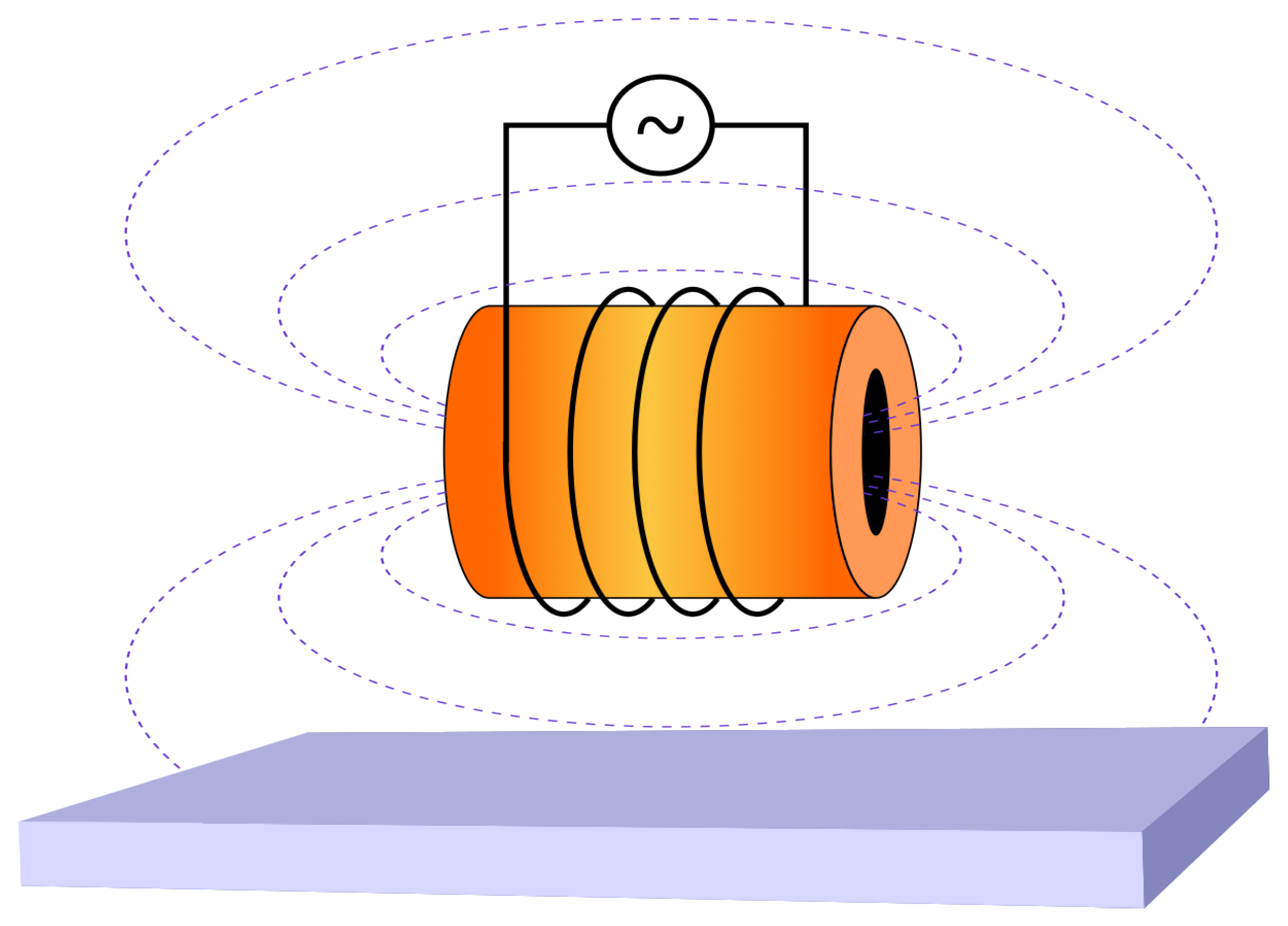
2. Mathematical Model. Problem Statement
2.1. Determination of NEMF
2.2. Determination of Thermal Field
2.3. Methodology of Solving the Formulated Initial-Boundary Problems
3. Results and Discussion
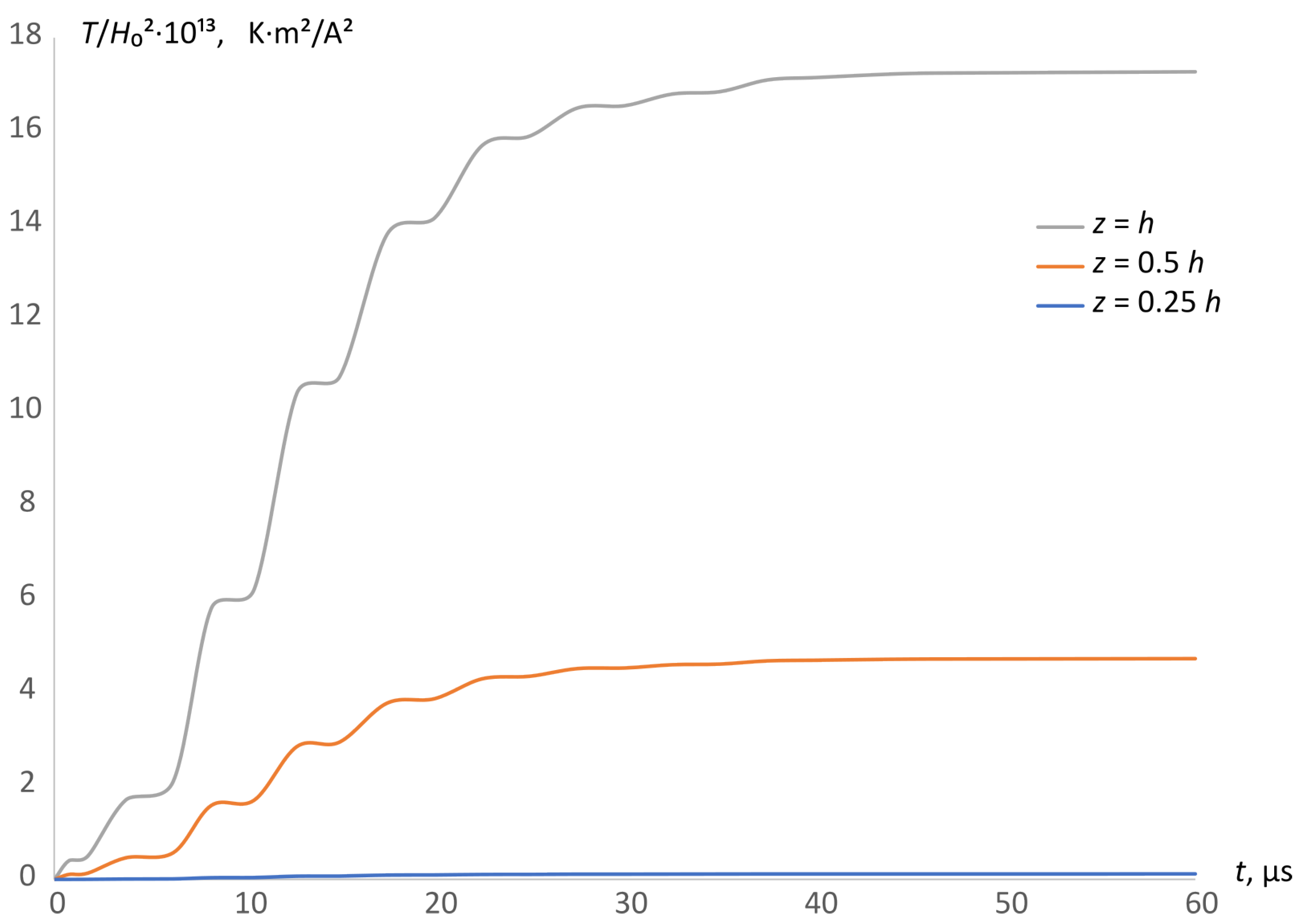
3.1. Research of Thermal Behaviour of the Plate Element Subject to Short-Term Conductive Heating by NEMF in MIMS
3.2. Numerical Analysis
4. Conclusions
- 1
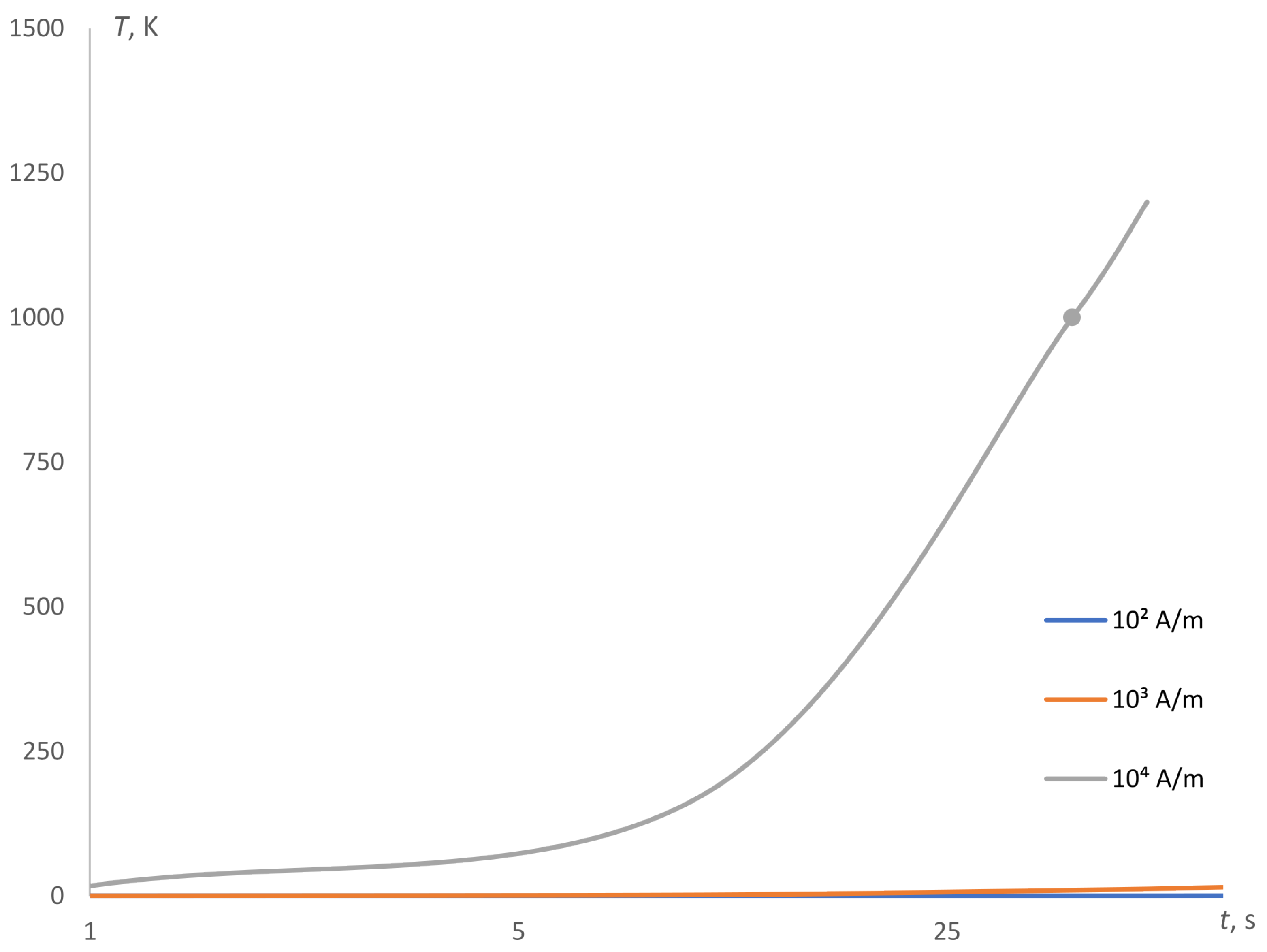
- There are obtained parameters (time duration and strength) of the optimal modes at short-term induction heating of an electrically conductive alloy steel plate element with chrome, nickel and titanium:
- (a)
- Time duration of inductive heating NEMF values are found within the limits from 10 s to 40–50 s;
- (b)
- The value of electromagnetic field strength is established approximately as A/m as well;
- (c)
- Strength value A/m is optimal for the sufficient temperature level under the short-term inductive heating. In particular, while 40–50 s, the melting temperature of the plate element cannot be obtained. That is why the considered mathematical model is valid for low temperatures;
- (d)
- Minor values A/m or A/m are not effective for inductive short-term heating.
- 2
- In the case of stationary electromagnetic fields, the strength is mostly used with values = 10–10 A/m. So the heating process needs a substantially longer time duration in comparison to A/m under NEMF action to achieve the necessary temperature values. In particular, we are interested in temperature K because it is widely used in technological thermal heating. NEMF, perhaps, does not lead to an essential energies economy, but we can state that the short time duration is an advantage of our method. Besides that, under short-time heating mode it is easier to satisfy the necessary strength value A/m.
- 3
- Analytical function takes into account the moments of switching on and off for generators of high frequency electromagnetic fluctuations.
- 4
- Qualitative and quantitative results in the paper are applicable to describing short-time induction heating modes of the electrical conductive plate elements in different devices while technologically processing. Finally, it is worth mentioning that the proposed method can be used to study similar problems.
- 5
- The obtained NEMF characteristics may serve as a theoretical basis for forecasting the rational modes of electrically conductive plate element processing by induction heating and can also be used to predict the optimal parameters (frequency, duration of thermal heating, strength) of the electromagnetic field and choose the rational mode of thermal functioning of the plate element as a constructive part of the technological equipment, in particular in aerospace vehicles and robotized systems etc.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| NEMF | Non-stationary electromagnetic field |
| MIMS | Mode of impulse modulating signal |
References
- Serpilli, M.; Dumont, S.; Rizzoni, R.; Lebon, F. Interface Models in Coupled Thermoelasticity. Technologies 2021, 9, 17. [Google Scholar] [CrossRef]
- Hetnarski, R. Encyclopedia of Thermal Stresses; Springer: Dordrecht, The Netherlands, 2014. [Google Scholar] [CrossRef]
- Batygin, Y.; Shinderuk, S.; Chaplygin, E. Mutual influence of currents in a flat inductor system with solenoid between two massive conductors. Electr. Eng. Electromech. 2021, 6, 25–30. [Google Scholar] [CrossRef]
- Asai, S. Electromagnetic Processing of Materials; Springer: Dordrecht, The Netherlands, 2012. [Google Scholar] [CrossRef]
- Smetana, M.; Capova, K.; Chudacik, V.; Palcek, P.; Oravcova, M. Influence of the Heat Treatment on Magnetic Properties of Austenitic Steels. Stud. Appl. Electromagn. Mech. 2017, 42, 83–90. [Google Scholar] [CrossRef]
- Montoya, E.; Sebastian, T.; Schultheiss, H.; Heinrich, B.; Camley, R.; Celinski, Z. Magnetization Dynamics. In Handbook of Surface Science; North-Holland: Amsterdam, The Netherlands, 2015; Volume 5, pp. 113–167. [Google Scholar] [CrossRef]
- Hachkevych, O.; Musii, R. Mathematical modeling in thermomechanics of electroconductive bodies under the action of the pulsed electromagnetic fields with modulation of amplitude. Math. Model. Comput. 2019, 6, 30–36. [Google Scholar] [CrossRef]
- Bobart, G.F. Induction heating. AccessScience. 2020. Available online: https://www.accessscience.com/content/341500 (accessed on 2 June 2022).
- Touzani, R.; Rappaz, J. Mathematical Models for Eddy Currents and Magnetostatics With Selected Applications; Springer: Dordrecht, The Netherlands, 2013. [Google Scholar] [CrossRef]
- Gantsevich, S.; Gurevich, V. Joule heat release during current flow through a nanowire. Phys. Solid State 2016, 58, 1711–1715. [Google Scholar] [CrossRef]
- Torteman, B.; Kessler, Y.; Liberzon, A. Micro-beam resonator parametrically excited by electro-thermal Joule’s heating and its use as a flow sensor. Nonlinear Dyn. 2019, 98, 3051–3065. [Google Scholar] [CrossRef]
- Ibrahim, S.M.; Kumar, P.V.; Lorenzini, G.; Lorenzini, E. Influence of Joule Heating and Heat Source on Radiative MHD Flow over a Stretching Porous Sheet with Power-Law Heat Flux. J. Engin. Thermophys. 2019, 28, 332–344. [Google Scholar] [CrossRef]
- Moshizi, S.A.; Pop, I. Conjugated Effect of Joule Heating and Magnetohydrodynamic on Laminar Convective Heat Transfer of Nanofluids Inside a Concentric Annulus in the Presence of Slip Condition. Int. J. Thermophys. 2016, 37, 72. [Google Scholar] [CrossRef]
- Solov’ev, S. Influence of Joule Dissipation on Heat Exchange and Magnetic Hydrodynamics of Liquid in a Spherical Layer. Part I. J. Eng. Phys. Thermophy 2017, 90, 1251–1265. [Google Scholar] [CrossRef]
- Musii, R.; Melnyk, N.; Nakonechnyi, A.; Goshko, L.; Bandyrskyi, B. Determination and analysis of the temperature field of a continuous electrically conductive ball with short-term induction heating. Appl. Quest. Math. Model. 2021, 4, 149–158. (In Ukrainian) [Google Scholar] [CrossRef]
- Altenbach, H.; Naumenko, K.; Lavinsky, D.; Konkin, V. Variational Formulation of Problems of Thermodeformation of Electrically Conductive Bodies in Electromagnetic Field. In Bulletin of NTU “KhPI”: Series “Dynamics and Strength of Machines”. 2019, pp. 3–6. Available online: http://jdsm.khpi.edu.ua/article/view/187409 (accessed on 2 June 2022). (In Ukrainian).
- Popov, V.; Shchukin, V. Numerical Simulation of Metal Surface Layer Modification Using High-Frequency Induction Heating. Inorg. Mater. Appl. Res 2019, 10, 616–621. [Google Scholar] [CrossRef]
- Bermúdez, A.; Gómez, D.; Muñiz, M.; Salgado, P.; Vázquez, R. Numerical Modelling of Industrial Induction. In Advances in Induction and Microwave Heating of Mineral and Organic Materials; IntechOpen: London, UK, 2011; Chapter 4. [Google Scholar] [CrossRef]
- Yi, H.Z.B.; Wang, J.; Zheng, X. Preliminary investigation on plate bending with multiple-line induction heating. J. Mar. Sci. Technol. 2020, 25, 455–466. [Google Scholar] [CrossRef]
- Bae, K.; Yang, Y.; Hyun, C. Analysis of triangle heating technique using high frequency induction heating in forming process of steel plate. Int. J. Precis. Eng. Manuf. 2012, 13, 539–545. [Google Scholar] [CrossRef]
- Glebov, A.; Karpov, S.; Karpushkin, S. Modeling of Three-Dimensional Fields of Eddy Currents during Induction Heating of Process Equipment. Russ. Electr. Engin. 2018, 89, 204–209. [Google Scholar] [CrossRef]
- Cao, Q.; Li, Z.; Lai, Z. Analysis of the effect of an electrically conductive die on electromagnetic sheet metal forming process using the finite element-circuit coupled method. Int. J. Adv. Manuf. Technol. 2019, 101, 549–563. [Google Scholar] [CrossRef]
- Madzharov, N.D.; Nemkov, V.S. Technological inductive power transfer systems. J. Electr. Eng. 2017, 68, 235–244. [Google Scholar] [CrossRef][Green Version]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Musii, R.; Pukach, P.; Kohut, I.; Vovk, M.; Šlahor, Ľ. Determination and Analysis of Joule’s Heat and Temperature in an Electrically Conductive Plate Element Subject to Short-Term Induction Heating by a Non-Stationary Electromagnetic Field. Energies 2022, 15, 5250. https://doi.org/10.3390/en15145250
Musii R, Pukach P, Kohut I, Vovk M, Šlahor Ľ. Determination and Analysis of Joule’s Heat and Temperature in an Electrically Conductive Plate Element Subject to Short-Term Induction Heating by a Non-Stationary Electromagnetic Field. Energies. 2022; 15(14):5250. https://doi.org/10.3390/en15145250
Chicago/Turabian StyleMusii, Roman, Petro Pukach, Ihor Kohut, Myroslava Vovk, and Ľudomír Šlahor. 2022. "Determination and Analysis of Joule’s Heat and Temperature in an Electrically Conductive Plate Element Subject to Short-Term Induction Heating by a Non-Stationary Electromagnetic Field" Energies 15, no. 14: 5250. https://doi.org/10.3390/en15145250
APA StyleMusii, R., Pukach, P., Kohut, I., Vovk, M., & Šlahor, Ľ. (2022). Determination and Analysis of Joule’s Heat and Temperature in an Electrically Conductive Plate Element Subject to Short-Term Induction Heating by a Non-Stationary Electromagnetic Field. Energies, 15(14), 5250. https://doi.org/10.3390/en15145250






