Techno-Economic Comparison of Utility-Scale Compressed Air and Electro-Chemical Storage Systems
Abstract
:1. Introduction
- Pb-Acid based installations ranging from 1 MW–1.4 MWh to 36 MW–24 MWh;
- Na-S facilities, from 1 MW–7 MWh to 34 MW–245 MWh;
- Li-ion based storage systems, from 6 MW–10 MWh to 32 MW–8 MWh;
- RFB based systems, from 0.2 MW–0.8 MWh to 2 MW–12 MWh.
2. Storage Systems Description and Modelling
2.1. D-CAES Plant Description and Modelling
2.2. BES Techno-Economic Model
3. Techno-Economic Performance Assessment
- one operating cycle per day at rated design condition;
- D-CAES lifetime equal to 30 years. It is foreseen the replacement of the GE after 15 years of operation;
- Na-S and Li-ion systems life duration assumed equal to 15 and 10 years respectively, as reported in Table 2;
- cost of natural gas used in D-CAES varying from 0.20 to 0.30 €/Sm3;
- annual interest rate equal to 5%.
- tCH = tDS = 6 h;
- tCH = tDS = 10 h
4. Conclusions
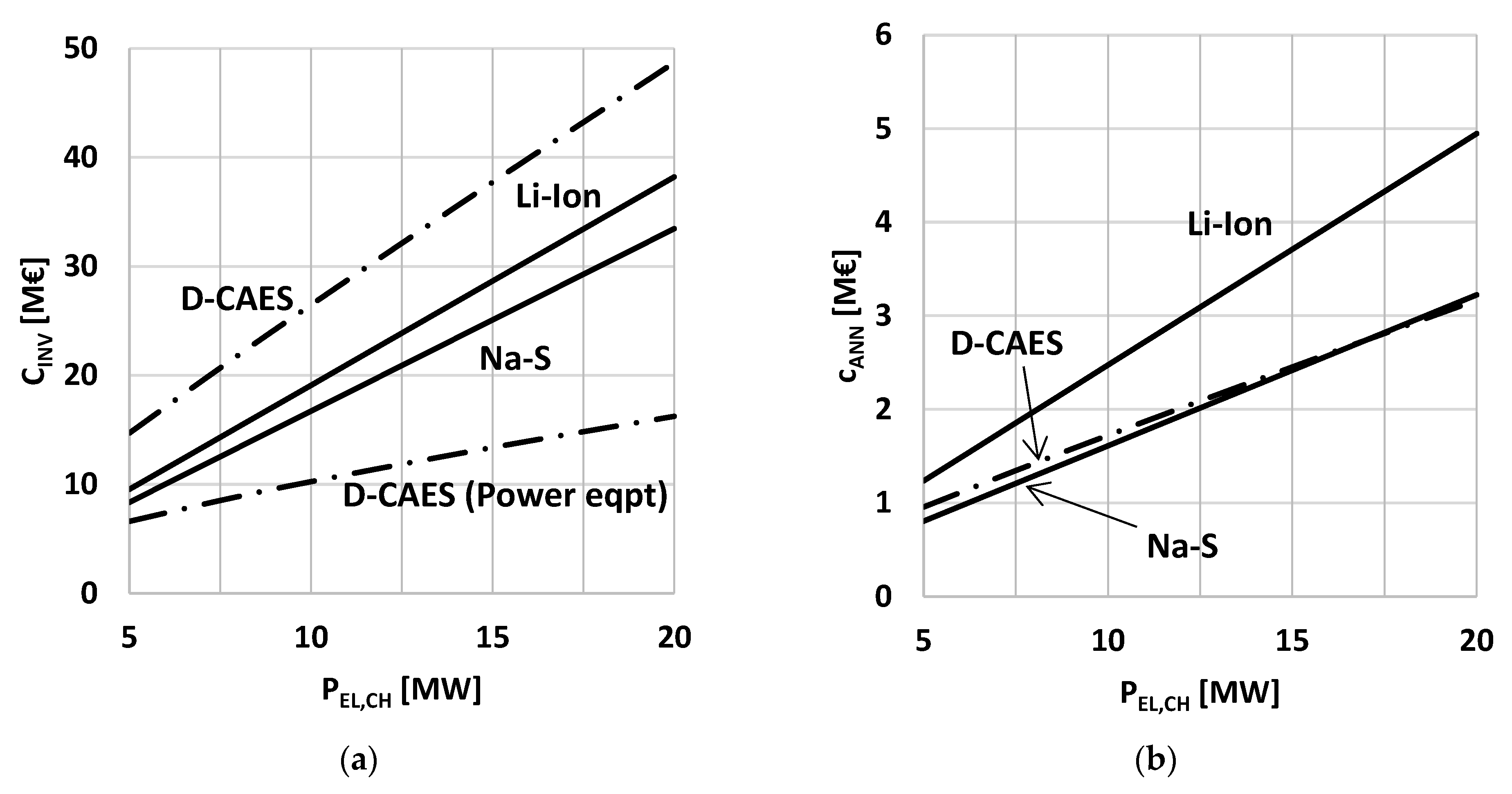
- D-CAES systems with artificial storage require investment cost significantly higher than Na-S and Li-Ion battery-based systems. Anyway, the really long lifetime of D-CAES system leads to annualized capital costs considerably lower than those of Li-ion and comparable to those evaluated for Na-S storage.
- In all cases taken into consideration, Na-S battery-based systems show a better economic performance in comparison with Li-ion based ones.
- The economic performance of both D-CAES and BES improves by increasing the storage capacity of the system. The D-CAES performance improvement rate, however, is higher than that estimated for BES based systems.
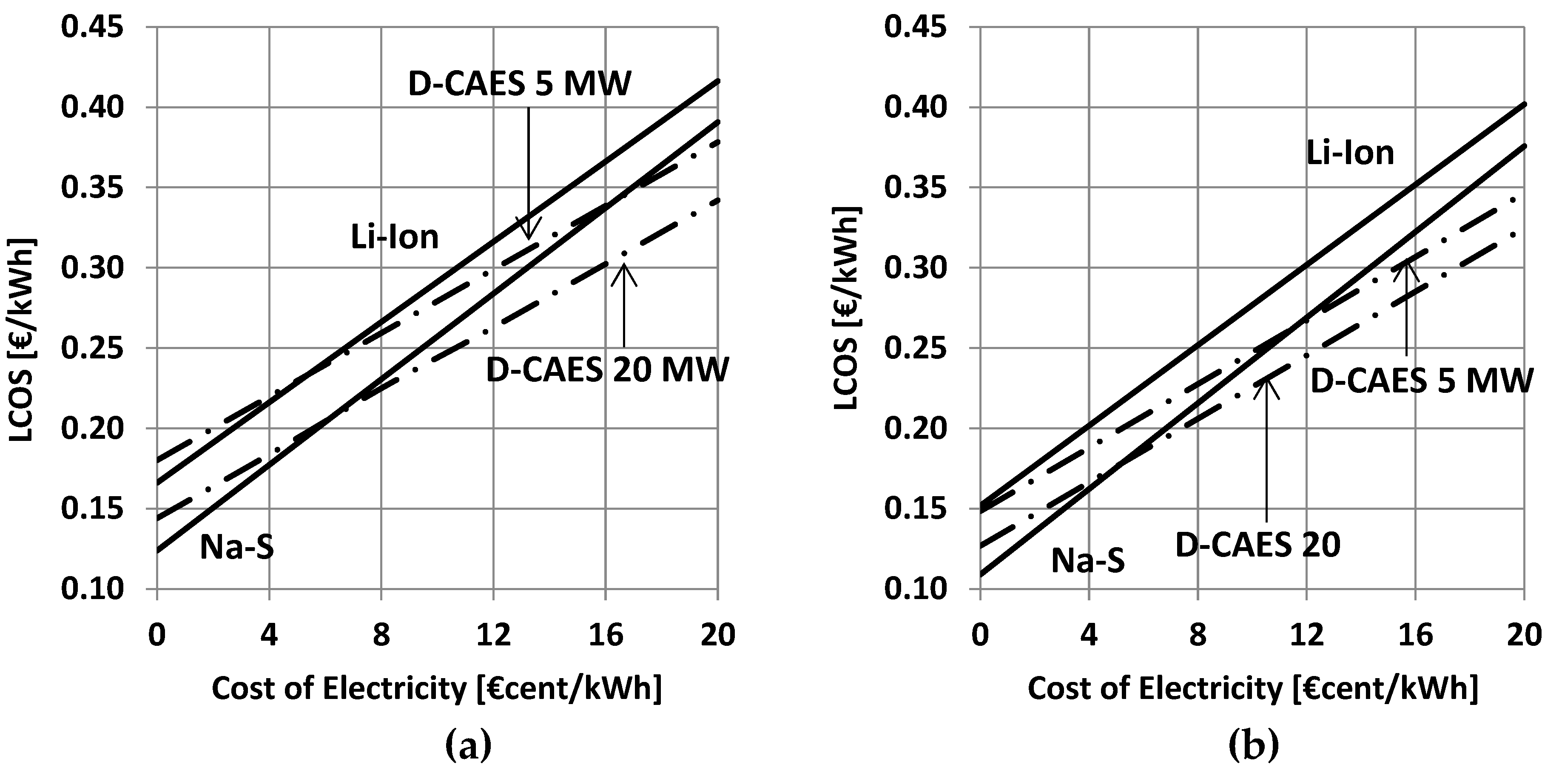
- The LCOS of D-CAES systems shows a lower sensitivity to the price of electricity in respect of BES based storage facilities.
- D-CAES based solutions can achieve a LCOS lower than that shown by Na-S batteries, provided that the size of the system and the price of electricity are large enough.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Appendix A.1. Charge Phase Model
- A perfect gas behavior is assumed for air. The isentropic exponent k (the ratio of specific heat at constant pressure to the specific heat at constant volume) is assumed equal to 1.4.
- The N compression stages constituting the compressor train operate at constant polytropic efficiency ηPS. Moreover, it is assumed that, at any time, the N compression stages work at the same pressure ratio βS(t):where β(t) is the overall pressure ratio at time t.
- Air temperature at the exit of each intercooler and at the exit of the aftercooler is assumed to remain constant.
- Bearing mechanical losses and losses in the reversible electric machine are taken into consideration by introducing the electric-mechanic efficiency ηEM.
- According to [34], the stored air temperature TST is considered constant during the charging, the storage period and the discharge phase.
Appendix A.2. Discharging Phase Model
References
- Xing, L.; Wang, J.; Dooner, M.; Clark, J. Overview of current development in electrical energy storage technologies and the application potential in power system operation. Appl. Energy 2015, 137, 511–536. [Google Scholar]
- May, G.J.; Davidson, A.; Mohanov, B. Lead batteries for utility energy storage: A review. J. Energy Storage 2018, 15, 145–157. [Google Scholar] [CrossRef]
- Rossi, A.; Stabile, M.; Puglisi, C.; Fabretti, D.; Merlo, M. Evaluation of the energy storage system Impact on the Italian ancillary market. Sustain. Energy Grid Netw. 2019, 18, 100178. [Google Scholar] [CrossRef]
- Guittet, M.; Capezzali, M.; Gaudard, L.; Romerio, F.; Vuille, F.; Avellan, F. Study of the drivers and asset management of pumped-storage power plants historical and geographical perspective. Energy 2016, 111, 560–579. [Google Scholar] [CrossRef]
- Blakers, A.; Stocks, M.; Lu, B.; Cheng, C. A review of pumped hydro energy storage. Prog. Energy 2021, 3, 022003. [Google Scholar] [CrossRef]
- TERNA. Report di Esercizio I Anno di Sperimentazione. Sperimentazione di Progetti Pilota di Accumulo Energetico a Batterie di Tipo Energy Intensiv. Available online: http://download.terna.it/terna/0000/0934/81.PDF (accessed on 27 May 2021).
- IRENA. Utility-Scale Batteries. Innovation Landscape Brief. Available online: https://www.irena.org/-/media/Files/IRENA/Agency/Publication/2019/Sep/IRENA_Utility-scale-batteries_2019.pdf (accessed on 10 June 2022).
- Goldman, S. SDG&E and AES Energy Storage Unveil World’s Largest Lithium Ion Battery-Based Energy Storage Installation. Available online: https://blog.fluenceenergy.com/sdge-and-aes-energy-storage-unveil-worlds-largest-battery-storage-installation (accessed on 10 June 2022).
- Brakels, R. Tesla Big Battery First Year: Lowers Electricity Prices, Makes Money, Is Not on Fire. Available online: https://www.solarquotes.com.au/blog/tesla-big-battery-first-year/ (accessed on 10 June 2022).
- Csereklyei, Z.; Kallies, A.; Diaz Valdivia, A. The status of and opportunities for utility-scale battery storage in Australia: A regulatory and market perspective. Util. Policy 2021, 73, 101313. [Google Scholar] [CrossRef]
- Zakeri, B.; Syri, S. Electrical energy storage systems: A comparative life cycle cost analysis. Renew. Sustain. Energy Rev. 2015, 42, 569–596. [Google Scholar] [CrossRef]
- Moustafa, M.G.; Sanad, M.M.S. Green fabrication of ZnAl2O4-coated LiFePO4 nanoparticles for enhanced electrochemical performance in Li-ion batteries. J. Alloys Compd. 2022, 903, 163910. [Google Scholar] [CrossRef]
- Sanad, M.M.S.; Azab, A.A.; Taha, T.A. Inducing lattice defects in calcium ferrite anode materials for improved electrochemical performance in lithium-ion batteries. Ceram. Int. 2022, 48, 12537–12548. [Google Scholar] [CrossRef]
- Mostafa, H.M.; Abdel Aleem, S.H.E.; Ali, S.G.; Ali, Z.M. Techno-economic assessment of energy storage systems using annualized life cycle cost storage (LCCOS) and levelized cost of energy (LCOE) metrics. J. Energy Storage 2020, 29, 101245. [Google Scholar] [CrossRef]
- Alotto, P.; Guarnieri, M.; Moro, F. Redox flow batteries for the storage of renewable energy: A review. Renew. Sustain. Energy Rev. 2014, 29, 325–335. [Google Scholar] [CrossRef]
- Colthorpe, A. First Phase of 800 MWh World Biggest Flow Battery Commissioned in China. Available online: https://www.energy-storage.news/first-phase-of-800mwh-world-biggest-flow-battery-commissioned-in-china/ (accessed on 21 August 2022).
- Rahman, M.M.; Oni, A.O.; Gemechu, E.; Kumar, A. Assessment of energy storage technologies: A review. Energy Convers. Manag. 2020, 223, 113295. [Google Scholar] [CrossRef]
- Darling, R.M. Techno-economic analyses of several redox flow batteries using levelized cost of energy storage. Curr. Opin. Chem. Eng. 2022, 37, 100855. [Google Scholar] [CrossRef]
- Rahman, M.M.; Oni, A.O.; Gemechu, E.; Kumar, A. The development of techno-economic models for the assessment of utility-scale electro-chemical battery storage systems. Appl. Energy 2021, 283, 116343. [Google Scholar] [CrossRef]
- Kim, Y.M.; Lee, J.H.; Kim, S.J.; Favrat, D. Potential and Evolution of Compressed Air Energy Storage: Energy and Exergy Analyses. Entropy 2012, 14, 1501–1521. [Google Scholar] [CrossRef]
- Budt, M.; Wolf, D.; Span, R.; Yan, J. A review on compressed air energy storage: Basic principles. Past milestones and recent developments. Appl. Energy 2016, 170, 250–268. [Google Scholar] [CrossRef]
- Chen, L.; Hu, P.; Sheng, C.; Xie, M. A Novel Compressed Air Energy Storage (CAES) Combined with Pre-Cooler and Using Low Grade Waste Heat as Heat Source. Energy 2017, 131, 259–266. [Google Scholar] [CrossRef]
- Li, Y.; Sciacovelli, A.; Peng, X.; Radcliffe, J.; Ding, Y. Integrating Compressed Air Energy Storage with a Diesel Engine for Electricity Generation in Isolated Areas. Appl. Energy 2016, 171, 26–36. [Google Scholar] [CrossRef]
- Salvini, C. Techno-Economic Analysis of CAES Systems Integrated into Gas-Steam Combined Plants. Energy Procedia 2016, 101, 870–877. [Google Scholar] [CrossRef]
- Salvini, C. Performance assessment of a CAES system integrated into a gas-steam combined plant. Energy Procedia 2017, 136, 264–269. [Google Scholar] [CrossRef]
- Crotogino, F.; Mohmeyer, K.U.; Scharf, R. Huntorf CAES: More than 20 Years of Successful Operation. In Proceedings of the Spring 2001 Meeting, Orlando, FL, USA, 15–18 April 2001. [Google Scholar]
- King, M.; Jain, A.; Bhakar, R.; Mathur, J.; Wang, J. Overview of Current Compressed Air Energy Storage Projects and Analysis of the Potential Underground Storage Capacity in India and the UK. Renew. Sustain. Energy Rev. 2021, 139, 110705. [Google Scholar] [CrossRef]
- Salvini, C.; Giovannelli, A. Techno-economic comparison of diabatic CAES with artificial air reservoir and battery energy storage systems. Energy Rep. 2022, 8, 601–607. [Google Scholar] [CrossRef]
- Salvini, C.; Giovannelli, A.; Sabatello, D. Analysis of diabatic compressed air energy storage systems with artificial reservoir using the levelized cost of storage method. Int. J. Energy Res. 2021, 45, 254–268. [Google Scholar] [CrossRef]
- Douglas, L.E. Industrial Chemical Process Design, 2nd ed.; McGraw-Hill: New York, NY, USA, 2014. [Google Scholar]
- Pedrick, J.; Marean, J.B. Compressed Air Energy Storage Engineering and Economic Study, Final Report, Nyserda Report 10-09, December 2009; NYSERDA: Albany, NY, USA, 2009. [Google Scholar]
- Sayer, J.; Pemberton, D.; Jewitt, J.; Pletka, R.; Fishbach, M.; Meyer, T.; Ward, M.; Bjorge, B.; Hargreaves, D.; Jordan, G. Mini-Compressed Air Energy Storage for Transmission and Congestion Relief and Wind Shaping Application, Final Report, Nyserda, Report 08-5, July 2008; NYSERDA: Albany, NY, USA, 2008. [Google Scholar]
- Liu, X.; Zhang, Z.; Xu, Y.; Chen, H.; Chen, Z.; Tan, C. Economic analysis of using above ground gas storage device for compressed air storage systems. J. Therm. Sci. 2014, 6, 535–543. [Google Scholar] [CrossRef]
- Salvini, C. Performance Analysis of Small Size Compressed Air Energy Storage Systems for Power Augmentation: Air Injection and Air Injection/Expander Schemes. Heat Transf. Eng. 2018, 39, 304–315. [Google Scholar] [CrossRef]
- Lincoln Electric, Welding Pressure Pipelines & Piping Systems—Procedures and Techniques. Available online: http://www.lincolnelectric.com/assets/global/Products/Consumable_PipelinerConsumables-Pipeliner-PipelinerLH-D90/c2420.pdf (accessed on 21 August 2022).
- Cerri, G.; Mazzoni, S.; Salvini, C. Steam cycle simulator for CHP plants”. In Proceedings of the ASME Turbo Expo, San Antonio, TX, USA, 3–7 June 2013. [Google Scholar] [CrossRef]
- Cerri, G.; Gazzino, M.; Botta, F.; Salvini, C. Production planning with hot section life prediction for optimum gas turbine management. Int. J. Gas Turbine Propuls. Power Syst. 2008, 2, 9–16. [Google Scholar] [CrossRef]
- Salvini, C. CAES systems integrated into gas-steam combined plant: Design point performance assessment. Energies 2018, 11, 415. [Google Scholar] [CrossRef]
- Foster-Pegg, R.W. Capital Cost of Gas-Turbine Heat Recovery Boilers. Chem. Eng. 1989, 14, 73–78. [Google Scholar]
- Salvini, C.; Giovannelli, A.; Varano, M. Economic Analysis of Small Size Gas Turbine Based CHP Plants in the Present Italian Context. Int. J. Heat Technol. 2016, 34, S443–S450. [Google Scholar] [CrossRef]
- PacifiCorp. Battery Energy Storage for the 2017 IRP. 2016. Available online: http://www.pacificorp.com/content/dam/pacificorp/doc/Energy_Sources/Integrated_Resource_Plan/2017_IRP/10018304_R-01-D_PacifiCorp_Battery_Energy_Storage_Study.pdf (accessed on 21 March 2019).
- Rahmann, C.; Mac-Clure, B.; Vittal, V.; Valencia, F. Break-Even Points of Battery Energy Storage Systems for Peak Shaving applications. Energies 2017, 10, 833. [Google Scholar] [CrossRef]
- Julch, V. Comparison of electricity storage options using levelized cost of storage (LCOS) method. Appl. Energy 2016, 183, 1594–1606. [Google Scholar] [CrossRef]
- Tesla Battery Cost Revealed Two Years after Blackout. Available online: https://www.abc.net.au/news/2018-09-27/tesla-battery-cost-revealed-two-years-after-blackout/10310680 (accessed on 30 June 2022).
- Cole, W.; Frazier, W. Cost Projection for Utility-Scale Battery Storage: 2020 Update. Available online: https://www.nrel.gov/docs/fy20osti/75385.pdf (accessed on 28 May 2021).
- Perry, H.; Green, D.W. Perry’s Chemical Engineers’ Handbook, 7th ed.; McGraw Hill: New York, NY, USA, 1998. [Google Scholar]
- Rivkin, S.L. Thermodynamic Properties of Gases; Springer: Berlin/Heidelberg, Germany, 1988. [Google Scholar]



| Ambient Temperature TAMB [°C] | 20 |
| Ambient Pressure pAMB [kPa] | 100 |
| Intercoolers/Aftercooler Outlet Temp. [°C] | 35 |
| Compression Polytropic Efficiency [%] | 85 |
| Mechanical-Electrical Efficiency [%] | 97 |
| Stored Air Temperature [°C] | 30 |
| Storage pressure [bar] | 100 |
| Natural Gas Lower Heating Value [MJ/kg] | 50 |
| Combustion Chamber Efficiency [%] | 99 |
| Air Pre-Heater Effectiveness [%] | 90 |
| Gas Expander inlet pressure [bar] | 20 |
| Gas Expander inlet temperature [°C] | 700 |
| Air Expander Polytropic Efficiency [%] | 85 |
| BES Type | Na-S | Li-Ion |
|---|---|---|
| Technical data | ||
| Efficiency [%] | 75 | 80 |
| Deep of Discharge DOD [%] | 80 | 80 |
| Life Duration [years] | 15 | 10 |
| Investment cost data | ||
| CPOWER [€/kW] | 350 | 250 |
| CSTORAGE [€/kWh] | 240 | 250 |
| Maintenance cost data | ||
| CM [€/kW/year] | 26 | 25 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salvini, C.; Giovannelli, A. Techno-Economic Comparison of Utility-Scale Compressed Air and Electro-Chemical Storage Systems. Energies 2022, 15, 6644. https://doi.org/10.3390/en15186644
Salvini C, Giovannelli A. Techno-Economic Comparison of Utility-Scale Compressed Air and Electro-Chemical Storage Systems. Energies. 2022; 15(18):6644. https://doi.org/10.3390/en15186644
Chicago/Turabian StyleSalvini, Coriolano, and Ambra Giovannelli. 2022. "Techno-Economic Comparison of Utility-Scale Compressed Air and Electro-Chemical Storage Systems" Energies 15, no. 18: 6644. https://doi.org/10.3390/en15186644
APA StyleSalvini, C., & Giovannelli, A. (2022). Techno-Economic Comparison of Utility-Scale Compressed Air and Electro-Chemical Storage Systems. Energies, 15(18), 6644. https://doi.org/10.3390/en15186644






