Analysis of Energy Dissipation on the Sealing Surface of Premium Connection Based on a Microslip Shear Layer Model
Abstract
:1. Introduction
2. Materials and Methods
2.1. Establishment of the Shear Layer Model
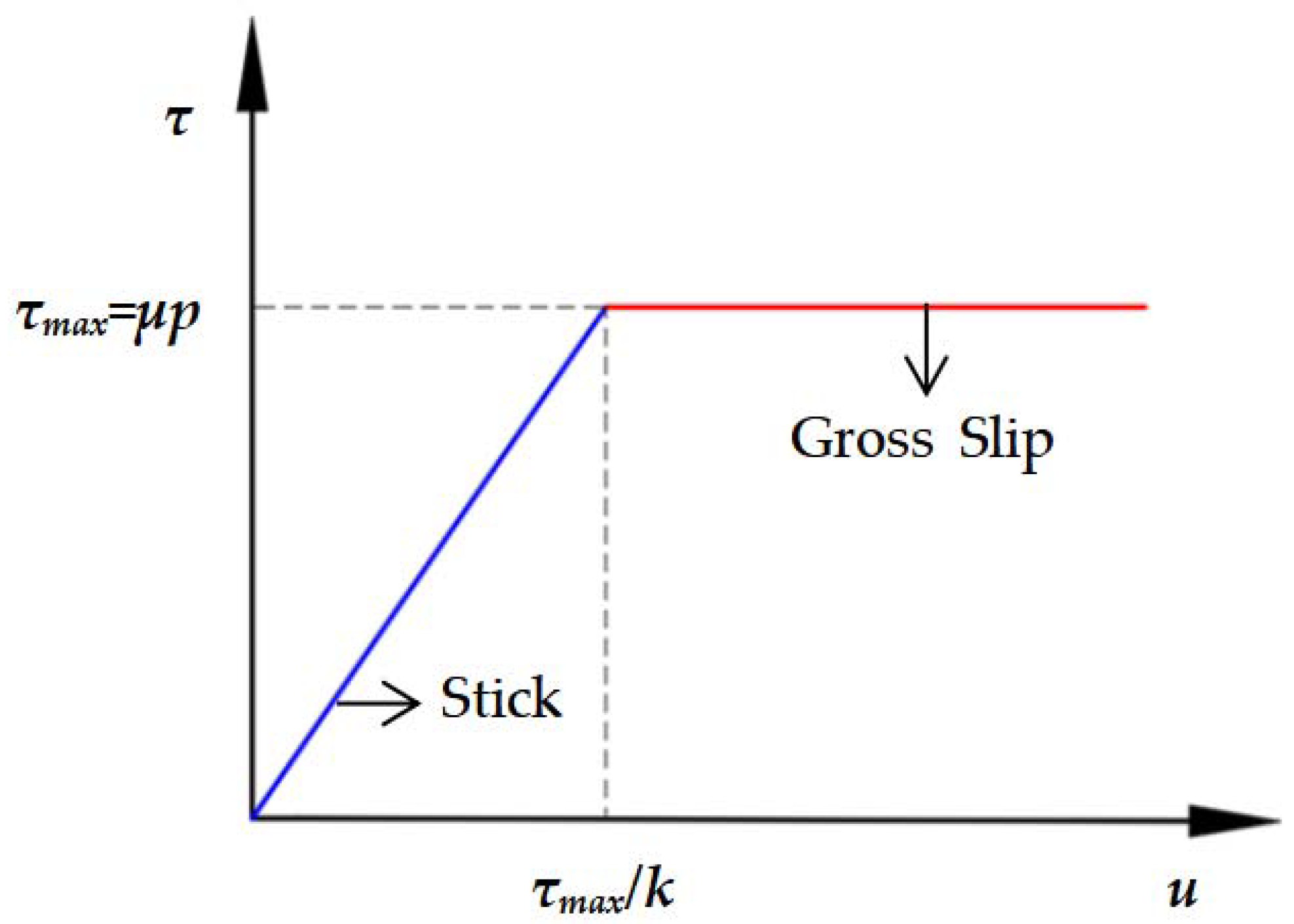
2.2. Stick–Microslip Critical Relationship
2.3. Force–Displacement Hysteresis Skeleton Curve
2.4. Force–Displacement Hysteresis Curve and Energy Dissipation Equation
2.5. Contact Pressure and Contact Length on the Sealing Surface
3. Results
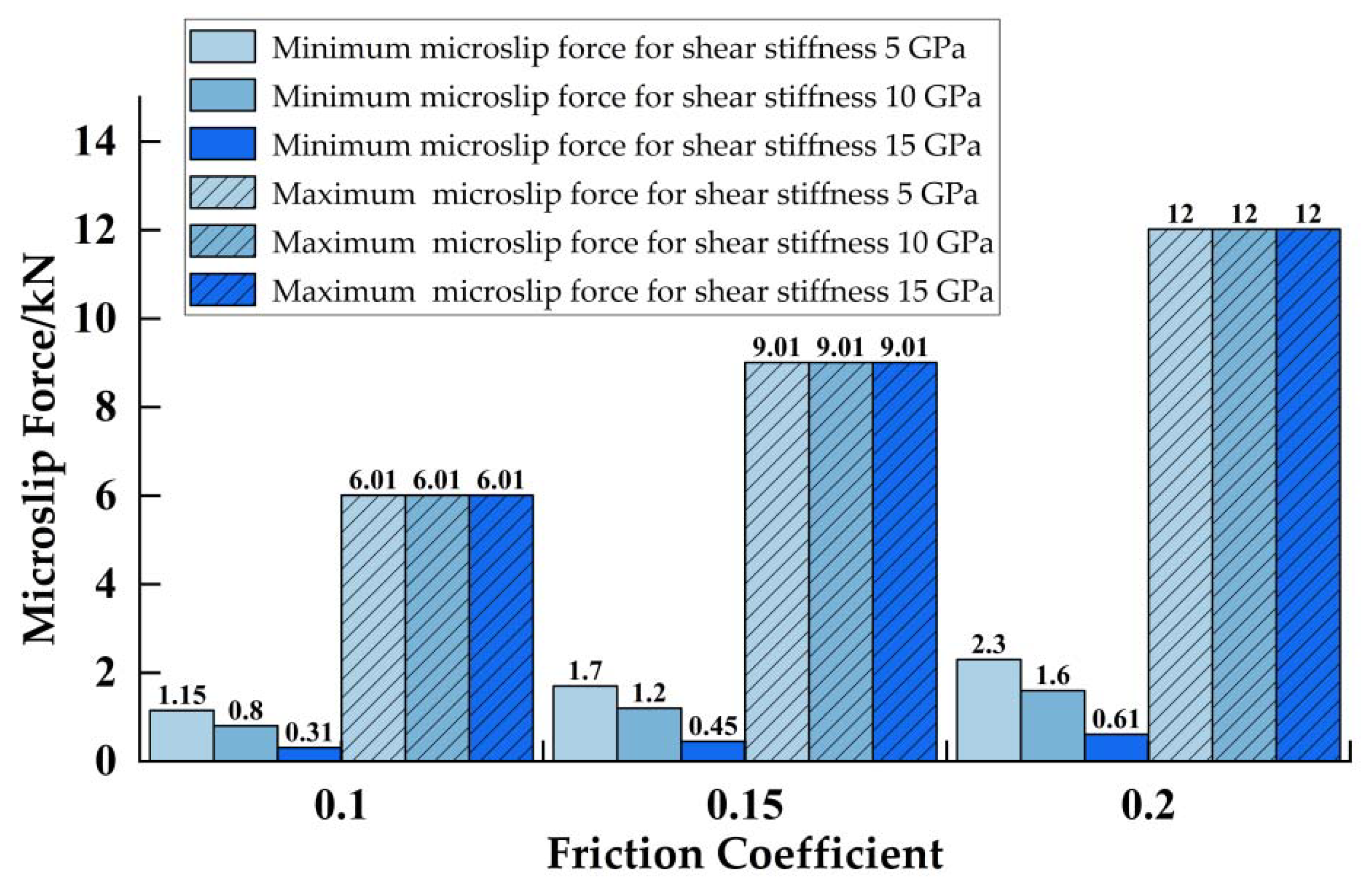
3.1. Stick–Slip Critical Relationship Analysis
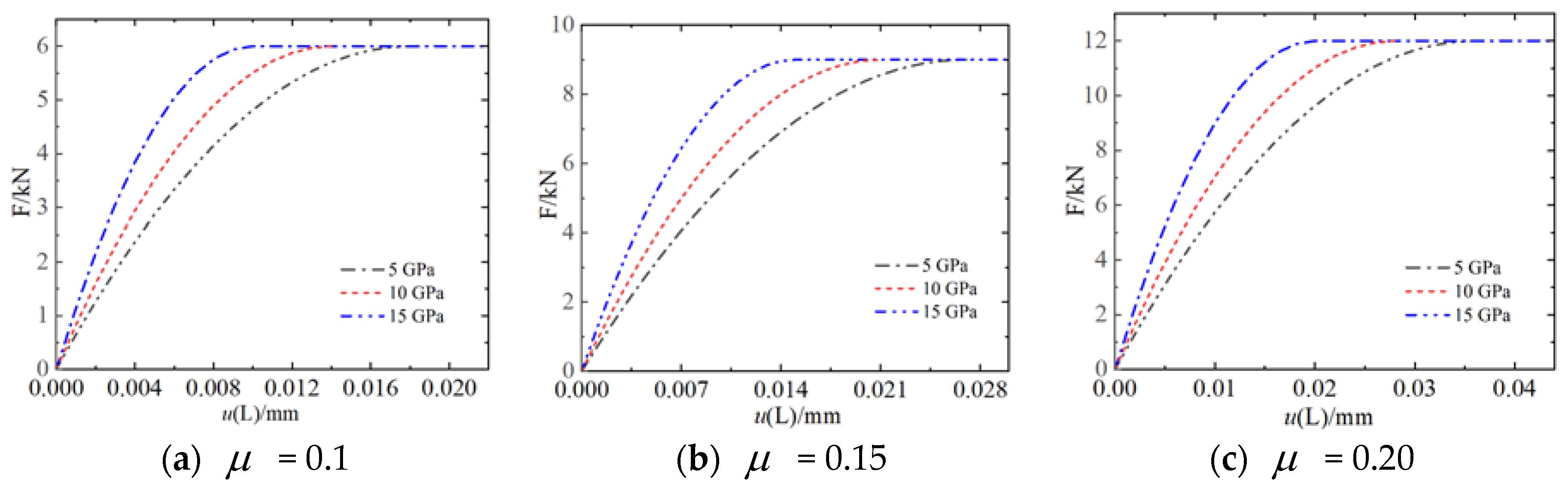
3.2. Force–Displacement Hysteresis Skeleton Curve Analysis
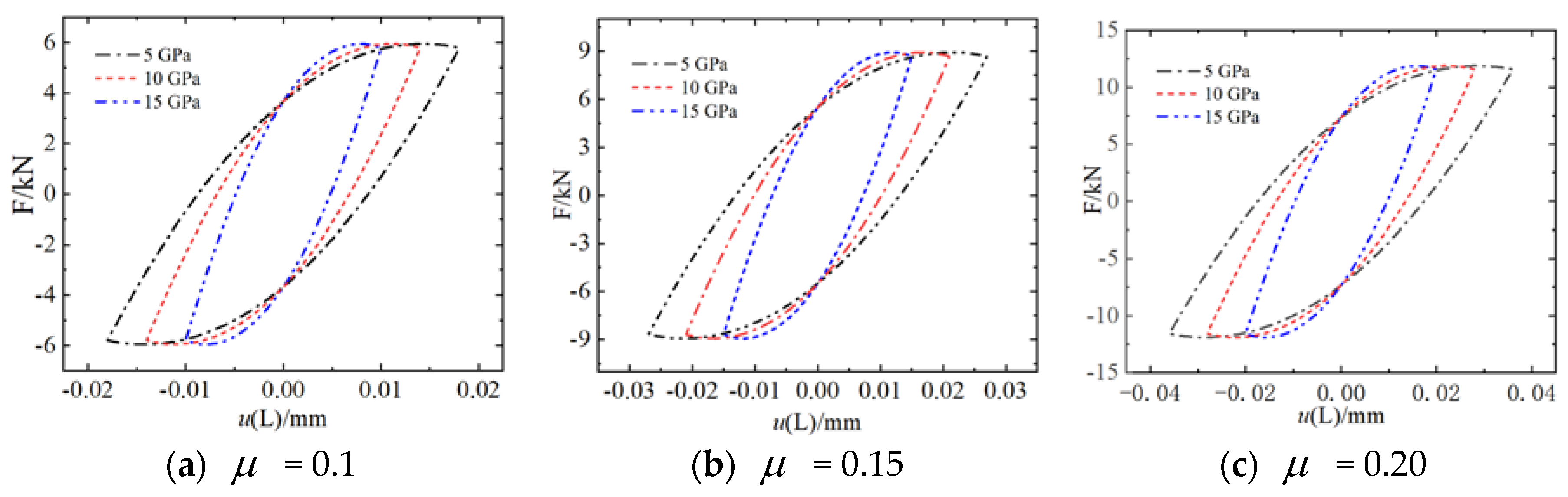
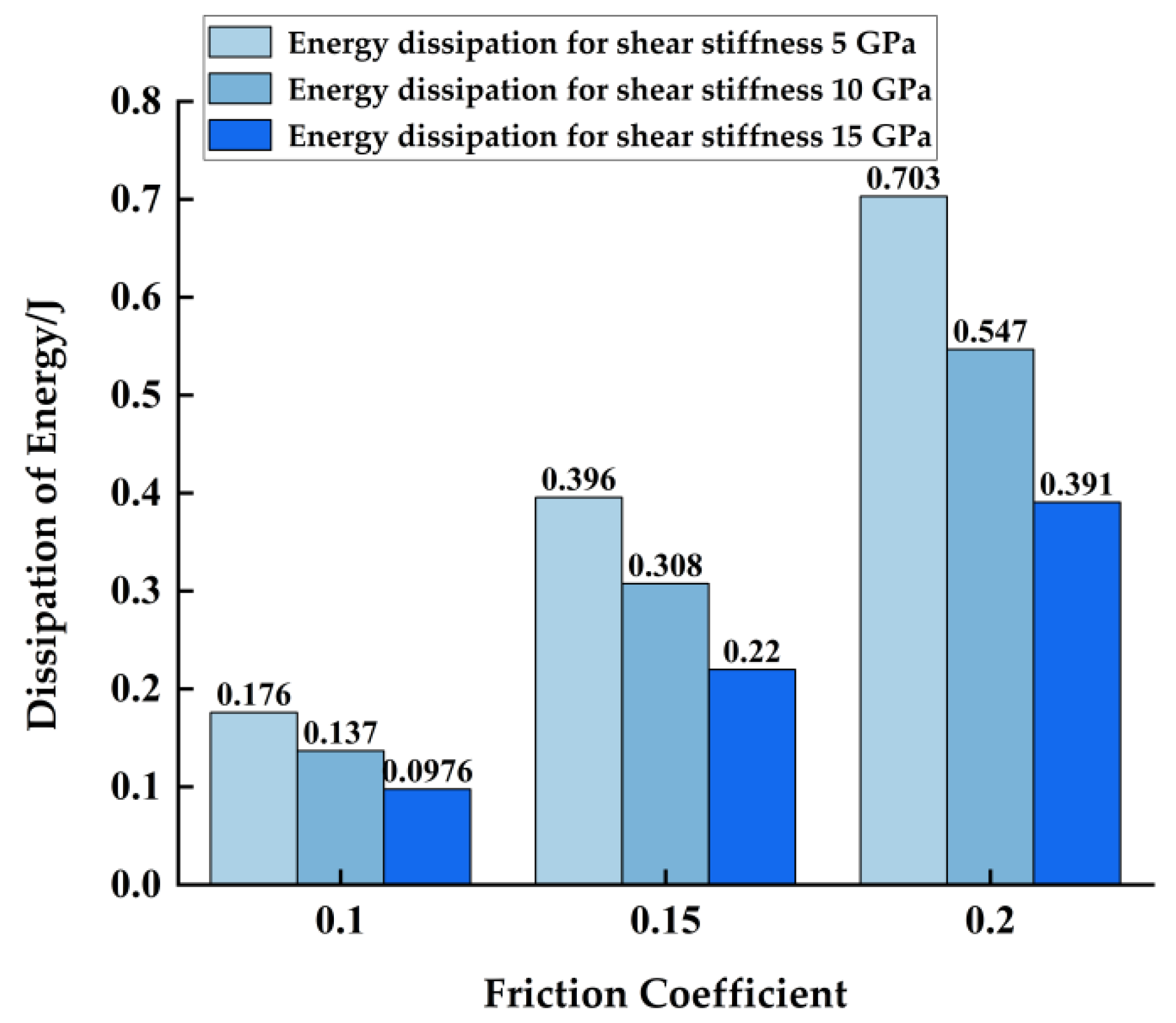
3.3. Force–Displacement Hysteresis Curve and Energy Dissipation Analysis
4. Discussion
5. Conclusions
- (1)
- When the tangential force is 0.31–12 kN, the sealing surface of the premium connection will produce energy dissipation caused by the microslip, and when the tangential force is more than 12 kN, the sealing surface will produce energy dissipation caused by the sliding friction.
- (2)
- The friction coefficient has an effect on both the minimum and maximum microslip tangential forces, while the shear layer stiffness only has an effect on the minimum microslip tangential forces. The greater the shear layer stiffness is, the smaller the minimum microslip tangential force causing the initial microslip is, the smaller the relative displacement of the contact surface is, and the easier it is to cause energy dissipation.
- (3)
- The influence of the friction coefficient on energy dissipation is two times greater than the shear layer stiffness by standardized regression coefficient analysis. The friction coefficient is positively correlated with energy dissipation, and the shear layer stiffness is negatively correlated with energy dissipation.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| Notation | |
| E | Young’s modulus, GPa |
| A | constant cross-section area of the elastic bar, mm2 |
| τ | shear friction force per unit length, N |
| u | tangential displacement, mm |
| k | stiffness per unit length of the shear layer, GPa |
| μ | friction coefficient |
| p | normal pressure on the sealing surface, MPa |
| P0 | the maximum value of a contact force along the length, N/mm |
| kp | slope of decreasing pressure |
| Fa | thread and shoulder torque binding force, kN |
| F | shear force, kN |
| ΔED | energy dissipation in per cycle, J |
| ln | stick length, mm |
| ust | sticking displacement, mm |
| usl | microslip displacement, mm |
| y | dependent variable |
| mean of the dependent variable | |
| xi | independent variables of different dimensions |
| average of the independent variables | |
| standard deviation of the independent variable | |
| standard deviation of the dependent variable | |
| regression coefficient |
References
- ISO 13679; Petroleum and Natural Gas Industries -Procedures for Testing Casing and Tubing Connection. International Organization for Standardization: Geneva, Switzerland, 2002.
- Chen, W.; Deng, X. Structural damping caused by micro-slip along frictional interfaces. Int. J. Mech. Sci. 2005, 47, 1191–1211. [Google Scholar] [CrossRef]
- Kogut, L.; Etsion, I. Elastic-plastic contact analysis of a sphere and a rigid flat. J. Appl. Mech. 2002, 69, 657–662. [Google Scholar] [CrossRef] [Green Version]
- Jackson, R.L.; Green, I. A finite element study of elasto-plastic hemispherical contact against a rigid flat. J. Trib. 2005, 127, 343–354. [Google Scholar] [CrossRef] [Green Version]
- Lin, L.P.; Lin, J.F. An elastoplastic microasperity contact model for metallic materials. J. Trib. 2005, 127, 666–672. [Google Scholar]
- Chung, J.C.; Lin, J.F. Variation in fractal properties and non-Gaussian distributions of microcontact between elastic-plastic rough surfaces with mean surface separation. J. Appl. Mech. 2006, 73, 143–152. [Google Scholar] [CrossRef]
- Xueliang, Z.; Nanshan, W.; Suhua, W. Elastoplastic fractal model for tangential contact damping energy dissipation of machine joint interfaces. J. Mech. Eng. 2013, 49, 43–49. [Google Scholar]
- Huang, W.; Gao, D.; Liu, Y. Inter-helical and intra-helical buckling analyses of tubular strings with connectors in horizontal wellbores. J. Pet. Sci. Eng. 2017, 152, 182–192. [Google Scholar] [CrossRef] [Green Version]
- Segalman, D.J. A Four-Parameter Iwan Model for Lap-Type Joints. J. Appl. Mech 2005, 72, 752–760. [Google Scholar] [CrossRef] [Green Version]
- Fujimoto, T.; Kagami, J.; Kawaguchi, T.; Hatazawa, T. Micro-displacement characteristics under tangential force. Wear 2000, 241, 136–142. [Google Scholar] [CrossRef]
- Wang, D.; Zhang, Z. A four-parameter model for nonlinear stiffness of a bolted joint with non-Gaussian surfaces. Acta Mech. 2020, 231, 1963–1976. [Google Scholar] [CrossRef]
- Oldfield, M.; Ojyang, H.; Mottershead, J. Experimental study and analytical modelling of a bolted joint subjected to harmonic excitation. Shock. Vib. Dig. 2006, 38, 246–247. [Google Scholar]
- Mahmoudi, M.; Kosari, M.; Lorestani, M.; Jalili Sadr Abad, M. Effect of contact surface type on the slip resistance in bolted connections. J. Constr. Steel Res. 2020, 166, 105943. [Google Scholar] [CrossRef]
- Xu, W.; Liu, Z.; Cheng, Q.; Chen, H.; Zhao, Y.; Li, Y. A mathematical model of a bolted connection based on Florida contact theory to study the influence of the friction coefficient on structural performance. Adv. Mech. Eng. 2019, 11, 1687814019851398. [Google Scholar] [CrossRef]
- Iwan, W.D. On a Class of Models for the Yielding Behavior of Continuous and Composite Systems. J. Appl. Mech. 1967, 34, 612–617. [Google Scholar] [CrossRef]
- Iwan, W.D. A Distributed-Element Model for Hysteresis and Its Steady-State Dynamic Response. J. Appl. Mech. 1966, 33, 893. [Google Scholar] [CrossRef]
- Segalman, D.J. An Initial Overview of Iwan Modeling for Mechanical Joints; Sandia National Lab. (SNL-NM): Albuquerque, NM, USA, 2001. [Google Scholar]
- Yang, B.D.; Chu, M.L.; Menq, C.H. Stick–slip–separation analysis and non-linear stiffness and damping characterization of friction contacts having variable normal load. J. Sound Vib. 1998, 210, 461–481. [Google Scholar] [CrossRef]
- Wang, Y.; Qian, C.; Kong, L.; Zhou, Q.; Gong, J. Design optimization for the thin-walled joint thread of a coring tool used for deep boreholes. Appl. Sci. 2020, 10, 2669. [Google Scholar] [CrossRef] [Green Version]
- Li, D.; Li, Y.; Chen, J.; Masoumi, H. An analytical model for axial performance of rock bolts under constant confining pressure based on continuously yielding criterion. Tunn. Undergr. Space Technol. 2021, 113, 103955. [Google Scholar] [CrossRef]
- Ju, B.-S.; Jeon, B.-G.; Kim, S.-W.; Son, H.-Y. Validation of Seismic Performance of Stainless Press-to-Connect Piping System under Cyclic Loadings. Appl. Sci. 2022, 12, 3896. [Google Scholar] [CrossRef]
- Qin, Z.; Cui, D.; Yan, S.; Chu, F. Hysteresis modeling of clamp band joint with macro-slip. Mech. Syst. Signal Process. 2016, 66–67, 89–110. [Google Scholar] [CrossRef]
- Oldfield, M.; Ouyang, H.; Mottershead, J.E. Simplified models of bolted joints under harmonic loading. Comput. Struct. 2005, 84, 25–33. [Google Scholar] [CrossRef]
- Majumdar, A.; Bhushan, B. Fractal Model of Elastic-Plastic Contact Between Rough Surfaces. J. Tribol. 1991, 113, 1–11. [Google Scholar] [CrossRef]
- Charalampakis, A.E.; Koumousis, V.K. A Bouc–Wen model compatible with plasticity postulates. J. Sound Vib. 2009, 322, 954–968. [Google Scholar] [CrossRef]
- Zaman, M.A.; Sikder, U. Bouc–Wen hysteresis model identification using Modified Firefly Algorithm. J. Magn. Magn. Mater. 2015, 395, 229–233. [Google Scholar] [CrossRef]
- Pereira Miguel, L.; Oliveira Teloli, R.d.; Silva, S.d. Harmonic Balance of Bouc-Wen Model to Identify Hysteresis Effects in Bolted Joints. In Vibration Engineering and Technology of Machinery; Springer: Cham, Switzerland, 2021; pp. 65–79. [Google Scholar]
- Shetty, D.; Allen, M.S.; Schoneman, J.D. Application of the Bouc-Wen Model to Bolted Joint Dynamics. In Nonlinear Structures & Systems; Springer: Cham, Switzerland, 2021; Volume 1, pp. 35–38. [Google Scholar]
- Peng, Z.; Zhou, C.G. Modeling of Nonlinear Isolation System Based on the Bouc–Wen Differential Model. Strength Mater. 2017, 49, 206–211. [Google Scholar] [CrossRef]
- Menq, C.H.; Bielak, J.; Griffin, J.H. The influence of microslip on vibratory response, part I: A new microslip model. J. Sound Vib. 1986, 107, 279–293. [Google Scholar] [CrossRef]
- Sun, D. Study on Energy Dissipation Mechanism and Prediction of Bolted Joints Structure. Ph.D. Thesis, Beijing Institute of Technology, Beijing, China, 2016. [Google Scholar]
- Segalman, D.J. Modelling joint friction in structural dynamics. Struct. Control. Health Monit. 2006, 13, 430–453. [Google Scholar] [CrossRef]
- Sun, D.; Liao, R. Investigation into the Effect of Interface Characteristics on the Energy Dissipation of the Bolted Joints. J. Comput. Theor. Nanosci. 2015, 12, 2821–2829. [Google Scholar] [CrossRef]
- Song, Y.; McFarland, D.M.; Bergman, L.A.; Vakakis, A.F. Effect of pressure distribution on energy dissipation in a mechanical lap joint. AIAA J. 2005, 43, 420–425. [Google Scholar] [CrossRef]

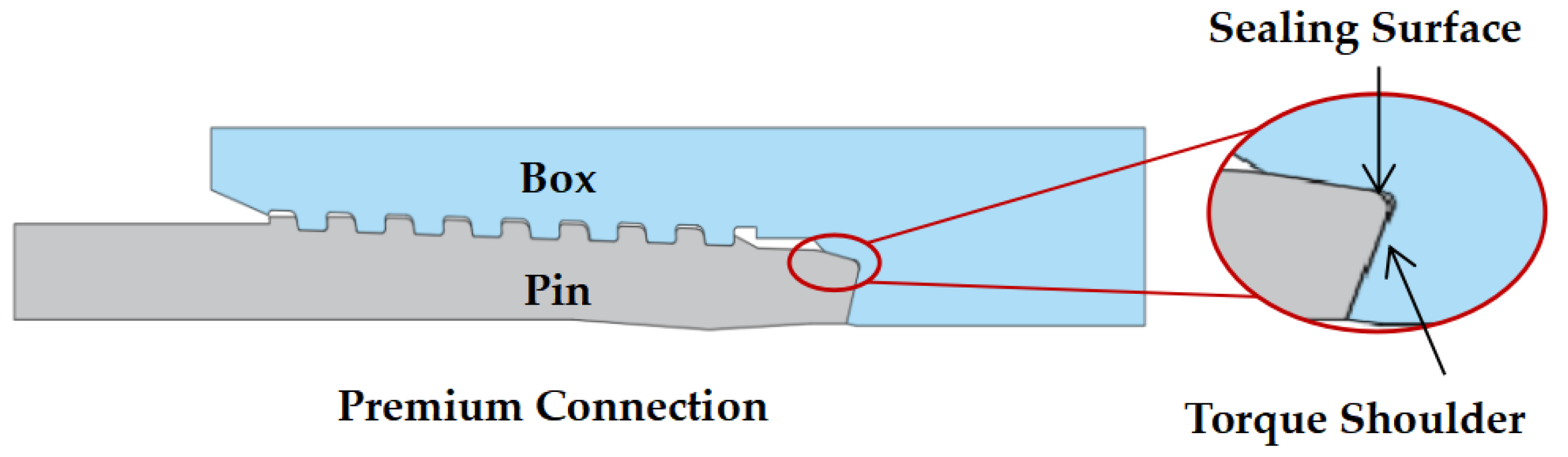



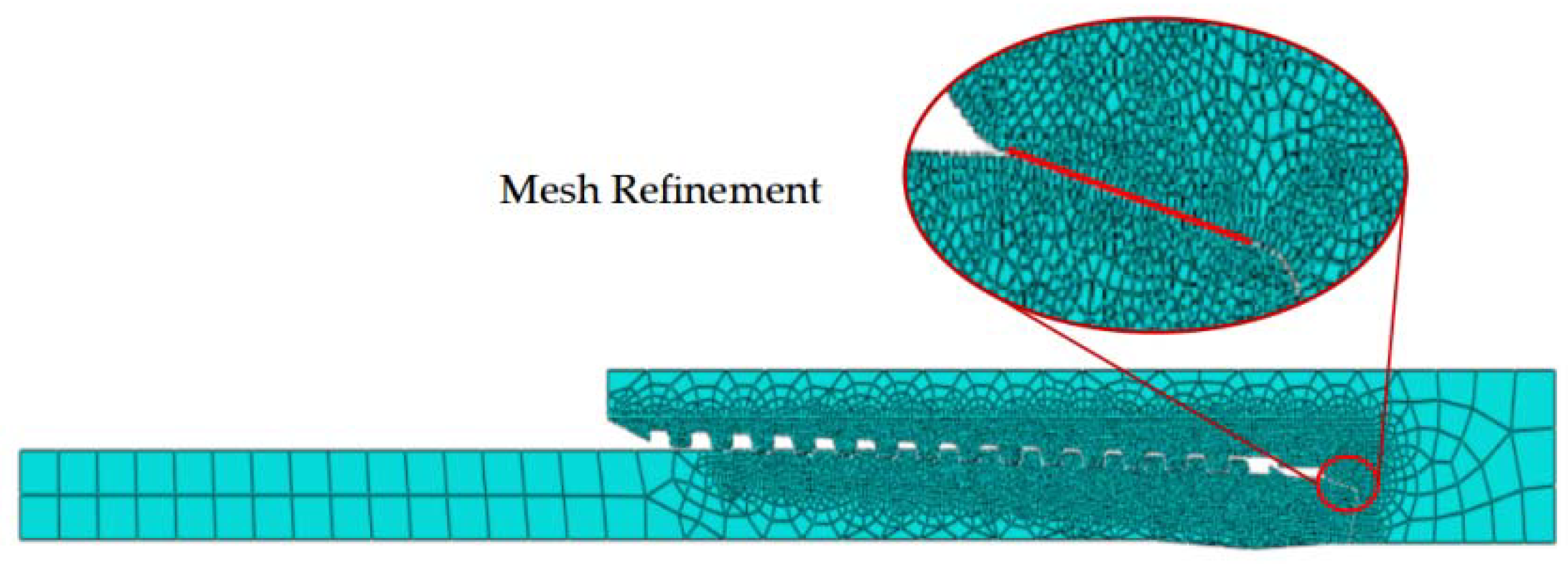
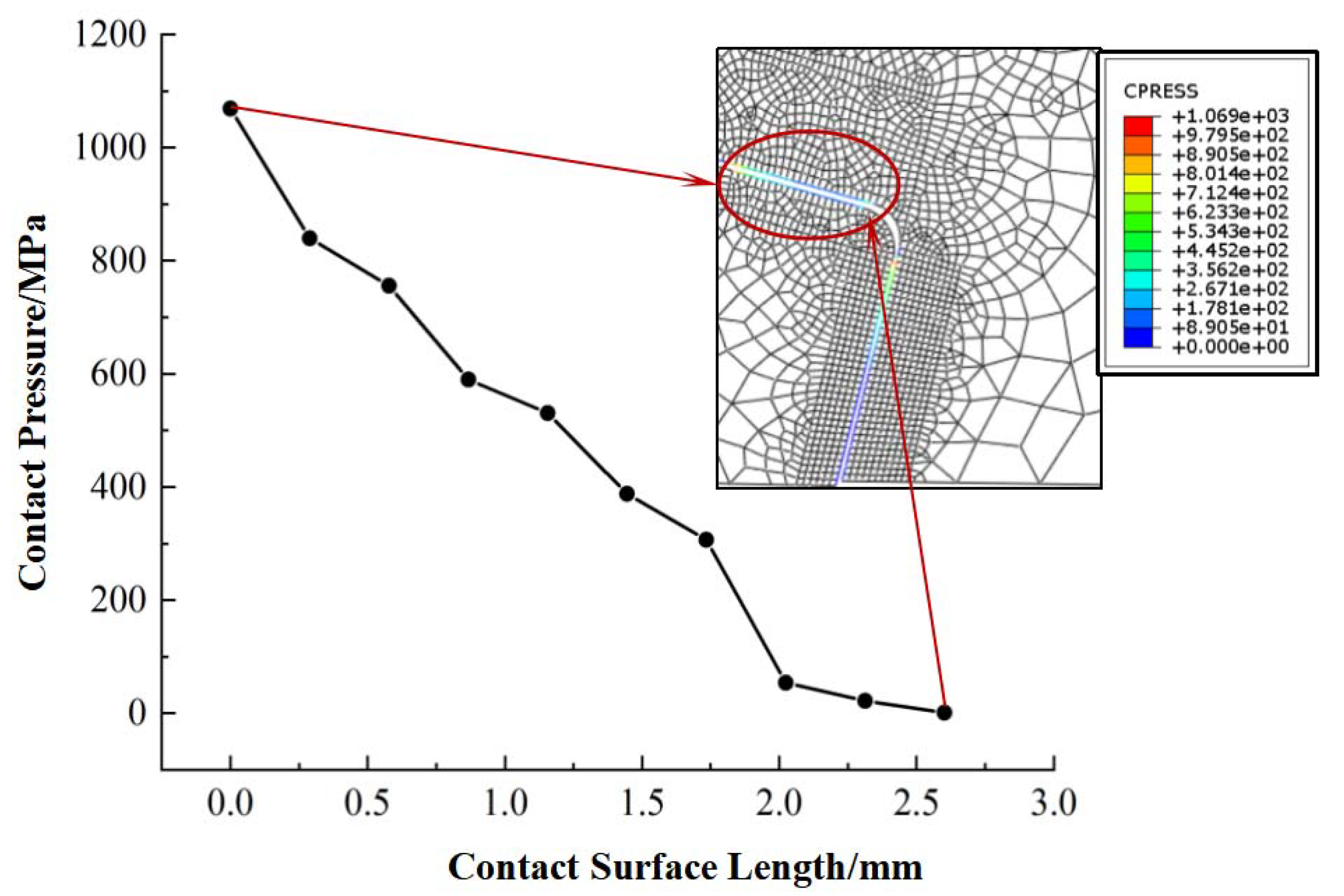


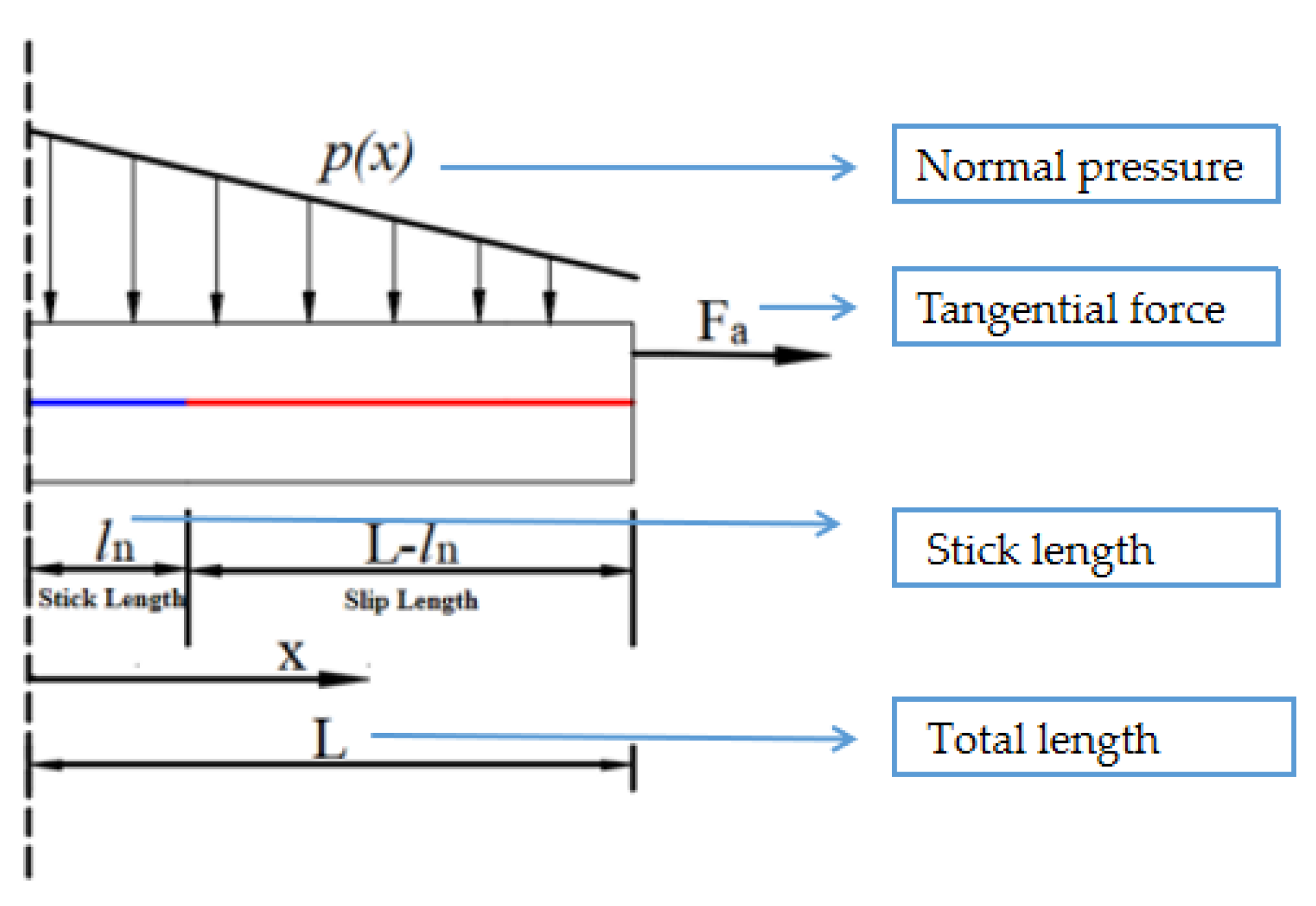
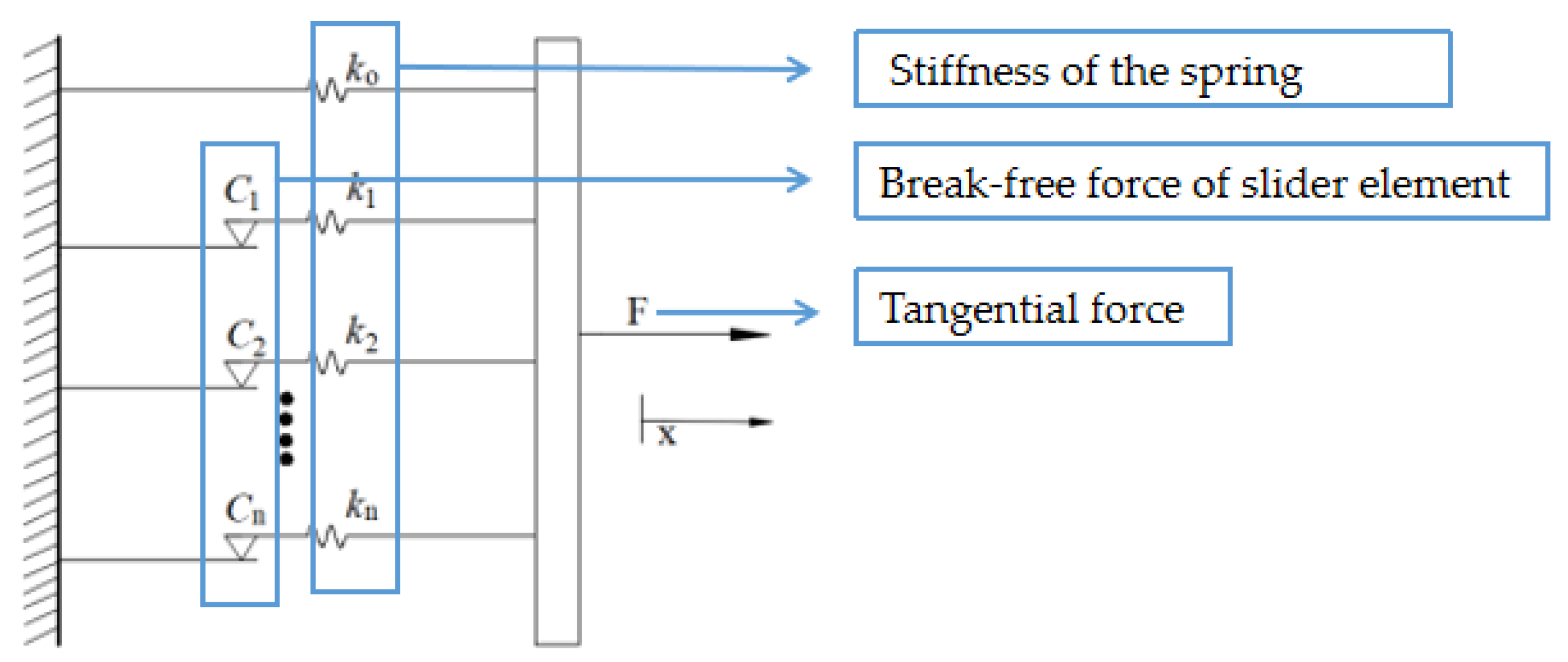
| Sealing Taper | Seal Interference | Shoulder Angle | Thread Taper | Pitch |
|---|---|---|---|---|
| 1/2 | 0.176 MM | −15° | 1/2 | 4.234 mm |
| The Coefficient of Friction | Shear Layer Stiffness |
|---|---|
| 0.10 | 5 GPa |
| 0.15 | 10 GPa |
| 0.20 | 15 GPa |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yu, Y.; Qu, Z.; Dou, Y.; Cao, Y. Analysis of Energy Dissipation on the Sealing Surface of Premium Connection Based on a Microslip Shear Layer Model. Energies 2022, 15, 8400. https://doi.org/10.3390/en15228400
Yu Y, Qu Z, Dou Y, Cao Y. Analysis of Energy Dissipation on the Sealing Surface of Premium Connection Based on a Microslip Shear Layer Model. Energies. 2022; 15(22):8400. https://doi.org/10.3390/en15228400
Chicago/Turabian StyleYu, Yang, Zhan Qu, Yihua Dou, and Yinping Cao. 2022. "Analysis of Energy Dissipation on the Sealing Surface of Premium Connection Based on a Microslip Shear Layer Model" Energies 15, no. 22: 8400. https://doi.org/10.3390/en15228400
APA StyleYu, Y., Qu, Z., Dou, Y., & Cao, Y. (2022). Analysis of Energy Dissipation on the Sealing Surface of Premium Connection Based on a Microslip Shear Layer Model. Energies, 15(22), 8400. https://doi.org/10.3390/en15228400




