Consequence of Blowby Flow and Idling Time on Oil Consumption and Particulate Emissions in Gasoline Engine
Abstract
:1. Introduction
2. Materials and Method
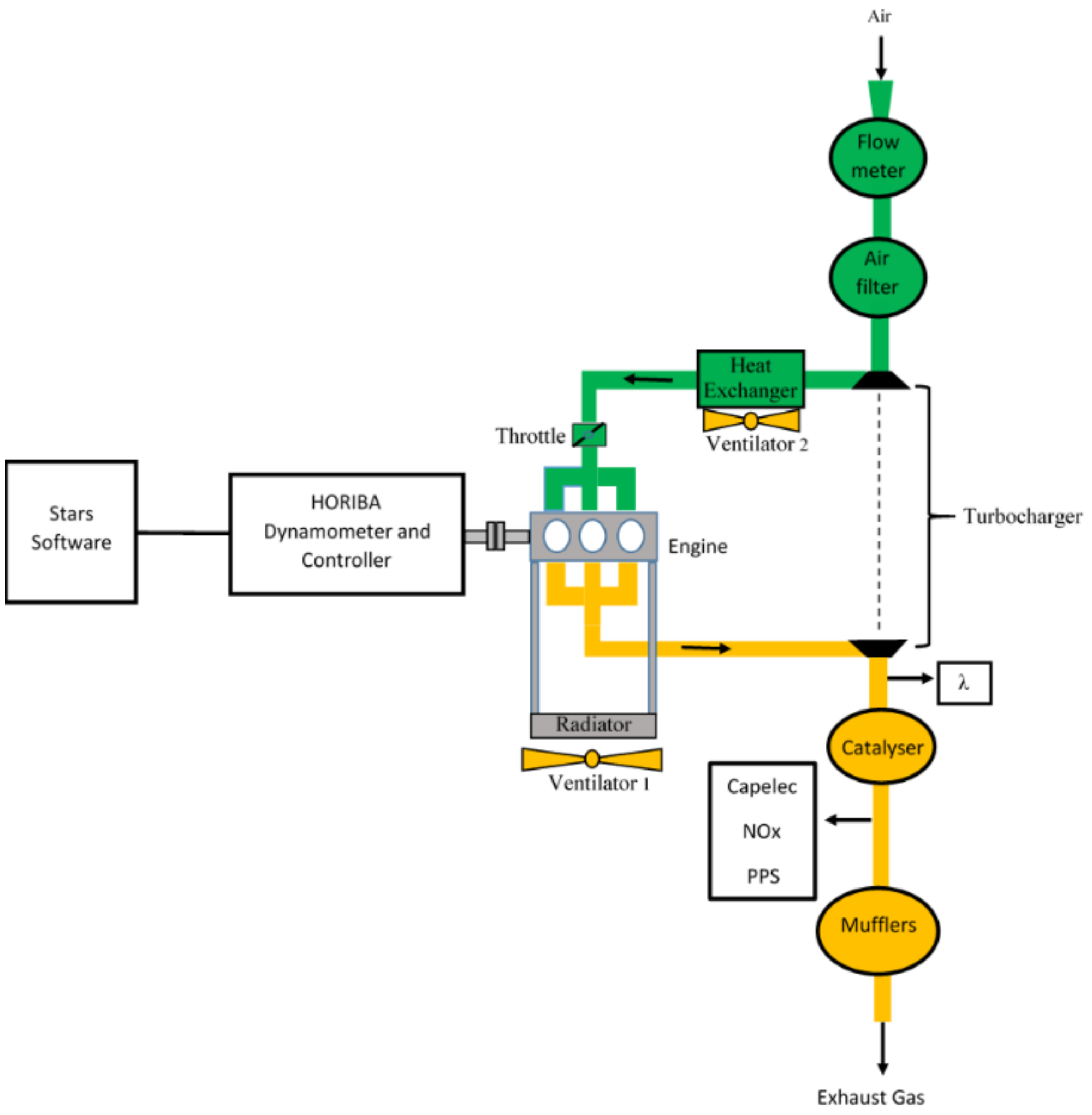
2.1 Description of Engine Bench
3. Blowby Simulation in Steady State
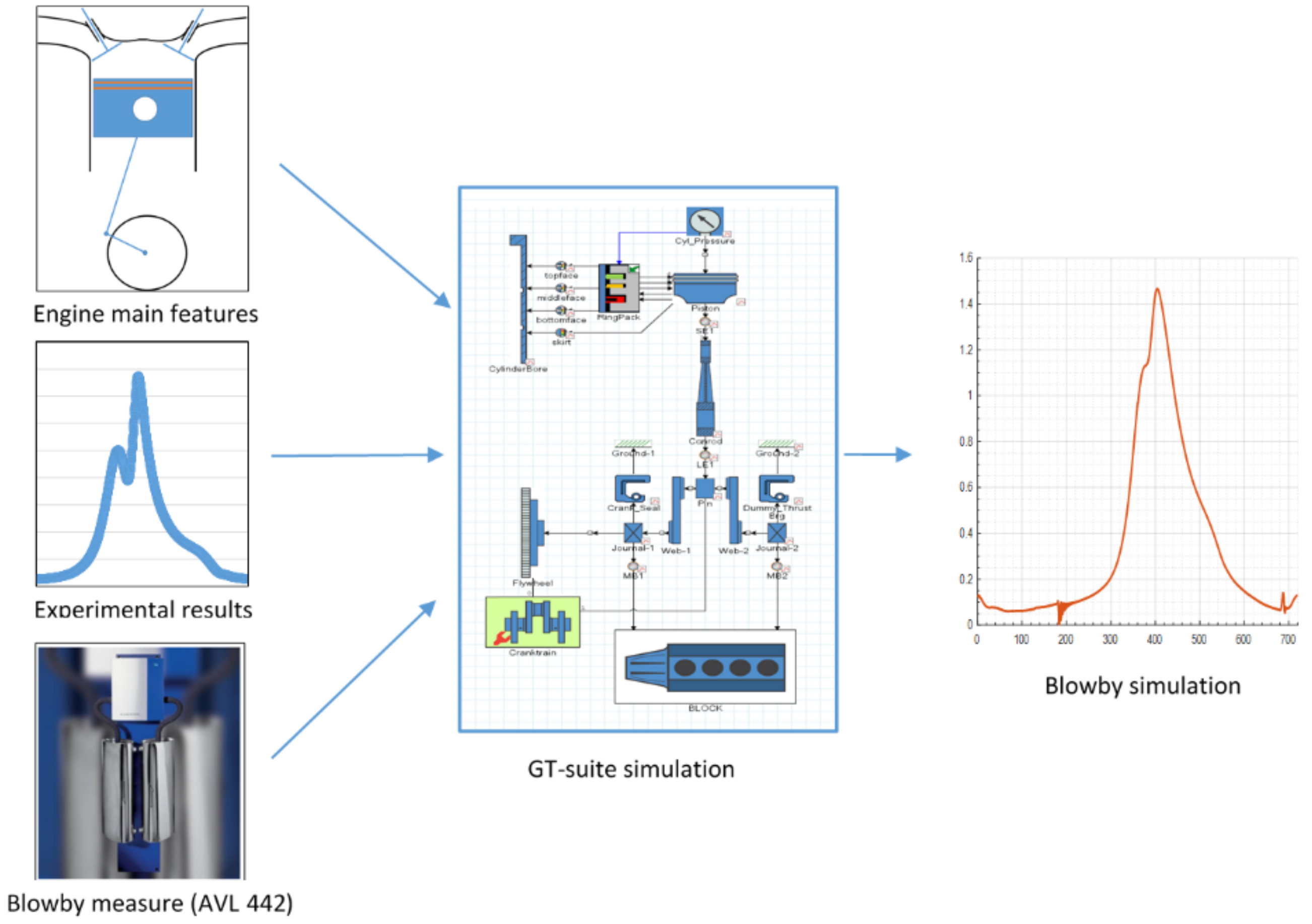
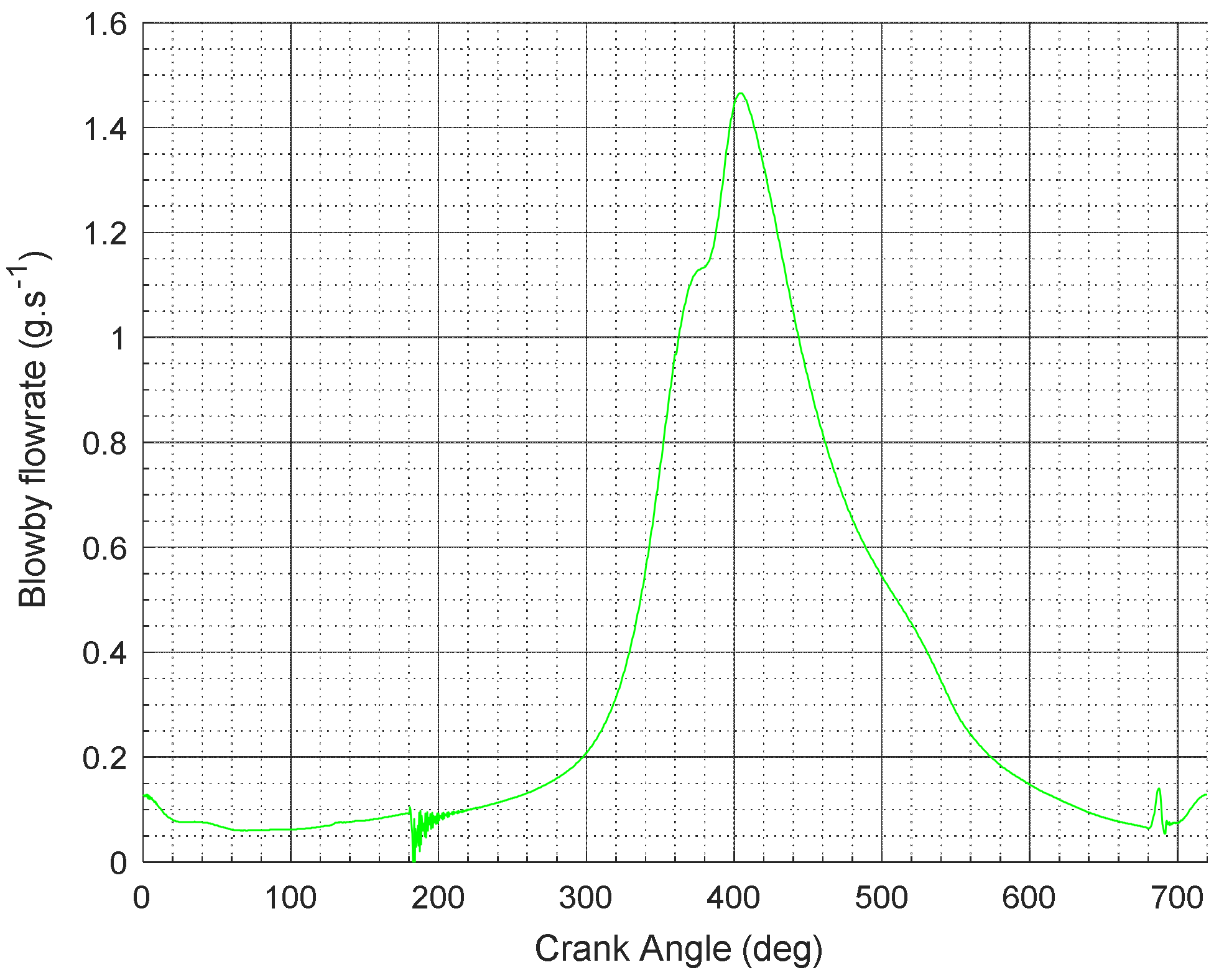
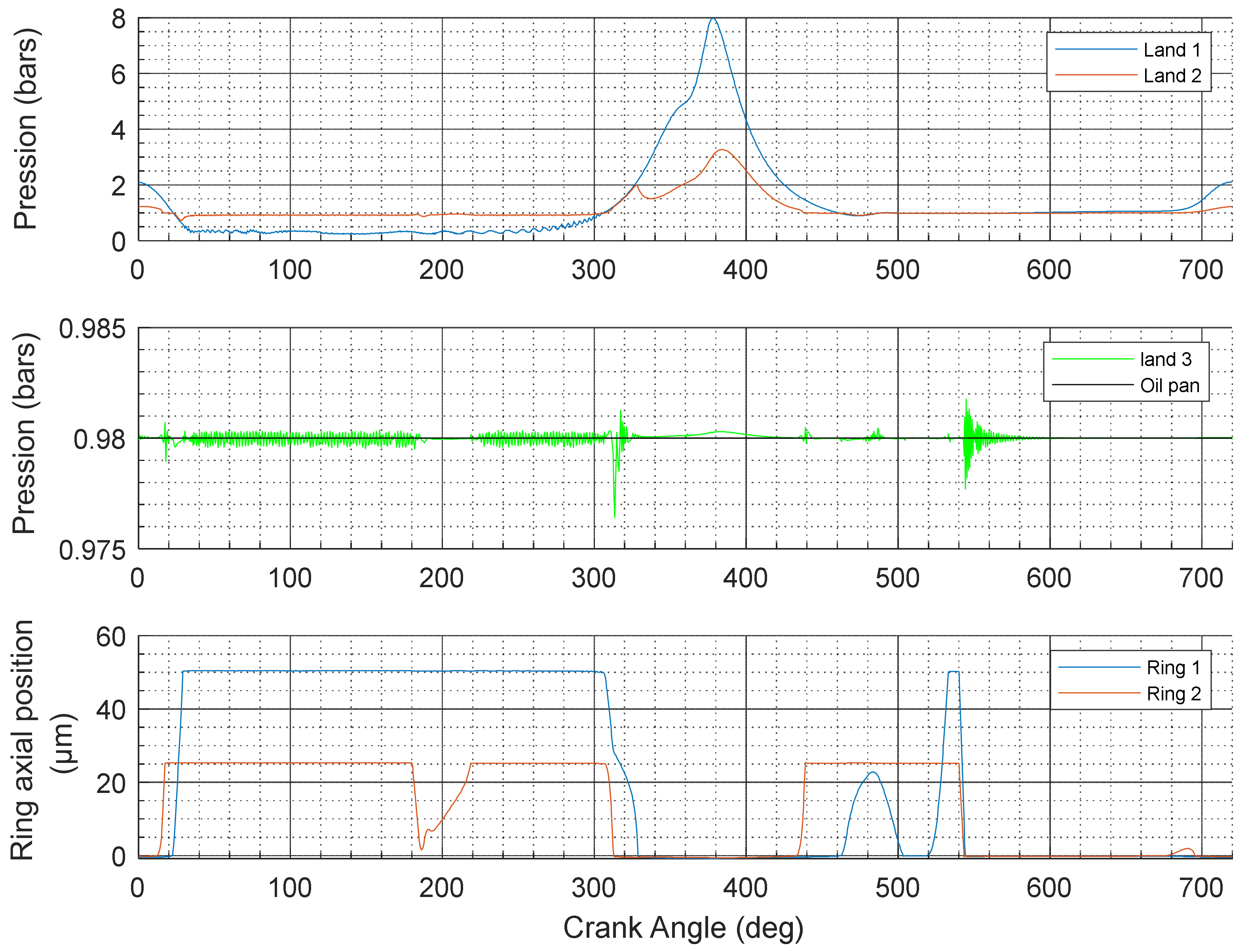
3.1. Building a Blowby Simulation Model
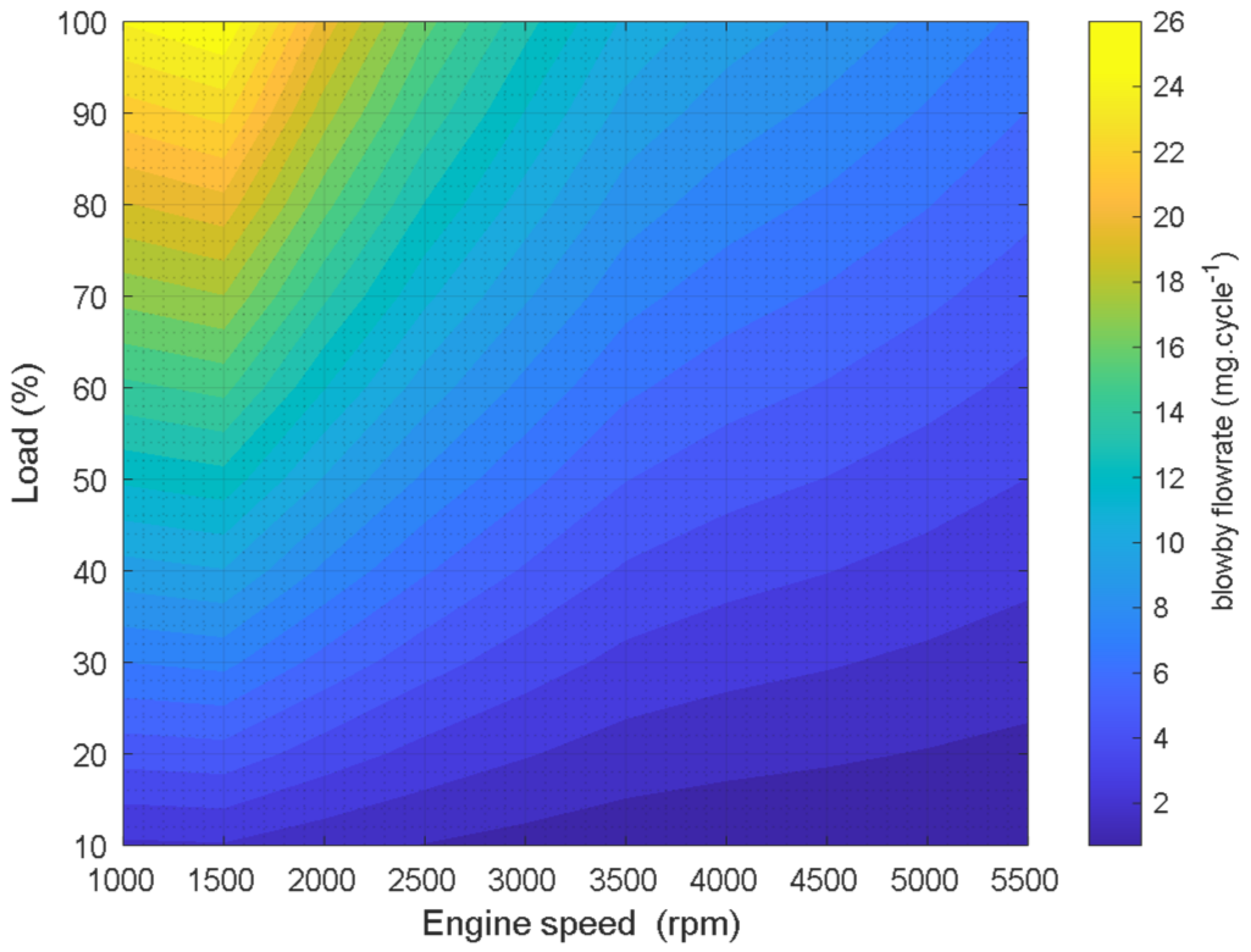
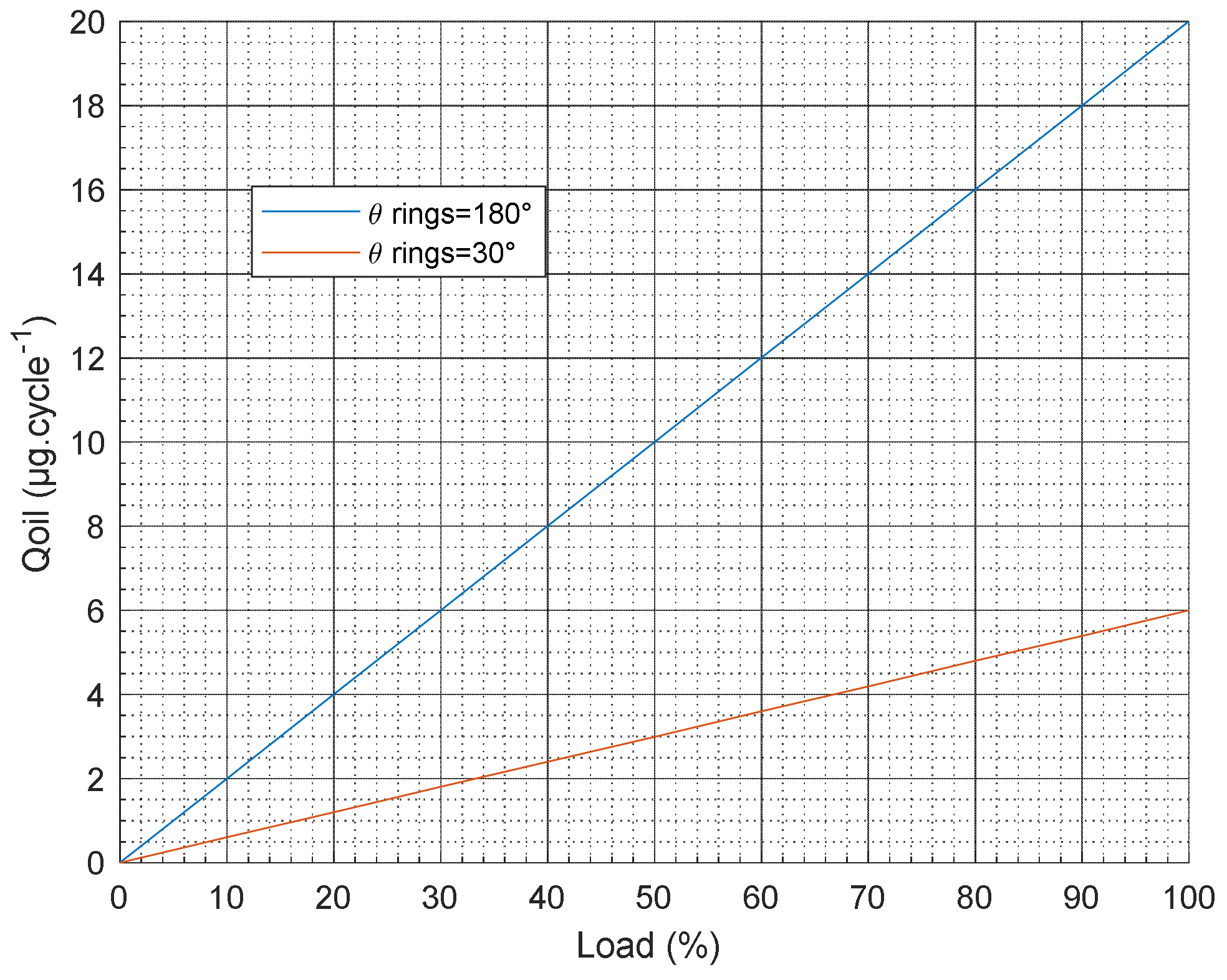
3.2. Analysis of Oil Flow Carried by Blowby Gases
3.3. Influence of Blowby on Particulate Emissions at Idle
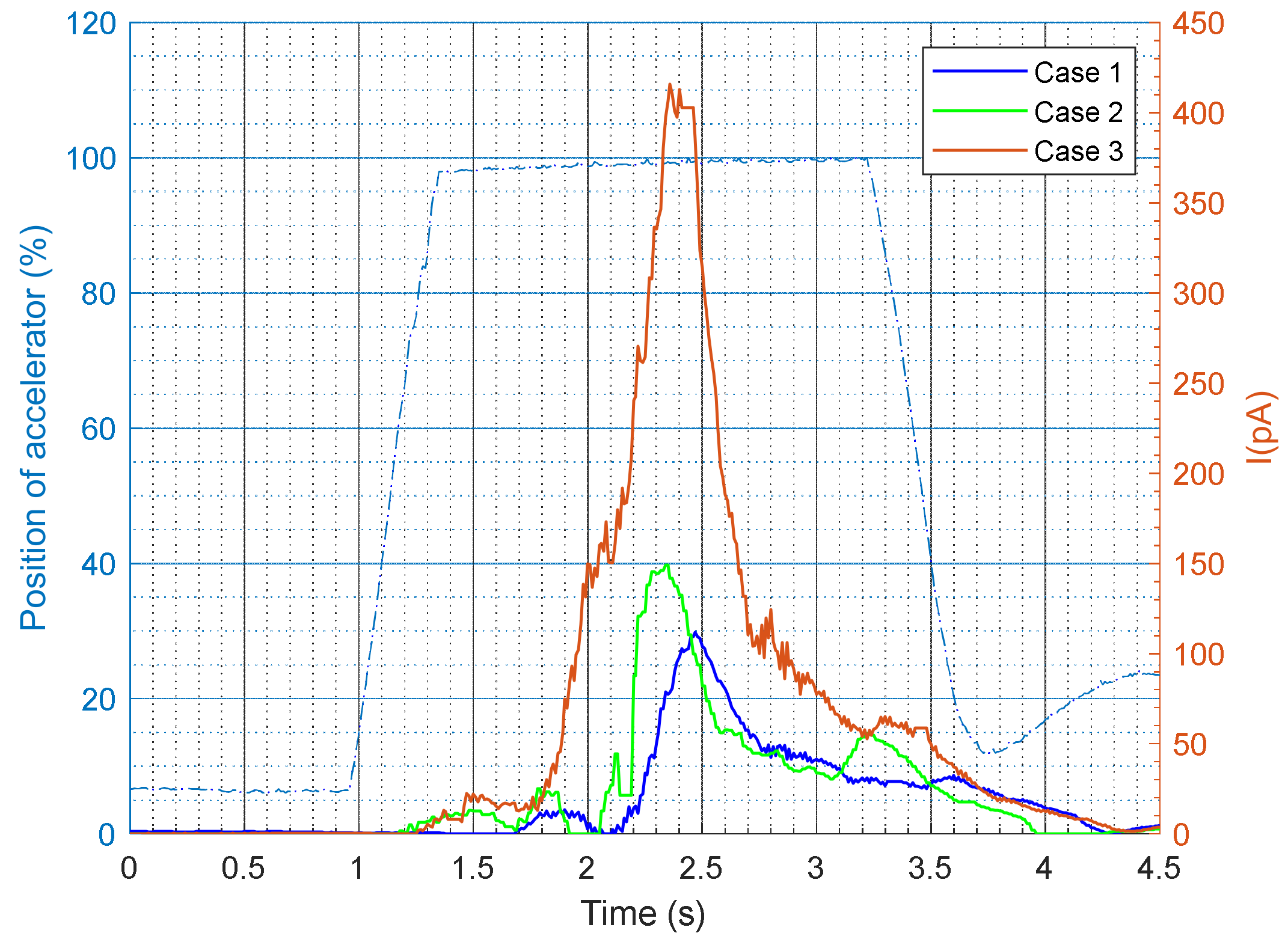
4. Influence of Idling Time between Two Accelerations on Particulate Emissions
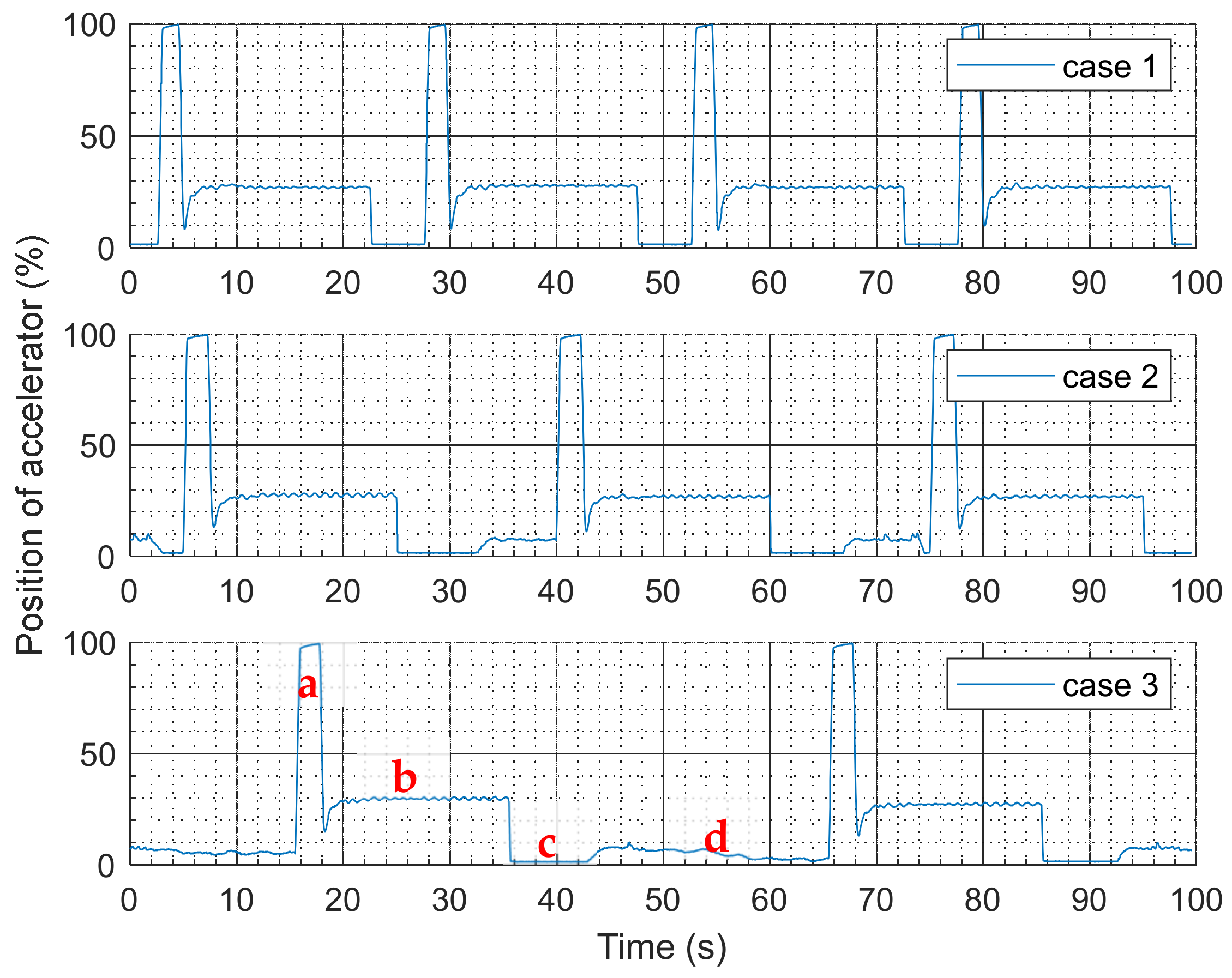
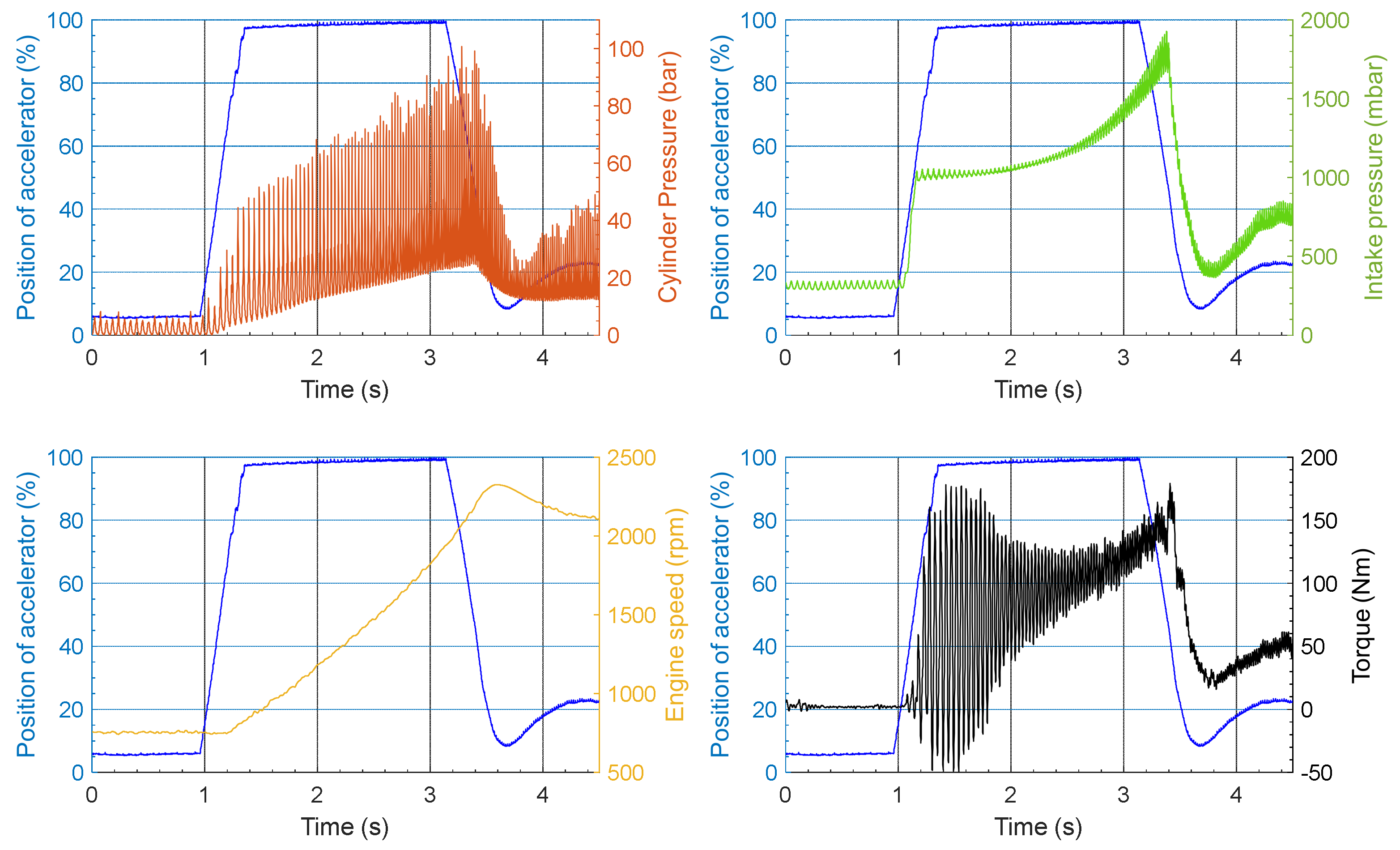
4.1. Setup
4.2. Particles Measuring Device
4.3. Test Results and Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| m | Kg | Ring mass |
| h | m | Variation of axial ring position in the groove |
| Fp | N | Pressure forces |
| Fi | N | Inertial forces |
| Ffr | N | Friction forces |
| Foil | N | Oil film pressure forces |
| Fa | N | Adhesion forces |
| m3.cycle−1 | Blowby flow | |
| Pa.s | Dynamic viscosity of air | |
| Pa.s | Dynamic viscosity of oil | |
| ° | Angle formed between the endgap of the first two rings | |
| m | Clearance between piston radius and cylinder radius | |
| hair | m | Clearance between piston and cylinder |
| and | - | Constants mainly related to the engine speed |
| WLTP | - | Worldwide Harmonized Light Vehicle Test Procedure |
| PAH | - | Polycyclic Aromatic Hydrocarbon |
| TGDI | - | Turbocharged Gasoline Direct Injection |
| PPS | - | Pegasor Particles Sensor |
| TDC | - | Top Dead Center |
| BDC | - | Bottom Dead Center |
| ICE | - | Internal Combustion Engine |
| Land 1 | - | Space between the piston top and the first ring |
| Land 2 | - | Space between the first two rings |
| Land 3 | - | Space between the second and third ring |
| pA | , avec n = test number | |
| - | Standard deviation |
References
- Commission Européenne. Règlement (UE) n°459/2012. J. Off. Union Eur. 2012, 2012, 16–24. [Google Scholar]
- Barbussse, S.; Plassat, G. Les Particules de Combustion Automobile et Leurs Dispositifs D’élimination. 2005. Available online: http://scholar.google.com/scholar?hl=en&btnG=Search&q=intitle:Les+particules+de+combustion+automobile+et+leurs+dispositifs+d??limination#0 (accessed on 5 October 2021).
- Marchal, C. Modélisation de la Formation et de L’oxydation des Suies Dans un Moteur Automobile. Ph.D. Thesis, Université d’Orléans, Orléans, France, 2008. [Google Scholar]
- Law, M.E.; Westmoreland, P.; Cool, T.A.; Wang, J.; Hansen, N.; Taatjes, C.; Kasper, T. Benzene precursors and formation routes in a stoichiometric cyclohexane flame. Proc. Combust. Inst. 2007, 31, 565–573. [Google Scholar] [CrossRef]
- Ketterer, J.E. Soot Formation in Direct Injection Spark Ignition Engines Under Cold-Idle Operating Conditions. Ph.D. Thesis, Massachusetts Institute of Technology, Cambridge, MA, USA, 2013. [Google Scholar]
- Krestinin, A. Detailed modeling of soot formation in hydrocarbon pyrolysis. Combust. Flame 2000, 121, 513–524. [Google Scholar] [CrossRef]
- Bockhorn, H. Detailed Mechanism and Modeling of Soot Particle Formation. In Soot Formation in Combustion; Springer: Berlin/Heidelberg, Germany, 1994. [Google Scholar]
- Kittelson, D.B. Engines and nanoparticles: A review. J. Aerosol Sci. 1998, 29, 575–588. [Google Scholar] [CrossRef]
- Cheng, W.K.; Hamrin, D.; Heywood, J.B.; Hochgreb, S.; Min, K.; Norris, M. An Overview of Hydrocarbon Emissions Mechanisms in Spark-Ignition Engines; Technical Papers; SAE International: Warrendale, PA, USA, 1993. [Google Scholar] [CrossRef]
- Thawko, A.; Yadav, H.; Eyal, A.; Shapiro, M.; Tartakovsky, L. Particle emissions of direct injection internal combustion engine fed with a hydrogen-rich reformate. Int. J. Hydrog. Energy 2019, 44, 28342–28356. [Google Scholar] [CrossRef]
- Swanson, J.; Ragatz, A.; Watts, W.; Kittelson, D.; Winsor, R. Nanoparticle measurements used to detect an engine oil control ring failure. Proc. Inst. Mech. Eng. Part D J. Automob. Eng. 2009, 223, 1071–1076. [Google Scholar] [CrossRef]
- Berthome, V. Etude des Émissions de Particules d’un Moteur à Combustion Interne de Type Allumage Commandé. Ph.D. Thesis, Ecole Centrale de Nantes, Nantes, France, 2021. [Google Scholar]
- Berthome, V.; Chalet, D.; Hetet, J.-F. Characterization of Particle Emissions of Turbocharged Direct Injection Gasoline Engine in Transients and Hot Start Conditions. J. Therm. Sci. 2021, 30, 2056–2070. [Google Scholar] [CrossRef]
- Amirante, R.; Distaso, E.; Tamburrano, P.; Reitz, R.D. Measured and Predicted Soot Particle Emissions from Natural Gas Engines; Technical Papers; SAE International: Warrendale, PA, USA, 2015; Volume 2015. [Google Scholar] [CrossRef]
- Delvigne, T. Oil Consumption Sources in a Modern Gasoline Engine Including Contribution of Blow-by Separator and Turbocharger: An Experimental Study Based on the Use of Radiotracers. SAE Int. J. Fuels Lubr. 2010, 3, 916–924. [Google Scholar] [CrossRef]
- Manni, M.; Carriero, M.; Roselli, A. A Study of Oil Consumption on a Diesel Engine with Independently Lubricated Turbocharger; Technical Papers; SAE International: Warrendale, PA, USA, 2002. [Google Scholar] [CrossRef]
- Yilmaz, E. Sources and Characteristics of Oil Consumption in a Spark-Ignition Engine. Ph.D. Thesis, Massachusetts Instititute of Technologie, Cambridge, MA, USA, 2003. [Google Scholar]
- Gohl, M.; Friedmann, M.; Holzmüller, J.; Wulff, R.; Braun, M. Impact of Lubricating Oil on Particle Emissions. In Proceedings of the SIA Powertrain & Power Electronics Congress, Port-Marly, France, 9–10 June 2021; pp. 47–54. [Google Scholar]
- Yilmaz, E.; Tian, T.; Wong, V.W.; Heywood, J.B. The Contribution of Different Oil Consumption Sources to Total Oil Consumption in a Spark Ignition Engine; Technical Papers; SAE International: Warrendale, PA, USA, 2004. [Google Scholar] [CrossRef]
- Berthome, V.; Chalet, D.; Hetet, J.-F. Impact of Blow-By Gas and Endgap Ring Position on the Variations of Particle Emissions in Gasoline Engines. Energies 2021, 14, 7492. [Google Scholar] [CrossRef]
- Premnath, M.S.V.; Khalek, I.; Michlberger, A. Effect of Lubricant Oil on Particle Emissions from a Gasoline Direct Injection Light-Duty Vehicle; Technical Papers; SAE International: Warrendale, PA, USA, 2018; pp. 1–11. [Google Scholar] [CrossRef]
- Amirante, R.; Distaso, E.; Napolitano, M.; Tamburrano, P.; Di Iorio, S.; Sementa, P.; Vaglieco, B.M.; Reitz, R.D. Effects of lubricant oil on particulate emissions from port-fuel and direct-injection spark-ignition engines. Int. J. Engine Res. 2017, 18, 606–620. [Google Scholar] [CrossRef]
- Jues, T. Modélisation et Simulation des gaz de Blow-by dans un Décanteur Automobile. Ph.D Thesis, L’Ecole Nationale Supérieure d’Arts et Métiers, Paris, France, 2010. [Google Scholar]
- Min, B.-S.; Kim, J.-S.; Oh, D.-Y.; Choi, J.-K.; Jin, J.-H. Dynamic Characteristics of Oil Consumption- Relationship Between the Instantaneous Oil Consumption and the Location of Piston Ring Gap; Technical Papers; SAE International: Warrendale, PA, USA, 1998. [Google Scholar] [CrossRef]
- Gohl, M.; Matz, G.; Preuss, A.-C.; Pischinger, S.; Günther, M.; Ebert, T. Investigation of Oil Sources in the Combustion Chamber of Direct Injection Gasoline Engines; Technical Papers; SAE International: Warrendale, PA, USA, 2018; Volume 2018, pp. 1–11. [Google Scholar] [CrossRef]
- Thirouard, B.; Hart, D.P. Investigation of Oil Transport Mechanisms on the Piston Second Land of a Single Cylinder Diesel Engine, Using Two-Dimensional-Laser-Induzed Fluorescence; Technical Papers; SAE International: Warrendale, PA, USA, 1998. [Google Scholar] [CrossRef]
- Delprete, C.; Selmani, E.; Bisha, A. Gas escape to crankcase: Impact of system parameters on sealing behavior of a piston cylinder ring pack. Int. J. Energy Environ. Eng. 2019, 10, 207–220. [Google Scholar] [CrossRef] [Green Version]
- Thirouard, B.; Tian, T. Oil Transport in the Piston Ring Pack (Part II): Zone Analysis and Macro Oil Transport Model Benoist; Technical Papers; SAE International: Warrendale, PA, USA, 2003. [Google Scholar] [CrossRef]
- Nakashima, K.; Ishihara, S.; Urano, K. Influence of Piston Ring Gaps on Lubricating Oil Flow into the Combustion Chamber; Technical Papers; SAE International: Warrendale, PA, USA, 1995. [Google Scholar] [CrossRef]
- Agarwal, V.K.; Ajay Paul, J. Optimization of Piston and Ringpack Design to Improve the Performance and Emission Characteristics of a Gasoline Engine; Technical Papers; SAE International: Warrendale, PA, USA, 2013; Volume 12. [Google Scholar] [CrossRef]
- Tian, T. Dynamic behaviours of piston rings and their practical impact. Part 2: Oil transport, friction and wear of ring/liner interface and the effects of piston and ring dynamics. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2002, 216, 209–228. [Google Scholar] [CrossRef]
- Iijima, N.; Miyamoto, T.; Takiguchi, M.; Kai, R.; Sato, M. An Experimental Study on Phenomena of Piston Ring Collapse; Technical Papers; SAE International: Warrendale, PA, USA, 2002. [Google Scholar] [CrossRef]
- Tian, T. Dynamic behaviours of piston rings and their practical impact. Part 1: Ring flutter and ring collapse and their effects on gas flow and oil transport. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2002, 216, 209–228. [Google Scholar] [CrossRef]
- Cheng, C.; Schock, H.; Richardson, D. The Dynamics of Second Ring Flutter and Collapse in Modern Diesel Engines. J. Eng. Gas Turbines Power 2015, 137, 111504. [Google Scholar] [CrossRef]
- Rabute, R.; Tian, T. Challenges Involved in Piston Top Ring Designs for Modern SI Engines. J. Eng. Gas Turbines Power 2001, 123, 448–459. [Google Scholar] [CrossRef]
- Wróblewski, P.; Koszalka, G. An Experimental Study on Frictional Losses of Coated Piston Rings with Symmetric and Asymmetric Geometry. SAE Int. J. Engines 2021, 14, 6. [Google Scholar] [CrossRef]
- Wróblewski, P.; Iskra, A. Problems of Reducing Friction Losses of a Piston-Ring-Cylinder Configuration in a Combustion Piston Engine with an Increased Isochoric Pressure Gain; Technical Papers; SAE International: Warrendale, PA, USA, 2020. [Google Scholar] [CrossRef]
- Zarenbin, V.; Kolesnikova, T.; Sakno, O.; Ollo, V.; Klimenko, V. Impact Evaluation of Piston Rings Mobility on a Gas Passage in an Internal Combustion Engine (ICE). Sci. J. Sil. Univ. Technol. Ser. Transp. 2019, 104, 187–201. [Google Scholar] [CrossRef]
- Turnbull, R.; Dolatabadi, N.; Rahmani, R.; Rahnejat, H. An assessment of gas power leakage and frictional losses from the top compression ring of internal combustion engines. Tribol. Int. 2020, 142, 105991. [Google Scholar] [CrossRef]
- Schneider, E.W.; Blossfeld, D.H. Method for Measurement of Piston Ring Rotation in an Operating Engine; Technical Papers; SAE International: Warrendale, PA, USA, 1990. [Google Scholar] [CrossRef]
- Uhlig, B.P.; Kirner, C.; Preuss, A.-C.; Wachtmeister, G. Real-Time Measurement of the Piston Ring Gap Positions and Their Effect on Exhaust Engine Oil Emission; Technical Papers; SAE International: Warrendale, PA, USA, 2018; pp. 1–8. [Google Scholar] [CrossRef]
- Thiel, N.; Weimar, H.-J.; Kamp, H.; Windisch, H. Advanced Piston Cooling Efficiency: A Comparison of Different New Gallery Cooling Concepts; Technical Papers; SAE International: Warrendale, PA, USA, 2007; Volume 2007, pp. 776–790. [Google Scholar] [CrossRef]
- Abril, S.; García, C.; León, J. Numerical and Experimental Analysis of the Potential Fuel Savings and Reduction in CO Emissions by Implementing Cylinder Bore Coating Materials Applied to Diesel Engines. Lubricants 2021, 9, 19. [Google Scholar] [CrossRef]
- Husberg, T.; Gjirja, S.; Denbratt, I.; Omrane, A.; Aldén, M.; Engström, J. Piston Temperature Measurement by Use of Thermographic Phosphors and Thermocouples in a Heavy-Duty Diesel Engine Run Under Partly Premixed Conditions; Technical Papers; SAE International: Warrendale, PA, USA, 2005. [Google Scholar] [CrossRef]
- AVL GmbH. AVL 442, Operating and Service Manual Calibration and Procedure, AT0203E-07, November 1996, AVL List GmbH A8020 Gra-Austria. Available online: https://www.avl.com/documents/10138/2699442/AVL+Blow+By+Meter+Product+Description (accessed on 22 October 2022).
- Koszalka, G.; Guzik, M. Mathematical Model of Piston Ring Sealing in Combustion Engine. Pol. Marit. Res. 2014, 21, 66–78. [Google Scholar] [CrossRef] [Green Version]
- Takiguchi, M.; Nakayama, K.; Furuhama, S.; Yoshida, H. Variation of Piston Ring Oil Film Thickness in an Internal Combustion Engine–Comparison Between Thrust and Anti-Thrust Sides; Technical Papers; SAE International: Warrendale, PA, USA, 1998. [Google Scholar] [CrossRef]
- Thirouard, B. Characterization and Modeling of the Fundamental Aspects of Oil Transport in the Piston Ring Pack of Internal Combustion Engines. Ph.D. Thesis, Massachusetts Instititute of Technologie, Cambridge, MA, USA, 2001. [Google Scholar]
- Tomizawa, K.; Ito, A. Development of a New Method to Measure the Rotational Force Acting on the Piston Rings of a Gasoline Engine; Technical Papers; SAE International: Warrendale, PA, USA, 2019; pp. 1–5. [Google Scholar] [CrossRef]
- Yilmaz, E.; Thirouard, B.; Tian, T.; Wong, V.W.; Heywood, J.B.; Lee, N. Analysis of Oil Consumption Behavior during Ramp Transients in a Production Spark Ignition Engine; Technical Papers; SAE International: Warrendale, PA, USA, 2001. [Google Scholar] [CrossRef]
- Pegasor. User Manual; Pegasor Ltd.: Tampere, Finland, 2014; Volume 3. [Google Scholar]
- Amanatidis, S.; Maricq, M.M.; Ntziachristos, L.; Samaras, Z. Measuring number, mass, and size of exhaust particles with diffusion chargers: The dual Pegasor Particle Sensor. J. Aerosol Sci. 2016, 92, 1–15. [Google Scholar] [CrossRef]
- Ntziachristos, L.; Amanatidis, S.; Samaras, Z.; Janka, K.; Tikkanen, J. Application of the Pegasor Particle Sensor for the Measurement of Mass and Particle Number Emissions. SAE Int. J. Fuels Lubr. 2013, 6, 521–531. [Google Scholar] [CrossRef]




| Engine Main Features | Main Parameters of Piston Rings | ||
|---|---|---|---|
| Engine | 4-stroke, TGDI, 3-cylinder | Piston diameter | 74.45 mm |
| Bore × Stroke | 75 mm × 90.48 mm | Ring 1 axial height | 1.2 mm |
| Displacement | 1199.9 cm3 | Ring 2 axial height | 1 mm |
| Valves | 12 | Ring 3 axial height | 2 mm |
| Compression ratio | 10.5: 1 | Ring 1 end gap | 0.2 mm |
| Maximum Power | 96 kW @ 5500 rpm | Ring 2 end gap | 0.4 mm |
| Maximum Torque | 230 Nm @ 1750 rpm | Ring 3 end gap | 0.2 mm |
| Engine speed (rpm) | 1000 | 1500 | 2000 | 2500 | 3000 | 3500 | 4000 | 4500 | 5000 | 5500 | 6000 |
| Qv (l.min−1) | 31 | 52 | 51 | 52 | 51 | 49 | 49 | 49 | 51 | 49 | 47 |
| Qm (mg.cycle−1) | 24.1 | 26.9 | 19.9 | 16.2 | 13.2 | 10.9 | 9.51 | 8.46 | 7.92 | 6.92 | 6.08 |
| Pressure (mbars) | Averaged Temperature (°C) | ||||||
|---|---|---|---|---|---|---|---|
| Atmospheric | Sample line PPS | Intake | Cooling | Oil | Engine bench | Exhaust | Sample line PPS |
| 1013 | 1538 | 26 | 93 | 96 | 17 | 356 | 170 |
| Case Number | Idling Time (s) | σ (%) | |
|---|---|---|---|
| 1 | 0 | 105 | 13 |
| 2 | 7 | 133 | 15 |
| 3 | 22 | 329 | 16 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Berthome, V.; Chalet, D.; Hetet, J.-F. Consequence of Blowby Flow and Idling Time on Oil Consumption and Particulate Emissions in Gasoline Engine. Energies 2022, 15, 8772. https://doi.org/10.3390/en15228772
Berthome V, Chalet D, Hetet J-F. Consequence of Blowby Flow and Idling Time on Oil Consumption and Particulate Emissions in Gasoline Engine. Energies. 2022; 15(22):8772. https://doi.org/10.3390/en15228772
Chicago/Turabian StyleBerthome, Vincent, David Chalet, and Jean-François Hetet. 2022. "Consequence of Blowby Flow and Idling Time on Oil Consumption and Particulate Emissions in Gasoline Engine" Energies 15, no. 22: 8772. https://doi.org/10.3390/en15228772
APA StyleBerthome, V., Chalet, D., & Hetet, J.-F. (2022). Consequence of Blowby Flow and Idling Time on Oil Consumption and Particulate Emissions in Gasoline Engine. Energies, 15(22), 8772. https://doi.org/10.3390/en15228772








