Active Disturbance Rejection Control of an Interleaved High Gain DC-DC Boost Converter for Fuel Cell Applications
Abstract
1. Introduction
- -
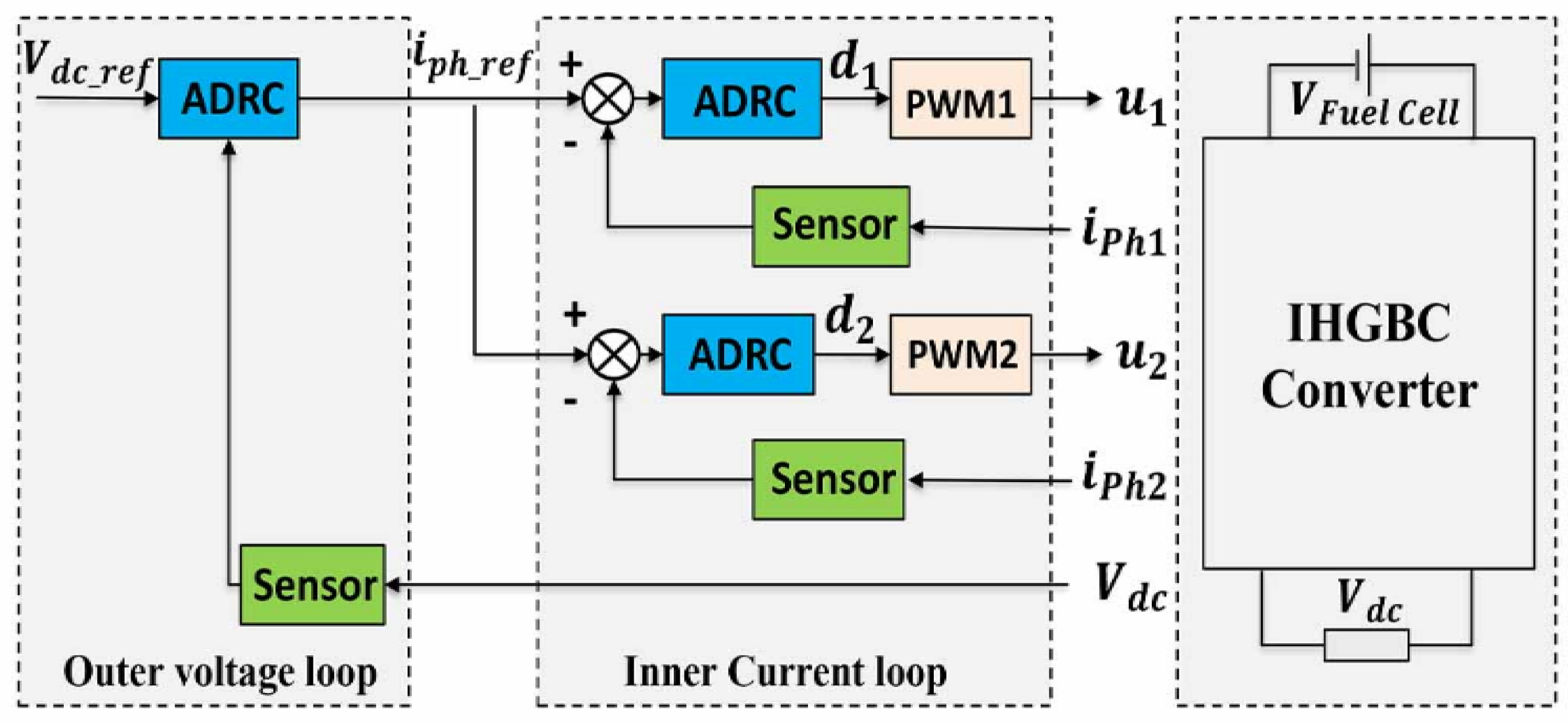
- A robust dual-loop control application and design based on a linear active disturbance rejection control that controls the external voltage loop and the internal current loop of an interleaved DC-DC boost converter.
- -
- This converter provides high efficiency and high voltage gain with reduced voltage stress on switches and diodes.
2. PEMFC Modelling
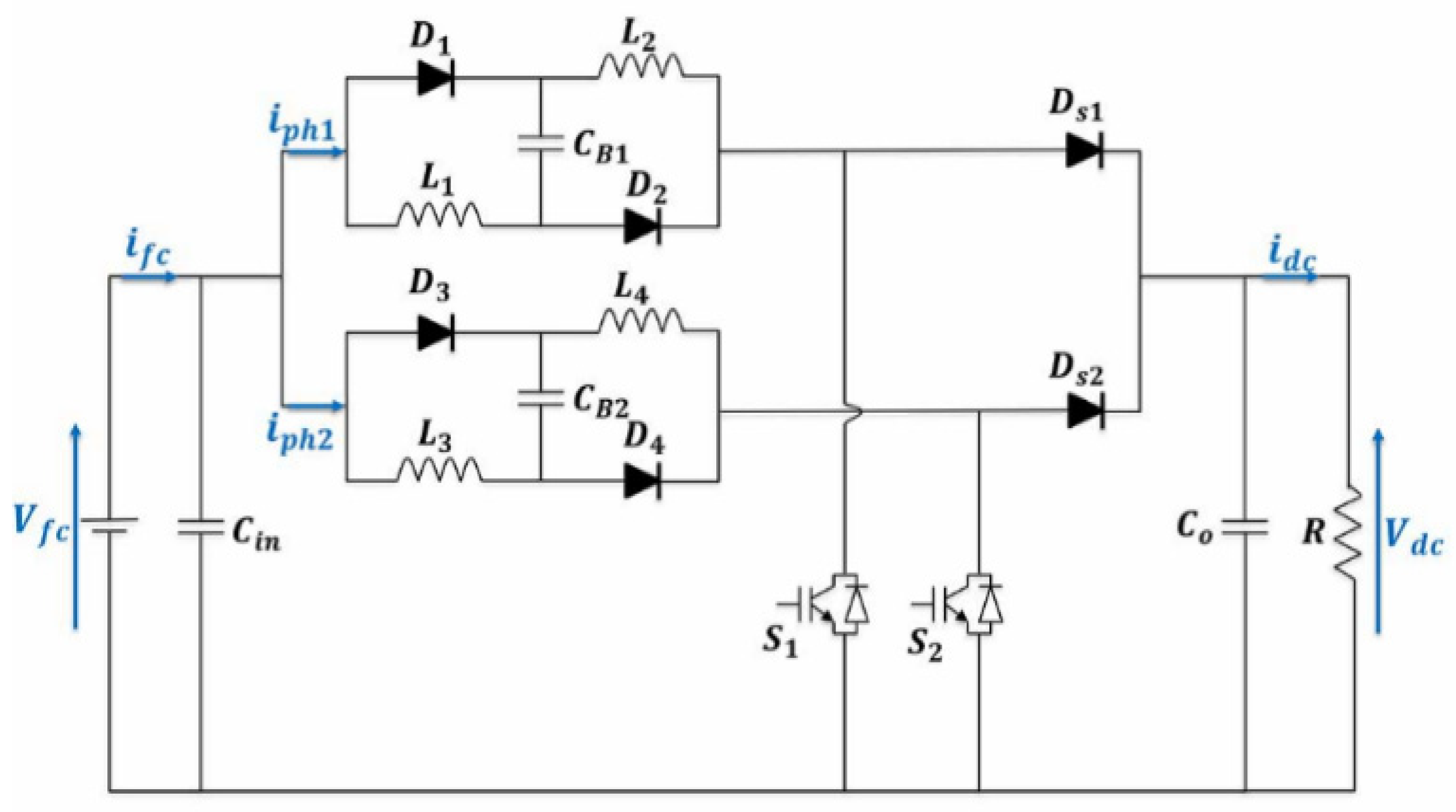
3. Modelling of the Proposed Converter
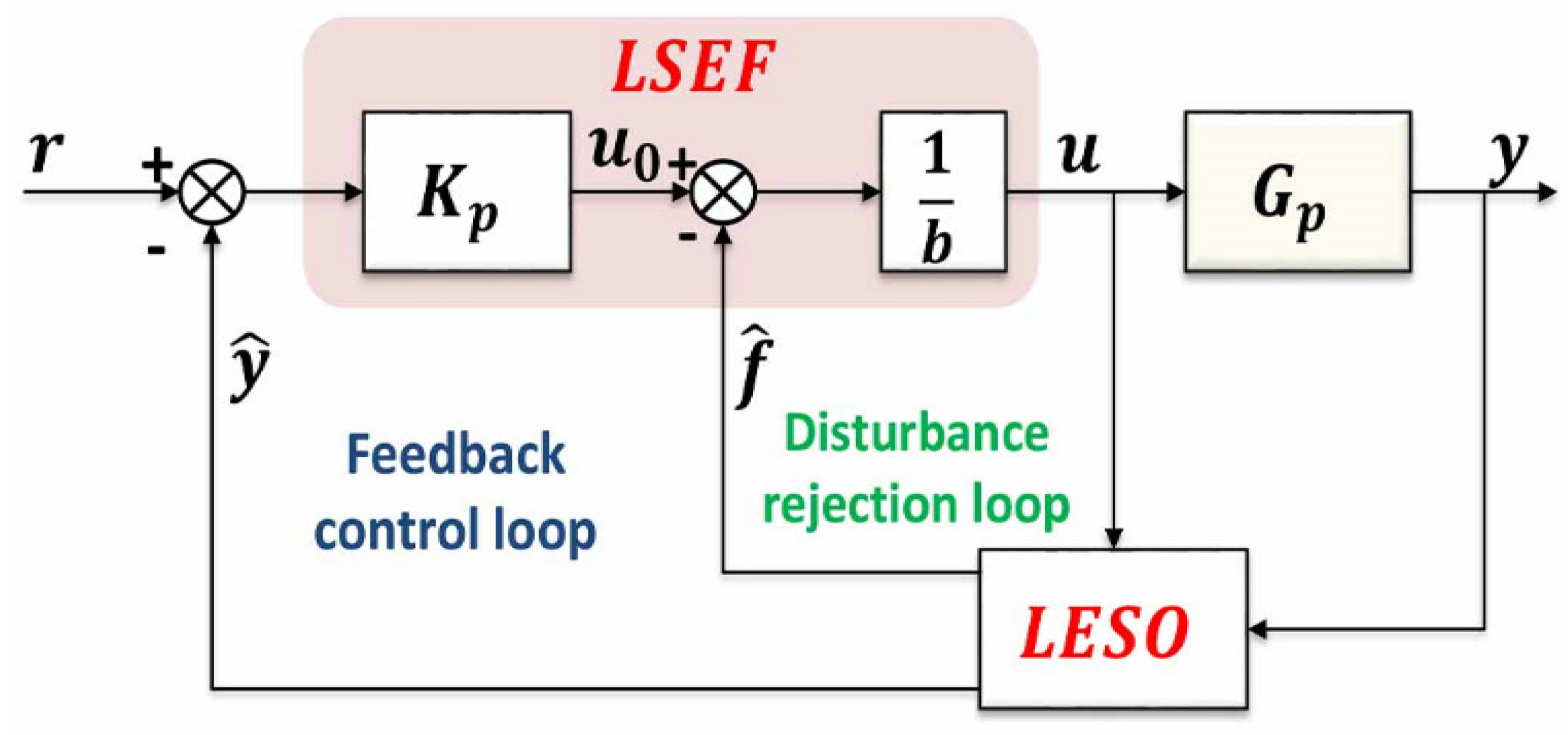
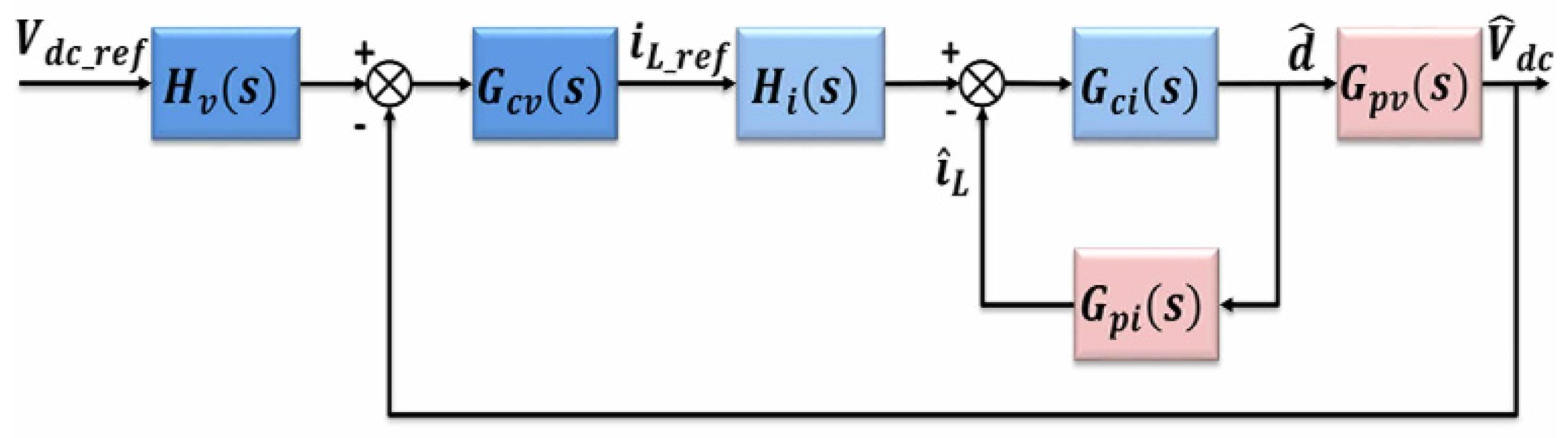
4. LADRC-Based Control Design
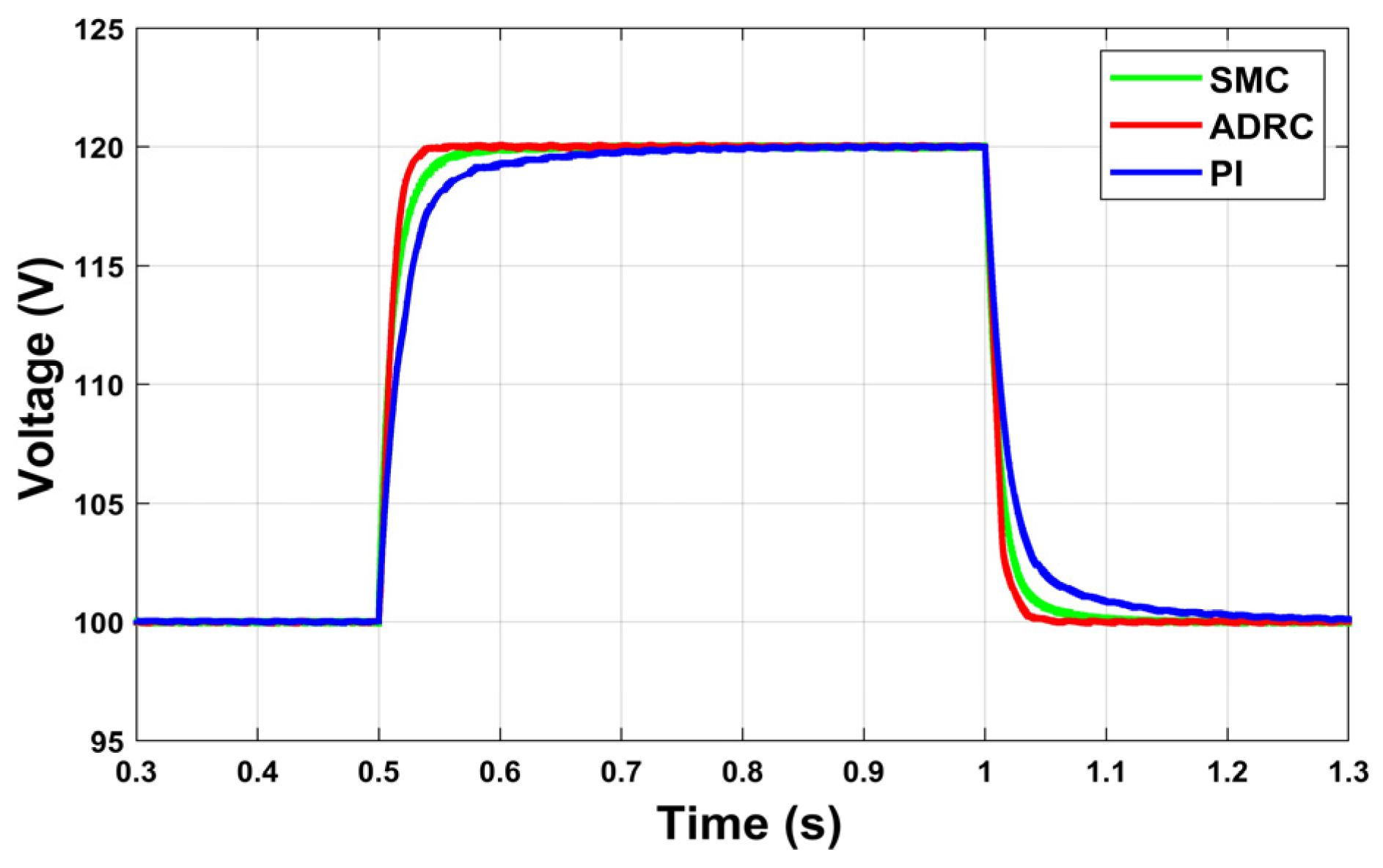
5. Simulation Results
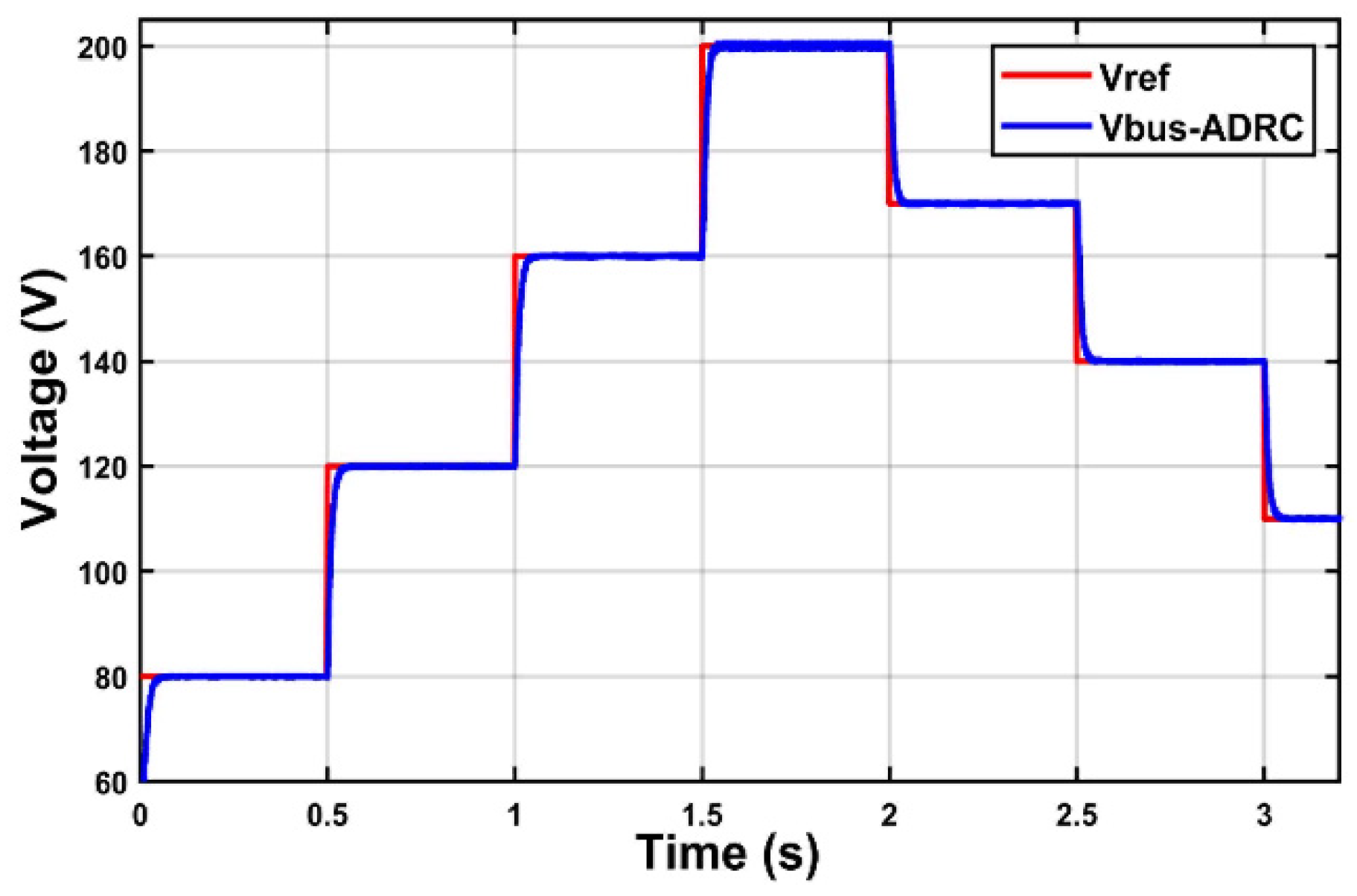
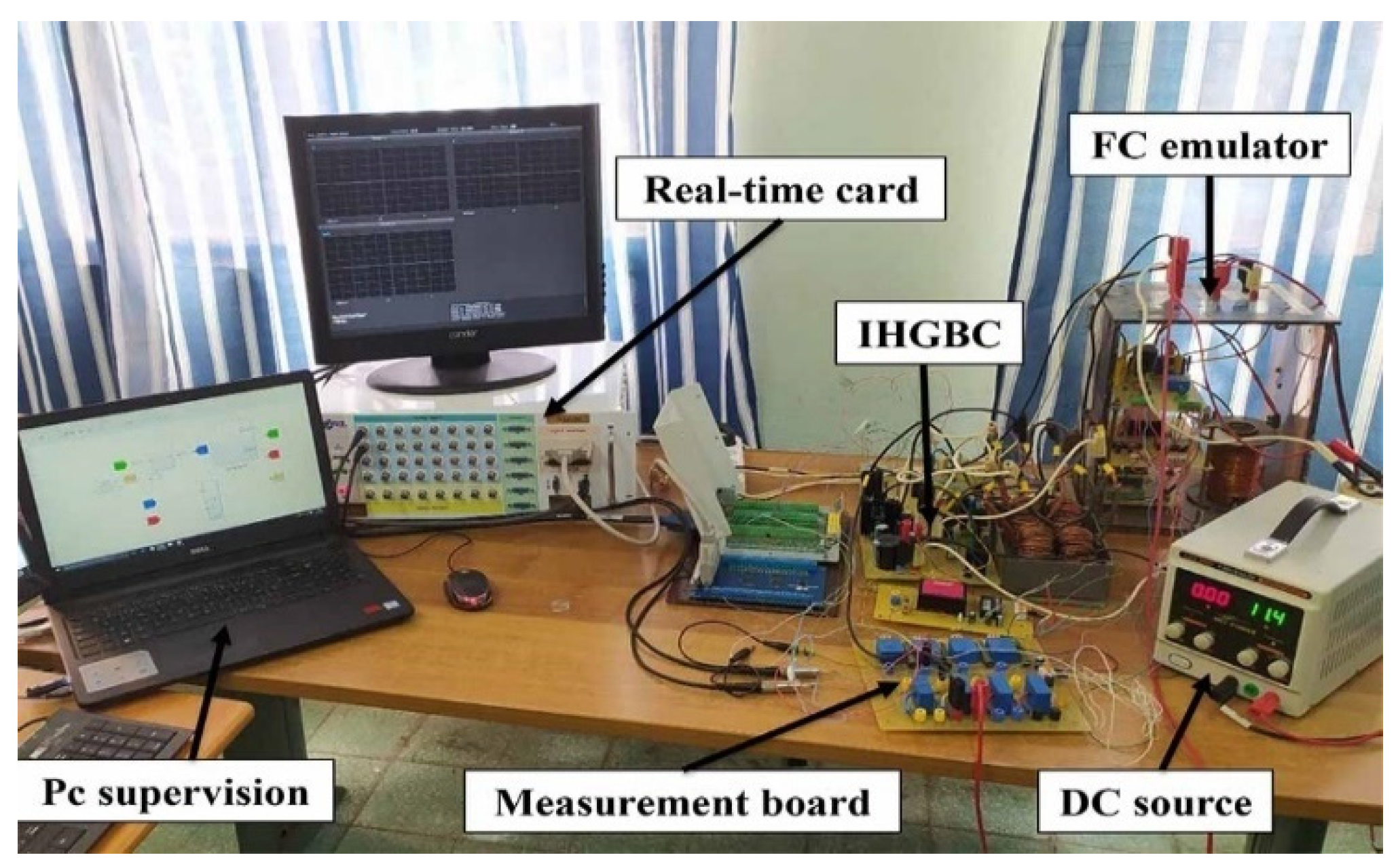
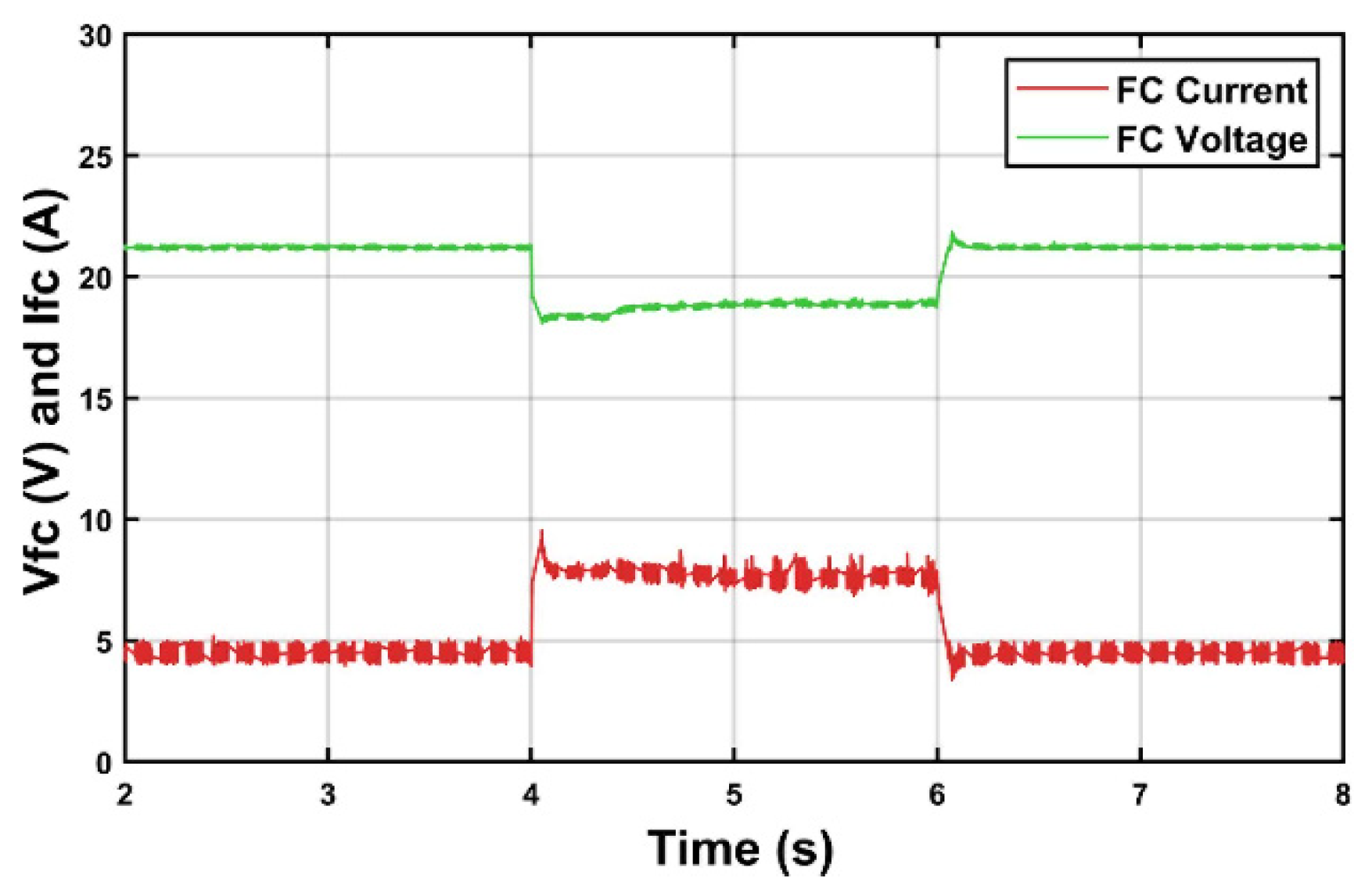
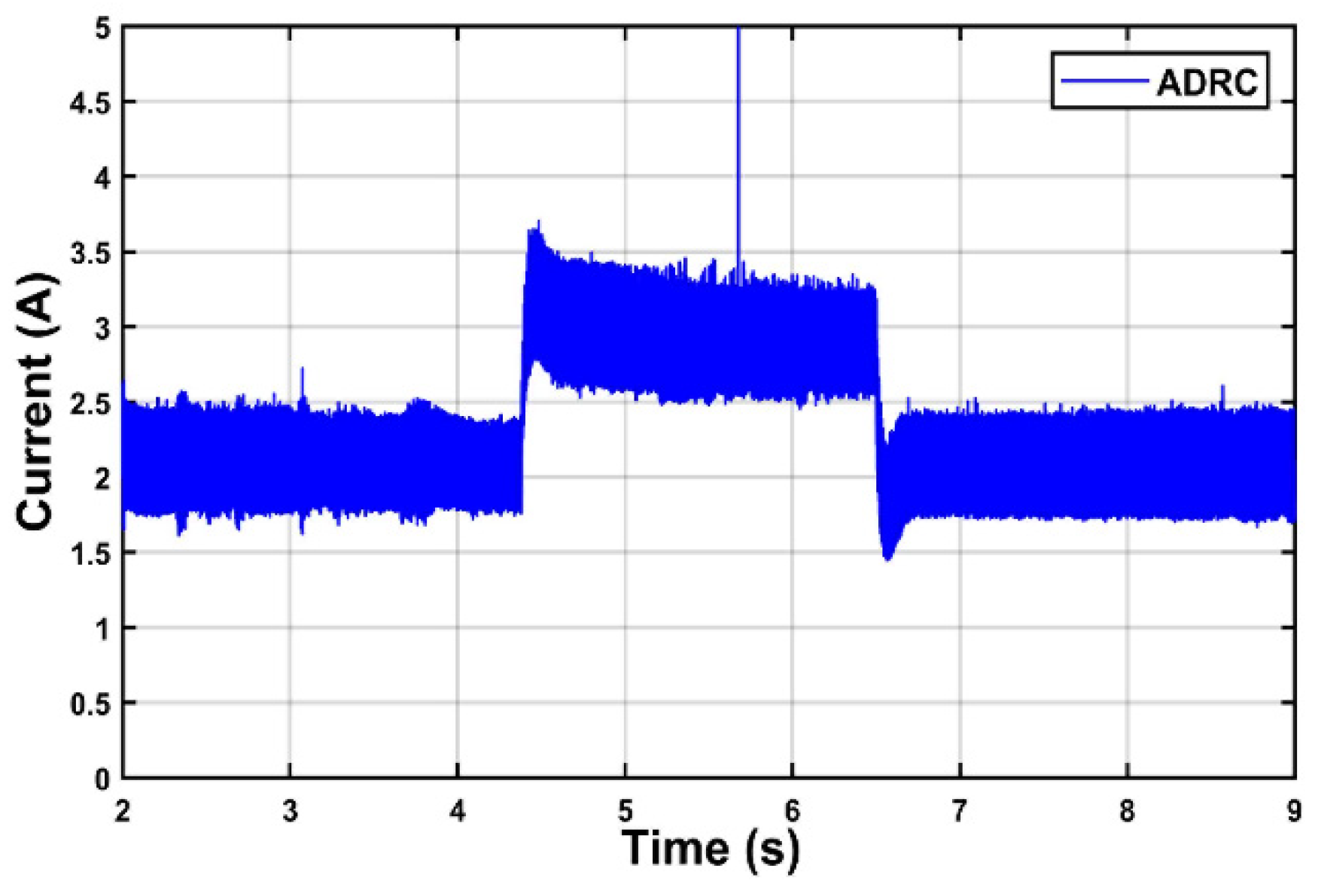
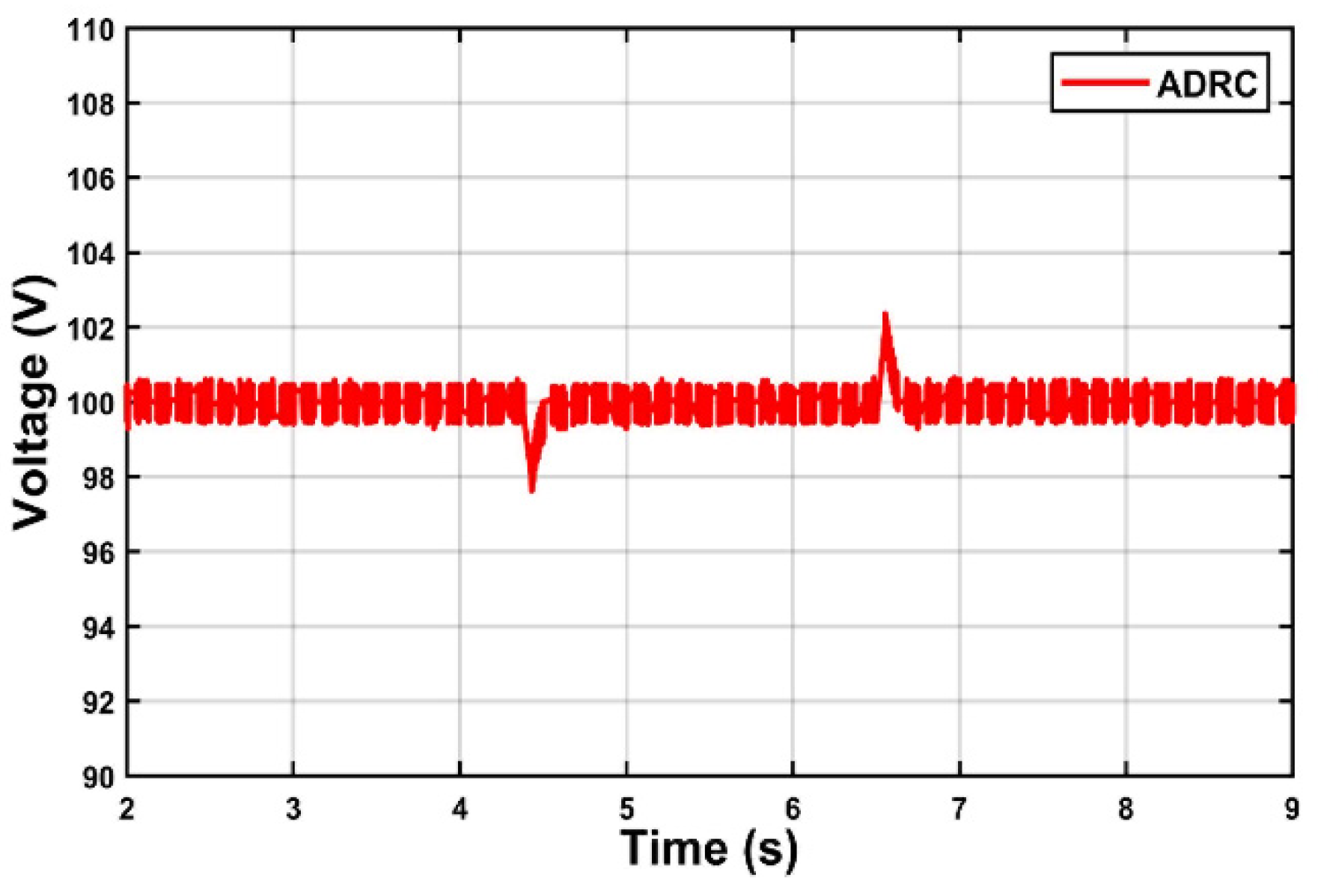
6. Experimental validation
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dafalla, A.M.; Wei, L.; Habte, B.T.; Guo, J.; Jiang, F. Membrane Electrode Assembly Degradation Modeling of Proton Exchange Membrane Fuel Cells: A Review. Energies 2022, 15, 9247. [Google Scholar] [CrossRef]
- Badoud, A.E.; Mekhilef, S.; Ould Bouamama, B. A Novel Hybrid MPPT Controller Based on Bond Graph and Fuzzy Logic in Proton Exchange Membrane Fuel Cell System: Experimental Validation. Arab. J. Sci. Eng. 2022, 47, 3201–3220. [Google Scholar] [CrossRef]
- Mounica, V.; Obulesu, Y.P. Hybrid Power Management Strategy with Fuel Cell, Battery, and Supercapacitor for Fuel Economy in Hybrid Electric Vehicle Application. Energies 2022, 15, 4185. [Google Scholar] [CrossRef]
- Smadi, A.A.; Khoucha, F.; Benrabah, A.; Benbouzid, M. An Improved Model for Fault Tolerant Control of a Flooding and Drying Phenomena in the Proton Exchange Membrane Fuel Cell. In Advances in Renewable Hydrogen and Other Sustainable Energy Carriers; Khellaf, A., Ed.; Springer Proceedings in Energy, Springer: Singapore, 2021. [Google Scholar] [CrossRef]
- Bi, H.; Wang, P.; Che, Y. A capacitor clamped H-type boost DC-DC converter with wide voltage-gain range for fuel cell vehicles. IEEE Trans. Veh. Technol. 2019, 68, 276–290. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhou, L.; Sumner, M.; Wang, P. Single-switch, wide voltage-gain range, boost DC–DC converter for fuel cell vehicles. IEEE Trans. Veh. Technol. 2018, 67, 134–145. [Google Scholar] [CrossRef]
- Guilbert, D.; Gaillard, A.; Mohammadi, A.; N’Diaye, A.; Djerdir, A. Investigation of the interactions between proton exchange membrane fuel cell and interleaved DC/DC boost converter in case of power switch faults. Int. J. Hydrogy Energy 2015, 40, 519–537. [Google Scholar] [CrossRef]
- Saadi, R.; Hammoudi, M.Y.; Kraa, O.; Ayad, M.Y.; Bahri, M. A robust control of a 4-leg floating interleaved boost converter for fuel cell electric vehicle application. Math. Comput. Simulat. 2020, 167, 32–47. [Google Scholar] [CrossRef]
- Kirubakaran, A.; Jain, S.; Nema, R. A review on fuel cell technologies and power electronic interface. Renew Sustain. Energy Rev. 2009, 13, 2430–2440. [Google Scholar] [CrossRef]
- Hasan, N.S.; Rosmin, N.; Osman, D.A.A.; Musta’amal, A.H. Reviews on multilevel converter and modulation techniques. Renew. Sustain. Energy Rev. 2017, 80, 163–174. [Google Scholar] [CrossRef]
- Kolli, A.; Gaillard, A.; De Bernardinis, A.; Bethoux, O.; Hissel, D.; Khatir, Z. A review on DC/DC converter architectures for power fuel cell applications, Energy Convers. Energy Convers. Manag. 2015, 105, 716–730. [Google Scholar] [CrossRef]
- Seguel, J.L.; Seleme, S.I., Jr.; Morais, L.M.F. Comparative Study of Buck-Boost, SEPIC, Cuk and Zeta DC-DC Converters Using Different MPPT Methods for Photovoltaic Applications. Energies 2022, 15, 7936. [Google Scholar] [CrossRef]
- Smadi, A.; Khoucha, F.; Benrabah, A.; Benbouzid, M. Interleaved High Gain DC/DC Boost Converter-Based Proton Exchange Membrane Fuel Cell Fault-Tolerant Control. Int. Rev. Electr. Eng. (IREE) 2022, 17, 447–459. [Google Scholar] [CrossRef]
- Hwu, K.I.; Yau, Y.T. High Step-Up Converter Based on Charge Pump and Boost Converter. IEEE Trans. Power Electron. 2012, 27, 2484–2494. [Google Scholar] [CrossRef]
- Mariscotti, A. Power Quality Phenomena, Standards, and Proposed Metrics for DC Grids. Energies 2021, 14, 6453. [Google Scholar] [CrossRef]
- Gerard, M.; Poirot-Crouzevier, J.P.; Hissel, D.; Pera, M.C. Ripple Current Effects on PEMFC Aging Test by Experimental and Modeling. ASME. J. Fuel. Cell Sci. Technol. 2011, 8, 021004. [Google Scholar] [CrossRef]
- Uno, M.; Tanaka, K. Pt/C catalyst degradation in proton exchange membrane fuel cells due to high-frequency potential cycling induced by switching power converters. J. Power Sources 2011, 196, 9884–9889. [Google Scholar] [CrossRef]
- Forouzesh, M.; Siwakoti, Y.P.; Gorji, S.A.; Blaabjerg, F.; Lehman, B. Step-Up DC-DC converters: A comprehensive review of voltage-boosting techniques, topologies, and applications. IEEE Trans. Power. Electron. 2017, 32, 9143–9178. [Google Scholar] [CrossRef]
- Kobaku, T.; Patwardhan, S.C.; Agarwal, V. Experimental evaluation of internal model control scheme on a DC–DC boost converter exhibiting nonminimum phase behavior. IEEE Trans. Power. Electron. 2017, 32, 8880–8891. [Google Scholar] [CrossRef]
- Wang, W.Y.; Ding, Y.H.; Ke, X.; Ma, X. Sliding mode control of direct coupled interleaved boost converter for fuel cell. In IOP Conference Series: Earth and Environmental Science; Conference 1; IOP Publishing: Bristol, UK, 2017; Volume 100. [Google Scholar] [CrossRef]
- Thammasiriroj, W.; Chunkag, V.; Phattanasak, M.; Pierfederici, S.; Davat, B.; Thounthong, P. Nonlinear single-loop control of the parallel converters for a fuel cell power source used in DC grid applications. Int. J. Electr. Power Energy Syst. 2015, 65, 41–48. [Google Scholar] [CrossRef]
- Hammoudi, M.Y.; Saadi, R.; Cardoso, A.; Benbouzid, M.; Mohamed, S. Practical implementation of H-infinity control for fuel cell-interleaved boost converter. Int. J. Model. Simulat. 2018, 40, 44–61. [Google Scholar] [CrossRef]
- Mumtaz, F.; Yahaya, N.Z.; Meraj, S.T.; Singh, B.; Kannan, R.; Ibrahim, O. Review on non-isolated DC-DC converters and their control techniques for renewable energy applications. Ain. Shams. Eng. J. 2021, 12, 3747–3763. [Google Scholar] [CrossRef]
- Venkatesan, M.; Rajamanickam, N.; Vishnuram, P.; Bajaj, M.; Blazek, V.; Prokop, L.; Misak, S. A Review of Compensation Topologies and Control Techniques of Bidirectional Wireless Power Transfer Systems for Electric Vehicle Applications. Energies 2022, 15, 7816. [Google Scholar] [CrossRef]
- Kanouni, A.; Badoud, A.; Mekhilef, S. A multi-objective model predictive current control with two-step horizon for double-stage grid-connected inverter PEMFC system. Int. J. Hydrog. Energy 2022, 47, 2685–2707. [Google Scholar] [CrossRef]
- Maroufi, O.; Choucha, A.; Chaib, L. Hybrid fractional fuzzy PID design for MPPT-pitch control of wind turbine-based bat algorithm. Electr. Eng. 2020, 102, 2149–2160. [Google Scholar] [CrossRef]
- Han, J. From PID to active disturbance rejection control, IEEE Trans. Ind. Electron. 2009, 56, 900–906. [Google Scholar] [CrossRef]
- Huang, Y.; Xue, W. Active disturbance rejection control: Methodology and theoretical analysis. ISA Trans. 2014, 53, 963–976. [Google Scholar] [CrossRef]
- Gao, Z. Scaling and bandwidth-parameterization based controller tuning. Proc. Amer. Control. Conf. 2003, 4989–4996. [Google Scholar]
- Li, H.; Liu, X.X.; Lu, J.W. Research on Linear Active Disturbance Rejection Control in DC/DC Boost Converter. Electronics 2019, 8, 1249. [Google Scholar] [CrossRef]
- Chiumeo, R.; Raggini, D.; Veroni, A.; Clerici, A. Comparative Analysis of PI and ADRC Control through CHIL Real Time Simulations of a DC-DC DAB into a Multi-Terminal MVDC/LVDC Distribution Network. Energies 2022, 15, 7631. [Google Scholar] [CrossRef]
- Wang, J.; Wang, X.; Luo, Z.; Assadian, F. Active Disturbance Rejection Control of Differential Drive Assist Steering for Electric Vehicles. Energies 2020, 13, 2647. [Google Scholar] [CrossRef]
- Li, H.; Qu, Y. A Composite Strategy for Harmonic Compensation in Standalone Inverter Based on Linear Active Disturbance Rejection Control. Energies 2019, 12, 2618. [Google Scholar] [CrossRef]
- Zuo, Y.; Zhu, X.; Li, Q.; Chao, Z.; Yi, D.; Xiang, Z. Active Disturbance Rejection Controller for Speed Control of Electrical Drives Using Phase-locking Loop Observer. IEEE Trans. Ind. Electron. 2019, 66, 1748–1759. [Google Scholar] [CrossRef]
- Alicia, L.; Keow, J.; Mayhall, A.; Cescon, M.; Chen, Z. Active disturbance rejection control of metal hydride hydrogen storage. Int. J. Hydrog. Energy 2021, 46, 837–851. [Google Scholar] [CrossRef]
- Benrabah, A.; Xu, D.; Gao, Z. Active disturbance rejection control of LCL filtered grid-connected inverter using pade approximation. IEEE Trans. Ind. Appl. 2018, 54, 6179–6189. [Google Scholar] [CrossRef]
- Pukrushpan, J.T.; Peng, H.; Stefanopoulou, A.G. Control oriented modeling and analysis for automotive fuel cell systems. J. Dyn. Sys. Meas. Control. 2004, 126, 14–25. [Google Scholar] [CrossRef]
- Wu, X.; Zhou, B. Fault tolerance control for proton exchange membrane fuel cell systems. J. Power Sources 2016, 324, 804–829. [Google Scholar] [CrossRef]
- De Bernardinis, A.; Candusso, D.; Harel, F.; Coquery, G. Power Electronics Interface for an Hybrid PEMFC Generating System with Fault Management Strategies for Transportation. In Proceedings of the European Conference on Power Electronics and Application, Barcelona, Spain, 8–10 September 2009. [Google Scholar]







| Parameters | Specifications |
|---|---|
| DC bus voltage Vbus | 80–200 V |
| Switching frequency, fs | 10 KHz |
| Inductors, L1, L2, L3, L4 | 440 μH |
| Boost capacitors CB1, CB2 | 47 μF /100 V |
| Input capacitor, Cin | 4.7 mF/400 V |
| Output capacitor, Co | 330 μF /400 V |
| , , | 1200, 6000, 21,000 |
| , , | 50, 450, 490 |
| PI parameters (voltage loop), Kp1, Ki1 | 0.4, 4.5 |
| PI parameters (current loop), Kp2, Ki2 | 0.5, 30 |
| Parameters | Specifications |
|---|---|
| Switching frequency, fs | 10 KHz |
| Inductors, L1, L2, L3, L4 | 440 μH |
| Boost capacitors CB1, CB2 | 47 μF/100 V |
| Input capacitor, Cin | 4.7 mF/400 V |
| Output capacitor, Co | 330 μF/400 V |
| Switch ‘S1′, ‘S2′ | IRFP260 (200 V, 50 A) |
| Diodes D1, D2, D3, D4, Do | RHRG3060 |
| Diodes Ds1, Ds2 | RHRG3060 |
| 500, 1750, 1600 | |
| 30, 270, 851 | |
| PI parameters (voltage loop), Kp1, Ki1 | 0.05, 0.5 |
| PI parameters (current loop), Kp2, Ki2 | 0.01, 10 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Smadi, A.A.; Khoucha, F.; Amirat, Y.; Benrabah, A.; Benbouzid, M. Active Disturbance Rejection Control of an Interleaved High Gain DC-DC Boost Converter for Fuel Cell Applications. Energies 2023, 16, 1019. https://doi.org/10.3390/en16031019
Smadi AA, Khoucha F, Amirat Y, Benrabah A, Benbouzid M. Active Disturbance Rejection Control of an Interleaved High Gain DC-DC Boost Converter for Fuel Cell Applications. Energies. 2023; 16(3):1019. https://doi.org/10.3390/en16031019
Chicago/Turabian StyleSmadi, Ahmed Abdelhak, Farid Khoucha, Yassine Amirat, Abdeldjabar Benrabah, and Mohamed Benbouzid. 2023. "Active Disturbance Rejection Control of an Interleaved High Gain DC-DC Boost Converter for Fuel Cell Applications" Energies 16, no. 3: 1019. https://doi.org/10.3390/en16031019
APA StyleSmadi, A. A., Khoucha, F., Amirat, Y., Benrabah, A., & Benbouzid, M. (2023). Active Disturbance Rejection Control of an Interleaved High Gain DC-DC Boost Converter for Fuel Cell Applications. Energies, 16(3), 1019. https://doi.org/10.3390/en16031019










