Start of Injection Influence on In-Cylinder Fuel Distribution, Engine Performance and Emission Characteristic in a RCCI Marine Engine
Abstract
:1. Introduction
2. Methodology
2.1. Experimental Setup
2.2. Model Initialization and Solver Setting
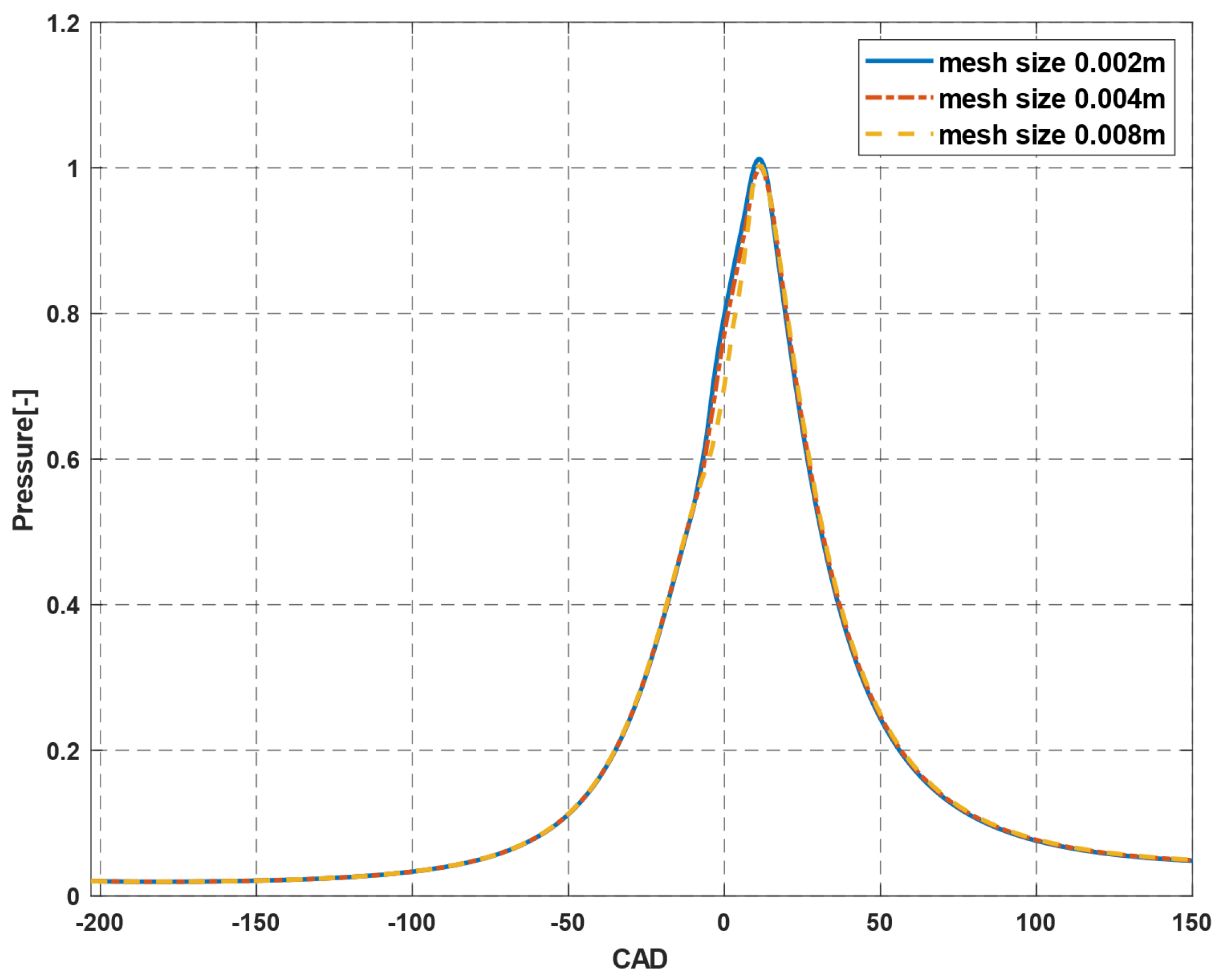
2.2.1. Geometry and Mesh Generation
2.2.2. Chemical Kinetic Mechanism and Liquid Fuel Properties
2.2.3. Combustion Modeling and Turbulence
2.3. Operating Points, Boundary Conditions and Initial Values
3. Results and Discussion
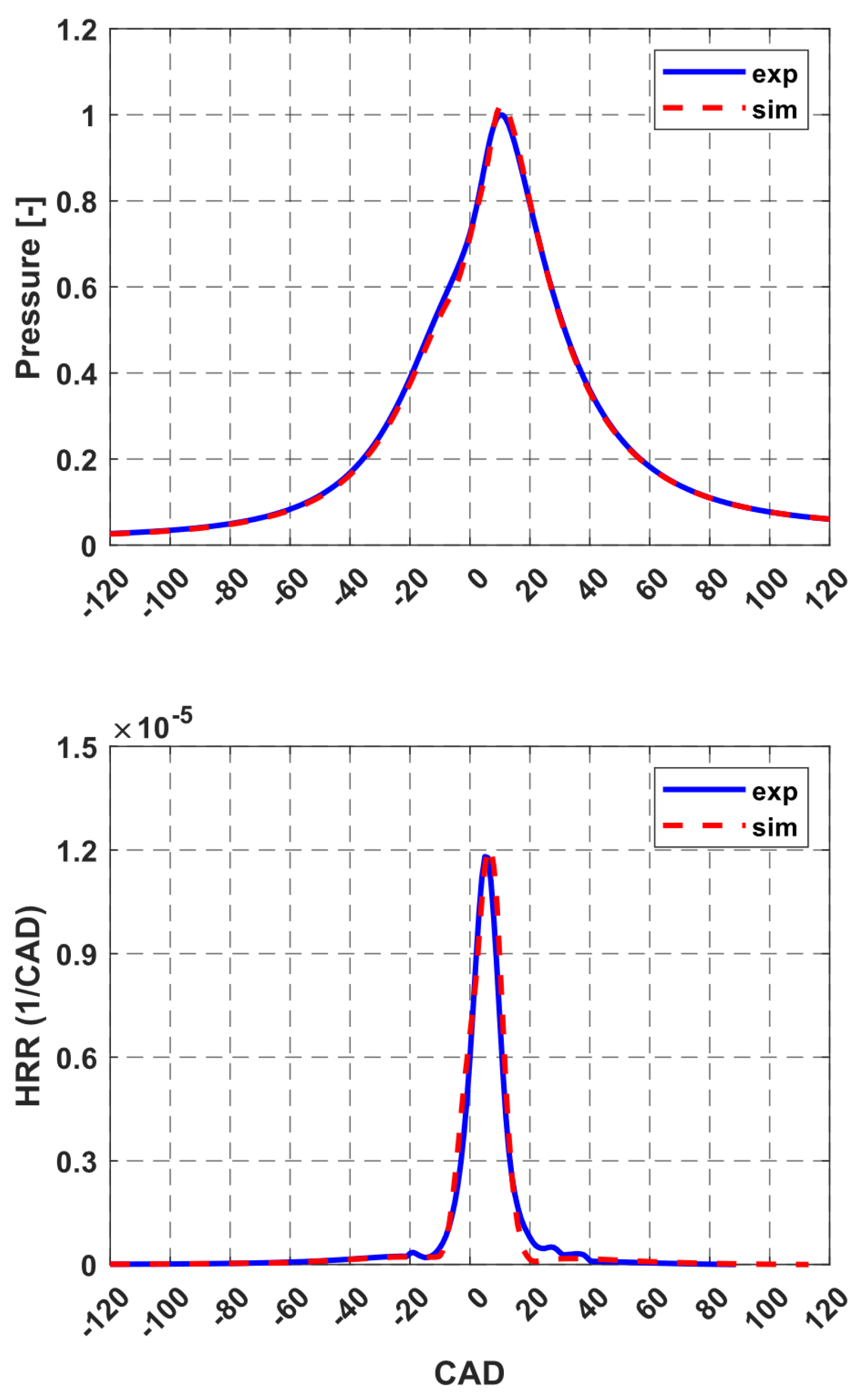
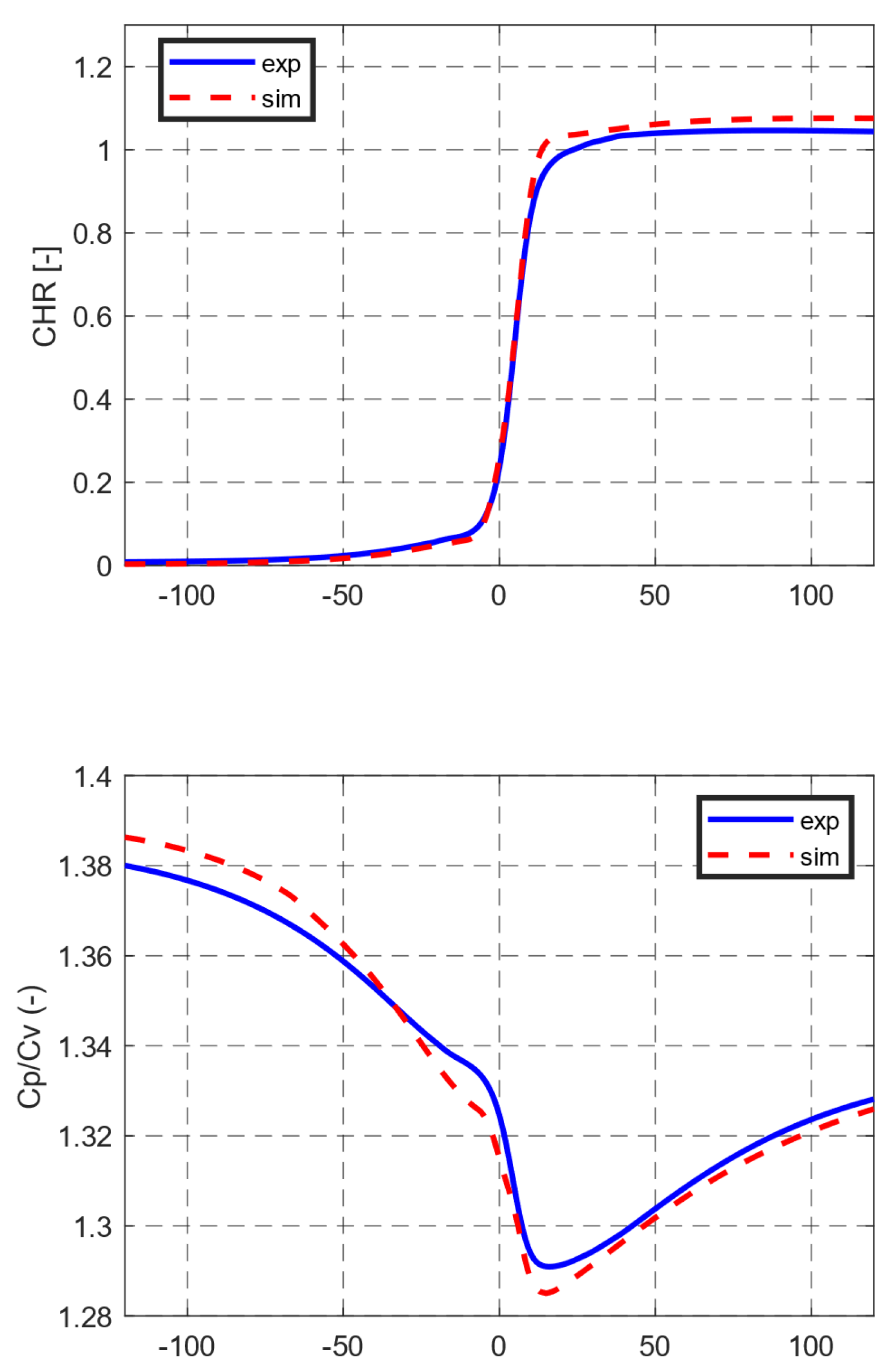
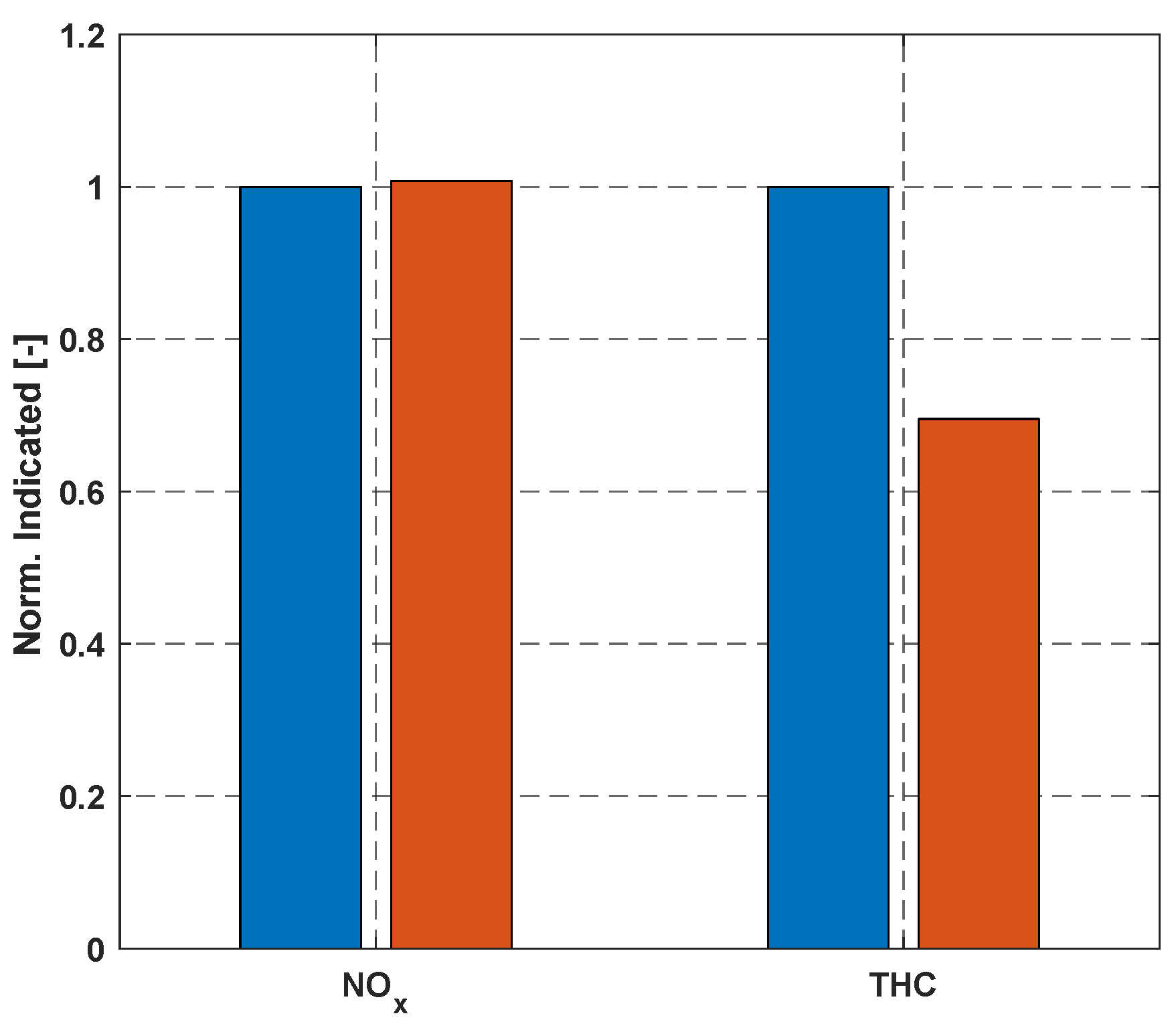
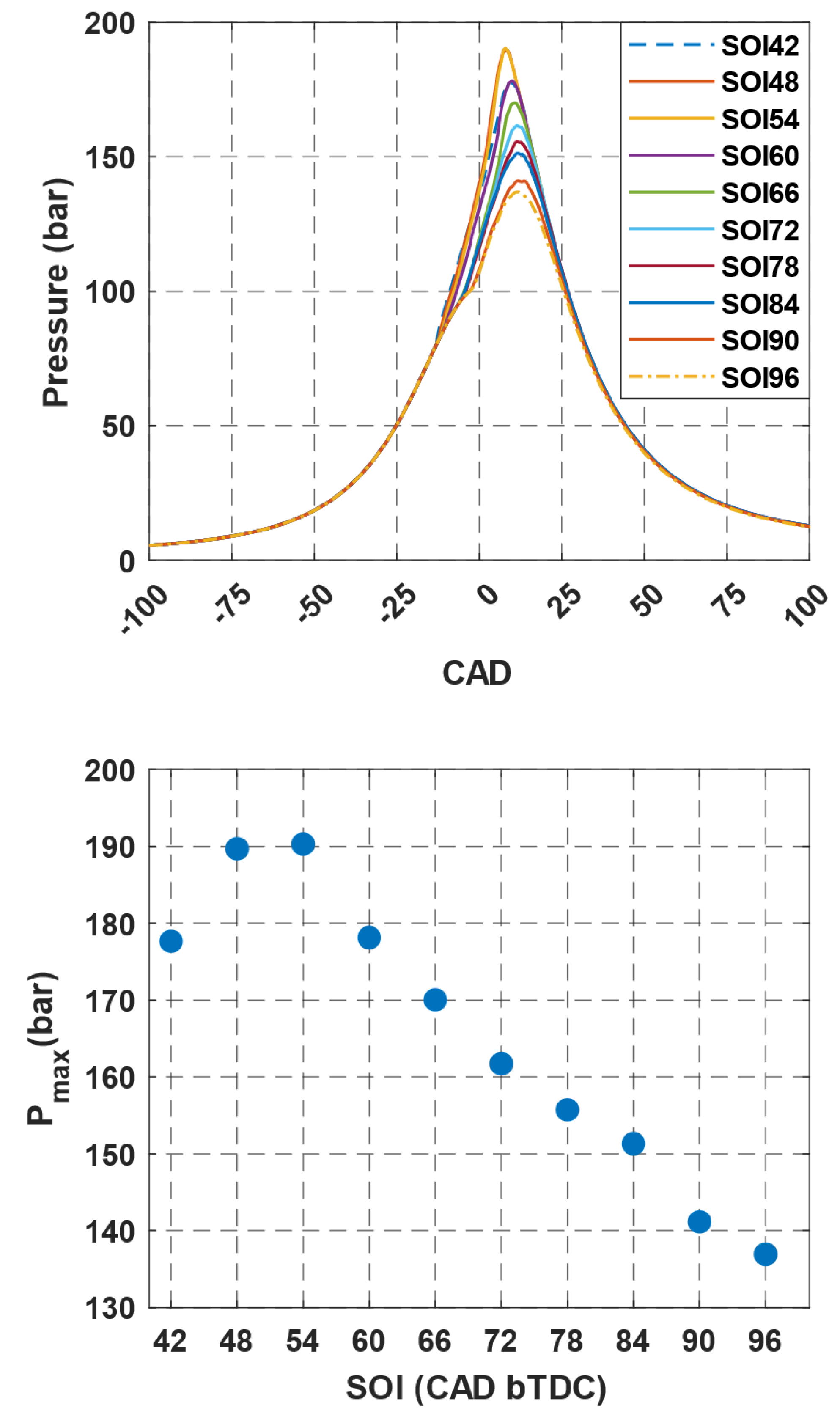
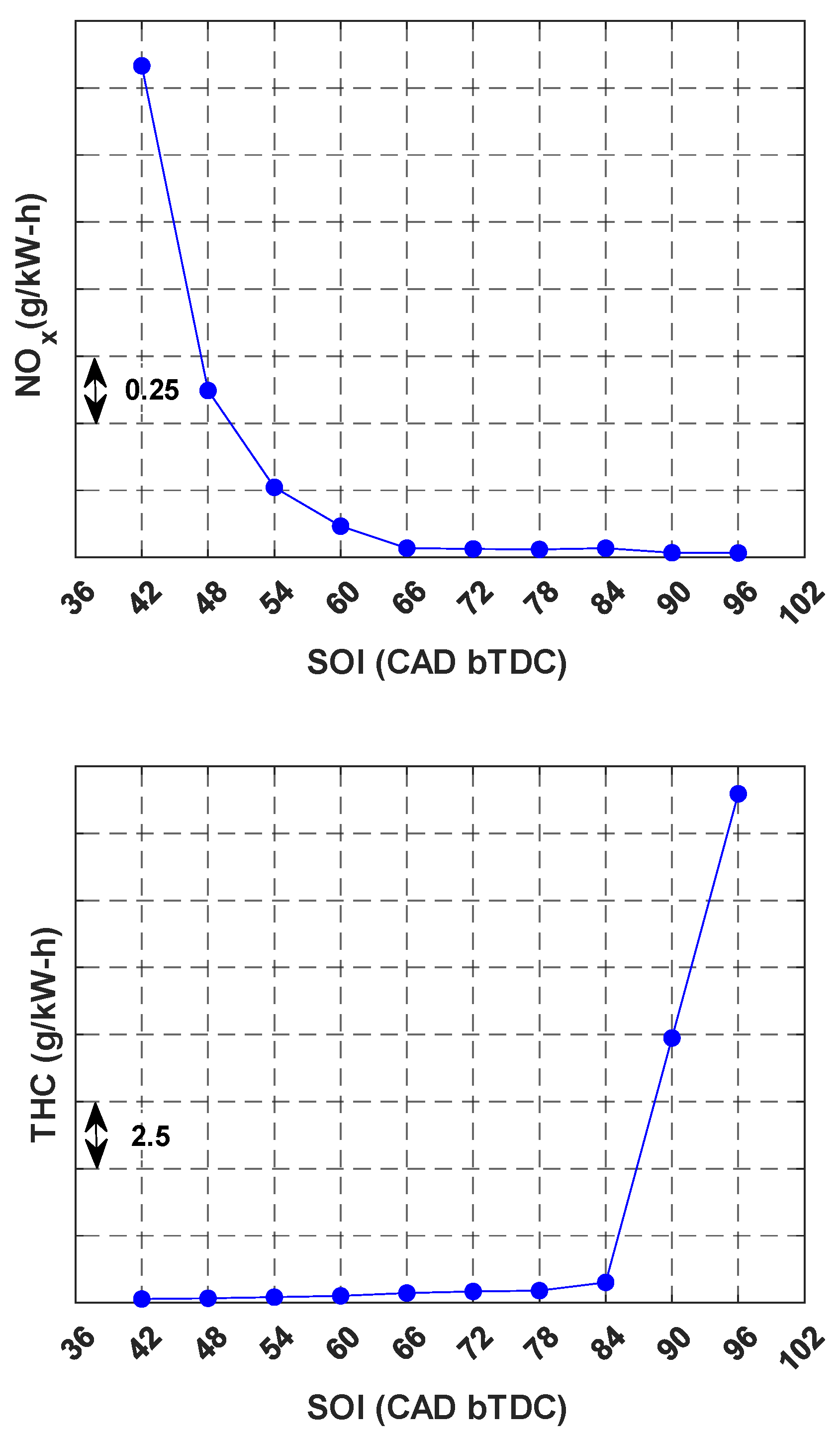
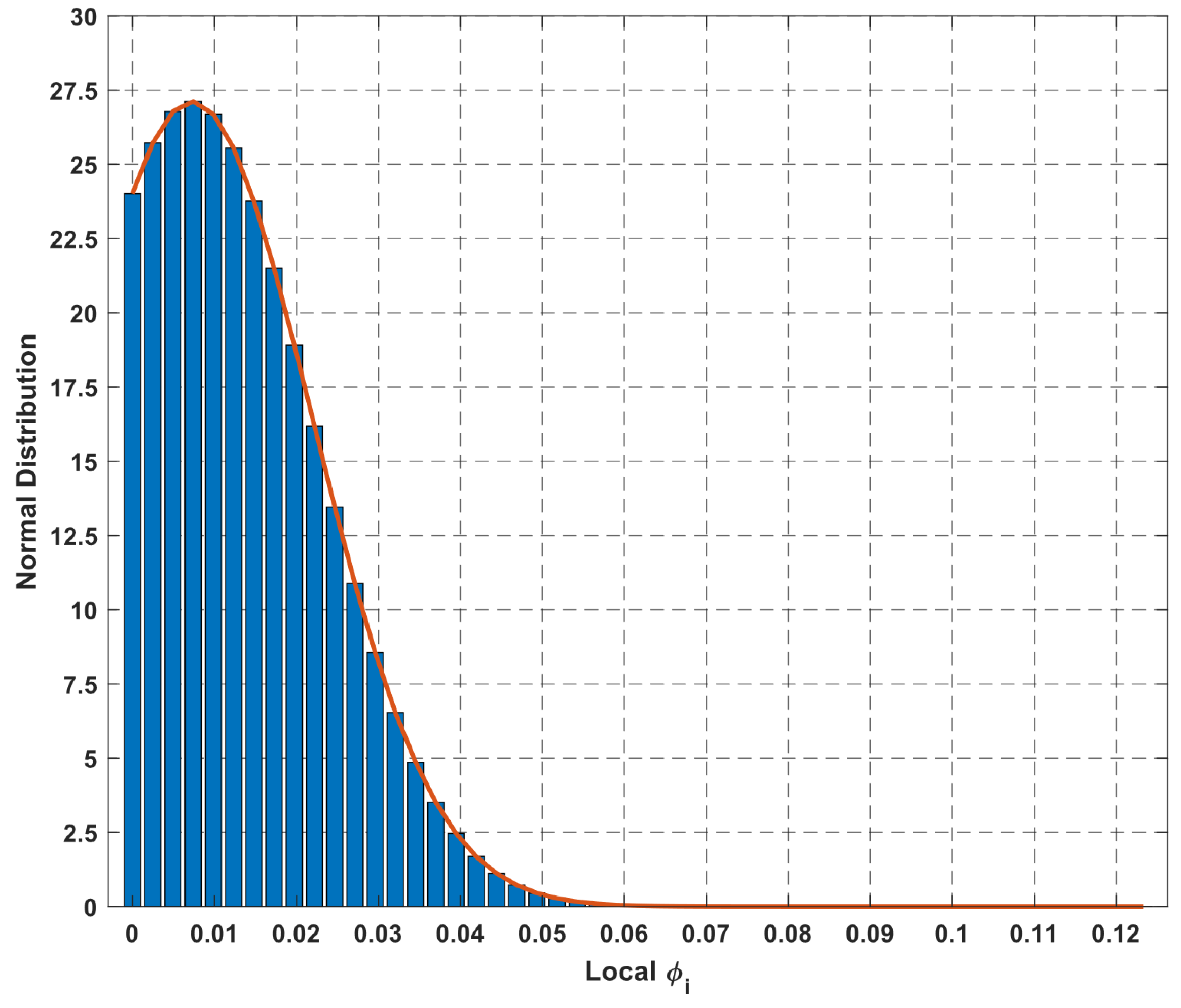
3.1. Model Validation and Results
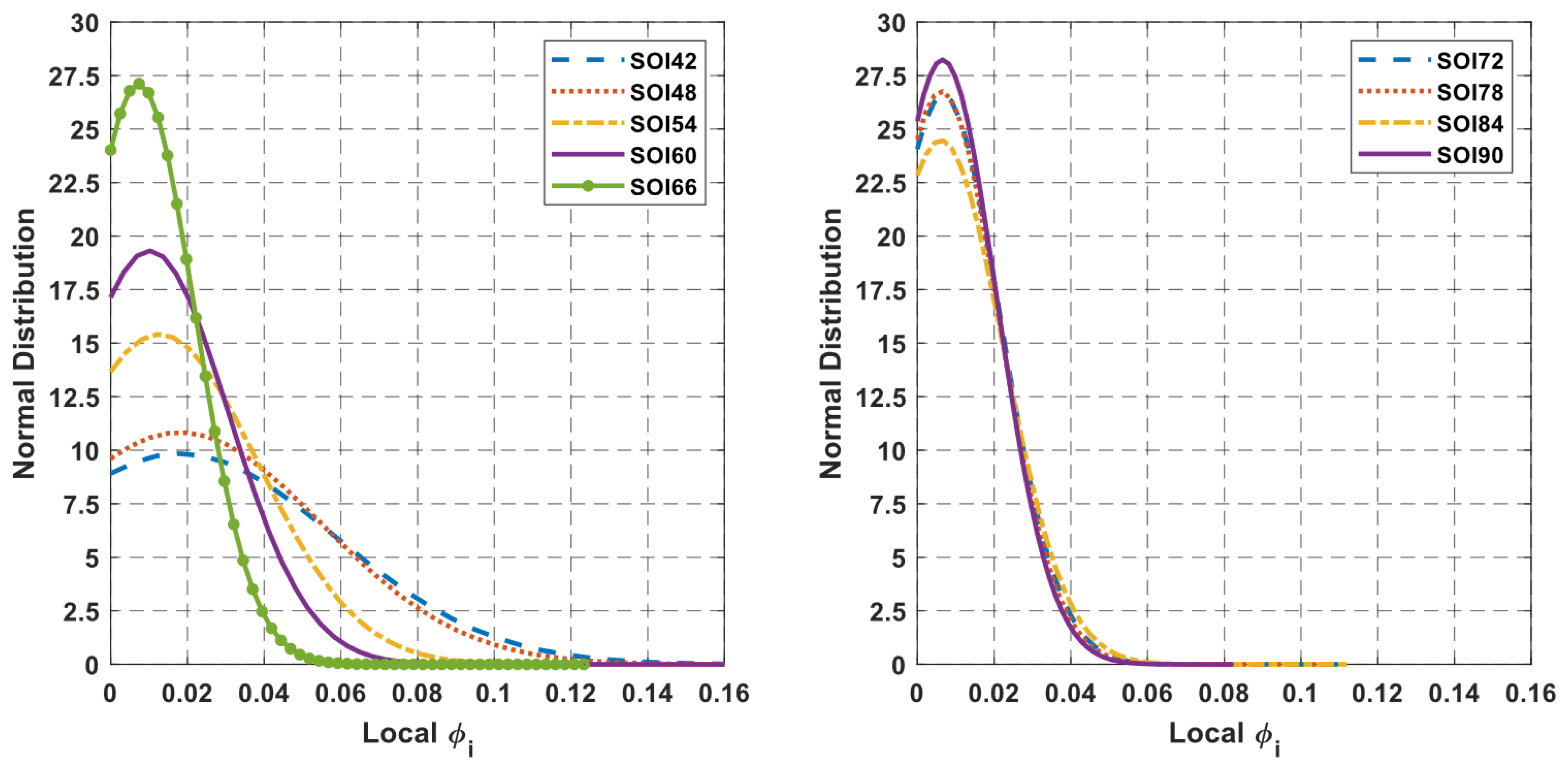
3.2. Injection Timing Effectiveness
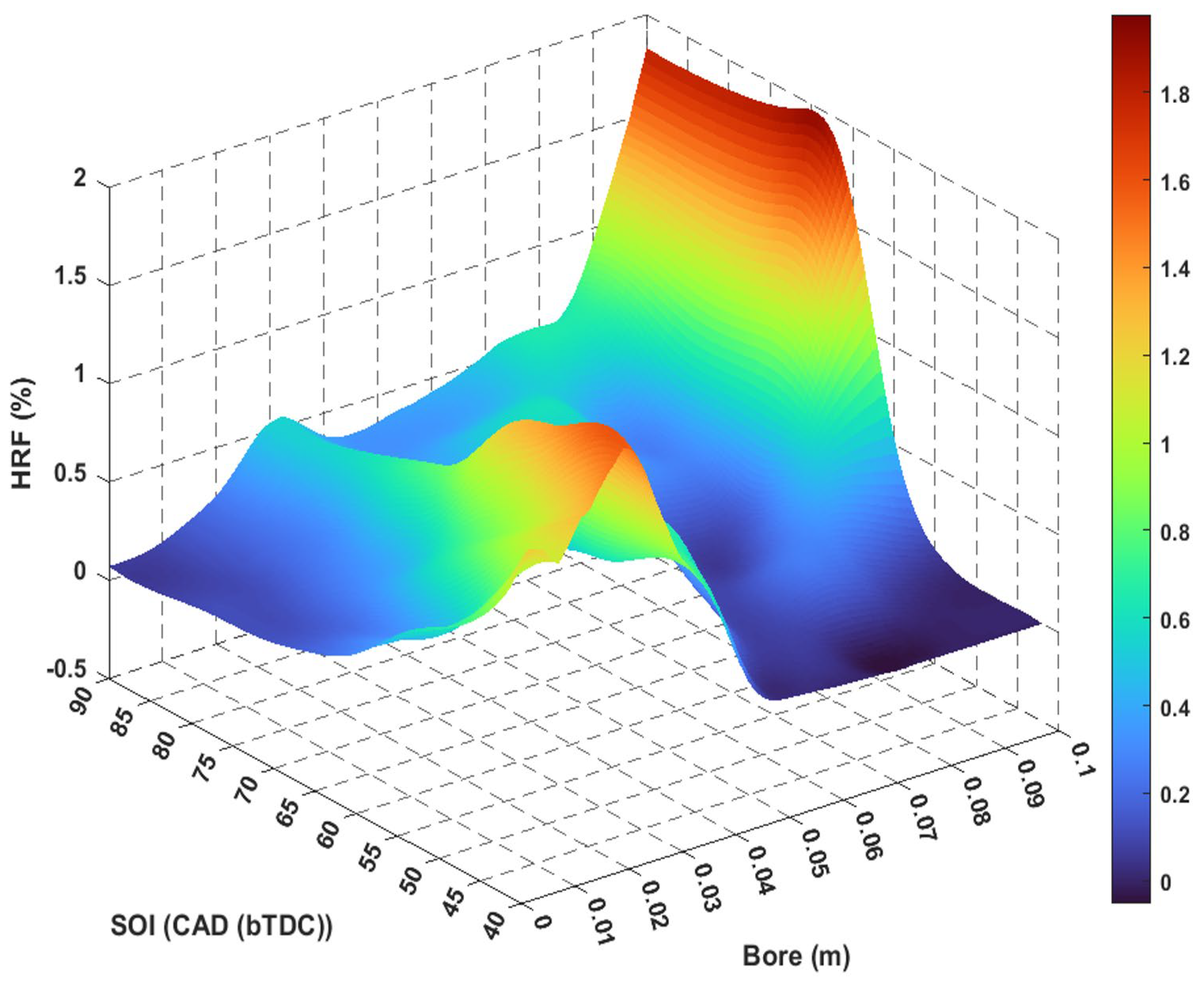
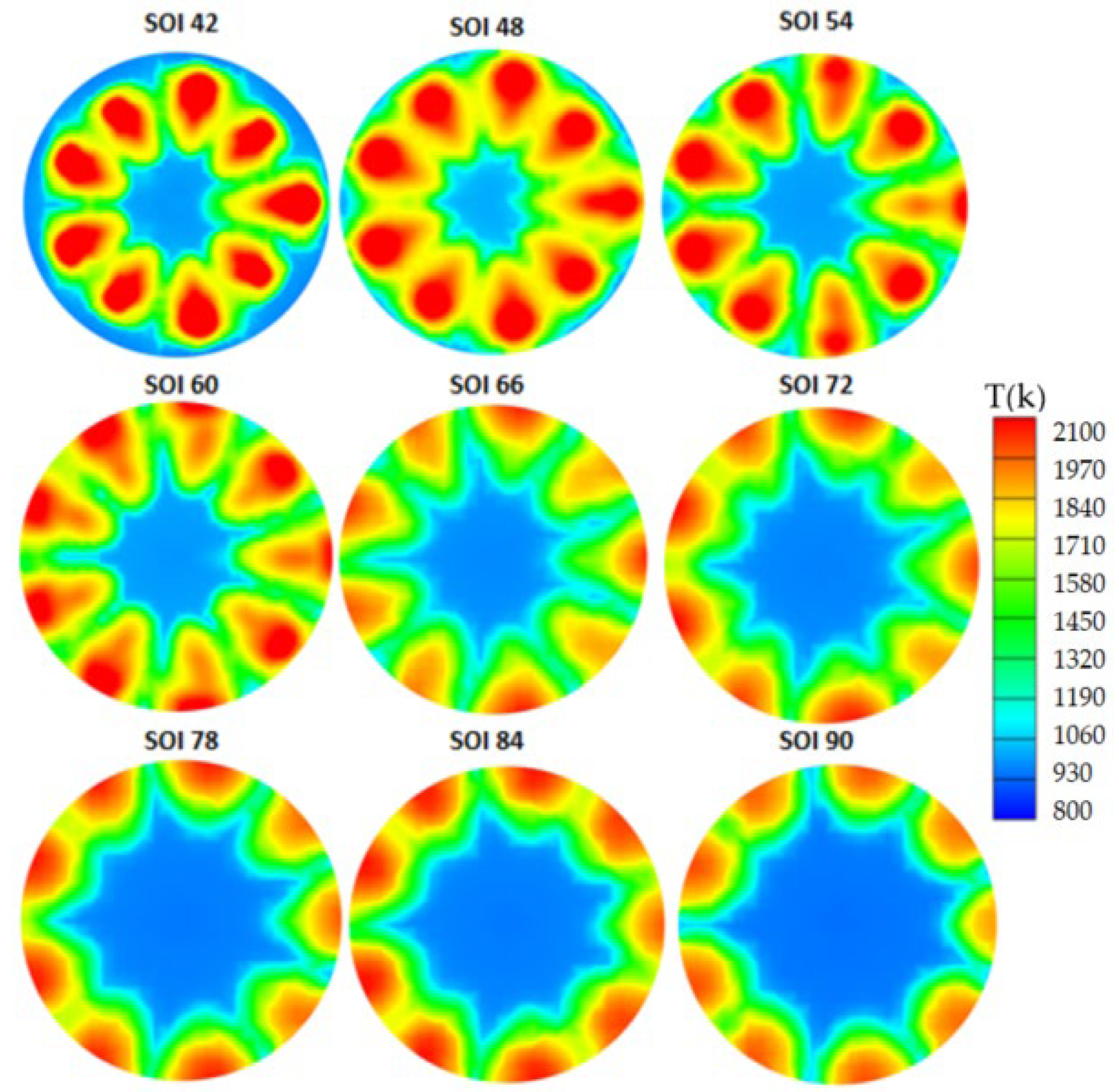
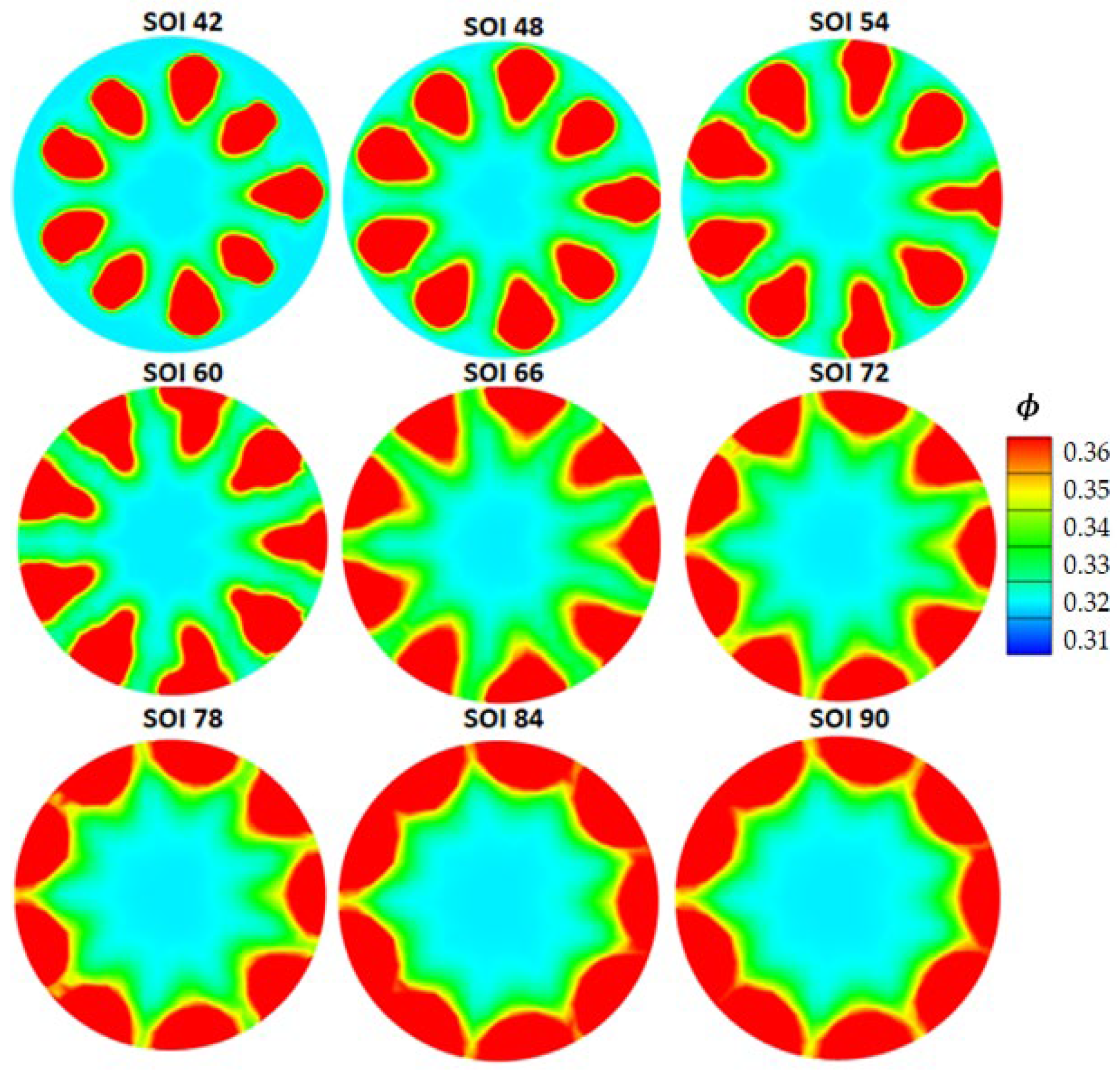
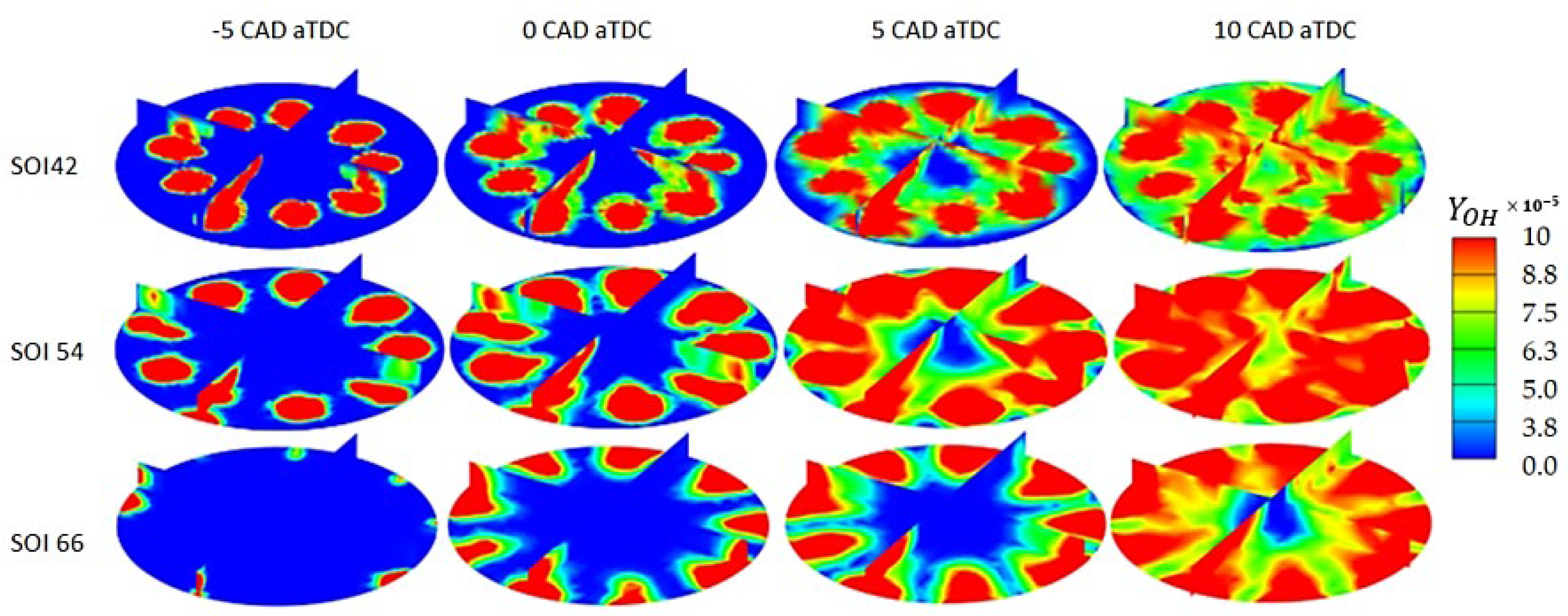
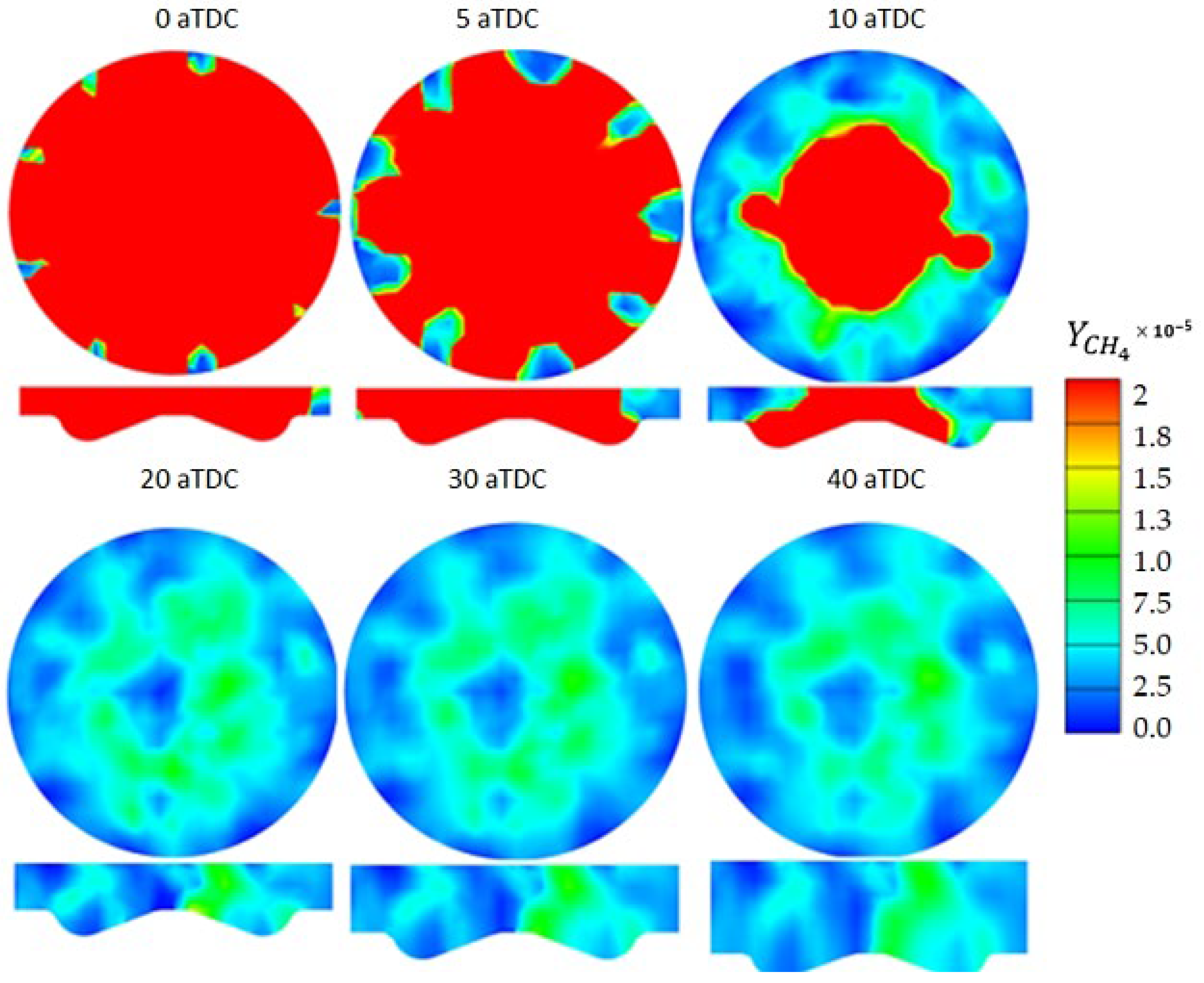
3.3. Flow and Species Visualization
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| RCCI | Reactivity Controlled Compression Ignition |
| NOx | Nitrogen Oxides |
| iNOx | indicated Nitrogen Oxides |
| CAD | Crank Angle Degrees |
| SOI | Start of Injection |
| CFD | Computational Fluid Dynamics |
| HCCI | Homogeneous Charge Compression Ignition |
| PM | Particulate Matter |
| HC | Hydrocarbon |
| CO | Carbon Monoxide |
| CO2 | Carbon Dioxide |
| IMEP | Indicated Mean Effective Pressure |
| CEM | Chemical Equilibrium Method |
| Probability Density Function | |
| IVC | Intake Valve Close |
| EGR | Exhaust Gas Recirculation |
| THC | Total Unburned Hydrocarbons |
| iTHC | indicated Total Unburned Hydrocarbons |
| RANS | Reynolds-Averaged Navier–Stokes |
| MFB | Mass Fraction Burned |
| HRF | High-Reactivity Fuel |
| CA50 | Crank Angle at 50% energy released |
| CA10 | Crank Angle at 10% energy released |
| CA90 | Crank Angle at 90% energy released |
| MZM | Multi-Zone Model |
| GT-Power | Engine Performance Simulation Software |
| RNG | Re-Normalization Group |
| O2 | Oxygen |
| N2 | Nitrogen |
| CAFE | Corporate Average Fuel Economy |
| COV | Coefficient of Variation |
| BD | Burn Duration |
| OH | Hydroxyl Radical |
| GRI | Gas Research Institute |
| LFO | Light Fuel Oil |
| SAGE | Semi-Analytical Green’s Function for Emission and Absorption |
| RIF | Radiation Interaction Factor |
| HOV | Heat of vaporization |
| RANS | Reynolds-averaged Navier-Stokes |
| RNG | Re-Normalization Group |
| TKE | Turbulent Kinetic Energy |
| KH | Kelvin–Helmholtz |
| RT | Rayleigh–Taylor |
References
- Li, J.; Yang, W.; Zhou, D. Review on the management of RCCI engines. Renew. Sustain. Energy Rev. 2017, 69, 65–79. [Google Scholar] [CrossRef]
- Reitz, R.D.; Duraisamy, G. Review of high efficiency and clean reactivity controlled compression ignition (RCCI) combustion in internal combustion engines. Prog. Energy Combust. Sci. 2015, 46, 12–71. [Google Scholar] [CrossRef]
- Benajes, J.; Novella, R.; Pastor, J.M.; Hernández-López, A.; Hasegawa, M.; Tsuji, N.; Emi, M.; Uehara, I.; Martorell, J.; Alonso, M. Optimization of the combustion system of a medium duty direct injection diesel engine by combining CFD modeling with experimental validation. Energy Convers. Manag. 2016, 110, 212–229. [Google Scholar] [CrossRef]
- Benajes, J.; Pastor, J.V.; García, A.; Boronat, V. A RCCI operational limits assessment in a medium duty compression ignition engine using an adapted compression ratio. Energy Convers. Manag. 2016, 126, 497–508. [Google Scholar] [CrossRef]
- Benajes, J.; García, A.; Pastor, J.M.; Monsalve-Serrano, J. Effects of piston bowl geometry on Reactivity Controlled Compression Ignition heat transfer and combustion losses at different engine loads. Energy 2016, 98, 64–77. [Google Scholar] [CrossRef]
- Liu, X.; Zhong, W.; Jiang, P.; Dai, L.; He, Z.; Wang, Q. Optimizing RCCI combustion for improved engine performance under low load conditions: Impact of low-reactivity fuel and direct injection timing. Fuel 2023, 351, 128871. [Google Scholar] [CrossRef]
- Wang, Y.; Zhu, Z.; Yao, M.; Li, T.; Zhang, W.; Zheng, Z. An investigation into the RCCI engine operation under low load and its achievable operational range at different engine speeds. Energy Convers. Manag. 2016, 124, 399–413. [Google Scholar] [CrossRef]
- Kakaee, A.-H.; Rahnama, P.; Paykani, A. Influence of fuel composition on combustion and emissions characteristics of natural gas/diesel RCCI engine. J. Nat. Gas Sci. Eng. 2015, 25, 58–65. [Google Scholar] [CrossRef]
- Molina, S.; García, A.; Pastor, J.M.; Belarte, E.; Balloul, I. Operating range extension of RCCI combustion concept from low to full load in a heavy-duty engine. Appl. Energy 2015, 143, 211–227. [Google Scholar] [CrossRef]
- Moradi, J.; Gharehghani, A.; Aghahasani, M. Application of machine learning to optimize the combustion characteristics of RCCI engine over wide load range. Fuel 2022, 324, 124494. [Google Scholar] [CrossRef]
- Loyte, A.; Suryawanshi, J.; Bhiogade, G.; Devarajan, Y.; Thandavamoorthy, R.; Mishra, R.; Natrayan, L. Influence of injection strategies on ignition patterns of RCCI combustion engine fuelled with hydrogen enriched natural gas. Environ. Res. 2023, 234, 116537. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Liu, J.; Ji, Q.; Sun, P.; Li, J.; Wei, M.; Liu, S. Experimental study on the high load extension of PODE/methanol RCCI combustion mode with optimized injection strategy. Fuel 2022, 314, 122726. [Google Scholar] [CrossRef]
- Bilgili, L. A systematic review on the acceptance of alternative marine fuels. Renew. Sustain. Energy Rev. 2023, 182, 113367. [Google Scholar] [CrossRef]
- Valera-Medina, A.; Xiao, H.; Owen-Jones, M.; David, W.I.F.; Bowen, P.J. Ammonia for power. Prog. Energy Combust. Sci. 2018, 69, 63–102. [Google Scholar] [CrossRef]
- Reiter, A.J.; Kong, S.-C. Combustion and emissions characteristics of compression-ignition engine using dual ammonia-diesel fuel. Fuel 2011, 90, 87–97. [Google Scholar] [CrossRef]
- Yousefi, A.; Guo, H.; Dev, S.; Liko, B.; Lafrance, S. Effects of ammonia energy fraction and diesel injection timing on combustion and emissions of an ammonia/diesel dual-fuel engine. Fuel 2022, 314, 122723. [Google Scholar] [CrossRef]
- Shen, Y.; Zhang, K.; Duwig, C. Investigation of wet ammonia combustion characteristics using LES with finite-rate chemistry. Fuel 2022, 311, 122422. [Google Scholar] [CrossRef]
- Ganesan, N.; Viswanathan, K.; Karthic, S.V.; Ekambaram, P.; Wu, W.; Vo, D.-V.N. Split injection strategies based RCCI combustion analysis with waste cooking oil biofuel and methanol in an open ECU assisted CRDI engine. Fuel 2022, 319, 123710. [Google Scholar] [CrossRef]
- Thomas, J.J.; Nagarajan, G.; Sabu, V.R.; Manojkumar, C.V.; Sharma, V. Performance and emissions of hexanol-biodiesel fuelled RCCI engine with double injection strategies. Energy 2022, 253, 124069. [Google Scholar] [CrossRef]
- Madihi, R.; Pourfallah, M.; Gholinia, M.; Armin, M.; Ghadi, A.Z. Thermofluids analysis of combustion, emissions, and energy in a biodiesel (C11H22O2)/natural gas heavy-duty engine with RCCI mode (Part I: Single/two-stage injection). Int. J. Thermofluids 2022, 16, 100227. [Google Scholar] [CrossRef]
- Madihi, R.; Pourfallah, M.; Gholinia, M.; Armin, M.; Ghadi, A.Z. Thermofluids analysis of combustion, emissions, and energy in a biodiesel (C11H22O2)/natural gas heavy-duty engine with RCCI mode (Part II: Fuel injection time/Fuel injection rate). Int. J. Thermofluids 2022, 16, 100200. [Google Scholar] [CrossRef]
- Li, J.; Yang, W.M.; Goh, T.N.; An, H.; Maghbouli, A. Study on RCCI (reactivity controlled compression ignition) engine by means of statistical experimental design. Energy 2014, 78, 777–787. [Google Scholar] [CrossRef]
- Li, J.; Yang, W.M.; An, H.; Zhao, D. Effects of fuel ratio and injection timing on gasoline/biodiesel fueled RCCI engine: A modeling study. Appl. Energy 2015, 155, 59–67. [Google Scholar] [CrossRef]
- Fakhari, A.H.; Gharehghani, A.; Salahi, M.M.; Mahmoudzadeh Andwari, A.; Mikulski, M.; Hunicz, J.; Könnö, J. Numerical Investigation of Ammonia-Diesel Fuelled Engine Operated in RCCI Mode. In Proceedings of the 16th International Conference on Engines & Vehicles, Capri, Italy, 10–14 September 2023. SAE Technical Paper 2023-24-0057. [Google Scholar]
- Ma, S.; Zheng, Z.; Liu, H.; Zhang, Q.; Yao, M. Experimental investigation of the effects of diesel injection strategy on gasoline/diesel dual-fuel combustion. Appl. Energy 2013, 109, 202–212. [Google Scholar] [CrossRef]
- Nazemi, M.; Shahbakhti, M. Modeling and analysis of fuel injection parameters for combustion and performance of an RCCI engine. Appl. Energy 2016, 165, 135–150. [Google Scholar] [CrossRef]
- Gharehghani, A.; Kakoee, A.; Andwari, A.M.; Megaritis, T.; Pesyridis, A. Numerical Investigation of an RCCI Engine Fueled with Natural Gas/Dimethyl-Ether in Various Injection Strategies. Energies 2021, 14, 1638. [Google Scholar] [CrossRef]
- Nemati, A.; Ong, J.C.; Pang, K.M.; Mayer, S.; Walther, J.H. A numerical study of the influence of pilot fuel injection timing on combustion and emission formation under two-stroke dual-fuel marine engine-like conditions. Fuel 2022, 312, 122651. [Google Scholar] [CrossRef]
- Yao, T.; Pei, Y.; Zhong, B.-J.; Som, S.; Lu, T.; Luo, K.H. A compact skeletal mechanism for n-dodecane with optimized semi-global low-temperature chemistry for diesel engine simulations. Fuel 2017, 191, 339–349. [Google Scholar] [CrossRef]
- Vasudev, A.; Mikulski, M.; Balakrishnan, P.R.; Storm, X.; Hunicz, J. Thermo-kinetic multi-zone modelling of low temperature combustion engines. Prog. Energy Combust. Sci. 2022, 91, 100998. [Google Scholar] [CrossRef]
- ISO 8217; FUEL STANDARD, Specifies the Requirements for Fuels for Use in Marine Diesel Engines and Boilers, Miami, FL, USA. 2017. Available online: https://www.iso.org/obp/ui/en/#iso:std:iso:8217:ed-6:v1:en (accessed on 8 May 2024).
- Vasudev, A.; Cafari, A.; Axelsson, M.; Mikulski, M.; Hyvonen, J. Towards Next Generation Control-Oriented Thermo-Kinetic Model for Reactivity Controlled Compression Ignition Marine Engines. In SAE Powertrains, Fuels & Lubricants Conference & Exhibition; SAE Technical Paper 2022-01-1033; SAE: Warrendale, PA, USA, 2022. [Google Scholar]
- Kakoee, A.; Vasudev, A.; Smulter, B.; Hyvonen, J.; Mikulski, M. A Predictive 1D Modeling Framework for Reactivity-Controlled Compression Ignition Engines, via a Chemistry-Based, Multizone Combustion Object. In Proceedings of the 16th International Conference on Engines & Vehicles, Capri, Italy, 10–14 September 2023; SAE International: Capri, Italy, 2023; p. 11. [Google Scholar]
- Heywood, J.B. Internal Combustion Engine Fundamentals; McGraw Hill: New York, NY, USA, 1988. [Google Scholar]
- Convergent Science; Version 3.1; Converge Manual; Convergent Science: Madison, WI, USA, 2023.
- Jafari, B.; Seddiq, M.; Mirsalim, S.M. Impacts of diesel injection timing and syngas fuel composition in a heavy-duty RCCI engine. Energy Convers. Manag. 2021, 247, 114759. [Google Scholar] [CrossRef]
- Ramesh, N.; Mallikarjuna, J.M. Evaluation of in-cylinder mixture homogeneity in a diesel HCCI engine—A CFD analysis. Eng. Sci. Technol. Int. J. 2016, 19, 917–925. [Google Scholar] [CrossRef]
- Kang, Y.; Li, X.; Shen, H.; Chen, Y.; Liu, D.; Chang, J. Effects of combustion chamber diameter on the performance and fuel–air mixing of a double swirl combustion system in a diesel engine. Fuel 2022, 324, 124392. [Google Scholar] [CrossRef]
- Singh, A.P.; Kumar, V.; Agarwal, A.K. Evaluation of comparative engine combustion, performance and emission characteristics of low temperature combustion (PCCI and RCCI) modes. Appl. Energy 2020, 278, 115644. [Google Scholar] [CrossRef]
- Žvar Baškovič, U.; Vihar, R.; Rodman Oprešnik, S.; Seljak, T.; Katrašnik, T. RCCI combustion with renewable fuel mix—Tailoring operating parameters to minimize exhaust emissions. Fuel 2022, 311, 122590. [Google Scholar] [CrossRef]

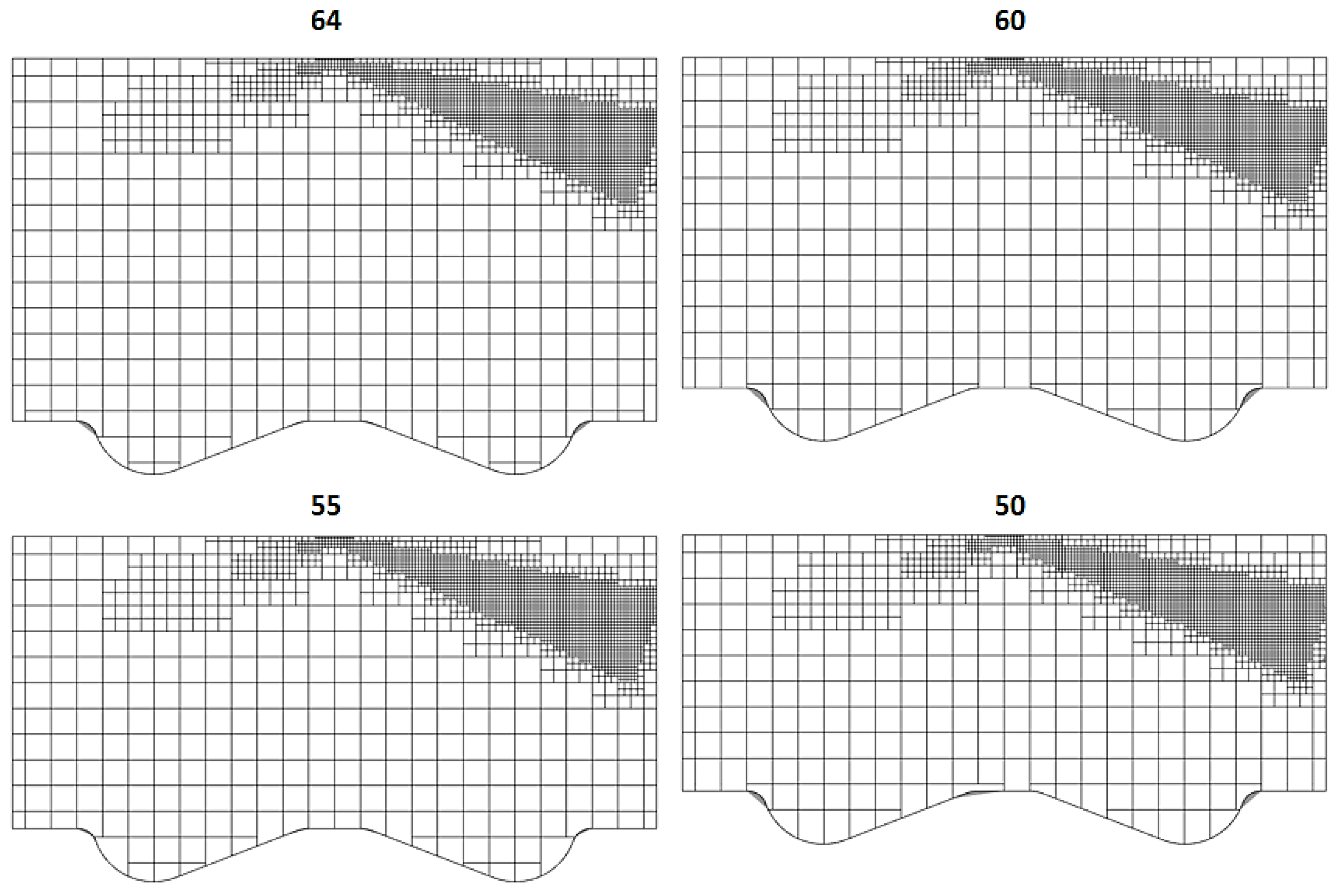



| Displacement/Nominal Speed | 8.80 L/1000 rpm |
| Stroke/Bore | 1.4 |
| Air System | Two-stage turbocharged (in series) |
| High reactivity fuel system | Common rail |
| Low reactivity fuel system | Low-pressure; multi-point, upstream of the intake valve |
| Valve train | 4 valves per cylinder, fully variable hydraulic valve train |
| Emission System | Horiba Mexa-One (NOx, CO, THC, CO2, O2) AVL415S (FSN-Soot) |
| Indicative System | AVL Indicom, Cylinder pressure tranducer Kistler 6124 A, 300 bar range, 30 pC/bar sensitivity.per |
| Engine Control system | Speed Goat/CanApe Rapid control protyping platfrom |
| Test fuels | ISO 8217 [31] compliant LFO/LNG (MN = 80) |
| Specifications/Case | B (Mid Load) | |
|---|---|---|
| Operating Points | Speed (rpm) | 1000 |
| Injected mass (mg) | ref − 28 | |
| SOI (CAD bTDC) | ref + 31 | |
| Injection duration (CAD) | 2.5 | |
| Boundary Conditions | T_piston (k) | ref − 84 |
| T_Head (k) | ref − 15 | |
| T_liner (k) | ref − 5 | |
| T_wall_exh_port | ref − 3.4 | |
| T_wall_int_port | ref − 2.4 | |
| P_exh_port | ref + 1.6 | |
| P_int_port | ref + 1.6 | |
| T_exh_port | ref − 31.1 | |
| T_int_port | ref + 6.5 | |
| Initial Values | Tivc (k) | ref + 14.8 |
| Pivc (bar) | ref + 1.92 | |
| Y_N2 | 0.7531 | |
| Y_O2 | 0.2288 | |
| Y_CH4 | 0.0168 | |
| Y_C2H6 | 0.0013 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kakoee, A.; Mikulski, M.; Vasudev, A.; Axelsson, M.; Hyvönen, J.; Salahi, M.M.; Mahmoudzadeh Andwari, A. Start of Injection Influence on In-Cylinder Fuel Distribution, Engine Performance and Emission Characteristic in a RCCI Marine Engine. Energies 2024, 17, 2370. https://doi.org/10.3390/en17102370
Kakoee A, Mikulski M, Vasudev A, Axelsson M, Hyvönen J, Salahi MM, Mahmoudzadeh Andwari A. Start of Injection Influence on In-Cylinder Fuel Distribution, Engine Performance and Emission Characteristic in a RCCI Marine Engine. Energies. 2024; 17(10):2370. https://doi.org/10.3390/en17102370
Chicago/Turabian StyleKakoee, Alireza, Maciej Mikulski, Aneesh Vasudev, Martin Axelsson, Jari Hyvönen, Mohammad Mahdi Salahi, and Amin Mahmoudzadeh Andwari. 2024. "Start of Injection Influence on In-Cylinder Fuel Distribution, Engine Performance and Emission Characteristic in a RCCI Marine Engine" Energies 17, no. 10: 2370. https://doi.org/10.3390/en17102370
APA StyleKakoee, A., Mikulski, M., Vasudev, A., Axelsson, M., Hyvönen, J., Salahi, M. M., & Mahmoudzadeh Andwari, A. (2024). Start of Injection Influence on In-Cylinder Fuel Distribution, Engine Performance and Emission Characteristic in a RCCI Marine Engine. Energies, 17(10), 2370. https://doi.org/10.3390/en17102370






