Multiphase LLC DC-Link Converter with Current Equalization Based on CM Voltage-Controlled Capacitor
Abstract
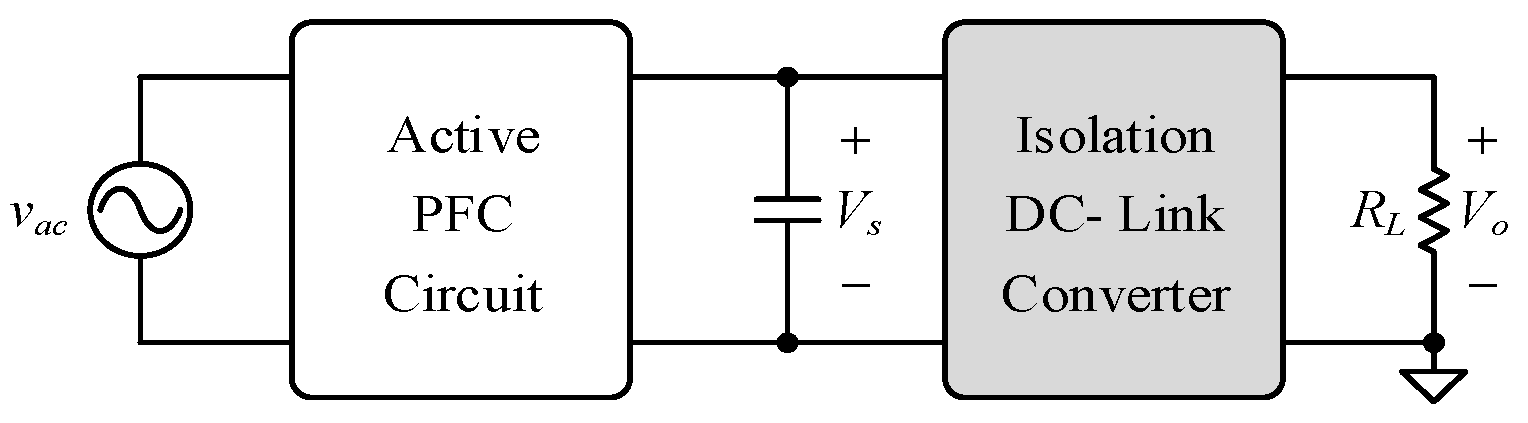
1. Introduction
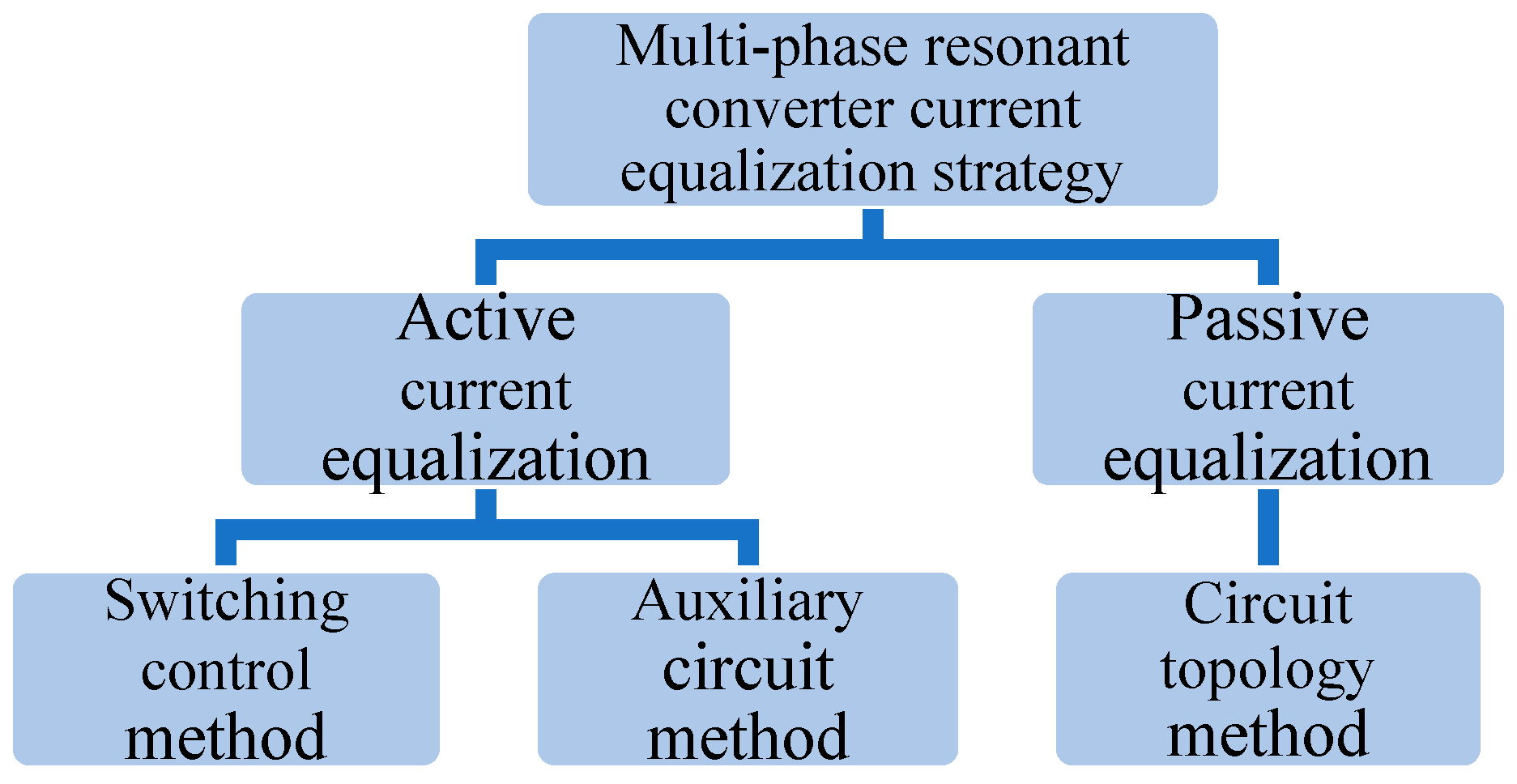
2. Principle of Current Equalization Based on a Variable Capacitor
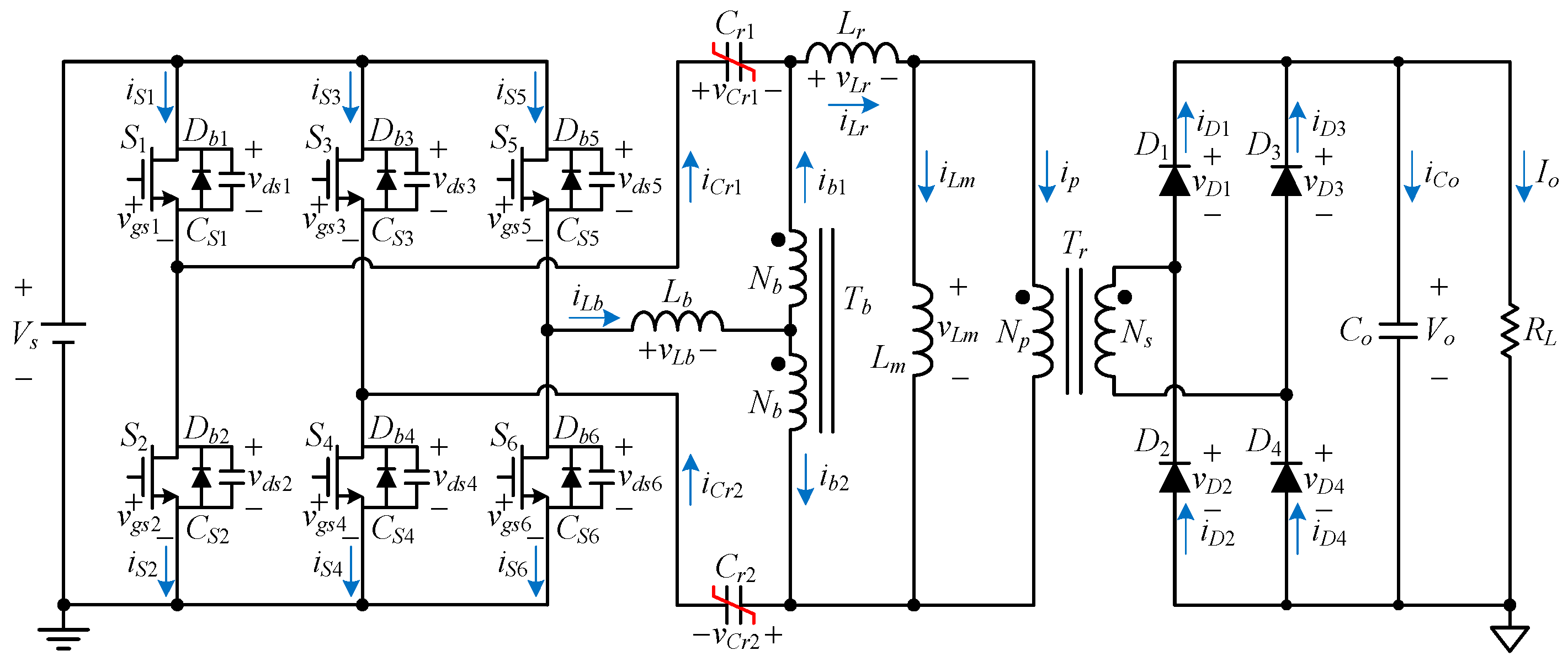
3. Common-Mode Bias Variable Capacitance Structure
3.1. Operating Principle
- (1)
- The full-bridge output voltage is regarded as ideal and is expressed in terms of two square wave voltage sources vx and vy with a duty cycle of 50%, and vx′ and vy′ follow tightly after Cr1 and Cr2;
- (2)
- The center-tapped transformer Tb is viewed as ideal, and the effect of magnetizing inductance is not considered;
- (3)
- The center terminal of this transformer Tb is referred to as the bias node, and its potential is indicated by vb;
- (4)
- The values of two ferroelectric resonance capacitors Cr1 and Cr2 can be expressed as functions of their trans-voltages, namely Cr (vCr1) and Cr (vCr2), respectively;
- (5)
- The equivalent resistance of the output load on the primary side of the transformer is expressed as Req.
3.2. Bias Operation Region
3.3. Ferroelectric Dielectric MLCCs
3.4. Proposed Common-Mode Bias Variable Capacitance Technique
- (1)
- The bias-controlled variable capacitor technique utilizes a high-permittivity MLCC, resulting in a small size for the resonant capacitor;
- (2)
- The bias circuit only supplies the resonant capacitor current in the steady state, such that the power required is quite low;
- (3)
- The common-mode bias structure can be constructed by a non-isolated auxiliary circuit;
- (4)
- The voltage ripple of the bias node is small, and this is favorable for the application of the switching bias circuit;
- (5)
- The application of the switching technology to bias circuits can significantly extend the bias operating range;
- (6)
- The common-mode bias structure with the switching circuit removes the need for additional filter capacitors;
- (7)
- The low dependency between the bias circuit and the main circuit allows each to be regulated independently;
- (8)
- All the switching elements, including the bias circuit, can achieve ZVS turn-on.
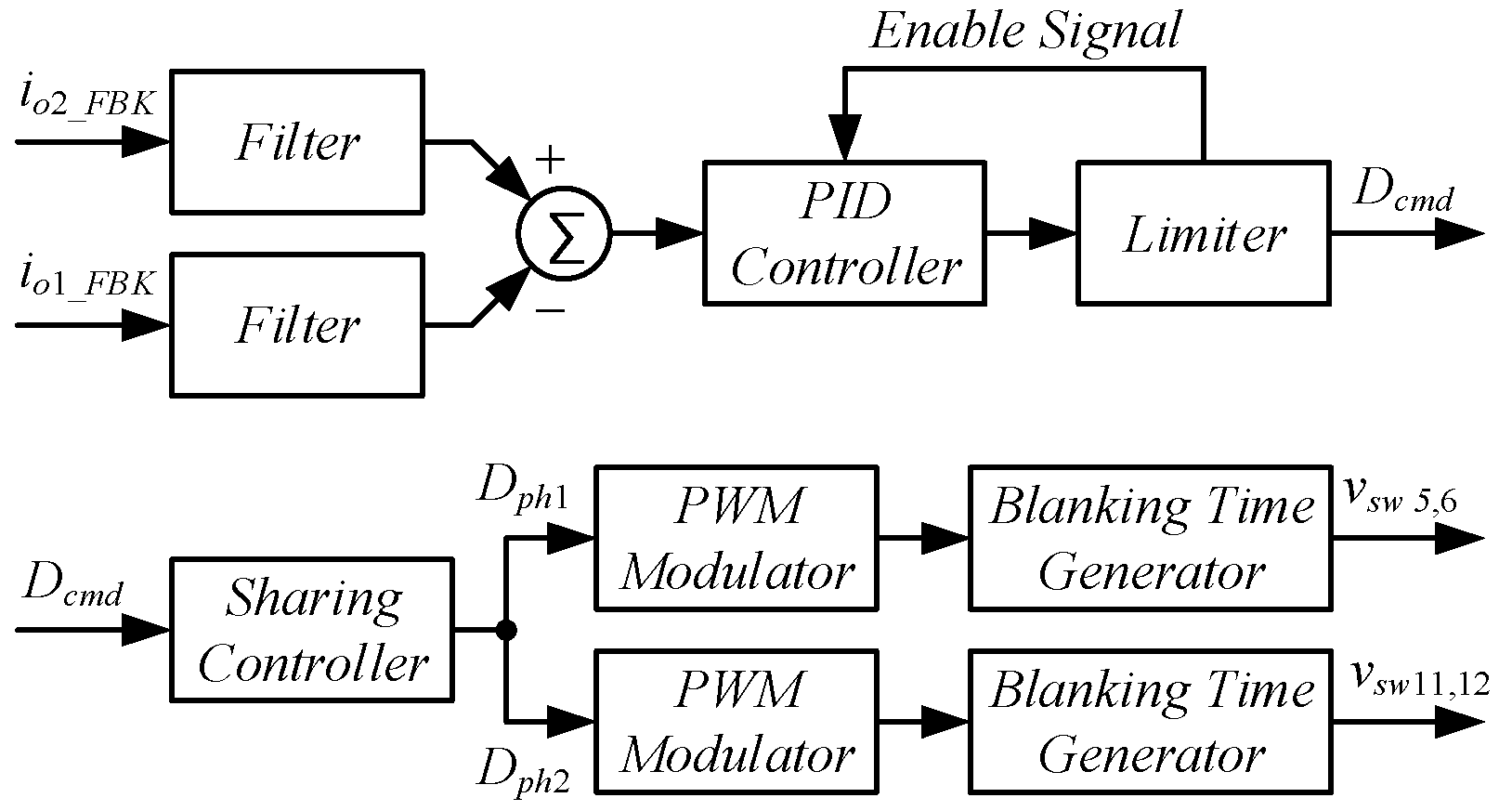
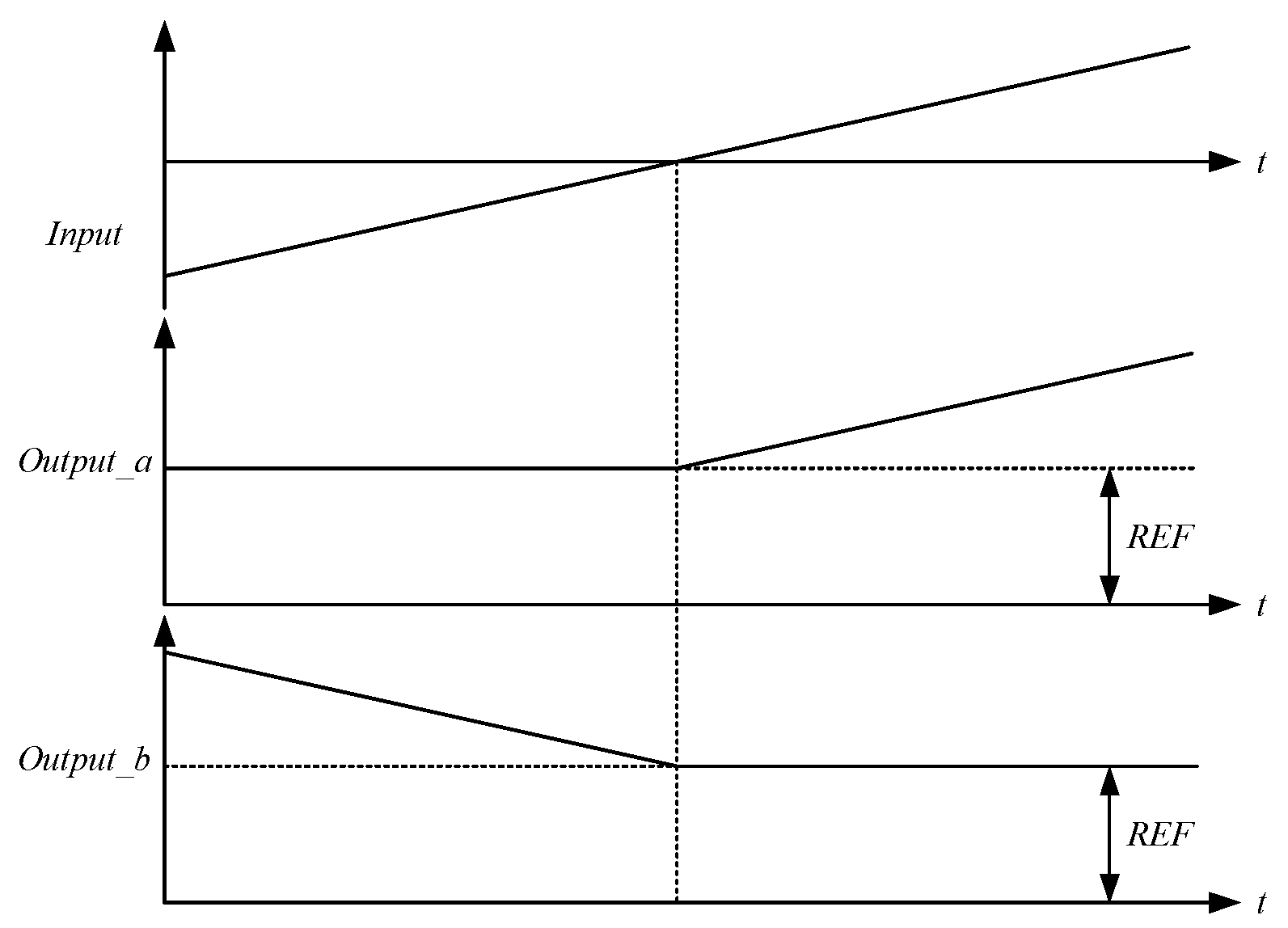
4. Current-Equalization Control
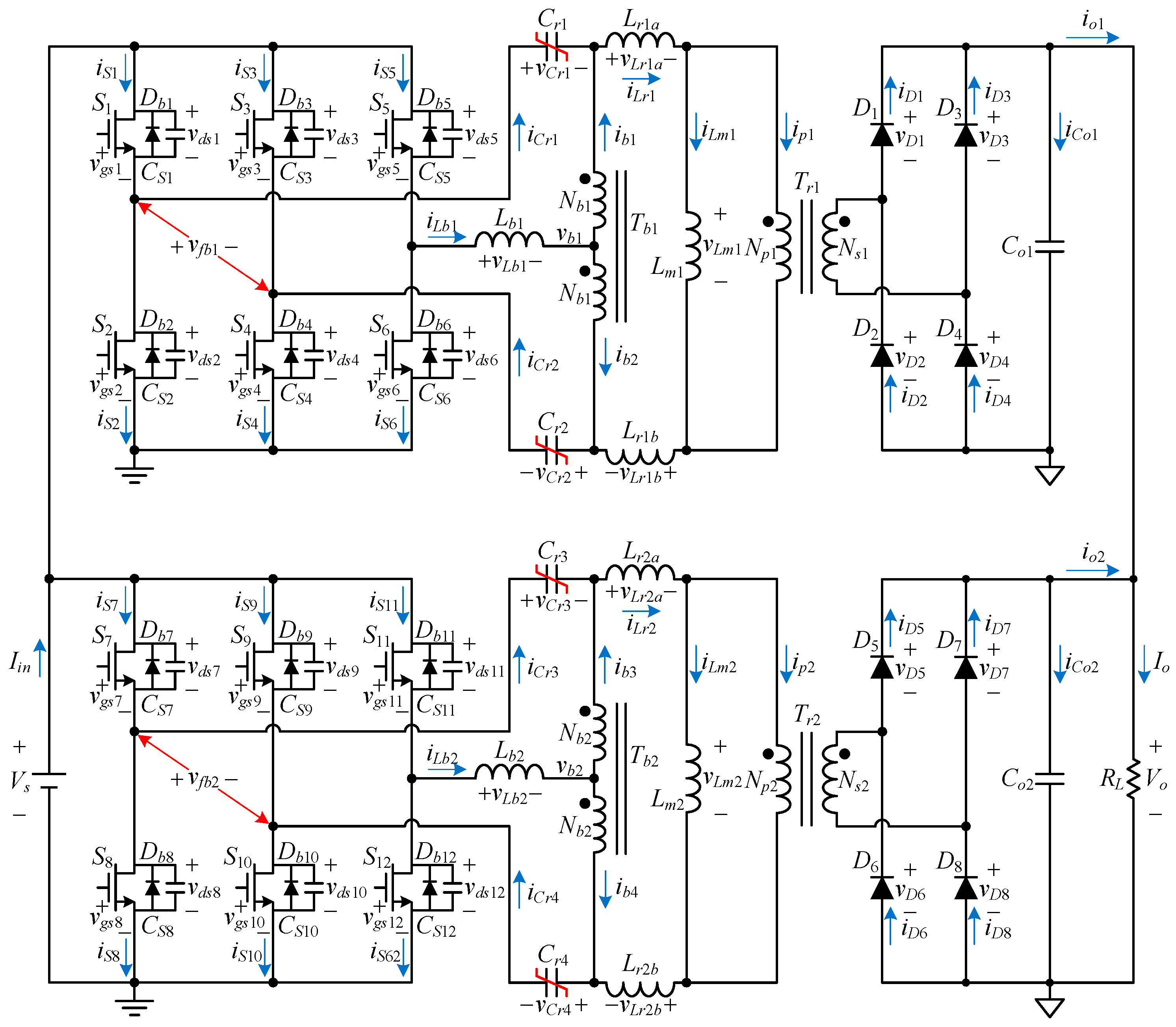
4.1. Main Power Stage
4.2. Switching Signals for Current Equalization Operation and Full-Bridge Operation
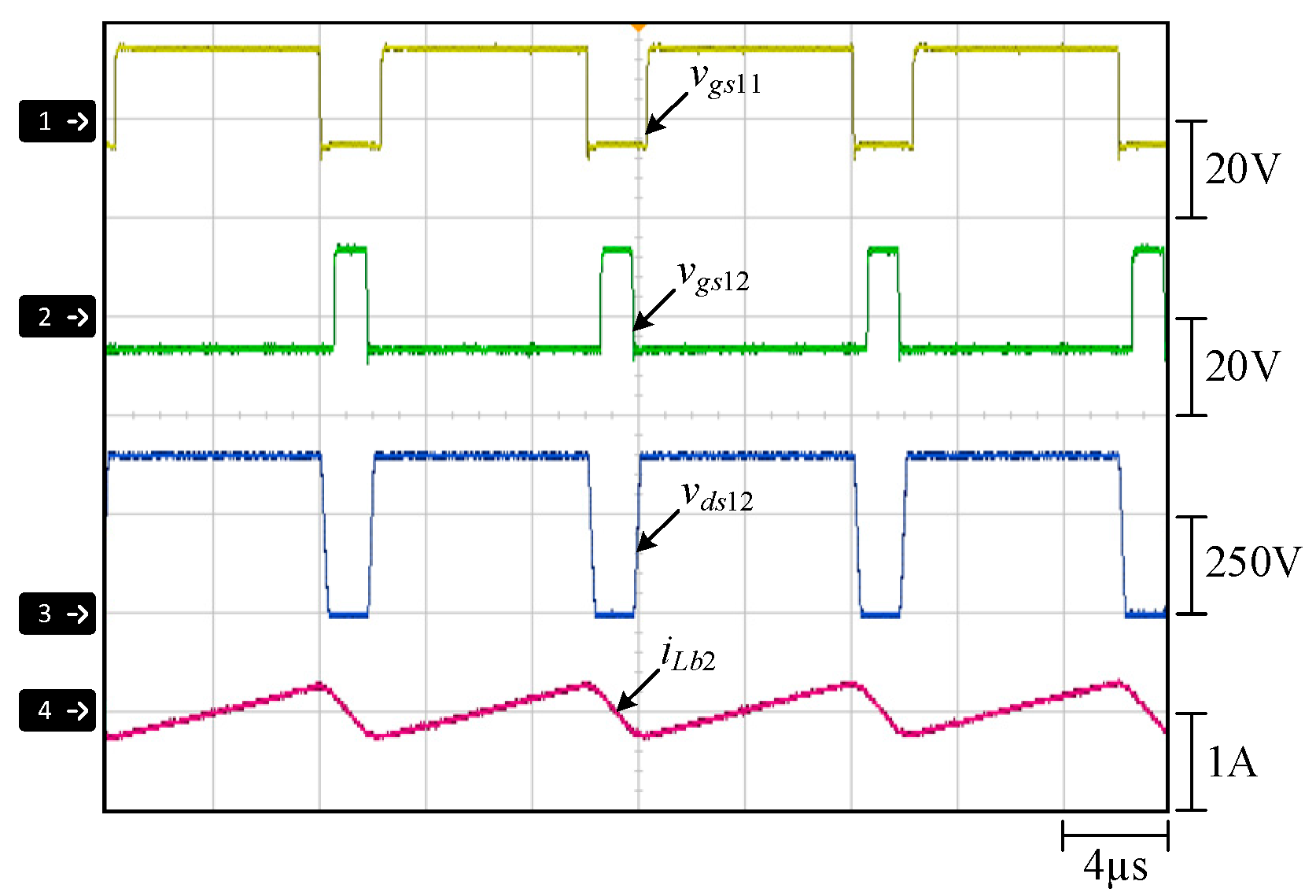
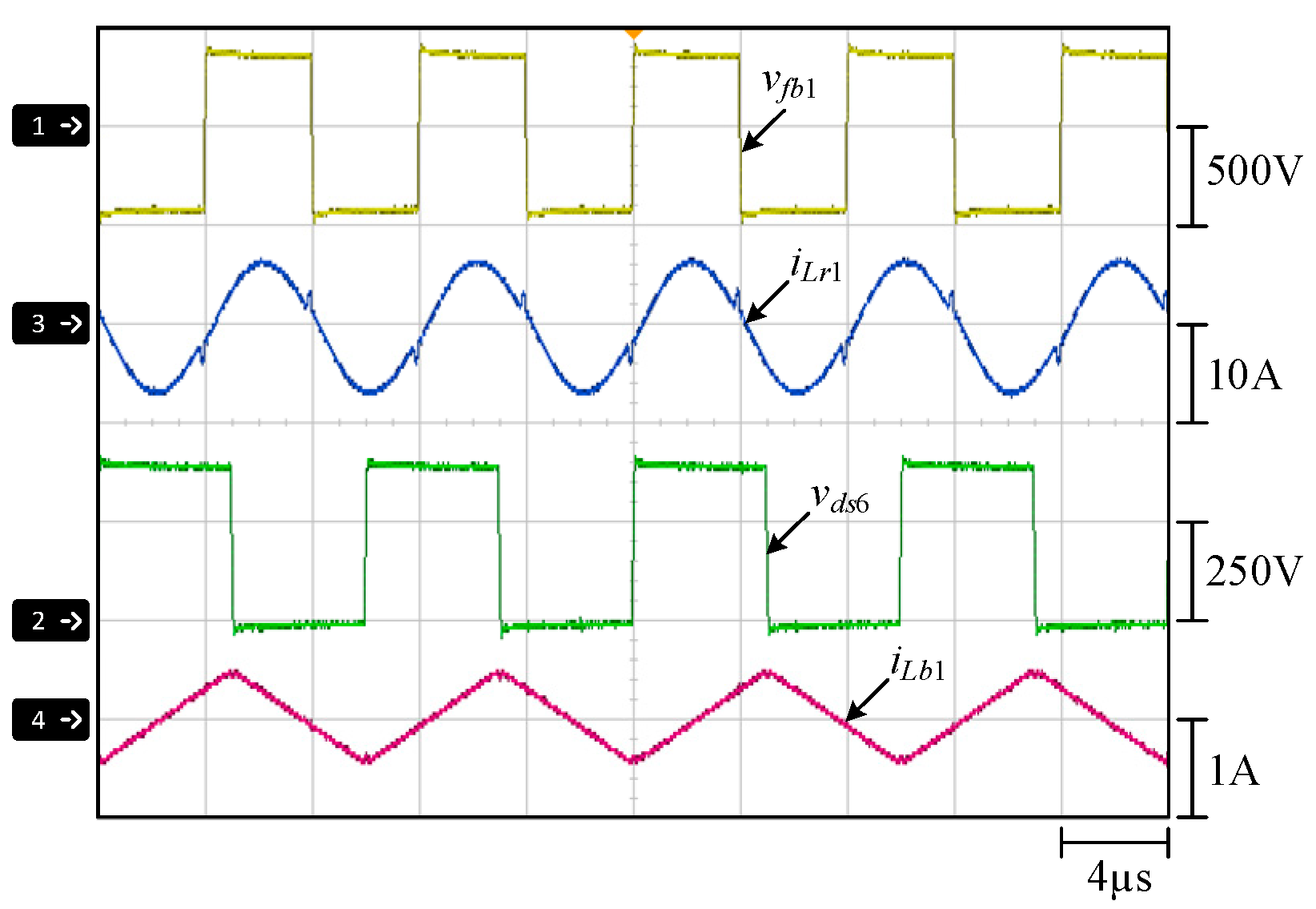
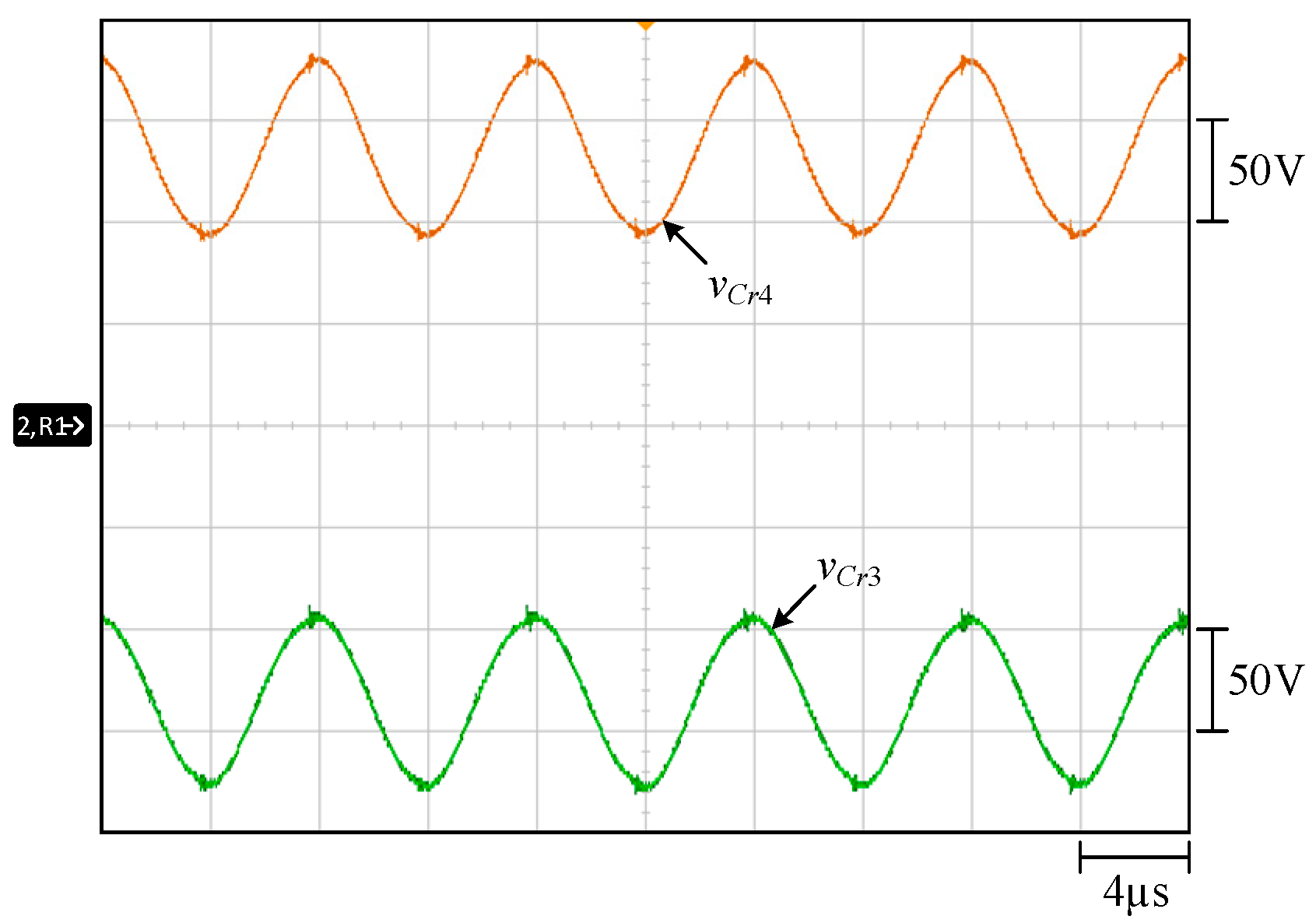
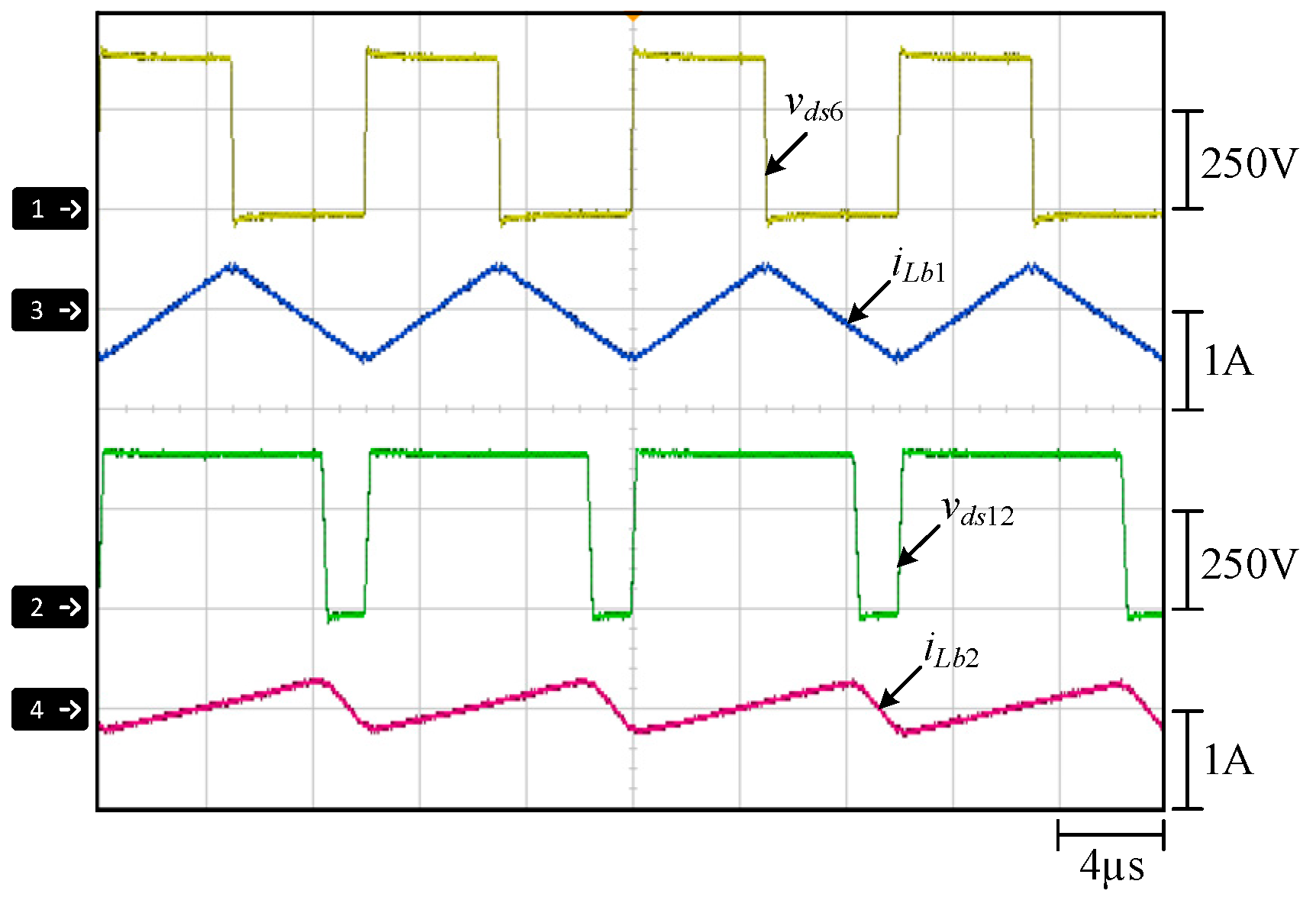
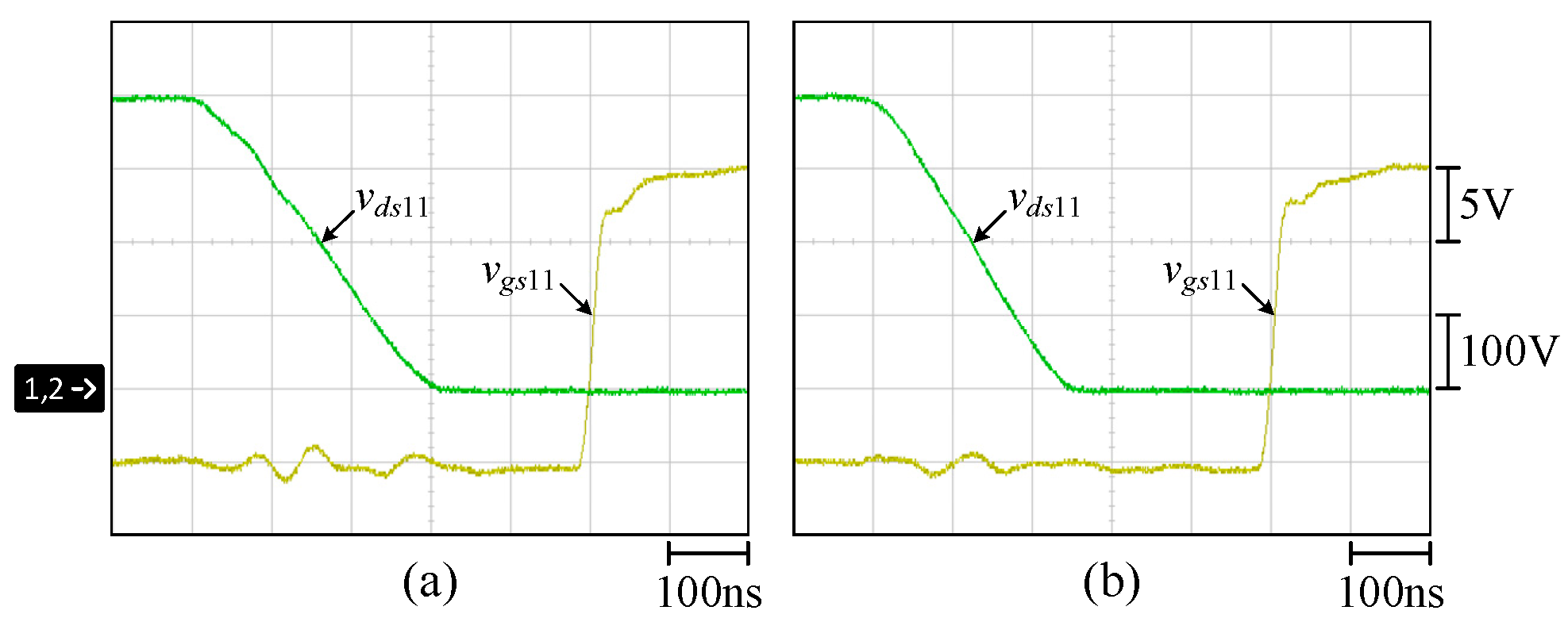
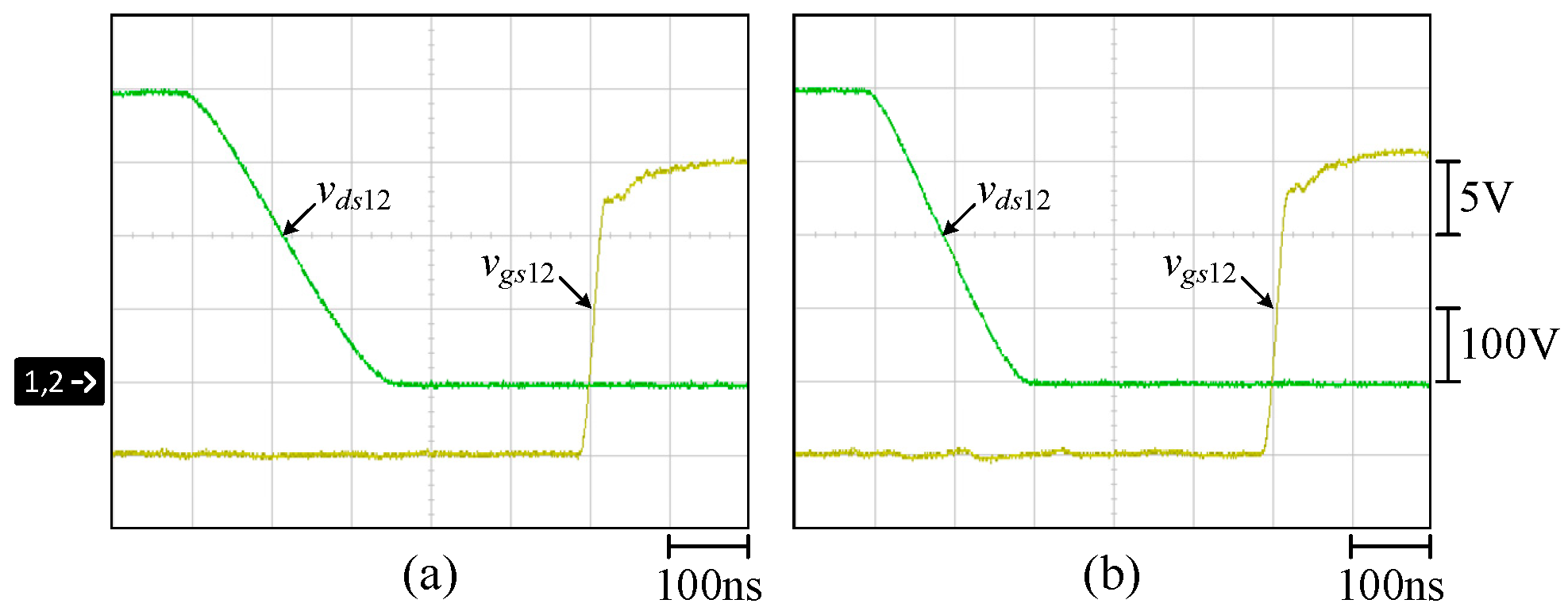
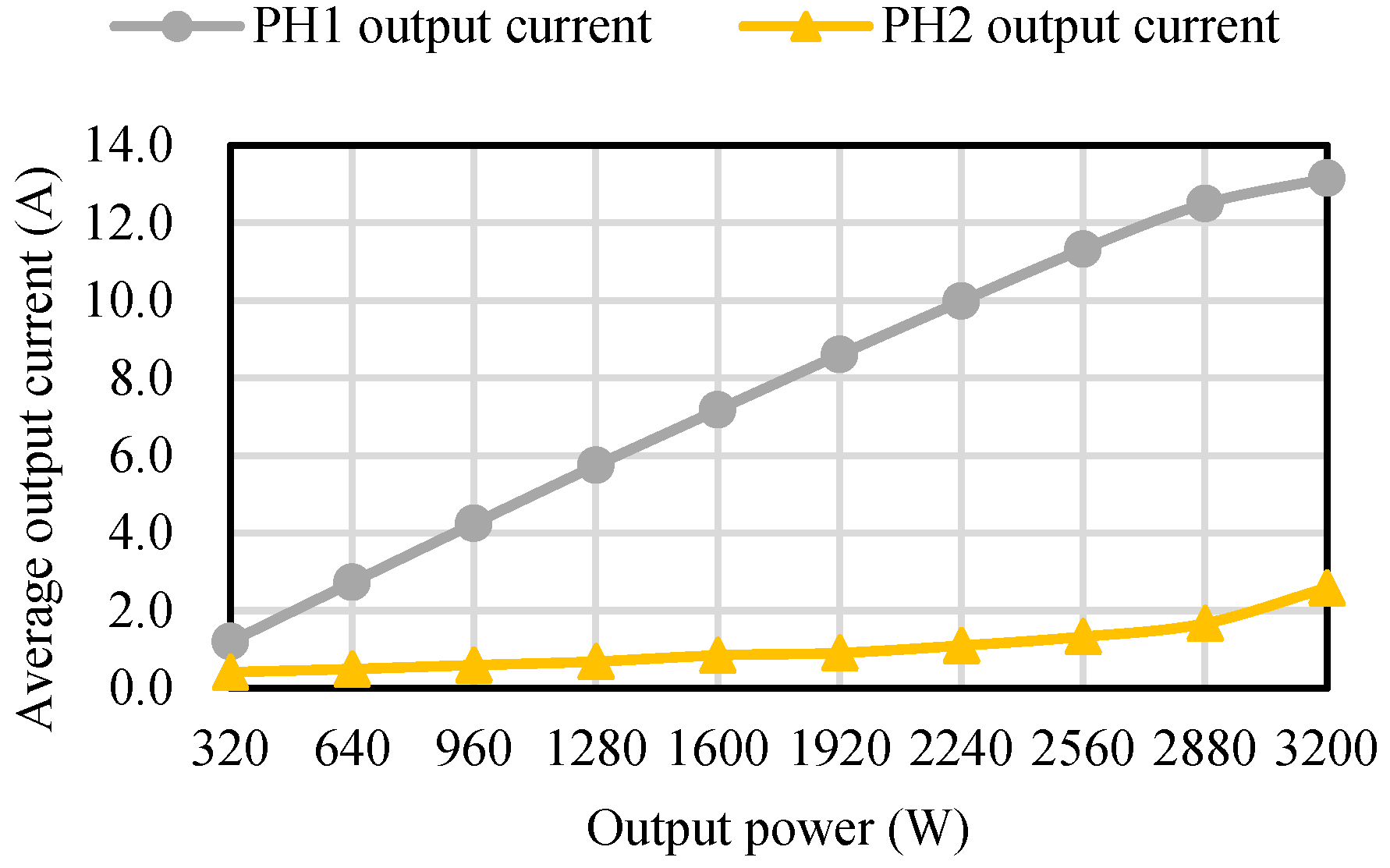
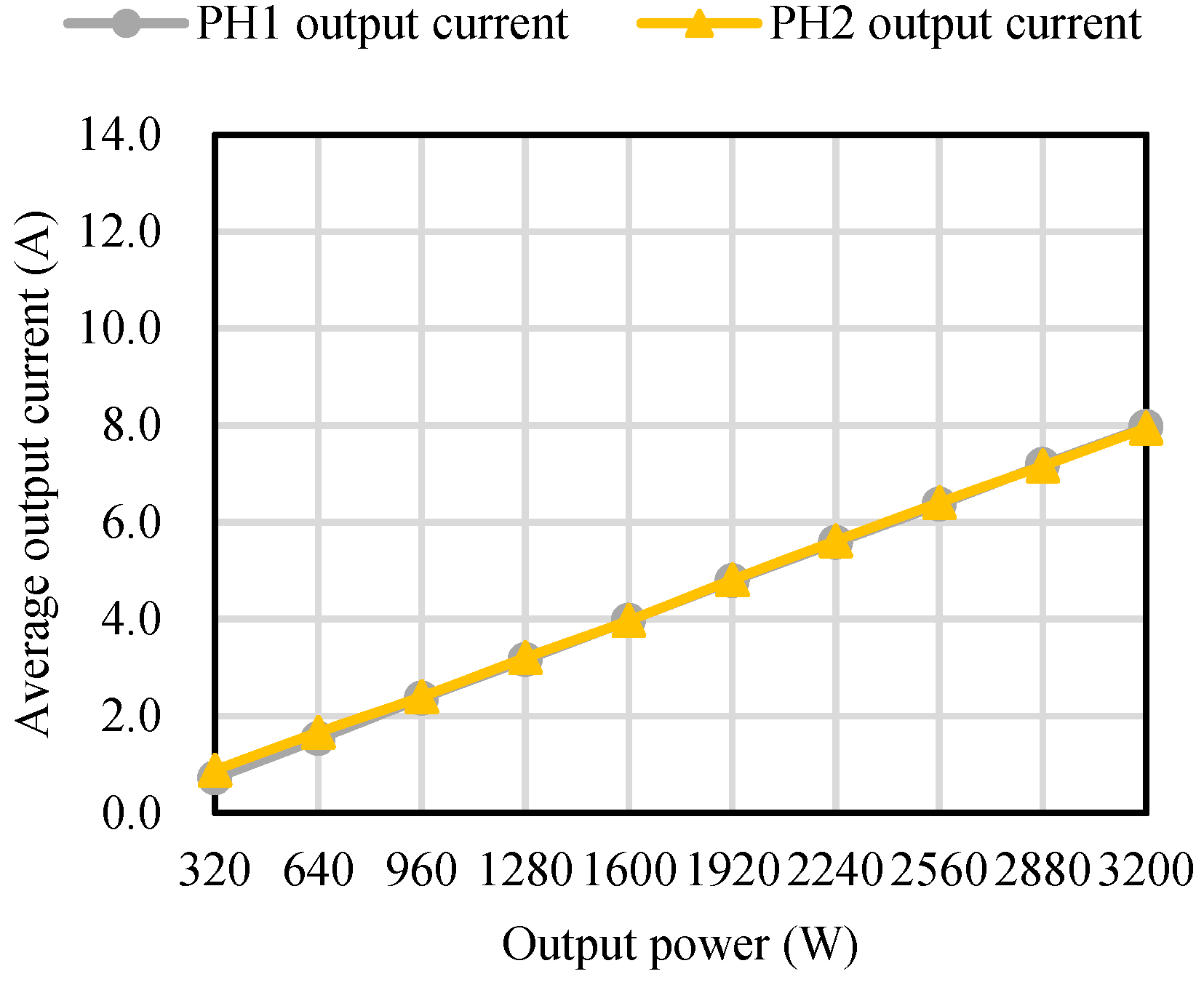
5. Experimental Results and Analyses
5.1. Circuit Specifications and Circuit
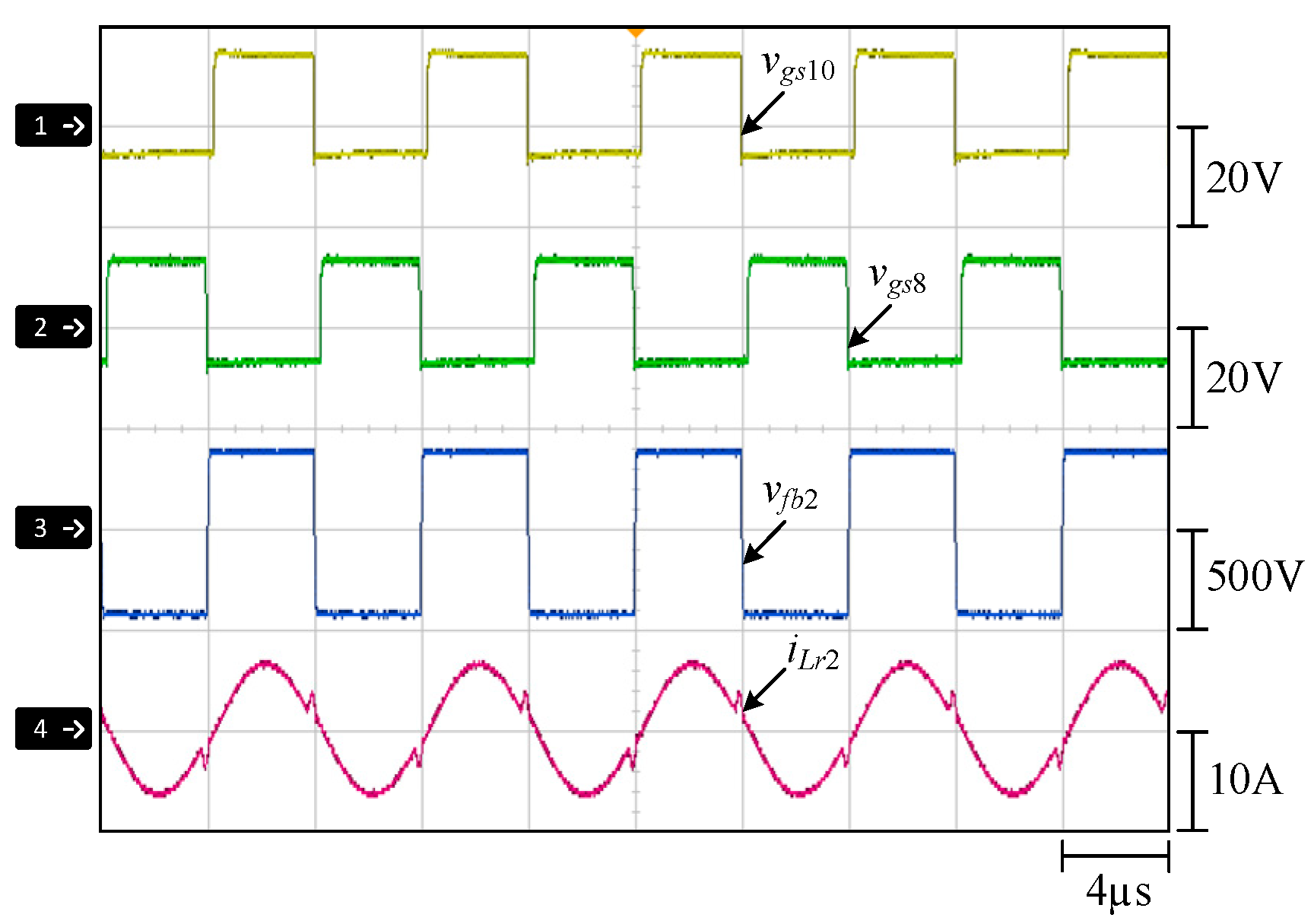
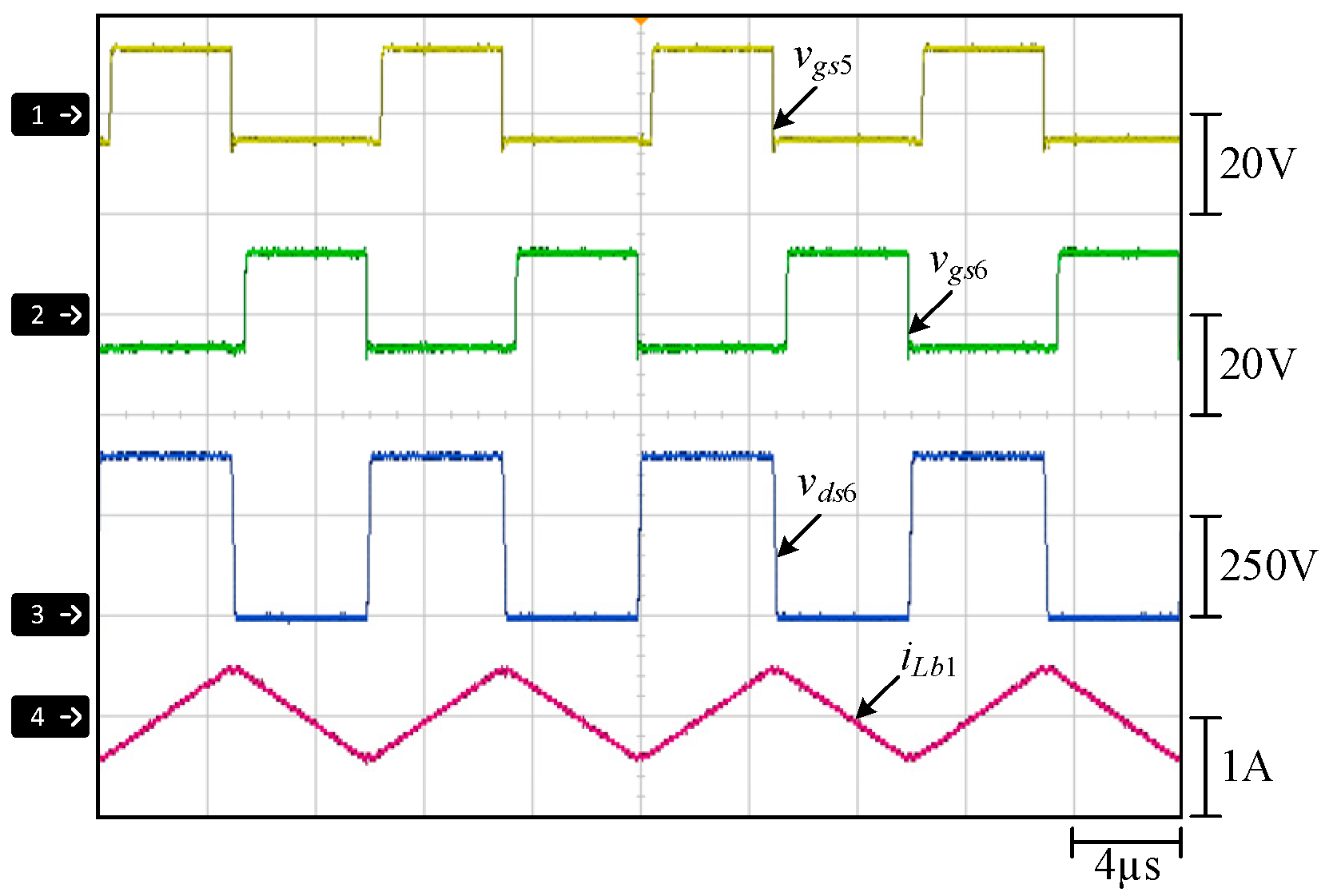
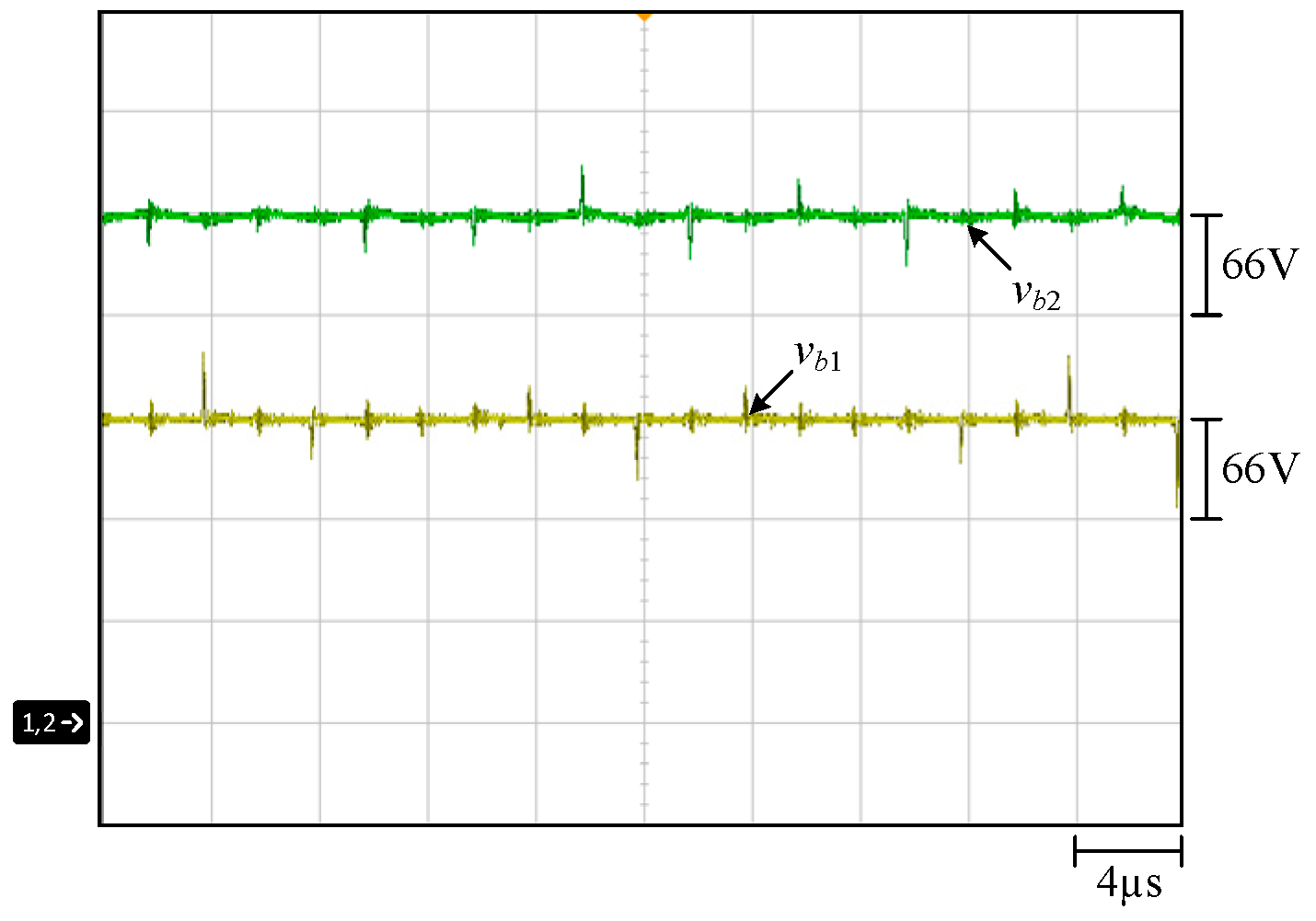
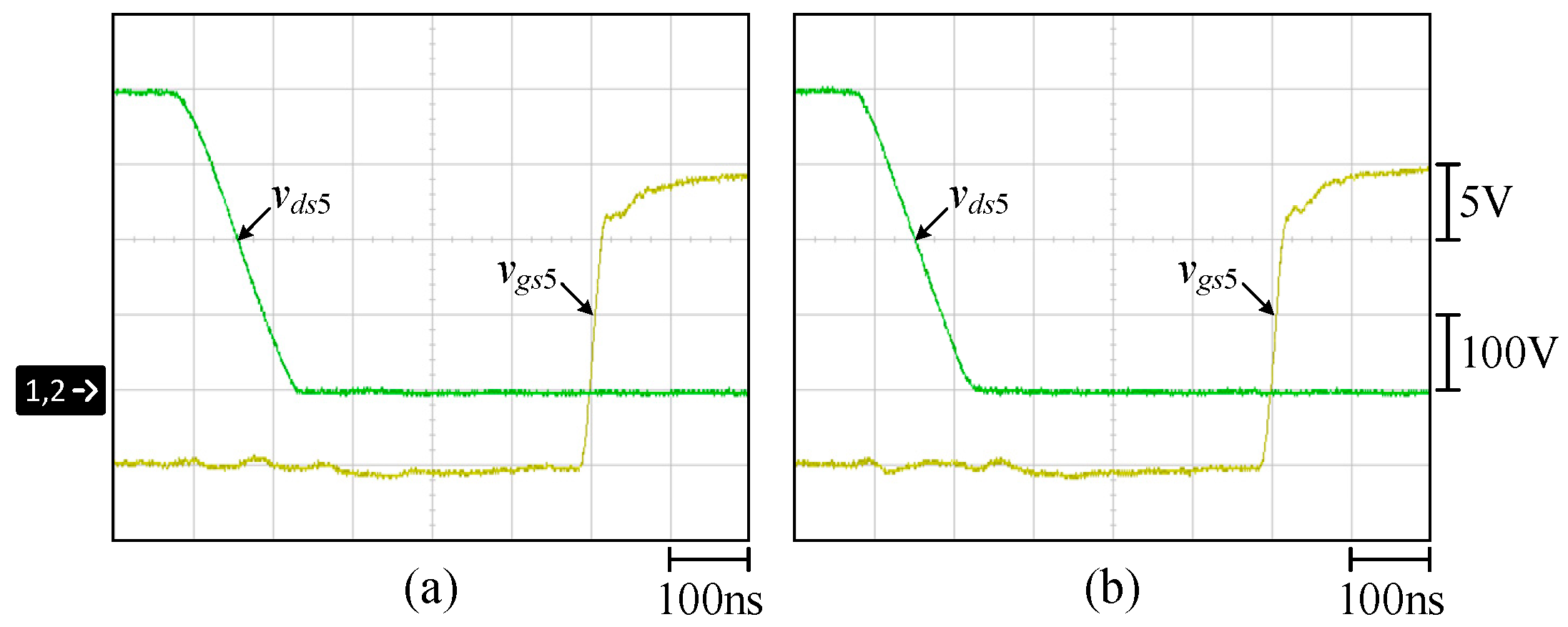
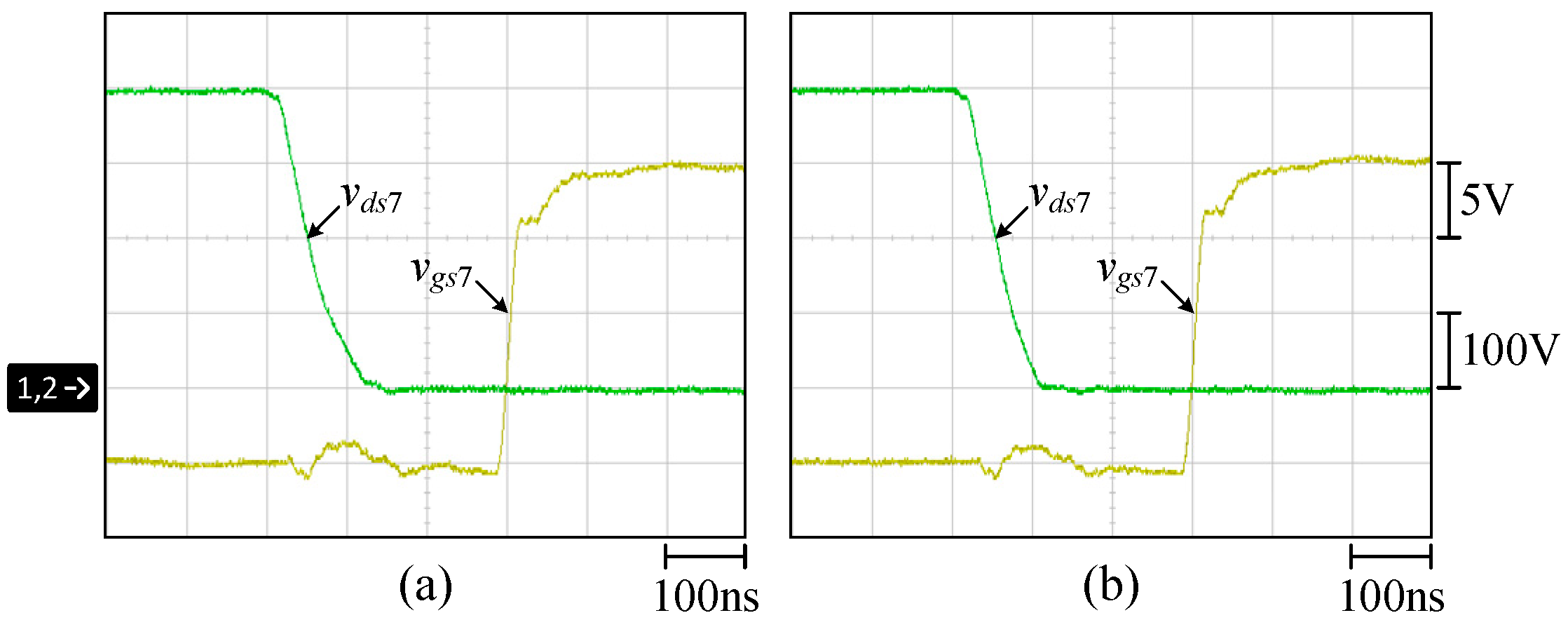
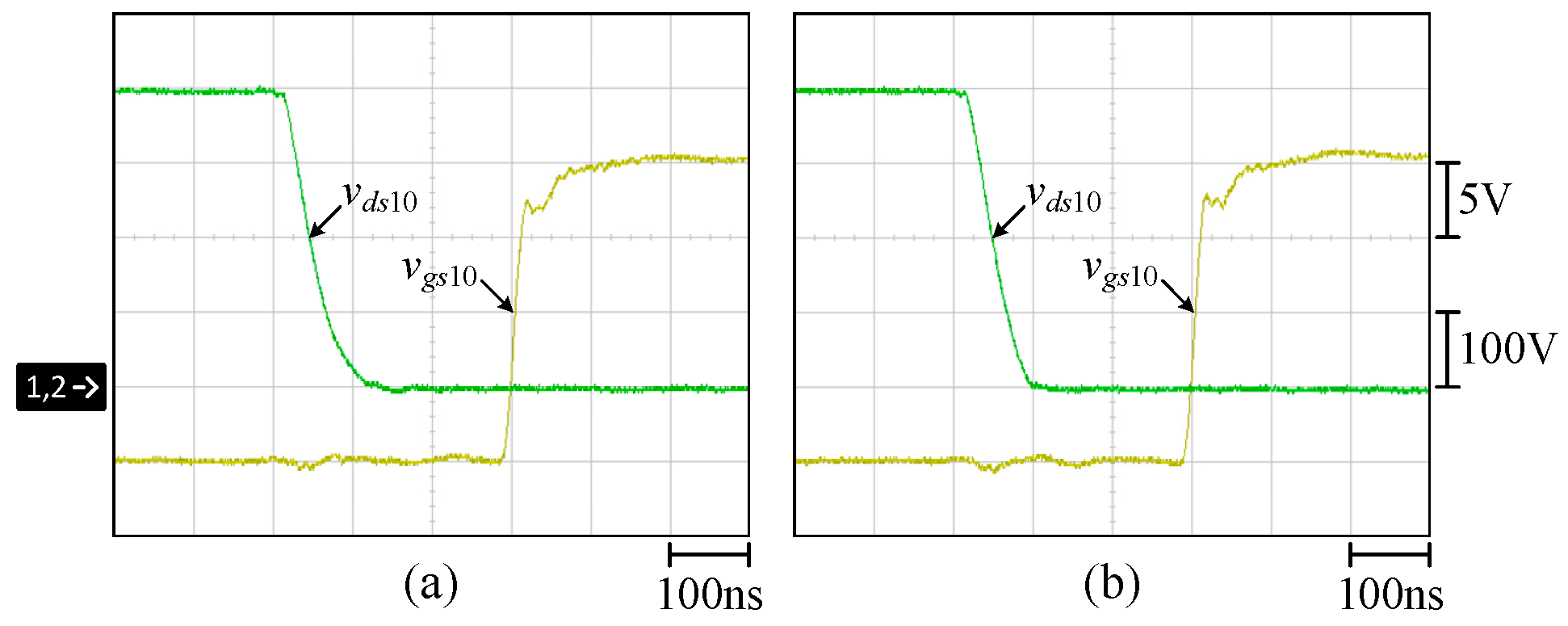
5.2. Measured Waveforms
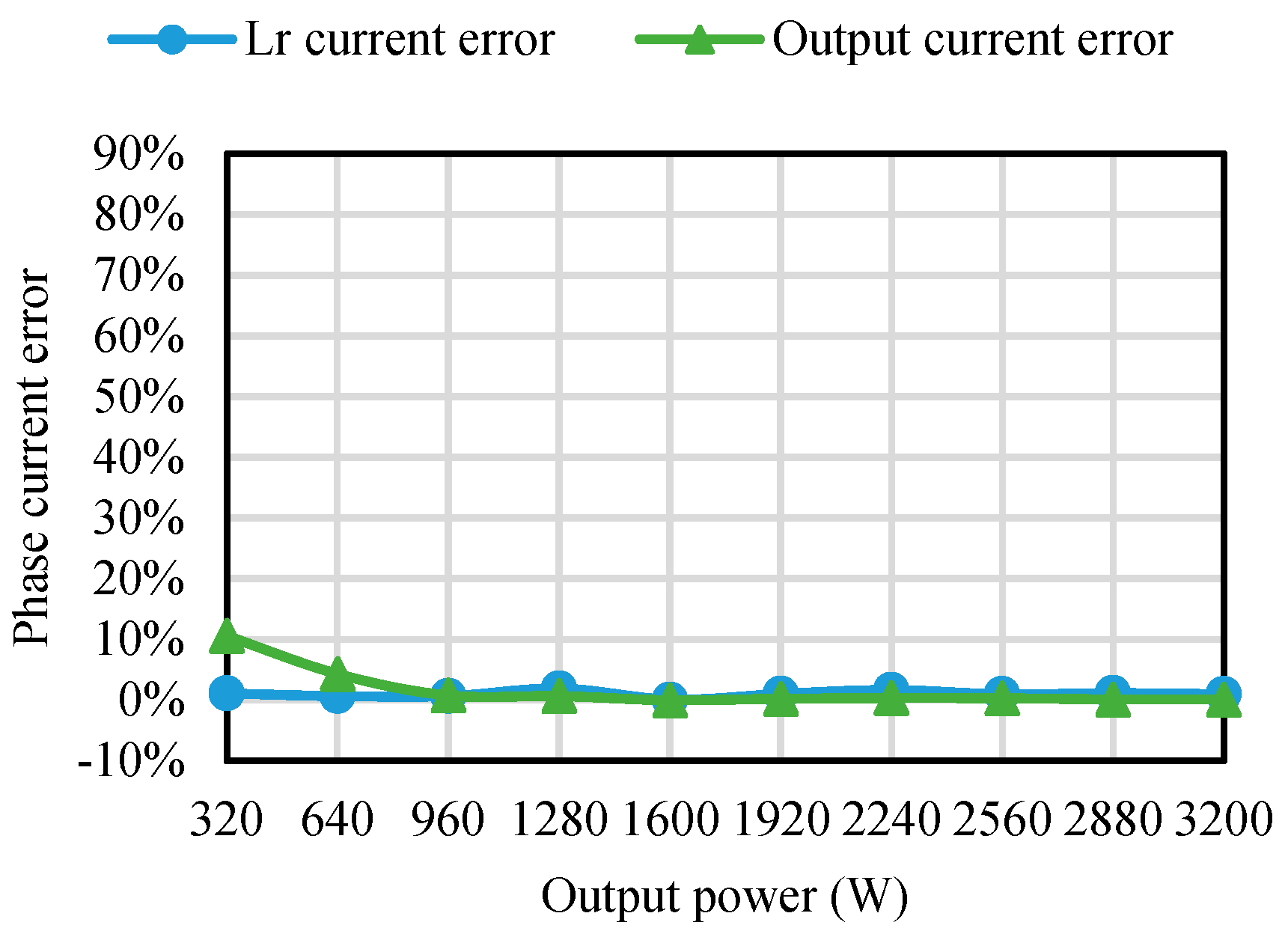
5.3. Current Error Measurement
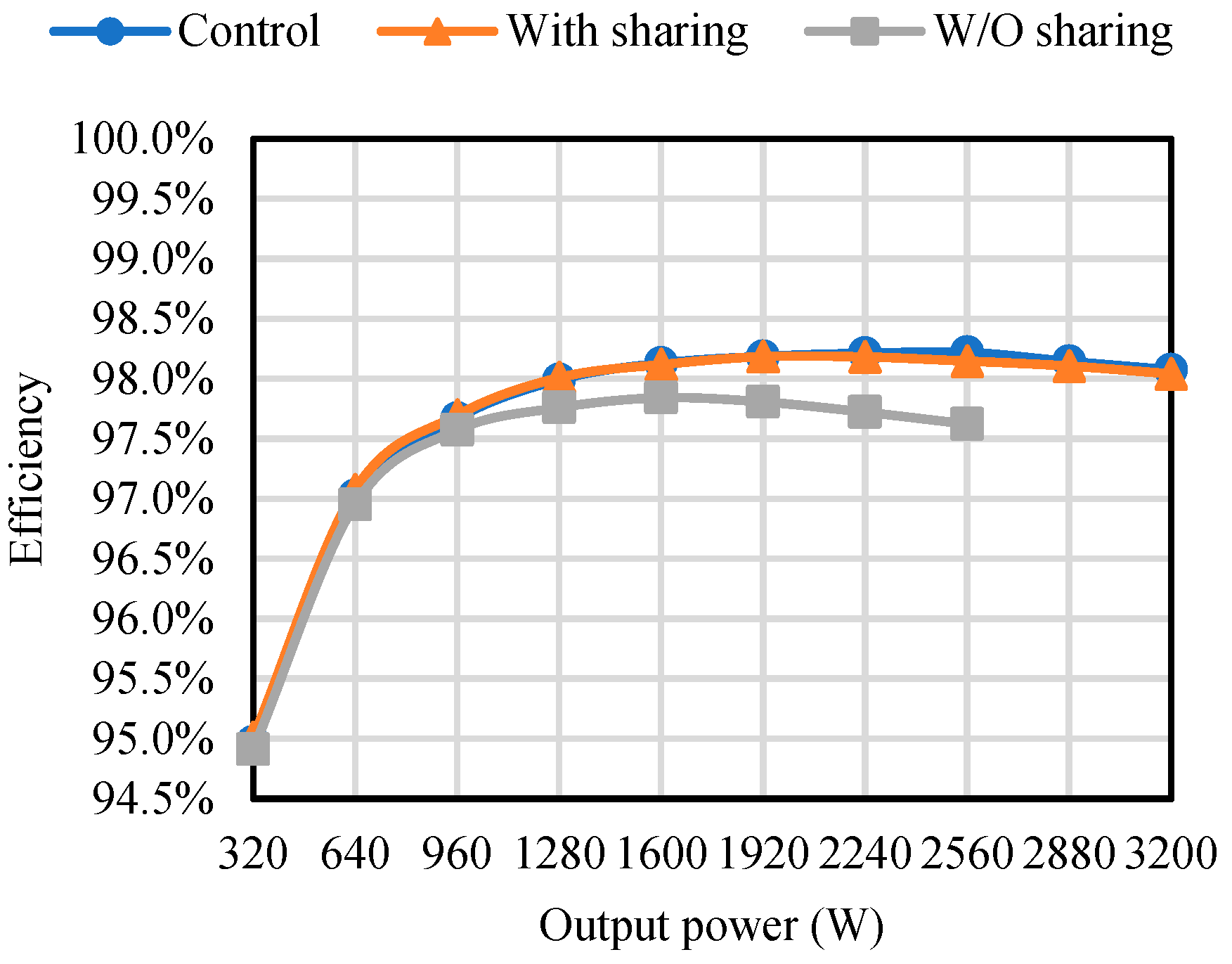
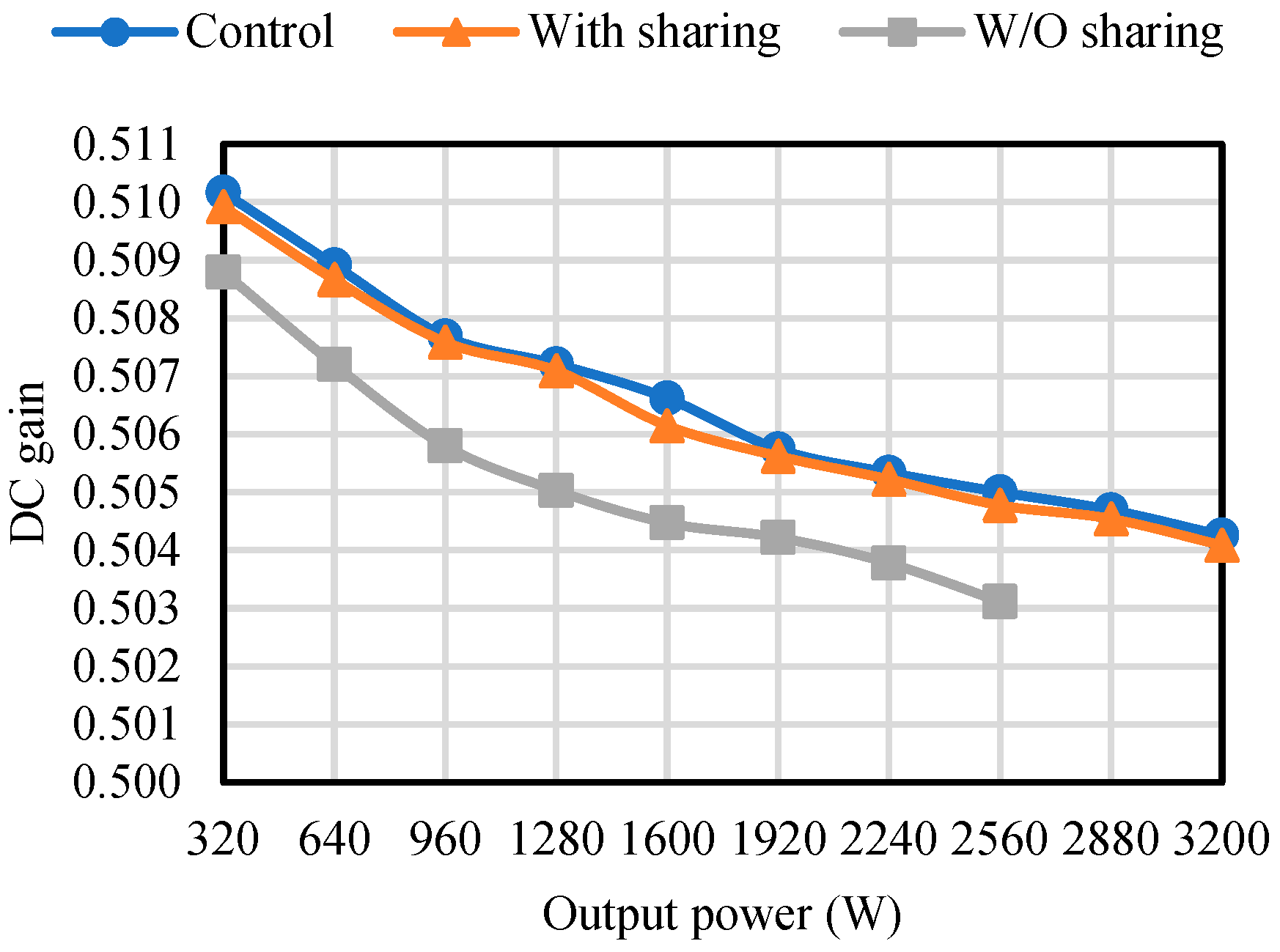
5.4. Measurement of Efficiency and DC Gain
6. Comparison of Related Literature
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Baba, D. Benefits of a Multiphase Buck Converter. Texas Instruments. 2012. Available online: https://www.ti.com/lit/an/slyt449/slyt449.pdf?ts=1652769828584&ref_url=https%253A%252F%252Fwww.google.com%252F (accessed on 5 February 2023).
- Wang, H.; Chen, Y.; Qiu, Y.; Fang, P.; Zhang, Y.; Wang, L.; Liu, Y.-F.; Afsharian, J.; Yang, Z. Common capacitor multiphase LLC converter with passive current sharing ability. IEEE Trans. Power Electron. 2018, 33, 370–387. [Google Scholar] [CrossRef]
- Wang, H.; Chen, Y.; Liu, Y.-F.; Afsharian, J.; Yang, Z. A passive current sharing method with common inductor multiphase LLC resonant converter. IEEE Trans. Power Electron. 2017, 32, 6994–7010. [Google Scholar] [CrossRef]
- Li, Y.; Shao, S.; Chen, H.; Zhang, J.; Sheng, K. High-gain high-efficiency IPOS LLC converter with coupled transformer and current sharing capability. IEEE CPSS Trans. Power Electron. Appl. 2020, 5, 63–73. [Google Scholar] [CrossRef]
- Yang, Y.; Yao, J.; Li, H.; Zhao, J. A novel current sharing method by grouping transformer’s secondary windings for a multiphase LLC resonant converter. IEEE Trans. Power Electron. 2020, 35, 4877–4890. [Google Scholar] [CrossRef]
- Ahmad, U.; Cha, H.; Naseem, N. Integrated current balancing transformer based input-parallel output-parallel LLC resonant converter modules. IEEE Trans. Power Electron. 2021, 36, 5278–5289. [Google Scholar]
- Kirshenboim, O.; Peretz, M. Combined multilevel and two-phase interleaved LLC converter with enhanced power processing characteristics and natural current sharing. IEEE Trans. Power Electron. 2018, 33, 5613–5620. [Google Scholar] [CrossRef]
- Shimada, T. Current sharing method using secondary voltage ripple for an interleaved LLC resonant converter. In Proceedings of the 2019 IEEE Conference on Power Electronics and Applications, Elazig, Turkey, 25–27 September 2019; pp. 1–7. [Google Scholar]
- Noah, M.; Endo, S.; Ishibashi, H.; Nanamori, K.; Imaoka, J.; Umetani, K.; Yamamoto, M. A current sharing method utilizing single balancing transformer for a multiphase LLC resonant converter with integrated magnetic. IEEE J. Emerg. Sel. Top. Power Electron. 2018, 6, 977–992. [Google Scholar] [CrossRef]
- Nakakohara, Y.; Otake, H.; Evans, T.; Tomohiko, M.; Tsuruya, M.; Nakahara, K. Three-phase LLC series resonant dc/dc converter using sic mosfets to realize high-voltage and high-frequency operation. IEEE Trans. Ind. Electron. 2016, 63, 2103–2110. [Google Scholar] [CrossRef]
- “3kW Dual-Phase LLC Evaluation Board.” Infineon. Available online: https://www.infineon.com/cms/en/product/evaluation-boards/eval_3kw_2llc_c7_20/ (accessed on 24 February 2023).
- Murata, K.; Kurokawa, F. An interleaved PFM LLC resonant converter with phase-shift compensation. IEEE Trans. Power Electron. 2016, 31, 2264–2272. [Google Scholar] [CrossRef]
- Arshadi, S.A.; Ordonez, M.; Eberle, W.; Saket, M.A.; Craciun, M.; Botting, C. Unbalanced three-phase LLC resonant converters: Analysis and trigonometric current balancing. IEEE Trans. Power Electron. 2019, 34, 2025–2038. [Google Scholar] [CrossRef]
- Two-Phase Interleaved LLC Resonant Converter Design with C2000TM Microcontrollers; Texas Instruments: Dallas, TX, USA, 2020; Available online: https://www.ti.com/lit/ug/tiduct9a/tiduct9a.pdf?ts=1652705048373&ref_url=https%253A%252F%252Fwww.ti.com%252Ftool%252FTIDM-1001 (accessed on 1 March 2023).
- Liu, F.; Zhou, R.G.X.; Ji, S.; Zhao, Q.; Zhang, X. An input-series-output-parallel converter system exhibiting natural input-voltage sharing and output-current sharing. IEEE Trans. Ind. Electron. 2021, 68, 1166–1177. [Google Scholar] [CrossRef]
- Chen, H.; Wu, X.; Shao, S. A current-sharing method for interleaved high-frequency LLC converter with partial energy processing. IEEE Trans. Ind. Electron. 2020, 67, 1498–1507. [Google Scholar] [CrossRef]
- Wang, M.; Zha, X.; Pan, S.; Gong, J.; Lin, W. A current-sharing method for interleaved high-frequency LLC converter with partial energy phase shift regulation. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 760–772. [Google Scholar] [CrossRef]
- Orietti, E.; Mattavelli, P.; Spiazzi, G.; Adragna, C.; Gattavari, G. Two-phase interleaved LLC resonant converter with current-controlled inductor. In Proceedings of the 2009 IEEE Brazilian Power Electronics Conference (BPEC), Bonito-Mato Grosso do Sul, Brazil, 27 September–1 October 2009; pp. 298–304. [Google Scholar]
- Hu, Z.; Qiu, Y.; Liu, Y.-F.; Sen, P.C. A control strategy and design method for interleaved LLC converters operating at variable switching frequency. IEEE Trans. Power Electron. 2014, 29, 4426–4437. [Google Scholar] [CrossRef]
- Thenathayalan, D.; Park, J. Highly flexible high-efficiency multiple-resonant wireless power transfer system using a controllable inductor. IEEE J. Emerg. Sel. Top. Power Electron. 2019, 7, 1914–1930. [Google Scholar] [CrossRef]
- Assadsangabi, B.; Mohamed Al, M.S.; Takahata, K. Planar variable inductor controlled by ferrofluid actuation. IEEE Trans. Magn. 2013, 49, 1402–1406. [Google Scholar] [CrossRef]
- Hu, Y.; Huber, L.; Jovanovic, M.M. Single-stage, universal-input ac/dc LED driver with current-controlled variable PFC boost inductor. IEEE Trans. Power Electron. 2012, 27, 1579–1588. [Google Scholar]
- Martins, M.; Perdigao, M.S.; Mendes, A.M.S.; Pinto, R.A.; Alonso, J.M. Analysis, design, and experimentation of a dimmable resonant-switched-capacitor LED driver with variable inductor control. IEEE Trans. Power Electron. 2017, 32, 3051–3062. [Google Scholar] [CrossRef]
- Alonso, J.M.M.; Perdigao, S.; Vaquero, D.G.; Calleja, A.J.; Saraiva, E.S. Analysis, design, and experimentation on constant-frequency dc-dc resonant converters with magnetic control. IEEE Trans. Power Electron. 2012, 27, 1369–1382. [Google Scholar] [CrossRef]
- Wei, Y.; Luo, Q.X.; Du, A.N.; Alonso, J.M.; Mantooth, H.A. Analysis and design of the LLC resonant converter with variable inductor control based on time-domain analysis. IEEE Trans. Ind. Electron. 2020, 67, 5432–5443. [Google Scholar] [CrossRef]
- Wei, Y.; Woldegiorgis, D.; Mantooth, A. Variable resonant and magnetizing inductor control for LLC resonant converter. In Proceedings of the 2020 IEEE International Symposium on Power Electronics for Distributed Generation Systems (PEDG), Dubrovnik, Croatia, 28 September–1 October 2020; pp. 149–153. [Google Scholar]
- Xu, G.; Koyama, Y.; Sogabe, Y.; Amemiya, N. Current sharing in spiral copper-plated multifilament coated conductors. IEEE Trans. Appl. Supercond. 2024, 34, 1–5. [Google Scholar] [CrossRef]
- Baek, D.-H.; Eun, Y.; Kwon, D.-S.; Kim, M.-O.; Chung, T.; Kim, J. Widely tunable variable capacitor with switching and latching mechanisms. IEEE Electron Device Lett. 2015, 36, 186–188. [Google Scholar] [CrossRef]
- Yang, H.-H.; Han, C.-H.; Choi, S.-J.; Choi, D.-H.; Yoon, J.-B. Signal power-insensitive analog mems tunable capacitor by immobilizing the movable plates. IEEE J. Microelectromech. Syst. 2015, 24, 1545–1556. [Google Scholar] [CrossRef]
- Patel, C.D.; Rebeiz, G.M. High-Q 3 b/4 b RF MEMS digitally tunable capacitors for 0.8–3 GHz applications. IEEE Microw. Wirel. Compon. Lett. 2012, 22, 394–396. [Google Scholar] [CrossRef]
- Gu, L.; Li, X. Rotational driven RF variable capacitors with post-CMOS processes. IEEE Electron Device Lett. 2008, 29, 195–197. [Google Scholar] [CrossRef]
- Yeh, J.A.; Chang, C.A.; Cheng, C.-C.; Huang, J.-Y.; Hsu, S.S.H. Microwave characteristics of liquid-crystal tunable capacitors. IEEE Electron Device Lett. 2005, 26, 451–453. [Google Scholar] [CrossRef]
- Harada, K.; Katsuki, A.M.; Fujiwara, N.H.; Matsushita, H. Resonant converter controlled by variable capacitance devices. IEEE Trans. Power Electron. 1993, 8, 404–410. [Google Scholar] [CrossRef]
- Ben-Yaakov, S. Characterization of ferroelectric ceramic capacitors. In Proceedings of the 2018 IEEE European Conference on Power Electronics and Applications (EPE ECCE Europe), Riga, Latvia, 17–21 September 2018; pp. 1–8. [Google Scholar]
- Tian, J.; Hu, A.P. A dc-voltage-controlled variable capacitor for stabilizing the ZVS frequency of a resonant converter for wireless power transfer. IEEE Trans. Power Electron. 2017, 32, 2312–2318. [Google Scholar] [CrossRef]
- Tishechkin, S.; Ben-Yaakov, S. Adaptive capacitance impedance matching (ACIM) of WPT systems by voltage controlled capacitors. In Proceedings of the 2019 IEEE PELS Workshop on Emerging Technologies: Wireless Power Transfer (WoW), London, UK, 18–21 June 2019; pp. 396–400. [Google Scholar]
- Zeng, H.; Peng, F.Z. Non-linear capacitor based variable capacitor for self-tuning resonant converter in wireless power transfer. In Proceedings of the 2018 IEEE Applied Power Electronics Conference and Exposition (APEC), San Antonio, TX, USA, 4–8 March 2018; pp. 1375–1379. [Google Scholar]
- EIA STANDARD, RS-198-B; Ceramic Dielectric Capacitors 1, 2 and 3. Engineering Department, Electronics Inmstitutes Association: Washington, DC, USA, 1971.
- “Design Support Software Simsurfing”. muRata: Kyoto, Japan, 2019. Available online: https://www.murata.com/en-us/tool/simsurfing (accessed on 3 March 2023).



























| Specification | Value |
|---|---|
| Number of Converter Phases (Nphase) | 2 |
| Switching Phase Difference (θshift) | 90° (=0.5 π) |
| Rated Input Voltage (Vs) | 400 V |
| DC Voltage Gain (Gv) | 0.5% |
| Output Power Range (Po) | 0.32~3.2 kW |
| Normal Resonance Frequency (fr) | 130 kHz |
| Full-Bridge Switching Frequency (fs) | 125 kHz |
| Bias Circuit Cut Frequency (fsb) | 100 kHz |
| Output Voltage Ripple (Vripple) | ≤2 Vpp |
| Resonant Inductance Deviation (ΔLr) | ~50% Lr |
| [5] | [7] | [16] | [17] | [18] | Proposed | ||
|---|---|---|---|---|---|---|---|
| Method | Topology Improvement | Partial Energy Processing | Variable Inductor | Variable Capacitor | |||
| Extendibility | No | No | Yes | Yes | Yes | Yes | |
| Phase Shift Switching | No | No | Yes | Yes | Yes | Yes | |
| Phase Shielding Ability | No | No | Yes | Yes | Yes | No | |
| Active Current Control | No | No | Yes | Yes | Yes | Yes | |
| Auxiliary Circuit Power Level | N/A | N/A | Medium | Medium | Low | Low | |
| Auxiliary Circuit Power Loss | N/A | N/A | Low | Low | Low | Lowest | |
| Current Error Ratio | 0.5% | 0.8% | 0.5% | 1.6% | 1.2% | 0.4% | |
| Peak Efficiency | 97.5% | 97.3% | 96.8% | 97.1% | 94.5% | 98.2% | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lee, Y.-L.; Hwu, K.-I. Multiphase LLC DC-Link Converter with Current Equalization Based on CM Voltage-Controlled Capacitor. Energies 2024, 17, 2793. https://doi.org/10.3390/en17112793
Lee Y-L, Hwu K-I. Multiphase LLC DC-Link Converter with Current Equalization Based on CM Voltage-Controlled Capacitor. Energies. 2024; 17(11):2793. https://doi.org/10.3390/en17112793
Chicago/Turabian StyleLee, Yue-Lin, and Kuo-Ing Hwu. 2024. "Multiphase LLC DC-Link Converter with Current Equalization Based on CM Voltage-Controlled Capacitor" Energies 17, no. 11: 2793. https://doi.org/10.3390/en17112793
APA StyleLee, Y.-L., & Hwu, K.-I. (2024). Multiphase LLC DC-Link Converter with Current Equalization Based on CM Voltage-Controlled Capacitor. Energies, 17(11), 2793. https://doi.org/10.3390/en17112793







