Perspective on the Development and Integration of Hydrogen Sensors for Fuel Cell Control
Abstract
:1. Introduction
2. State-of-the-Art Fuel Cell Control and Hydrogen Sensors
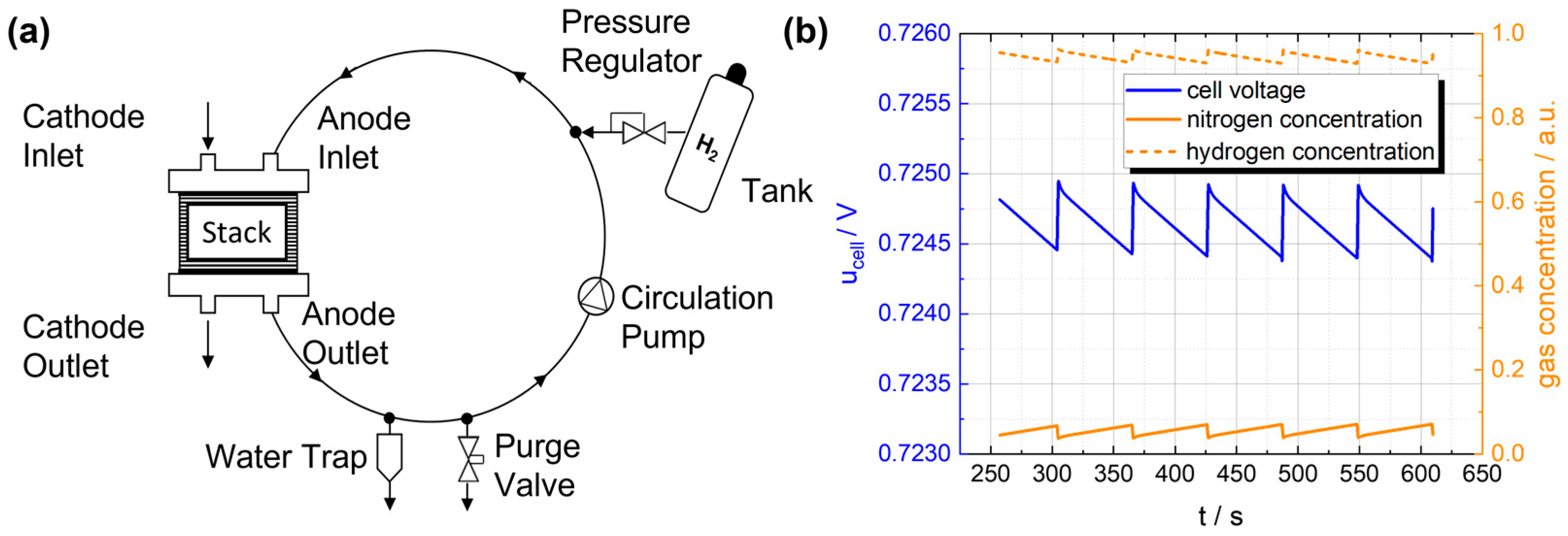
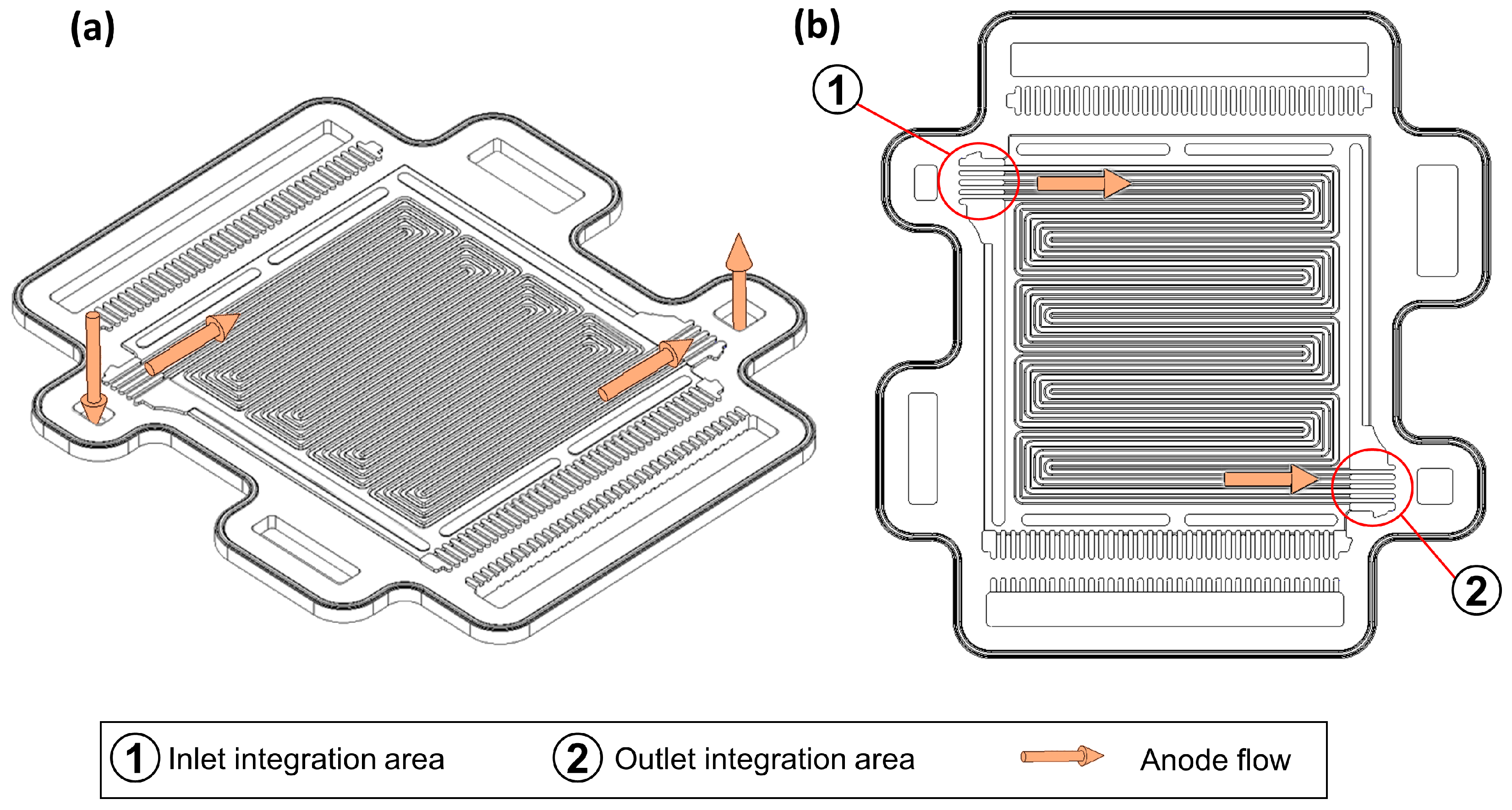
2.1. Fuel Cell System
2.2. Hydrogen Sensors
| Working Principle | Pros | Cons | Suitability |
|---|---|---|---|
| Catalytic reactions [14,40] | Fast reaction times, high sensitivity | Non-selective, requires oxygen | Poor |
| Metal oxide-based/ redox reactions [19] | Fast reaction times, simple, low-cost production | Requires oxygen to regenerate the oxide layer | Poor |
| Electrochemical [27] | Simple functionality, high sensitivity, low temperatures | Requires oxygen | Poor |
| Thermal conductivity [27] | Covers entire measuring range, no oxygen required | Reference gas, large set-up, non-selective | Poor |
| Propagation of sound waves [27] | Covers entire measuring range, simple functionality | Highly susceptible to humidity | Medium |
| Gas-sensitive metals [41] | Simple functionality, easy to manufacture, works without oxygen | Additives required to protect against hydrogen embrittlement | Good |
| Chemiresistive [34,35] | Low production costs, manufacturing on arbitrary surfaces | Highly susceptible to humidity | Medium |
| Organic | Low production costs, tailor-made materials, biocompatible | No proof of concept | Good |
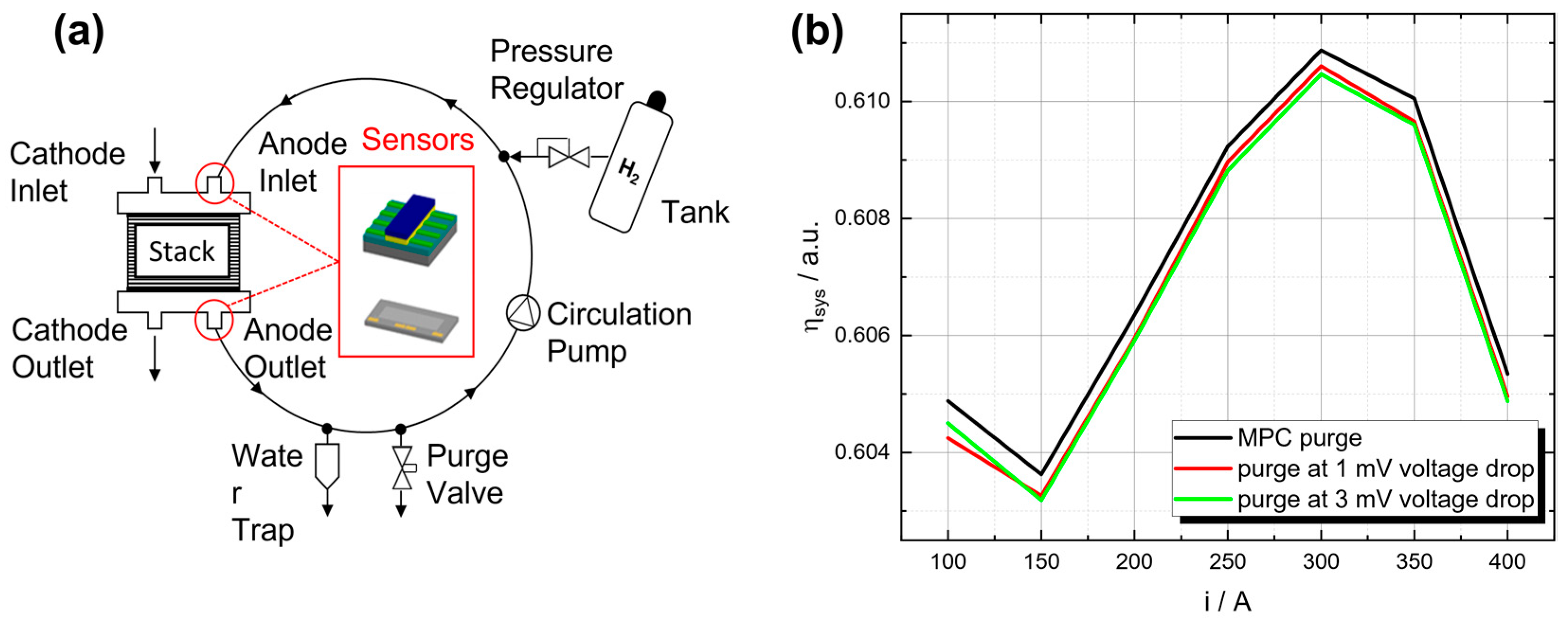
2.3. Fuel Cell Hydrogen Control
3. Requirements for New Hydrogen Sensors
4. Perspective
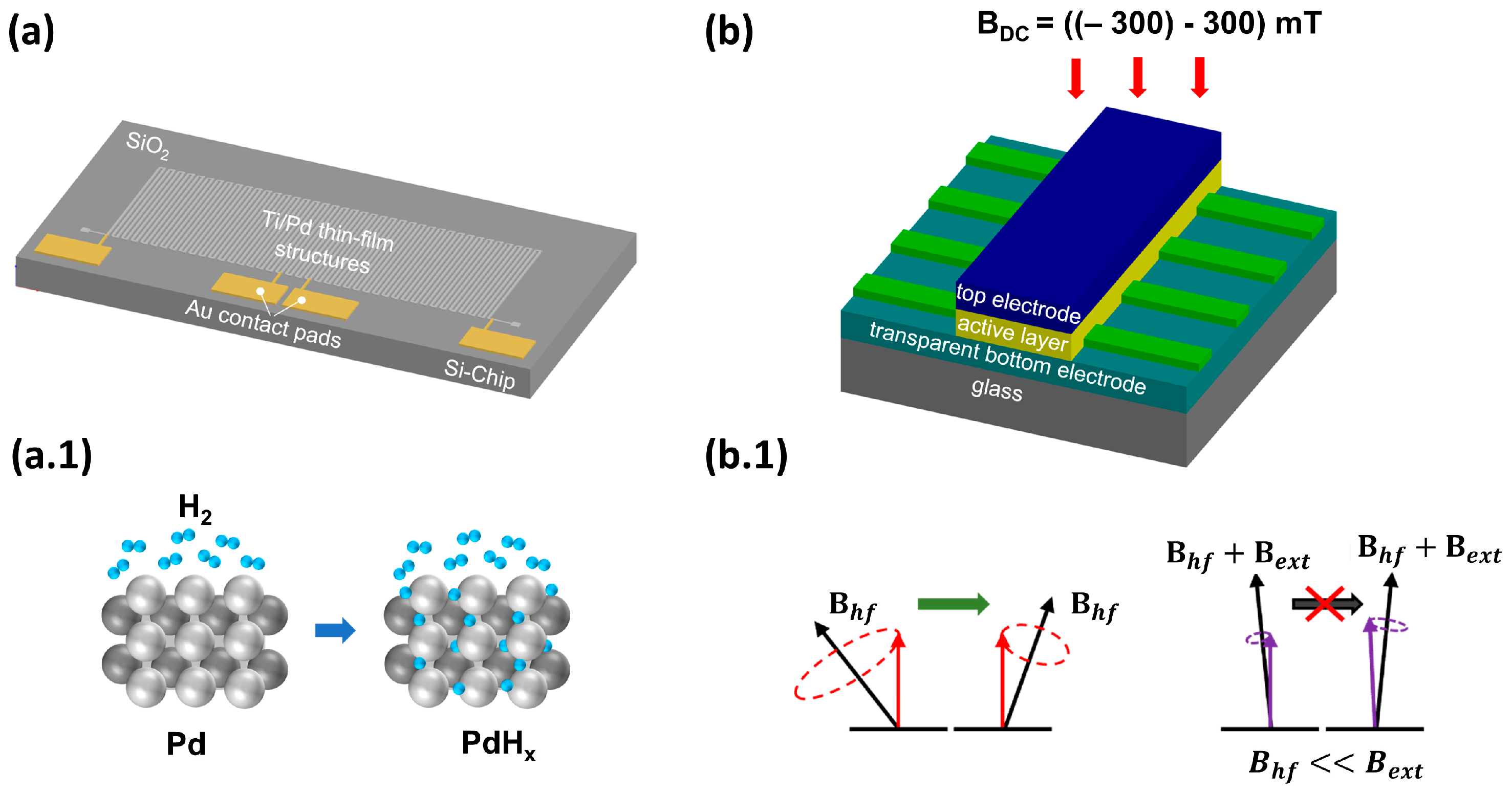
4.1. Development of Hydrogen Sensors
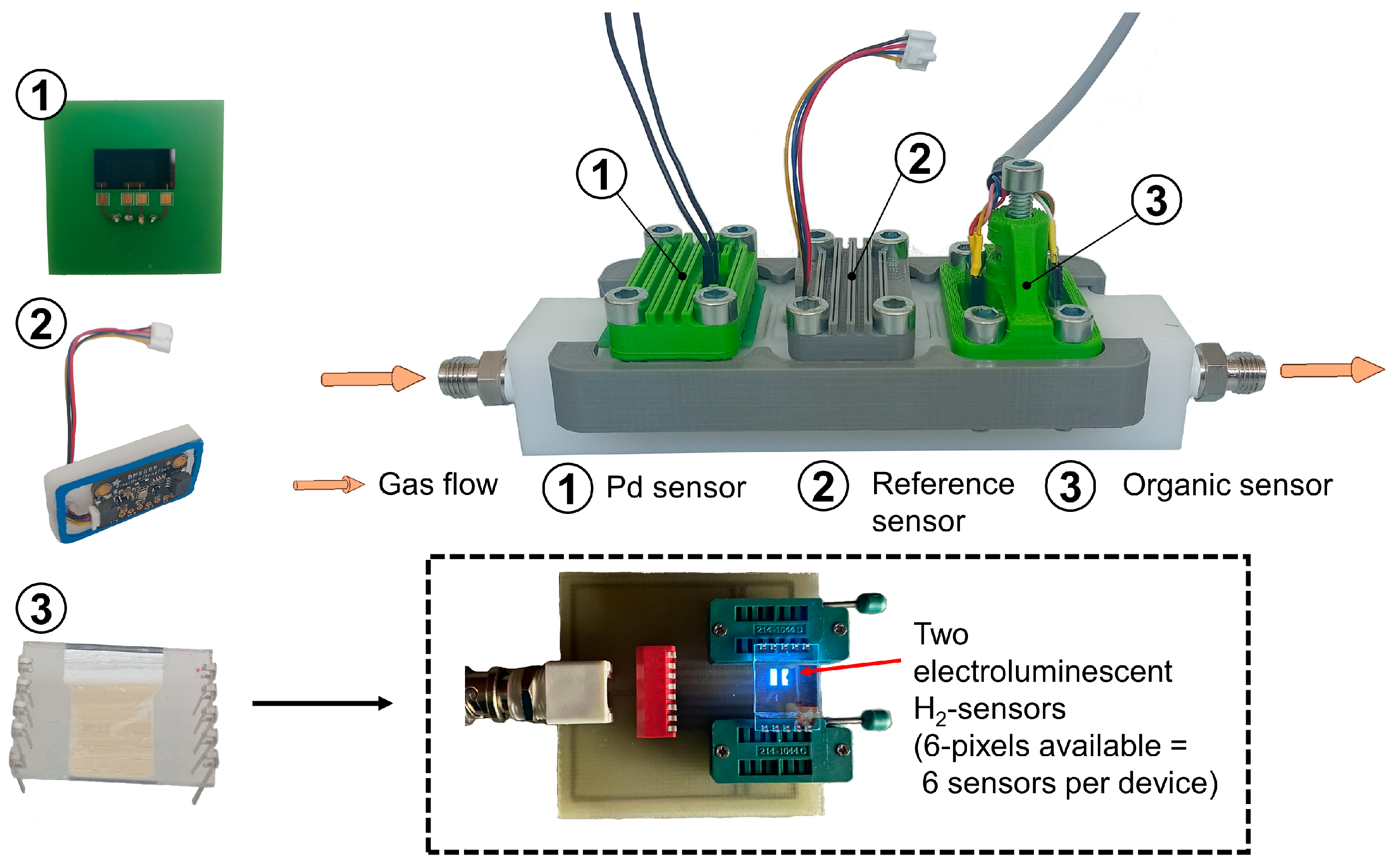
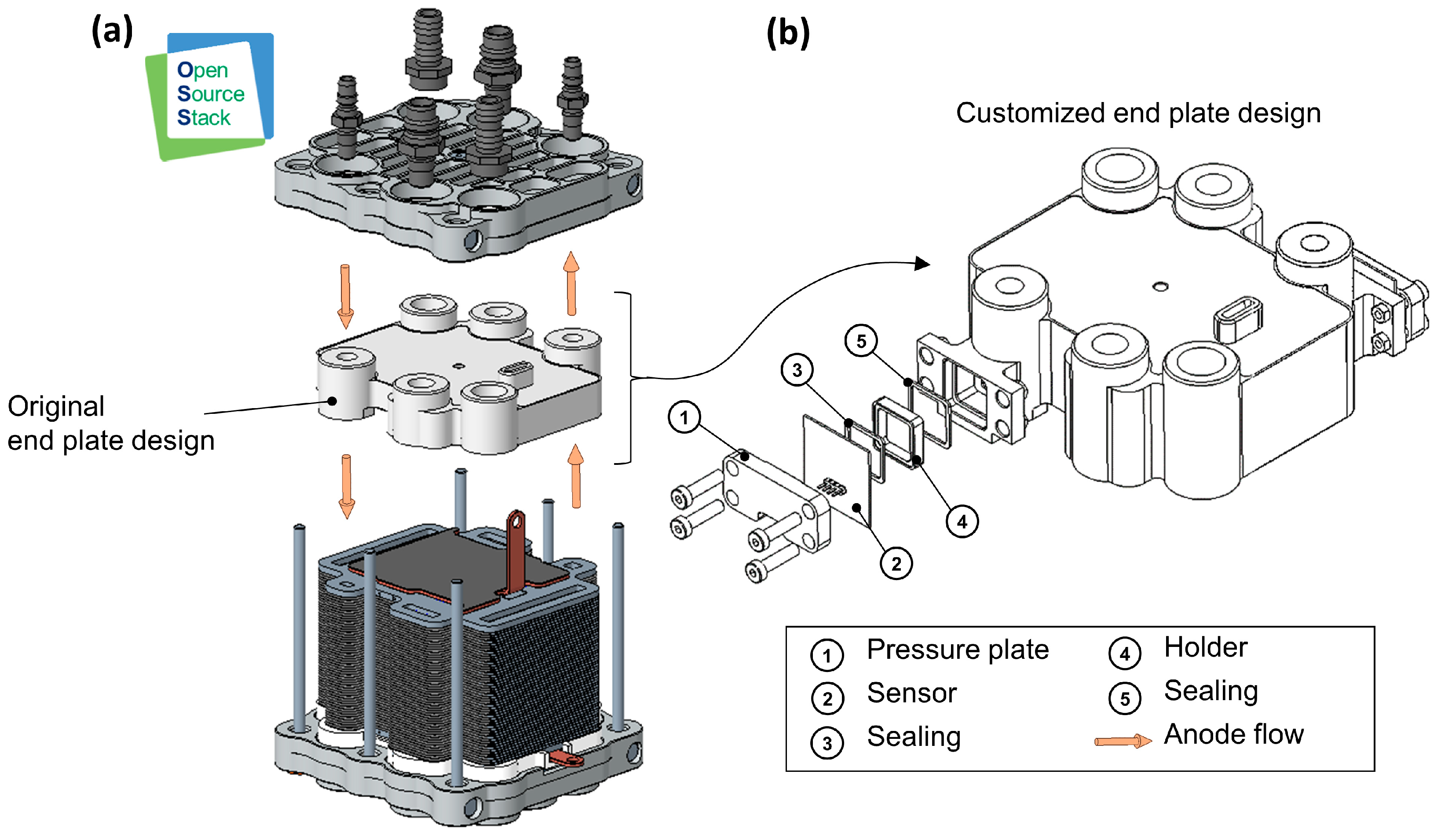
4.2. Sensor Integration
4.3. Enhancement of Data Analysis to Increase Computational Efficiency
4.4. Development of Advanced Fuel Cell Control
5. Conclusions and Outlook
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Morán-Durán, A.; Martínez-Sibaja, A.; Rodríguez-Jarquin, J.P.; Posada-Gómez, R.; González, O.S. PEM Fuel Cell Voltage Neural Control Based on Hydrogen Pressure Regulation. Processes 2019, 7, 434. [Google Scholar] [CrossRef]
- He, H.; Quan, S.; Wang, Y.-X. Hydrogen Circulation System Model Predictive Control for Polymer Electrolyte Membrane Fuel Cell-Based Electric Vehicle Application. Int. J. Hydrogen Energy 2020, 45, 20382–20390. [Google Scholar] [CrossRef]
- Tian, Y.; Zou, Q.; Lin, Z. Hydrogen Leakage Diagnosis for Proton Exchange Membrane Fuel Cell Systems: Methods and Suggestions on Its Application in Fuel Cell Vehicles. IEEE Access 2020, 8, 224895–224910. [Google Scholar] [CrossRef]
- Hauck, M.; Petzke, F.; Tafat, R.; Streif, S. Observability Analysis of PEM Fuel Cell Systems with Anode Recirculation. In Proceedings of the 2024 European Control Conference (ECC), Stockholm, Sweden, 25–28 June 2024; pp. 2423–2428. [Google Scholar]
- Tang, Q.; Li, B.; Yang, D.; Ming, P.; Zhang, C.; Wang, Y. Review of Hydrogen Crossover through the Polymer Electrolyte Membrane. Int. J. Hydrogen Energy 2021, 46, 22040–22061. [Google Scholar] [CrossRef]
- Tang, X.; Yang, M.; Shi, L.; Hou, Z.; Xu, S.; Sun, C. Adaptive State-of-Health Temperature Sensitivity Characteristics for Durability Improvement of PEM Fuel Cells. Chem. Eng. J. 2024, 491, 151951. [Google Scholar] [CrossRef]
- Quan, S.; Wang, Y.-X.; Xiao, X.; He, H.; Sun, F. Feedback Linearization-Based MIMO Model Predictive Control with Defined Pseudo-Reference for Hydrogen Regulation of Automotive Fuel Cells. Appl. Energy 2021, 293, 116919. [Google Scholar] [CrossRef]
- Liu, Z.; Chen, J.; Liu, H.; Yan, C.; Hou, Y.; He, Q.; Zhang, J.; Hissel, D. Anode Purge Management for Hydrogen Utilization and Stack Durability Improvement of PEM Fuel Cell Systems. Appl. Energy 2020, 275, 115110. [Google Scholar] [CrossRef]
- Hauck, M.; Petzke, F.; Streif, S. Model Predictive Purge Control for PEM Fuel Cell Systems with Anode Recirculation. In Proceedings of the 60th IEEE Conference on Decision and Control, Austin, TX, USA, 14–17 December 2021; IEEE: Piscataway, NJ, USA, 2021; pp. 6359–6364. [Google Scholar]
- Tang, Y.-Q.; Fang, W.-Z.; Lin, H.; Tao, W.-Q. Thin Film Thermocouple Fabrication and Its Application for Real-Time Temperature Measurement inside PEMFC. Int. J. Heat Mass Transf. 2019, 141, 1152–1158. [Google Scholar] [CrossRef]
- Wagner, E. Das System Brennstoffzelle: Wasserstoffsysteme Ganzheitlich Entwickeln; Hanser: Munich, Germany, 2023; ISBN 3-446-47505-2. [Google Scholar]
- Muck, N.; David, C. Integrating Fiber Sensing for Spatially Resolved Temperature Measurement in Fuel Cells. Energies 2023, 17, 16. [Google Scholar] [CrossRef]
- Awang, Z. Gas Sensors: A Review. Sens Transducers 2014, 168, 61–75. [Google Scholar]
- Lee, E.-B.; Hwang, I.-S.; Cha, J.-H.; Lee, H.-J.; Lee, W.-B.; Pak, J.J.; Lee, J.-H.; Ju, B.-K. Micromachined Catalytic Combustible Hydrogen Gas Sensor. Sens. Actuators B Chem. 2011, 153, 392–397. [Google Scholar] [CrossRef]
- Honeywell Model 705 HT Sensor. Available online: https://prod-edam.honeywell.com/content/dam/honeywell-edam/sps/his/it-it/products/gas-and-flame-detection/documents/hwa6110_h_model_705_high_temperature_sensor_ds0301_v3_flr_6_29_10.pdf?download=false (accessed on 11 September 2024).
- Samari, R. Low Power Combustible Gas Sensors. U.S. Patent 7,833,482 B2, 16 November 2010. Available online: https://patentimages.storage.googleapis.com/80/28/dc/9bbdd279400d25/US7833482.pdf (accessed on 11 September 2024).
- Pranti, A.S.; Loof, D.; Kunz, S.; Zielasek, V.; Bäumer, M.; Lang, W. Design and Fabrication Challenges of a Highly Sensitive Thermoelectric-Based Hydrogen Gas Sensor. Micromachines 2019, 10, 650. [Google Scholar] [CrossRef] [PubMed]
- FIGARO TGS 821—Special Sensor for Hydrogen Gas. Available online: https://Www.Figarosensor.Com/Product/Docs/TGS821.Pdf2024 (accessed on 11 September 2024).
- Ji, H.; Zeng, W.; Li, Y. Gas Sensing Mechanisms of Metal Oxide Semiconductors: A Focus Review. Nanoscale 2019, 11, 22664–22684. [Google Scholar] [CrossRef] [PubMed]
- Phanichphant, S. Semiconductor Metal Oxides as Hydrogen Gas Sensors. Procedia Eng. 2014, 87, 795–802. [Google Scholar] [CrossRef]
- Trinchi, A.; Kandasamy, S.; Wlodarski, W. High Temperature Field Effect Hydrogen and Hydrocarbon Gas Sensors Based on SiC MOS Devices. Sens. Actuators B Chem. 2008, 133, 705–716. [Google Scholar] [CrossRef]
- Currie, J.F.; Essalik, A.; Marusic, J. Micromachined Thin Film Solid State Electrochemical CO2, NO2 and SO2 Gas Sensors. Sens. Actuators B Chem. 1999, 59, 235–241. [Google Scholar] [CrossRef]
- EC Sense ES1-H2-5%. 2024. Available online: https://Ecsense.Com/Product/Es1-H2-5-Hydrogen-Gas-Sensor/ (accessed on 11 September 2024).
- Posifa Technologies MEMS THERMAL CONDUCTIVITY HYDROGEN SENSORS. Available online: https://posifatech.com/wp-content/uploads/2020/11/Datasheet_PGS1000_MEMS_TC_H2_RevA_C0.5.pdf (accessed on 11 September 2024).
- De Graaf, G.; Wolffenbuttel, R. Surface-Micromachined Thermal Conductivity Detectors for Gas Sensing. In Proceedings of the 2012 IEEE International Instrumentation and Measurement Technology Conference Proceedings, Graz, Austria, 13–16 May 2012; pp. 1861–1864. [Google Scholar]
- Tu, X.Z. Single Silicon Wafer Micromachined Thermal Conduction Sensor. U.S. Patent 9,580,305 B2, 28 February 2017. Available online: https://patentimages.storage.googleapis.com/34/71/6f/50dc76a7453f1d/US9580305.pdf (accessed on 11 September 2024).
- Hübert, T.; Boon-Brett, L.; Black, G.; Banach, U. Hydrogen Sensors–a Review. Sens. Actuators B Chem. 2011, 157, 329–352. [Google Scholar] [CrossRef]
- Yu, Z.; Berding, M.; Krishnamurthy, S. Spin Transport in Organics and Organic Spin Devices. IEE Proc.-Circuits Devices Syst. 2005, 152, 334–339. [Google Scholar] [CrossRef]
- Liu, K.; Ouyang, B.; Guo, X.; Guo, Y.; Liu, Y. Advances in Flexible Organic Field-Effect Transistors and Their Applications for Flexible Electronics. Npj Flex. Electron. 2022, 6, 1. [Google Scholar] [CrossRef]
- Rath, R.J.; Farajikhah, S.; Oveissi, F.; Dehghani, F.; Naficy, S. Chemiresistive Sensor Arrays for Gas/Volatile Organic Compounds Monitoring: A Review. Adv. Eng. Mater. 2023, 25, 2200830. [Google Scholar] [CrossRef]
- Chunhong, D.; Guangzhong, X.; Junsheng, Y. Fabrication and Properties of an OLED-Based Gas Sensor with ZnPc Sensing Film. In Proceedings of the IEEE 10th International Conference on Electronic Measurement & Instruments, Chengdu, China, 16–19 August 2011; Volume 1, pp. 164–167. [Google Scholar]
- Xie, G.; Jiang, Y.; Du, X.; Tai, H.; Li, W. P1. 8.8 Fabrication and Properties of an OLED-Based Gas Sensor with Poly (3-Hexylthiophene) Sensing Film. In Proceedings of the 14th International Meeting on Chemical Sensors—IMCS 2012, Nuremberg, Germany, 20–23 May 2012; pp. 1130–1133. [Google Scholar]
- Adachi, C.; Xie, G.; Reineke, S.; Zysman-Colman, E. Recent Advances in Thermally Activated Delayed Fluorescence Materials. Front. Chem. 2020, 8, 625910. [Google Scholar] [CrossRef] [PubMed]
- He, J.; Yan, X.; Liu, A.; You, R.; Liu, F.; Li, S.; Wang, J.; Wang, C.; Sun, P.; Yan, X. A Rapid-Response Room-Temperature Planar Type Gas Sensor Based on DPA-Ph-DBPzDCN for the Sensitive Detection of NH 3. J. Mater. Chem. A 2019, 7, 4744–4750. [Google Scholar] [CrossRef]
- He, J.; Liang, B.; Yan, X.; Liu, F.; Wang, J.; Yang, Z.; You, R.; Wang, C.; Sun, P.; Yan, X. A TPA-DCPP Organic Semiconductor Film-Based Room Temperature NH3 Sensor for Insight into the Sensing Properties. Sens. Actuators B Chem. 2021, 327, 128940. [Google Scholar] [CrossRef]
- Kalita, A.; Hussain, S.; Malik, A.H.; Subbarao, N.V.; Iyer, P.K. Vapor Phase Sensing of Ammonia at the Sub-Ppm Level Using a Perylene Diimide Thin Film Device. J. Mater. Chem. C 2015, 3, 10767–10774. [Google Scholar] [CrossRef]
- Li, L.; Gao, P.; Baumgarten, M.; Müllen, K.; Lu, N.; Fuchs, H.; Chi, L. High Performance Field-effect Ammonia Sensors Based on a Structured Ultrathin Organic Semiconductor Film. Adv. Mater. 2013, 25, 3419–3425. [Google Scholar] [CrossRef]
- Xiao, X.; Cheng, X.; Hou, X.; He, J.; Xu, Q.; Li, H.; Li, N.; Chen, D.; Lu, J. Ion-in-conjugation: Squaraine as an Ultrasensitive Ammonia Sensor Material. Small 2017, 13, 1602190. [Google Scholar] [CrossRef]
- Zang, Y.; Zhang, F.; Huang, D.; Di, C.; Meng, Q.; Gao, X.; Zhu, D. Specific and Reproducible Gas Sensors Utilizing Gas-phase Chemical Reaction on Organic Transistors. Adv. Mater. 2014, 26, 2862–2867. [Google Scholar] [CrossRef]
- Shin, W.; Matsumiya, M.; Qiu, F.; Izu, N.; Murayama, N. Thermoelectric Gas Sensor for Detection of High Hydrogen Concentration. Sens. Actuators B Chem. 2004, 97, 344–347. [Google Scholar] [CrossRef]
- Koo, W.-T.; Cho, H.-J.; Kim, D.-H.; Kim, Y.H.; Shin, H.; Penner, R.M.; Kim, I.-D. Chemiresistive Hydrogen Sensors: Fundamentals, Recent Advances, and Challenges. ACS Nano 2020, 14, 14284–14322. [Google Scholar] [CrossRef]
- Pei, Y.; Chen, F.; Jiao, J.; Liu, S. Analysis and Control Strategy Design for PEMFC Purging Process. Energy 2024, 290, 130233. [Google Scholar] [CrossRef]
- Gao, Y.; Lin, M. Research on the Performance Characteristics of Hydrogen Circulation Pumps for PEMFC Vehicles. Int. J. Hydrogen Energy 2023, 50, 1255–1272. [Google Scholar] [CrossRef]
- Lee, Y.-A.; Han, S.; An, H.; Park, J.; Singh, R.; Kim, H.Y.; Seo, H. Confined Interfacial Alloying of Multilayered Pd-Ni Nanocatalyst for Widening Hydrogen Detection Capacity. Sens. Actuators B Chem. 2021, 330, 129378. [Google Scholar] [CrossRef]
- Gong, J.; Wang, Z.; Tang, Y.; Sun, J.; Wei, X.; Zhang, Q.; Tian, G.; Wang, H. MEMS-Based Resistive Hydrogen Sensor with High Performance Using a Palladium-Gold Alloy Thin Film. J. Alloys Compd. 2023, 930, 167398. [Google Scholar] [CrossRef]
- Yoon, J.-H.; Kim, B.-J.; Kim, J.-S. Design and Fabrication of Micro Hydrogen Gas Sensors Using Palladium Thin Film. Mater. Chem. Phys. 2012, 133, 987–991. [Google Scholar] [CrossRef]
- Hoffmann, M.; Wienecke, M.; Lengert, M.; Weidner, M.H.; Heeg, J. Palladium Based Mems Hydrogen Sensors. In Proceedings of the 2023 IEEE 36th International Conference on Micro Electro Mechanical Systems (MEMS), Munich, Germany, 15–19 January 2023; pp. 822–825. [Google Scholar]
- Wu, H.; Kustra, S.; Gates, E.M.; Bettinger, C.J. Topographic Substrates as Strain Relief Features in Stretchable Organic Thin Film Transistors. Org. Electron. 2013, 14, 1636–1642. [Google Scholar] [CrossRef]
- Yan, H.; Chen, Z.; Zheng, Y.; Newman, C.; Quinn, J.R.; Dötz, F.; Kastler, M.; Facchetti, A. A High-Mobility Electron-Transporting Polymer for Printed Transistors. Nature 2009, 457, 679–686. [Google Scholar] [CrossRef]
- Siegel, A.C.; Phillips, S.T.; Dickey, M.D.; Lu, N.; Suo, Z.; Whitesides, G.M. Foldable Printed Circuit Boards on Paper Substrates. Adv. Funct. Mater. 2010, 20, 28–35. [Google Scholar] [CrossRef]
- Sasabe, H.; Kido, J. Development of High Performance OLEDs for General Lighting. J. Mater. Chem. C 2013, 1, 1699–1707. [Google Scholar] [CrossRef]
- Kaltenbrunner, M.; White, M.S.; Głowacki, E.D.; Sekitani, T.; Someya, T.; Sariciftci, N.S.; Bauer, S. Ultrathin and Lightweight Organic Solar Cells with High Flexibility. Nat. Commun. 2012, 3, 770. [Google Scholar] [CrossRef]
- Kim, O.; Yoo, S.J.; Kim, J.Y.; Cho, S.K.; Park, H.S.; Lee, S.Y.; Seo, B.; Jang, J.H.; Lim, K.H.; Park, H.-Y. Impact of Fuel Starvation–Induced Anode Carbon Corrosion in Proton Exchange Membrane Fuel Cells on the Structure of the Membrane Electrode Assembly and Exhaust Gas Emissions: A Quantitative Case Study. J. Power Sources 2024, 615, 235032. [Google Scholar] [CrossRef]
- Yang, B.; Li, J.; Li, Y.; Guo, Z.; Zeng, K.; Shu, H.; Cao, P.; Ren, Y. A Critical Survey of Proton Exchange Membrane Fuel Cell System Control: Summaries, Advances, and Perspectives. Int. J. Hydrogen Energy 2022, 47, 9986–10020. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, H.; He, S.; Wang, W.; Gao, M.; Moiseevna, K.E.; Anatolievna, V.V. Dynamic Analysis and Control Optimization of Hydrogen Supply for the Proton Exchange Membrane Fuel Cell and Metal Hydride Coupling System with a Hydrogen Buffer Tank. Energy Convers. Manag. 2023, 291, 117339. [Google Scholar] [CrossRef]
- Li, X.; Qi, Y.; Li, S.; Tunestål, P.; Andersson, M. A Multi-input and Single-output Voltage Control for a Polymer Electrolyte Fuel Cell System Using Model Predictive Control Method. Int. J. Energy Res. 2021, 45, 12854–12863. [Google Scholar] [CrossRef]
- Kamal, E.; Aitouche, A. Fuzzy Observer-Based Fault Tolerant Control against Sensor Faults for Proton Exchange Membrane Fuel Cells. Int. J. Hydrogen Energy 2020, 45, 11220–11232. [Google Scholar] [CrossRef]
- Fu, H.; Shen, J.; Sun, L.; Lee, K.Y. In-Depth Characteristic Analysis and Wide Range Optimal Operation of Fuel Cell Using Multi-Model Predictive Control. Energy 2021, 234, 121226. [Google Scholar] [CrossRef]
- Mei, J.; Meng, X.; Tang, X.; Li, H.; Hasanien, H.; Alharbi, M.; Dong, Z.; Shen, J.; Sun, C.; Fan, F. An Accurate Parameter Estimation Method of the Voltage Model for Proton Exchange Membrane Fuel Cells. Energies 2024, 17, 2917. [Google Scholar] [CrossRef]
- Yuan, H.; Dai, H.; Wei, X.; Ming, P. Model-Based Observers for Internal States Estimation and Control of Proton Exchange Membrane Fuel Cell System: A Review. J. Power Sources 2020, 468, 228376. [Google Scholar] [CrossRef]
- Liang, W.; Liu, J.; Li, J.; Zhao, W.; Ao, C.; Wang, X.; Sun, P.; Ji, Q. Optimization Design of Proton Exchange Membrane Fuel Cell Cooling Plate Based on Dual-Objective Function Topology Theory. Int. Commun. Heat Mass Transf. 2024, 153, 107404. [Google Scholar] [CrossRef]
- Li, J.; Wu, T.; Cheng, C.; Li, J.; Zhou, K. A Review of the Research Progress and Application of Key Components in the Hydrogen Fuel Cell System. Processes 2024, 12, 249. [Google Scholar] [CrossRef]
- Muñoz-Perales, V.; Van der Heijden, M.; García-Salaberri, P.A.; Vera, M.; Forner-Cuenca, A. Engineering Lung-Inspired Flow Field Geometries for Electrochemical Flow Cells with Stereolithography 3D Printing. ACS Sustain. Chem. Eng. 2023, 11, 12243–12255. [Google Scholar] [CrossRef]
- Becker, H.; Murawski, J.; Shinde, D.V.; Stephens, I.E.; Hinds, G.; Smith, G. Impact of Impurities on Water Electrolysis: A Review. Sustain. Energy Fuels 2023, 7, 1565–1603. [Google Scholar] [CrossRef]
- Zhang, B.; Zhang, S.-X.; Yao, R.; Wu, Y.-H.; Qiu, J.-S. Progress and Prospects of Hydrogen Production: Opportunities and Challenges. J. Electron. Sci. Technol. 2021, 19, 100080. [Google Scholar] [CrossRef]
- Peterson, D. DOE Hydrogen and Fuel Cells Program Record. Reversible Fuel Cell Targets, Record 20001, 23 June 2020. Available online: https://www.hydrogen.energy.gov/docs/hydrogenprogramlibraries/pdfs/20001-reversible-fuel-cell-targets.pdf?sfvrsn=1ac6de44_1 (accessed on 11 September 2024).
- Flanagan, T.B.; Oates, W. The Palladium-Hydrogen System. Annu. Rev. Mater. Sci. 1991, 21, 269–304. [Google Scholar] [CrossRef]
- Das, S.S.; Kopnov, G.; Gerber, A. Positive vs Negative Resistance Response to Hydrogenation in Palladium and Its Alloys. AIP Adv. 2020, 10, 065129. [Google Scholar] [CrossRef]
- Adams, B.D.; Chen, A. The Role of Palladium in a Hydrogen Economy. Mater. Today 2011, 14, 282–289. [Google Scholar] [CrossRef]
- Nemanič, V. Hydrogen Permeation Barriers: Basic Requirements, Materials Selection, Deposition Methods, and Quality Evaluation. Nucl. Mater. Energy 2019, 19, 451–457. [Google Scholar] [CrossRef]
- Li, Y.; Barzagli, F.; Liu, P.; Zhang, X.; Yang, Z.; Xiao, M.; Huang, Y.; Luo, X.; Li, C.; Luo, H. Mechanism and Evaluation of Hydrogen Permeation Barriers: A Critical Review. Ind. Eng. Chem. Res. 2023, 62, 15752–15773. [Google Scholar] [CrossRef]
- Wagner, S.; Pundt, A. Electrical Resistivity and Hydrogen Solubility of PdHc Thin Films. Acta Mater. 2010, 58, 1387–1394. [Google Scholar] [CrossRef]
- Das, S.S.; Kopnov, G.; Gerber, A. Kinetics of the Lattice Response to Hydrogen Absorption in Thin Pd and CoPd Films. Molecules 2020, 25, 3597. [Google Scholar] [CrossRef]
- Sharif, P.; Alemdar, E.; Ozturk, S.; Caylan, O.; Haciefendioglu, T.; Buke, G.; Aydemir, M.; Danos, A.; Monkman, A.P.; Yildirim, E. Rational Molecular Design Enables Efficient Blue TADF− OLEDs with Flexible Graphene Substrate. Adv. Funct. Mater. 2022, 32, 2207324. [Google Scholar] [CrossRef]
- Cole, C.M.; Kunz, S.V.; Baumann, T.; Blinco, J.P.; Sonar, P.; Barner-Kowollik, C.; Yambem, S.D. Flexible Ink-Jet Printed Polymer Light-Emitting Diodes Using a Self-Hosted Non-Conjugated TADF Polymer. Macromol. Rapid Commun. 2023, 44, 2300015. [Google Scholar] [CrossRef] [PubMed]
- Wu, F.; Zhao, X.; Zhu, H.; Tang, X.; Ning, Y.; Chen, J.; Chen, X.; Xiong, Z. Identifying the Exciplex-to-Exciplex Energy Transfer in Tricomponent Exciplex-Based OLEDs through Magnetic Field Effect Measurements. ACS Photonics 2022, 9, 2713–2723. [Google Scholar] [CrossRef]
- Nguyen, T.; Sheng, Y.; Wohlgenannt, M.; Anthopoulos, T. On the Role of Hydrogen in Organic Magnetoresistance: A Study of C60 Devices. Synth. Met. 2007, 157, 930–934. [Google Scholar] [CrossRef]
- Deng, J.; Jia, W.; Chen, Y.; Liu, D.; Hu, Y.; Xiong, Z. Guest Concentration, Bias Current, and Temperature-Dependent Sign Inversion of Magneto-Electroluminescence in Thermally Activated Delayed Fluorescence Devices. Sci. Rep. 2017, 7, 44396. [Google Scholar] [CrossRef]
- Braun, F.; Scharff, T.; Bange, S.; Jiang, W.; Darwish, T.A.; Burn, P.L.; Mkhitaryan, V.V.; Lupton, J.M. Low-Temperature Magnetoelectroluminescence of Organic Light-Emitting Diodes: Separating Excitonic Effects from Carrier-Pair Singlet-Triplet Mixing. Phys. Rev. B 2024, 110, 014204. [Google Scholar] [CrossRef]
- Weber, D.; Morgenstern, A.; Beer, D.; Zahn, D.R.; Deibel, C.; Salvan, G.; Schondelmaier, D. Exciplex-Driven Blue OLEDs: Unlocking Multifunctionality Applications. Appl. Phys. A 2024, 130, 412. [Google Scholar] [CrossRef]
- Tanioka, A.; Fukushima, N.; Hasegawa, K.; Miyasaka, K.; Takahashi, N. Permeation of Gases across the Poly (Chloro-p-xylylene) Membrane. J. Appl. Polym. Sci. 1994, 54, 219–229. [Google Scholar] [CrossRef]
- Bian, L.; Shu, Y.; Wang, X. A Molecular Dynamics Study on Permeability of Gases through Parylene AF8 Membranes. Polym. Adv. Technol. 2012, 23, 1520–1528. [Google Scholar] [CrossRef]
- Ortigoza-Diaz, J.; Scholten, K.; Larson, C.; Cobo, A.; Hudson, T.; Yoo, J.; Baldwin, A.; Weltman Hirschberg, A.; Meng, E. Techniques and Considerations in the Microfabrication of Parylene C Microelectromechanical Systems. Micromachines 2018, 9, 422. [Google Scholar] [CrossRef]
- Lu, Q.; Yang, Z.; Meng, X.; Yue, Y.; Ahmad, M.A.; Zhang, W.; Zhang, S.; Zhang, Y.; Liu, Z.; Chen, W. A Review on Encapsulation Technology from Organic Light Emitting Diodes to Organic and Perovskite Solar Cells. Adv. Funct. Mater. 2021, 31, 2100151. [Google Scholar] [CrossRef]
- Tankelevičiūtė, E.; Samuel, I.D.; Zysman-Colman, E. The Blue Problem: OLED Stability and Degradation Mechanisms. J. Phys. Chem. Lett. 2024, 15, 1034–1047. [Google Scholar] [CrossRef] [PubMed]
- Zhu, X.-D.; Tian, Q.-S.; Zheng, Q.; Wang, Y.-K.; Yuan, Y.; Li, Y.; Jiang, Z.-Q.; Liao, L.-S. Deep-Blue Thermally Activated Delayed Fluorescence Materials with High Glass Transition Temperature. J. Lumin. 2019, 206, 146–153. [Google Scholar] [CrossRef]
- Tavgeniene, D.; Beresneviciute, R.; Blazevicius, D.; Krucaite, G.; Jacunskaite, G.; Sudheendran Swayamprabha, S.; Jou, J.-H.; Grigalevicius, S. 3-(N, N-Diphenylamino) Carbazole Donor Containing Bipolar Derivatives with Very High Glass Transition Temperatures as Potential TADF Emitters for OLEDs. Coatings 2022, 12, 932. [Google Scholar] [CrossRef]
- Keller, N. Beitrag Zur Methodischen Auslegung von Polymerelektrolytmembran-Brennstoffzellensystemen. Univ. Chemnitz 2023, 1, 277–289. [Google Scholar] [CrossRef]
- Hasanpour, S.; Rashidi, A.; Walsh, T.; Pagan, E.; Milani, A.S.; Akbari, M.; Djilali, N. Electrode-Integrated Textile-Based Sensors for in Situ Temperature and Relative Humidity Monitoring in Electrochemical Cells. ACS Omega 2021, 6, 9509–9519. [Google Scholar] [CrossRef]
- Foucart, S.; Rauhut, H.; Foucart, S.; Rauhut, H. An Invitation to Compressive Sensing; Springer: Berlin/Heidelberg, Germany, 2013; ISBN 0-8176-4947-6. [Google Scholar]
- Nagel, N.; Schäfer, M.; Ullrich, T. A New Upper Bound for Sampling Numbers. Found. Comput. Math. 2022, 22, 445–468. [Google Scholar] [CrossRef]
- Dolbeault, M.; Krieg, D.; Ullrich, M. A Sharp Upper Bound for Sampling Numbers in L2. Appl. Comput. Harmon. Anal. 2023, 63, 113–134. [Google Scholar] [CrossRef]
- Bartel, F.; Lüttgen, K.; Nagel, N.; Ullrich, T. Efficient Recovery of Non-Periodic Multivariate Functions from Few Scattered Samples. arXiv 2023, arXiv:230607140. [Google Scholar]
- Bartel, F.; Schäfer, M.; Ullrich, T. Constructive Subsampling of Finite Frames with Applications in Optimal Function Recovery. Appl. Comput. Harmon. Anal. 2023, 65, 209–248. [Google Scholar] [CrossRef]
- Krieg, D.; Ullrich, M. Function Values Are Enough for L_2 L 2-Approximation. Found. Comput. Math. 2021, 21, 1141–1151. [Google Scholar] [CrossRef]
- Feng, J.; Xing, L.; Wang, B.; Wei, H.; Xing, Z. Effects of Working Fluids on the Performance of a Roots Pump for Hydrogen Recirculation in a PEM Fuel Cell System. Appl. Sci. 2020, 10, 8069. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hauck, M.; Bickmann, C.; Morgenstern, A.; Nagel, N.; Meinecke, C.R.; Schade, A.; Tafat, R.; Viriato, L.; Kuhn, H.; Salvan, G.; et al. Perspective on the Development and Integration of Hydrogen Sensors for Fuel Cell Control. Energies 2024, 17, 5158. https://doi.org/10.3390/en17205158
Hauck M, Bickmann C, Morgenstern A, Nagel N, Meinecke CR, Schade A, Tafat R, Viriato L, Kuhn H, Salvan G, et al. Perspective on the Development and Integration of Hydrogen Sensors for Fuel Cell Control. Energies. 2024; 17(20):5158. https://doi.org/10.3390/en17205158
Chicago/Turabian StyleHauck, Michael, Christopher Bickmann, Annika Morgenstern, Nicolas Nagel, Christoph R. Meinecke, Alexander Schade, Rania Tafat, Lucas Viriato, Harald Kuhn, Georgeta Salvan, and et al. 2024. "Perspective on the Development and Integration of Hydrogen Sensors for Fuel Cell Control" Energies 17, no. 20: 5158. https://doi.org/10.3390/en17205158
APA StyleHauck, M., Bickmann, C., Morgenstern, A., Nagel, N., Meinecke, C. R., Schade, A., Tafat, R., Viriato, L., Kuhn, H., Salvan, G., Schondelmaier, D., Ullrich, T., Unwerth, T. v., & Streif, S. (2024). Perspective on the Development and Integration of Hydrogen Sensors for Fuel Cell Control. Energies, 17(20), 5158. https://doi.org/10.3390/en17205158







