Selecting an Installation Site for MW-Scale Water Electrolysis Systems Based on Grid Voltage Stability
Abstract
1. Introduction
2. Considerations to Select Optimal Electrolysis Connection Locations
2.1. Required Specifications for the Electrolysis System Operation
2.1.1. Electrolyzer Stack
2.1.2. Electrical Balance of Plant
2.1.3. Mechanical Balance of Plant
2.2. Fault in the Power Transmission System
2.3. Calcutate the Power Load Margin in Voltage Stability to Maintain the Operation of the Electrolysis System
- : load margin based on voltage stability.
- : maximum load point after renewable energy is disconnected in the grid system.
- : operating load oint after renewable energy is disconnected in the grid system.
2.4. Calculate the Connectable Power Margin in the Power Load Margin and Power Supply Margin of the Distribution System
- : power supply margin.
- : permissible power of power cable line.
- : total load power of a feeder line in a power distribution system.
3. Simulation of the Proposed Optimal Location Selection Method for Electrolysis System
3.1. Simulation Model
3.1.1. Load Model for the Electrolysis System
3.1.2. Power Distribution System Model
3.1.3. Power Transmission System Model
3.2. Simulation Results
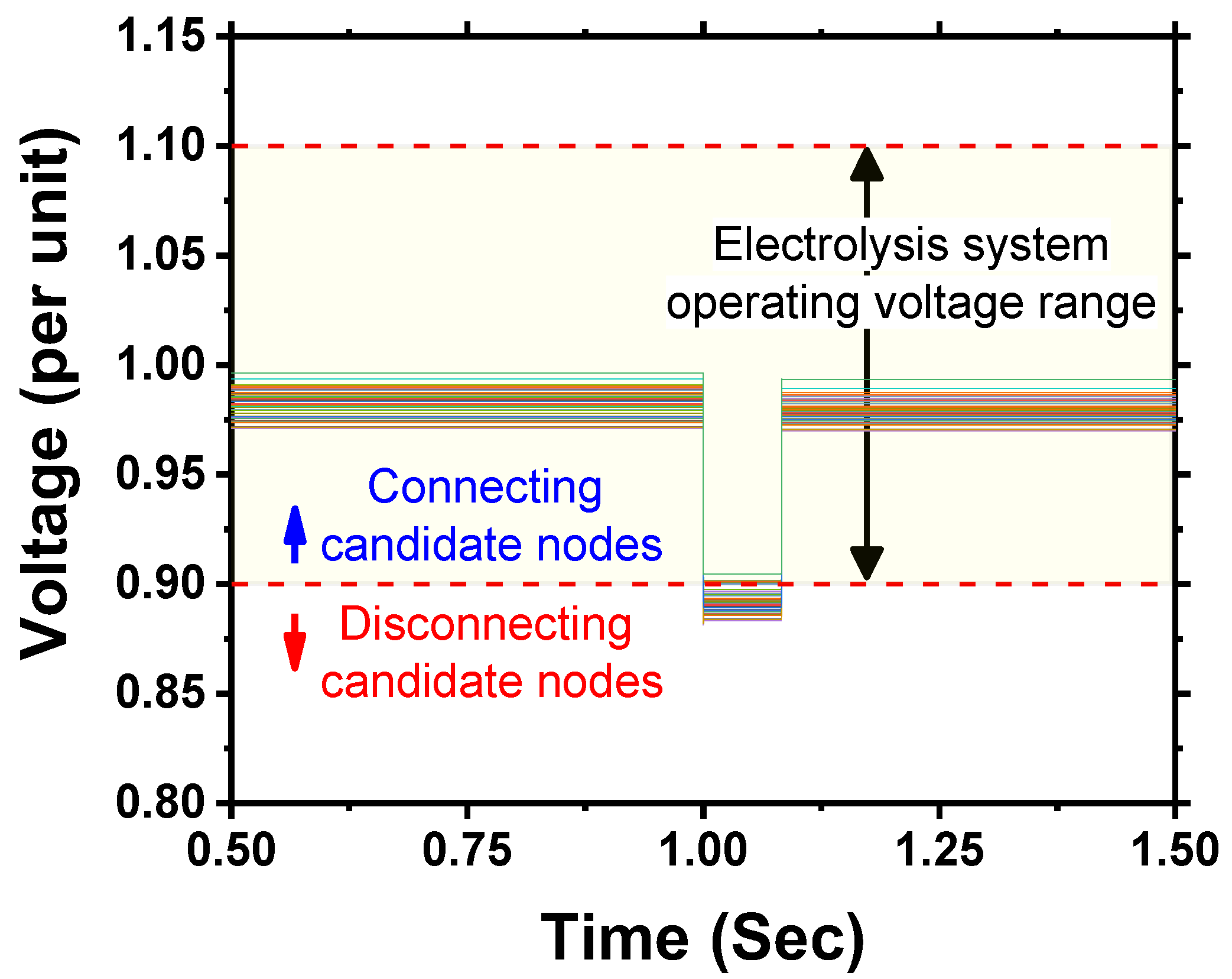
3.2.1. Low-Voltage Occurrence of the Distribution System by a Transmission System Fault
3.2.2. Calculation of Connectable Power Margin
3.2.3. Optimal Connection Selection Results for the Electrolysis System
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Züttel, A. Materials for Hydrogen Storage. Mater. Today 2003, 6, 24–33. [Google Scholar] [CrossRef]
- Eberle, U.; Felderhoff, M.; Schüth, F. Chemical and Physical Solutions for Hydrogen Storage. Angew. Chem. Int. Ed. 2009, 48, 6608–6630. [Google Scholar] [CrossRef]
- Barthelemy, H.; Weber, M.; Barbier, F. Hydrogen Storage: Recent Improvements and Industrial Perspectives. Int. J. Hydrogen Energy 2017, 42, 7254–7262. [Google Scholar] [CrossRef]
- Masson-Delmotte, V.; Zhai, P.; Pirani, A.; Connors, S.L.; Péan, C.; Berger, S.; Caud, N.; Chen, Y.; Goldfarb, L.; Gomis, M.I.; et al. Climate Change 2021: The Physical Science Basis; The Working Group I Contribution to the Sixth Assessment Report; IPCC: Geneva, Switzerland, 2021; Volume 2, p. 2391.
- Stott, P.A.; Christidis, N.; Otto, F.E.L.; Sun, Y.; Vanderlinden, J.-P.; van Oldenborgh, G.J.; Vautard, R.; von Storch, H.; Walton, P.; Yiou, P.; et al. Attribution of Extreme Weather and Climate-Related Events. WIREs Clim. Change 2016, 7, 23–41. [Google Scholar] [CrossRef] [PubMed]
- Loock, M. Unlocking the Value of Digitalization for the European Energy Transition: A Typology of Innovative Business Models. Energy Res. Soc. Sci. 2020, 69, 101740. [Google Scholar] [CrossRef]
- Vernay, A.-L.; Sebi, C.; Arroyo, F. Energy Community Business Models and Their Impact on the Energy Transition: Lessons Learnt from France. Energy Policy 2023, 175, 113473. [Google Scholar] [CrossRef]
- Bryant, S.T.; Straker, K.; Wrigley, C. The Discourses of Power—Governmental Approaches to Business Models in the Renewable Energy Transition. Energy Policy 2019, 130, 41–59. [Google Scholar] [CrossRef]
- Carley, S.; Konisky, D.M. The Justice and Equity Implications of the Clean Energy Transition. Nat. Energy 2020, 5, 569–577. [Google Scholar] [CrossRef]
- Carley, S.; Evans, T.P.; Graff, M.; Konisky, D.M. A Framework for Evaluating Geographic Disparities in Energy Transition Vulnerability. Nat. Energy 2018, 3, 621–627. [Google Scholar] [CrossRef]
- Meinshausen, M.; Lewis, J.; McGlade, C.; Gütschow, J.; Nicholls, Z.; Burdon, R.; Cozzi, L.; Hackmann, B. Realization of Paris Agreement Pledges May Limit Warming Just below 2 °C. Nature 2022, 604, 304–309. [Google Scholar] [CrossRef]
- International Energy Agency. Global Hydrogen Review 2022; International Energy Agency: Paris, France, 2022.
- Gan, L.; Jiang, P.; Lev, B.; Zhou, X. Balancing of Supply and Demand of Renewable Energy Power System: A Review and Bibliometric Analysis. Sustain. Futur. 2020, 2, 100013. [Google Scholar] [CrossRef]
- Mohamad, F.; Teh, J.; Lai, C.-M. Optimum Allocation of Battery Energy Storage Systems for Power Grid Enhanced with Solar Energy. Energy 2021, 223, 120105. [Google Scholar] [CrossRef]
- El-Bidairi, K.S.; Nguyen, H.D.; Mahmoud, T.S.; Jayasinghe, S.D.G.; Guerrero, J.M. Optimal Sizing of Battery Energy Storage Systems for Dynamic Frequency Control in an Islanded Microgrid: A Case Study of Flinders Island, Australia. Energy 2020, 195, 117059. [Google Scholar] [CrossRef]
- Immendoerfer, A.; Tietze, I.; Hottenroth, H.; Viere, T. Life-Cycle Impacts of Pumped Hydropower Storage and Battery Storage. Int. J. Energy Environ. Eng. 2017, 8, 231–245. [Google Scholar] [CrossRef]
- Mayyas, A.; Wei, M.; Levis, G. Hydrogen as a Long-Term, Large-Scale Energy Storage Solution When Coupled with Renewable Energy Sources or Grids with Dynamic Electricity Pricing Schemes. Int. J. Hydrogen Energy 2020, 45, 16311–16325. [Google Scholar] [CrossRef]
- Pijarski, P.; Kacejko, P. Voltage Optimization in MV Network with Distributed Generation Using Power Consumption Control in Electrolysis Installations. Energies 2021, 14, 993. [Google Scholar] [CrossRef]
- Idaho National Laboratory. Dynamic Modeling and Validation of Electrolyzers in Real Time Grid Simulation; Idaho National Laboratory: Idaho Falls, ID, USA, 2016.
- Cozzolino, R.; Bella, G. A Review of Electrolyzer-Based Systems Providing Grid Ancillary Services: Current Status, Market, Challenges and Future Directions. Front. Energy Res. 2024, 12, 1358333. [Google Scholar] [CrossRef]
- Petrollese, M.; Concas, G.; Lonis, F.; Cocco, D. Techno-Economic Assessment of Green Hydrogen Valley Providing Multiple End-Users. Int. J. Hydrogen Energy 2022, 47, 24121–24135. [Google Scholar] [CrossRef]
- Tsukase, N.; Araki, T.; Haleem, A.A.; Nagasawa, K.; Kuroda, Y.; Mitsushima, S. Numerical Simulation of the Distribution of Reverse Currents in a Practical Alkaline Water Electrolysis Stack Immediately after Electrolysis. Int. J. Hydrogen Energy 2024, 49, 701–712. [Google Scholar] [CrossRef]
- Abdel Haleem, A.; Huyan, J.; Nagasawa, K.; Kuroda, Y.; Nishiki, Y.; Kato, A.; Nakai, T.; Araki, T.; Mitsushima, S. Effects of Operation and Shutdown Parameters and Electrode Materials on the Reverse Current Phenomenon in Alkaline Water Analyzers. J. Power Sources 2022, 535, 231454. [Google Scholar] [CrossRef]
- Norazahar, N.; Khan, F.; Rahmani, N.; Ahmad, A. Degradation Modelling and Reliability Analysis of PEM Electrolyzer. Int. J. Hydrogen Energy 2024, 50, 842–856. [Google Scholar] [CrossRef]
- Kuhnert, E.; Hacker, V.; Bodner, M. A Review of Accelerated Stress Tests for Enhancing MEA Durability in PEM Water Electrolysis Cells. Int. J. Energy Res. 2023, 2023, 3183108. [Google Scholar] [CrossRef]
- Liu, C.; Wrubel, J.; Padgett, E.; Bender, G. The Impacts of Membrane Pinholes on PEM Water Electrolysis. J. Power Sources 2023, 581, 233507. [Google Scholar] [CrossRef]
- Liu, T.; Wang, X.; Jiang, X.; Deng, C.; Niu, S.; Mao, J.; Zeng, W.; Liu, M.; Liao, H. Mechanism of Corrosion and Sedimentation of Nickel Electrodes for Alkaline Water Electrolysis. Mater. Chem. Phys. 2023, 303, 127806. [Google Scholar] [CrossRef]
- Leng, Y.; Chen, G.; Mendoza, A.J.; Tighe, T.B.; Hickner, M.A.; Wang, C.-Y. Solid-State Water Electrolysis with an Alkaline Membrane. J. Am. Chem. Soc. 2012, 134, 9054–9057. [Google Scholar] [CrossRef]
- Operator, A.E.M. Black System Event in South Australia on 28 September 2016; AEMO: Melbourne, Australia, 2017. [Google Scholar]
- Meng, X.; Pian, Z. Chapter 4—Derivation of Distribution Network Vulnerability Indicators Based on Voltage Stability. In Intelligent Coordinated Control of Complex Uncertain Systems for Power Distribution Network Reliability; Meng, X., Pian, Z., Eds.; Elsevier: Oxford, UK, 2016; pp. 65–89. ISBN 978-0-12-849896-5. [Google Scholar]
- Shin, J.-R.; Kim, B.-S.; Park, J.-B.; Lee, K.Y. A New Optimal Routing Algorithm for Loss Minimization and Voltage Stability Improvement in Radial Power Systems. IEEE Trans. Power Syst. 2007, 22, 648–657. [Google Scholar] [CrossRef]
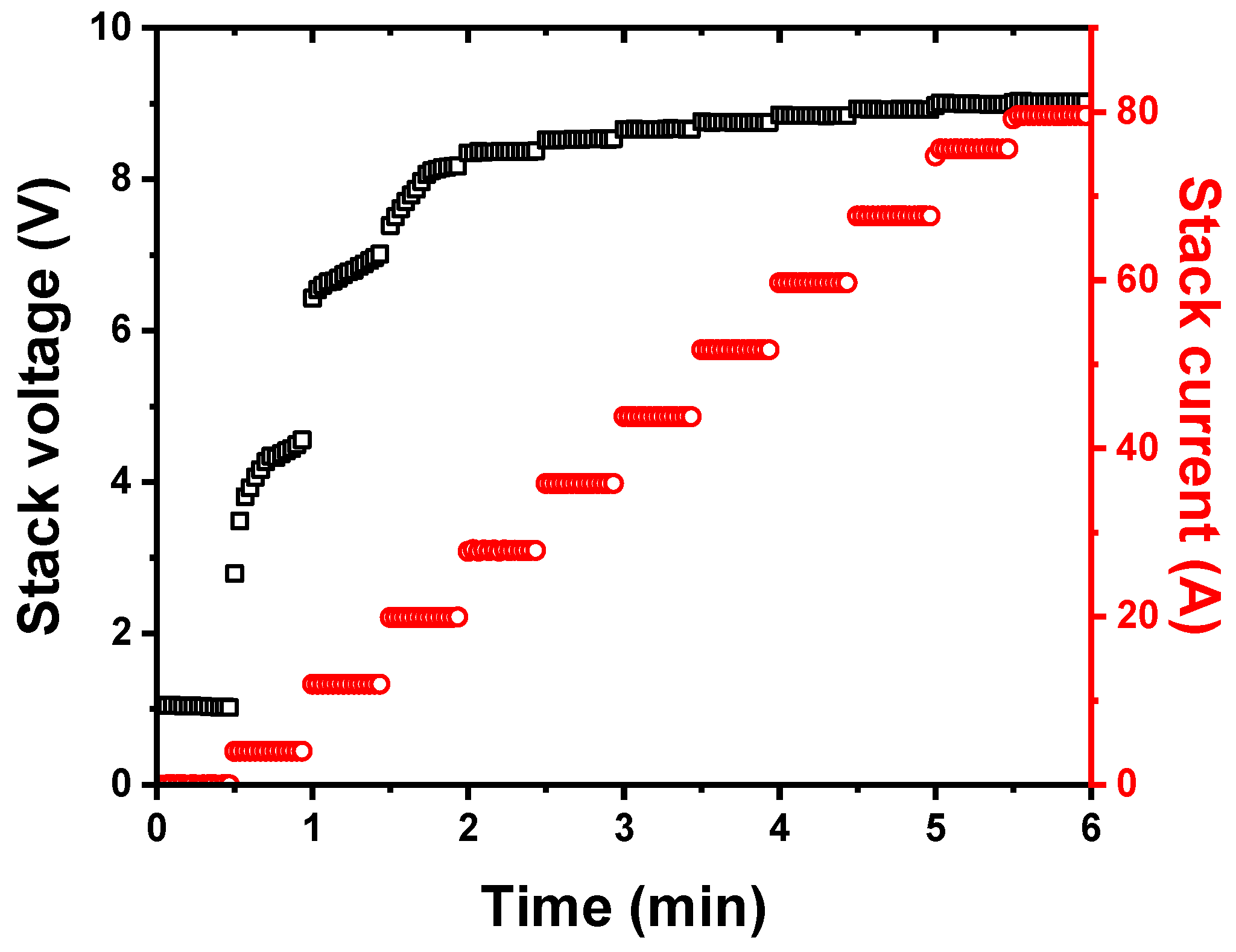
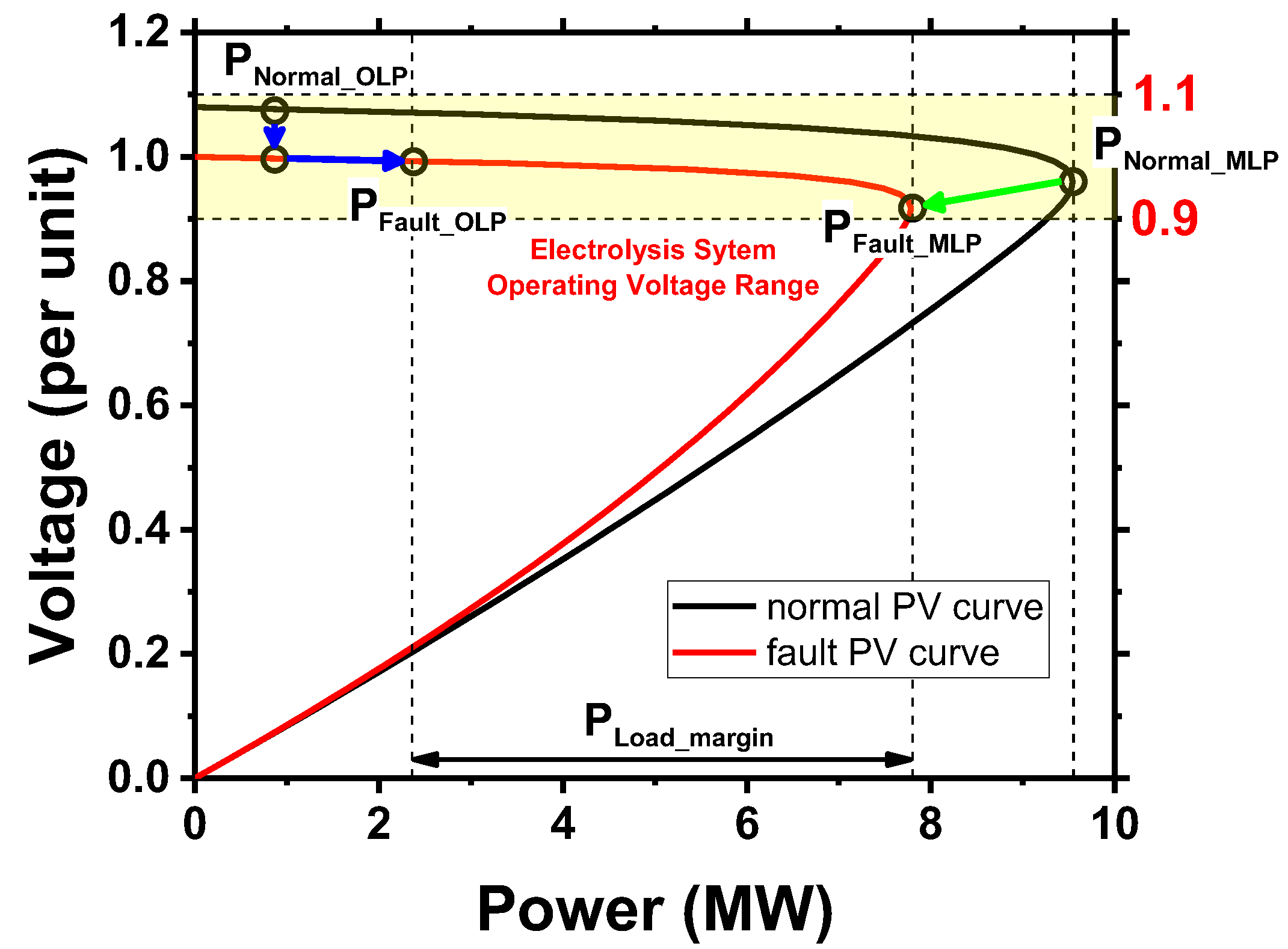

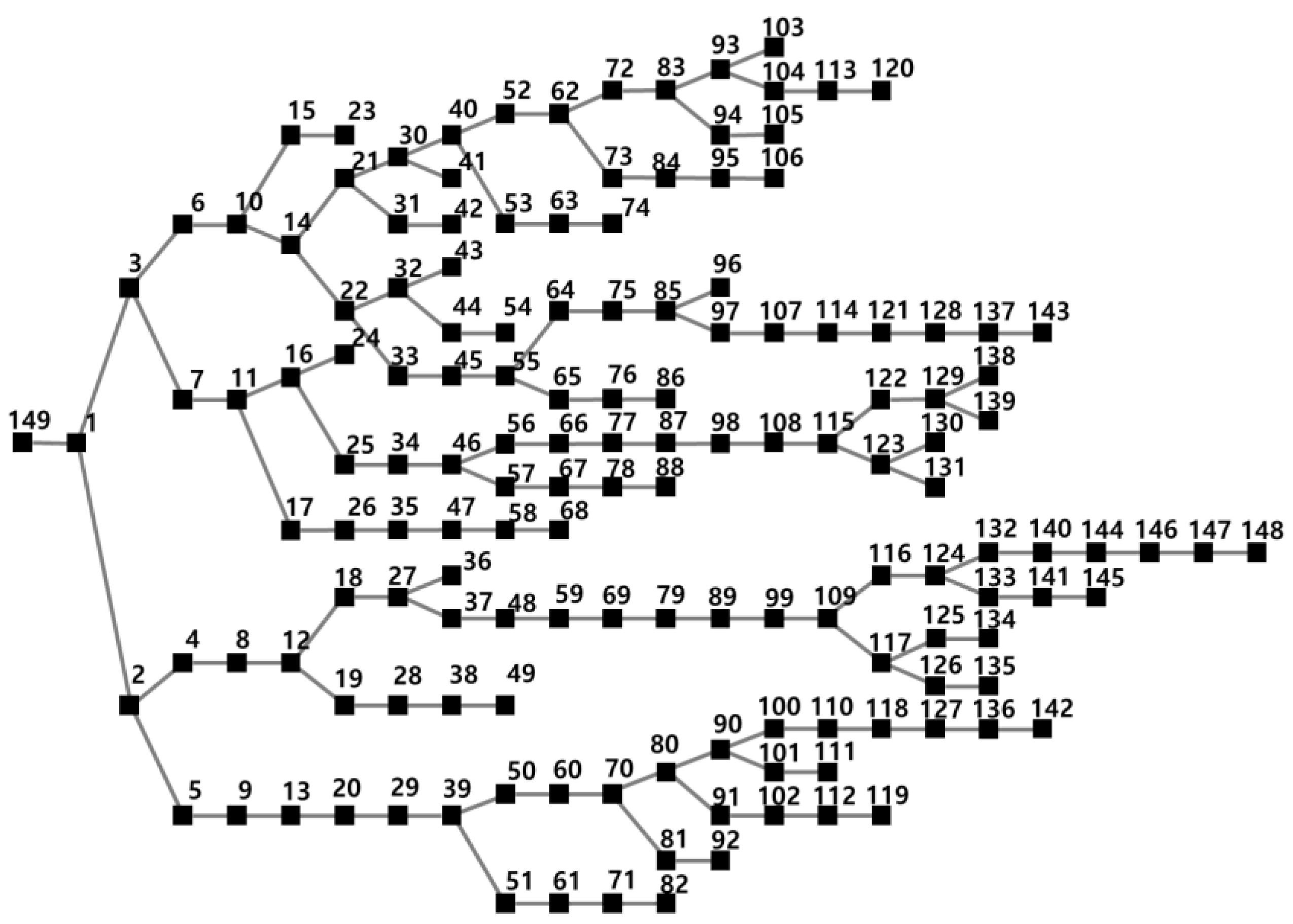

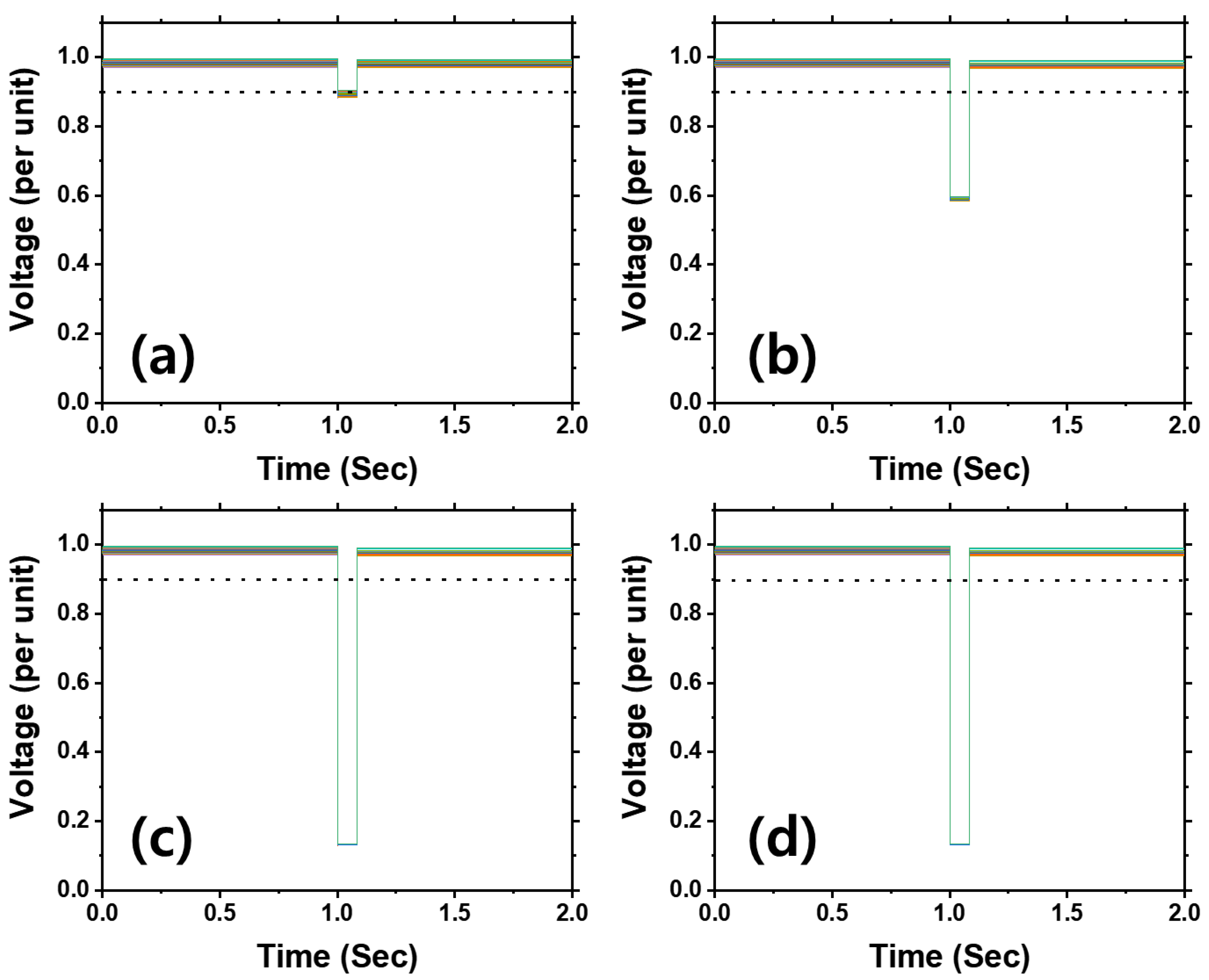

| Rated AC Power (kVA) | 600 |
| Rated AC Voltage (V) | 440 (3-Phase, 3-Wire) |
| Rated AC Current (A) | 787.3 |
| Rated Grid Frequency (Hz) | 60 |
| Grid Voltage operation range (V) | 396~484 (90~110% of rated voltage) |
| Grid Frequency operation range (Hz) | 60 ± 0.2 |
| Input Rated Voltage (VAC) | 380~480 (+10%~−15%, 3-Phase) |
| Input Rated Frequency (Hz) | 50~60 |
| Input Rated voltage (VAC) | 100~120, 200~240 |
| Input Rated Frequency (Hz) | 50~60 |
| Active Power (kW) | Reactive Power (kVar) | |
|---|---|---|
| Electrical Balance of Plant (included with tack) | 1015.22 | 45.44 |
| Mechanical Balance of Plant | 99.31 | 45.88 |
| Total | 1114.54 | 91.32 |
| Active Power (kW) | Reactive Power (kVAR) | Apparent Power (kVA) | |
|---|---|---|---|
| DL#1 | 4974.288 | 2400.288 | 5523.489 |
| DL#2 | 5271.843 | 2550.394 | 5855.512 |
| DL#3 | 4774.266 | 2318.778 | 5308.010 |
| DL#4 | 4786.223 | 2319.390 | 5318.624 |
| Bus No. | Power (MW) | DL | |
|---|---|---|---|
| Solar | 37 | 1.25 | #3 |
| Solar | 44 | 1.25 | #1 |
| Solar | 79 | 1.25 | #3 |
| Solar | 85 | 1.25 | #1 |
| Solar | 106 | 1.25 | #1 |
| Solar | 121 | 1.25 | #1 |
| Solar | 137 | 1.25 | #1 |
| Solar | 144 | 1.25 | #3 |
| Water Electrolyzer | 25 | 1 | #1 |
| Water Electrolyzer | 64 | 1 | #2 |
| Water Electrolyzer | 82 | 1 | #3 |
| Water Electrolyzer | 134 | 1 | #4 |
| Bus | Min. Voltage | Bus | Min. Voltage | Bus | Min. Voltage | Bus | Min. Voltage |
|---|---|---|---|---|---|---|---|
| 1 | 0.905 | 38 | 0.898 | 75 | 0.901 | 112 | 0.891 |
| 2 | 0.905 | 39 | 0.892 | 76 | 0.900 | 113 | 0.900 |
| 3 | 0.905 | 40 | 0.901 | 77 | 0.886 | 114 | 0.901 |
| 4 | 0.905 | 41 | 0.901 | 78 | 0.887 | 115 | 0.885 |
| 5 | 0.905 | 42 | 0.901 | 79 | 0.893 | 116 | 0.890 |
| 6 | 0.905 | 43 | 0.901 | 80 | 0.891 | 117 | 0.890 |
| 7 | 0.905 | 44 | 0.901 | 81 | 0.891 | 118 | 0.891 |
| 8 | 0.901 | 45 | 0.901 | 82 | 0.891 | 119 | 0.891 |
| 9 | 0.896 | 46 | 0.887 | 83 | 0.900 | 120 | 0.900 |
| 10 | 0.901 | 47 | 0.891 | 84 | 0.901 | 121 | 0.901 |
| 11 | 0.891 | 48 | 0.895 | 85 | 0.901 | 122 | 0.883 |
| 12 | 0.898 | 49 | 0.898 | 86 | 0.900 | 123 | 0.885 |
| 13 | 0.895 | 50 | 0.892 | 87 | 0.885 | 124 | 0.890 |
| 14 | 0.901 | 51 | 0.892 | 88 | 0.887 | 125 | 0.890 |
| 15 | 0.901 | 52 | 0.900 | 89 | 0.892 | 126 | 0.890 |
| 16 | 0.890 | 53 | 0.900 | 90 | 0.891 | 127 | 0.891 |
| 17 | 0.891 | 54 | 0.901 | 91 | 0.891 | 128 | 0.901 |
| 18 | 0.897 | 55 | 0.900 | 92 | 0.891 | 129 | 0.883 |
| 19 | 0.898 | 56 | 0.887 | 93 | 0.900 | 130 | 0.885 |
| 20 | 0.894 | 57 | 0.887 | 94 | 0.900 | 131 | 0.884 |
| 21 | 0.901 | 58 | 0.891 | 95 | 0.901 | 132 | 0.890 |
| 22 | 0.901 | 59 | 0.894 | 96 | 0.901 | 133 | 0.890 |
| 23 | 0.901 | 60 | 0.891 | 97 | 0.901 | 134 | 0.890 |
| 24 | 0.890 | 61 | 0.892 | 98 | 0.885 | 135 | 0.890 |
| 25 | 0.890 | 62 | 0.900 | 99 | 0.891 | 136 | 0.891 |
| 26 | 0.891 | 63 | 0.900 | 100 | 0.891 | 137 | 0.902 |
| 27 | 0.896 | 64 | 0.900 | 101 | 0.891 | 138 | 0.882 |
| 28 | 0.898 | 65 | 0.900 | 102 | 0.891 | 139 | 0.882 |
| 29 | 0.892 | 66 | 0.886 | 103 | 0.900 | 140 | 0.890 |
| 30 | 0.901 | 67 | 0.887 | 104 | 0.900 | 141 | 0.890 |
| 31 | 0.901 | 68 | 0.891 | 105 | 0.900 | 142 | 0.891 |
| 32 | 0.901 | 69 | 0.893 | 106 | 0.901 | 143 | 0.901 |
| 33 | 0.901 | 70 | 0.891 | 107 | 0.901 | 144 | 0.890 |
| 34 | 0.888 | 71 | 0.891 | 108 | 0.885 | 145 | 0.890 |
| 35 | 0.891 | 72 | 0.900 | 109 | 0.890 | 146 | 0.889 |
| 36 | 0.896 | 73 | 0.900 | 110 | 0.891 | 147 | 0.889 |
| 37 | 0.895 | 74 | 0.900 | 111 | 0.891 | 148 | 0.889 |
| DL | Bus | (MVA) | DL | Bus | (MVA) |
|---|---|---|---|---|---|
| #3 | 8 | 217.67 | #1 | 72 | 108.21 |
| #1 | 10 | 126.76 | #1 | 33 | 107.92 |
| #1 | 15 | 126.67 | #1 | 83 | 107.42 |
| #1 | 23 | 126.65 | #1 | 94 | 107.26 |
| #1 | 14 | 114.37 | #1 | 93 | 107.04 |
| #1 | 21 | 113.48 | #1 | 104 | 106.83 |
| #1 | 31 | 113.37 | #1 | 103 | 106.66 |
| #1 | 42 | 113.28 | #1 | 105 | 106.61 |
| #1 | 30 | 112.29 | #1 | 45 | 106.38 |
| #1 | 41 | 112.27 | #1 | 55 | 103.39 |
| #1 | 40 | 110.69 | #1 | 65 | 103.07 |
| #1 | 53 | 110.58 | #1 | 64 | 102.98 |
| #1 | 63 | 110.45 | #1 | 76 | 102.98 |
| #1 | 74 | 110.29 | #1 | 86 | 102.95 |
| #1 | 52 | 109.69 | #1 | 75 | 102.66 |
| #1 | 22 | 109.44 | #1 | 85 | 101.69 |
| #1 | 32 | 109.35 | #1 | 96 | 101.29 |
| #1 | 43 | 109.30 | #1 | 97 | 101.06 |
| #1 | 44 | 109.23 | #1 | 107 | 100.84 |
| #1 | 54 | 109.18 | #1 | 114 | 100.56 |
| #1 | 106 | 109.14 | #1 | 121 | 100.22 |
| #1 | 95 | 109.06 | #1 | 128 | 100.08 |
| #1 | 84 | 109.04 | #1 | 137 | 99.90 |
| #1 | 62 | 109.01 | #1 | 143 | 99.54 |
| #1 | 73 | 109.01 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, Y.-J.; Kang, M.; Kim, H.-T.; Kim, H.; Choi, S.; Choi, B.-B. Selecting an Installation Site for MW-Scale Water Electrolysis Systems Based on Grid Voltage Stability. Energies 2025, 18, 2037. https://doi.org/10.3390/en18082037
Kim Y-J, Kang M, Kim H-T, Kim H, Choi S, Choi B-B. Selecting an Installation Site for MW-Scale Water Electrolysis Systems Based on Grid Voltage Stability. Energies. 2025; 18(8):2037. https://doi.org/10.3390/en18082037
Chicago/Turabian StyleKim, Yong-Jung, Moonho Kang, Hyun-Tae Kim, Haelee Kim, Sungyun Choi, and Baeck-Bum Choi. 2025. "Selecting an Installation Site for MW-Scale Water Electrolysis Systems Based on Grid Voltage Stability" Energies 18, no. 8: 2037. https://doi.org/10.3390/en18082037
APA StyleKim, Y.-J., Kang, M., Kim, H.-T., Kim, H., Choi, S., & Choi, B.-B. (2025). Selecting an Installation Site for MW-Scale Water Electrolysis Systems Based on Grid Voltage Stability. Energies, 18(8), 2037. https://doi.org/10.3390/en18082037






