Research on Measures to Limit Short-Circuit Current by Changing the Structure of the Power Grid
Abstract
1. Introduction
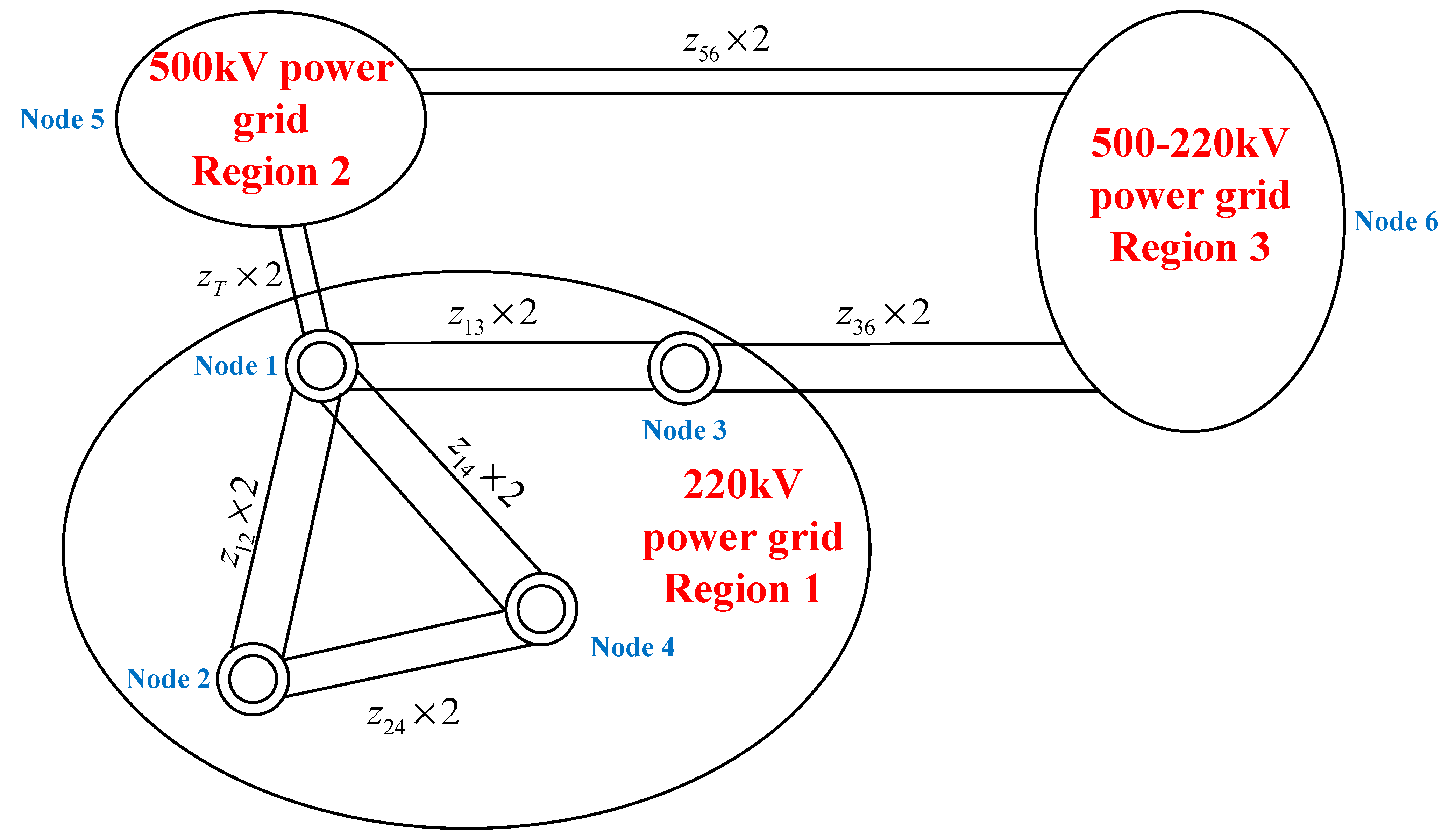
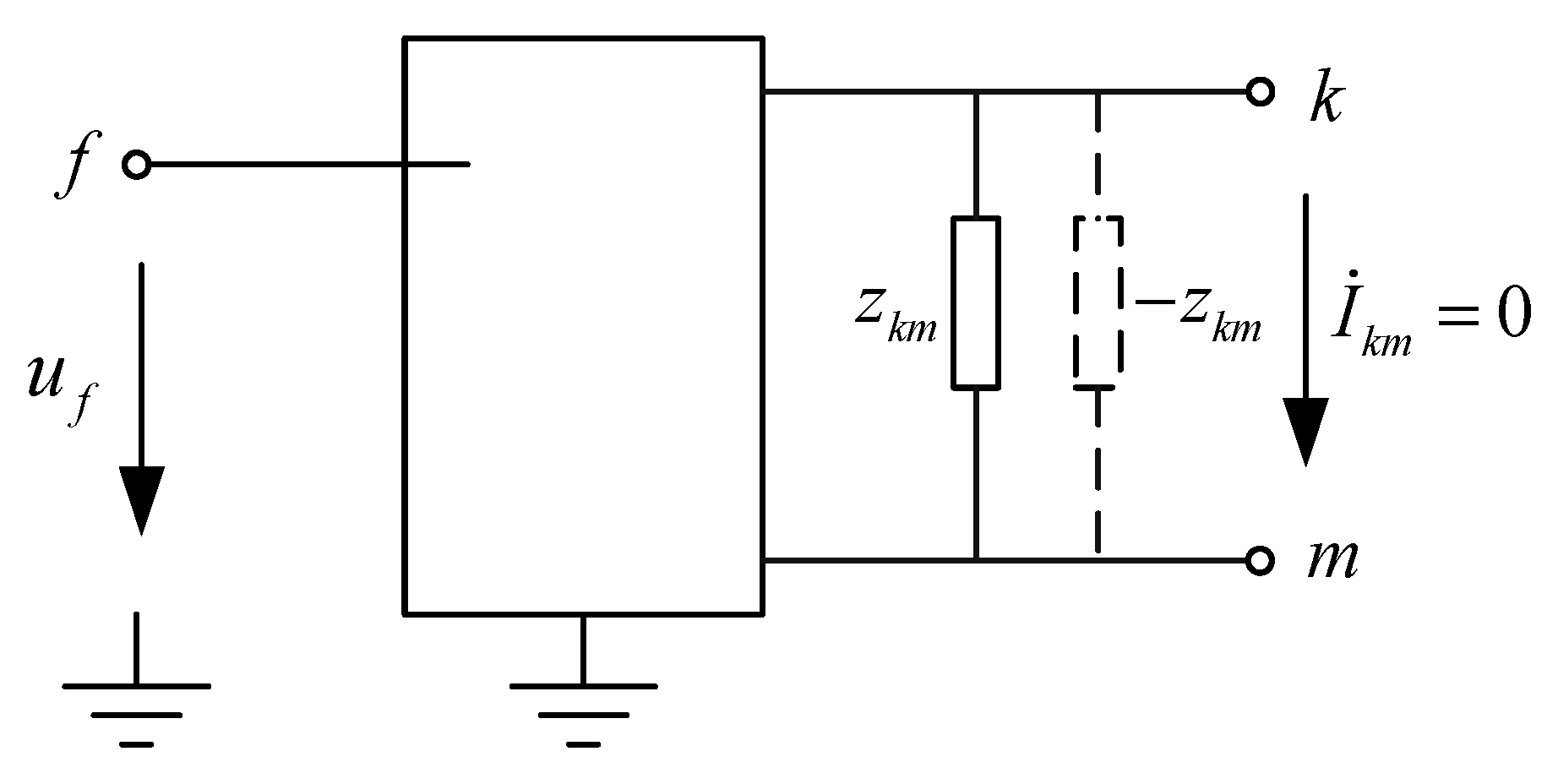
2. Constructing Impedance Matrix Using Additional Branch Method
3. Current-Limiting Measures for Changing Power Grid Structures
3.1. Significant Changes in Power Grid Structure
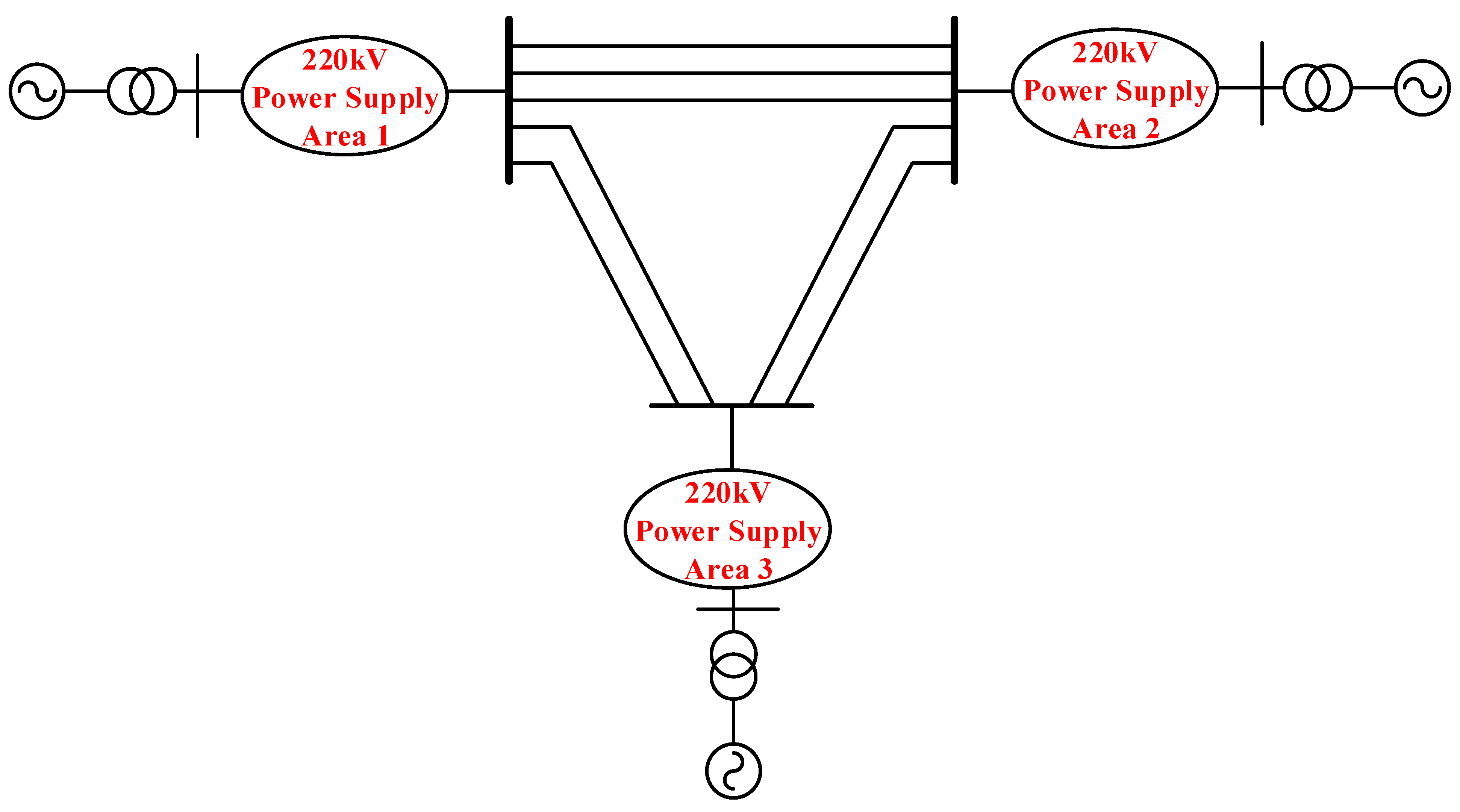
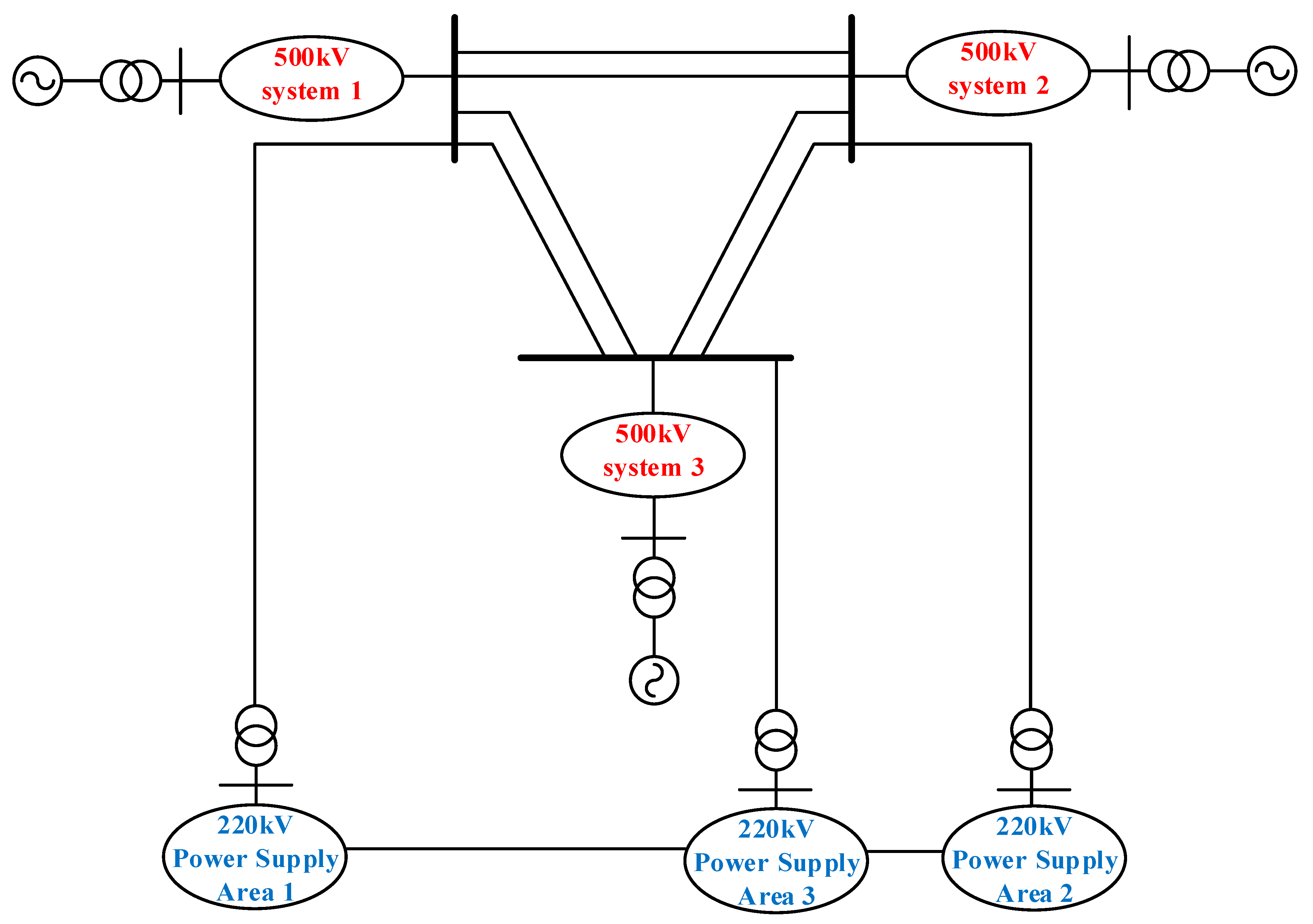
3.1.1. Power Grid Layering and Zoning
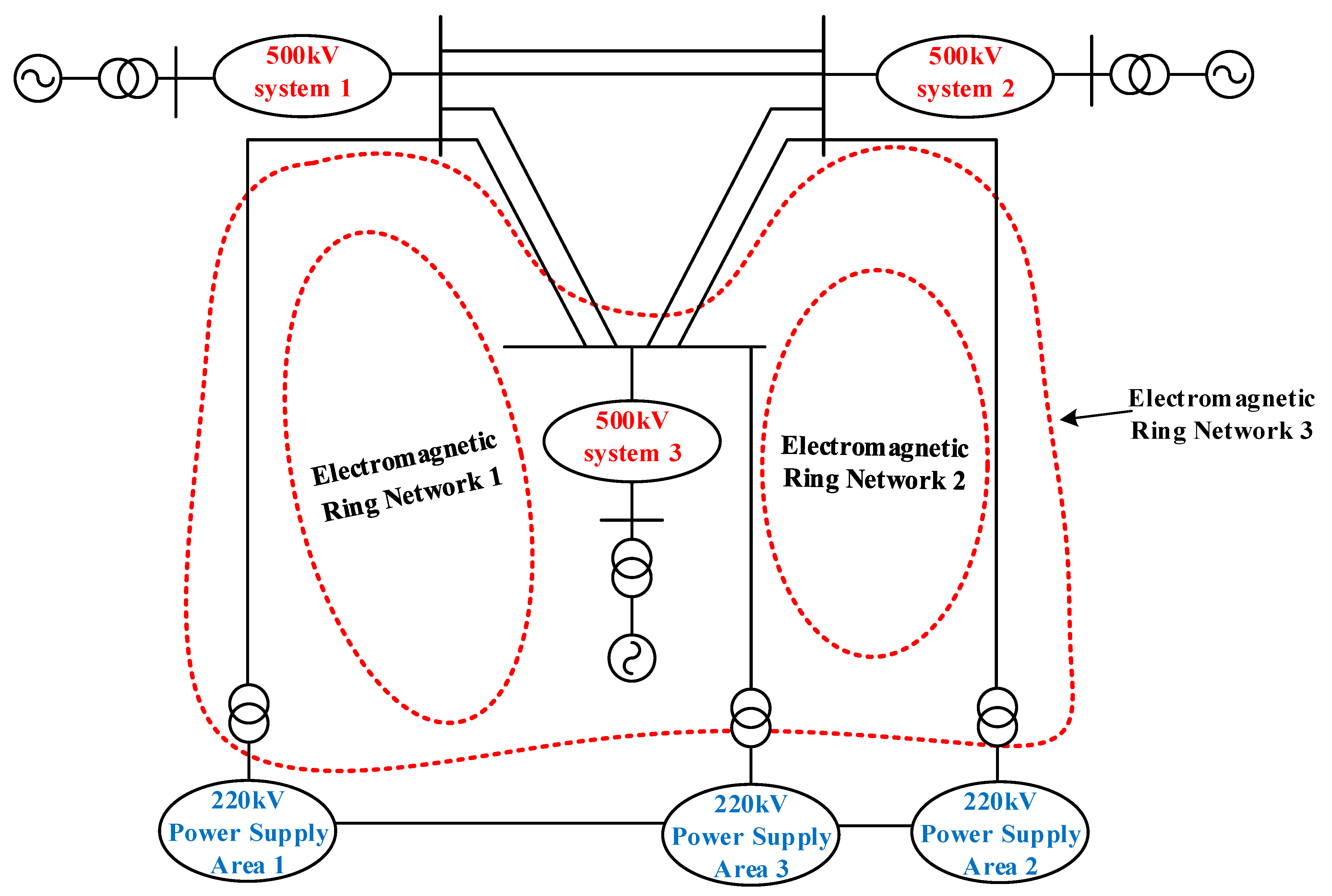
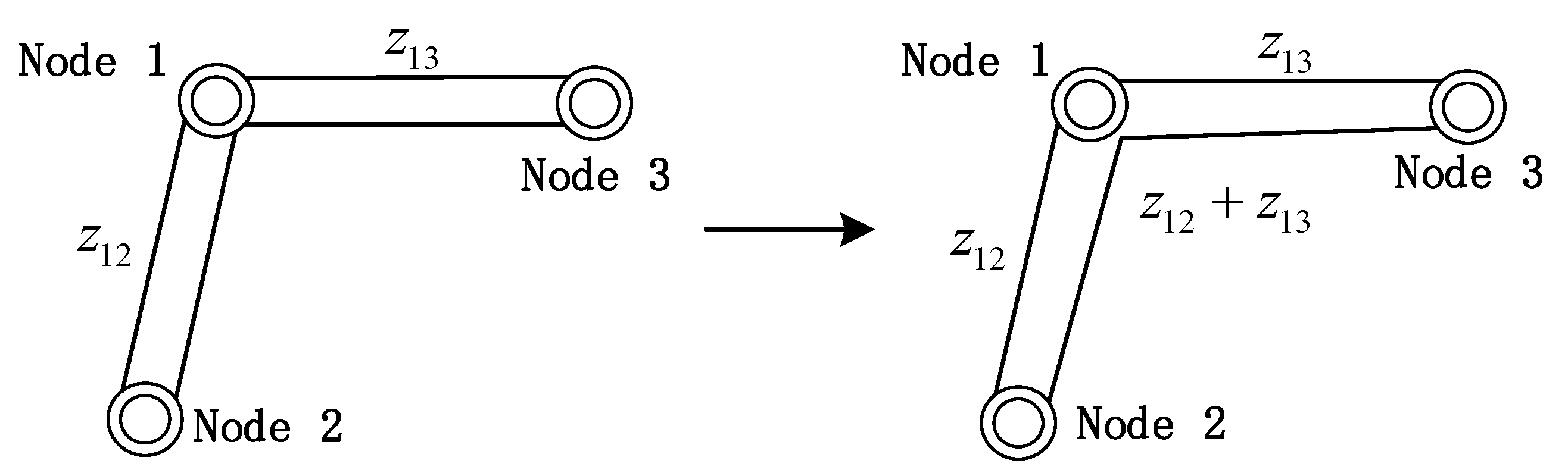
3.1.2. Electromagnetic Ring Network Breaking
- (1)
- Disconnect Line 1 between Node 1 and Node 3.
- (2)
- Disconnect Line 2 between Node 1 and Node 3.
3.2. Partial Changes in Power Grid Structure
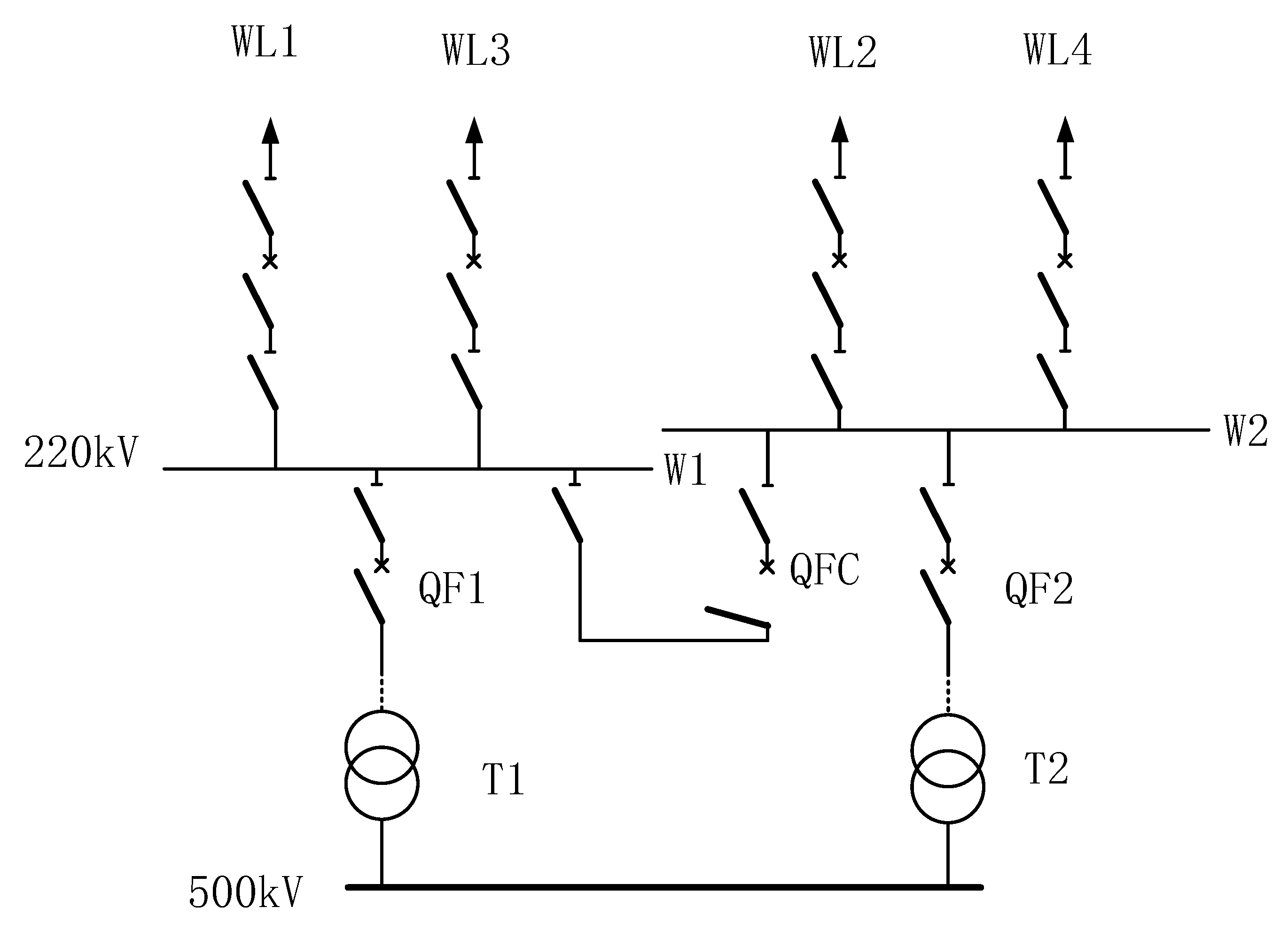
3.2.1. Bus Splitting
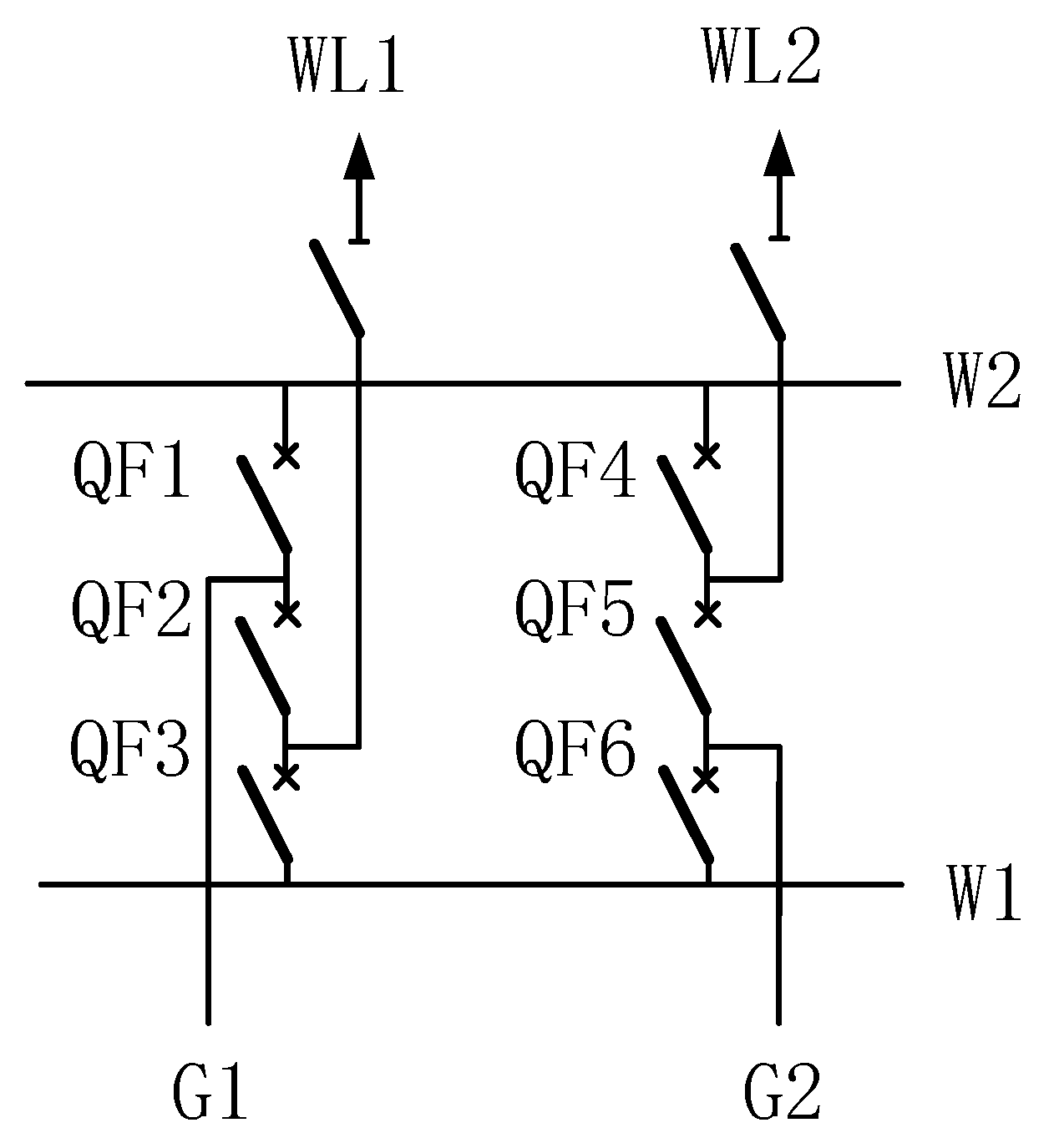
3.2.2. Dynamic Opening and Closing Circuit Breaker
3.3. Measures for Switching Power Grid Lines
3.3.1. Line Interruption
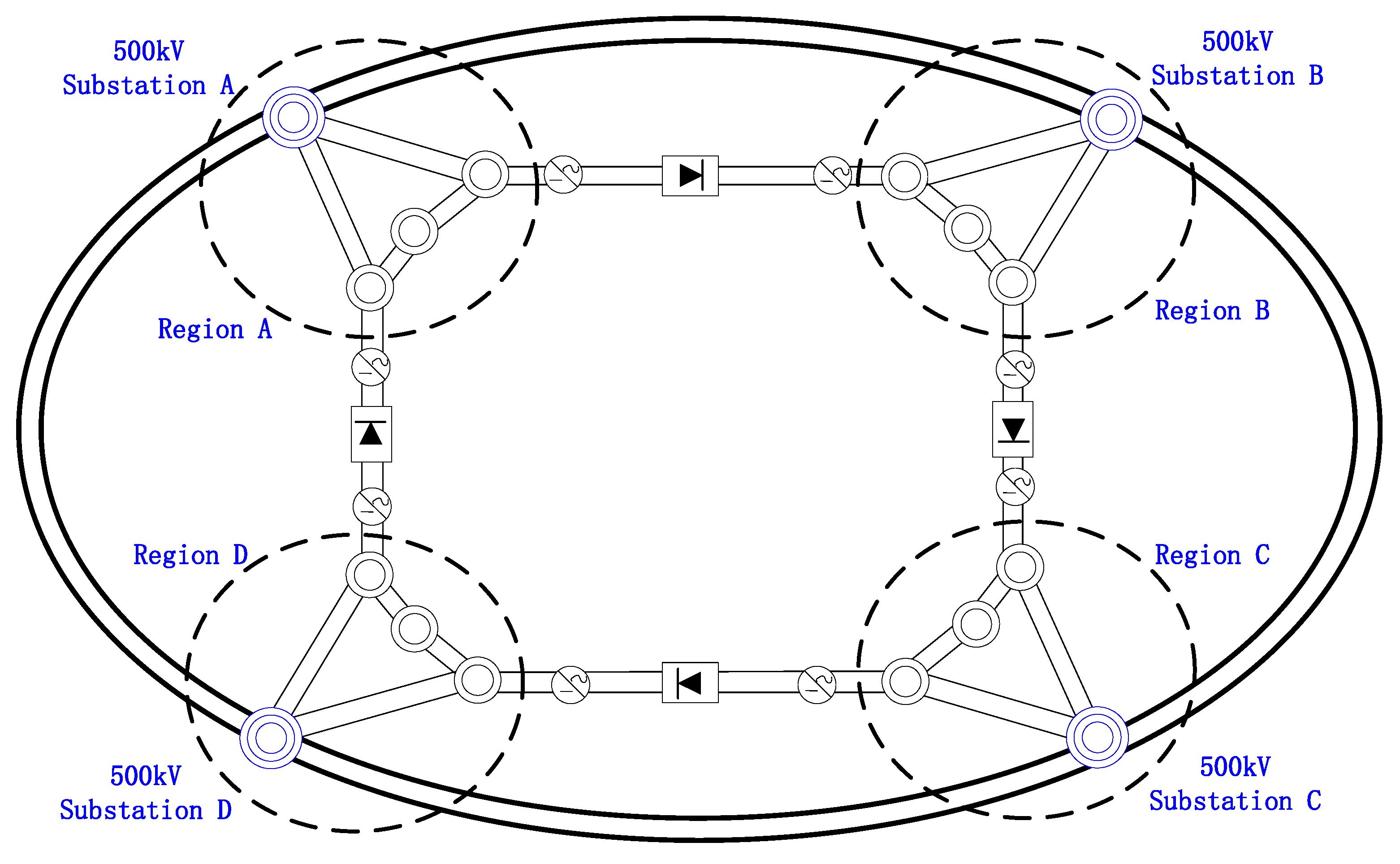
3.3.2. Adopting Direct Current Transmission
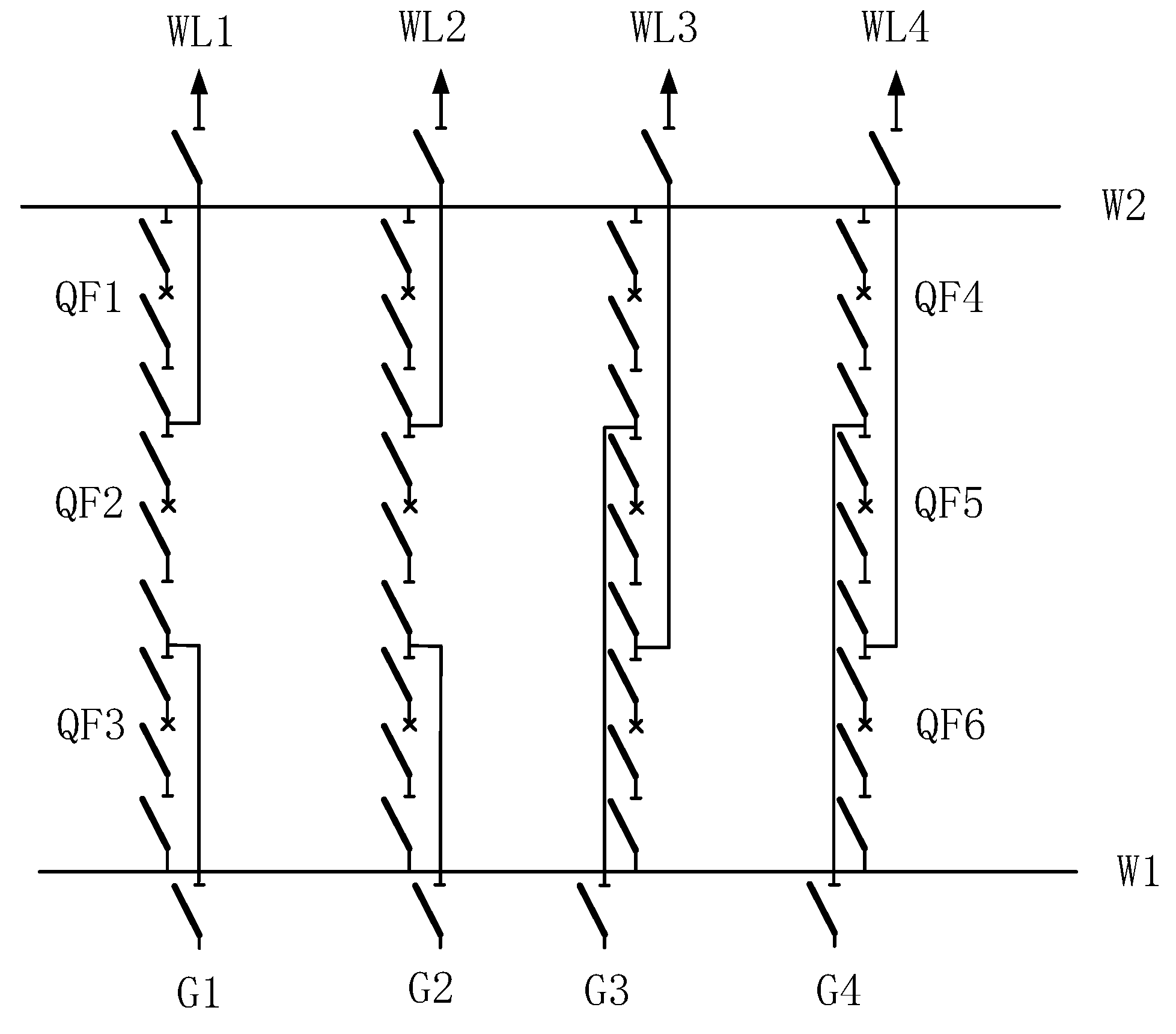
3.3.3. Line Output String
- (1)
- Disconnect line 1 between node 2 and node 1.
- (2)
- Disconnect line 1 between node 3 and node 1.
- (3)
- Add a new line between node 2 and node 3.
4. Simulation Analysis of Current-Limiting Cases
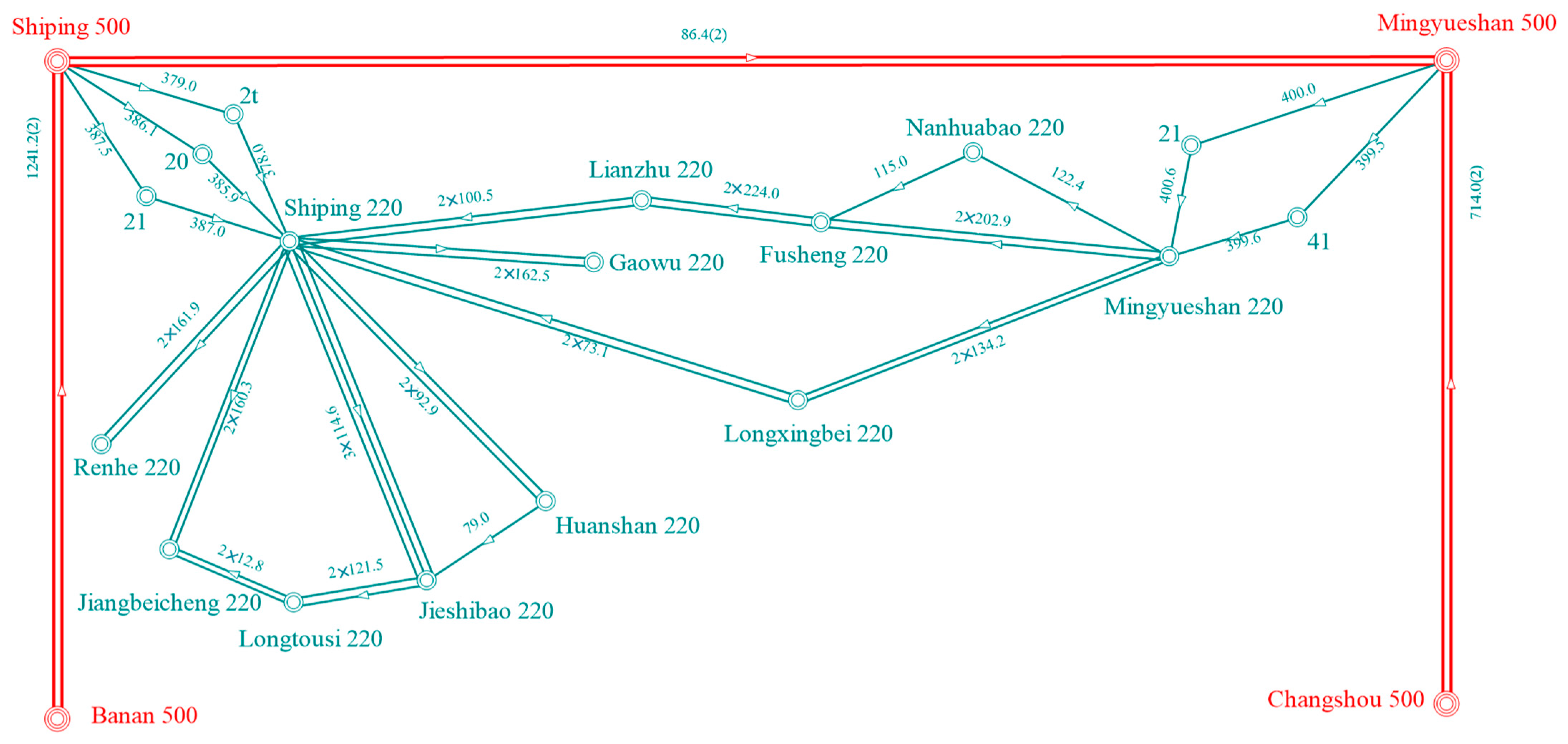
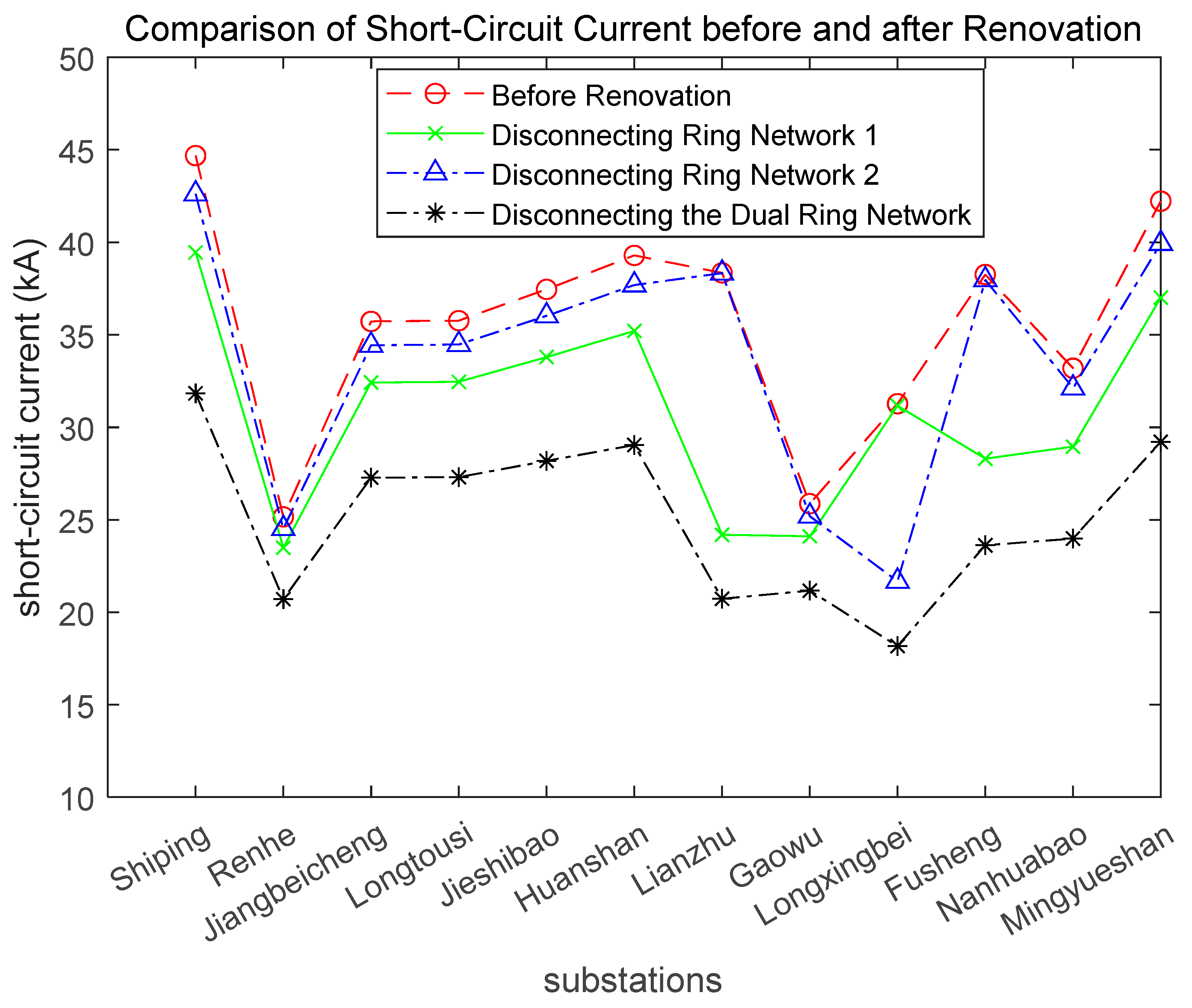
4.1. Simulation and Calculation of Measures for Significant Changes in Power Grid Structure
- (1)
- Disconnecting Ring Network 1: Break the dual circuit of Shiping 220 kV–Lianzhu 220 kV.
- (2)
- Disconnecting Ring Network 2: Break the dual circuit of Shiping 220 kV–Longxingbei 220 kV.
- (3)
- Disconnecting the Dual Ring Network: Break the dual circuit of Shiping 220 kV–Lianzhu 220 kV and break the dual circuit of Shiping 220 kV–Longxingbei 220 kV.
4.2. Simulation and Calculation of Measures for Partial Changes in Power Grid Structure
4.3. Simulation and Calculation of Measures for Switching Power Grid Lines
- (1)
- Line interruption: Disconnect branch 1 between STATIONN and STATIONS, and retain the single return line.
- (2)
- Line output string: At the STATIONN station, “jump line” is used to complete the line output stringing of STATIONS-STATIONN-North.
- (3)
- Adopting direct current transmission: Transform the North and South AC double circuit branches into DC lines. After introducing the DC system, the power grid is shown in Figure 18.
5. Conclusions
- (1)
- There are generally two factors that cause changes in the grid impedance matrix: one is the change in the topology of the power system; the other is the change in the parameters of the power system. Short-circuit-current-limiting measures can be categorized based on their operational forms into two types: changing the structure of the power system and modifying the equipment of the power system. Changing the structure corresponds to the first factor, while modifying the equipment corresponds to the second factor, which causes changes in the impedance matrix.
- (2)
- The layered and zoned management of the power grid is a fundamental measure in solving the problem of excessive short-circuit currents and is an inevitable trend in the long-term development of the grid. However, the implementation process is relatively long-term and requires a high level of grid development. Electromagnetic loop network disconnection can effectively reduce the short-circuit current levels in lower-level grids, but it may result in a loss of partial supply reliability for those grids.
- (3)
- Bus-splitting operation has low costs and simple operation, effectively targeting and limiting the short-circuit currents of specific “split-bus” substations. However, it lacks the flexibility and reliability in power supply that comes with double-bus connections and may also lead to uneven load distribution. Dynamic circuit breaker operation can compensate for the shortcomings of traditional bus-splitting operations, but the entire system is expensive and has technological issues that prevent large-scale use.
- (4)
- Line interruption has a good current-limiting effect, is easy to operate, and does not incur cost issues, but it may lead to overloads in other lines and also waste grid resources. DC transmission offers a high level of controllability over short-circuit currents, but it has high requirements for power mutual assistance and supply reliability. A line output string is simple and effective for current limiting, but there should be certain limitations on the number of branches connected to the substations implementing this measure.
- (5)
- While each current-limiting measure effectively limits short-circuit currents, it also brings about corresponding issues for grid operation. Therefore, in addition to short-circuit calculations, comprehensive consideration should be given to stability calculations, power flow calculations, and economic costs. After comprehensive evaluation, the optimal solution to address the problem of excessive short-circuit currents should be obtained.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Eroshenko, S.; Egorov, A.; Senyuk, M.; Khalyasmaa, A. Practical experience in short-circuit current limiting measures implementation. In Proceedings of the 2017 IEEE Conference of Russian Young Researchers in Electrical and Electronic Engineering (EIConRus), St. Petersburg and Moscow, Russia, 1–3 February 2017; pp. 1326–1331. [Google Scholar]
- Jin, X.; Duan, K.Y.; Zhuang, W.Q. Short-circuit current limiting methods for power networks. J. Electr. Power Sci. Technol. 2008, 23, 78–82. [Google Scholar]
- Schlabbach, J. Limitation of Short-Circuit Currents. In Short Circuit Currents; The Institution of Engineering and Technology: Stevenage, UK, 2005; pp. 225–244. [Google Scholar] [CrossRef]
- Vorkunov, O.; Glotkina, L.; Sinicin, A. Short-Circuit Current Limitation. In Proceedings of the 2024 International Conference on Industrial Engineering, Applications and Manufacturing (ICIEAM), Sochi, Russia, 20–24 May 2024; pp. 315–320. [Google Scholar]
- Liu, B.; Li, Y.; Xi, J.; Xu, Y. Analysis of Pulling Switch to Limit Short-circuit Current in 3/2 Wiring Substation. Autom. Electr. Power Syst. 2014, 38, 131–135. [Google Scholar]
- Han, G.; Han, L.; Wu, L. Application and development of methods on limiting power grid’s short-circuit current. Power Syst. Prot. Control 2010, 38, 141–144. [Google Scholar]
- Yuan, J.; Liu, W.; Dong, M.; Shi, K.; Fan, Y. Application of Measures Limiting Short Circuit Currents in Northwest China Power Grid. Power Syst. Technol. 2007, 31, 42–45. [Google Scholar]
- Xiong, W.; Lu, H. Analysis of short circuit current level of Guangdong Province power system and limiting measures. J. Guizhou Univ. Technol. 2002, 31, 32–35. [Google Scholar]
- Lu, B.; Huang, M.; Huang, Y.; Tian, B. Application of short-circuit current limitation technology to Ningxia Power Grid. East China Electr. Power 2008, 36, 40–42. [Google Scholar]
- Pan, X.; Wang, H.; Liu, F.; Gao, Z.; Tian, C.; Zhang, H. A New Method of Short-circuit Current Limitation for Henan 500 kV Power Grid. In Proceedings of the 2022 7th Asia Conference on Power and Electrical Engineering (ACPEE), Hangzhou, China, 15–17 April 2022; pp. 45–49. [Google Scholar]
- Qi, S.; Pi, J.; Wu, H.; Ma, X. Grid Optimization Based on Sensitivity Analysis for Limiting Short-circuit Current of Power System. In Proceedings of the 2022 IEEE 6th Conference on Energy Internet and Energy System Integration (EI2), Chengdu, China, 11–13 November 2022; pp. 1096–1101. [Google Scholar]
- Zhang, K.; Hu, J.; Lu, X.; Peng, Z.; Ma, P.; Gao, F. Influence of fast switching fault current limiter on electromagnetic transient of extra-high voltage transmission line. J. Phys. Conf. Ser. 2024, 2903, 012007. [Google Scholar] [CrossRef]
- Ren, H.; Zhang, Y. Analysis of Transient Recovery Voltage of 500 kV Line Considering Current Limiting Reactor. J. Phys. Conf. Ser. 2023, 2488, 012036. [Google Scholar] [CrossRef]
- Zhang, L.; Yu, Y.; Liu, S.; Guo, J. Research on Short-Circuit Current Suppression Based on Equivalent Sensitivity. In Proceedings of the 2020 International Conference on Urban Engineering and Management Science (ICUEMS), Zhuhai, China, 24–26 April 2020; pp. 251–255. [Google Scholar]
- Yang, Z.; Li, L.; Li, Y.; Wang, K. The Issue of Short-circuit Current Being out of Limitation in Guangdong Power Grid and Related Countermeasures. South. Power Syst. Technol. 2011, 5, 90–93. [Google Scholar]
- Viktorovich, K.A.; Petrovich, Y.Y.; Aleksandrovich, M.A. The Problem of Short-circuit Current Limitation in Energy–saving Systems of Transportation and Electricity Distribution. In Proceedings of the 2021 12th International Symposium on Advanced Topics in Electrical Engineering (ATEE), Bucharest, Romania, 25–27 March 2021; pp. 1–5. [Google Scholar]
- Safonov, E.; Frolov, V.; Zhiligotov, R.; Petrenya, Y. On the Problems of Current Limitations in Networks Based on Power Semiconductor Devices. Energies 2023, 16, 5905. [Google Scholar] [CrossRef]
- Luo, F.; Zhang, C.; Liu, F.; Bai, S.; Xiang, L.; Zhong, H. Research on Limiting Measures for DC Component of Short Circuit Current Based on Fault Current Limiter and Selection of Installation Site. In Proceedings of the 2019 IEEE Asia Power and Energy Engineering Conference (APEEC), Chengdu, China, 29–31 March 2019; pp. 89–93. [Google Scholar]
- Ma, K. Research and Application of 220kV Short-Circuit Current Limiting Measures Based on Fast Switch. In Proceedings of the 2022 IEEE Asia-Pacific Conference on Image Processing, Electronics and Computers (IPEC), Dalian, China, 14–16 April 2022; pp. 948–951. [Google Scholar]
- Castro, L.; Guillen, D.; Trillaud, F. On Short-Circuit Current Calculations Including Superconducting Fault Current Limiters (ScFCLs). IEEE Trans. Power Deliv. 2018, 33, 2513–2523. [Google Scholar] [CrossRef]
- Yu, Y.; Pan, T.; Rui, J.; Chen, S.; Fan, Y. Design and analysis of a peak current-limiting device using an oscillating zero-crossing circuit. Electr. Power Syst. Res. 2025, 242, 111465. [Google Scholar] [CrossRef]
- Han, L.; Qiu, W.; Xiao, Z. Overview on Measures of Short-Circuit Current Limitation on Power System. Electr. Power Technol. Econ. 2009, 21, 33–37. [Google Scholar]
- Sun, S.; Zhou, G.; Song, Y.; Tang, X.; Yuan, Z.; Qi, X. Research on Optimization Method of Short-Circuit Current-Limiting Measures Based on Combination Assignment. Energies 2024, 17, 5724. [Google Scholar] [CrossRef]






| Voltage Level (kV) | Short-Circuit Current Control Value (kA) | Voltage Level (kV) | Short-Circuit Current Control Value (kA) |
|---|---|---|---|
| 1000 | 50 | 110 | 40 |
| 750 | 63 | 66 | 31.5 |
| 500 | 63 | 35 | 25 |
| 330 | 63 | 20 | 20 |
| 220 | 50 | 10 | 20 |
| Current-Limiting Measures | Selected Condition |
|---|---|
| Power grid layering and zoning | The structure of the upper and lower power grids is strong, especially in limiting the short-circuit current of the lower power grids, which has a significant effect |
| Electromagnetic ring network breaking | Having the conditions for developing a higher voltage level power grid and ensuring that the reliability of power supply is not affected after partitioning the lower-level power grid |
| Bus splitting | When there are multiple branches connected to the busbar, it is advisable to have at least four connecting transformers connected to the busbar |
| Dynamic opening and closing of circuit breaker | There are locations within the station where monitoring and control systems are installed, and changing the structure of the power grid has a significant impact on its characteristics |
| Line interruption | The power flow of the line is small |
| Adopting direct current transmission | It has high requirements for power mutual benefit and power supply reliability, especially suitable for the transformation of inter-zone interconnection lines in the power grid |
| Line output string | The substation has four or more lines |
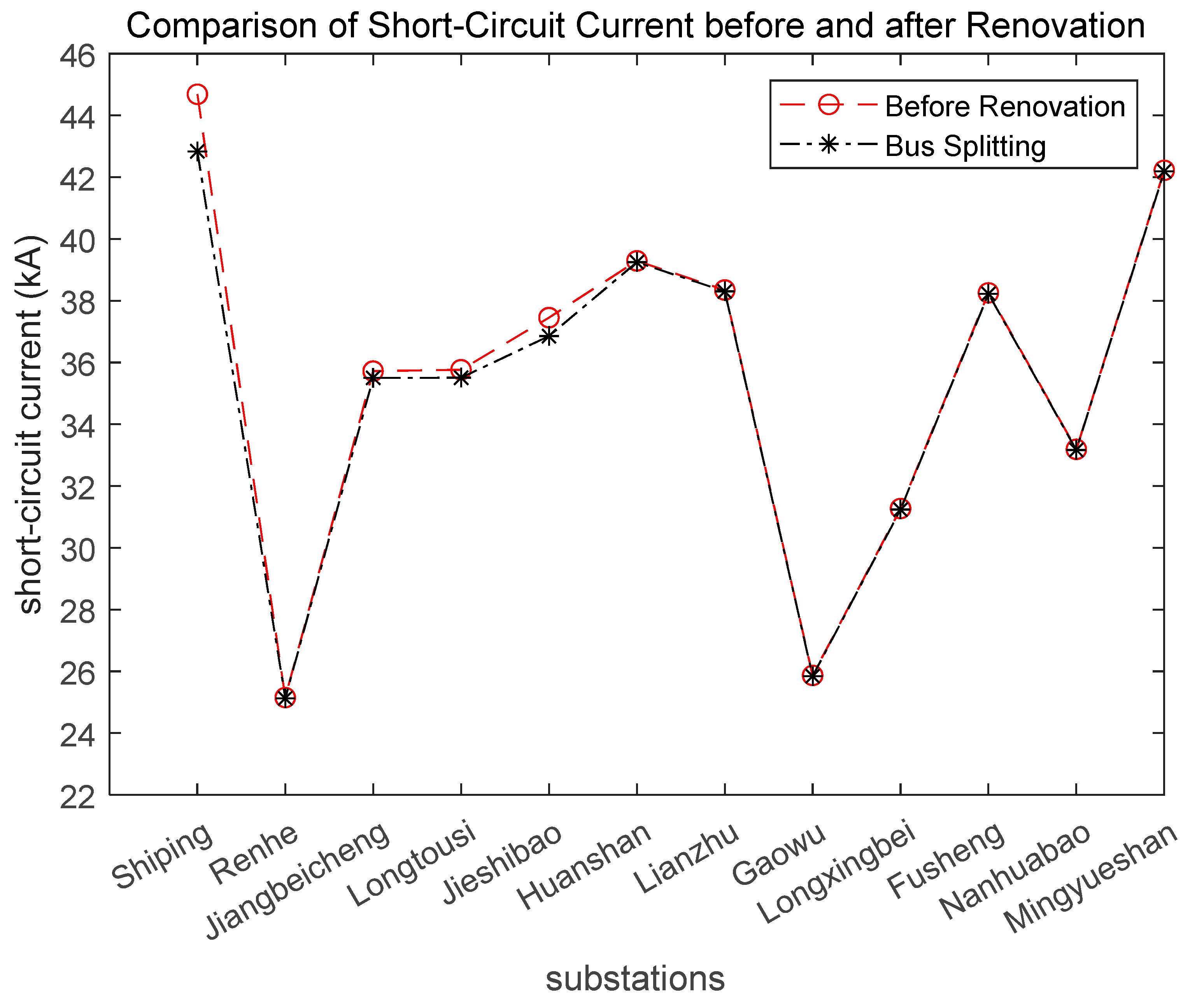
| Substation | Short-Circuit Current (kA) | Substation | Short-Circuit Current (kA) |
|---|---|---|---|
| Shiping | 44.688 | Lianzhu | 38.348 |
| Renhe | 25.153 | Gaowu | 25.872 |
| Jiangbeicheng | 35.723 | Longxingbei | 31.274 |
| Longtousi | 35.767 | Fusheng | 38.259 |
| Jieshibao | 37.455 | Nanhuabao | 33.191 |
| Huanshan | 39.292 | Mingyueshan | 42.226 |
| Substation | Short-Circuit Current (kA) | Substation | Short-Circuit Current (kA) |
|---|---|---|---|
| Shiping 1/Shiping 2 | 42.838/41.157 | Lianzhu | 38.306 |
| Renhe | 25.127 | Gaowu | 25.845 |
| Jiangbeicheng | 35.505 | Longxingbei | 31.240 |
| Longtousi | 35.509 | Fusheng | 38.225 |
| Jieshibao | 36.857 | Nanhuabao | 33.170 |
| Huanshan | 39.249 | Mingyueshan | 42.195 |
| Substation | Short-Circuit Current (kA) |
|---|---|
| STATIONN | 20.00 |
| NORTH | 33.22 |
| STATIONS | 10.36 |
| SOUTH | 19.17 |
| Current-Limiting Measures | Substation | Short-Circuit Current (kA) |
|---|---|---|
| Line interruption | STATIONN | 19.18 |
| NORTH | 33.16 | |
| STATIONS | 8.96 | |
| SOUTH | 18.33 | |
| Line output string | STATIONN | 17.77 |
| NORTH | 32.39 | |
| STATIONS | 9.70 | |
| SOUTH | 18.58 | |
| Direct current transmission | STATIONN | 19.98 |
| NORTH | 28.25 | |
| STATIONS | 8.43 | |
| SOUTH | 11.01 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, S.; Zhou, G.; Song, Y.; Tang, X.; Zhou, Y.; Yuan, Z. Research on Measures to Limit Short-Circuit Current by Changing the Structure of the Power Grid. Energies 2025, 18, 2098. https://doi.org/10.3390/en18082098
Sun S, Zhou G, Song Y, Tang X, Zhou Y, Yuan Z. Research on Measures to Limit Short-Circuit Current by Changing the Structure of the Power Grid. Energies. 2025; 18(8):2098. https://doi.org/10.3390/en18082098
Chicago/Turabian StyleSun, Shuqin, Guanghao Zhou, Yunting Song, Xiaojun Tang, Yuguang Zhou, and Zhenghai Yuan. 2025. "Research on Measures to Limit Short-Circuit Current by Changing the Structure of the Power Grid" Energies 18, no. 8: 2098. https://doi.org/10.3390/en18082098
APA StyleSun, S., Zhou, G., Song, Y., Tang, X., Zhou, Y., & Yuan, Z. (2025). Research on Measures to Limit Short-Circuit Current by Changing the Structure of the Power Grid. Energies, 18(8), 2098. https://doi.org/10.3390/en18082098





