Internal Model Current Decoupling Control Strategy for Induction Motors

Abstract

In order to improve the current dynamic performance of induction motor (IM) drive systems, an internal model current decoupling control strategy is proposed to suppress the stator’s internal coupling effect. First, the IM mathematical model in the complex frequency domain is established, and the expressions of the coupling terms are derived. Then, according to the zero-pole distribution diagram and Bode plots, the design details for the structure and parameters of the internal model controller are presented in a continuous domain, and the impact of stator inductance mismatch on decoupling performance is analyzed. In addition to considering sampling and control delays, the IM mathematical model is established in the discrete domain, and the principle of the controller parameter is presented. Finally, the experimental results prove that the proposed internal model current decoupling control strategy can effectively improve the dynamic performance of IMs. Compared with the traditional feedforward current decoupling control strategy, the proposed method has superior decoupling performance under the operating conditions of low switching frequency. At the same time, a better steady-state performance is obtained by the proposed internal model control strategy.

1. Introduction

Induction motors (IMs) have advantages such as high efficiency, high torque output capability, and high reliability [1,2] and are widely used in industrial fields such as high-speed railways and electric vehicles [3,4]. Various control strategies for induction motors have also been proposed, including constant voltage frequency ratio control [5], vector control [6], direct torque control [7], and model prediction control [8]. Among them, vector control based on rotor flux orientation can achieve independent control of flux and torque, providing IMs with the speed regulation characteristics of DC motors [9], so it is currently the most mature and widely used motor control technology. However, after Clarke and Park’s transformation, there is a cross-coupling term in the mathematical model of the IM, which leads to an incomplete linear relationship between voltage and current in the d–q axis. In cases of high speed or parameter mismatch, the dynamic performance of the control system will deteriorate [10].

Feedforward decoupling compensates for the output voltage by adding a decoupling term to the reference of the stator current; this decoupling principle is simple, easy to implement, and widely used in engineering [11]. However, the cross-coupling in IMs is caused by the actual stator current, and the inconsistency between the exact value and the reference value may cause incomplete decoupling. In addition, the decoupling effect is poor under the conditions of low carrier ratio and parameter mismatch. In the literature [12], the state equation is transformed and a diagonalization matrix is constructed, so the linearization of the current control equation is achieved. According to the zero pole shift theory, the literature [13] introduces complex vector decoupling in the PI controller, improving decoupling performance in high-speed intervals. The literature [14] analyzes the impact of carrier ratio reduction on coupling effects; to improve the decoupling performance under a low carrier ratio, the delay compensation is introduced into the complex vector decoupling controller. In [15], a compensation angle is added to compensate for the angle delay, and a decoupling algorithm combining model predictive control is proposed to compensate for the time delay. However, the above methods rely on the accuracy of speed measurement and parameters, so the coupling effect cannot be decoupled completely.

The literature [16] considers voltage coupling an uncertain disturbance and adopts a disturbance observer (DOB) decoupling method. Although DOB decoupling is superior to diagonalization decoupling under inaccurate parameters, the decoupling performance is affected by nonlinear factors in the inverter. In [17], a sliding mode observer is adopted to estimate the stator current and feed it back to the input, achieving the decoupling of the d-axis and q-axis currents and solving the problem of poor robustness in feedforward decoupling. However, current oscillation occurs when the motor operates under high-speed conditions. The literature [18] proposes a current decoupling method based on an extended state observer (ESO), which obtains comprehensive observations and compensates for cross-coupling and disturbances caused by other factors through ESO to achieve decoupling. However, an increase in ESO bandwidth will reduce the stability of the control system. The literature [19] adopts an improved neural network algorithm for decoupling controllers, which improves the dynamic response speed, but the computational burden of the algorithm is too high.

Internal model decoupling is a mature control method developed from process control, which separates uncertain factors from the controlled object model [20]. It can dynamically track the reference and eliminate the influence of uncertain interference without a completely accurate model. Compared with other decoupling methods, internal model decoupling has the advantages of simple tuning and easy adjustment, and higher robustness can be obtained. This paper studies the parameter design methods of the internal model current decoupling controller in a continuous domain (s-domain). Then, to improve the decoupling performance under low switching frequency, the internal model current decoupling controller is modified in a discrete domain (z-domain). Finally, the experimental verifications are completed. The chapters are organized as follows: In Section 2, the d–q axis cross-coupling characteristic is analyzed based on the mathematical model of the IM. In Section 3, a current decoupling controller based on the internal model principle is proposed. In Section 4, the experimental results are presented. Finally, the conclusion is drawn in Section 5.

2. The Mathematical Model and Coupling Effect Analysis of the IM

This section first establishes the mathematical model of the induction motor, and on this basis, the expression of the complex vector transfer function is derived, and its coupling effect is analyzed.

2.1. Model of Induction Motor

Assuming that the magnetic potential generated by the stator and rotor in the air gap is sinusoidally distributed and ignoring the effects of ferromagnetic saturation, eddy currents, and teeth slots, the flux linkage and voltage equations of the squirrel-cage induction motor in the d–q coordinate system are expressed as follows [21]:

$$\left\{\begin{array}{l}{\psi }_{\mathrm{sd}}=L_{\mathrm{s}}{i}_{\mathrm{sd}}+L_{\mathrm{m}}{i}_{\mathrm{rd}}\hfill \\ {\psi }_{\mathrm{sq}}=L_{\mathrm{s}}{i}_{\mathrm{sq}}+L_{\mathrm{m}}{i}_{\mathrm{rq}}\hfill \\ {\psi }_{\mathrm{rd}}=L_{\mathrm{r}}{i}_{\mathrm{rd}}+L_{\mathrm{m}}{i}_{\mathrm{sd}}\hfill \\ {\psi }_{\mathrm{rq}}=L_{\mathrm{r}}{i}_{\mathrm{rq}}+L_{\mathrm{m}}{i}_{\mathrm{sq}}\hfill \end{array}\right.$$

(1)

$$\left\{\begin{array}{l}{u}_{\mathrm{sd}}=R_{\mathrm{s}}{i}_{\mathrm{sd}}+p{\psi }_{\mathrm{sd}}-{\psi }_{\mathrm{sq}}{\omega }_{\mathrm{e}}\hfill \\ u_{\mathrm{sq}}=R_{\mathrm{s}}{i}_{\mathrm{sq}}+p{\psi }_{\mathrm{sq}}+{\psi }_{\mathrm{sd}}{\omega }_{\mathrm{e}}\hfill \\ 0=R_r{i}_{\mathrm{rd}}+p{\psi }_{\mathrm{rd}}-{\psi }_{\mathrm{rq}}\left({\omega }_{\mathrm{e}}-{\omega }_{\mathrm{r}}\right)\hfill \\ 0=R_{\mathrm{r}}{i}_{\mathrm{rq}}+p{\psi }_{\mathrm{rq}}+{\psi }_{\mathrm{rd}}\left({\omega }_{\mathrm{e}}-{\omega }_{\mathrm{r}}\right)\hfill \end{array}\right.$$

(2)

In the above two equations, ψ_sd and ψ_sq represent the stator flux linkage in the d-axis and q-axis, respectively, ψ_rd and ψ_rq represent the rotor flux linkage in the d-axis and q-axis, respectively, i_sd and i_sq represent the stator current in the d-axis and q-axis respectively, i_rd and i_rq represent the rotor current in the d-axis and q-axis, respectively, u_sd and u_sq represent the stator voltage in the d-axis and q-axis, respectively, u_rd and u_rq represent the rotor voltage in the d-axis and q-axis, respectively, L_s represents the stator inductance, L_m represents the excitation inductance, L_r represents the rotor inductance, R_s represents the stator resistance, R_r represents the rotor resistance, p represents the differential operator, ω_e represents the synchronous angular frequency, and ω_r represents the rotor electrical angular frequency.

In the d–q coordinate system, ψ_rq is equal to zero, and the rotor flux linkage ψ_r is equal to ψ_rd. According to Equations (1) and (2), the relationship between ψ_r and i_sd is obtained as follows:

$${\psi }_{\mathrm{r}}=L_{\mathrm{m}}{\displaystyle \frac{i_{\mathrm{sd}}}{T_{\mathrm{r}}s+1}}$$

(3)

where T_r is the rotor time constant, which is equal to L_r/R_r.

The spatial vectors are defined as follows:

$$\left\{\begin{array}{l}{u}_{\mathrm{s}}=u_{\mathrm{sd}}+j{u}_{\mathrm{sq}}\hfill \\ u_{\mathrm{r}}=u_{\mathrm{rd}}+j{u}_{\mathrm{rq}}\hfill \\ {\Psi }_{\mathrm{s}}={\psi }_{\mathrm{sd}}+j{\psi }_{\mathrm{sq}}\hfill \\ {\Psi }_{\mathrm{r}}={\psi }_{\mathrm{rd}}+j{\psi }_{\mathrm{rq}}\hfill \\ i_{\mathrm{s}}=i_{\mathrm{sd}}+j{i}_{\mathrm{sq}}\hfill \\ i_{\mathrm{r}}=i_{\mathrm{rd}}+j{i}_{\mathrm{rq}}\hfill \end{array}\right.$$

(4)

where j represents the imaginary component, and u_s, u_r, ψ_s, ψ_r, i_s, and i_r donate spatial vectors of state voltage, rotor voltage, stator flux linkage, rotor flux linkage, stator current and rotor current, respectively. Then, the flux linkage equation of Formula (1) and the voltage equation of Formula (2) can, respectively, be expressed in the form of spatial vectors.

$$\left\{\begin{array}{l}{\psi }_{\mathrm{s}}=L_{\mathrm{s}}{i}_{\mathrm{s}}+L_{\mathrm{m}}{i}_{\mathrm{r}}\hfill \\ {\psi }_{\mathrm{r}}=L_{\mathrm{m}}{i}_{\mathrm{s}}+L_{\mathrm{r}}{i}_{\mathrm{r}}\hfill \end{array}\right.$$

(5)

$$\left\{\begin{array}{l}{u}_{\mathrm{s}}=R_{\mathrm{s}}{i}_{\mathrm{s}}+p{\psi }_{\mathrm{s}}+j{\omega }_{\mathrm{e}}{\psi }_{\mathrm{s}}\hfill \\ \mathbf{0}=R_r{i}_{\mathrm{r}}+p{\psi }_{\mathrm{r}}+j\left({\omega }_{\mathrm{c}}-{\omega }_{\mathrm{r}}\right){\psi }_{\mathrm{r}}\hfill \end{array}\right.$$

(6)

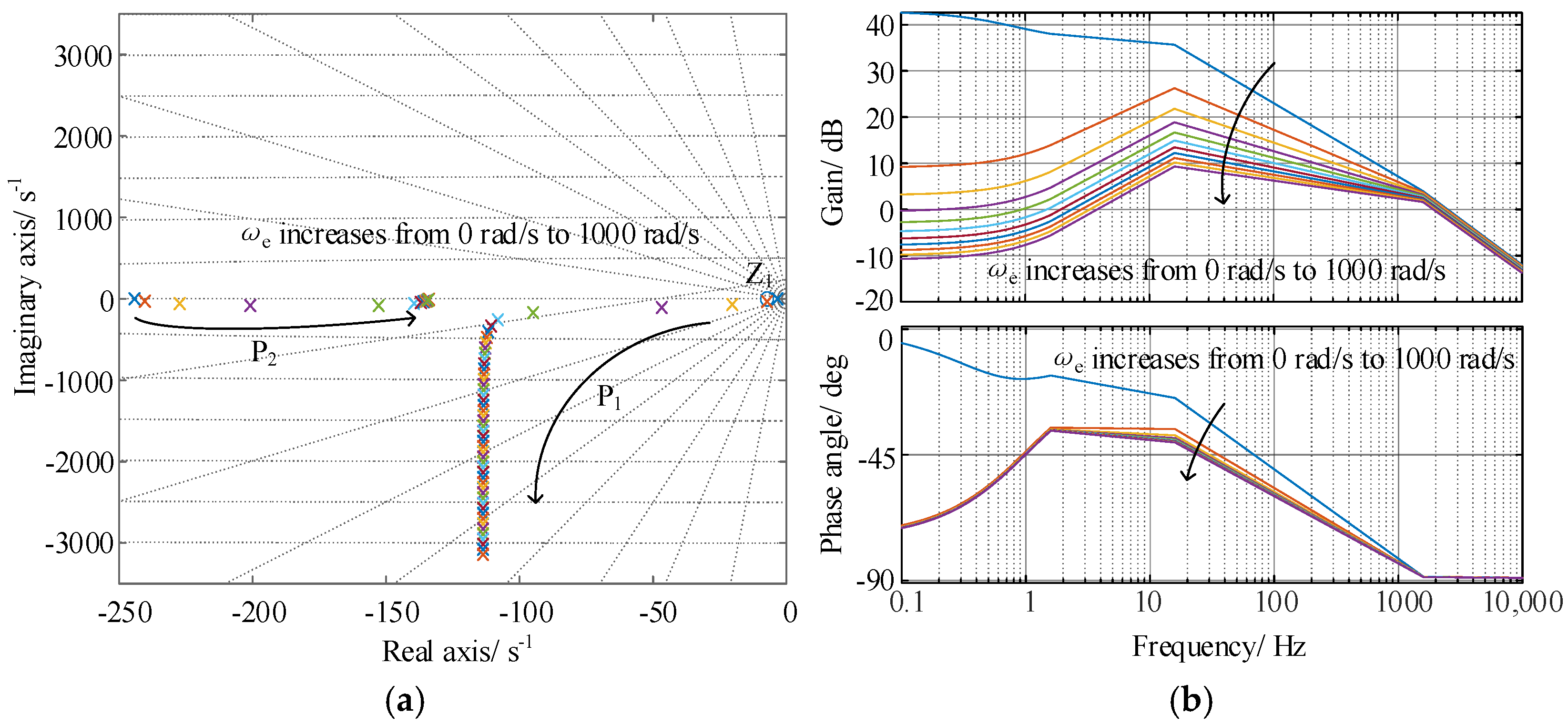
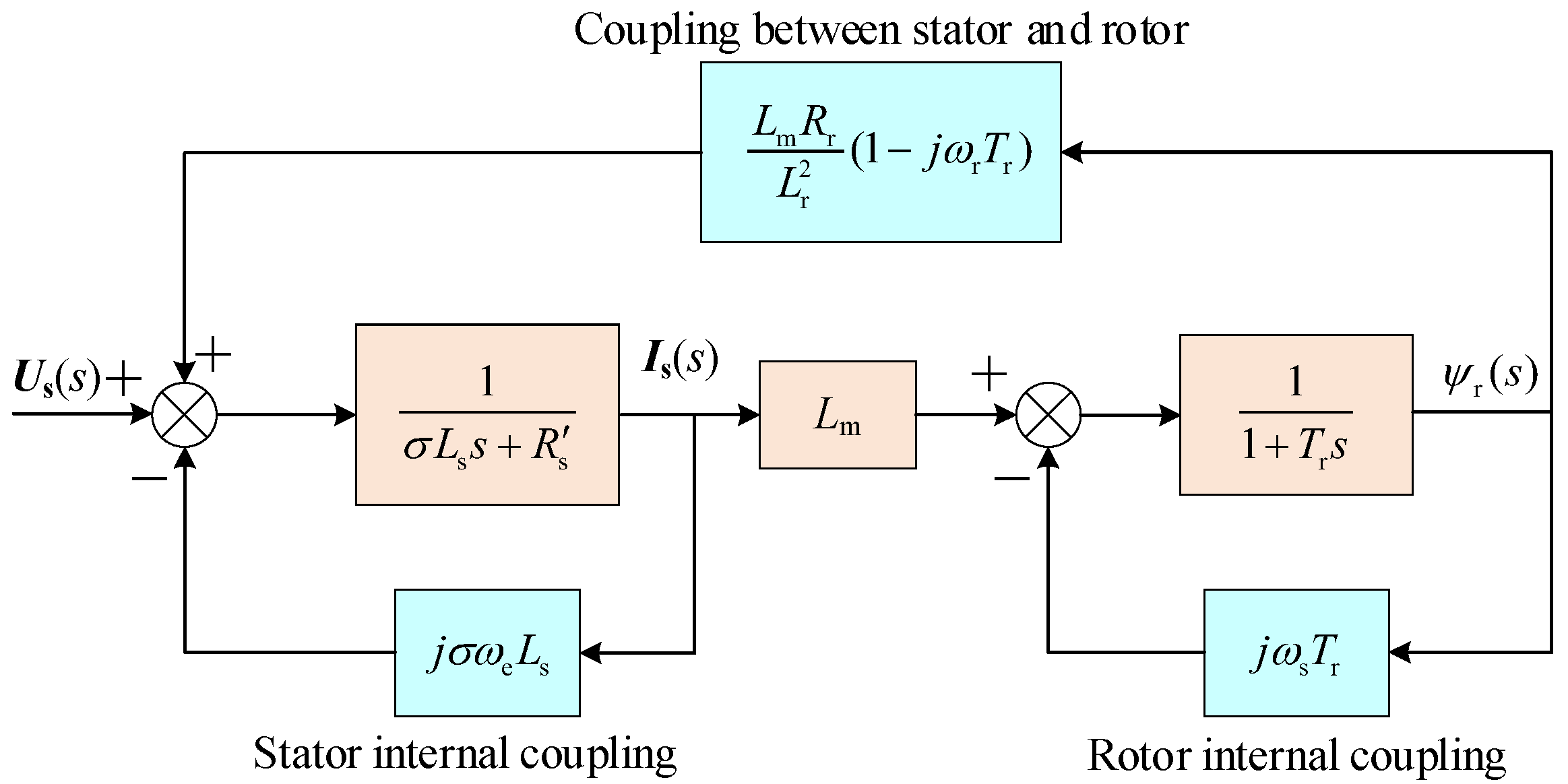
2.2. Coupling Effect Analysis

According to Equations (5) and (6), in the d–q coordinate system, the s-domain transfer functions of rotor flux linkage and stator voltage can be obtained as follows:

$${\Psi }_{\mathrm{r}}(s)={\displaystyle \frac{L_{\mathrm{m}}}{T_{\mathrm{r}}s+1+j{\omega }_{\mathrm{s}}{T}_{\mathrm{r}}}}{I}_{\mathrm{s}}(s)$$

(7)

$$U_{\mathrm{s}}(s)=\left(\sigma L_{\mathrm{s}}s+R_{\mathrm{s}}^{\prime }+j\sigma L_{\mathrm{s}}{\omega }_{\mathrm{e}}\right)I_{\mathrm{s}}(s)-{\displaystyle \frac{L_{\mathrm{m}}{R}_{\mathrm{r}}}{L_{\mathrm{r}}^2}}\left(1-j{\omega }_{\mathrm{r}}{T}_{\mathrm{r}}\right){\Psi }_{\mathrm{r}}(s)$$

(8)

where s represents the complex frequency domain operator, ψ_s(s), I_s(s), and U_s(s) are the s-domain expressions of rotor flux, stator current, and stator voltage vectors, respectively, magnetic leakage coefficient σ = 1 − L²_m/(L_rL_s), and stator equivalent resistance $R_{\mathrm{s}}^{\prime }=R_{\mathrm{s}}+R_{\mathrm{r}}{L}_{\mathrm{m}}^2/L_{\mathrm{r}}^2$.

Substituting Equation (7) into Equation (8), the transfer function G_{m_f}(s) from the stator voltage u_s to the stator current i_s of the IM can be obtained as follows:

$$G_{\mathrm{m}\_\mathrm{f}}(s)={\displaystyle \frac{T_{\mathrm{r}}s+j{\omega }_{\mathrm{e}}{T}_{\mathrm{r}}+1}{\left(R_{\mathrm{s}}^{\prime }+\sigma L_{\mathrm{s}}s+j\sigma L_{\mathrm{s}}{\omega }_{\mathrm{e}}\right)\left(T_{\mathrm{r}}s+j{\omega }_{\mathrm{e}}{T}_{\mathrm{r}}+1\right)-L_{\mathrm{m}}^2{R}_{\mathrm{r}}\left(1-j{\omega }_{\mathrm{r}}{T}_{\mathrm{r}}\right)/L_{\mathrm{r}}^2}}$$

(9)

The parameters of the IM and inverter used in the theoretical analysis, simulation, and experiment are presented in

Table 1. Parameters of IMs and controllers.

Parameter	Value
Rated Power	1 kW
Number of Pole Pairs	2
Rated Voltage	48 V
Rated Speed	3000 rpm
Stator Resistance	0.25 Ω
Rotor Resistance	0.25 Ω
Stator Inductance	0.5 mH
Rotor Inductance	0.5 mH
Excitation Inductance	0.5 mH
Conduction Voltage Drop of Switching Tube	0.7 V
Conduction Voltage Drop of Diode	0.5 V

. According to Equation (9), when the synchronous speed ω_e rises from 0 to 1000 rad/s, the distribution of zeros and poles and the frequency characteristics are plotted in Figure 1. When ω_e = 0 rad/s, G_{m_f}(s) has a pair of zeros, Z_1, and poles, P₁, that can cancel each other out, as well as a pole, P₂, far from the imaginary axis. As the synchronous speed ω_e increases, the imaginary axis component of pole P₁ gradually increases, presenting a coupled and increasingly underdamped state, which leads to an increase in oscillations during the dynamic process.

From Equations (7) and (8), the space vector structure diagram of the induction motor in the s-domain can be obtained, as shown in Figure 2.

The degree of current cross-coupling is determined by the imaginary component of the transfer function G_{m_f}(s). According to Figure 2, the coupling of the IM includes the following three parts:

(1)

Stator internal coupling $-j\sigma L_{\mathrm{s}}{\omega }_{\mathrm{e}}{I}_{\mathrm{s}}(s)$: This is generated by the rotating coordinate transformationl the coupling degree is directly proportional to the synchronous angular frequency ω_e and the stator current I_s(s). Under the condition of high speed and large current, this coupling term has a significant impact on system performance;

(2)

The coupling term between the stator and rotor $L_{\mathrm{m}}{R}_{\mathrm{r}}\left(1-j{\omega }_{\mathrm{r}}{T}_r\right){\Psi }_{\mathrm{r}}(s)/L_{\mathrm{r}}^2$: This term is directly proportional to the rotor angular frequency ω_r, and the coupling between the stator and rotor increases as the speed increases. According to Equation (7), the rotor flux ψ_r(s) lags behind the stator current I_s, and this part of the coupling component can be regarded as an external disturbance to the system, which is considered as electromotive force E_s(s);

(3)

Rotor internal coupling $-j{\omega }_{\mathrm{s}}{T}_{\mathrm{r}}$: Its magnitude depends on the slip frequency ω_s, and the rotor flux ψ_r(s), and is related to the rotor time constant T_r. ω_s is usually small, so this term has little effect on the dynamic performance of the IM. When the motor operates with no load and zero speed, there is no internal coupling in the rotor.

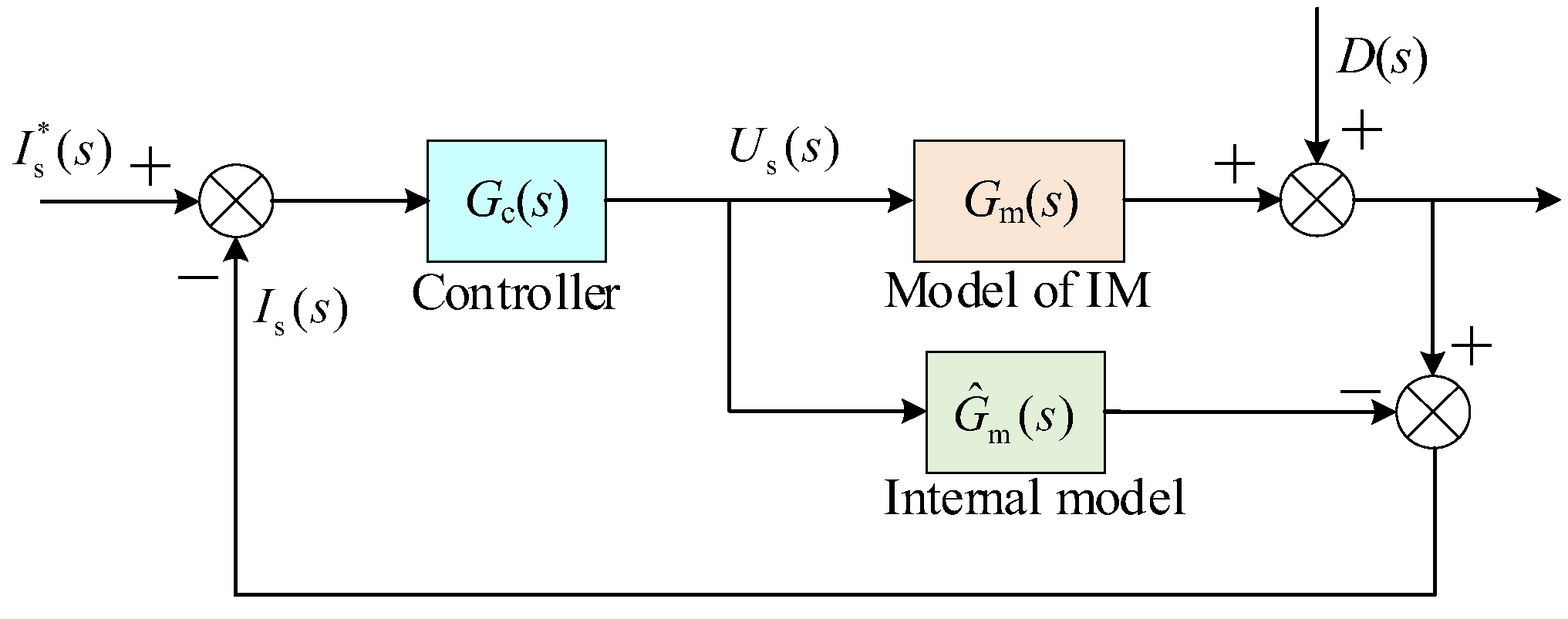
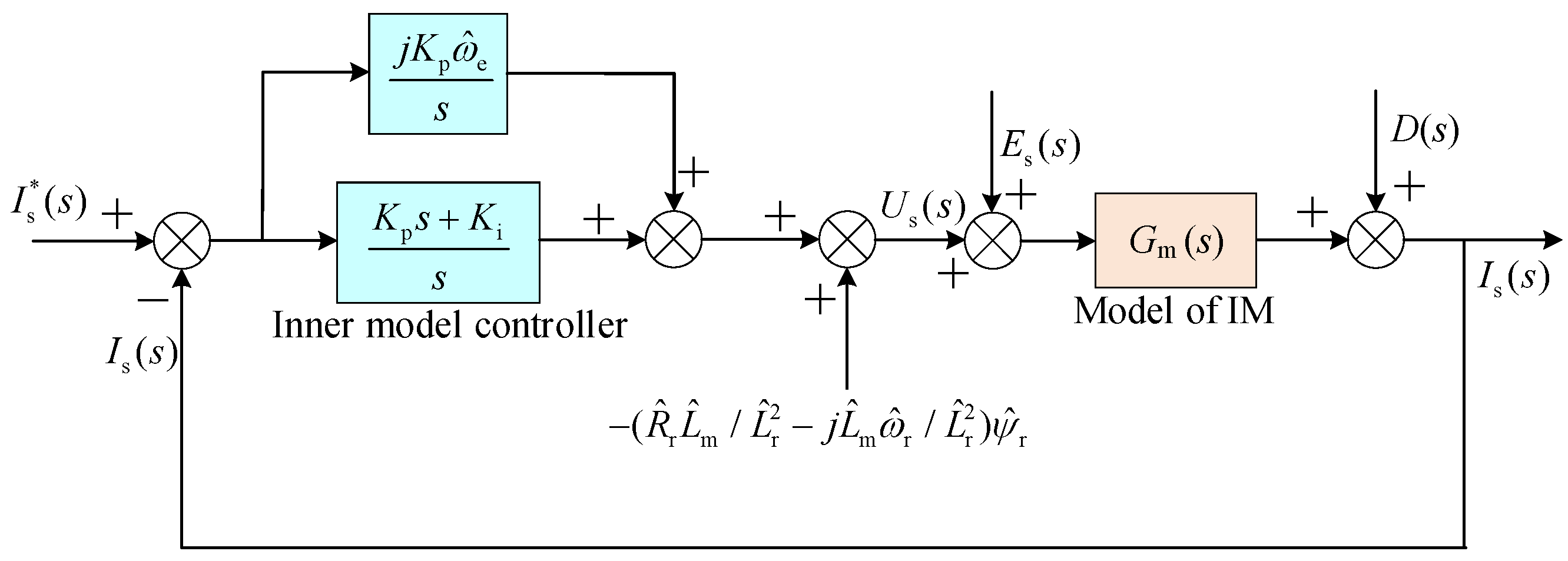
3. Internal Model Current Decoupling Controller for IM

Current coupling is considered an external disturbance, based on the theory of internal model control; the internal model current controller is first designed in the s-domain, then is discretized to reflect the characteristics of digital control, and the parameter optimization of the current decoupling controller is designed in the z-domain.

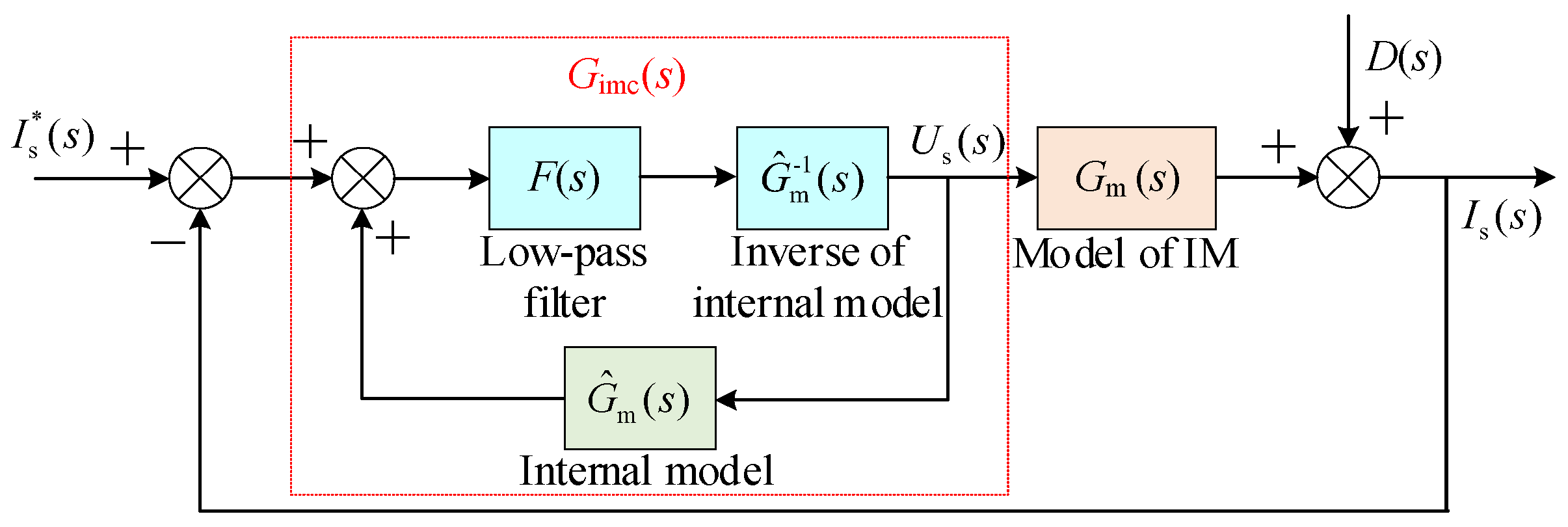
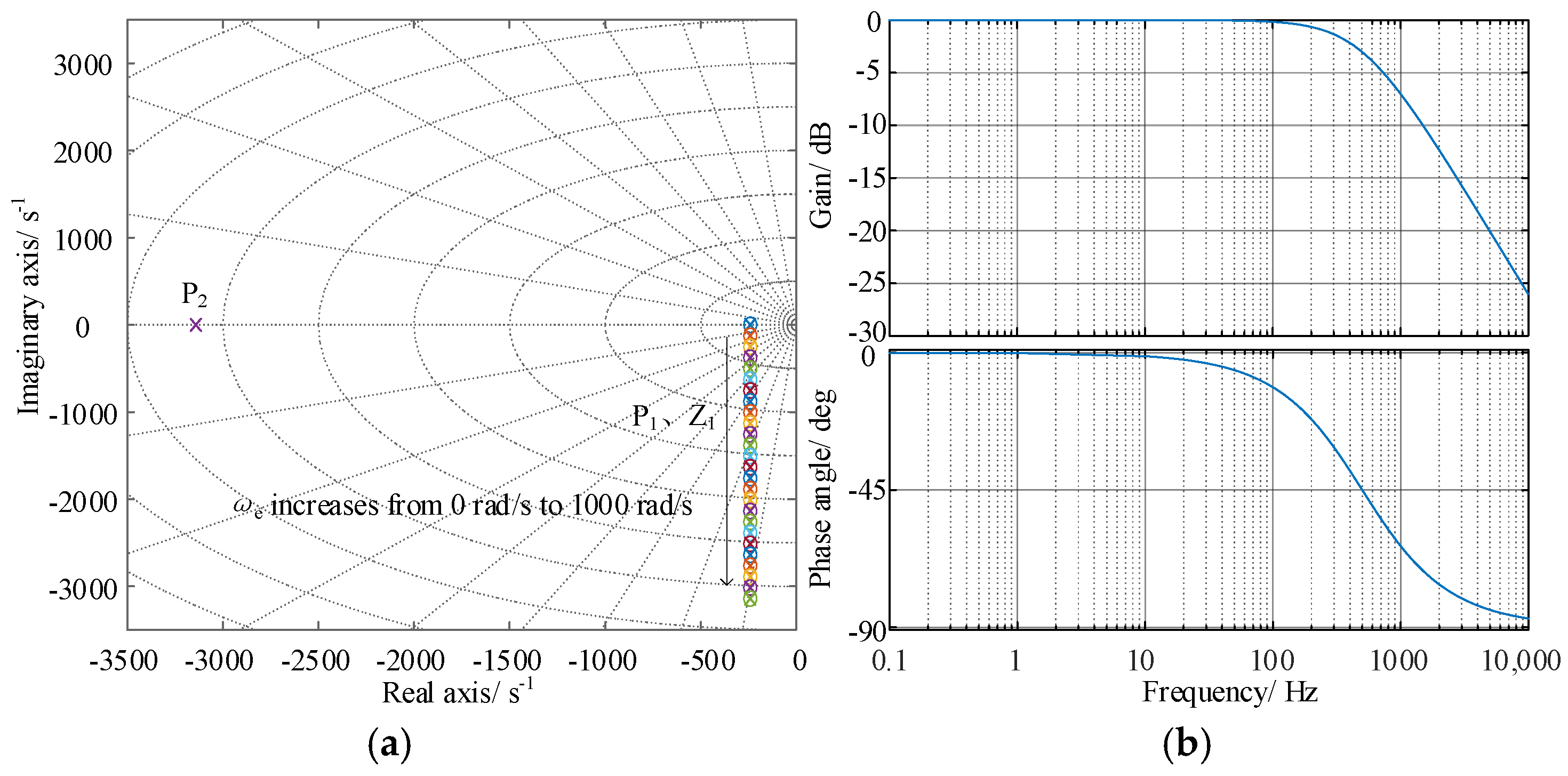
3.1. Design of Internal Model Current Decoupling Controller in Continuous Domain

As shown in Figure 3, an s-domain current control system IM is designed based on the internal model control principle, where $I_{\mathrm{s}}^{*}$(s) represents the reference of the stator current, D(s) represents the current disturbance caused by coupling, I_s(s) represents the actual stator current, G_m(s) is the first term of Equation (8), Ĝ_m(s) is the internal model, and G_c(s) is the controller to be designed.

According to Figure 3, the I_s(s) can be expressed as follows:

$$I(s)={\displaystyle \frac{G_{\mathrm{c}}(s)G_{\mathrm{m}}(s)}{1+G_{\mathrm{c}}(s)\left[G_{\mathrm{m}}(s)-{\widehat{G}}_{\mathrm{m}}(s)\right]}}{I}_{\mathrm{s}}^{*}(s)+{\displaystyle \frac{1-G_{\mathrm{c}}(s){\widehat{G}}_{\mathrm{m}}(s)}{1+G_{\mathrm{c}}(s)\left[G_{\mathrm{m}}(s)-{\widehat{G}}_{\mathrm{m}}(s)\right]}}D(s)$$

(10)

when ${\widehat{G}}_{\mathrm{m}}(s)=G_{\mathrm{m}}(s)$, if $G_{\mathrm{c}}(s)={\widehat{G}}_{\mathrm{m}}^{-1}(s)$, $I_{\mathrm{s}}(s)=I_{\mathrm{s}}^{*}(s)$ will be obtained. Therefore, if the model of the IM is accurate, it can overcome the interference and achieve zero-error tracking. From Equation (10), it can be obtained that ${\widehat{G}}_{\mathrm{m}}^{-1}(s)=\widehat{\sigma }{\widehat{L}}_{\mathrm{s}}s+{\widehat{R}}_{\mathrm{s}}^{\prime }+j{\omega }_{\mathrm{e}}\widehat{\sigma }{\widehat{L}}_{\mathrm{s}}$, where symbol “ˆ” denotes the estimated value. It can be seen that the differential item occurs in Ĝ_m(s), a low-pass filter F(s) is introduced in the control loop, and its transfer function is defined as follows:

$$F(s)={\displaystyle \frac{1}{\alpha s+1}}$$

(11)

At this time, the transfer function of $G_{\mathrm{c}}(s)$ is expressed as follows:

$$G_{\mathrm{c}}(s)={\widehat{G}}_{\mathrm{m}}^{-1}(s)L(s)={\displaystyle \frac{\widehat{\sigma }{\widehat{L}}_{\mathrm{s}}s+{\widehat{R}}_{\mathrm{s}}^{\prime }+j{\omega }_{\mathrm{e}}\widehat{\sigma }{\widehat{L}}_s}{\alpha s+1}}$$

(12)

The equivalent structure of the internal model control system is shown in Figure 4.

According to Figure 4, the expression of the internal model current controller G_imc(s) is as follows:

$$G_{\mathrm{imc}}(s)={\displaystyle \frac{F(s)}{1-F(s)}}{\widehat{G}}_{\mathrm{m}}^{-1}(s)={\displaystyle \frac{1}{\alpha }}\left({\displaystyle \frac{\widehat{\sigma }{\widehat{L}}_{\mathrm{s}}s+{\widehat{R}}_{\mathrm{s}}^{\prime }+j{\widehat{\omega }}_{\mathrm{c}}\widehat{\sigma }{\widehat{L}}_{\mathrm{s}}}{s}}\right)$$

(13)

$G_{\mathrm{imc}}(s)$ and the back electromotive force compensation term $-\left({\widehat{R}}_{\mathrm{r}}{\widehat{L}}_{\mathrm{m}}/L_{\mathrm{r}}^2-j{\widehat{L}}_{\mathrm{m}}{\widehat{\omega }}_{\mathrm{r}}/{\widehat{L}}_{\mathrm{r}}\right){\widehat{\psi }}_{\mathrm{r}}$ are introduced into the current loop. The complete structure of the s-domain internal model current control system is shown in Figure 5.

As shown in Figure 6, when $1/\alpha ={\omega }_{\mathrm{b}}$, the internal model current controller can be regarded as a PI controller, K_p + K_i/s, with an added cross-decoupling term, j${\widehat{\omega }}_{\mathrm{e}}$K_p/s, and a back-EMF compensation term, $-\left(R_{\mathrm{r}}{L}_{\mathrm{m}}/L_{\mathrm{r}}^2-j{L}_{\mathrm{m}}{\omega }_{\mathrm{r}}/L_{\mathrm{r}}\right){\psi }_{\mathrm{r}}$. Combined with Figure 4, the cross-decoupling term $j{\widehat{\omega }}_{\mathrm{e}}{K}_{\mathrm{p}}/s$ in Figure 6 can eliminate the stator’s internal coupling, and the back-EMF compensation term can eliminate the influence of the coupling between the stator and rotor. Since the slip frequency is usually small, the influence of the rotor internal coupling can be ignored. Therefore, in the s-domain, the internal model current controller can completely decouple the d-axis and q-axis currents when the switching frequency is high.

When the internal model current controller is used, the current open-loop transfer function is expressed as (14). When the parameter is accurate, G_o(s) is equal to ω_b/s, and the system has no coupling, which verifies the above analysis.

$$G_{\mathrm{o}}(s)=\left({\displaystyle \frac{K_{\mathrm{p}}s+K_{\mathrm{i}}+j{\widehat{\omega }}_{\mathrm{e}}{K}_{\mathrm{p}}}{s}}\right)\cdot \left({\displaystyle \frac{1}{\sigma L_{\mathrm{s}}s+R_{\mathrm{s}}^{\prime }+j{\omega }_{\mathrm{e}}\sigma L_{\mathrm{s}}}}\right)$$

(14)

When synchronous speed ω_e varies within the range of [0 rad/s, 1000π rad/s], and the internal model controller parameters are accurate, the zero-pole distribution diagram of the current closed-loop transfer function is drawn and shown in Figure 7. It can be seen that due to the cross-decoupling term $j{\widehat{\omega }}_{\mathrm{e}}{K}_{\mathrm{p}}/s$, the internal model controller generates a zero Z₁ at the same position as the pole P₁, eliminating the internal coupling of the stator caused by the rotation coordinate transformation. The positions of Z₁ and P₁ are completely coincident, so the internal model controller can eliminate the increase in current control coupling and the decrease in system stability at high synchronous speed, and complete decoupling is achieved.

When $\sigma L_{\mathrm{s}}=2\widehat{\sigma }{\widehat{L}}_s$, the zero and pole distribution of the current closed-loop transfer function under the internal model controller is shown in Figure 8a. Compared with Figure 7a, as the synchronous speed ω_e increases, there is a slight position deviation between the zero Z₁ and pole P₁. However, according to the Bode plots shown in Figure 8b, the mismatch of the stator inductance parameter has little impact on the cut-off frequency and phase, which illustrates that a slight position deviation can be ignored and the zero Z₁ can significantly weaken the impact of the pole P₁ on dynamic performance. Therefore, the proposed internal model control scheme has good decoupling performance. Meanwhile, it is insensitive to stator leakage inductance.

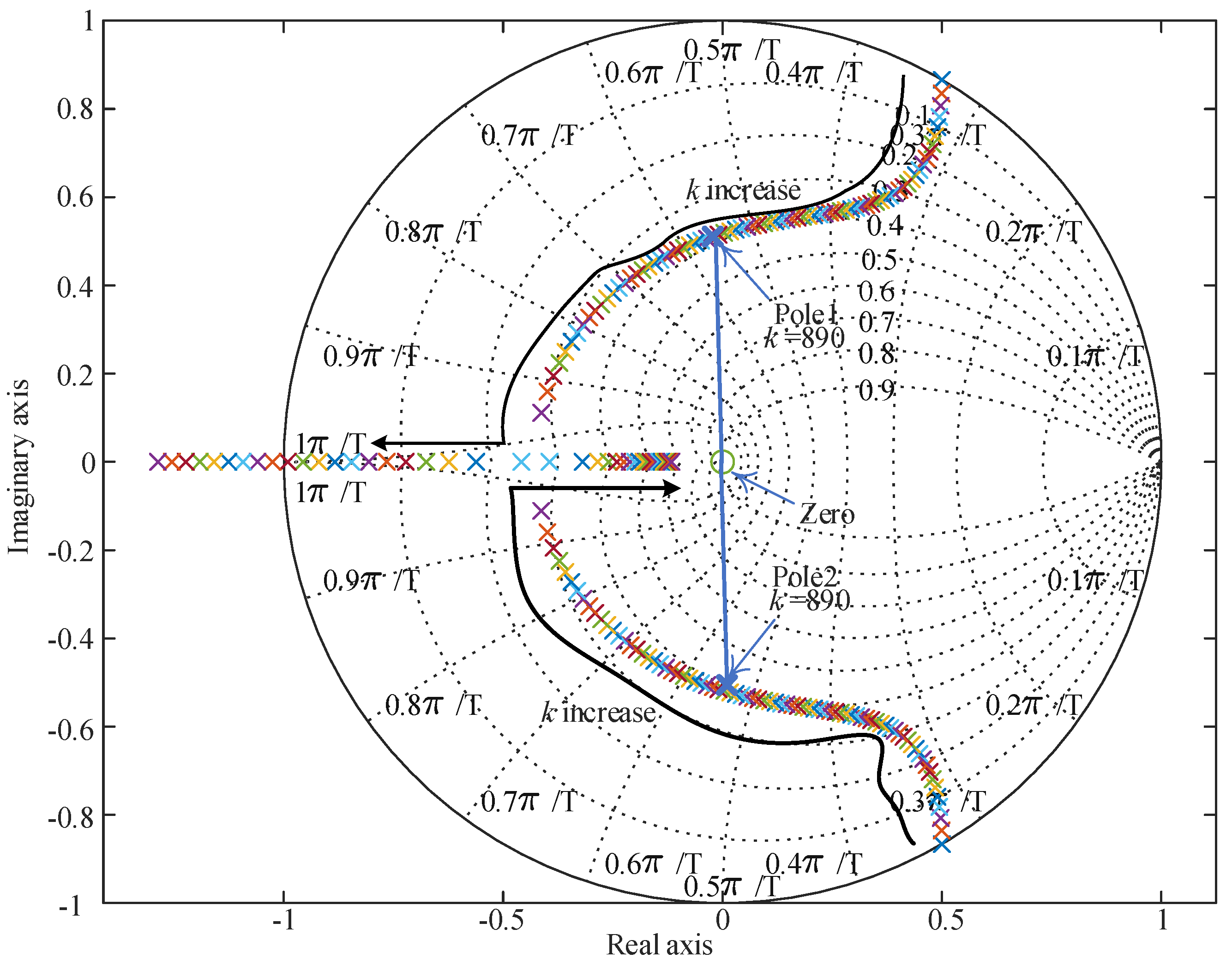
3.2. Design of Internal Model Current Decoupling Controller in Discrete Domain

When the switching frequency is low, the current internal model controller should be designed in the discrete domain (z-domain). The z-domain equivalent structure diagram of the internal model control system is shown in Figure 9, where z⁻¹ represents the model of the sampling and control delay, and the zero-order holder represents the model of the inverter.

F(z) is used to reduce the high-frequency oscillation; its z-domain transfer function is expressed as follows:

$$F(z)={\displaystyle \frac{k^2{T}_{\mathrm{s}}^2{z}^{-2}}{(1+\sqrt{2}k{T}_{\mathrm{s}})-(1+\sqrt{2}k{T}_{\mathrm{s}})z^{-1}+(1+k^2{T}_{\mathrm{s}}^2)z^{-2}}}$$

(15)

where k is the coefficient of F(z), and T_s is the switching period.

The s-domain transfer function G_zoh(s) of the zero-order holder is expressed as follows:

$$G_{\mathrm{zoh}}(s)={\displaystyle \frac{1-e^{T_{\mathrm{s}}s}}{s}}$$

(16)

Considering the sampling delay and zero-order holder, the G_m(s) is discretized, the transfer function of G_m(z) is obtained as follows:

$$\begin{array}{ll}{G}_{\mathrm{m}}(z)& =z^{-1}Z[G_{\mathrm{zoh}}(s)G_{\mathrm{m}}(s)]\\ & =z^{-1}(1-z^{-1})Z[{\displaystyle \frac{1}{s(\sigma L_{\mathrm{s}}s+R_{\mathrm{s}}^{\prime }+j\sigma L_{\mathrm{s}}{\omega }_{\mathrm{e}})}}]\\ & ={\displaystyle \frac{(1-e^{-({R^{\prime }}_{\mathrm{s}}/\sigma L_{\mathrm{s}}+j{\omega }_{\mathrm{e}})T_{\mathrm{s}}})z^{-2}}{e^{j{\omega }_{\mathrm{e}}{T}_{\mathrm{s}}}({R^{\prime }}_{\mathrm{s}}+j{\omega }_{\mathrm{e}}\sigma L_{\mathrm{s}})(1-e^{-({R^{\prime }}_{\mathrm{s}}/\sigma L_{\mathrm{s}}+j{\omega }_{\mathrm{e}})T_{\mathrm{s}}}{z}^{-1})}}\end{array}$$

(17)

The transfer function of G_imc(z) is derived as follows:

$$G_{\mathrm{imc}}(z)={\displaystyle \frac{k^2{T}_{\mathrm{s}}^2{e}^{j{\omega }_{\mathrm{e}}{T}_{\mathrm{s}}}({R^{\prime }}_{\mathrm{s}}+j{\omega }_{\mathrm{e}}\sigma L_{\mathrm{s}})(z-e^{-({R^{\prime }}_{\mathrm{s}}/\sigma L_{\mathrm{s}}+j{\omega }_{\mathrm{e}})T_{\mathrm{s}}})}{(1-e^{-({R^{\prime }}_{\mathrm{s}}/\sigma L_{\mathrm{s}}+j{\omega }_{\mathrm{e}})T_{\mathrm{s}}})[(1+\sqrt{2}k{T}_{\mathrm{s}})-(1+\sqrt{2}k{T}_{\mathrm{s}})z^{-1}+(1+k^2{T}_{\mathrm{s}}^2)z^{-2}]}}$$

(18)

The open-loop transfer function G_open(z) of Figure 9 can be derived as follows:

$$G_{\mathrm{open}}(z)=G_{\mathrm{imc}}(z)G_{\mathrm{m}}(z)={\displaystyle \frac{k^2{T}_{\mathrm{s}}^2{z}^{-1}}{(1+\sqrt{2}k{T}_{\mathrm{s}})-(1+\sqrt{2}k{T}_{\mathrm{s}})z^{-1}+(1+k^2{T}_{\mathrm{s}}^2)z^{-2}}}$$

(19)

The closed-loop transfer function G_close(z) of Figure 9 is derived as follows:

$$G_{\mathrm{close}}(z)={\displaystyle \frac{G_{\mathrm{open}}(z)}{1+G_{\mathrm{open}}(z)}}={\displaystyle \frac{k^2{T}_{\mathrm{s}}^{2}z}{(1+\sqrt{2}k{T}_{\mathrm{s}})z^2+(k^2{T}_{\mathrm{s}}^2-\sqrt{2}k{T}_{\mathrm{s}}-1)z^1+k^2{T}_{\mathrm{s}}^2+1}}$$

(20)

It can be seen that no item in G_close(z) contains ω_e. When k varies within the range of [0, 2000], the zero-pole distribution diagram of the current closed-loop transfer function is shown in Figure 10. There are two poles and one zero, and the zero is located at the center of the unit circle. As k increases, the two conjugate poles move to the left while approaching the real axis. According to the discrete-time control theory, as the distance between the pole and the center of the unit circle decreases, the dynamic response becomes faster. As the z-domain conjugate poles shift to the left, the imaginary part of the corresponding s-domain conjugate poles increases, leading to an increase in oscillations during the dynamic process. In Figure 10, when k is greater than 890, the distance between the pole and the center of the unit circle decreases marginally, so k is determined as 890 in this paper.

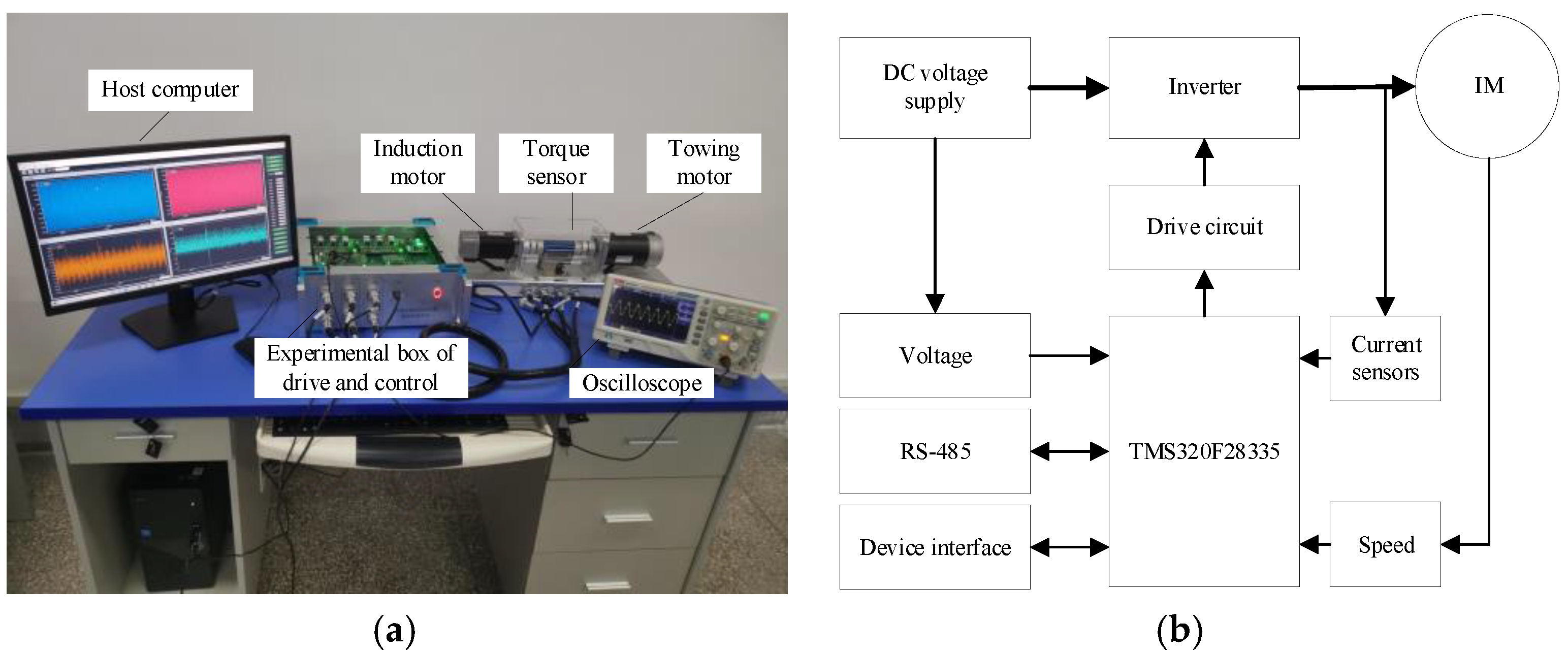
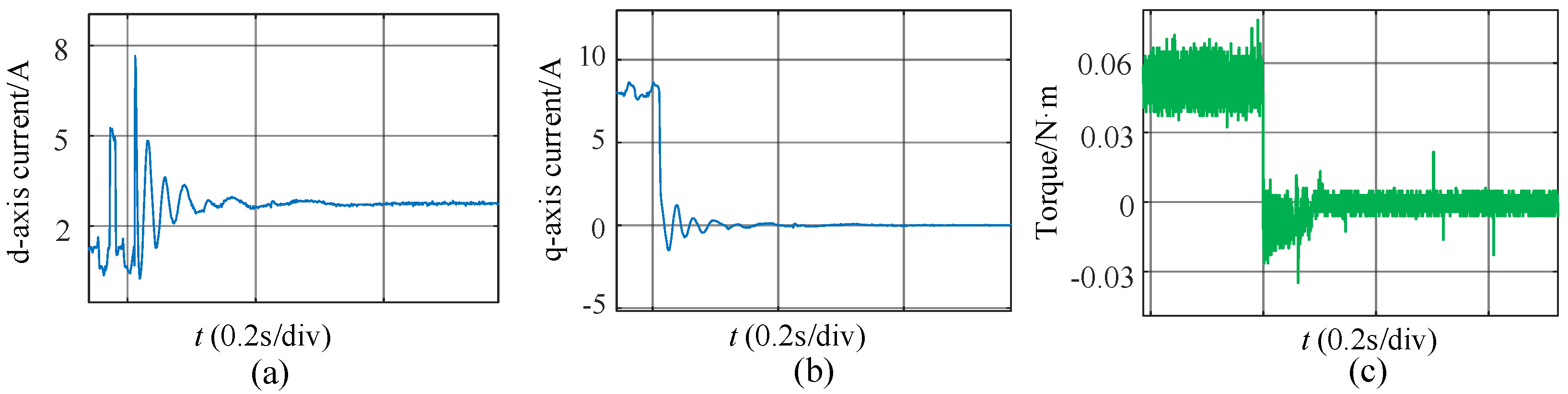
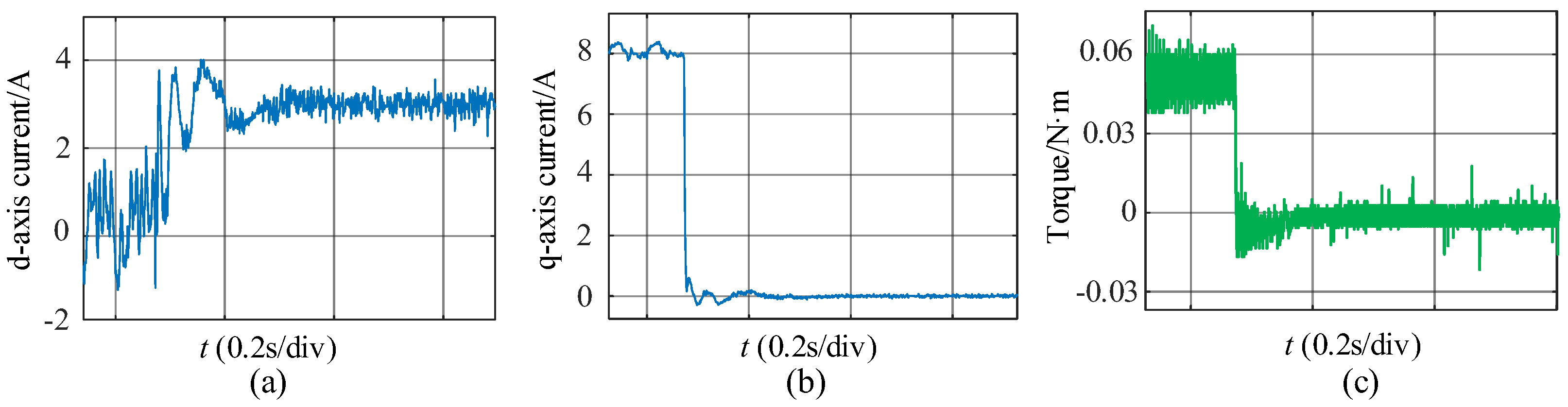
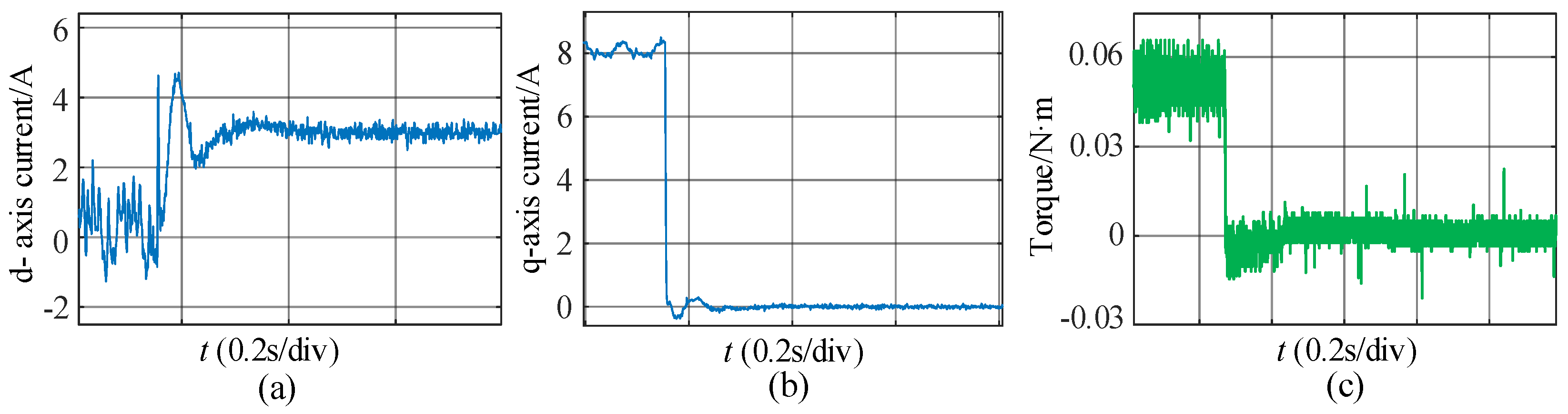
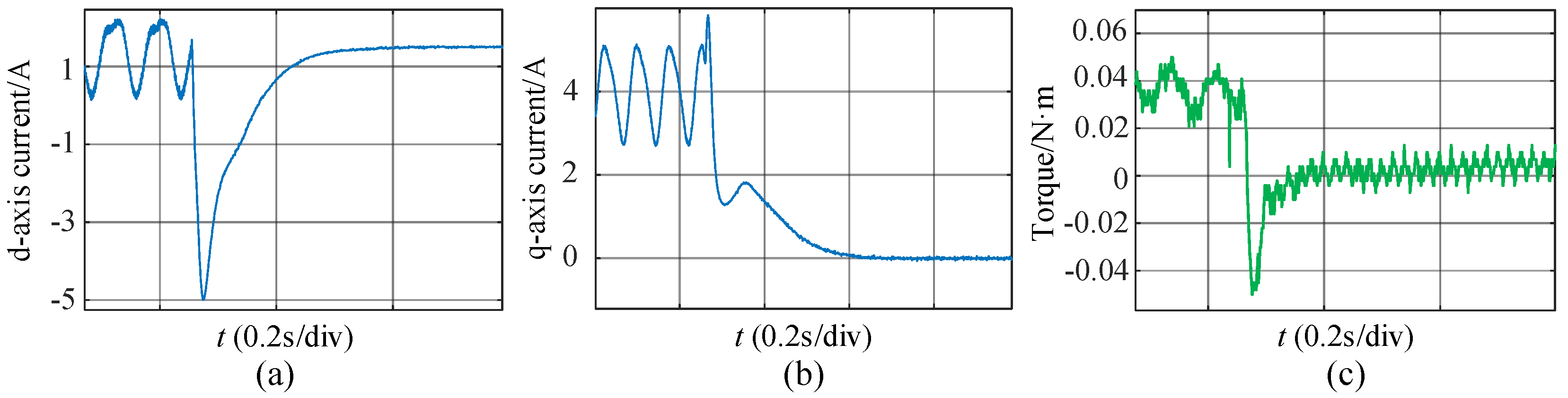
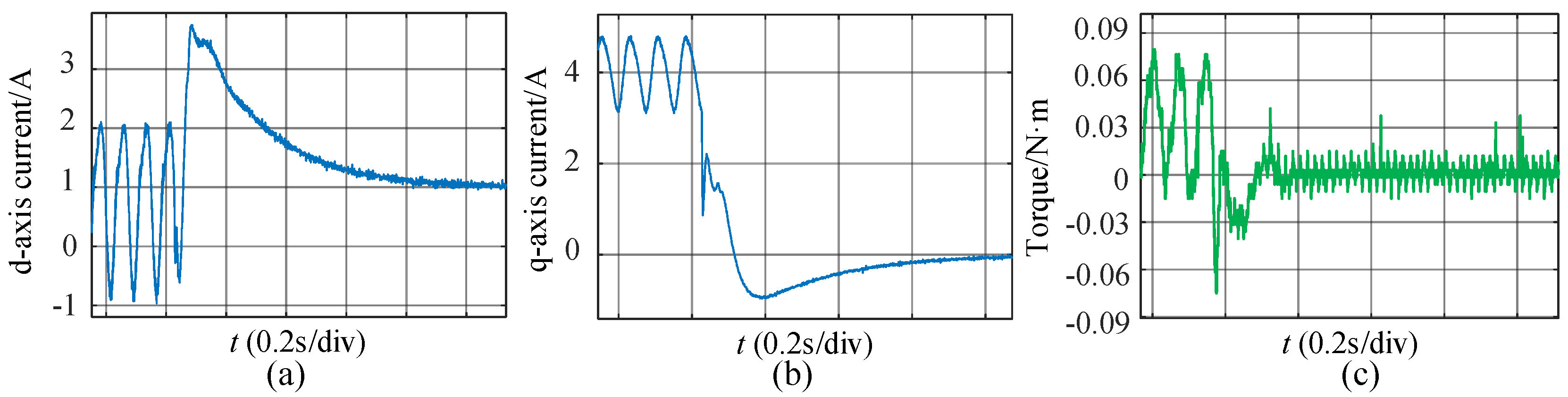
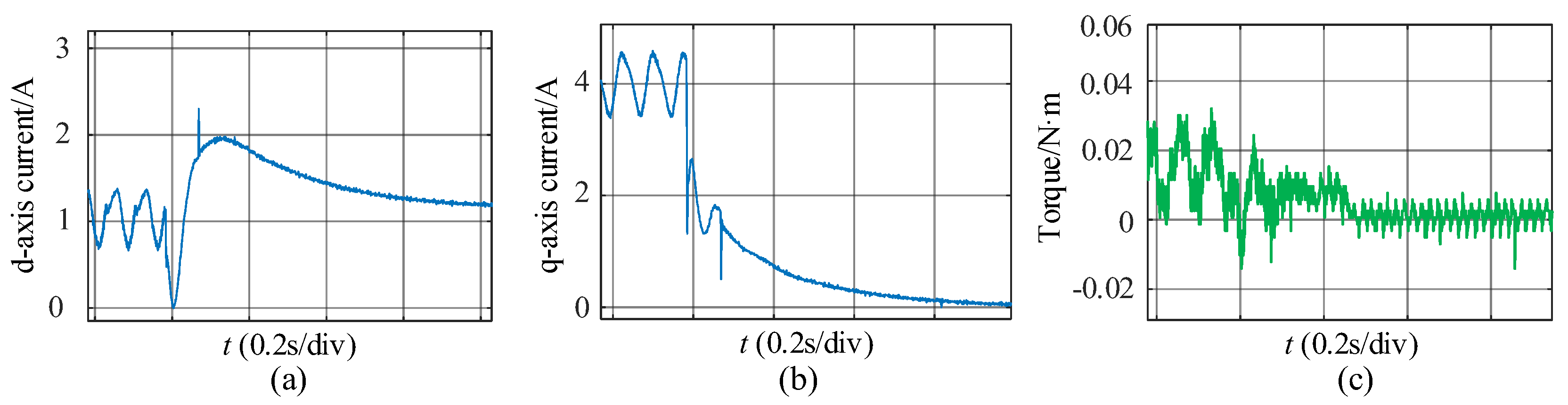
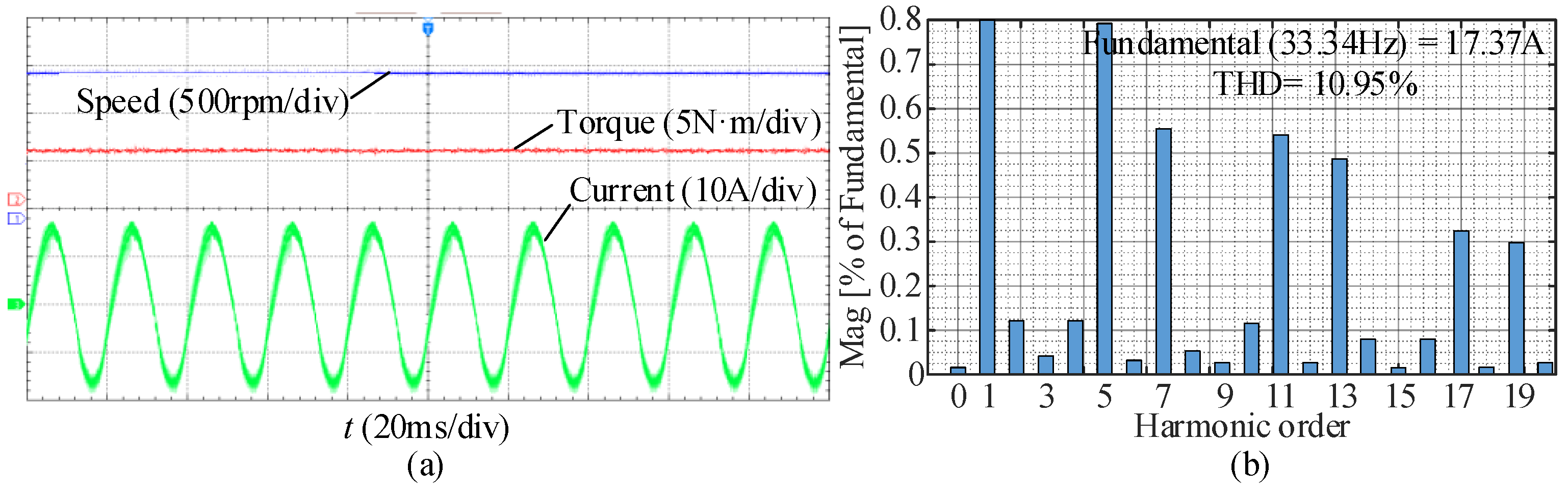
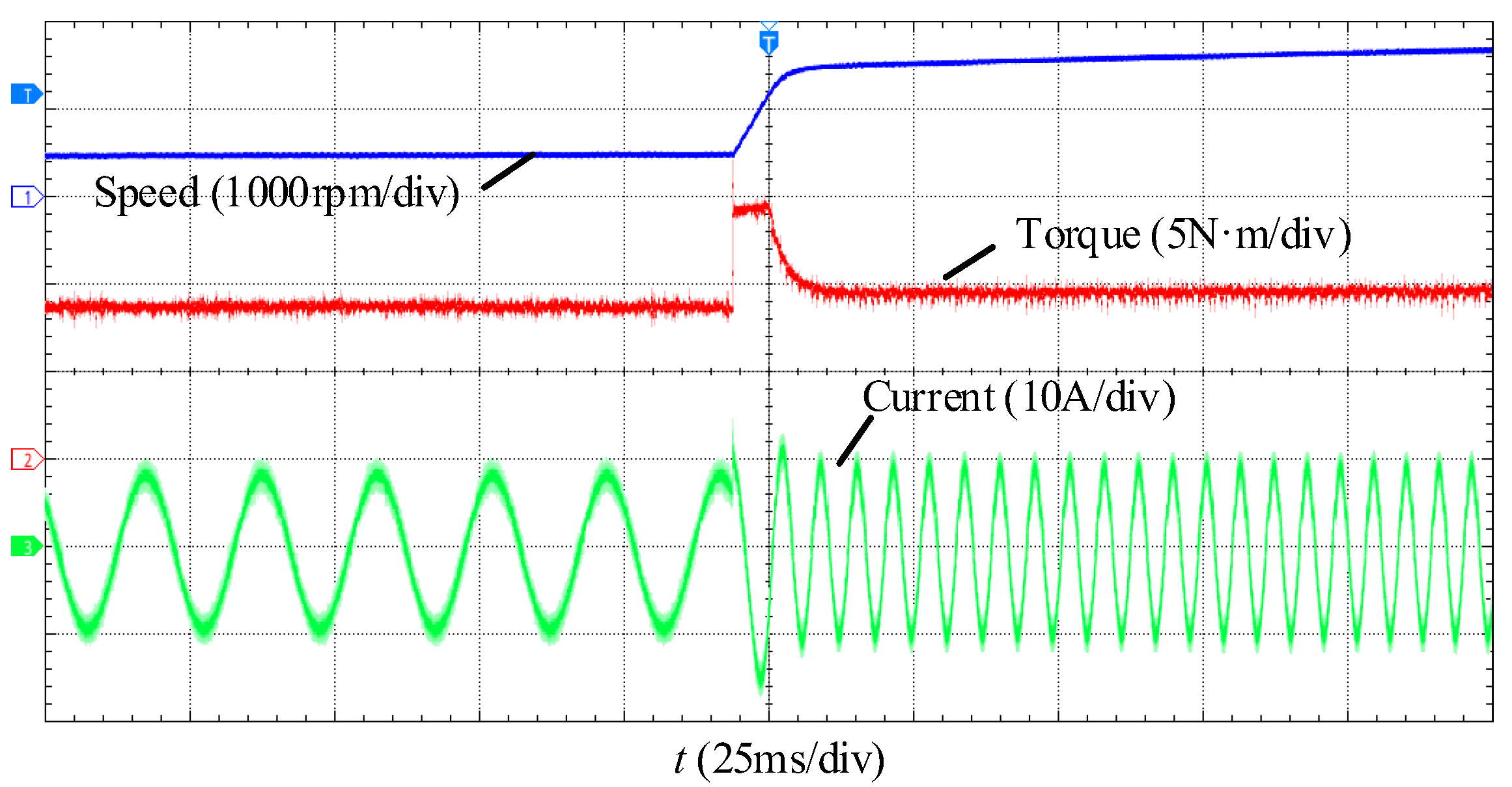
4. Experimental Results

The experimental images and structure diagram used in this paper are shown in Figure 11. The experimental system mainly consists of four parts: an induction motor towing platform, an experimental box of drive and control, a cSPACE upper computer, and related sensors. The parameters of the induction motor have been listed in Table 1. The sensors used in the experimental system mainly include a torque sensor (type: WTQ1050D, bandwidth: 6 kHz, Nanli Measurement and Control Equipment Co., Ltd., Donguan, China), a hall current sensor (type: ACS711ELCTR-25AB-T, Allegro MicroSystems, Worcester, MA, USA), and photoelectric incremental encoders (type: TS5700N8501, Tamagawa, Tokyo, Japan) with a wire count of 2500PPR. The control algorithm is implemented by the digital signal processor (type: TMS320F28335, Texas instruments, Dallas, TX, USA), and the adopted numerical integration algorithm is the forward Euler method. The driving system is mainly composed of six driving chips (type: IRS923STRPBF, Infineon, Munich, Germany). In addition, the experimental box includes a Power module, RS-485 bus, USB to serial port, torque signal amplifier, etc.

The PWM frequency is set to 15 kHz, the reference of the speed is 2500 rpm, and the IM operates with no load. The references of i_d and i_q are set as 1 A and 8 A, respectively. When the IM operates in a steady state, the references of i_d and i_q are step-changed to 3 A and 0 A at different times. The dynamic experimental waveforms of torque, i_d and i_q, under three different control methods are shown in Figure 12, Figure 13, and Figure 14, respectively.

Figure 12 shows the d-axis and q-axis current and torque responses when using a PI current controller without decoupling compensation. From Figure 13a,b, it can be observed that the d-axis and q-axis current exhibit oscillatory decay, with the d-axis current oscillating peak exceeding 7 A and the q-axis current oscillating peak reaching −2 A, which indicates a strong d–q axis cross-coupling. Figure 13 shows the feedforward decoupling dynamic response under the same conditions. Compared with no decoupling shown in Figure 12, the peak value of d-axis current oscillation during feedforward decoupling in Figure 13a has decreased but still exceeds 6 A. The electromagnetic torque of Figure 13c exhibits negative overshoot due to cross-coupling, with a peak overshoot of approximately 0.03 N·m. Figure 14 shows the experimental waveform when using the proposed internal model current controller. Due to the presence of cross-integration terms, it can be observed that the overshoot of the d-axis stator current and q-axis stator current relative to the references are significantly reduced, the peak value of the d-axis stator current oscillation is below 4 A, and the peak value of electromagnetic torque overshoot in Figure 15c is only 0.015 N·m, which is about 50% lower than that with no decoupling and feedforward decoupling.

Due to the fact that the motor parameter involved in the cross-decoupling term in the internal model current controller is stator leakage inductance, Figure 15 shows the decoupling effect of the internal model current controller under $\sigma L_{\mathrm{s}}=2\widehat{\sigma }{\widehat{L}}_s$. Compared to experimental waveforms in Figure 14 without parameter mismatch, the dynamic responses of the d-axis stator current, the q-axis current, and electromagnetic torque have little variation, and the experimental results are consistent with the theoretical analysis. Therefore, the proposed internal model control scheme has the ability to suppress the effects of the stator inductance mismatch.

Next, the effectiveness of the designed internal model current controller in the z-domain will be verified. The switching frequency is reduced to 2 kHz, the reference of the speed is 500 rpm, and the IM operates with no load. The references of i_d and i_q are set as 1 A and 4 A, respectively. When the IM operates at a steady state, the reference of i_q is step-changed to 0A. The dynamic experimental waveforms of torque, i_d and i_q, under four different control methods are shown in Figure 16, Figure 17, Figure 18, and Figure 19, respectively.

Figure 16 shows the d-axis and q-axis current and torque responses when using a PI current controller without decoupling compensation. Compared with Figure 12, due to a low switching frequency, the ripple of the d-axis current increases, and with the sudden change in the q-axis stator current, i_d exhibits attenuation oscillation. As a result, an overshoot of −0.15 N·m occurs in electromagnetic torque, as shown in Figure 16c. Figure 17 shows the feedforward decoupling dynamic response under the same conditions. Compared with no decoupling shown in Figure 16, the d-axis stator current no longer decays and oscillates, but there is an impulse of −5A. The overshoot of electromagnetic torque is −0.05 N·m, which is less than that of decoupling. Figure 18 shows the dynamic experimental waveforms under the designed internal model current decoupling controller in the s-domain; the overshoot of the d-axis stator current is 3.6 A, and the dynamic performance of the electromagnetic torque is weaker than that of feedforward decoupling, which indicates that the designed internal model current decoupling controller in the s-domain does not have excellent dynamic performance at low switching frequency. Figure 19 shows the dynamic experimental waveforms under the designed internal model current decoupling controller in the z-domain; the overshoot of the d-axis stator current is limited, the electromagnetic torque variation in Figure 19c is smoother, and the overshoot is significantly limited to only −0.01 N·m. Therefore, the theoretical analysis in Part 3.2 of Section 3 is correct.

Then, the steady state under the designed internal model current controller in the z-domain is compared with the traditional PI current controller with feedforward decoupling. The switching frequency is reduced to 2 kHz, the reference of the speed is 1500 rpm, the IM operates with the load of 5 N·m, and the reference of i_d is set as 5 A. The steady-state waveforms and FFT analysis results are shown in Figure 20 and Figure 21.

Since the switching frequency is low, in Figure 20, the ripple of the torque under the traditional PI current controller is great, and the THD of the current is 14.38%. Since the proposed internal model current decoupling controller in the z-domain can compensate for control delay caused by low switching frequency, in Figure 21, the torque ripple and the current harmonic distortion are less than those under the traditional PI current controller, so a better steady-state performance can be obtained by the proposed control method.

To further verify the effectiveness of the proposed internal model control method under speed increasing, the dynamic experimental waveforms when the speed changes from 400 rpm to 1600 rpm with a load of 8 N·m are shown in Figure 22. The acceleration time is shorter than 15 ms, and no overshoot or oscillation occurs in the dynamic process, which shows that good dynamic performance can be obtained by the proposed internal model control method.

5. Conclusions

This paper proposes an internal model current decoupling control strategy to suppress the stator internal coupling effect; the coupling characteristic of IMs is analyzed, and the design details for the structure and parameters of the internal model controller are presented in continuous and discrete domains, respectively. Finally, its feasibility and effectiveness have been proven by the experimental results, and the following conclusions are formed:

(1)

There is a complex pole in the IM mathematical model: as the synchronous speed increases, the imaginary axis component of the complex pole increases, resulting in an increasing oscillation during the dynamic process;

(2)

The internal model controller generates a zero at the same position as the complex pole, eliminating the stator’s internal coupling. Meanwhile, it is insensitive to stator leakage inductance;

(3)

Considering sampling and control delay, an internal model controller is designed in a discrete domain, the parameter is determined according to the phase of the pole and the distance between the pole and the center of the unit circle. A good dynamic performance can be obtained under the switching frequency of 2 kHz.