Inverse Identification of Elastic Properties of Constituents of Discontinuously Reinforced Composites
Abstract
1. Introduction
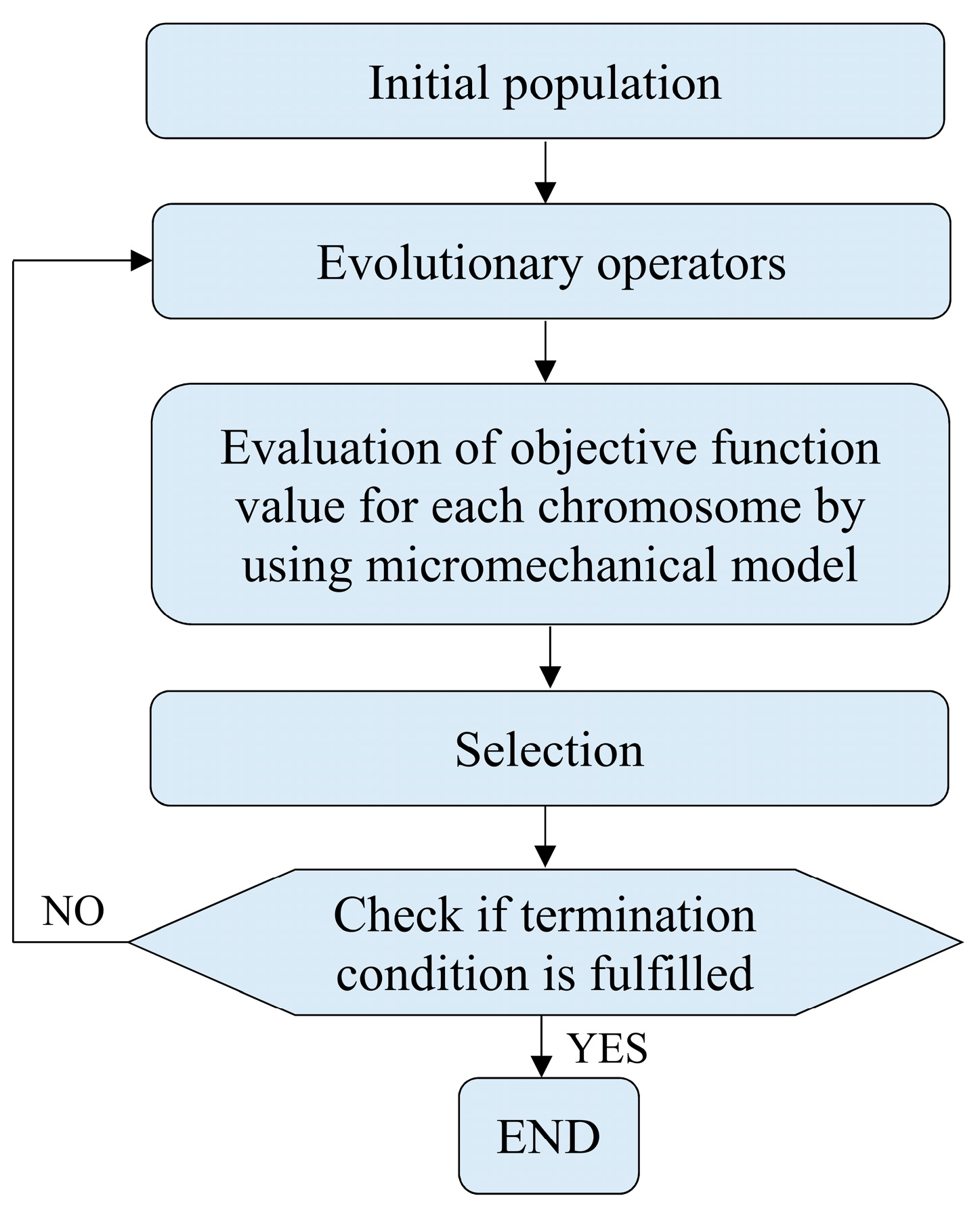
2. Identification Procedure
2.1. Optimization Problem
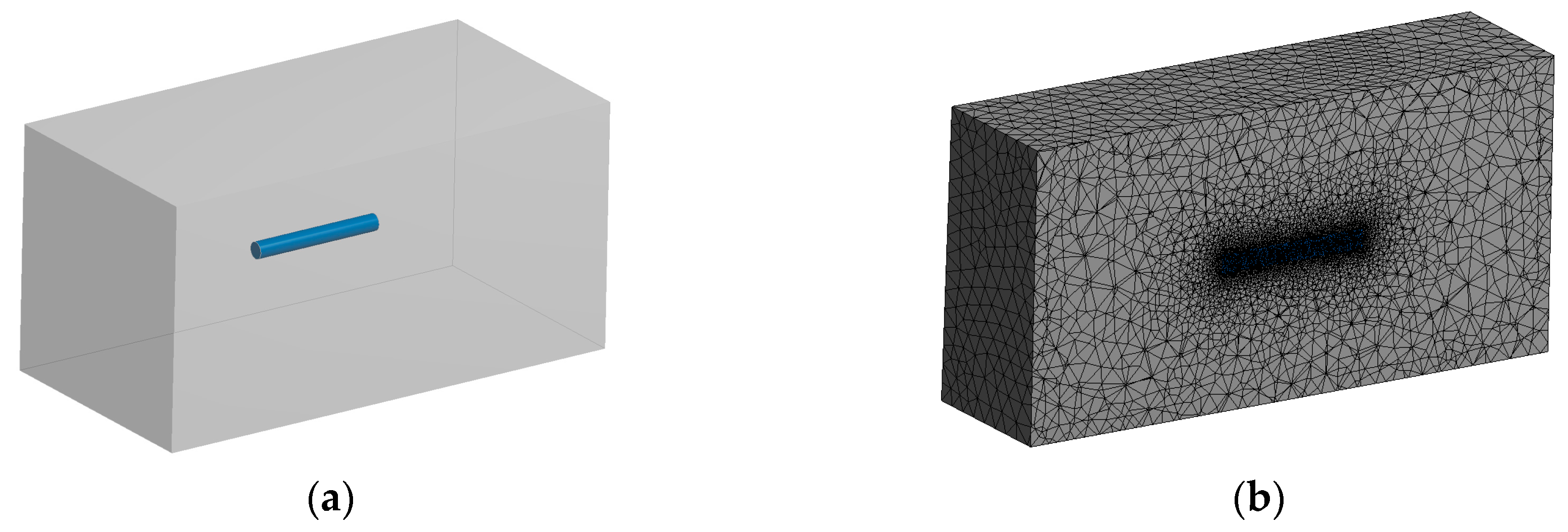
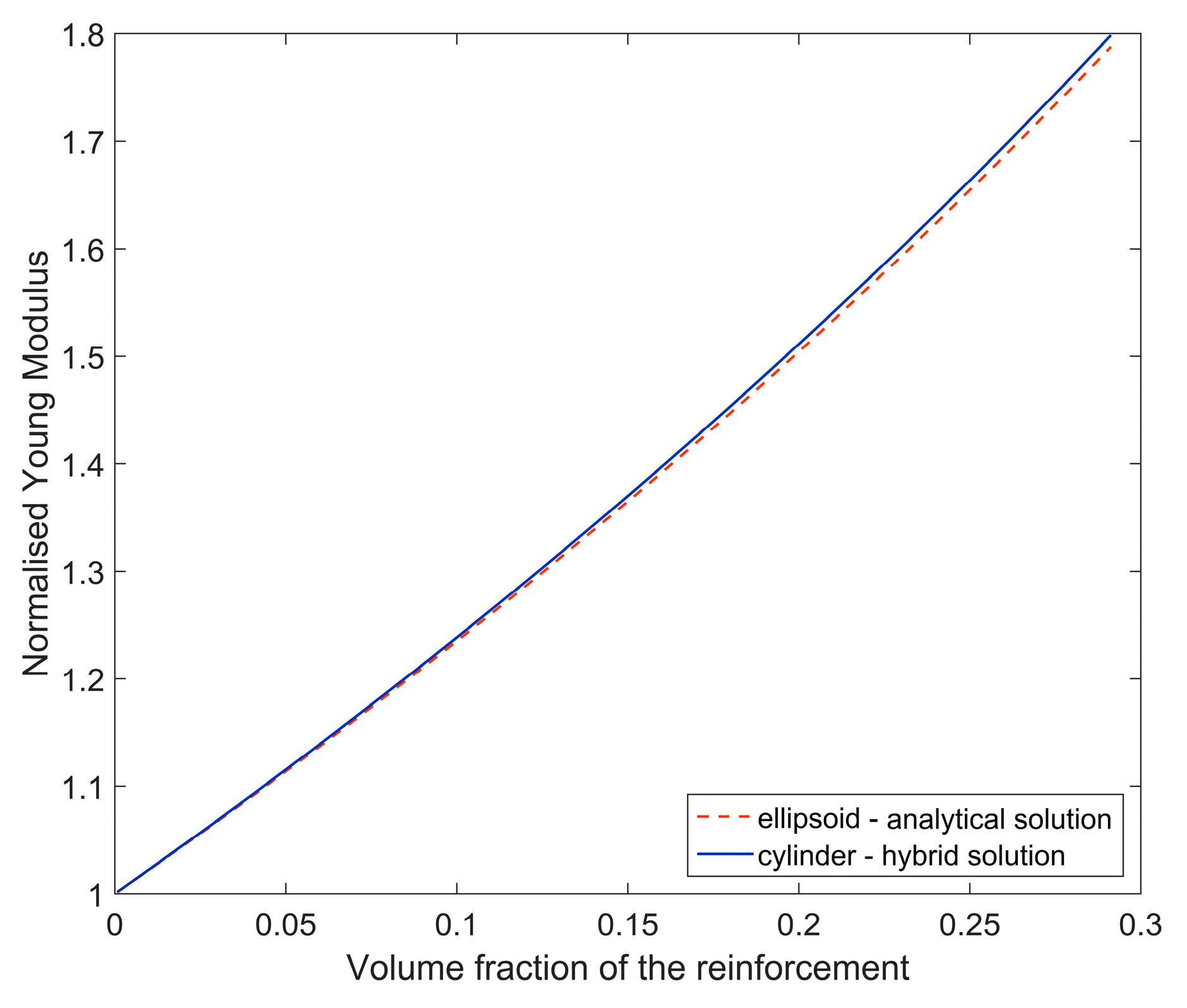
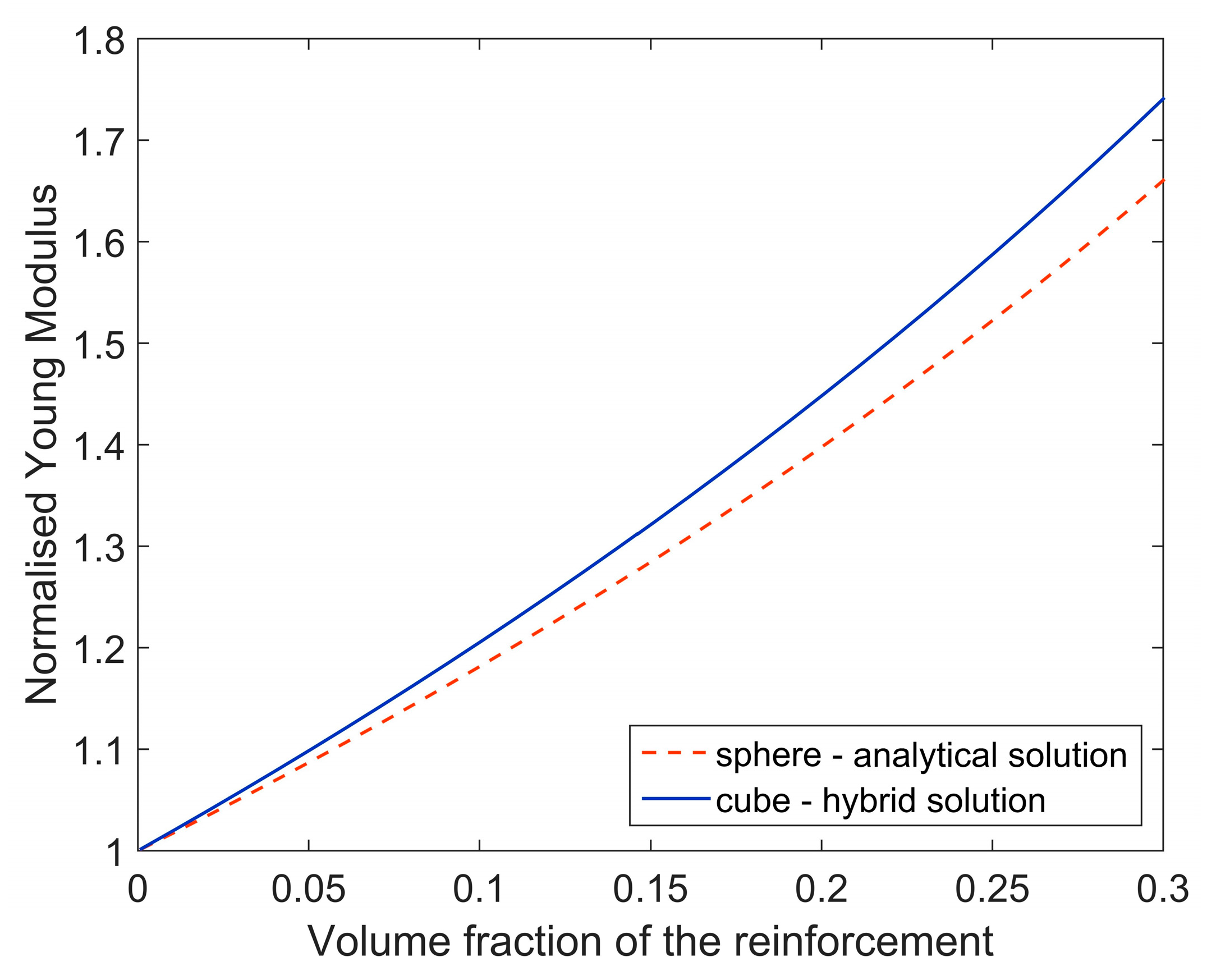
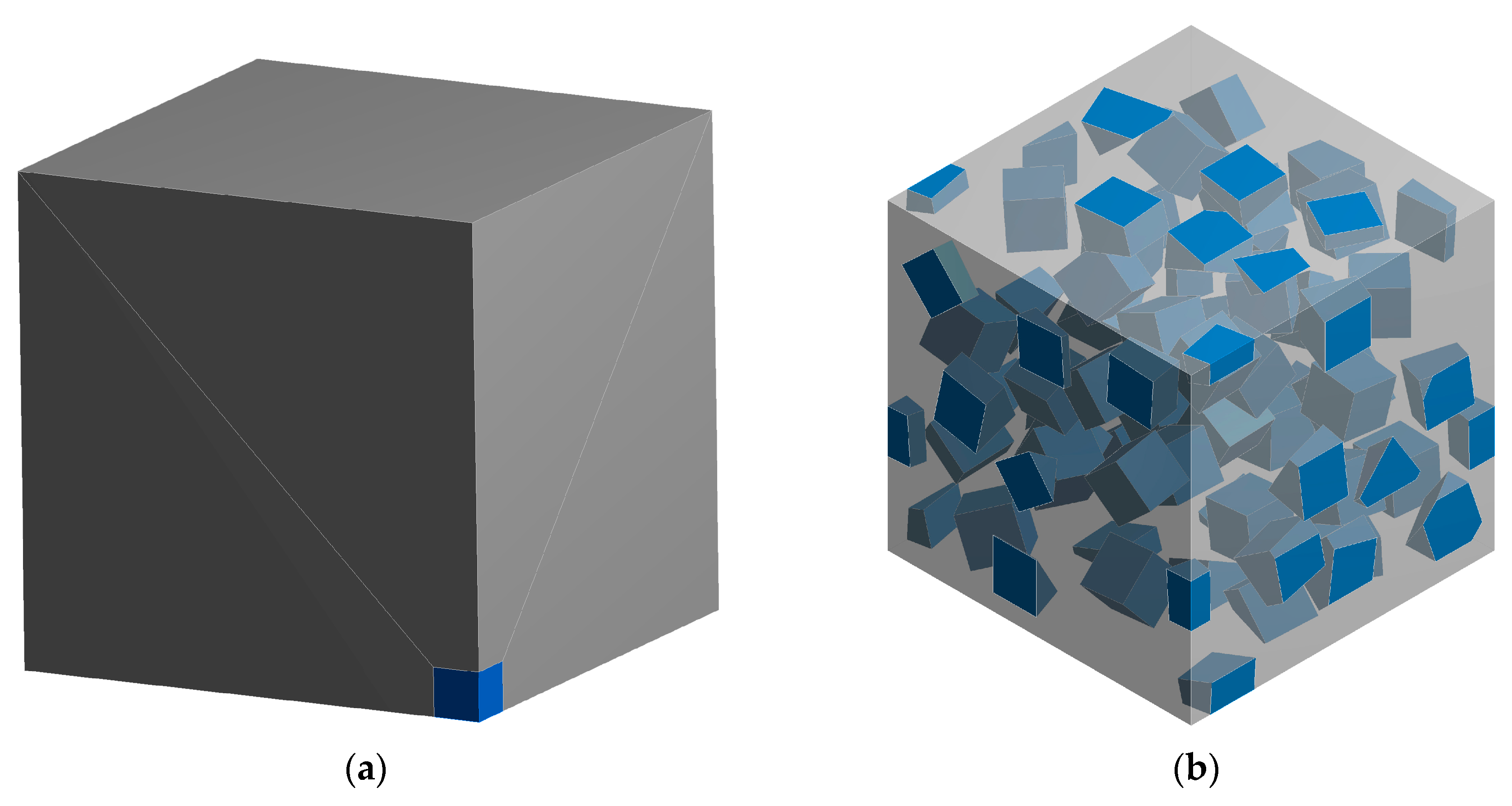
2.2. Micromechanical Modeling
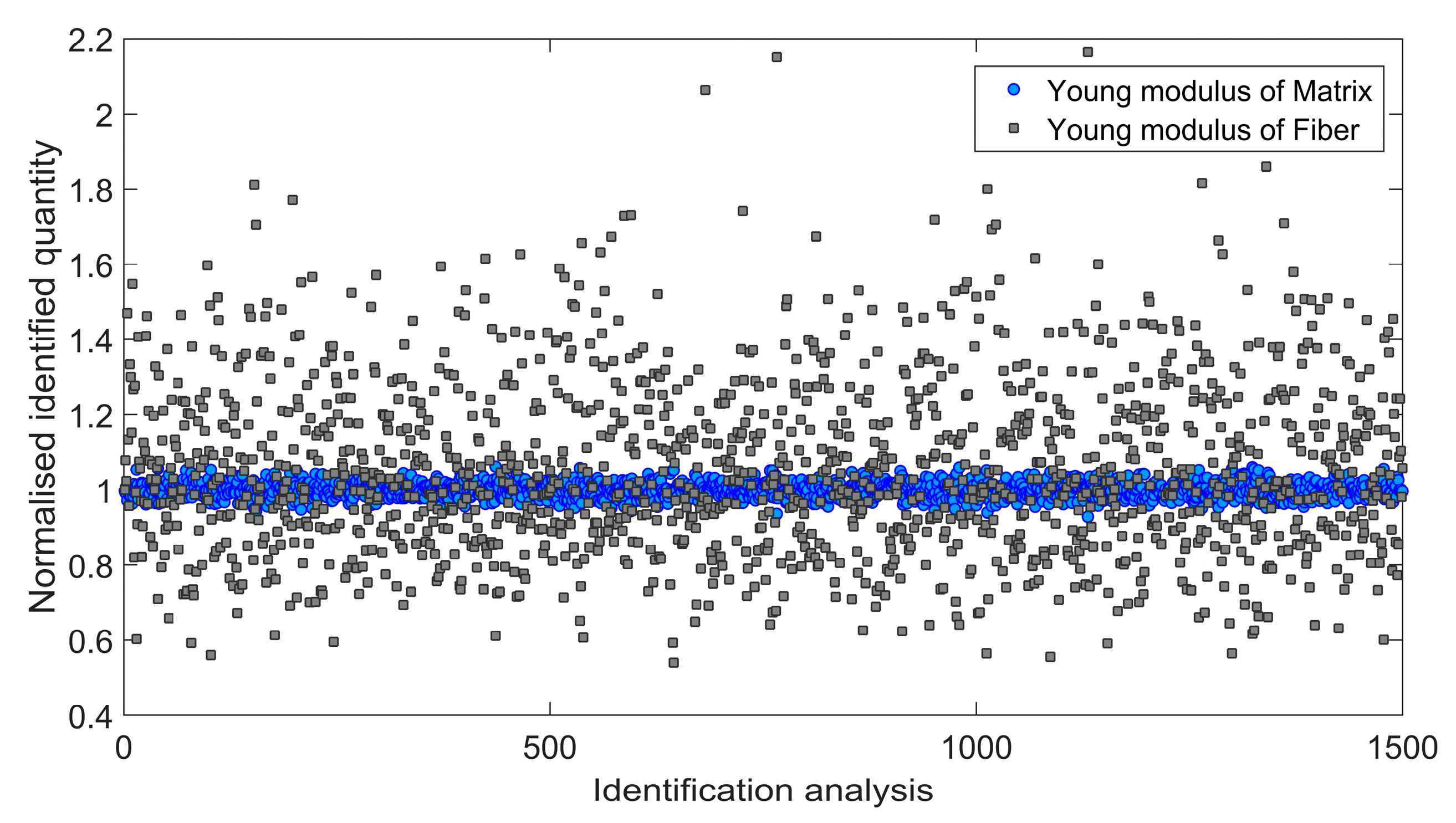
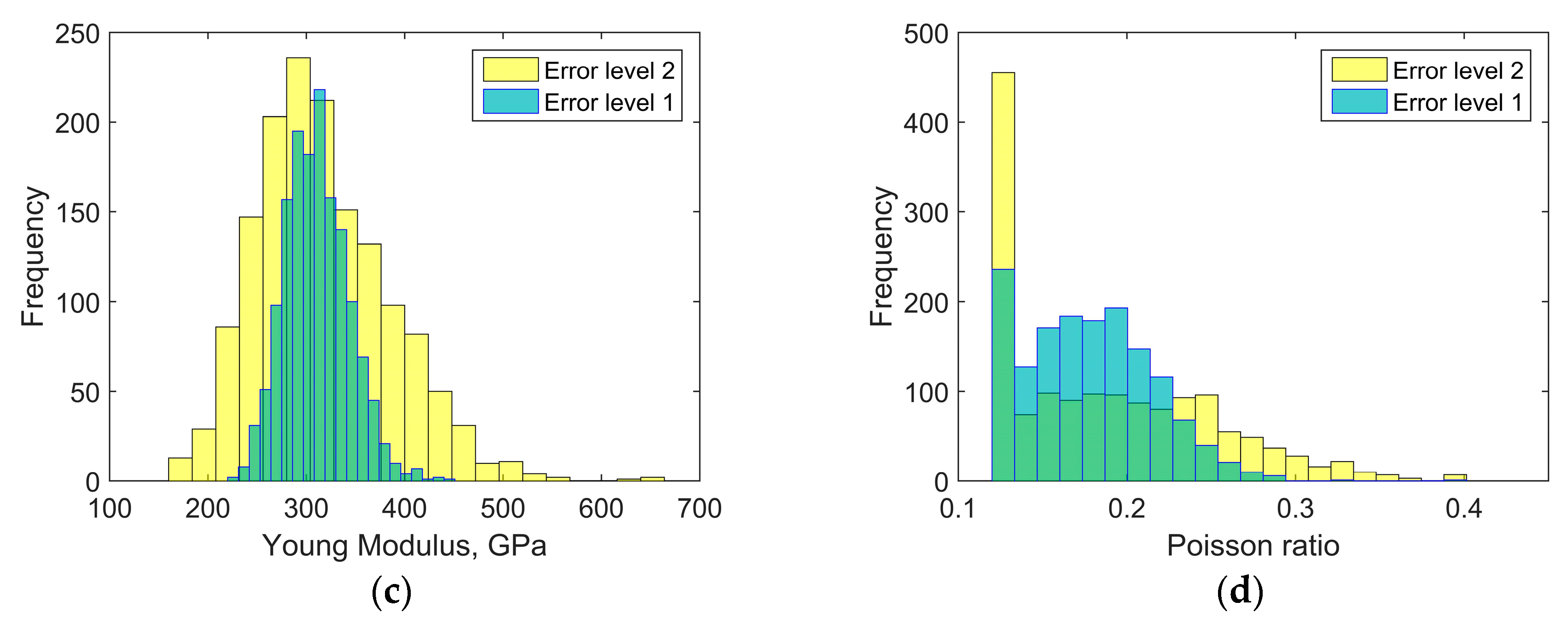
3. Results and Discussion
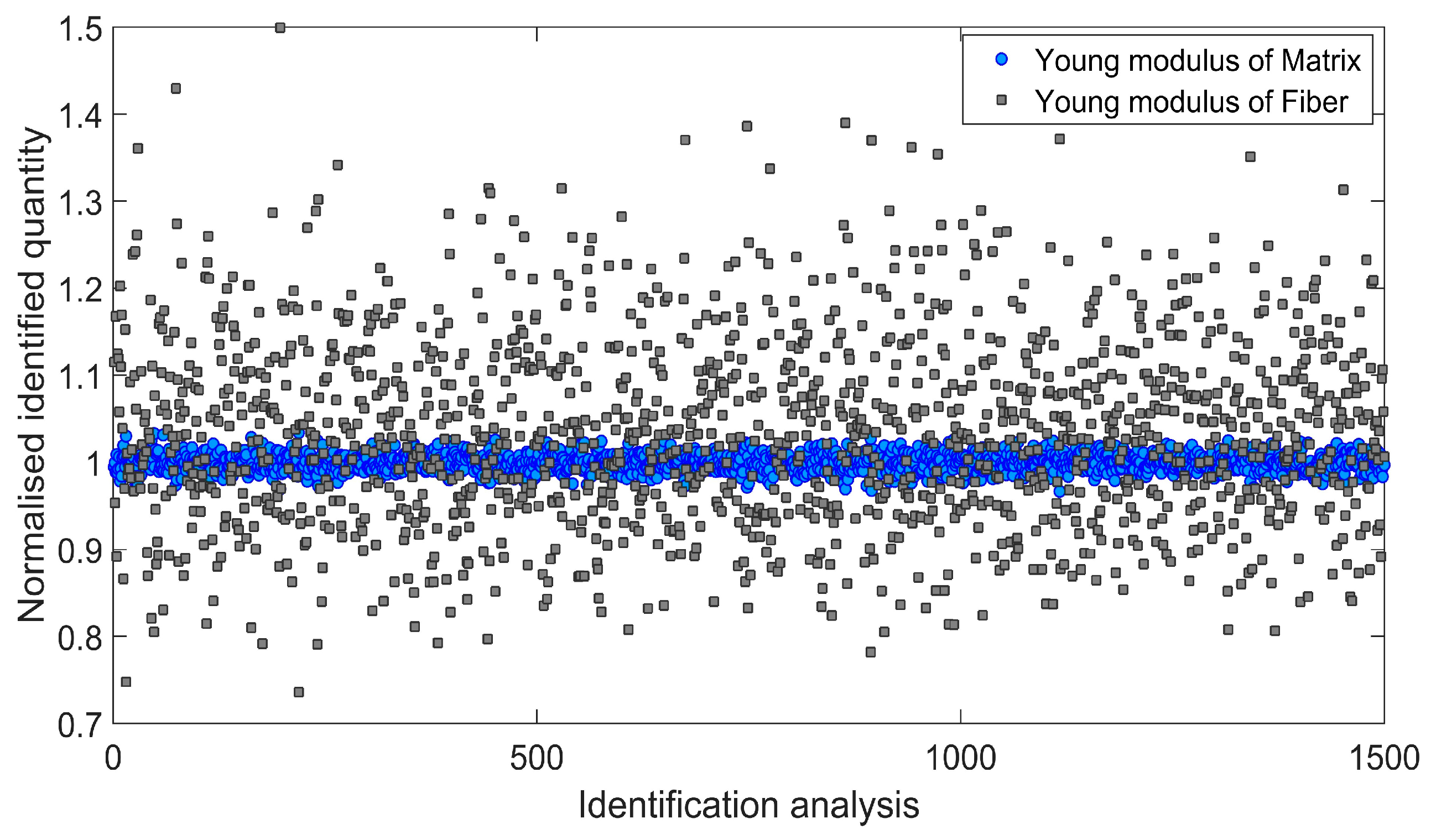
3.1. Composite Reinforced with Short Fibers
3.2. Composite Reinforced with Cubic Particles
4. Concluding Remarks
Funding
Conflicts of Interest
References
- Wang, H.; Li, G.; Zhao, Y.; Chen, G. In situ fabrication and microstructure of Al2O3 particles reinforced aluminum matrix composites. Mater. Sci. Eng. A 2010, 527, 2881–2885. [Google Scholar] [CrossRef]
- Ma, F.; Liu, P.; Li, W.; Liu, X.; Chen, X.; Zhang, K.; Pan, D.; Lu, W. The mechanical behavior dependence on the TiB whisker realignment during hot-working in titanium matrix composites. Sci. Rep. 2016, 6, 1–9. [Google Scholar] [CrossRef] [PubMed]
- Tong, X.C.; Ghosh, A.K. Fabrication of in situ TiC reinforced aluminum matrix composites. J. Mater. Sci. 2001, 36, 4059–4069. [Google Scholar] [CrossRef]
- Zhang, D.; Bai, F.; Sun, L.; Wang, Y.; Wang, J. Compression properties and electrical conductivity of in-situ 20 vol.% nano-sized TiCx/Cu composites with different particle size and morphology. Materials 2017, 10, 499. [Google Scholar] [CrossRef] [PubMed]
- Barile, C.; Casavola, C.; Pappalettere, C. The influence of stitching and unconventional fibres orientation on the tensile properties of CFRP laminates. Compos. Part B Eng. 2017, 110, 248–254. [Google Scholar] [CrossRef]
- Santhanakrishnan Balakrishnan, V.; Wartig, K.; Tsombanis, N.; Seidlitz, H. Influence of processing parameters on the impact behaviour of glass/polyamide-6 composite. Compos. Part B Eng. 2019, 159, 292–299. [Google Scholar] [CrossRef]
- Fernández, R.; Cabeza, S.; Mishurova, T.; Fernández-Castrillo, P.; González-Doncel, G.; Bruno, G. Residual stress and yield strength evolution with annealing treatments in an age-hardenable aluminum alloy matrix composite. Mater. Sci. Eng. A 2018, 731, 344–350. [Google Scholar] [CrossRef]
- Constantinides, G.; Ravi Chandran, K.S.; Ulm, F.J.; Van Vliet, K.J. Grid indentation analysis of composite microstructure and mechanics: Principles and validation. Mater. Sci. Eng. A 2006, 430, 189–202. [Google Scholar] [CrossRef]
- Hardiman, M.; Vaughan, T.J.; McCarthy, C.T. A review of key developments and pertinent issues in nanoindentation testing of fibre reinforced plastic microstructures. Compos. Struct. 2017, 180, 782–798. [Google Scholar] [CrossRef]
- Singh, D.R.P.; Chawla, N.; Tang, G.; Shen, Y.L. Micropillar compression of Al/SiC nanolaminates. Acta Mater. 2010, 58, 6628–6636. [Google Scholar] [CrossRef]
- Mayer, C.R.; Yang, L.W.; Singh, S.S.; Llorca, J.; Molina-Aldareguia, J.M.; Shen, Y.L.; Chawla, N. Anisotropy, size, and aspect ratio effects on micropillar compression of Al-SiC nanolaminate composites. Acta Mater. 2016, 114, 25–32. [Google Scholar] [CrossRef]
- Kuś, W.; Burczyński, T. Bioinspired Algorithms in Multiscale Optimization. In Computer Methods in Mechanics. Advanced Structured Materials; Springer: Berlin, Germany, 2010. [Google Scholar]
- Kaiser, J.M.; Stommel, M. Inverse determination of modeling parameters to consider inhomogeneities of semicrystalline thermoplastics in structure simulations. Arch. Appl. Mech. 2013, 83, 889–897. [Google Scholar] [CrossRef]
- Beluch, W.; Burczyński, T. Two-scale identification of composites’ material constants by means of computational intelligence methods. Arch. Civ. Mech. Eng. 2014, 14, 636–646. [Google Scholar] [CrossRef]
- Herrera-Solaz, V.; Llorca, J.; Dogan, E.; Karaman, I.; Segurado, J. An inverse optimization strategy to determine single crystal mechanical behavior from polycrystal tests: Application to AZ31 Mg alloy. Int. J. Plast. 2014, 57, 1–15. [Google Scholar] [CrossRef]
- Comellas, E.; Valdez, S.I.; Oller, S.; Botello, S. Optimization method for the determination of material parameters in damaged composite structures. Compos. Struct. 2015, 122, 417–424. [Google Scholar] [CrossRef]
- Grédiac, M. The use of full-field measurement methods in composite material characterization: Interest and limitations. Compos. Part A Appl. Sci. Manuf. 2004, 35, 751–761. [Google Scholar] [CrossRef]
- Rahmani, B.; Mortazavi, F.; Villemure, I.; Levesque, M. A new approach to inverse identification of mechanical properties of composite materials: Regularized model updating. Compos. Struct. 2013, 105, 116–125. [Google Scholar] [CrossRef]
- Barile, C.; Casavola, C.; Pappalettera, G.; Pappalettere, C. Innovative mechanical characterization of materials by combining ESPI and numerical modelling. Int. J. Mech. 2016, 10, 115–123. [Google Scholar]
- Mrozek, A.; Kuś, W.; Burczyński, T. Nano level optimization of graphene allotropes by means of a hybrid parallel evolutionary algorithm. Comput. Mater. Sci. 2015, 106, 161–169. [Google Scholar] [CrossRef]
- Han, Y.; Lu, W. Evolutionary design of nonuniform cellular structures with optimized Poisson’s ratio distribution. Mater. Des. 2018, 141, 384–394. [Google Scholar] [CrossRef]
- Ogierman, W.; Kokot, G. Homogenization of inelastic composites with misaligned inclusions by using the optimal pseudo-grain discretization. Int. J. Solids Struct. 2017, 113, 230–240. [Google Scholar] [CrossRef]
- Poteralski, A. Hybrid artificial immune strategy in identification and optimization of mechanical systems. J. Comput. Sci. 2017, 23, 216–225. [Google Scholar] [CrossRef]
- Silva-Santos, C.H.; Goulart, P.R.; Bertelli, F.; Garcia, A.; Cheung, N. An artificial immune system algorithm applied to the solution of an inverse problem in unsteady inward solidification. Adv. Eng. Softw. 2018, 121, 178–187. [Google Scholar] [CrossRef]
- Burczyński, T.; Szczepanik, M. Intelligent optimal design of spatial structures. Comput. Struct. 2013, 127, 102–115. [Google Scholar] [CrossRef]
- He, L.; Su, H.; Xu, J.; Zhang, L. Inverse identification of constitutive parameters of Ti2AlNb intermetallic alloys based on cooperative particle swarm optimization. Chin. J. Aeronaut. 2018, 31, 1774–1785. [Google Scholar] [CrossRef]
- Zheng, Y.; Fish, J. Toward realization of computational homogenization in practice. Int. J. Numer. Methods Eng. 2008, 73, 361–380. [Google Scholar] [CrossRef]
- Kouznetsova, V.; Brekelmans, W.A.M.; Baaijens, F.P.T. Approach to micro-macro modeling of heterogeneous materials. Comput. Mech. 2001, 27, 37–48. [Google Scholar] [CrossRef]
- González, C.; LLorca, J. Mechanical behavior of unidirectional fiber-reinforced polymers under transverse compression: Microscopic mechanisms and modeling. Compos. Sci. Technol. 2007, 67, 2795–2806. [Google Scholar] [CrossRef]
- Burczyński, T.; Kuś, W.; Brodacka, A. Multiscale modeling of osseous tissues. J. Theor. Appl. Mech. 2010, 48, 855–870. [Google Scholar]
- Ogierman, W.; Kokot, G. Generation of representative volume elements of heterogeneous materials with distributed orientations of inclusions. Compos. Struct. 2018, 201, 636–646. [Google Scholar] [CrossRef]
- Eshelby, J.D. The determination of the elastic field of an ellipsoidal inclusion, and related problems. Proc. R. Soc. Lond. A. Math. Phys. Sci. 1957, 241, 376–396. [Google Scholar] [CrossRef]
- Benveniste, Y. Revisiting the generalized self-consistent scheme in composites: Clarification of some aspects and a new formulation. J. Mech. Phys. Solids 2008, 56, 2984–3002. [Google Scholar] [CrossRef]
- Benveniste, Y. A new approach to the application of Mori–Tanaka’s theory in composite materials. Mech. Mater. 1987, 6, 147–157. [Google Scholar] [CrossRef]
- Nemat-Nasser, S.; Hori, M. Micromechanics: Overall Properties of Heterogeneous Materials, 2nd ed.; Elsevier Science Publishers: Amsterdam, The Netherlands, 1999. [Google Scholar]
- Tucker, I.C.; Liang, E. Stiffness predictions for unidirectional short-fibre composites: Review and evaluation. Compos. Sci. Technol. 1999, 59, 655–671. [Google Scholar] [CrossRef]
- Pettermann, H.E.; Huber, C.O.; Luxner, M.H.; Nogales, S.; Böhm, H.J. An incremental Mori–tanaka homogenization scheme for finite: Strain thermoelastoplasticity of mmcs. Materials 2010, 3, 434–451. [Google Scholar] [CrossRef]
- Sadowski, P.; Kowalczyk-Gajewska, K.; Stupkiewicz, S. Consistent treatment and automation of the incremental Mori–Tanaka scheme for elasto-plastic composites. Comput. Mech. 2017, 60, 1–19. [Google Scholar] [CrossRef]
- Schjødt-Thomsen, J.; Pyrz, R. The Mori–Tanaka stiffness tensor: Diagonal symmetry, complex fibre orientations and non-dilute volume fractions. Mech. Mater. 2001, 33, 531–544. [Google Scholar] [CrossRef]
- Advani, S.G.; Tucker, C.L., III. The use of tensors to describe and predict fibre orientation in short fibre composites. J. Rheol. 1987, 31, 751–784. [Google Scholar] [CrossRef]
- Ogierman, W.; Kokot, G. A study on fiber orientation influence on the mechanical response of a short fiber composite structure. Acta Mech. 2016, 227, 173–183. [Google Scholar] [CrossRef]
- Brassart, L.; Doghri, I.; Delannay, L. Homogenization of elasto-plastic composites coupled with a nonlinear finite element analysis of the equivalent inclusion problem. Int. J. Solids Struct. 2010, 47, 716–729. [Google Scholar] [CrossRef]
- Srinivasulu, G.; Velmurugan, R.; Jayasankar, S. A hybrid method for computing the effective properties of composites containing arbitrarily shaped inclusions. Comput. Struct. 2015, 150, 63–70. [Google Scholar] [CrossRef]
- Klusemann, B.; Böhm, H.J.; Svendsen, B. Homogenization methods for multi-phase elastic composites with non-elliptical reinforcements: Comparisons and benchmarks. Eur. J. Mech. A/Solids 2012, 34, 21–37. [Google Scholar] [CrossRef]
- Ogierman, W. Hybrid Mori-Tanaka/Finite Element Method: An Efficient Homogenization of Composite Materials with Various Reinforcement Shape and Orientation. In Proceedings of the 13th World Congress on Computational Mechanics (WCCM XIII) and 2nd Pan American Congress on Computational Mechanics, New York, NY, USA, 22–27 July 2018. [Google Scholar]
- Chiang, C.R. Computation of the bounds on the elastic moduli of a fiber-reinforced composite by Monte Carlo simulations. Acta Mech. 2011, 217, 257–267. [Google Scholar] [CrossRef]
- Kamiński, M.; Lauke, B. Uncertainty in effective elastic properties of particle filled polymers by the Monte Carlo simulation. Compos. Struct. 2015, 123, 374–382. [Google Scholar] [CrossRef]
- Fedeliński, P.; Górski, G.; Czyż, T.; Dziatkiewicz, G.; Ptaszny, J. Analysis of effective properties of materials by using the boundary element method. Arch. Mech. 2014, 66, 19–35. [Google Scholar]
- Ptaszny, J.; Hatłas, M. Evaluation of the FMBEM efficiency in the analysis of porous structures. Eng. Comput. 2018, 35, 843–866. [Google Scholar] [CrossRef]





| Phase | Young Modulus (MPa) | Poisson Ratio |
|---|---|---|
| Matrix | 70,000 | 0.33 |
| Fiber | 300,000 | 0.20 |
| Volume Fraction of Fibers | Young Modulus (MPa) | Poisson Ratio |
|---|---|---|
| 0.05 | Ec5% = 74,955.83 | νc5% = 0.32446 |
| 0.10 | Ec10% = 80,486.48 | νc10% = 0.31897 |
| 0.15 | Ec15% = 85,820.04 | νc15% = 0.31383 |
| Input Data | Analysis Number | Identified Elastic Constants | |||
|---|---|---|---|---|---|
| Em | Er | vm | vr | ||
| Ec5%, νc5% | 1 | 68,383.3, 2.3% | 512,943.6, 71.0% | 0.33013, 0.0% | 0.16642, 16.8% |
| 2 | 69,287.1, 1.0% | 393,597.7, 31.2% | 0.32500, 1.5% | 0.38210, 91.1% | |
| 3 | 72,330.0, 3.3% | 152,002.5, 49.3% | 0.32536, 1.4% | 0.31336, 56.7% | |
| Ec10%, νc10% | 1 | 65,642.5, 6.2% | 663,675.3,121.2% | 0.32863, 0.4% | 0.21423, 7.1% |
| 2 | 79,190.7, 13.1% | 92,946.0, 69.0% | 0.32298, 2.1% | 0.27911, 39.6% | |
| 3 | 71,523.3, 2.2% | 250,707.2, 16.4% | 0.32895, 0.3% | 0.19039, 4.8% | |
| Ec15%, νc15% | 1 | 77,654.0, 10.9% | 155,795.0, 48.1% | 0.31281, 5.2% | 0.32879, 64.4% |
| 2 | 62,787.5, 10.3% | 709,421.2, 136.5% | 0.32567, 1.3% | 0.25722, 28.6% | |
| 3 | 60,758.4, 13.2% | 929,659.3, 209.9% | 0.32381, 1.9% | 0.32073, 60.4% | |
| Ec5%, νc5%, Ec10%, νc10% | 1 | 69,708.4, 0.4% | 331,100.2, 10.4% | 0.33001, 0.0% | 0.16767, 16.2% |
| 2 | 69,708.4, 0.4% | 331,097.8, 10.4% | 0.33001, 0.0% | 0.16766, 16.2% | |
| 3 | 69,708.4, 0.4% | 331,098.0, 10.4% | 0.33001, 0.0% | 0.16766, 16.2% | |
| Ec10%, νc10%, Ec15%, νc15% | 1 | 70,608.3, 0.9% | 288,238.8, 3.9% | 0.32927, 0.2% | 0.18351, 8.2% |
| 2 | 70,608.3, 0.9% | 288,237.8, 3.9% | 0.32927, 0.2% | 0.18351, 8.2% | |
| 3 | 70,608.4, 0.9% | 288,237.4, 3.9% | 0.32927, 0.2% | 0.18353, 8.2% | |
| Ec5%, νc5%, Ec15%, νc15% | 1 | 69,934.1, 0.1% | 308,332.5, 2.8% | 0.32982, 0.1% | 0.17573, 12.1% |
| 2 | 69,934.1, 0.1% | 308,332.6, 2.8% | 0.32982, 0.1% | 0.17573, 12.1% | |
| 3 | 69,934.04, 0.1% | 308,333.81, 2.8% | 0.32982, 0.1% | 0.17573, 12.1% | |
| Ec5%, νc5%, Ec15%, νc15%, Ec15%, νc15% | 1 | 70,001.2, 0.0% | 308,842.0, 2.9% | 0.32976, 0.1% | 0.17571, 12.1% |
| 2 | 70,001.2, 0.0% | 308,841.9, 2.9% | 0.32976, 0.1% | 0.17571, 12.1% | |
| 3 | 70,001.2, 0.0% | 308,841.8, 2.9% | 0.32976, 0.1% | 0.17571, 12.1% | |
| Volume Fraction of Fibers | Young Modulus (Mpa) | Poisson Ratio |
|---|---|---|
| 0.05 | Μ = 74,955.83 | μ = 0.32446 |
| s1 = 500.00 | s1 = 0.0015 | |
| s2 = 1000.00 | s2 = 0.0030 | |
| 0.10 | μ = 80,486.48 | μ = 0.31897 |
| s1 = 500.00 | s1 = 0.0015 | |
| s2 = 1000.00 | s2 = 0.0030 | |
| 0.15 | μ = 85,820.04 | μ = 0.31383 |
| s1 = 500.00 | s1 = 0.0015 | |
| s2 = 1000.00 | s2 = 0.0030 |
| Phase | Young Modulus (MPa) | Poisson Ratio |
|---|---|---|
| Matrix | 70,000 | 0.30 |
| Particle | 415,000 | 0.16 |
| Volume Fraction of Fibers | Young Modulus(MPa) | Poisson Ratio |
|---|---|---|
| 0.10 | 82,012.65 | 0.28828 |
| 0.15 | 88,707.98 | 0.28269 |
| Micromechanical Model | Identified Elastic Constants | |||
|---|---|---|---|---|
| Em | Ei | vm | vi | |
| Mori–Tanaka (M-T) | 70,180.5, 0.3% | 518,546.8, 22.2% | 0.29849, 0.5% | 0.12000, 18.2% |
| Hybrid M-T/FE | 70,042.9, 0.1% | 432,482.4, 4.1% | 0.29924, 0.3% | 0.15423, 3.7% |
© 2018 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ogierman, W. Inverse Identification of Elastic Properties of Constituents of Discontinuously Reinforced Composites. Materials 2018, 11, 2332. https://doi.org/10.3390/ma11112332
Ogierman W. Inverse Identification of Elastic Properties of Constituents of Discontinuously Reinforced Composites. Materials. 2018; 11(11):2332. https://doi.org/10.3390/ma11112332
Chicago/Turabian StyleOgierman, Witold. 2018. "Inverse Identification of Elastic Properties of Constituents of Discontinuously Reinforced Composites" Materials 11, no. 11: 2332. https://doi.org/10.3390/ma11112332
APA StyleOgierman, W. (2018). Inverse Identification of Elastic Properties of Constituents of Discontinuously Reinforced Composites. Materials, 11(11), 2332. https://doi.org/10.3390/ma11112332





