Atomic Simulations of Grain Structures and Deformation Behaviors in Nanocrystalline CoCrFeNiMn High-Entropy Alloy
Abstract
:1. Introduction
2. Materials and Methods
3. Results
3.1. Melting Properties
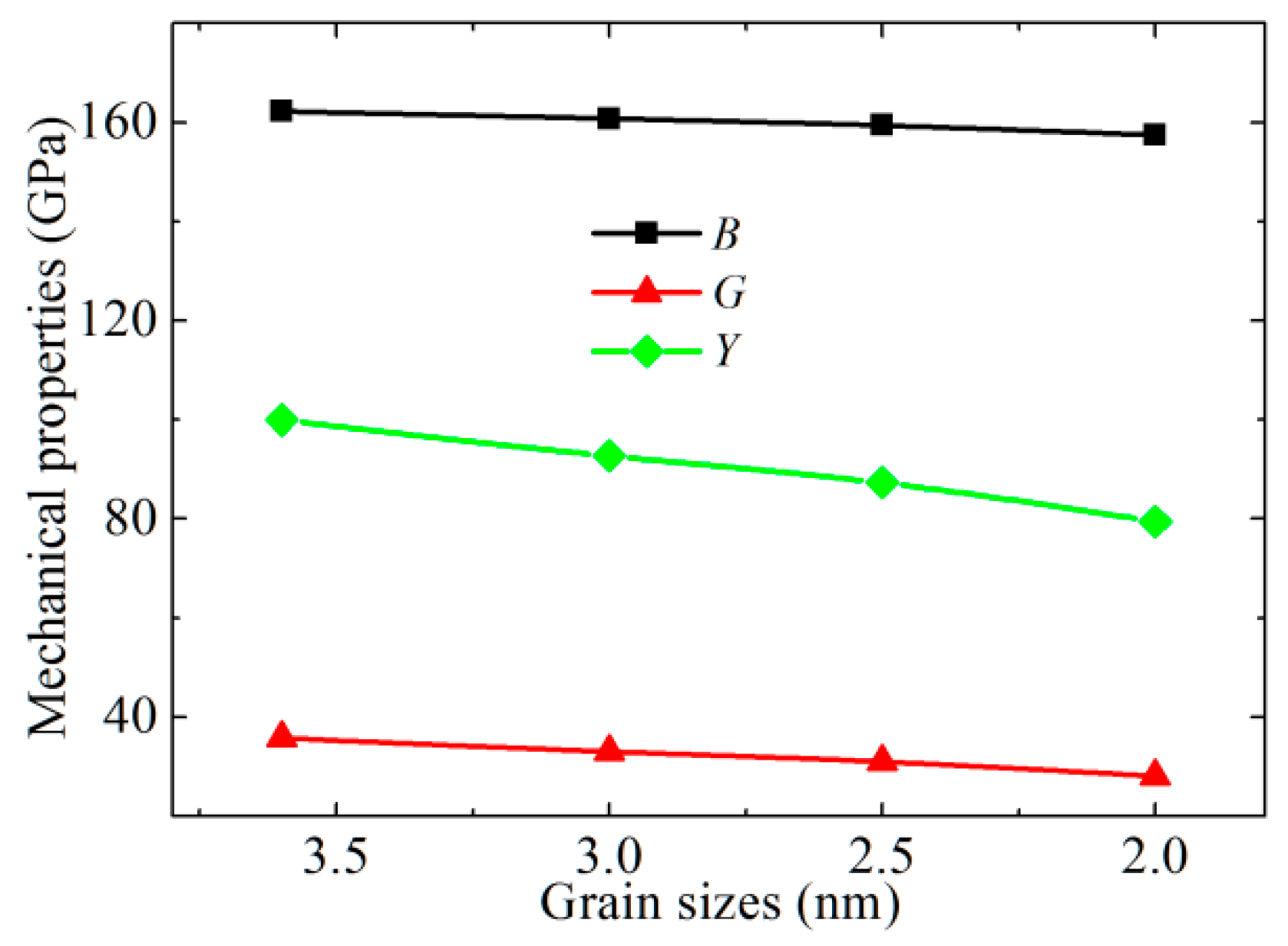
3.2. Elastic Properties
3.3. Stress–Strain Relation
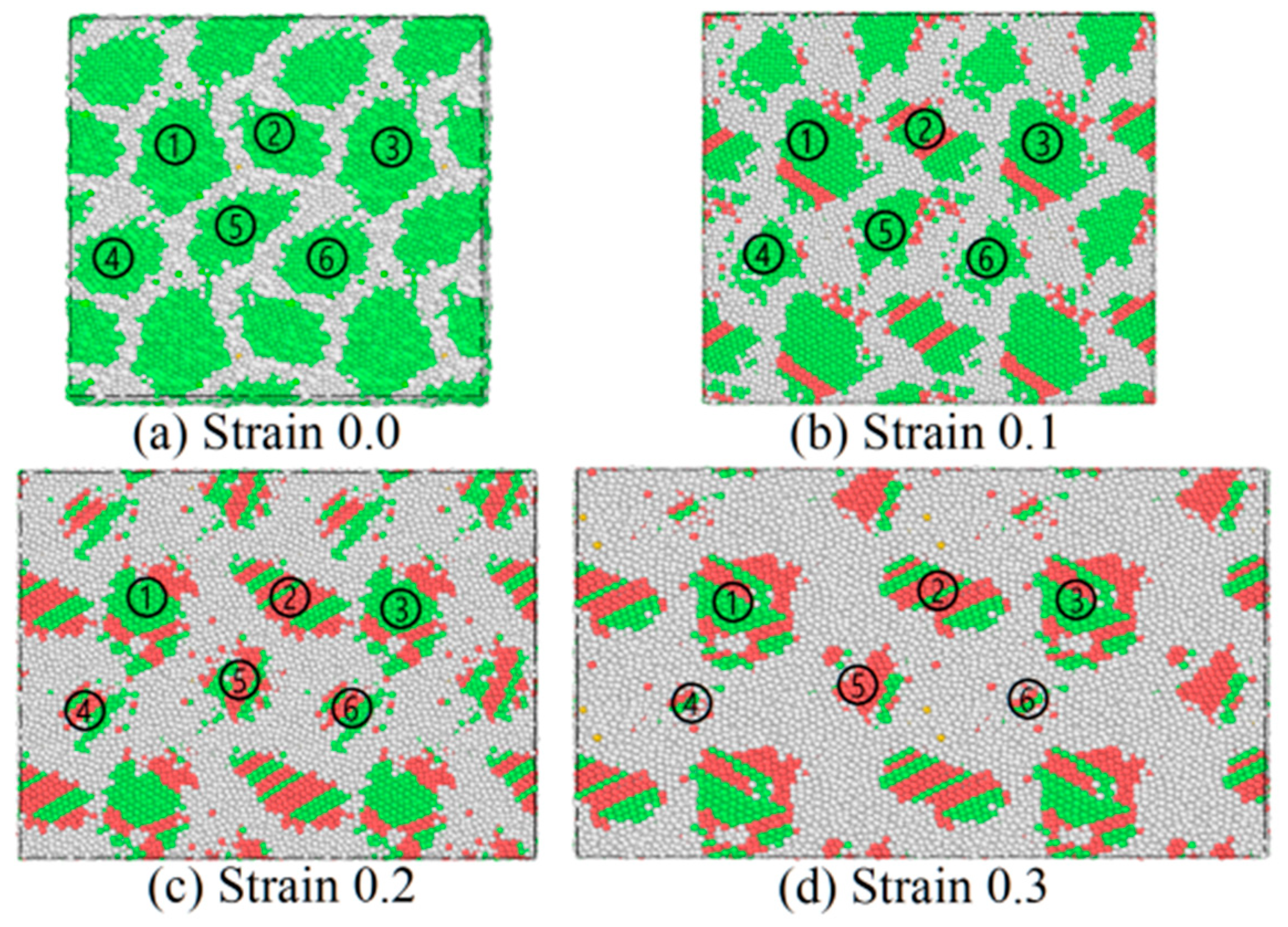
3.4. Structure Character under Uniaxial Strain
3.5. Deformation Mechanism under Uniaxial Tension
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Zhang, W.; Liaw, P.K.; Zhang, Y. Science and technology in high-entropy alloys. Sci. China Mater. 2018, 61, 2–22. [Google Scholar] [CrossRef] [Green Version]
- Miracle, D.B.; Senkov, O.N. A critical review of high entropy alloys and related concepts. Acta Mater. 2017, 122, 448–511. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Zuo, T.T.; Tang, Z.; Gao, M.C.; Dahmen, K.A.; Liaw, P.K.; Lu, Z.P. Microstructures and properties of high-entropy alloys. Prog. Mater. Sci. 2014, 61, 1–93. [Google Scholar] [CrossRef]
- Salishchev, G.A.; Tikhonovsky, M.A.; Shaysultanov, D.G.; Stepanov, N.D.; Kuznetsov, A.V.; Kolodiy, I.V.; Tortika, A.S.; Senkov, O.N. Effect of Mn and V on structure and mechanical properties of high-entropy alloys based on CoCrFeNi system. J. Alloy. Compd. 2014, 591, 11–21. [Google Scholar] [CrossRef] [Green Version]
- Shahmir, H.; Mousavi, T.; He, J.Y.; Lu, Z.P.; Kawasaki, M.; Langdon, T.G. Microstructure and properties of a CoCrFeNiMn high-entropy alloy processed by equal-channel angular pressing. Mater. Sci. Eng. A 2017, 705, 411–419. [Google Scholar] [CrossRef]
- Stepanov, N.D.; Shaysultanov, D.G.; Chernichenko, R.S.; Yurchenko, N.Y.; Zherebtsov, S.V.; Tikhonovsky, M.A.; Salishchev, G.A. Effect of thermomechanical processing on microstructure and mechanical properties of the carbon-containing CoCrFeNiMn high entropy alloy. J. Alloy. Compd. 2017, 693, 394–405. [Google Scholar]
- Heczel, A.; Kawasaki, M.; Labar, J.L.; Jang, J.I.; Langdon, T.G.; Gubicza, J. Defect structure and hardness in nanocrystalline CoCrFeMnNi High-Entropy Alloy processed by High-Pressure Torsion. J. Alloy. Compd. 2017, 711, 143–154. [Google Scholar] [CrossRef] [Green Version]
- Yu, P.F.; Zhang, L.J.; Cheng, H.; Zhang, H.; Ma, M.Z.; Li, Y.C.; Li, G.; Liaw, P.K.; Liu, R.P. The high-entropy alloys with high hardness and soft magnetic property prepared by mechanical alloying and high-pressure sintering. Intermetallics 2016, 70, 82–87. [Google Scholar] [Green Version]
- Dang, C.Q.; Surjadi, J.U.; Gao, L.B.; Lu, Y. Mechanical Properties of Nanostructured CoCrFeNiMn High-Entropy Alloy (HEA) Coating. Front. Mater. 2018, 5, 41. [Google Scholar] [CrossRef]
- Tian, F.Y.; Varga, L.K.; Shen, J.; Vitos, L. Calculating elastic constants in high-entropy alloys using the coherent potential approximation: Current issues and errors. Comp. Mater. Sci. 2016, 111, 350–358. [Google Scholar] [CrossRef]
- Korchuganov, A.V. Onset of plastic deformation in non-equiatomic fcc CoCrFeMnNi high-entropy alloys under high-rate loading. Lett. Mater. 2018, 8, 311–316. [Google Scholar] [CrossRef]
- Plimpton, S. Fast Parallel Algorithms for Short-Range Molecular Dynamics. J. Comput. Phys. 1995, 117, 1–19. [Google Scholar] [CrossRef] [Green Version]
- Choi, W.-M.; Jo, Y.H.; Sohn, S.S.; Lee, S.; Lee, B.-J. Understanding the physical metallurgy of the CoCrFeMnNi high-entropy alloy: an atomistic simulation study. npj Comput. Mater. 2018, 4, 1. [Google Scholar] [CrossRef]
- Hirel, P. Atomsk: A tool for manipulating and converting atomic data files. Comput. Phys. Commun. 2015, 197, 212–219. [Google Scholar] [CrossRef]
- Stukowski, A. Visualization and analysis of atomistic simulation data with OVITO–the Open Visualization Tool. Model. Simul. Mater. Sci. Eng. 2009, 18, 015012. [Google Scholar] [CrossRef] [Green Version]
- Bhattacharjee, P.P.; Sathiaraj, G.D.; Zaid, M.; Gatti, J.R.; Lee, C.; Tsai, C.-W.; Yeh, J.-W. Microstructure and texture evolution during annealing of equiatomic CoCrFeMnNi high-entropy alloy. J. Alloy. Compd. 2014, 587, 544–552. [Google Scholar] [CrossRef]
- Stepanov, N.D.; Shaysultanov, D.G.; Salishchev, G.A.; Tikhonovsky, M.A.; Oleynik, E.E.; Tortika, A.S.; Senkov, O.N. Effect of V content on microstructure and mechanical properties of the CoCrFeMnNiVx high entropy alloys. J. Alloy. Compd. 2015, 628, 170–185. [Google Scholar] [CrossRef]
- Yan, H.-J.; Zhuang, J.-C.; Zhou, P.; Li, Q.; Zhou, C.Q.; Fu, P. Molecular dynamics simulation of thermal physical properties of molten iron. Int. J. Heat Mass. Tran. 2017, 109, 755–760. [Google Scholar] [CrossRef]
- Hill, R. Elastic properties of reinforced solids: Some theoretical principles. J. Mech. Phys. Solids. 1963, 11, 357–372. [Google Scholar] [CrossRef]
- Alagarsamy, K.; Fortier, A.; Mishra, R.; Kumar, N. Investigation of Thermo-Mechanical Processing and Mechanical Properties of CoCrFeNiMn High Entropy Alloy for Peripheral Vascular Stent Application. In Proceedings of the ASME 2016 11th International Manufacturing Science and Engineering Conference, Blacksburg, VA, USA, 27 June 27–1 July 2016; p. V001T02A059. [Google Scholar]
- Laplanche, G.; Gadaud, P.; Horst, O.; Otto, F.; Eggeler, G.; George, E.P. Temperature dependencies of the elastic moduli and thermal expansion coefficient of an equiatomic, single-phase CoCrFeMnNi high-entropy alloy. J. Alloy. Compd. 2015, 623, 348–353. [Google Scholar] [CrossRef] [Green Version]



| Atom Type | Co | Cr | Fe | Ni | Mn | CoCrFeNiMn |
|---|---|---|---|---|---|---|
| Diffusion coefficient (m2·s−1) | 15.3 × 10−9 | 14.7 × 10−9 | 14.5 × 10−9 | 14.3 × 10−9 | 16.5 × 10−9 | 15.0 × 10−9 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hou, J.; Li, Q.; Wu, C.; Zheng, L. Atomic Simulations of Grain Structures and Deformation Behaviors in Nanocrystalline CoCrFeNiMn High-Entropy Alloy. Materials 2019, 12, 1010. https://doi.org/10.3390/ma12071010
Hou J, Li Q, Wu C, Zheng L. Atomic Simulations of Grain Structures and Deformation Behaviors in Nanocrystalline CoCrFeNiMn High-Entropy Alloy. Materials. 2019; 12(7):1010. https://doi.org/10.3390/ma12071010
Chicago/Turabian StyleHou, Junling, Qiang Li, Chuanbao Wu, and Limei Zheng. 2019. "Atomic Simulations of Grain Structures and Deformation Behaviors in Nanocrystalline CoCrFeNiMn High-Entropy Alloy" Materials 12, no. 7: 1010. https://doi.org/10.3390/ma12071010
APA StyleHou, J., Li, Q., Wu, C., & Zheng, L. (2019). Atomic Simulations of Grain Structures and Deformation Behaviors in Nanocrystalline CoCrFeNiMn High-Entropy Alloy. Materials, 12(7), 1010. https://doi.org/10.3390/ma12071010





