Characterisation of Natural Fibres for Sustainable Discontinuous Fibre Composite Materials
Abstract
:1. Introduction
2. Materials
3. Experimental Work
3.1. Physical Characterisation
3.1.1. Visual Characterisation
3.1.2. Density Characterisation
3.1.3. Surface Analysis
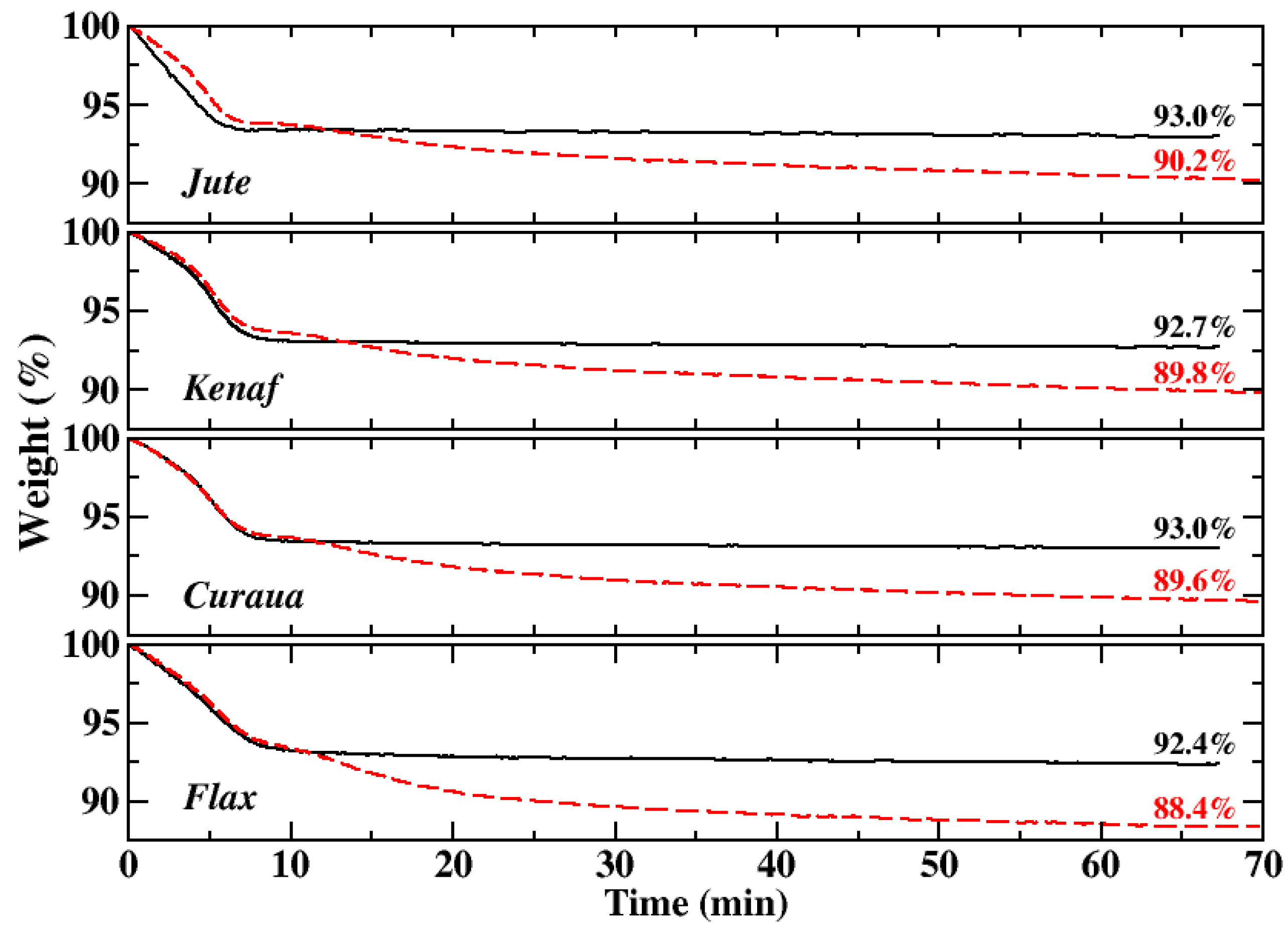
3.2. Thermal Analysis
3.3. Mechanical Characterisation
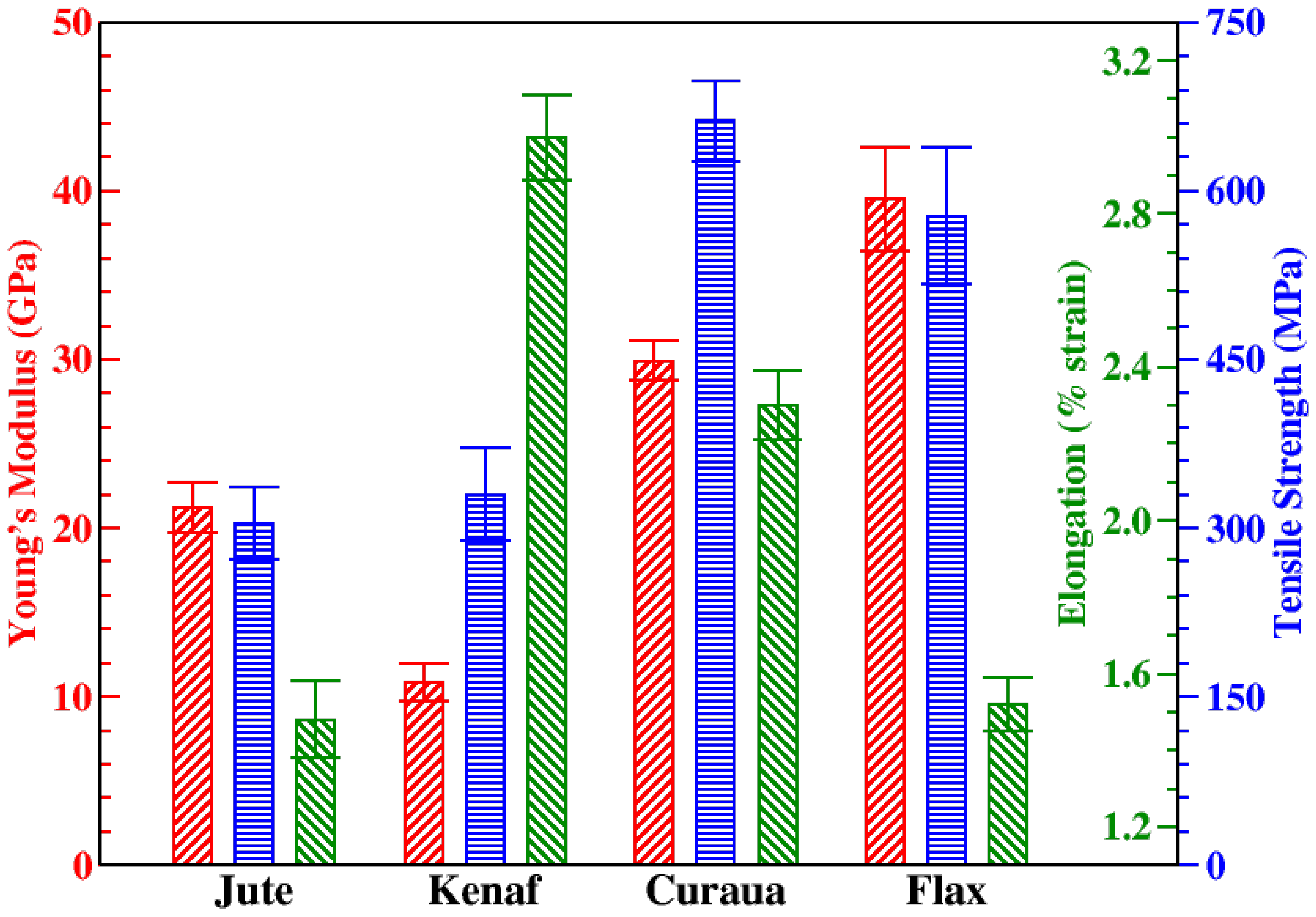
3.3.1. Single Fibre Tensile Test
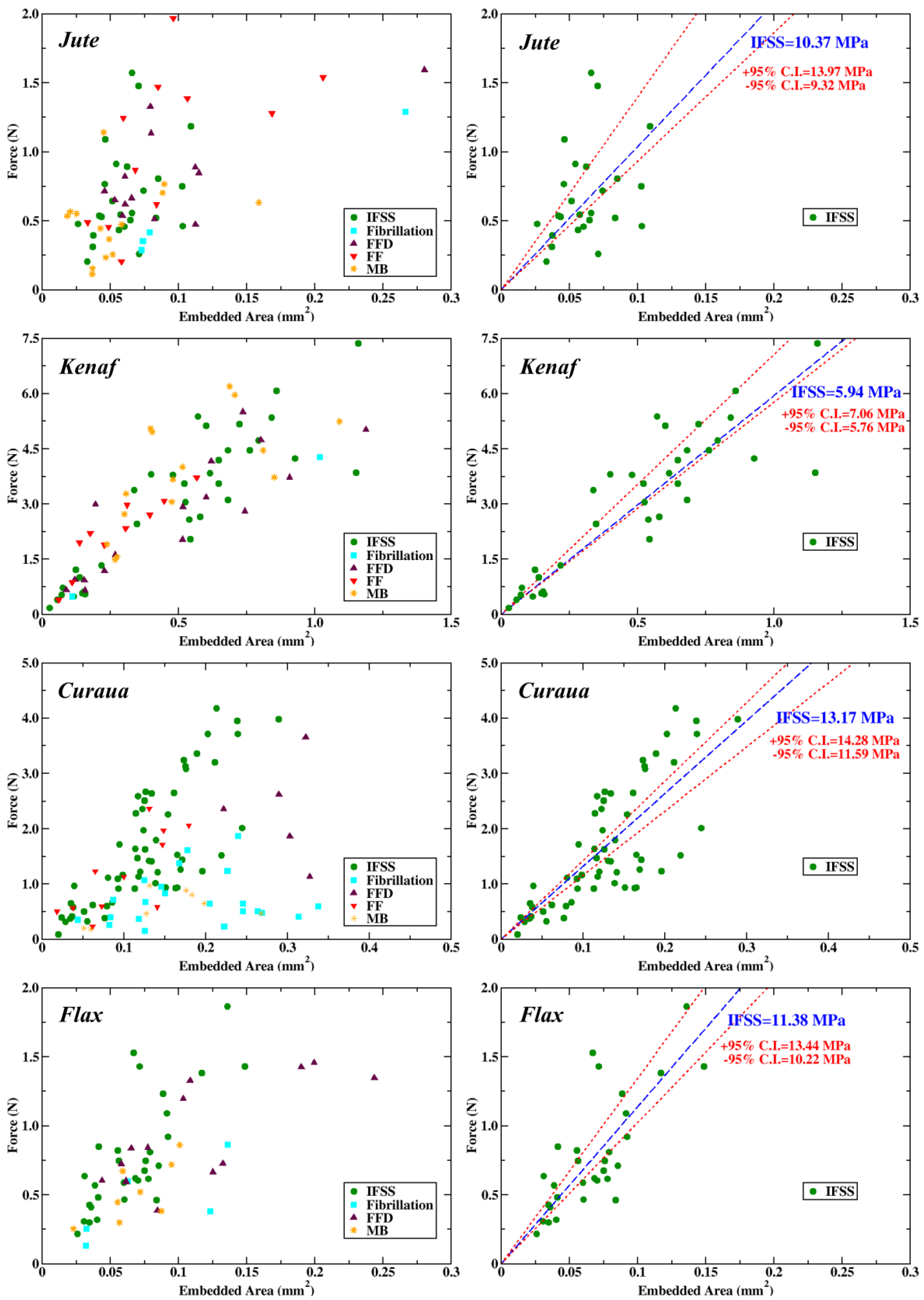
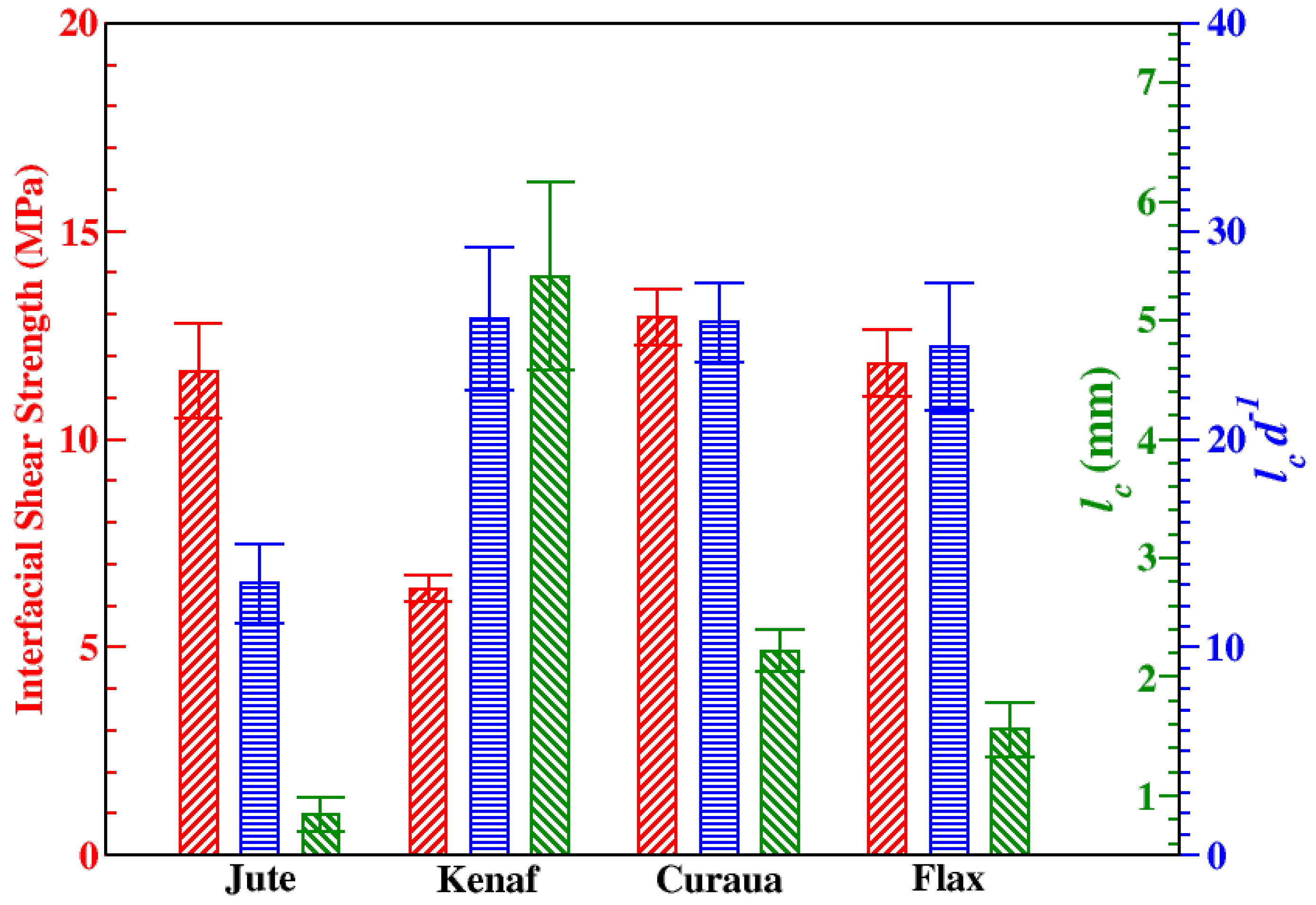
3.3.2. Microbond Test
4. Discussion
Natural Fibres as Alternatives to Glass Fibres in ADFRCs
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- U.K. Composites. End-of-Life Solutions for FRP Composites; Technical Sheet; U.K. Composites: Berkhamsted, UK, 2015. [Google Scholar]
- Tapper, R.J.; Longana, M.L.; Norton, A.; Potter, K.D.; Hamerton, I. An evaluation of life cycle assessment and its application to the closed-loop recycling of carbon fibre reinforced polymers. Compos. Part B Eng. 2020, 184, 107665. [Google Scholar] [CrossRef]
- Pickering, K.; Efendy, M.A.; Le, T. A review of recent developments in natural fibre composites and their mechanical performance. Compos. Part A Appl. Sci. Manuf. 2016, 83, 98–112. [Google Scholar] [CrossRef] [Green Version]
- Summerscales, J.; Dissanayake, N.P.; Virk, A.S.; Hall, W. A review of bast fibres and their composites. Part 1—Fibres as reinforcements. Compos. Part A Appl. Sci. Manuf. 2010, 41, 1329–1335. [Google Scholar] [CrossRef] [Green Version]
- Saheb, D.N.; Jog, J.P. Natural fiber polymer composites: A review. Adv. Polym. Technol. 1999, 18, 351–363. [Google Scholar] [CrossRef]
- Fangueiro, R.; Rana, S. Natural Fibres: Advances in Science and Technology Towards Industrial Applications From Science to Market; Springer: Dordrecht, The Netherlands, 2016. [Google Scholar] [CrossRef]
- Wang, W.; Sain, M.; Cooper, P. Study of moisture absorption in natural fiber plastic composites. Compos. Sci. Technol. 2006, 66, 379–386. [Google Scholar] [CrossRef]
- Fukuda, H.; Chou, T.W. A probabilistic theory of the strength of short-fibre composites with variable fibre length and orientation. J. Mater. Sci. 1982, 17, 1003–1011. [Google Scholar] [CrossRef]
- Fu, S.Y.; Lauke, B. Effects of fiber length and fiber orientation distributions on the tensile strength of short-fiber-reinforced polymers. Compos. Sci. Technol. 1996, 56, 1179–1190. [Google Scholar] [CrossRef]
- Matthew Such, C.W.; Potter, K. Aligned Discontinuous fibre composites: A short history. J. Multifunct. Compos. 2014, 2, 155–168. [Google Scholar] [CrossRef]
- Yu, H.; Potter, K.; Wisnom, M. A novel manufacturing method for aligned discontinuous fibre composites (High Performance-Discontinuous Fibre method). Compos. Part A Appl. Sci. Manuf. 2014, 65, 175–185. [Google Scholar] [CrossRef]
- Longana, M.L.; Ondra, V.; Yu, H.; Potter, K.D.; Hamerton, I. Reclaimed Carbon and Flax Fibre Composites: Manufacturing and Mechanical Properties. Recycling 2018, 3, 52. [Google Scholar] [CrossRef] [Green Version]
- Drzal, L.; Madhukar, M. Fibre-matrix adhesion and its relationship to composite mechanical properties. J. Mater. Sci. 1993, 28, 569–610. [Google Scholar] [CrossRef]
- Spārniņš, E.; Nyström, B.; Andersons, J. Interfacial shear strength of flax fibers in thermoset resins evaluated via tensile tests of UD composites. Int. J. Adhes. Adhes. 2012, 36, 39–43. [Google Scholar] [CrossRef]
- Wong, S.; Shanks, R.; Hodzic, A. Effect of additives on the interfacial strength of poly(l-lactic acid) and poly(3-hydroxy butyric acid)-flax fibre composites. Compos. Sci. Technol. 2007, 67, 2478–2484. [Google Scholar] [CrossRef]
- Arbelaiz, A.; Cantero, G.; Fernández, B.; Mondragon, I.; Gañán, P.; Kenny, J. Flax fiber surface modifications: Effects on fiber physico mechanical and flax/polypropylene interface properties. Polym. Compos. 2005, 26, 324–332. [Google Scholar] [CrossRef]
- Zafeiropoulos, N.; Baillie, C.; Hodgkinson, J. Engineering and characterisation of the interface in flax fibre/polypropylene composite materials. Part II. The effect of surface treatments on the interface. Compos. Part A Appl. Sci. Manuf. 2002, 33, 1185–1190. [Google Scholar] [CrossRef]
- Seghini, M.; Touchard, F.; Sarasini, F.; Chocinski-Arnault, L.; Mellier, D.; Tirillò, J. Interfacial adhesion assessment in flax/epoxy and in flax/vinylester composites by single yarn fragmentation test: Correlation with micro-CT analysis. Compos. Part A Appl. Sci. Manuf. 2018, 113, 66–75. [Google Scholar] [CrossRef]
- Joffe, R.; Andersons, J.; Wallström, L. Interfacial shear strength of flax fiber/thermoset polymers estimated by fiber fragmentation tests. J. Mater. Sci. 2005, 40, 2721–2722. [Google Scholar] [CrossRef]
- Huber, T.; Müssig, J. Fibre matrix adhesion of natural fibres cotton, flax and hemp in polymeric matrices analyzed with the single fibre fragmentation test. Compos. Interfaces 2008, 15, 335–349. [Google Scholar] [CrossRef]
- Gaur, U.; Miller, B. Microbond method for determination of the shear strength of a fiber/resin interface: Evaluation of experimental parameters. Compos. Sci. Technol. 1989, 34, 35–51. [Google Scholar] [CrossRef]
- Khalil, H.; Ismail, H.; Rozman, H.; Ahmad, M. The effect of acetylation on interfacial shear strength between plant fibres and various matrices. Eur. Polym. J. 2001, 37, 1037–1045. [Google Scholar] [CrossRef]
- Baley, C.; Busnel, F.; Grohens, Y.; Sire, O. Influence of chemical treatments on surface properties and adhesion of flax fibre–polyester resin. Compos. Part A Appl. Sci. Manuf. 2006, 37, 1626–1637. [Google Scholar] [CrossRef]
- Wang, F.; Lu, M.; Zhou, S.; Lu, Z.; Ran, S. Effect of Fiber Surface Modification on the Interfacial Adhesion and Thermo-Mechanical Performance of Unidirectional Epoxy-Based Composites Reinforced with Bamboo Fibers. Molecules 2019, 24, 2682. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Vincent, J.F.V. A Unified Nomenclature for Plant Fibres for Industrial Use. Appl. Compos. Mater. 2000, 7, 269–271. [Google Scholar] [CrossRef]
- Gurit, Prime™20lv Datasheet; Technical Sheet; Gurit UK: Newport, UK, 2019.
- ASTM International. Standard Test Methods for Apparent Porosity, Liquid Absorption, Apparent Specific Gravity, and Bulk Density of Refractory Shapes by Vacuum Pressure; ASTM International: West Conshohocken, PA, USA, 2016. [Google Scholar]
- Cook, J.G. Handbook of Textile Fibres: Volume 1: Natural Fibres; Woodhead Publishing: Cambridge, UK, 2001. [Google Scholar]
- Lewin, M. Handbook of Fiber Chemistry, 3rd ed.; CRC Press: Boca Raton, FL, USA, 2010. [Google Scholar]
- Sawsen, C.; Fouzia, K.; Mohamed, B.; Moussa, G. Optimizing the formulation of flax fiber-reinforced cement composites. Constr. Build. Mater. 2014, 54, 659–664. [Google Scholar] [CrossRef]
- Amiri, A.; Triplett, Z.; Moreira, A.; Brezinka, N.; Alcock, M.; Ulven, C.A. Standard density measurement method development for flax fiber. Ind. Crop. Prod. 2017, 96, 196–202. [Google Scholar] [CrossRef]
- Madsen, B.; Lilholt, H. Physical and mechanical properties of unidirectional plant fibre composites—An evaluation of the influence of porosity. Compos. Sci. Technol. 2003, 63, 1265–1272. [Google Scholar] [CrossRef]
- Bourmaud, A.; Beaugrand, J.; Shah, D.U.; Placet, V.; Baley, C. Towards the design of high-performance plant fibre composites. Prog. Mater. Sci. 2018, 97, 347–408. [Google Scholar] [CrossRef]
- Bismarck, A.; Aranberri-Askargorta, I.; Springer, J.; Lampke, T.; Wielage, B.; Stamboulis, A.; Shenderovich, I.; Limbach, H.H. Surface characterization of flax, hemp and cellulose fibers; Surface properties and the water uptake behavior. Polym. Compos. 2002, 23, 872–894. [Google Scholar] [CrossRef]
- Cordeiro, N.; Gouveia, C.; Moraes, A.; Amico, S. Natural fibers characterization by inverse gas chromatography. Carbohydr. Polym. 2011, 84, 110–117. [Google Scholar] [CrossRef]
- Wesson, S.P.; Vajo, J.J.; Ross, S. Determination of specific surface areas of glass filaments by BET and CAEDMON methods. J. Colloid Interface Sci. 1983, 94, 552–563. [Google Scholar] [CrossRef]
- Spinacé, M.A.; Lambert, C.S.; Fermoselli, K.K.; Paoli, M.A.D. Characterization of lignocellulosic curaua fibres. Carbohydr. Polym. 2009, 77, 47–53. [Google Scholar] [CrossRef]
- Ramiah, M. Thermogravimetric and differential thermal analysis of cellulose, hemicellulose, and lignin. J. Appl. Polym. Sci. 1970, 14, 1323–1337. [Google Scholar] [CrossRef]
- ASTM International. Standard Test Method for Tensile Strength and Young’s Modulus of Fibers; ASTM International: West Conshohocken, PA, USA, 2014. [Google Scholar]
- Komuraiah, A.; Kumar, N.S.; Prasad, B.D. Chemical Composition of Natural Fibers and its Influence on their Mechanical Properties. Mech. Compos. Mater. 2014, 50, 359–376. [Google Scholar] [CrossRef]
- Mortazavi, S.M.; Kamali Moghaddam, M. An analysis of structure and properties of a natural cellulosic fiber (Leafiran). Fibers Polym. 2010, 11, 877–882. [Google Scholar] [CrossRef]
- Ouajai, S.; Shanks, R. Composition, structure and thermal degradation of hemp cellulose after chemical treatments. Polym. Degrad. Stab. 2005, 89, 327–335. [Google Scholar] [CrossRef]
- Swolfs, Y.; Verpoest, I.; Gorbatikh, L. A review of input data and modelling assumptions in longitudinal strength models for unidirectional fibre-reinforced composites. Compos. Struct. 2016, 150, 153–172. [Google Scholar] [CrossRef]
- Eichhorn, S.J.; Baillie, C.A.; Zafeiropoulos, N.; Mwaikambo, L.Y.; Ansell, M.P.; Dufresne, A.; Entwistle, K.M.; Herrera-Franco, P.J.; Escamilla, G.C.; Groom, L.; et al. Review: Current international research into cellulosic fibres and composites. J. Mater. Sci. 2001, 36, 2107–2131. [Google Scholar] [CrossRef]
- Sarker, F.; Karim, N.; Afroj, S.; Koncherry, V.; Novoselov, K.S.; Potluri, P. High-Performance Graphene-Based Natural Fiber Composites. ACS Appl. Mater. Interfaces 2018, 10, 34502–34512. [Google Scholar] [CrossRef]
- Doan, T.T.L.; Brodowsky, H.; Mäder, E. Jute fibre/epoxy composites: Surface properties and interfacial adhesion. Compos. Sci. Technol. 2012, 72, 1160–1166. [Google Scholar] [CrossRef]
- Pitkethly, M.J.; Favre, J.P.; Gaur, U.; Jakubowski, J.; Mudrich, S.F.; Caldwell, D.L.; Drzal, L.T.; Nardin, M.; Wagner, H.D.; Di Landro, L.; et al. A round-robin programme on interfacial test methods. Compos. Sci. Technol. 1993, 48, 205–214. [Google Scholar] [CrossRef]
- Bryce, D.; Yang, L.; Thomason, J. An investigation of fibre sizing on the interfacial strength of glass-fibre epoxy composites. In Proceedings of the ECCM18—18th European Conference on Composite Materials, Athens, Greece, 24–28 June 2018. [Google Scholar]
- Kang, S.K.; Lee, D.B.; Choi, N.S. Fiber/epoxy interfacial shear strength measured by the microdroplet test. Compos. Sci. Technol. 2009, 69, 245–251. [Google Scholar] [CrossRef]
- Charlet, K. CHAPTER 3 Natural Fibres as Composite Reinforcement Materials: Description and New Sources. Natl. Polym. 2012, 1, 37–62. [Google Scholar] [CrossRef]
- Toray Carbon Fibres, Torayca T300 and Toray T700s Technical Data Sheet; Technical Sheet; Toray Composite Materials America Inc.: Tacoma, WA, USA, 2019.
- Wambua, P.; Ivens, J.; Verpoest, I. Natural fibres: Can they replace glass in fibre reinforced plastics? Compos. Sci. Technol. 2003, 63, 1259–1264. [Google Scholar] [CrossRef]
- Correa, J.P.; Montalvo-Navarrete, J.M.; Hidalgo-Salazar, M.A. Carbon footprint considerations for biocomposite materials for sustainable products: A review. J. Clean. Prod. 2019, 208, 785–794. [Google Scholar] [CrossRef]
- Bensadoun, F.; Verpoest, I.; Baets, J.; Müssig, J.; Graupner, N.; Davies, P.; Gomina, M.; Kervoelen, A.; Baley, C. Impregnated fibre bundle test for natural fibres used in composites. J. Reinf. Plast. Compos. 2017, 36, 942–957. [Google Scholar] [CrossRef]
- Cox, H.L. The elasticity and strength of paper and other fibrous materials. Br. J. Appl. Phys. 1952, 3, 72–79. [Google Scholar] [CrossRef]
- Yu, H.; Longana, M.L.; Jalalvand, M.; Wisnom, M.R.; Potter, K.D. Hierarchical pseudo-ductile hybrid composites combining continuous and highly aligned discontinuous fibres. Compos. Part A Appl. Sci. Manuf. 2018, 105, 40–56. [Google Scholar] [CrossRef] [Green Version]



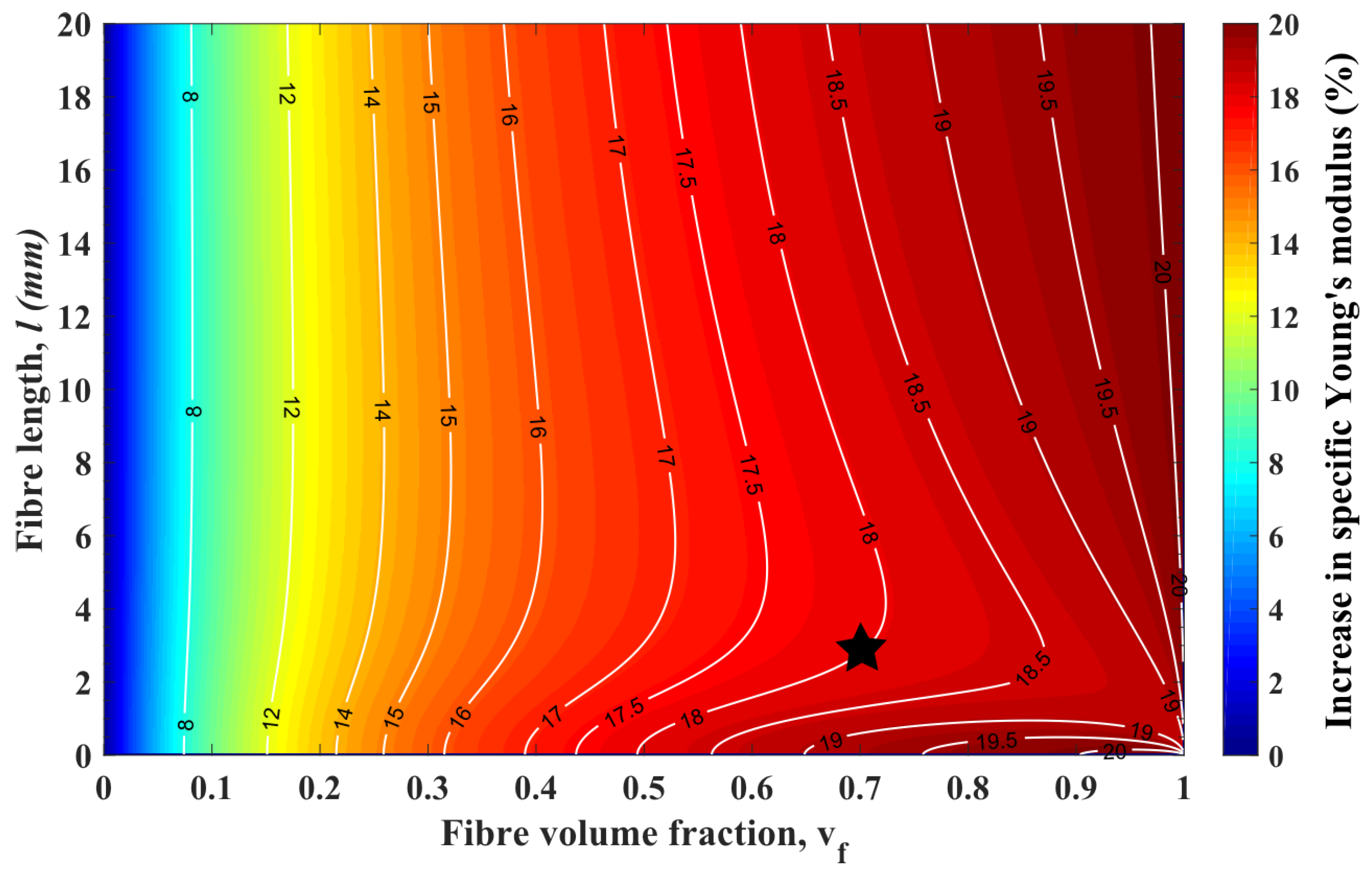
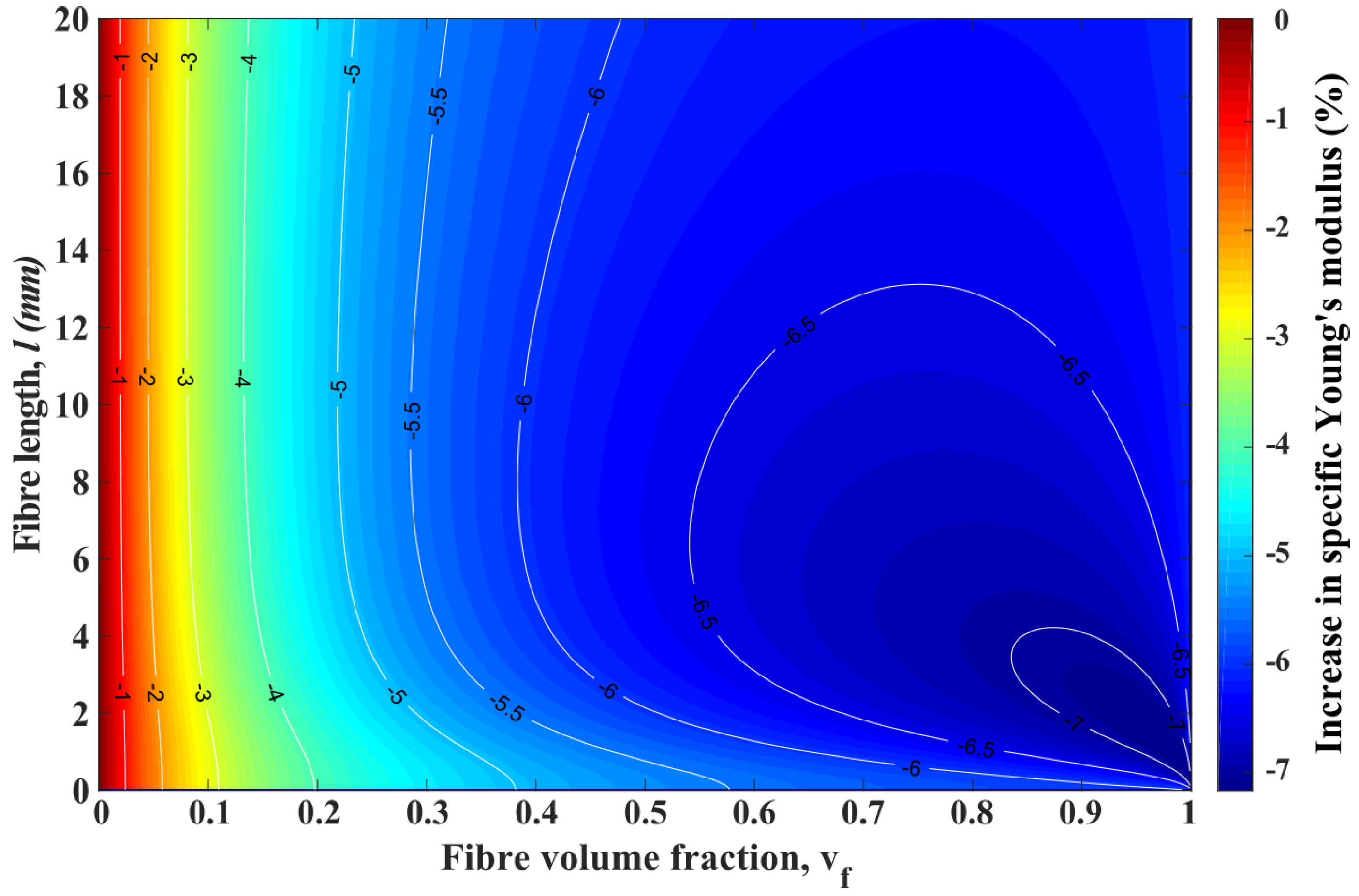
| Fibre | Apparent | Apparent | Water | Bulk |
|---|---|---|---|---|
| Density | Porosity | Absorption | Density | |
| (g cm−3) | (%) | (%) | (g cm−3) | |
| Jute | 1.51 ± 0.01 | 54.86 ± 2.20 | 81.08 ± 7.75 | 0.68 ± 0.04 |
| Kenaf | 1.57 ± 0.02 | 50.53 ± 1.20 | 65.21 ± 3.76 | 0.78 ± 0.03 |
| Curaua | 1.50 ± 0.01 | 54.58 ± 5.61 | 81.98 ± 17.57 | 0.68 ± 0.08 |
| Flax | 1.54 ± 0.01 | 51.72 ± 0.15 | 69.60 ± 0.19 | 0.74 ± 0.00 |
| Fibre | SBET | Sgeo | SR | SSA |
|---|---|---|---|---|
| (m2 g−1) | 10−2 (m2 g−1) | (μm−1) | ||
| Jute | 2.28 ± 1.07 | 4.14 ± 0.38 | 55.11 ± 26.35 | 3.44 ± 1.61 |
| Kenaf | 1.17 ± 0.12 | 1.22 ± 0.07 | 96.04 ± 11.36 | 1.84 ± 0.19 |
| Curaua | 1.32 ± 0.61 | 3.07 ± 0.09 | 43.02 ± 19.97 | 1.98 ± 0.92 |
| Flax | 0.37 ± 0.18 | 4.07 ± 0.32 | 9.05 ± 4.37 | 0.57 ± 0.27 |
| Specific Young’s Modulus | Specific Strength | Failure | |
|---|---|---|---|
| This Study | (GPa cm3 g−1) | (MPa cm3 g−1) | Strain (%) |
| Jute | 14.04 ± 1.00 | 201.36 ± 21.52 | 1.48 ± 0.10 |
| Kenaf | 6.93 ± 0.71 | 210.52 ± 26.45 | 3.00 ± 0.11 |
| Curaua | 19.96 ± 0.77 | 441.53 ± 24.07 | 2.30 ± 0.09 |
| Flax | 25.64 ± 2.01 | 375.36 ± 39.72 | 1.52 ± 0.07 |
| Glass [50] | 28–30 | 940–1350 | 2.5–3.4 |
| Jute [50] | 7–39 | 270–650 | 1.2–2.0 |
| Kenaf [50] | 12–42 | 538 | 3.0 |
| Curaua [50] | 8.4–36 | 360–1000 | 3.0–4.3 |
| Flax [50] | 26–76 | 240–1070 | 1.2–3.3 |
| Carbon [51] | 128–130 | 1900–2700 | 1.5–2.1 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kandemir, A.; Pozegic, T.R.; Hamerton, I.; Eichhorn, S.J.; Longana, M.L. Characterisation of Natural Fibres for Sustainable Discontinuous Fibre Composite Materials. Materials 2020, 13, 2129. https://doi.org/10.3390/ma13092129
Kandemir A, Pozegic TR, Hamerton I, Eichhorn SJ, Longana ML. Characterisation of Natural Fibres for Sustainable Discontinuous Fibre Composite Materials. Materials. 2020; 13(9):2129. https://doi.org/10.3390/ma13092129
Chicago/Turabian StyleKandemir, Ali, Thomas R. Pozegic, Ian Hamerton, Stephen J. Eichhorn, and Marco L. Longana. 2020. "Characterisation of Natural Fibres for Sustainable Discontinuous Fibre Composite Materials" Materials 13, no. 9: 2129. https://doi.org/10.3390/ma13092129
APA StyleKandemir, A., Pozegic, T. R., Hamerton, I., Eichhorn, S. J., & Longana, M. L. (2020). Characterisation of Natural Fibres for Sustainable Discontinuous Fibre Composite Materials. Materials, 13(9), 2129. https://doi.org/10.3390/ma13092129







