Simulations and Experiments on the Micro-Milling Process of a Thin-Walled Structure of Al6061-T6
Abstract
:1. Introduction
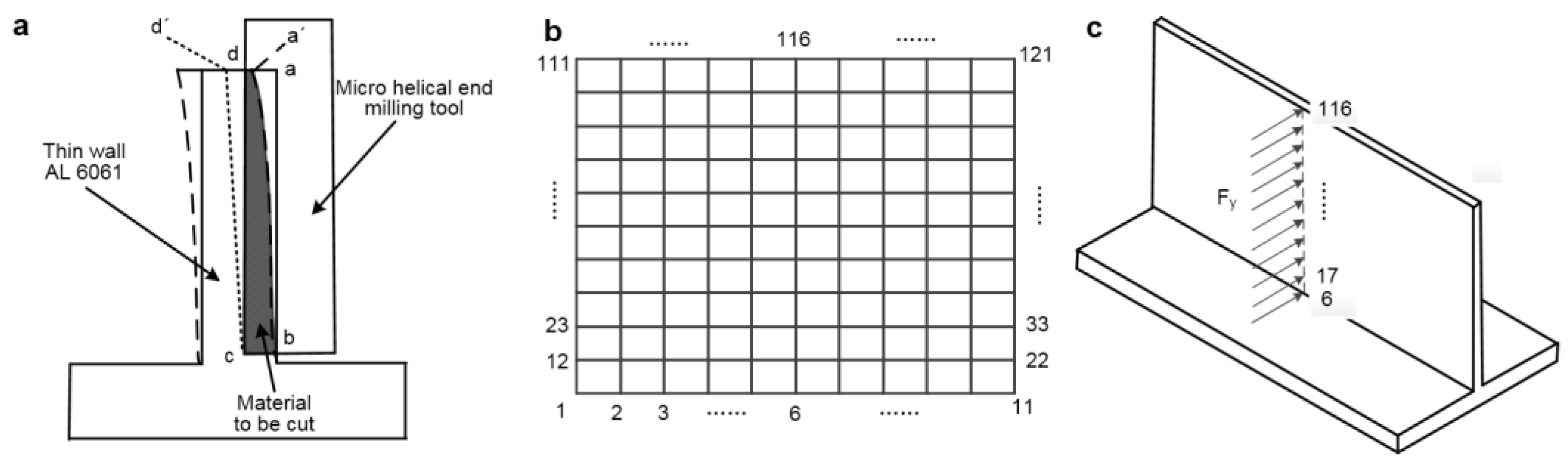
2. Modeling of a Micro Thin-Walled Structure by FEM
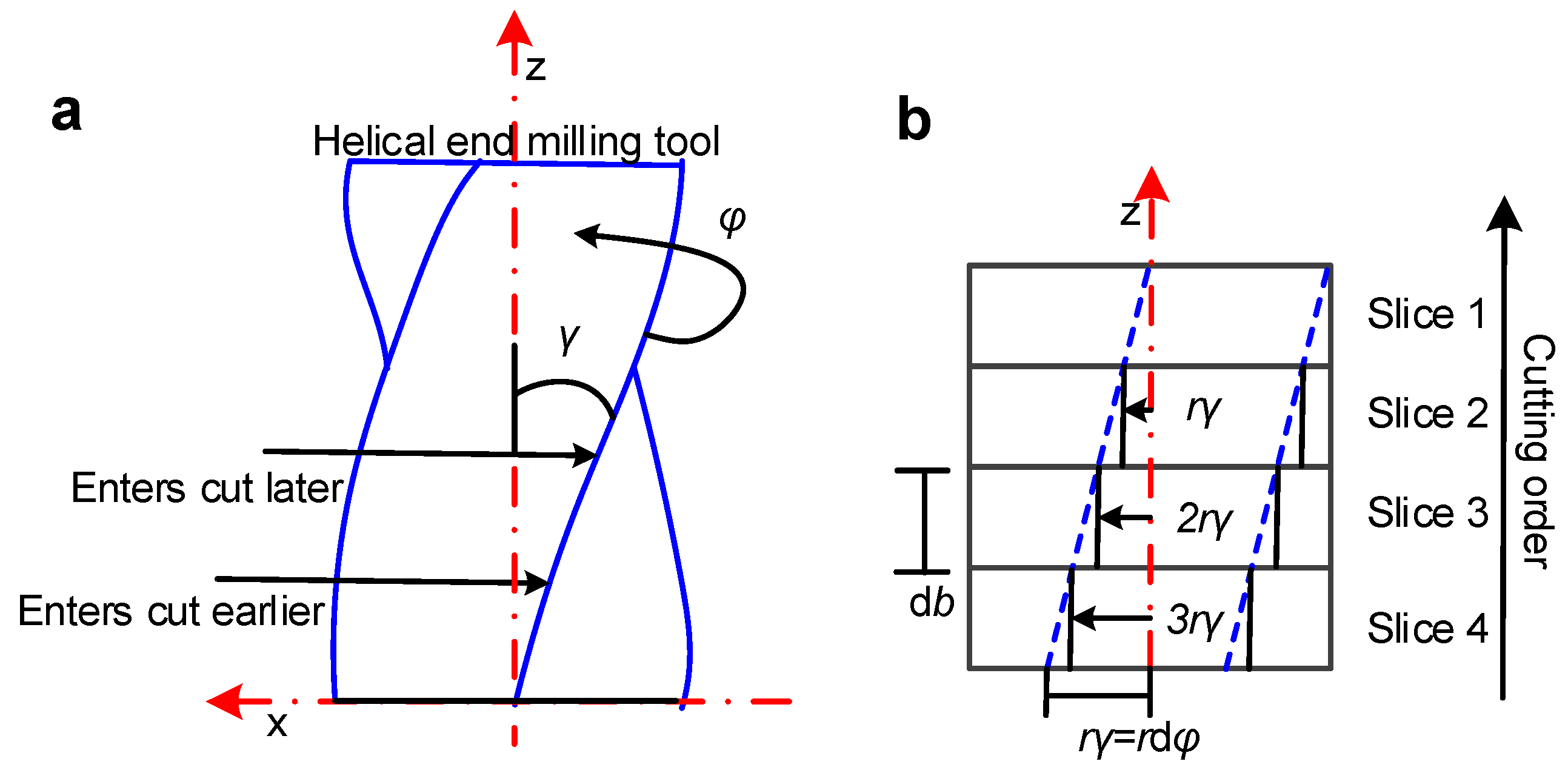
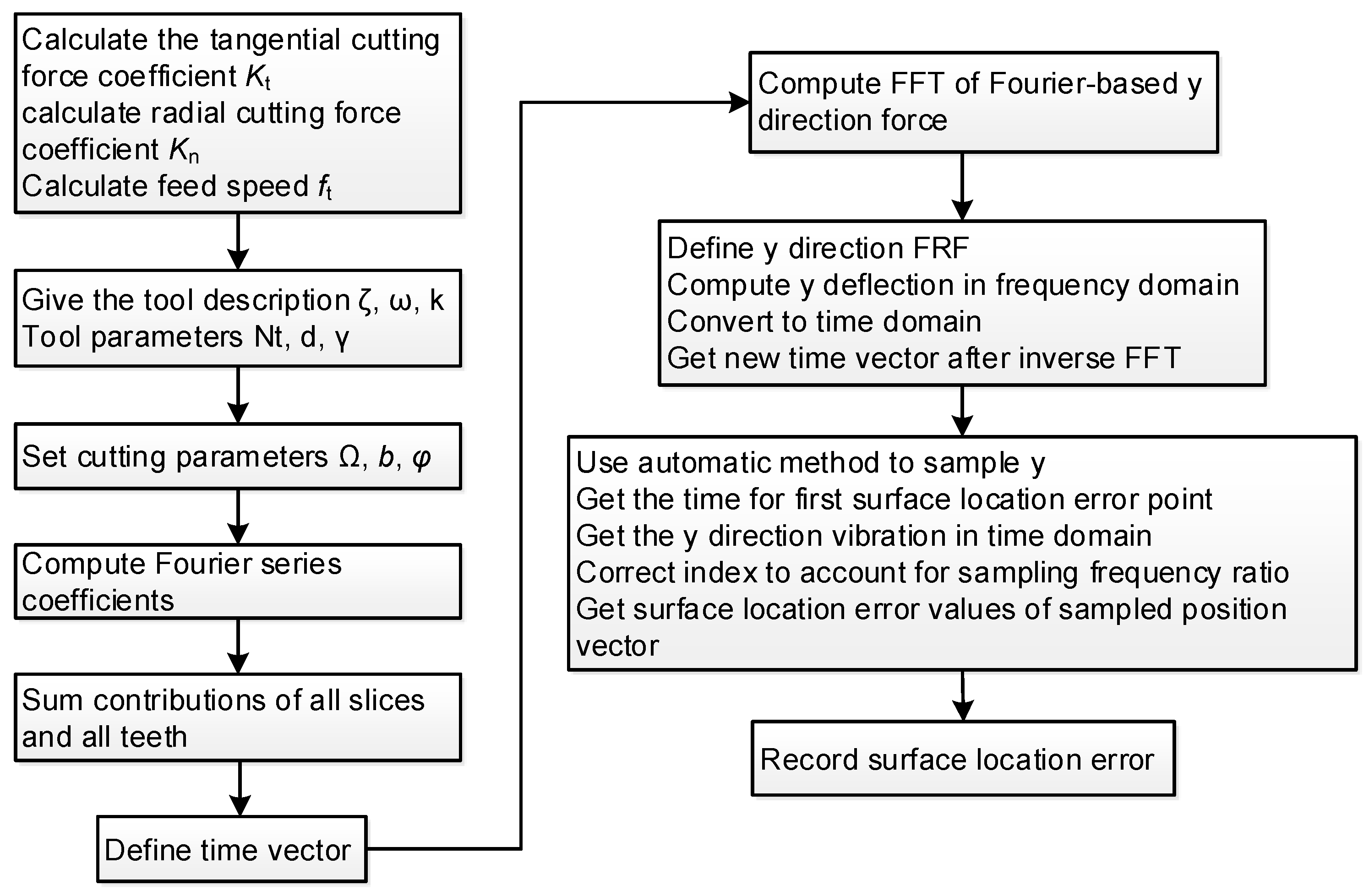
2.1. Modeling of the Cutting Force of Micro Thin-Walled Structures

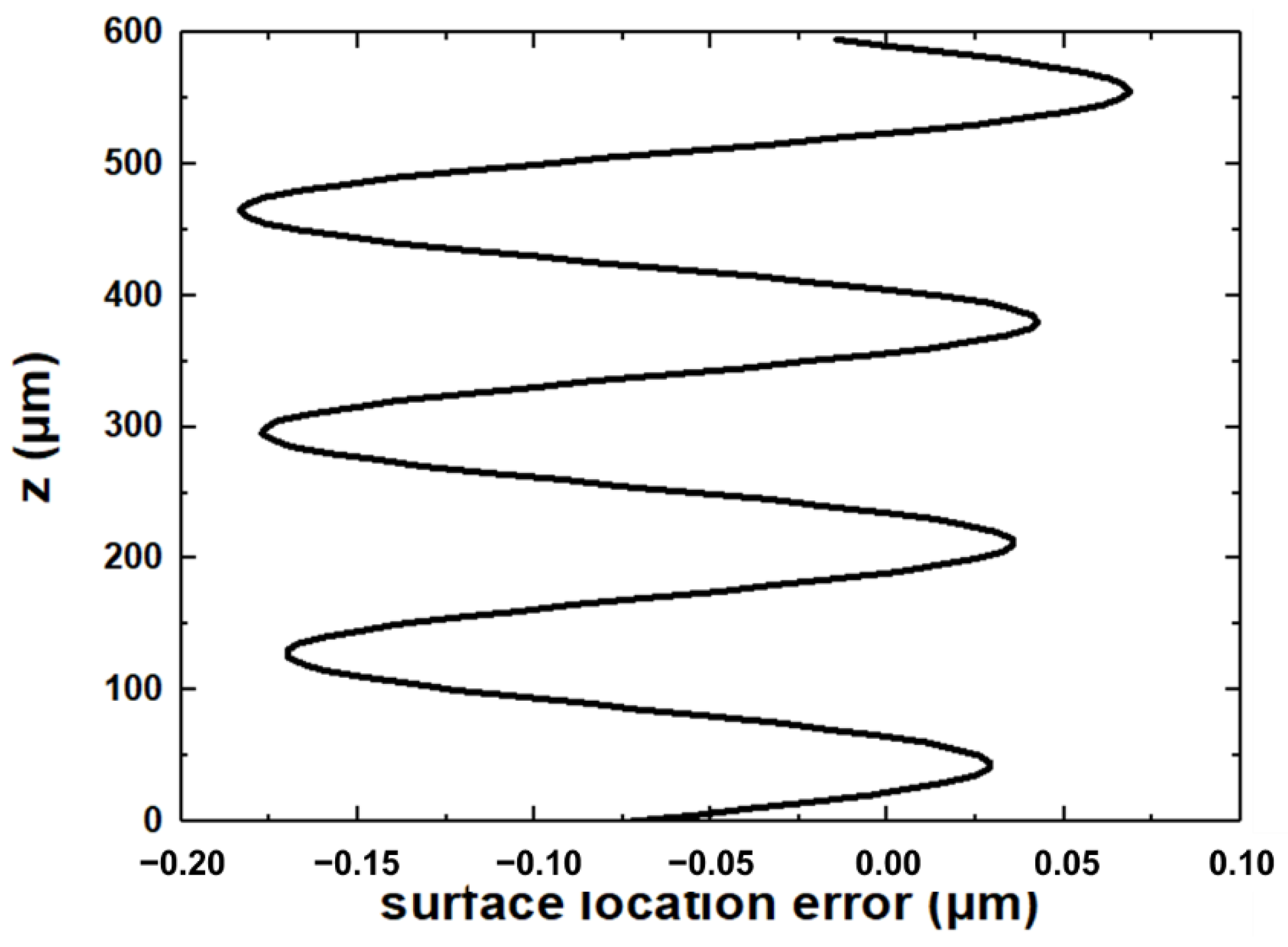
2.2. Analysis of Surface Location Error
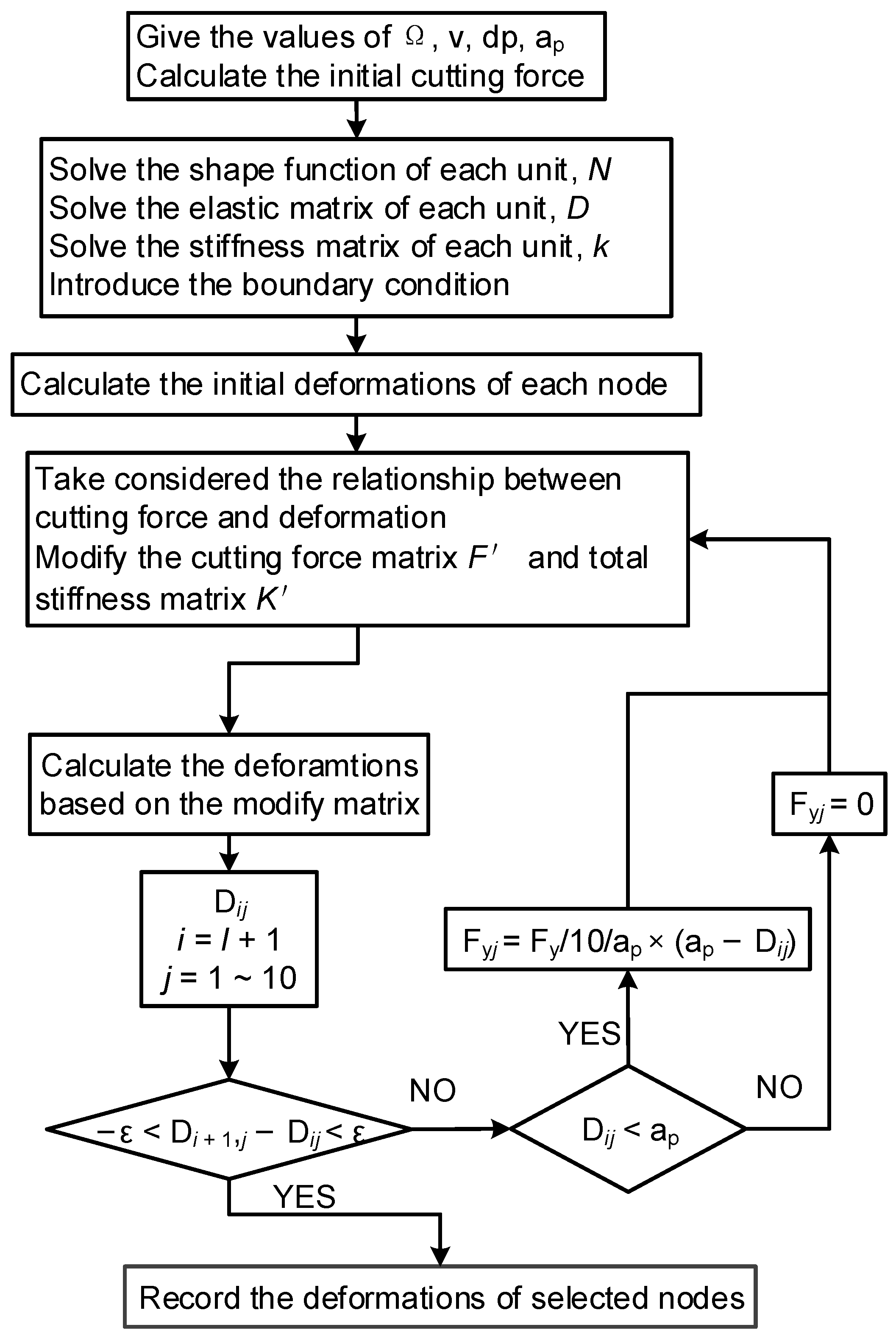
2.3. Error Prediction of Cutting Deformation and Error
3. DEFORM-3D Modeling and Simulation
3.1. Three-Dimensional Modeling of Milling Micro Thin-Walled Structure
3.2. Material Properties
3.3. Friction and Heat Transfer
4. Experimental Processes
5. Results and Discussion
5.1. Deformation of Iterative FEM
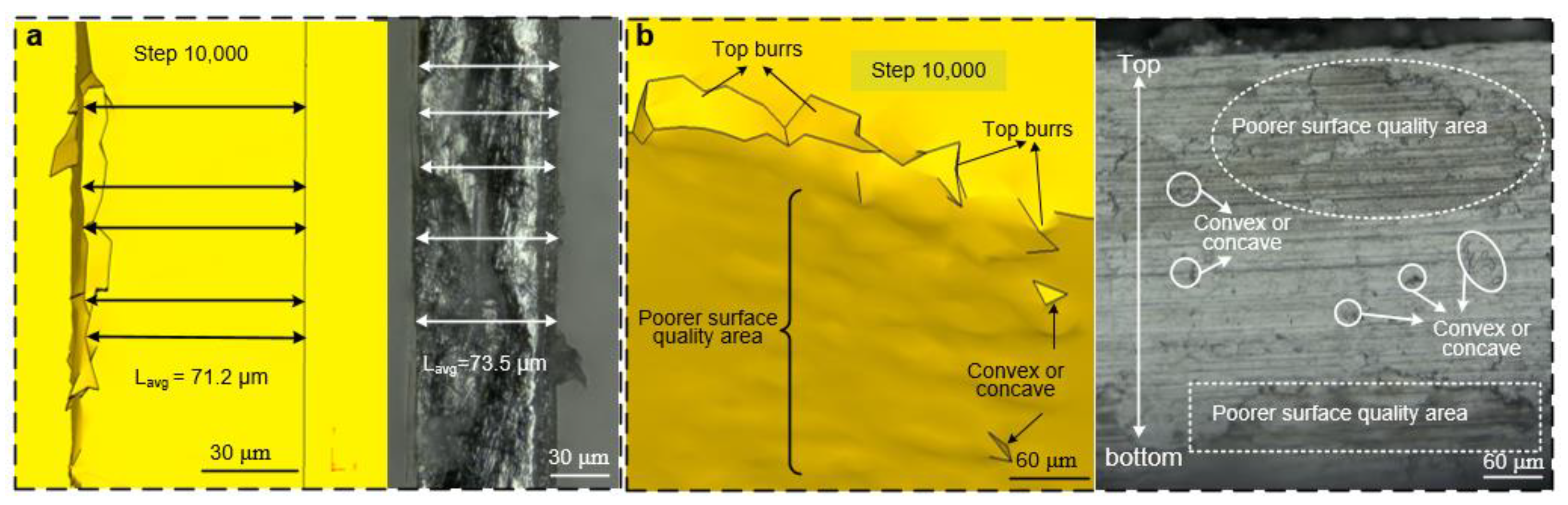
5.2. Deformations of Deform-3D Simulation and Experiments
6. Conclusions
- The thickness of the thin-walled structure obtained by the iterative FEM simulation, DEFORM-3D simulation and experiments are all larger than the ideal value, illustrating that there is a deformation in the process of micro-milling processing.
- The final machining thickness error among the three methods is less than 2.5 μm, providing a reference for calculating the deformation and final thickness of thin-walled structures according to the milling force.
- Affected by the vertical stiffness, the deformation at the top of the thin-walled structure is smaller than that at the bottom. The weak stiffness at the top of the thin-walled structure leads to a higher surface location error.
- Some defects (convex or concave poorer surface quality area, and top burr) appears on the thin-walled structure surface, which directly affect the machining quality. Decreasing the deformations and defects is necessary to improve the surface quality and processing accuracy.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Dang, X.B.; Wan, M.; Yang, Y.; Zhang, W.H. Efficient prediction of varying dynamic characteristics in thin-wall milling using freedom and mode reduction methods. Int. J. Mech. Sci. 2019, 150, 202–216. [Google Scholar] [CrossRef]
- Wu, G.; Li, G.X.; Pan, W.C.; Wang, X.; Ding, S.L. A prediction model for the milling of thin-wall parts considering thermal-mechanical coupling and tool wear. Int. J. Adv. Manuf. Technol. 2020, 107, 4645–4659. [Google Scholar]
- Wan, M.; Zhang, W.H.; Qin, G.H.; Wang, Z.P. Strategies for error prediction and error control in peripheral milling of thin-walled workpiece. Int. J. Mach. Tools. Manuf. 2008, 48, 1366–1374. [Google Scholar] [CrossRef]
- Wan, M.; Gao, T.Q.; Feng, J.; Zhang, W.H. On improving chatter stability of thin-wall milling by prestressing. J. Mater. Process. Technol. 2019, 264, 32–44. [Google Scholar] [CrossRef]
- Tang, A.J.; Liu, Z.Q. Deformations of thin-walled plate due to static end milling force. J. Mater. Process. Technol. 2008, 206, 345–351. [Google Scholar]
- Gang, L. Study on deformation of titanium thin-walled part in milling process. J. Mater. Process. Technol. 2009, 209, 2788–2793. [Google Scholar] [CrossRef]
- Ning, H.; Wang, Z.G.; Jiang, C.Y.; Zhang, B. Finite element method analysis and control stratagem for machining deformation of thin-walled components. J. Mater. Process. Technol. 2003, 139, 332–336. [Google Scholar] [CrossRef]
- Guo, H.; Zuo, D.W.; Wu, H.B.; Xu, F.; Tong, G.Q. Prediction on milling distortion for aero-multi-frame parts. Mater. Sci. Eng. A 2009, 499, 230–233. [Google Scholar] [CrossRef]
- Bolar, G.; Joshi, S.N. Three-dimensional numerical modeling, simulation and experimental validation of milling of a thin-wall component. Proc. Inst. Mech. Eng. Part. B J. Eng. Manuf. 2017, 231, 792–804. [Google Scholar] [CrossRef]
- Guo, W.C.; Wu, C.J.; Ding, Z.S.; Zhou, Q.Z. Prediction of surface roughness based on a hybrid feature selection method and long short-term memory network in grinding. Int. J. Adv. Manuf. Technol. 2021, 112, 2853–2871. [Google Scholar] [CrossRef]
- Sun, Y.; Su, Z.P.; Gong, Y.D.; Ba, D.; Yin, G.Q.; Zhang, H.; Zhou, L.H. Analytical and experimental study on micro-grinding surface-generated mechanism of DD5 single-crystal superalloy using micro-diamond pencil grinding tool. Arch. Civ. Mech. Eng. 2021, 21, 1–22. [Google Scholar] [CrossRef]
- Kou, Z.J.; Wan, Y.; Liu, Z.Q.; Cai, Y.K.; Liang, X.C. Deformation control in micro-milling of thin-walled structures. Int. J. Adv. Manuf. Technol. 2015, 81, 967–974. [Google Scholar] [CrossRef]
- Sridhar, G.; Ramesh, B.P. Effect of a milling cutter diameter on distortion due to the machining of thin wall thin floor components. Adv. Prod. Eng. Manag. 2015, 10, 140–152. [Google Scholar] [CrossRef] [Green Version]
- Guo, Y.Y.; Zhong, H.Y.; He, W.T.; Tao, G.F. Experiment study on efficient flank milling of Ti6AL4V thin-walled components. Int. J. Multimed. Ubiquitous. Eng. 2016, 11, 79–86. [Google Scholar] [CrossRef]
- Annoni, M.; Rebaioli, L.; Semeraro, Q. Thin wall geometrical quality improvement in micro milling. Int. J. Adv. Manuf. Technol. 2015, 79, 881–895. [Google Scholar] [CrossRef] [Green Version]
- Kolluru, K.; Axinte, D. Coupled interaction of dynamic responses of tool and workpiece in thin wall milling. J. Mater. Process. Technol. 2013, 213, 1565–1574. [Google Scholar] [CrossRef]
- Duan, Z.J.; Li, C.H.; Ding, W.F.; Zhang, Y.B.; Yang, M.; Gao, T.; Cao, H.J.; Xu, X.F.; Wang, D.Z.; Mao, C.; et al. Milling force model for aviation aluminum alloy: Academic insight and perspective analysis. Chin. J. Mech. Eng. 2021, 34, 1–35. [Google Scholar] [CrossRef]
- Rai, J.K.; Xirouchakis, P. Finite element method based machining simulation environment for analyzing part errors induced during milling of thin-walled components. Int. J. Mach. Tools. Manuf. 2008, 48, 629–643. [Google Scholar] [CrossRef]
- Daniyan, I.A.; Fameso, F.; Ale, F.; Bello, K.A.; Tlhabadira, I. Modelling, simulation and experimental validation of the milling operation of titanium alloy (Ti6Al4V). Int. J. Adv. Manuf. Technol. 2020, 109, 1853–1866. [Google Scholar] [CrossRef]
- Xu, J.T.; Xu, L.K.; Geng, Z.; Sun, Y.W.; Tang, K. 3D surface topography simulation and experiments for ball-end NC milling considering dynamic feedrate. CIRP J. Manuf. Sci. Technol. 2020, 31, 210–223. [Google Scholar] [CrossRef]
- Ratchev, S.; Liu, S.H.; Huang, W.J.; Becker, A.A. Milling error prediction and compensation in machining of low-rigidity parts. Int. J. Mach. Tools. Manuf. 2004, 44, 1629–1641. [Google Scholar] [CrossRef]
- Cheng, Y.N.; Zuo, D.G.; Wu, M.Y.; Feng, X.M.; Zhang, Y. Study on simulation of machining deformation and experiments for thin-walled parts of titanium alloy. Int. J. Control. Autom. 2015, 8, 401–410. [Google Scholar] [CrossRef]
- Huang, X.M.; Sun, J.; Li, J.F. Finite element simulation and experimental investigation on the residual stress-related monolithic component deformation. Int. J. Adv. Manuf. Technol. 2015, 77, 1035–1041. [Google Scholar] [CrossRef]
- Li, P.; Zdebski, D.; Langen, H.H.; Hoogstrate, A.M.; Oosterling, J.A.J.; Schmidt, R.H.M.; Allen, D.M. Micro milling of thin ribs with high aspect ratios. J. Micromech. Microeng. 2010, 20, 115013. [Google Scholar] [CrossRef] [Green Version]
- Rai, J.K.; Xirouchakis, P. FEM-based prediction of workpiece transient temperature distribution and deformations during milling. Int. J. Adv. Manuf. Technol. 2009, 42, 429–449. [Google Scholar] [CrossRef] [Green Version]
- Thepsonthi, T.; Özel, T. Experimental and finite element simulation based investigations on micro-milling Ti-6Al-4V titanium alloy: Effects of cBN coating on tool wear. J. Mater. Process. Technol. 2013, 213, 532–542. [Google Scholar] [CrossRef]
- Özel, T.; Sima, M.; Srivastava, A.K.; Kaftanoglu, B. Investigations on the effects of multi-layered coated inserts in machining Ti–6Al–4V alloy with experiments and finite element simulations. CIRP Ann. Manuf. Technol. 2010, 59, 77–82. [Google Scholar] [CrossRef]
- Arrazola, P.J.; Özel, T.; Umbrello, D.; Davies, M.; Jawahir, I.S. Recent advances in modelling of metal machining processes. CIRP. Ann. Manuf. Technol. 2013, 62, 695–718. [Google Scholar] [CrossRef]
- Liu, Y.; Li, P.F.; Liu, K.; Zhang, Y.M. Micro milling of copper thin wall structure. Int. J. Adv. Manuf. Technol. 2017, 90, 405–412. [Google Scholar] [CrossRef]
- Jin, X.L.; Altintas, Y. Prediction of micro-milling forces with finite element method. J. Mater. Process. Technol. 2012, 212, 542–552. [Google Scholar] [CrossRef]
- Johnson, G.R.; Cook, W.H. A constitutive model and data for materials subjected to large strains, high strain rates, and high temperatures. Eng. Fract. Mech. 1983, 21, 541–547. [Google Scholar]
- Jagadeesh, S.K.; Ramesh, C.S.; Mallikarjuna, J.M.; Keshavamurthy, R. Prediction of cooling curves during solidification of Al 6061-SiCp based metal matrix composites using finite element analysis J. Mater. Process. Technol. 2013, 213, 532–542. [Google Scholar] [CrossRef]
- Jia, Z.Y.; Lu, X.H.; Gu, H.; Ruan, F.X.; Liang, S.Y. Deflection prediction of micro-milling Inconel 718 thin-walled parts. J. Mater. Process. Technol. 2021, 291, 117003. [Google Scholar] [CrossRef]
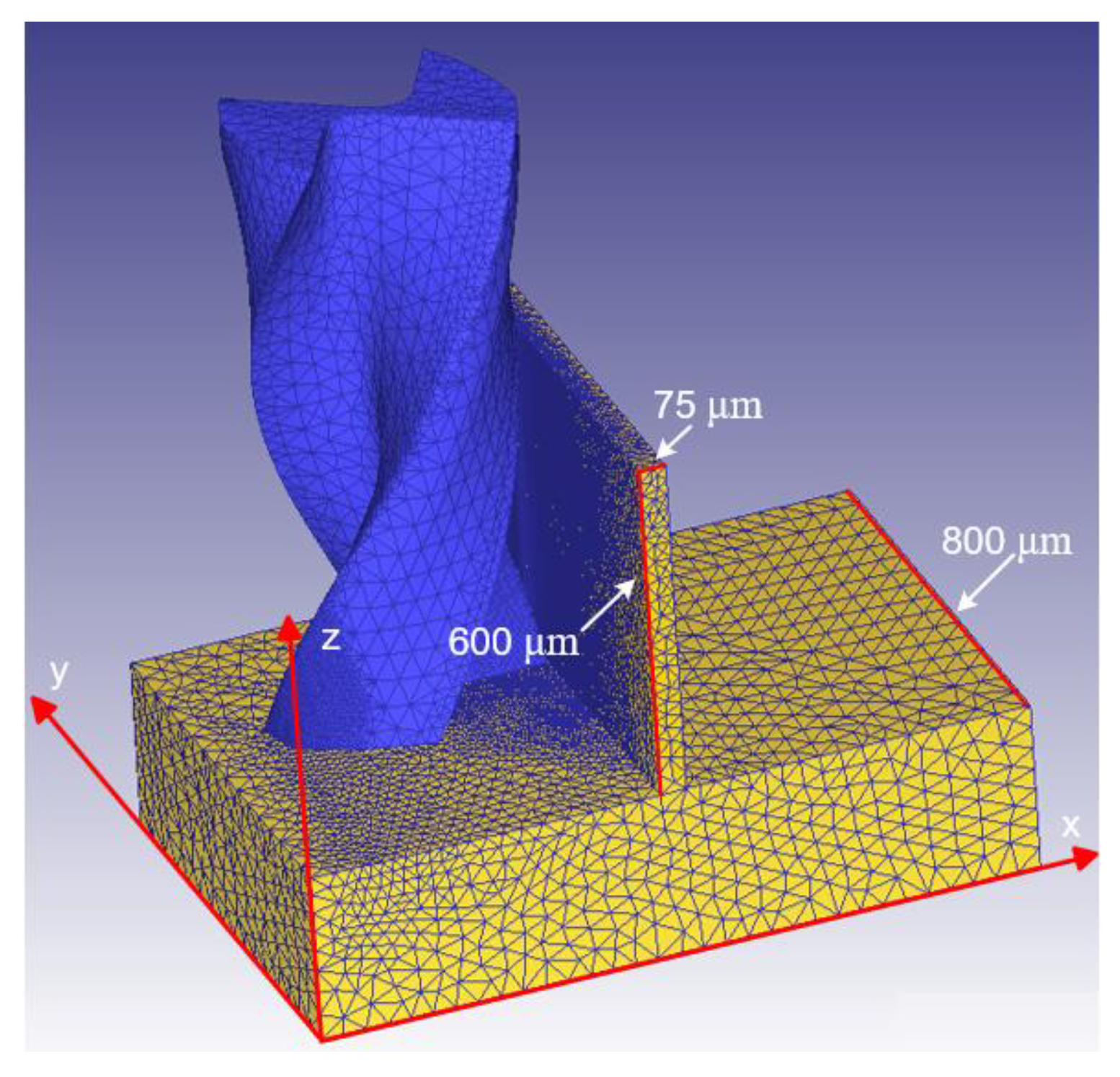


| Items | Values | Items | Values |
|---|---|---|---|
| αt | 490 | αn | 128.8 |
| dt | –0.351 | dn | –0.3355 |
| βt | 2123 | βn | 894.8 |
| pt | –0.719 | pn | –0.9272 |
| qt | 1.055 | qn | 0.9696 |
| A (MPa) | B (MPa) | C | N | m | Tm (K) | Tr (K) | (s−1) |
|---|---|---|---|---|---|---|---|
| 324 | 114 | 0.011 | 0.35 | 1.34 | 293.15 | 923.15 | 1 |
| Density (kg⋅m−3) | Elastic Modulus (GPa) | Poisson’s Ratio | Conductivity (W∙m−1∙K−1) | Specific Heat (J∙kg−1∙K−1) | Inelastic Heat Fraction |
|---|---|---|---|---|---|
| 2700 | 68.9 | 0.33 | 167 | 896 | 23.6 |
| Rake Angle (°) | Relief Angle (°) | Edge Radius (μm) | Cutter Diameter (μm) | Helix Angle (°) |
|---|---|---|---|---|
| –2 | 17 | 4 | 500 | 30 |
| Methods | DEFORM-3D Simulation | Iterative Simulation | Experiment |
|---|---|---|---|
| Thickness (μm) | 71.2 | 72.5 | 73.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, Q.; Zhou, J.; Li, P. Simulations and Experiments on the Micro-Milling Process of a Thin-Walled Structure of Al6061-T6. Materials 2022, 15, 3568. https://doi.org/10.3390/ma15103568
Sun Q, Zhou J, Li P. Simulations and Experiments on the Micro-Milling Process of a Thin-Walled Structure of Al6061-T6. Materials. 2022; 15(10):3568. https://doi.org/10.3390/ma15103568
Chicago/Turabian StyleSun, Qi, Jianzhong Zhou, and Pengfei Li. 2022. "Simulations and Experiments on the Micro-Milling Process of a Thin-Walled Structure of Al6061-T6" Materials 15, no. 10: 3568. https://doi.org/10.3390/ma15103568
APA StyleSun, Q., Zhou, J., & Li, P. (2022). Simulations and Experiments on the Micro-Milling Process of a Thin-Walled Structure of Al6061-T6. Materials, 15(10), 3568. https://doi.org/10.3390/ma15103568





