The Confinement-Affected Strength Variety of Anisotropic Rock Mass
Abstract
1. Introduction
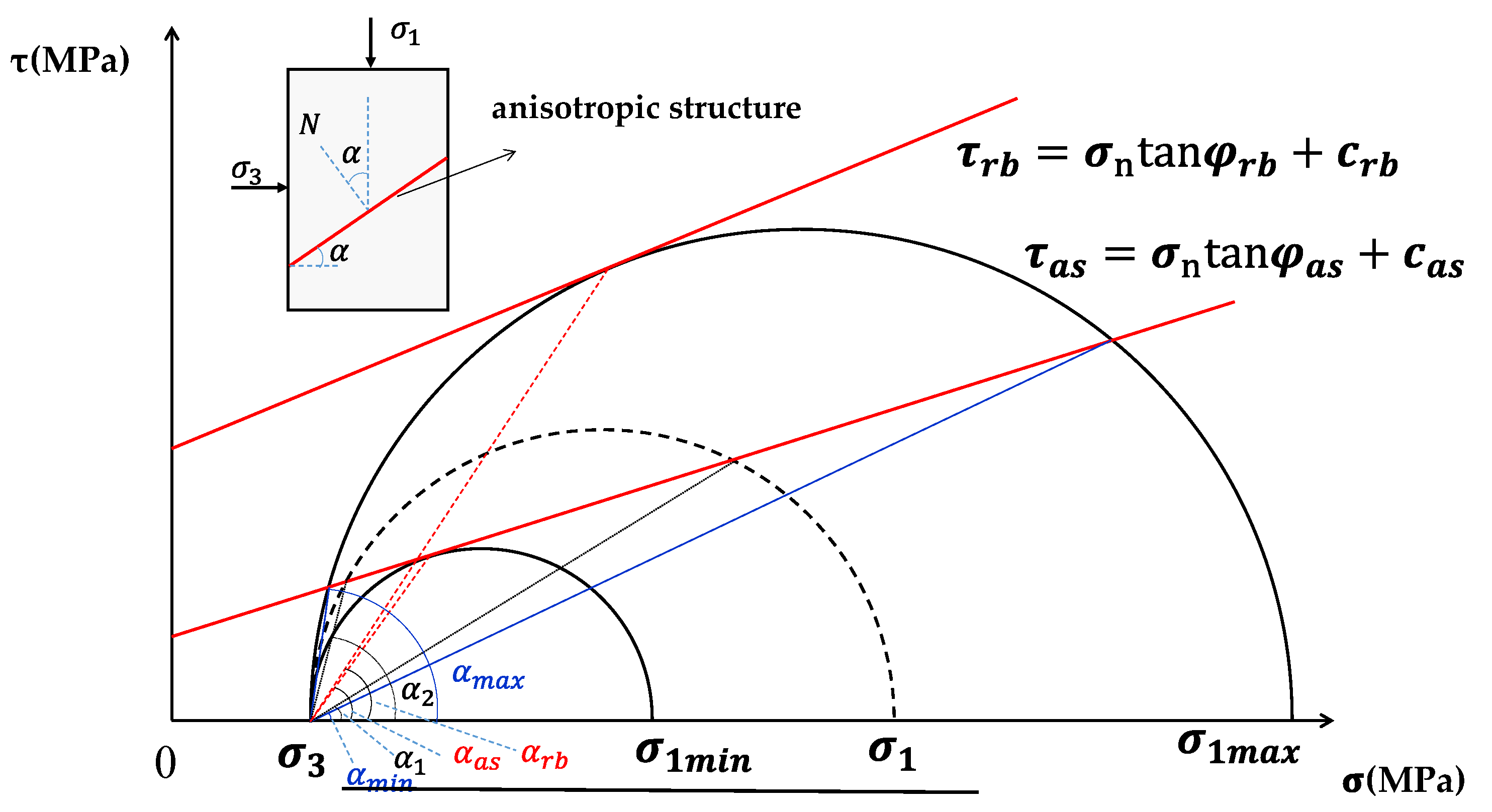
2. Theoretical Analysis on Strength of Anisotropic Rock Mass
3. Verification Study of Existing Experimental Data
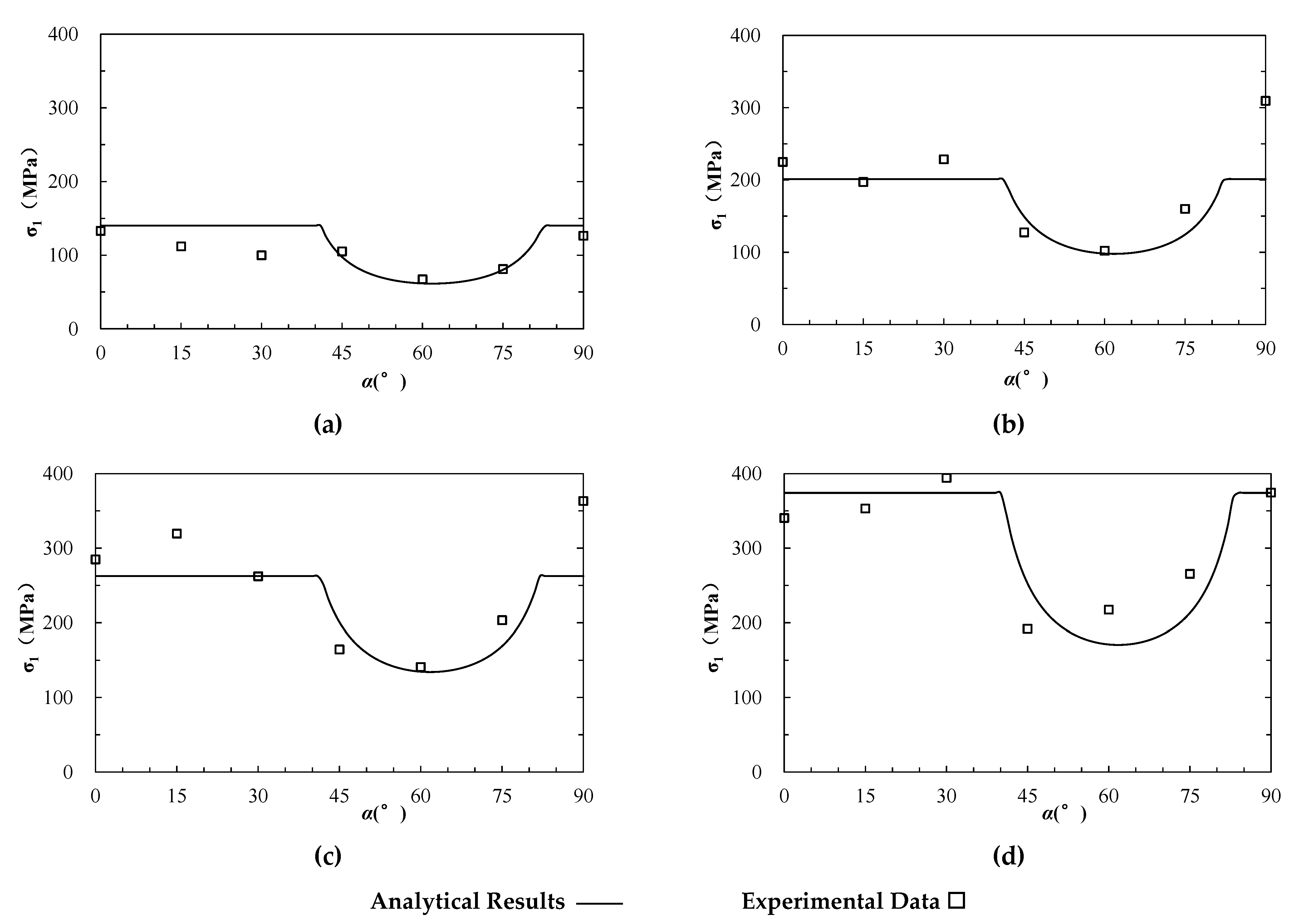
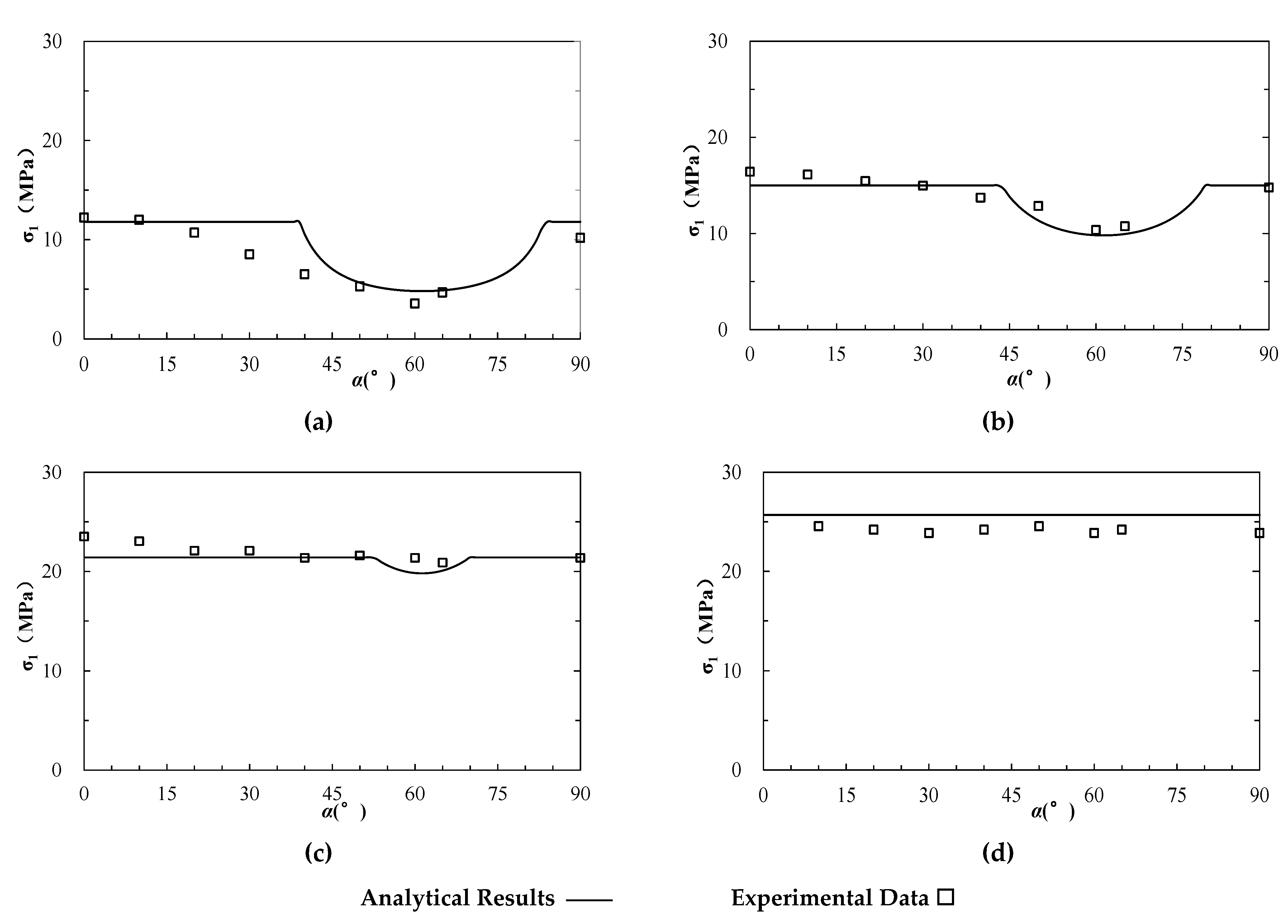
3.1. Dolomitic Limestone
3.2. Lyonian Sandstone
3.3. Plaster of Paris with Artificial Contact Joint
4. Discussion
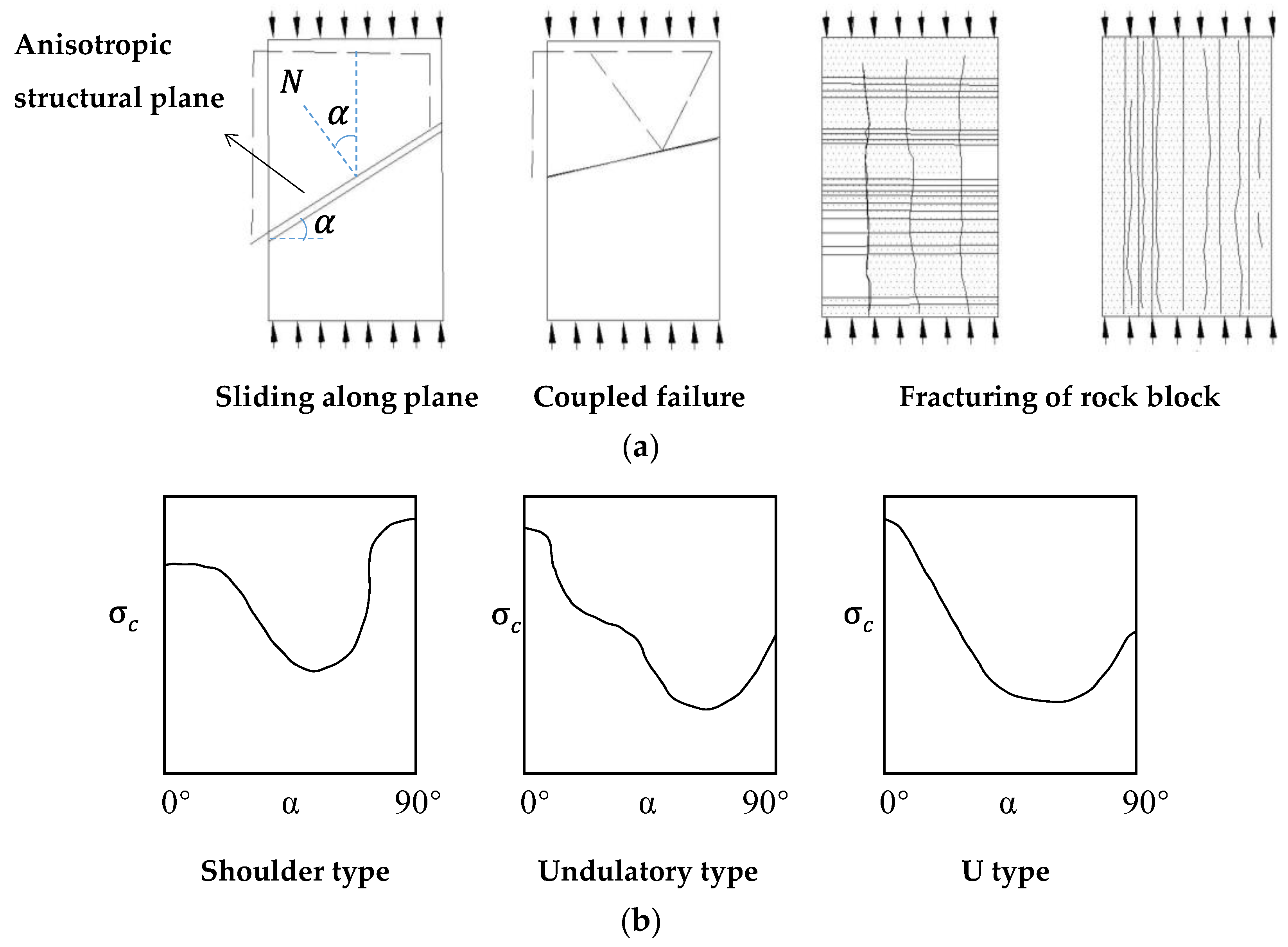
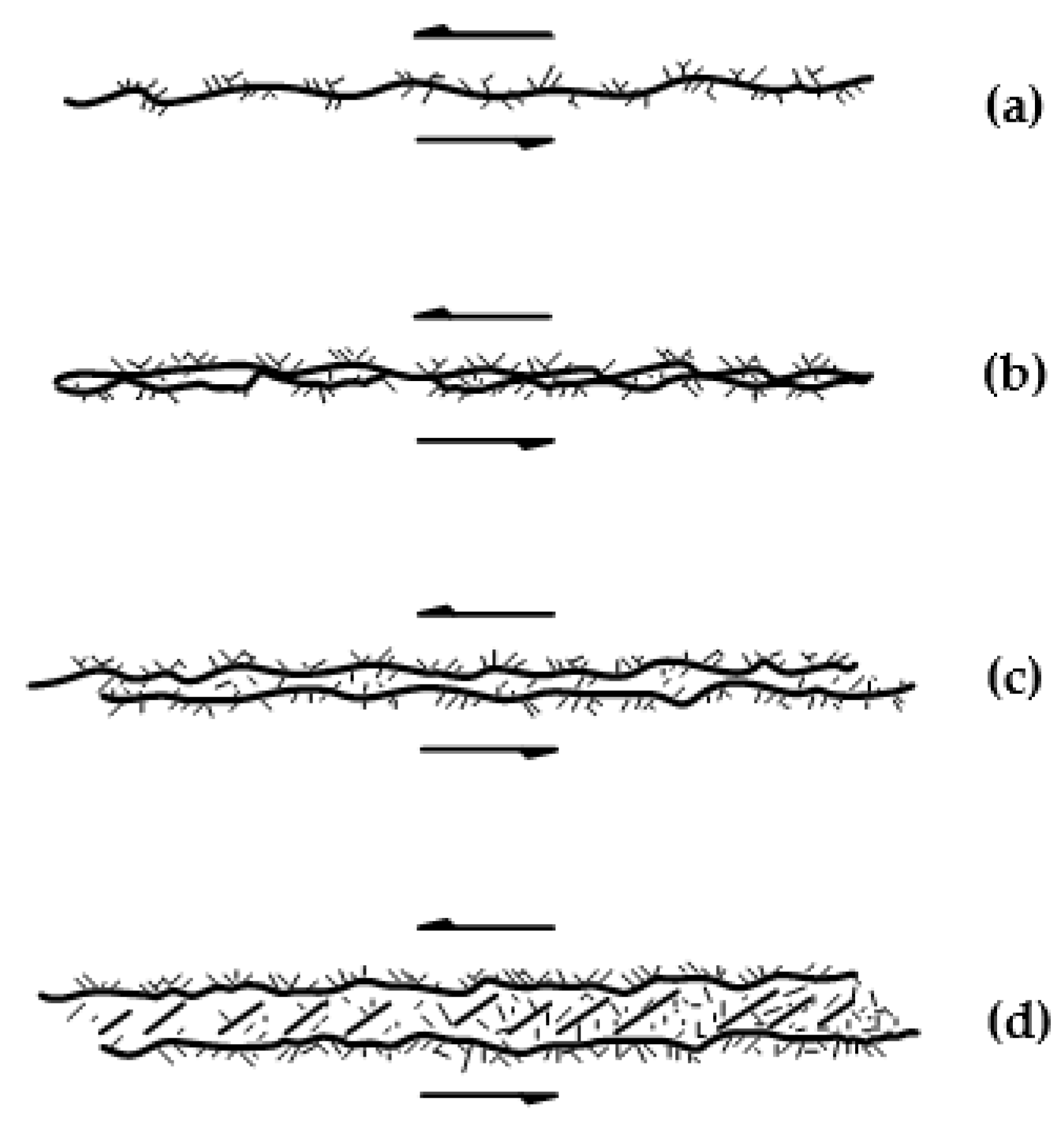
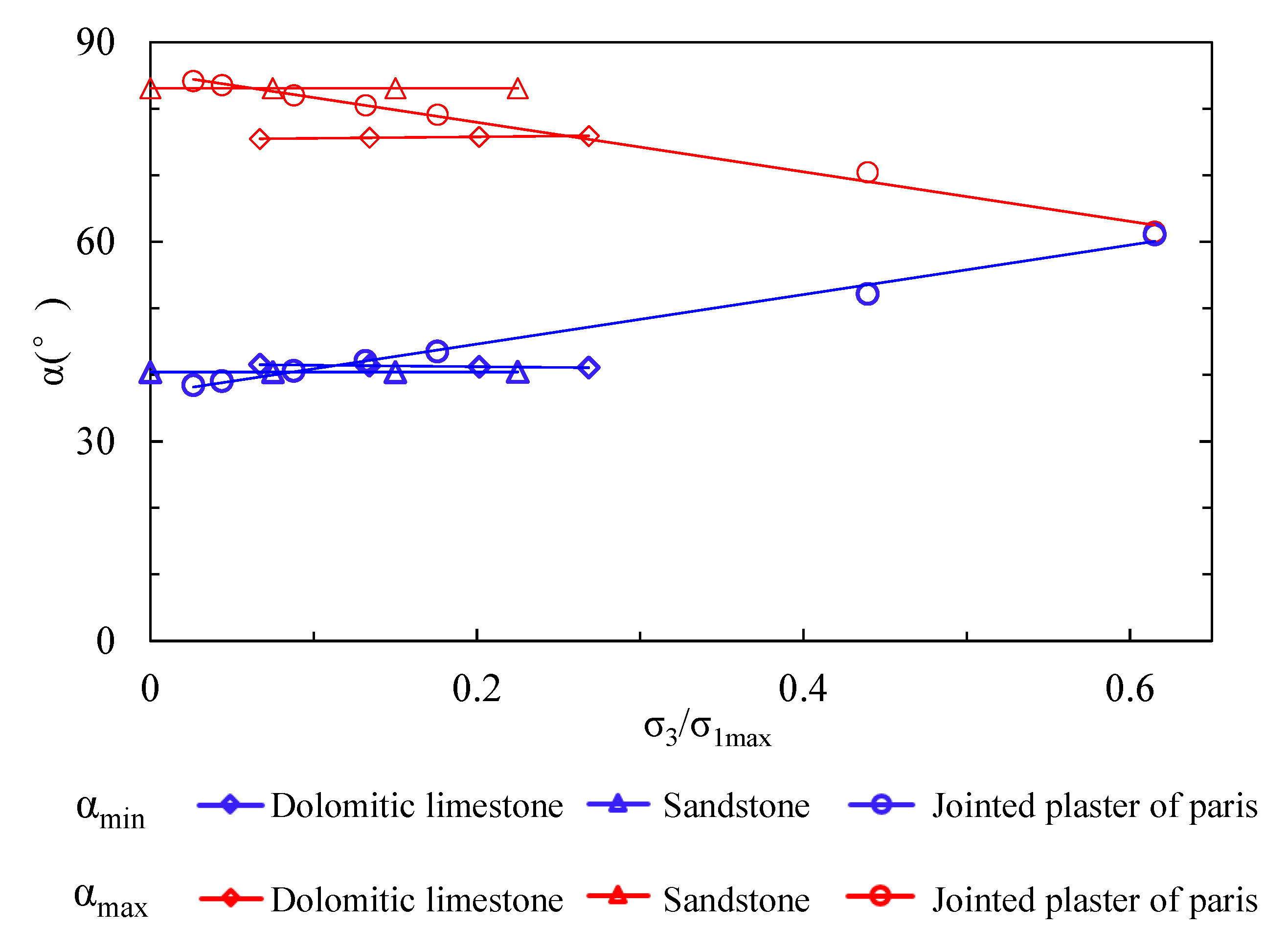
4.1. The Effects of Confinement on the Possibility of Anisotropic Structural Plane-Controlled Strength
4.2. The Effects of Confinement on Strength Anisotropy Degree
4.3. Further Discussions
5. Conclusions Remarks
- (1)
- The anisotropic structural planes incorporate both weak-filled layers and hard contact discontinuities, which cause strength anisotropy and different failure modes of rock mass at low confining pressures;
- (2)
- The commonly used Mohr–Coulomb strength criterion is adopted to depict the strength of both rock block and anisotropic structural plane, based on which the formulas to estimate anisotropic strength under certain confining pressures are developed. The formulas compare well with the compression experiments data of various anisotropic rock types under different confining pressures;
- (3)
- The possibility of anisotropic structural plane-controlled rock strength as confining pressure increases is not definite but theoretically related to the comparison between and ;
- (4)
- Likewise, the tendency of strength anisotropy degree with increasing confinement is not definite either. As confining pressure increases, the anisotropic strength ratio (ASD) always decreases, while the anisotropic strength difference (ASD) increases or decreases depending on the friction strength of the rock block and anisotropic structural plane;
- (5)
- The different anisotropic structural plane types may lead to distinct behaviors under high confinement, i.e., soft-filled layers or hard contact discontinuities-induced anisotropy.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
References
- Jaeger, J.C. Shear fracture of anisotropic rocks. Geol. Mag. 1960, 97, 65–72. [Google Scholar] [CrossRef]
- Sun, G. Rock Mass Structure Mechanics; Science Press: Beijing, China, 1988. [Google Scholar]
- Ramamurthy, T.; Arora, V.K. Strength predictions for jointed rocks in confined and unconfined states. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1994, 31, 9–22. [Google Scholar] [CrossRef]
- Zangerl, C.; Loew, S.; Eberhardt, E. Structure, geometry and formation of brittle discontinuities in anisotropic crystalline rocks of the Central Gotthard Massif, Switzerland. Eclogae Geol. Helv. 2006, 99, 271–290. [Google Scholar] [CrossRef]
- Bidgoli, M.N.; Jing, L. Anisotropy of strength and deformability of fractured rocks. J. Rock Mech. Geotech. Eng. 2014, 6, 156–164. [Google Scholar] [CrossRef]
- Guo, S.; Qi, S.; Zou, Y.; Zheng, B. Numerical Studies on the Failure Process of Heterogeneous Brittle Rocks or Rock-Like Materials under Uniaxial Compression. Materials 2017, 10, 378. [Google Scholar] [CrossRef]
- Guo, S.; Qi, S.; Zhan, Z.; Ma, L.; Getahun, E.; Zhang, S. Numerical study on the progressive failure of heterogeneous geomaterials under varied confining stresses. Eng. Geol. 2022, 269, 10556. [Google Scholar] [CrossRef]
- Margielewski, W. Structural control and types of movements of rock mass in anisotropic rocks: Case studies in the Polish Flysch Carpathians. Geomorphology 2006, 77, 47–68. [Google Scholar] [CrossRef]
- Wang, S.H.; Lee, C.I.; Ranjith, P.G.; Tang, C.A. Modelling the effects of heterogeneity and anisotropy on the excavation damage/disturbed zone (EDZ). Rock Mech. Rock Eng. 2009, 42, 229–258. [Google Scholar] [CrossRef]
- Zhang, Z.X.; Xu, Y.; Kulatilake, P.H.S.W.; Huang, X. Physical model test and numerical analysis on the behavior of stratified rock masses during underground excavation. Int. J. Rock Mech. Min. Sci. 2012, 49, 134–147. [Google Scholar] [CrossRef]
- Jiang, Q.; Feng, X.; Hatzor, Y.H.; Hao, X.; Li, S. Mechanical anisotropy of columnar jointed basalts: An example from the Baihetan hydropower station, China. Eng. Geol. 2014, 175, 35–45. [Google Scholar] [CrossRef]
- Shen, B.; Siren, T.; Rinne, M. Modelling fracture propagation in anisotropic rock mass. Rock Mech. Rock Eng. 2015, 48, 1067–1081. [Google Scholar] [CrossRef]
- Özbek, A.; Gul, M.; Karacan, E.; Alca, O. Anisotropy effect on strengths of metamorphic rocks. J. Rock Mech. Geotech. Eng. 2018, 10, 164–175. [Google Scholar] [CrossRef]
- Sun, X.; Chen, F.; Miao, C.; Song, P.; Li, G.; Zhao, C.; Xia, X. Physical modeling of deformation failure mechanism of surrounding rocks for the deep buried tunnel in soft rock strata during the excavation. Tunn. Undergr. Space Technol. 2018, 74, 247–261. [Google Scholar] [CrossRef]
- He, J.; Qi, S.; Zhan, Z.; Guo, S.; Li, C.; Zheng, B.; Huang, X.; Zou, Y.; Yang, G.; Liang, N. Seismic response characteristics and deformation evolution of the bedding rock slope using a large-scale shaking table. Landslides 2021, 18, 2835–2853. [Google Scholar] [CrossRef]
- Guo, X.; Deng, P.; Liu, Q.; Xu, X.; Wang, N.; Jiang, Y.; Yu, Y. Progressive fracture and swelling of anisotropic rock masses around deep tunnels: A new floor heave mechanical mechanism. Arab. J. Geosci. 2022, 15, 1325. [Google Scholar] [CrossRef]
- Singh, D.; Singh, P.K.; Kainthola, A.; Pandey, H.K.; Kumar, S.; Singh, T.N. Analysis of failure pattern in cut slopes of bedded sandstone: A case study. Environ. Earth Sci. 2022, 81, 398. [Google Scholar] [CrossRef]
- Donath, F.A. Experimental study of shear failure in anisotropic rocks. Geol. Soc. Am. Bull. 1961, 72, 985–990. [Google Scholar] [CrossRef]
- Saeidi, Q.; Rasouli, V.; Vaneghi, R.G.; Gholami, R.; Torabi, S.R. A modified failure criterion for transversely isotropic rocks. Geosci. Front. 2014, 5, 215–225. [Google Scholar] [CrossRef]
- Acharya, D.; Raina, A.K.; Panthee, S. Relationship between point load index and compressive strength of foliated metamorphic rocks at different loading angels. Arab. J. Geosci. 2022, 15, 490. [Google Scholar] [CrossRef]
- Singh, V.K.; Singh, D.; Singh, T.N. Prediction of strength properties of some schistose rocks from petrographic properties using artificial neural networks. Int. J. Rock Mech. Min. Sci. 2001, 38, 269–284. [Google Scholar] [CrossRef]
- Nasseri, M.H.B.; Rao, K.S.; Ramamurthy, T. Anisotropic strength and deformational behavior of Himalayan schists. Int. J. Rock Mech. Min. Sci. 2003, 40, 3–23. [Google Scholar] [CrossRef]
- Saroglou, H.; Tsiambaos, G. A modified Hoek- Brown failure criterion for anisotropic intact rock. Int. J. Rock Mech. Min. Sci. 2008, 45, 223–234. [Google Scholar] [CrossRef]
- Ghazvinian, A.; Vaneghi, R.G.; Hadei, M.R.; Azinfar, M.J. Shear behavior of inherently anisotropic rocks. Int. J. Rock Mech. Min. Sci. 2013, 61, 96–110. [Google Scholar] [CrossRef]
- Rao, K.S.; Rao, G.V.; Ramamurthy, T. A strength criterion for anisotropic rocks. Indian. Geotech. J. 1986, 16, 317–333. [Google Scholar]
- Niandou, H.; Shao, J.; Henry, J.P.; Fourmaintraux, D. Laboratory investigation of the mechanical behavior of Tournemire shale. Int. J. Rock Mech. Min. Sci. 1997, 34, 3–16. [Google Scholar] [CrossRef]
- Cheng, C.; Li, X.; Qian, H. Anisotropic failure strength of shale with increasing confinement: Behaviors, factors and mechanism. Materials 2017, 10, 1310. [Google Scholar] [CrossRef]
- Tien, Y.M.; Kuo, M.C. A failure criterion for transversely isotropic rocks. Int. J. Rock Mech. Min. Sci. 2001, 38, 399–412. [Google Scholar] [CrossRef]
- McGill, G.E.; Raney, J.A. Experimental study of faulting in an anisotropic, inhomogeneous dolomitic limestone. Geol. Soc. Am. Bull. 1970, 81, 2949–2958. [Google Scholar] [CrossRef]
- Xu, X.; Liu, B.; Li, S.; Yang, L.; Song, J.; Li, M.; Mei, J. Experimental study on conductivity anisotropy of limestone considering the bedding directional effect in the whole process of uniaxial compression. Materials 2016, 9, 165. [Google Scholar] [CrossRef]
- Ajalloeian, R.; Lashkaripour, R.G. Strength anisotropies in mudrocks. Bull. Eng. Geol. Environ. 2000, 59, 195–199. [Google Scholar] [CrossRef]
- Zhang, T.; Xu, W.; Wang, H.; Wang, R.; Yan, L.; Hu, M. Anisotropic mechanical behavior of columnar jointed rock masses subjected to cyclic loading: An experimental investigation. Int. J. Rock Mech. Min. Sci. 2021, 144, 104954. [Google Scholar] [CrossRef]
- Tien, Y.M.; Kuo, M.C.; Juang, C.H. An experimental investigation of failure mechanism of simulated transversely isotropic rocks. Int. J. Rock Mech. Min. Sci. 2006, 43, 1163–1181. [Google Scholar] [CrossRef]
- Wang, P.; Liu, C.; Qi, Z.; Liu, Z.; Cai, M. A rough discrete fracture network model for geometrical modeling of jointed rock mass and the anisotropic behavior. Appl. Sci. 2022, 17, 1720. [Google Scholar] [CrossRef]
- Ramamurthy, T. Strength and modulus responses of anisotropic rocks. In Comprehensive Rock Engineering, Vol. 1. Fundamentals; Hudson, J.A., Ed.; Pergamon Press: Oxford, UK, 1993; pp. 313–329. [Google Scholar]
- Singh, B.; Geol, R.K.; Mehrotra, V.K.; Gary, S.K.; Allu, M.R. Effect of intermediate principal stress on strength of anisotropic rock mass. Tunn. Undergr. Space Technol. 1998, 13, 71–79. [Google Scholar]
- Guo, S.; Qi, S. Numerical study on progressive failure of hard rock samples with an unfilled undulate joint. Eng. Geol. 2015, 193, 173–182. [Google Scholar] [CrossRef]
- Wu, N.; Liang, Z.; Li, Y.; Li, H.; Li, W.; Zhang, M. Stress-dependent anisotropy index of strength and deformability of jointed rock mass: Insights from a numerical study. Bull. Eng. Geol. Environ. 2019, 78, 5905–5917. [Google Scholar] [CrossRef]
- Zhou, R. The Effect of Confining Pressure and the Coefficient of Confining Pressure; Engineering Geomechanics Research; Geological Publishing House: Beijing, China, 1985; pp. 209–214. [Google Scholar]
- Guo, S.; Qi, S.; Huang, X. Anisotropy of rock mass strength and its transformation critical confining stress. Chin. J. Rock Mech. Eng. 2013, 32, 3222–3227. (In Chinese) [Google Scholar]
- Saroglou, C.; Qi, S.; Guo, S.; Wu, F. ARMR, a new classification system for the rating of anisotropic rock masses. Bull. Eng. Geol. Environ. 2019, 78, 3611–3626. [Google Scholar] [CrossRef]
- Guo, S.; Qi, S.; Saroglou, C. A-BQ, A classification system for anisotropic rock mass based on China National Standard. J. Cen. South. Univ. 2020, 27, 3090–3102. [Google Scholar] [CrossRef]
- Walsh, J.B.; Brace, W.F. A fracture criterion for brittle anisotropic rocks. J. Geophys. Res. 1964, 69, 3449. [Google Scholar] [CrossRef]
- Nova, R. The failure of transversely isotropic rocks in triaxial compression. Int. J. Rock Mech. Min. Sci. Geomech. Abstr. 1980, 17, 325–332. [Google Scholar] [CrossRef]
- Cazacu, O.; Cristescu, N.D.; Shao, J.F.; Henry, J.P. A new failure criterion for transversely isotropic rocks. Int. J. Rock Mech. Min. Sci. 1998, 35, 130. [Google Scholar] [CrossRef]
- Duveau, G.; Shao, J. A modified single plane of weakness theory for the failure of highly stratified rocks. Int. J. Rock Mech. Min. Sci. 1998, 35, 807–813. [Google Scholar] [CrossRef]
- Pouya, A.; Ghoreychi, M. Determination of rock mass strength properties by homogenization. Int. J. Numer. Anal. Met. 2001, 25, 1285–1303. [Google Scholar] [CrossRef]
- Chen, X.; Yang, Q.; Qiu, K.B.; Feng, J.L. An anisotropic strength criterion for jointed rock masses and its application in wellbore stability analyses. Int. J. Numer. Anal. Met. 2008, 32, 607–631. [Google Scholar] [CrossRef]
- Halakatevakis, N.; Sofianos, A.I. Strength of a blocky rock mass based on an extended plane of weakness theory. Int. J. Rock Mech. Min. Sci. 2010, 47, 568–582. [Google Scholar] [CrossRef]
- Triantafyllidis, T.; Gerolymatou, E. Estimation of the strength of stratified rock mass. Rock Mech. Rock Eng. 2014, 47, 535–547. [Google Scholar] [CrossRef]
- Guo, S.; Qi, S.; Zhan, Z.; Zheng, B. Plastic- strain- dependent strength model to simulate the cracking process of brittle rocks with an existing non-persistent joint. Eng. Geol. 2017, 231, 114–125. [Google Scholar] [CrossRef]
- Shen, J.; Shu, Z.; Cai, M.; Du, S. A shear strength model for anisotropic blocky rock masses with persistent joints. Int. J. Rock Mech. Min. Sci. 2020, 134, 104430. [Google Scholar] [CrossRef]
- Shi, X.; Yang, X.; Meng, Y.; Li, G. Modified Hoek- Brown failure criterion for anisotropic rocks. Environ. Earth Sci. 2016, 75, 995. [Google Scholar] [CrossRef]
- Wang, Z.; Qi, C.; Ban, L.; Yu, H.; Wang, H.; Fu, Z. Modified Hoek- Brown failure criterion for anisotropic intact rock under high confining pressures. Bull. Eng. Geol. Environ. 2022, 81, 333. [Google Scholar] [CrossRef]
- Gao, Z.; Zhao, J.; Yao, Y. A generalized anisotropic failure criterion for geomaterials. Int. J. Solids. Struct. 2010, 47, 3166–3185. [Google Scholar] [CrossRef]
- Asadi, M. Optimized Mamdani fuzzy models for predicting the strength of intact rocks and anisotropic rock masses. J. Rock Mech. Geotech. Eng. 2016, 8, 218–224. [Google Scholar] [CrossRef]
- Wen, L.; Luo, Z.; Yang, S.; Qin, Y.; Wang, W. Correlation of Geo-Mechanics Parameters with Uniaxial Compressive Strength and P-Wave Velocity on Dolomitic Limestone Using a Statistical Method. Geotech. Geol. Eng. 2019, 37, 1079–1094. [Google Scholar] [CrossRef]
- Youash, Y.Y. Experimental deformation of layered rocks. In Proceedings of the 1st ISRM Congress, Lisbon, Portugal, 25 September–1 October 1966. [Google Scholar]
- ISRM. Rock Characterization, Testing and Monitoring, ISRM Suggested Methods; Pergamon Press: Oxford, UK, 1981. [Google Scholar]
- Tsidzi, K. The influence of foliation on point load strength anisotropy of foliated rocks. Eng. Geol. 1990, 29, 49–58. [Google Scholar] [CrossRef]
- Hoek, E.; Brown, E.T. The Hoek-Brown failure criterion and GSI-2018 edition. J. Rock Mech. Geotech. Eng. 2019, 11, 445–463. [Google Scholar] [CrossRef]
- Barton, N.; Choubey, V. The shear strength of rock joints in theory and practice. Rock Mech. 1977, 10, 1–54. [Google Scholar] [CrossRef]
- Byerlee, J. Friction of Rocks. Pure. Appl. Geophys. 1978, 116, 615–626. [Google Scholar] [CrossRef]
- Ge, Y.; Kulatilake, P.H.S.W.; Tang, H.; Xiong, C. Investigation of natural rock joint roughness. Comput. Geotech. 2014, 55, 290–305. [Google Scholar] [CrossRef]
- Zheng, B.; Qi, S.; Luo, G.; Liu, F.; Huang, X.; Guo, S. Characterization of discontinuity morphology based on extensive 3D fractal dimension by integrating laser scanning with ArcGIS. Bull. Eng. Geol. Environ. 2021, 80, 2261–2281. [Google Scholar] [CrossRef]
- Barton, N. Review of a new shear-strength criterion for rock joints. Eng. Geol. 1973, 7, 287–332. [Google Scholar] [CrossRef]



| Rock Type | (MPa) | (°) | (MPa) | (°) | (Mpa) | (Mpa) | Variation with Increasing Confinement | ||
|---|---|---|---|---|---|---|---|---|---|
| Possibility of Anisotropic Structural Plane-Controlled Strength | Anisotropic Strength Ratio | Anisotropic Strength Difference | |||||||
| Dolomitic limestone | 78.23 | 34.5 | 63.56 | 27 | 113.8 | 124.8 | Increase | Decrease | Increase |
| Sandstone | 26.92 | 47.92 | 16.45 | 33.5 | 25.57 | 24.93 | Decrease | Decrease | Increase |
| Jointed plaster of Paris | 3.67 | 21.26 | 0.86 | 32.56 | 9.30 | 1.35 | Decrease | Decrease | Decrease |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guo, S.; Qi, S.; Zheng, B.; Xue, L.; Wang, X.; Liang, N.; Zou, Y.; Tang, F.; Faisal, W.M.; Wen, W.; et al. The Confinement-Affected Strength Variety of Anisotropic Rock Mass. Materials 2022, 15, 8444. https://doi.org/10.3390/ma15238444
Guo S, Qi S, Zheng B, Xue L, Wang X, Liang N, Zou Y, Tang F, Faisal WM, Wen W, et al. The Confinement-Affected Strength Variety of Anisotropic Rock Mass. Materials. 2022; 15(23):8444. https://doi.org/10.3390/ma15238444
Chicago/Turabian StyleGuo, Songfeng, Shengwen Qi, Bowen Zheng, Lei Xue, Xueliang Wang, Ning Liang, Yu Zou, Fengjiao Tang, Waqar Muhammad Faisal, Weiluan Wen, and et al. 2022. "The Confinement-Affected Strength Variety of Anisotropic Rock Mass" Materials 15, no. 23: 8444. https://doi.org/10.3390/ma15238444
APA StyleGuo, S., Qi, S., Zheng, B., Xue, L., Wang, X., Liang, N., Zou, Y., Tang, F., Faisal, W. M., Wen, W., Li, Y., & Yu, X. (2022). The Confinement-Affected Strength Variety of Anisotropic Rock Mass. Materials, 15(23), 8444. https://doi.org/10.3390/ma15238444










